Research on Effective Thermal Conductivity in Porous Media Embedded with Randomly Distributed Damaged Tree-like Bifurcation Networks
Abstract
:1. Introduction
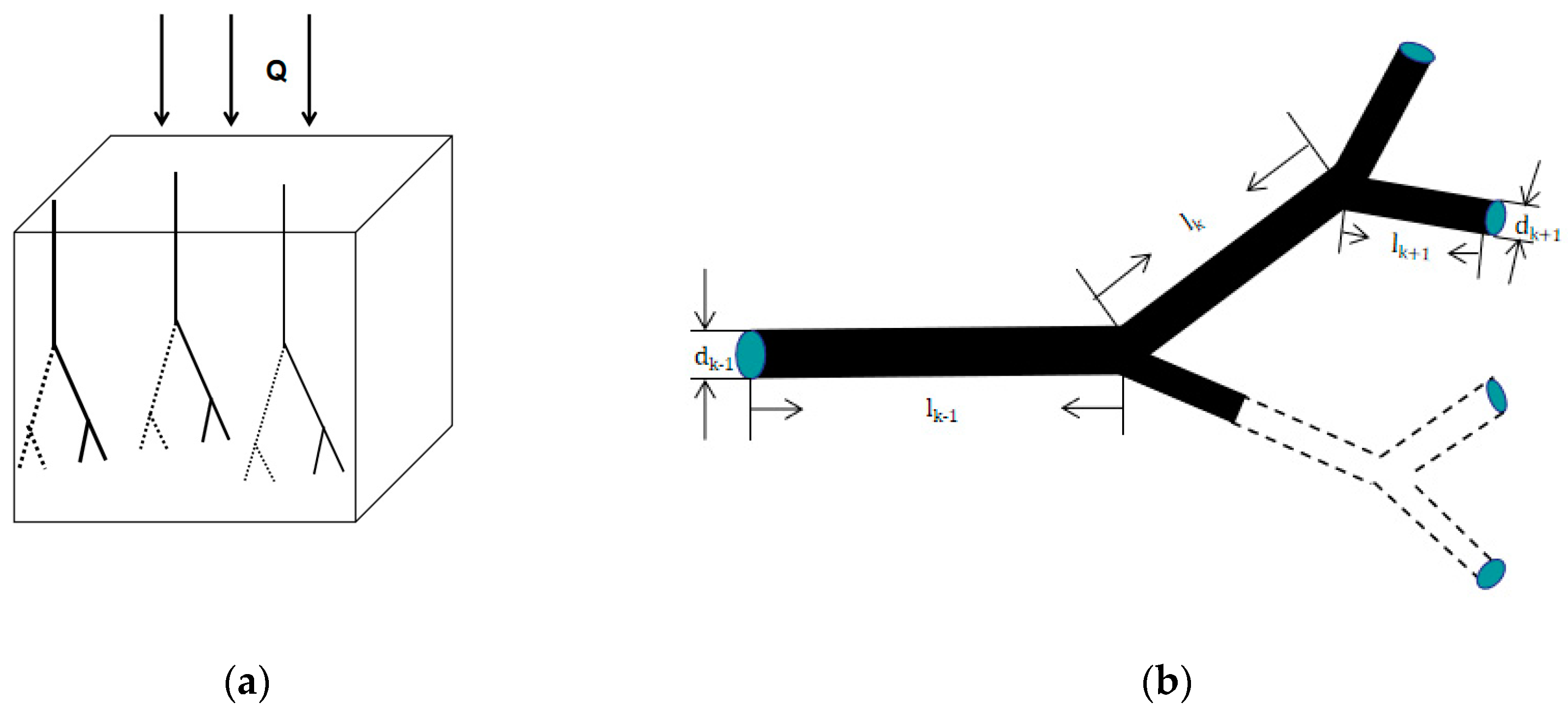
2. Fractal Characteristics of Porous Media
3. Fractal Model of Effective Thermal Conductivity of Porous Media
3.1. The Effective Thermal Conductivity of Heat Conduction
3.2. The Effective Thermal Conductivity of Heat Convection
3.3. The Total Effective Thermal Conductivity of Porous Media
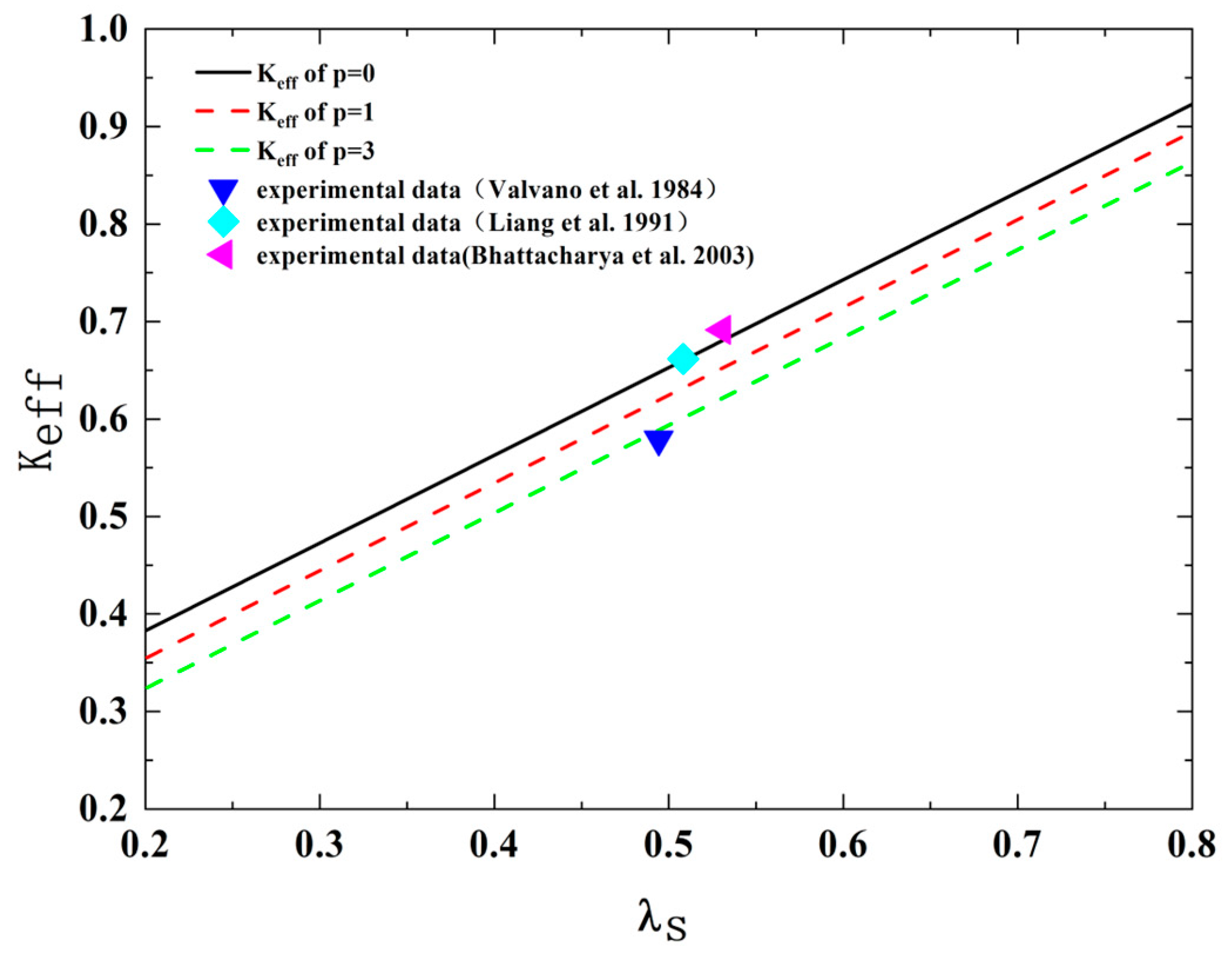
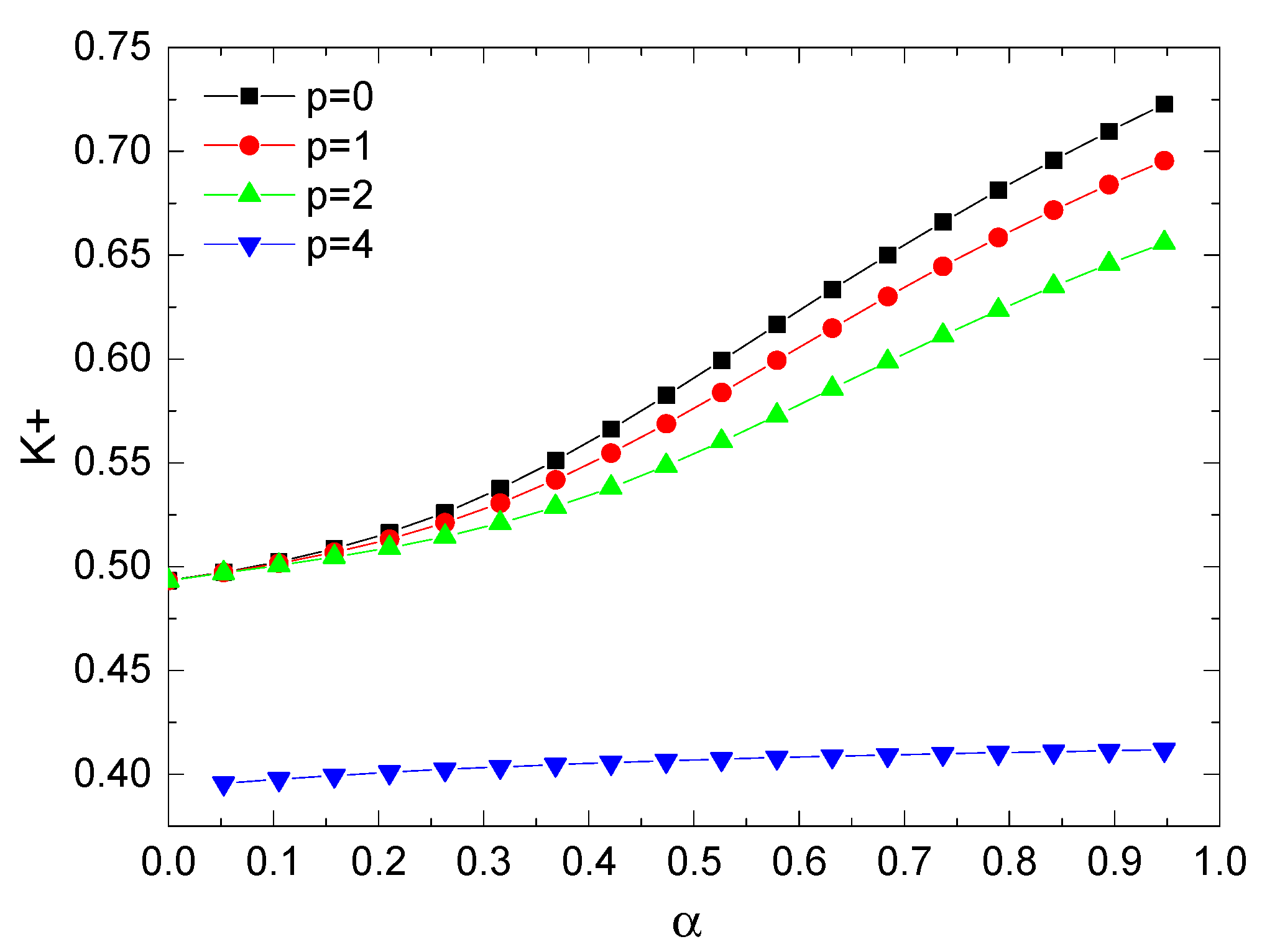
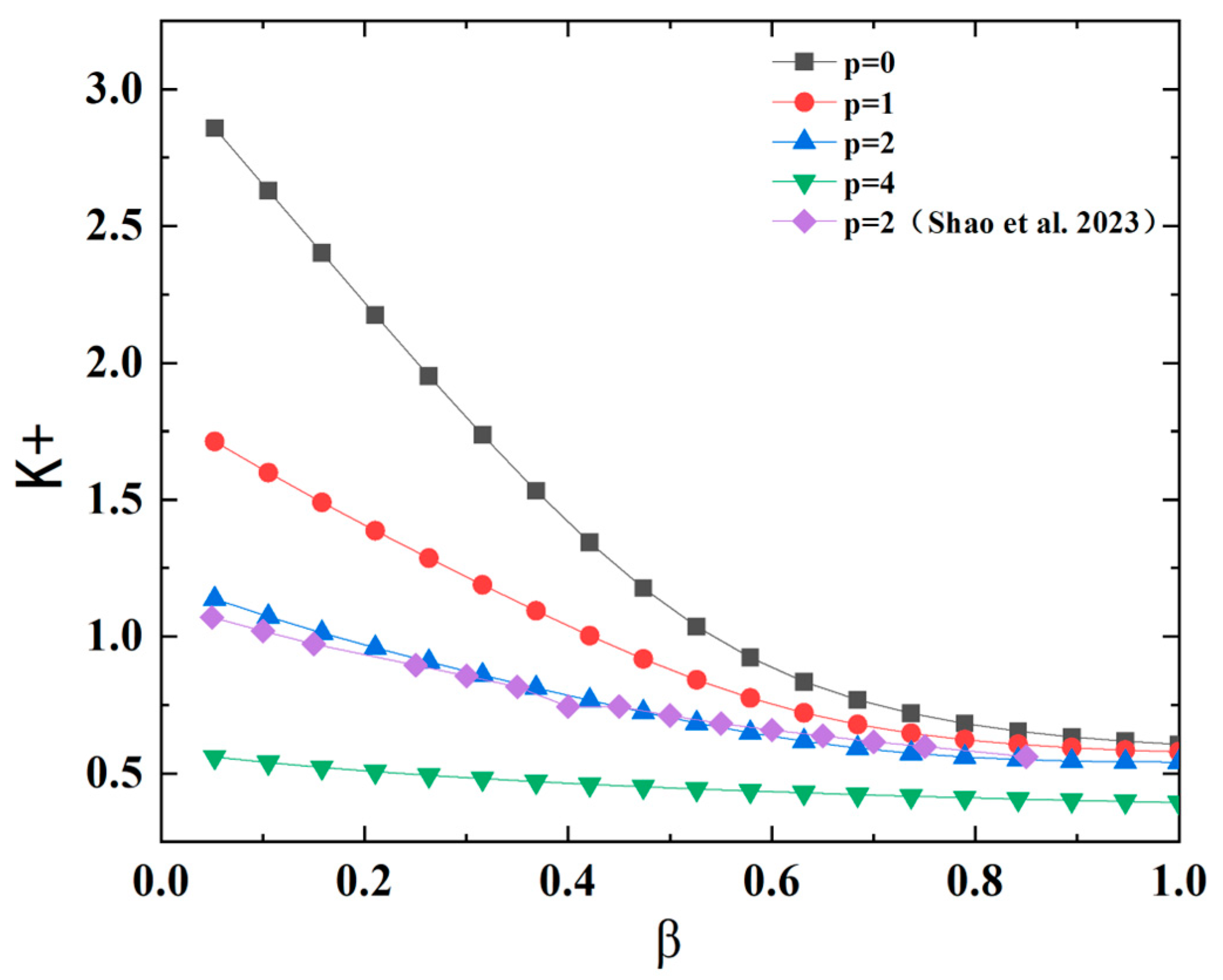
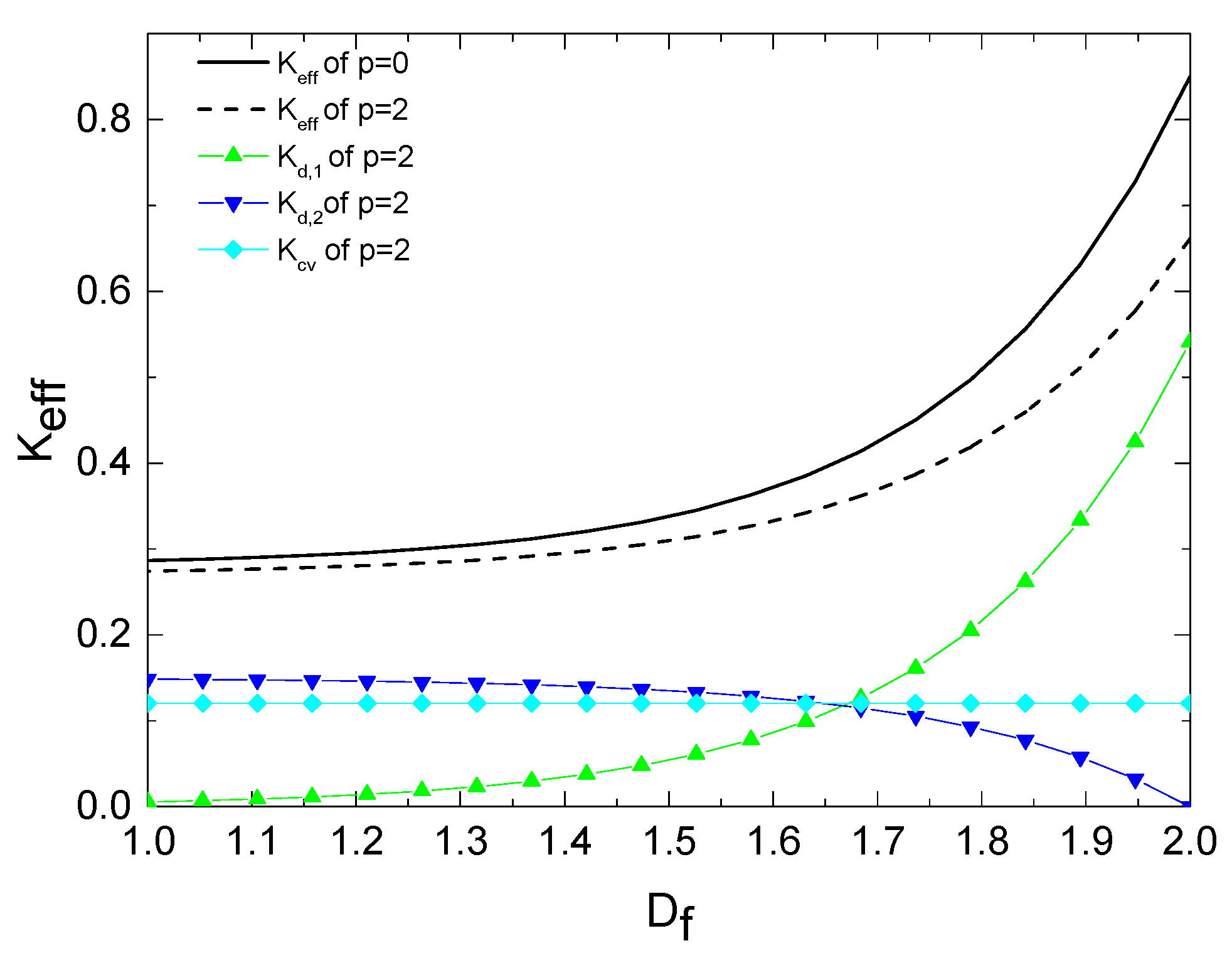
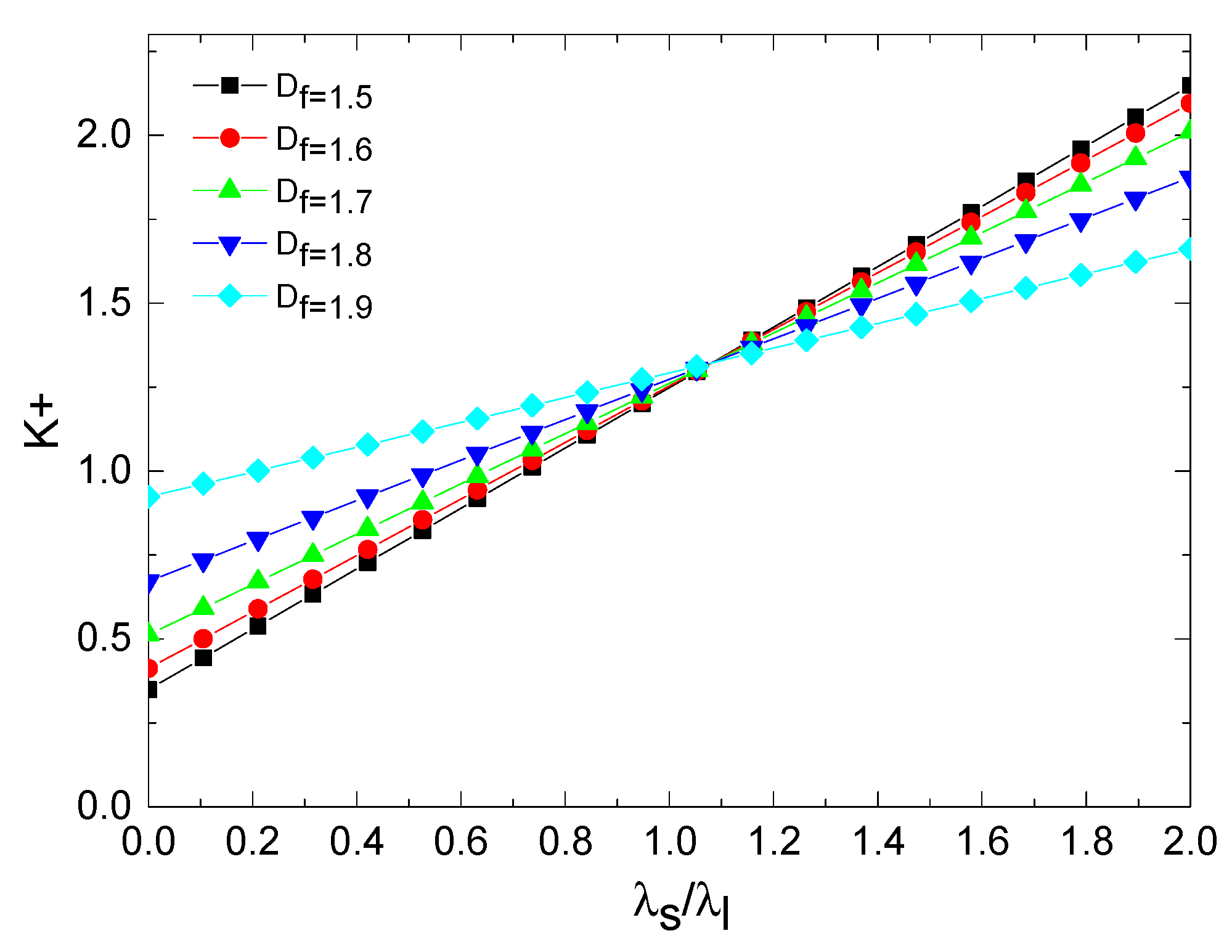
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols | Description | the coefficient of heat convection | |
| branching number | the thermal resistance of the media matrix | ||
| L | diameter scale | the thermal resistance of the network | |
| the length of the kth branching level | the porosity of the cross-section of the media | ||
| the diameter of the kth branching level | temperature difference | ||
| dimension of the main channels’ fractal | the porosity of the cross-section of the media | ||
| areal porosity | the total thermal resistance of the network | ||
| Euclidean dimension | temperature difference | ||
| n | the branching number | the heat convection flow of a single main pipe | |
| fractal dimension | the tree-like bifurcation network’s single main pipe’s heat convection area | ||
| Euclidean dimension | the heat convection flow in the undamaged part | ||
| the primary channels’ largest diameter | the heat convection flow in the damaged part | ||
| the primary channels’ minimum diameter | the heat convection area of the undamaged part | ||
| the length ratio | the heat convection area of the damaged part | ||
| the diameter ratio | the effective thermal conductivity of the media matrix part | ||
| the kth level channel’s single channel’s thermal resistance | the effective thermal conductivity of the network part | ||
| total number of levels in a branch | the average diameter of the main channels | ||
| the number of damaged channels | the thermal conductivity of heat convection | ||
| the equivalent length | the total heat flow | ||
| the total volume | the total heat convection area | ||
| the single pipe’s volume at the branching level | the individual heat flow | ||
| the thermal conductivity of the fluid part | the individual heat convection area | ||
| the thermal conductivity of the media matrix | the thickness of the thermal boundary layer of thermal convection caused by fluid flow is mainly related to the characteristics of the fluid | ||
| the overall main channel cross-sectional area | the coefficient of heat convection | ||
| the area encompassing the whole spectrum of media | the effective thermal conductivity | ||
| the ETC of network part | the dimensionless thermal conductivity | ||
| the ETC of the media matrix part | |||
| the ETC of the whole porous media | |||
| the Nusselt number | |||
| the thermal conductivity of heat convection |
References
- He, Y.L.; Xie, T. Advances of thermal conductivity model of nanoscale silica aerogel insulation material. Appl. Therm. Eng. 2015, 81, 28–50. [Google Scholar] [CrossRef]
- Mao, N.; Ye, J.; Quan, Z.Z.; Zhang, H.N.; Wu, D.Q.; Qin, X.H.; Wang, R.W.; Yu, J.Y. Tree-like structure driven water transfer in 1D fiber assemblies for Functional Moisture-Wicking Fabrics. Mater. Des. 2020, 186, 108305. [Google Scholar] [CrossRef]
- Liang, M.; Fu, C.; Xiao, B.; Luo, L.; Wang, Z. A fractal study for the effective electrolyte diffusion through charged porous media. Int. J. Heat Mass Transf. 2019, 137, 365–371. [Google Scholar] [CrossRef]
- Zhang, N.; Wang, Z.Y. Review of soil thermal conductivity and predictive models. Int. J. Therm. Sci. 2017, 117, 172–183. [Google Scholar] [CrossRef]
- Liu, H.; Zhao, X. Thermal conductivity analysis of high porosity structures with open and closed pores. Int. J. Heat Mass Transfer 2022, 183, 122089. [Google Scholar] [CrossRef]
- Pan, Y.; Zhao, R.; Nian, Y. Study on the flow and heat transfer characteristics of pin-fin manifold microchannel heat sink. Int. J. Heat Mass Transfer 2022, 183, 122052. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W H Freeman: San Francisco, CA, USA, 1982. [Google Scholar]
- Yu, B.M.; Li, J. Some fractal characters of porous media. Fractals 2001, 9, 365–372. [Google Scholar] [CrossRef]
- Yu, B.M.; Lee, L.J.; Cao, H. A fractal in-plane permeability model for fabrics. Polym. Compos. 2002, 23, 201–221. [Google Scholar] [CrossRef]
- Yu, B.M.; Cheng, P. A fractal model for permeability of bi-dispersed porous media. Int. J. Heat Mass Transfer 2002, 45, 2983–2993. [Google Scholar] [CrossRef]
- Zheng, Q.; Wang, H.L.; Guo, X.Y. Research on the effect of surface roughness on gas diffusion coefficient of porous media embedded with a fractal-like tree network. Fractals 2021, 29, 2150195. [Google Scholar] [CrossRef]
- Miao, T.J.; Chen, A.M.; Zhang, L.W. A novel fractal model for permeability of damaged tree-like branching networks. Int. J. Heat Mass Transfer 2018, 127, 278–285. [Google Scholar] [CrossRef]
- Kou, J.L.; Chen, Y.Y.; Zhou, X.Y.; Lu, H.J.; Wu, F.M.; Fan, J.T. Optimal structure of tree-like branching networks for fluid flow. Phys. A 2014, 393, 527–534. [Google Scholar] [CrossRef]
- Zheng, Q.; Wang, H.L.; Jiang, J.; Xu, C. Fractal analysis of surface roughness effects on gas diffusion in porous nanofibers. Fractals 2020, 28, 2050125. [Google Scholar] [CrossRef]
- Zheng, Q.; Yu, B.M.; Wang, S.F.; Luo, L. A diffusivity model for gas diffusion through fractal porous media. Chem. Eng. Sci. 2020, 68, 650–655. [Google Scholar] [CrossRef]
- Zheng, Q.; Xu, J.; Yang, B.; Yu, B.M. Research on the effective gas diffusion coefficient in dry porous media embedded with a fractal-like tree network. Phys. A 2013, 392, 1557–1566. [Google Scholar] [CrossRef]
- Wang, F.Y.; Cheng, H. A fractal permeability model for 2D complex tortuous fractured porous media. J. Pet. Sci. Eng. 2020, 188, 106938. [Google Scholar] [CrossRef]
- Zheng, Q.; Yu, B.M. A fractal permeability model for gas flow through dual-porosity media. J. Appl. Phys. 2012, 111, 024316. [Google Scholar] [CrossRef]
- Zhang, J.; Raza, A.; Khan, U.; Ali, Q.; Zaib, A.; Weera, W.; Galal, A. Thermophysical Study of Oldroyd-B Hybrid Nanofluid with Sinusoidal Conditions and Permeability: A Prabhakar Fractional Approach. Fractal Fract. 2022, 6, 357. [Google Scholar] [CrossRef]
- Xie, B.; Hu, Y.H.; Liu, L.T. Thermal conduction model of asymmetric structural aramid nanofiber aerogel membranes based on fractal theory. Int. J. Heat Mass Transfer 2023, 208, 124086. [Google Scholar] [CrossRef]
- Shi, Q.T.; Feng, C.; Li, B.; Ming, P.W.; Zhang, C.M. Fractal model for the effective thermal conductivity of microporous layer. Int. J. Heat Mass Transfer 2023, 205, 123884. [Google Scholar] [CrossRef]
- Xiao, B.Q. A fractal model for predicting the effective thermal conductivity of roughened porous media with microscale conductivity. Fractals 2021, 29, 2150114. [Google Scholar] [CrossRef]
- Shen, Y.Q.; Xu, P. A generalized thermal conductivity model for unsaturated porous media with fractal geometry. Int. J. Heat Mass Transfer 2020, 152, 119540. [Google Scholar] [CrossRef]
- Jing, D.L.; He, L.; Wang, X.M. Optimization analysis of fractal tree-like microchannel network for electroviscous flow to realize minimum hydraulic resistance. Int. J. Heat Mass Transfer 2018, 125, 749–752. [Google Scholar] [CrossRef]
- Xu, L.; Xu, Y.; Gu, H. Thermal-hydraulic performance of flat-plate microchannel with fractal tree-like structure and self-affine rough wall. Eng. Appl. Comput. Fluid Mech. 2023, 17, e2153174. [Google Scholar] [CrossRef]
- Chen, Y.P.; Cheng, P. Heat transfer and pressure drop in fractal tree-like microchannel nets. Int. J. Heat Mass Transfer 2002, 45, 2643–2648. [Google Scholar] [CrossRef]
- Li, Y.; Yu, B.M. Study of the starting pressure gradient in branching network. Sci. China Technol. Sci. 2010, 53, 2397–2403. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.Z.; Lou, G.; Kou, J.L. A fractal model for effective thermal conductivity of dual-porosity media with randomly distributed tree-like networks. Fractals 2021, 29, 2150146. [Google Scholar] [CrossRef]
- Miao, T.J.; Chen, A.M.; Xu, Y.; Yang, S.S.; Yu, B.M. Optimal structure of damaged tree-like branching networks for the equivalent thermal conductivity. Therm. Sci. 2016, 102, 89–99. [Google Scholar] [CrossRef]
- Xiao, B.Q.; Fang, J.; Long, G.B.; Tao, Y.Z.; Huang, Z.J. Analysis Of Thermal Conductivity Of Damaged Tree-Like Bifurcation Network With Fractal Roughened Surfaces. Fractals 2022, 30, 1–13. [Google Scholar] [CrossRef]
- Shao, Y.H.; Yang, H.; Guo, X.Y.; Wang, H.L.; Zhu, L.M.; Ma, X.; Chen, R.J.; Ruan, S.F.; Ren, L.L.; Zheng, Q. Thermal conductivity model of porous media embedded with a damaged tree-like branching network considering the influence of roughness. Fractal Fract. 2023, 7, 5. [Google Scholar] [CrossRef]
- Xia, Y.L.; Zheng, S.; Yang, S.S.; Yi, S. Analysis on effective thermal conductivity model of porous media with rough fractal-like tree network. J. Cent. China Norm. Univ. 2022, 2, 255–261. [Google Scholar]
- Xu, P.; Yu, B.M.; Yun, M.J.; Zou, M.Q. Heat conduction in fractal tree-like branched networks. Int. J. Heat Mass Transfer 2006, 49, 3746–3751. [Google Scholar] [CrossRef]
- Valvano, J.W.; Allen, J.T.; Bowman, H.F. The simultaneous measurement of thermal conductivity, thermal diffusivity, and perfusion in small volumes of tissue. J. Biomech. Eng. 1984, 106, 192–197. [Google Scholar] [CrossRef] [PubMed]
- Liang, X.G.; Ge, X.S.; Zhang, Y.P. A convenient method of measuring the thermal conductivity of biological tissue. Phys. Med. Biol. 1991, 36, 1599. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharya, A.; Mahajan, R.L. Temperature dependence of thermal conductivity of biological tissues. Physiol. Meas. 2003, 24, 769–783. [Google Scholar] [CrossRef] [PubMed]
- Tien, C.L.; Vafai, K. Convective and radiative heat transfer in porous media. Adv. Appl. Mech. 1989, 27, 225–281. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, Y.; Guo, X.; Wang, H.; Zhu, L.; Zheng, Q. Research on Effective Thermal Conductivity in Porous Media Embedded with Randomly Distributed Damaged Tree-like Bifurcation Networks. Fractal Fract. 2023, 7, 853. https://doi.org/10.3390/fractalfract7120853
Shao Y, Guo X, Wang H, Zhu L, Zheng Q. Research on Effective Thermal Conductivity in Porous Media Embedded with Randomly Distributed Damaged Tree-like Bifurcation Networks. Fractal and Fractional. 2023; 7(12):853. https://doi.org/10.3390/fractalfract7120853
Chicago/Turabian StyleShao, Yihao, Xiuya Guo, Huili Wang, Limei Zhu, and Qian Zheng. 2023. "Research on Effective Thermal Conductivity in Porous Media Embedded with Randomly Distributed Damaged Tree-like Bifurcation Networks" Fractal and Fractional 7, no. 12: 853. https://doi.org/10.3390/fractalfract7120853
APA StyleShao, Y., Guo, X., Wang, H., Zhu, L., & Zheng, Q. (2023). Research on Effective Thermal Conductivity in Porous Media Embedded with Randomly Distributed Damaged Tree-like Bifurcation Networks. Fractal and Fractional, 7(12), 853. https://doi.org/10.3390/fractalfract7120853