Miniaturization and Bandwidth Enhancement of Fractal-Structured Two-Arm Sinuous Antenna Using Gap Loading with Meandering
Abstract
:1. Introduction
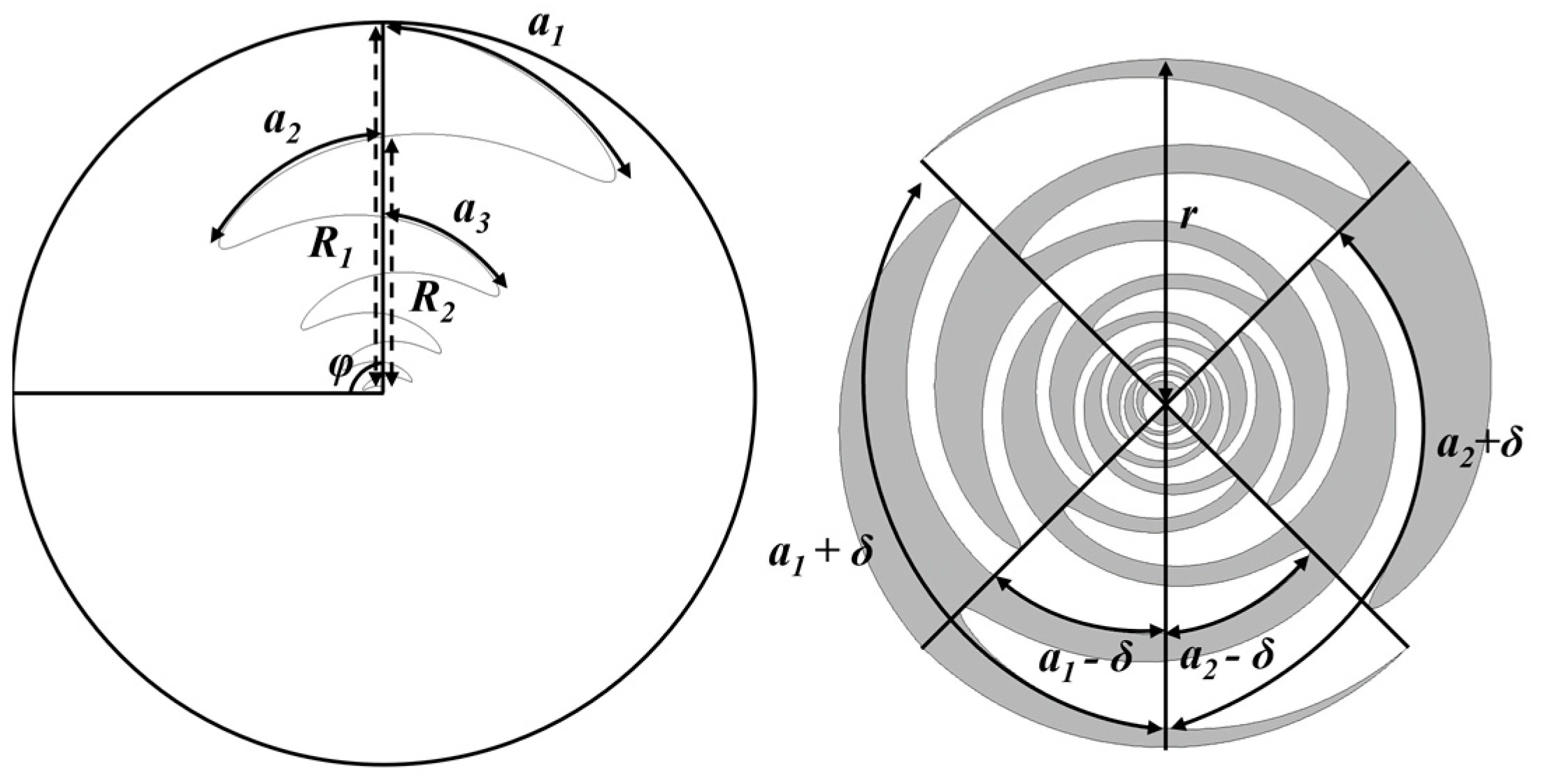
2. Antenna Principle
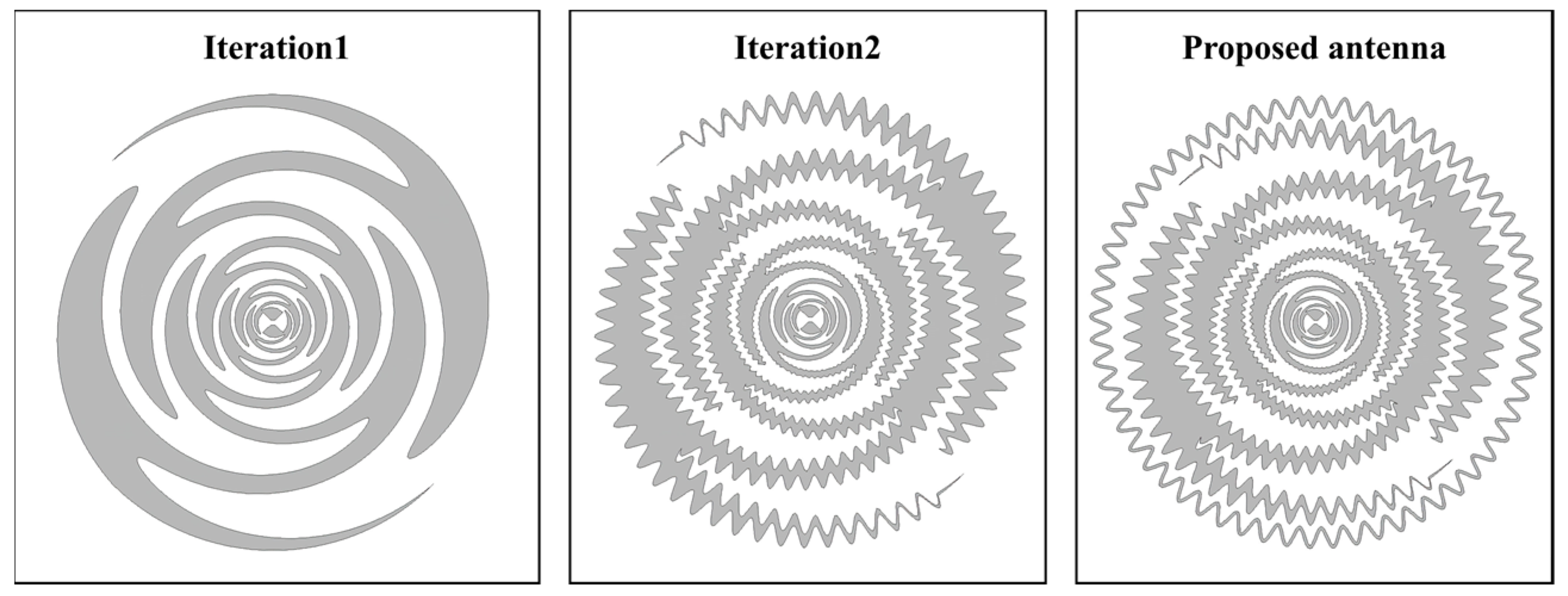
3. The Proposed Antenna Design
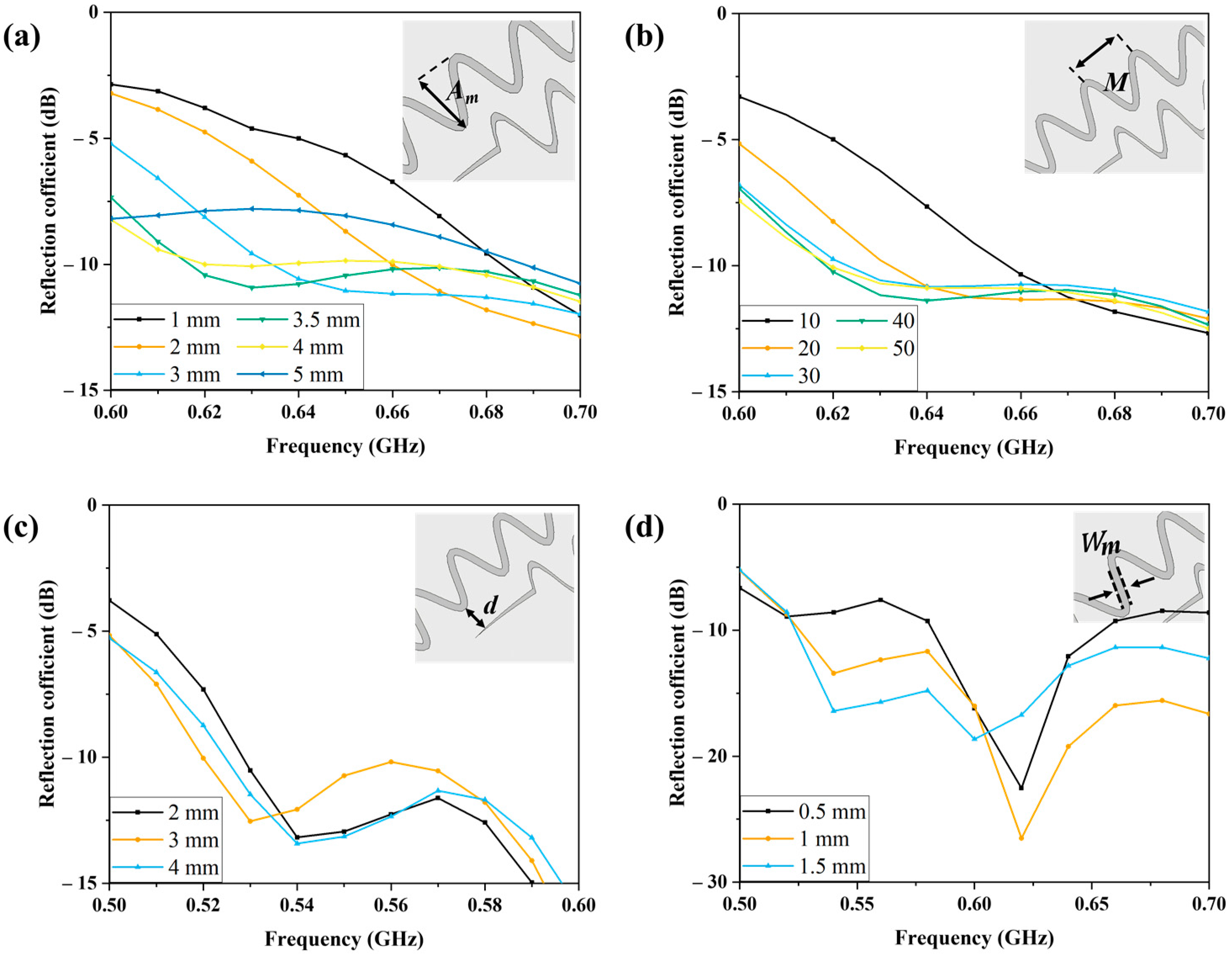
3.1. Applying the Meander Shape to a Basic Sinuous Antenna
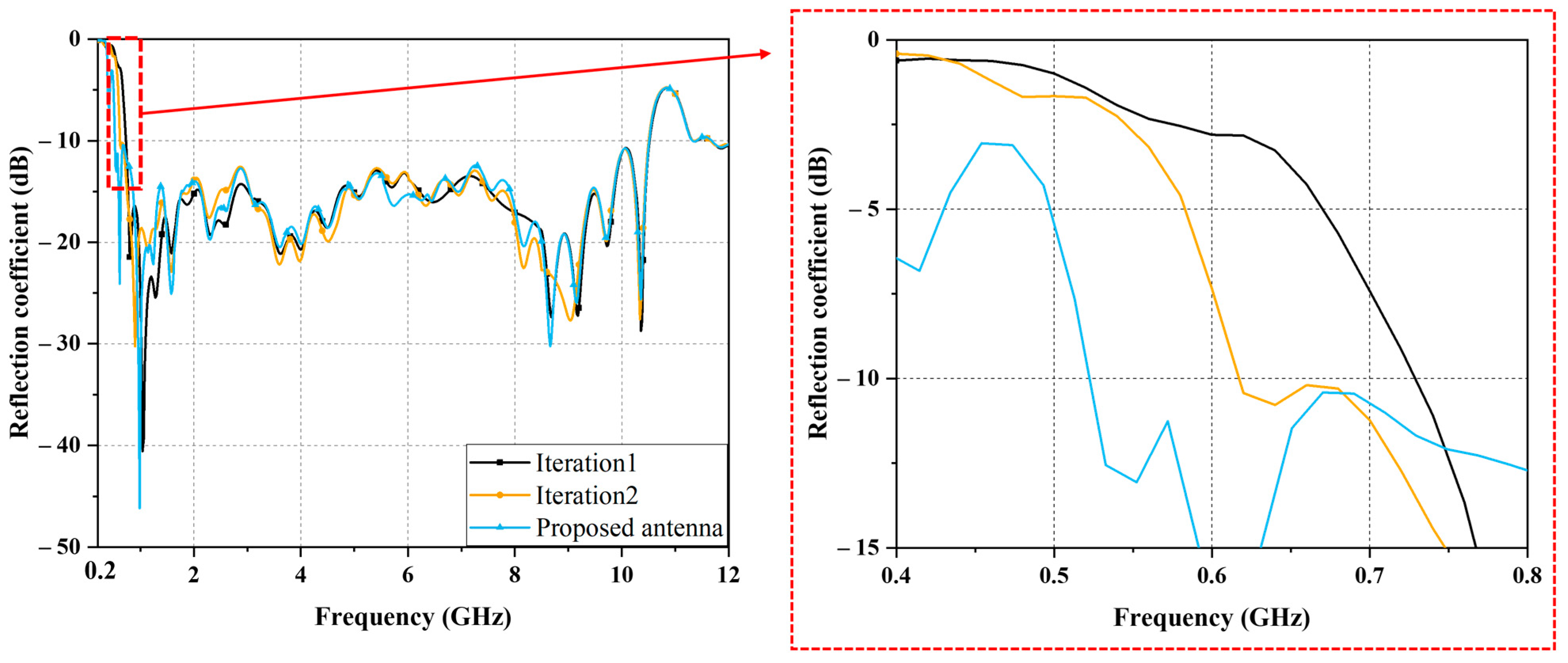
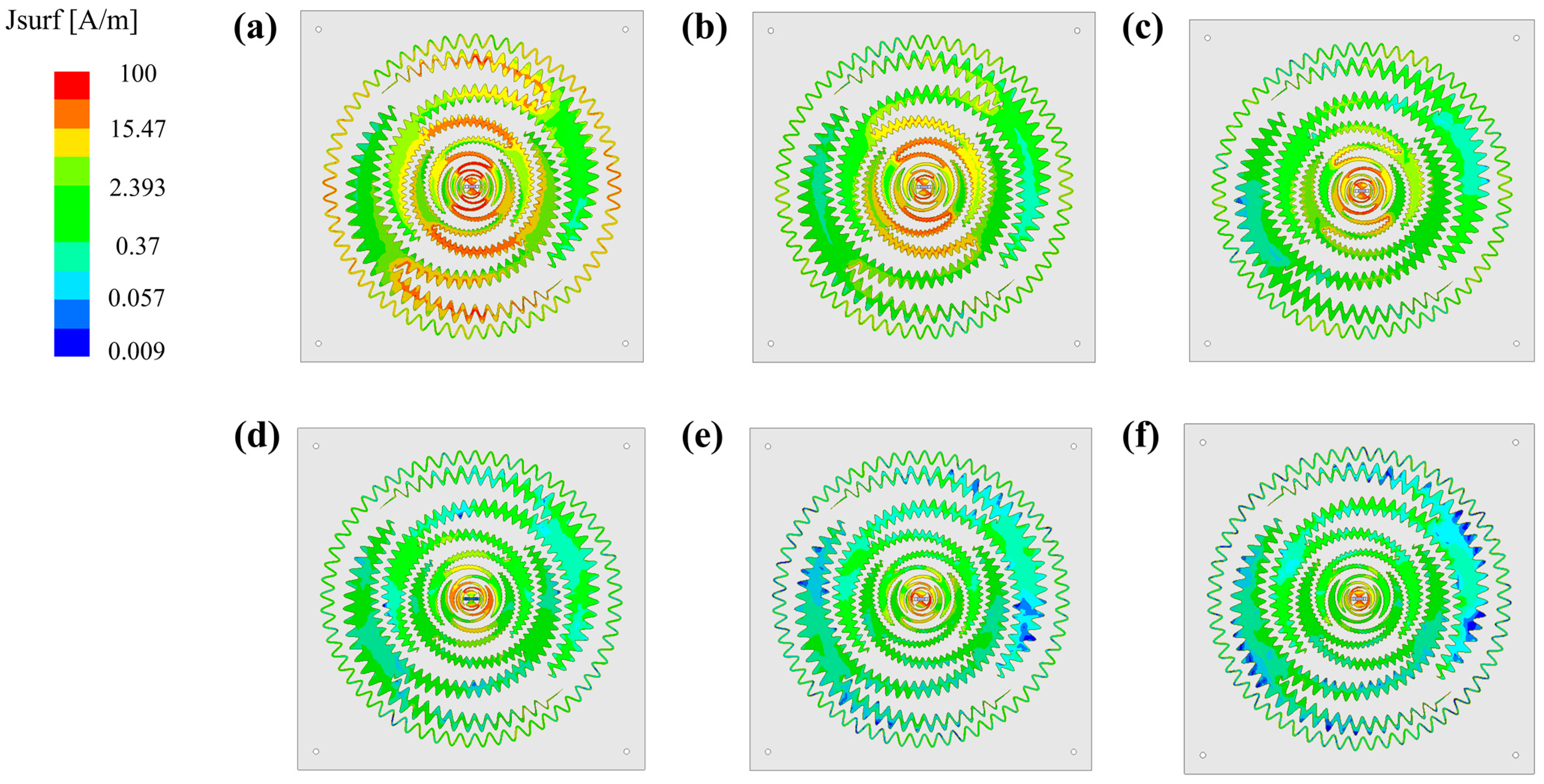
3.2. Proposed Antenna Simulation
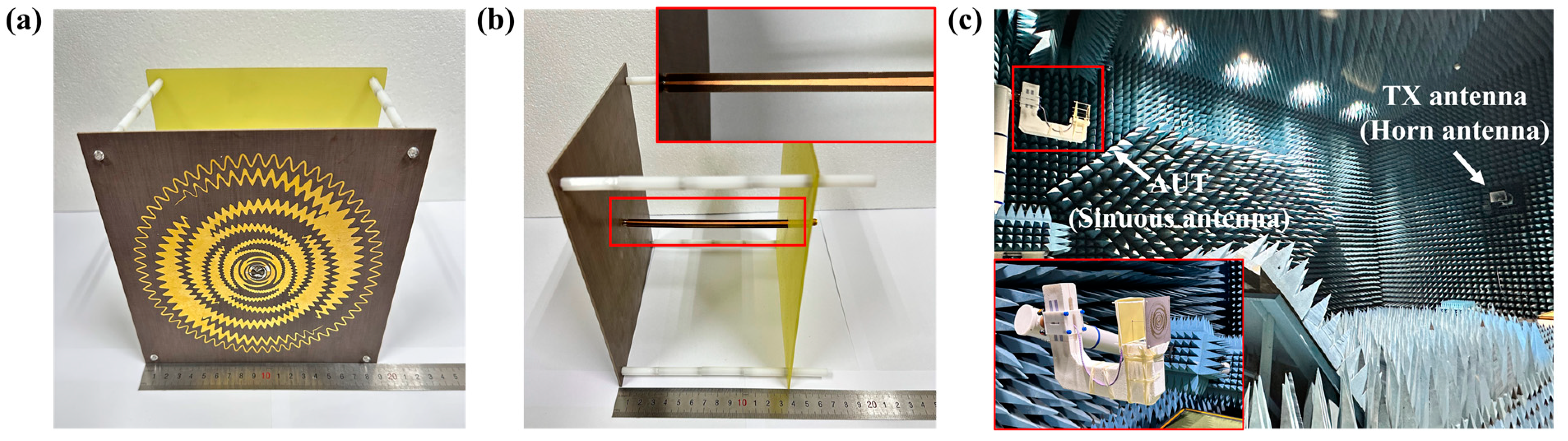
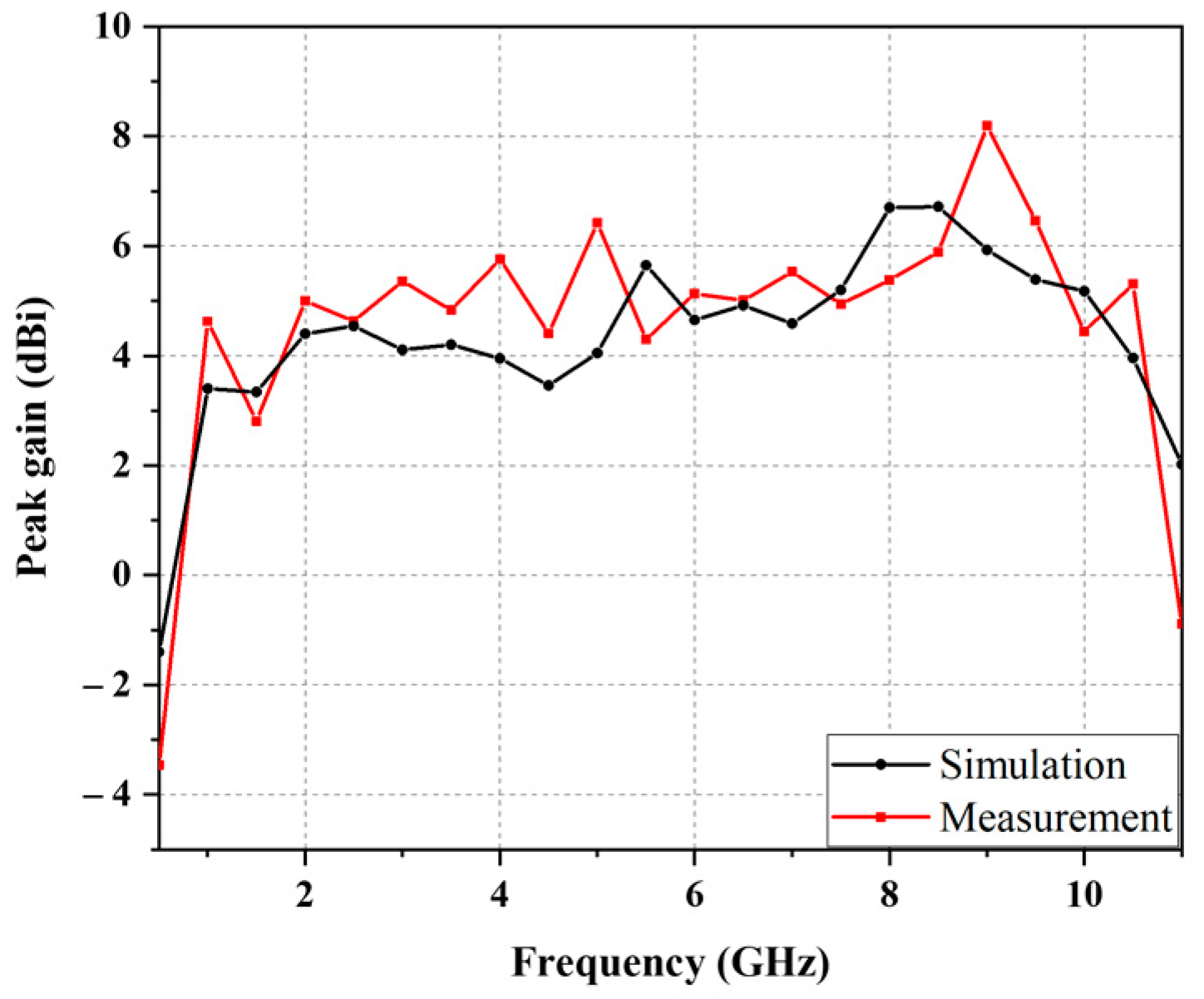
4. Experiment and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chou, H.; Su, H. Dual-Band Hybrid Antenna Structure with Spatial Diversity for DTV and WLAN Applications. IEEE Trans. Antennas Propag. 2017, 65, 4850–4853. [Google Scholar] [CrossRef]
- Bisht, N.; Malik, P.K.; Das, S.; Islam, T.; Asha, S.; Alathbah, M. Design of a Modified MIMO Antenna Based on Tweaked Spherical Fractal Geometry for 5G New Radio (NR) Band N258. Fractal Fract. 2023, 258, 10. [Google Scholar]
- Han, B.; Wang, S.; Shi, X. Design of Compact Fragment-Type Antenna Array for Microwave-Based Head Imaging Application. J. Electromagn. Eng. Sci. 2023, 23, 344–350. [Google Scholar] [CrossRef]
- Koohestani, M.; Azadi-Tinat, N.; Skrivervik, A.K. Compact Slit-Loaded ACS-Fed Monopole Antenna for Bluetooth and UWB Systems with WLAN Band-Stop Capability. IEEE Access 2023, 11, 7540–7550. [Google Scholar] [CrossRef]
- Voronov, A.; Sydoruk, O.; Syms, R.R.A. Waveguide Antenna Topologies for Distributed High-Frequency Near-Field Communication and Localization. IEEE Trans. Antennas Propag. 2023, 71, 5026–5035. [Google Scholar] [CrossRef]
- Vallappil, A.K.; Khawaja, B.A.; Rahim, M.K.A.; Uzair, M.; Jamil, M.; Awais, Q. Minkowski–Sierpinski Fractal Structure-Inspired 2 × 2 Antenna Array for Use in Next-Generation Wireless Systems. Fractal Fract. 2023, 7, 158. [Google Scholar] [CrossRef]
- Benkhadda, O.; Saih, M.; Ahmad, S.; Al-Gburi, A.J.A.; Zakaria, Z.; Chaji, K.; Reha, A. A Miniaturized Tri-Wideband Sierpinski Hexagonal-Shaped Fractal Antenna for Wireless Communication Applications. Fractal Fract. 2023, 7, 115. [Google Scholar] [CrossRef]
- Ooi, S.Y.; Chee, P.S.; Lim, E.H.; Low, J.H.; Bong, F.L. A Zeroth-Order Slot-Loaded Cap-Shaped Patch Antenna with Omnidirectional Radiation Characteristic for UHF RFID Tag Design. IEEE Trans. Antennas Propag. 2023, 71, 131–139. [Google Scholar] [CrossRef]
- Nguyen, T.D.; Kim, S.E.; Jung, C.W. Compact, Flexible and Transparent Antenna Using MMF for Conformal Wi-Fi 7 Applications. J. Electr. Eng. Technol. 2023, 23, 4341–4352. [Google Scholar] [CrossRef]
- Paun, M.A.; Nichita, M.V.; Paun, V.A.; Paun, V.P. Minkowski’s Loop Fractal Antenna Dedicated to Sixth Generation (6G) Communication. Fractal Fract. 2022, 6, 402. [Google Scholar] [CrossRef]
- Liu, Z.; Zhang, Y.; He, Y.; Li, Y. A Compact-Size and High-Efficiency Cage Antenna for 2.4-GHz WLAN Access Points. IEEE Trans. Antennas Propag. 2022, 70, 12317–12321. [Google Scholar] [CrossRef]
- Tong, X.; Jiang, Z.H.; Yu, C.; Wu, F.; Xu, X.; Hong, W. Low-Profile, Broadband, Dual-Linearly Polarized, and Wide-Angle Millimeter-Wave Antenna Arrays for Ka-Band 5G Applications. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 2038–2042. [Google Scholar] [CrossRef]
- Wu, D.L.; Chen, J.H.; Yang, K.Y.; Zhu, W.J.; Ye, L.H. A Compact Dual-Polarized Patch Antenna With L-Shaped Short Pins. IEEE Antennas Wirel. Propag. Lett. 2023, 22, 689–693. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Pal, J.; Patra, K.; Gupta, B. Bandwidth-Enhanced Miniaturized Patch Antenna Operating at Higher Order Dual-Mode Resonance Using Modal Analysis. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 274–278. [Google Scholar] [CrossRef]
- Chang, L.; Liu, H. Low-Profile and Miniaturized Dual-Band Microstrip Patch Antenna for 5G Mobile Terminals. IEEE Trans. Antennas Propag. 2022, 70, 2328–2333. [Google Scholar] [CrossRef]
- Jung, J.I.; Yang, J.R. 5.8-GHz Patch Antenna with an Enhanced Defected Ground Structure for Size Reduction and Increased Bandwidth. J. Electromagn. Eng. Sci. 2022, 22, 245–251. [Google Scholar] [CrossRef]
- Vallappil, A.K.; Khawaja, B.A.; Rahim, M.K.A.; Iqbal, M.N.; Chattha, H.T.; Ali, M.F. bin M. A Compact Triple-Band UWB Inverted Triangular Antenna with Dual-Notch Band Characteristics Using SSRR Metamaterial Structure for Use in Next-Generation Wireless Systems. Fractal Fract. 2022, 6, 422. [Google Scholar] [CrossRef]
- Naji, D.K. Miniature Slotted Semi-Circular Dual-Band Antenna for WiMAX and WLAN Applications. J. Electromagn. Eng. Sci. 2020, 20, 115–124. [Google Scholar] [CrossRef]
- Tumakov, D.; Chikrin, D.; Kokunin, P. Miniaturization of a Koch-Type Fractal Antenna for Wi-Fi Applications. Fractal Fract. 2020, 4, 25. [Google Scholar] [CrossRef]
- DuHamel, R.H. Dual Polarized Sinuous Antennas. U.S. Patent No. 4,658,262, 14 April 1987. [Google Scholar]
- Kang, Y.; Kim, K.; Scott, W.R. Modification of Sinuous Antenna Arms for UWB Radar Applications. IEEE Trans. Antennas Propag. 2015, 63, 5229–5234. [Google Scholar] [CrossRef]
- Crocker, D.A.; Scott, W.R. On the Design of Sinuous Antennas for UWB Radar Applications. IEEE Antennas Wirel. Propag. Lett. 2019, 18, 1347–1351. [Google Scholar] [CrossRef]
- Gamec, J.; Repko, M.; Gamcová, M.; Gladišová, I.; Kurdel, P.; Nekrasov, A.; Fidge, C. Low Profile Sinuous Slot Antenna for UWB Sensor Networks. Electronics 2019, 8, 127. [Google Scholar] [CrossRef]
- Crocker, D.A.; Scott, W.R. Compensation of Dispersion in Sinuous Antennas for Polarimetric Ground Penetrating Radar Applications. Remote Sens. 2019, 11, 1937. [Google Scholar] [CrossRef]
- Crocker, D.A.; Scott, W.R. An Unbalanced Sinuous Antenna for Near-Surface Polarimetric Ground-Penetrating Radar. IEEE Open J. Antennas Propag. 2020, 1, 435–447. [Google Scholar] [CrossRef]
- Stults, A.H. Impulse Loading of Sinuous Antennas by Ferroelectric Generators. In Proceedings of the 2008 IEEE International Power Modulators and High-Voltage Conference, Las Vegas, NV, USA, 27–31 May 2008; pp. 156–158. [Google Scholar] [CrossRef]
- Kim, D.; Park, C.Y.; Kim, Y.; Kim, H.; Yoon, Y.J. Four-Arm Sinuous Antenna With Low Input Impedance for Wide Gain Bandwidth. IEEE Access 2022, 10, 35265–35272. [Google Scholar] [CrossRef]
- Zhang, H.S.; Xiao, K.; Qiu, L.; Chai, S.L. Four-Arm Sinuous Antenna for Direction Finding System. In Proceedings of the 2014 IEEE International Wireless Symposium (IWS 2014), Xi’an, China, 24–26 March 2014; pp. 1–4. [Google Scholar] [CrossRef]
- Bellion, A.; Le Meins, C.; Julien-Vergonjanne, A.; Monédière, T. A New Compact Dually Polarized Direction Finding Antenna on the UHF Band. In Proceedings of the 2008 IEEE Antennas and Propagation Society International Symposium, San Diego, CA, USA, 5–11 July 2008; pp. 11–14. [Google Scholar] [CrossRef]
- Lorho, N.; Lirzin, G.; Chousseaud, A.; Razban, T.; Bikiny, A.; Lestieux, S. Miniaturization of an UWB Dual-Polarized Antenna. In Proceedings of the 2015 IEEE International Conference on Ubiquitous Wireless Broadband (ICUWB), Montreal, QC, Canada, 4–7 October 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Johnson, R.C.J.H. Antenna Engineering Handbook, 3rd ed.; McGraw-Hill: New York, NY, USA, 1993. [Google Scholar]
- Liu, Q.; Ruan, C.-L.; Peng, L.; Wu, W.-X. A novel compact archimedean spiral antenna with gap-loading. Prog. Electromagn. Res. Lett. 2008, 3, 169–177. [Google Scholar] [CrossRef]
- Pozar, D.M. Microwave Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2011; ISBN 9780470631553. [Google Scholar]
- Wang, S.F.; Xie, Y.Z. Design and Optimization of High-Power UWB Combined Antenna Based on Klopfenstein Impedance Taper. IEEE Trans. Antennas Propag. 2017, 65, 6960–6967. [Google Scholar] [CrossRef]
- Wang, W.; Garcia, N.C.; Chisum, J.D. The Systematic Design of Noncommensurate Impedance Matching Tapers for Ultrawideband Gradient-Index Lens Antennas. IEEE Trans. Antennas Propag. 2022, 70, 35–45. [Google Scholar] [CrossRef]
- Elmansouri, M.A.; Bargeron, J.B.; Filipovic, D.S. Simply-Fed Four-Arm Spiral-Helix Antenna. IEEE Trans. Antennas Propag. 2014, 62, 4864–4868. [Google Scholar] [CrossRef]
- Buck, M.C.; Filipović, D.S. Two-Arm Sinuous Antennas. IEEE Trans. Antennas Propag. 2008, 56, 1229–1235. [Google Scholar] [CrossRef]
- Sammeta, R.; Filipovic, D.S. Improved Efficiency Lens-Loaded Cavity-Backed Transmit Sinuous Antenna. IEEE Trans. Antennas Propag. 2014, 62, 6000–6009. [Google Scholar] [CrossRef]
- Zhang, X.L.; Chen, K.; Liang, Z.Y.; Liu, L.; Wang, Z. Bin A High-Gain Wideband Sinuous Antenna Loaded with Lens and Cone-Shaped Reflective Backplate. In Proceedings of the 2022 International Conference on Microwave and Millimeter Wave Technology (ICMMT), Harbin, China, 12–15 August 2022; Volume 2, pp. 1–3. [Google Scholar] [CrossRef]
- Edwards, J.M.; O’Brient, R.; Lee, A.T.; Rebeiz, G.M. Dual-Polarized Sinuous Antennas on Extended Hemispherical Silicon Lenses. IEEE Trans. Antennas Propag. 2012, 60, 4082–4091. [Google Scholar] [CrossRef]
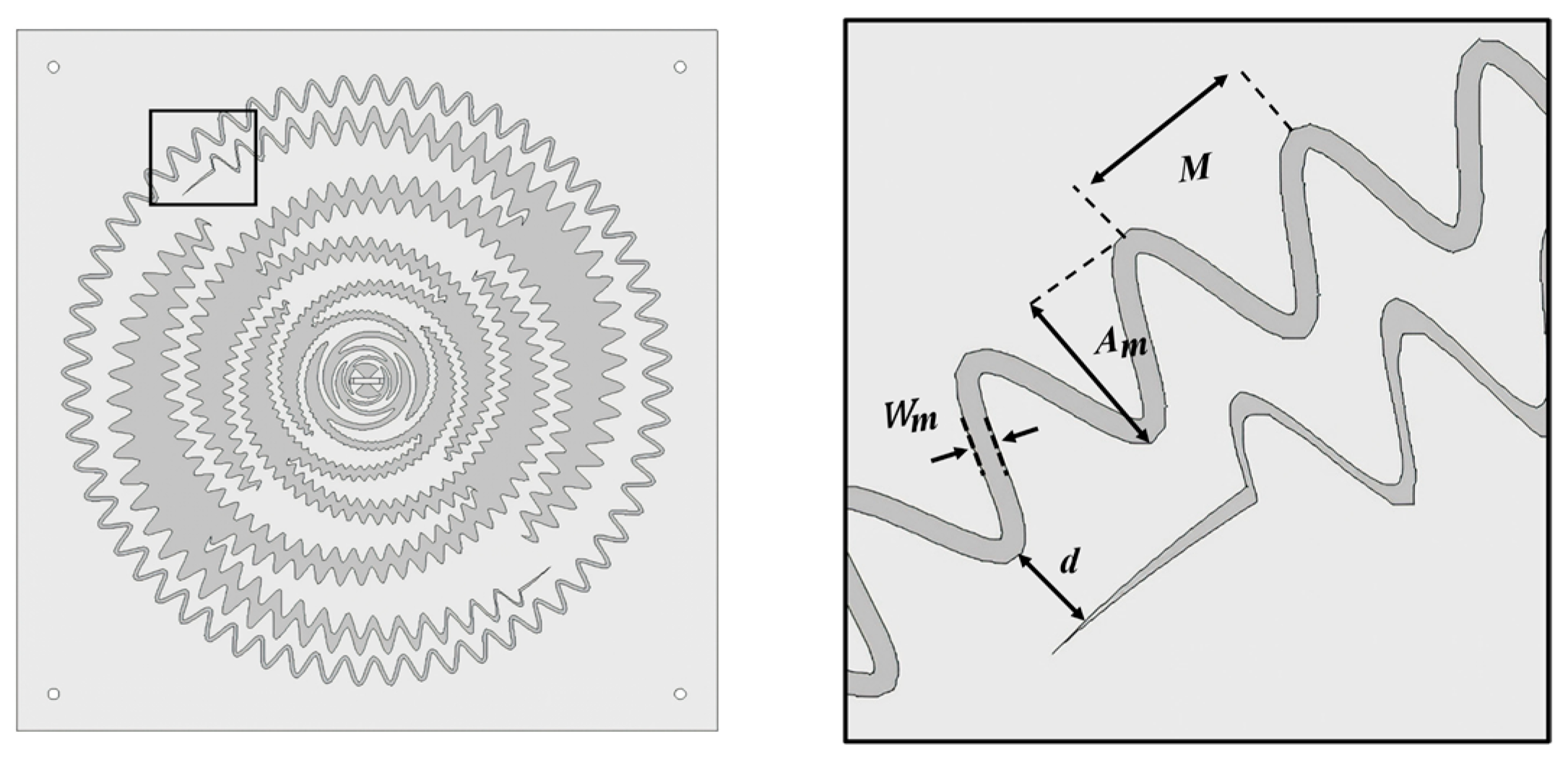



| Parameter | Dimension | Parameter | Dimension (mm) | Parameter | Dimension (mm) |
|---|---|---|---|---|---|
| 70.8722 mm | 2.168 | 4.57 | |||
| τ | 0.707 | 2.069 | 3.625 | ||
| 90° | 1.972 | 2.998 | |||
| 45° | 1.874 | 2.596 | |||
| 2 | 1.776 | 2.222 | |||
| Cell | 8 | 1.678 | 1.939 | ||
| 3.5 mm | 1.581 | 1.704 | |||
| 1 mm | 1.483 | 1.467 | |||
| 30 | 1.385 | 1.287 | |||
| 4 mm | 1.287 | 1.123 | |||
| 12.5218 mm | 1.189 | 1.004 | |||
| 70.0931 mm | 1.091 | 0.891 | |||
| 7 mm | 0.993 | 0.81 | |||
| 0.896 | 0.758 | ||||
| 0.798 | 0.714 | ||||
| 0.7 | 0.676 |
| Ref. | Antenna Type | Reflection Coefficient Bandwidth (GHz)/Fractional Bandwidth (%) | Gain (dBi) | Antenna Width × Length |
|---|---|---|---|---|
| [22] | 4-arm sinuous w/balun | 1–10/163.6 | −1~6 | 0.495 × 0.495 |
| [24] | 4-arm sinuous w/balun | 0.8~10/170.4 | N/A | 0.79 × 0.79 |
| [27] | 4-arm sinuous w/balun | 0.45~6/172.1 | −1~5.5 | 0.64 × 0.64 |
| [28] | 4-arm sinuous w/balun | 0.4~2/133.3 | N/A | 0.955 × 0.955 |
| [38] | 4-arm sinuous on dielectric lens | 0.6~2.5/115 | 3.9~12 | 0.7 × 0.7 |
| [40] | 4-arm sinuous on dielectric lens | 6~24/120 | N/A | 1.2 × 1.2 |
| [23] | 2-arm sinuous w/CPWG | 0.46~4.5/162.9 | 2.9~5.7 | 0.67 × 0.63 |
| [37] | 2-arm sinuous w/balun | 2~18/160 | 4.3~5.1 | 0.495 × 0.495 |
| [39] | 2-arm sinuous on dielectric lens w/balun | 1~10/163.6 | 6~12 | 0.554 × 0.554 |
| This work | 2-arm sinuous w/balun | 0.74~10.53/173.7 (SIM.) | 2.8~5.7 (SIM.) | 0.552 × 0.552 |
| This work | 2-arm fractal-structured sinuous w/balun | 0.51~10.72/181.8 | −3.5~8.2 | 0.443 × 0.443 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.; Keun, J.; Yoo, T.; Lim, S. Miniaturization and Bandwidth Enhancement of Fractal-Structured Two-Arm Sinuous Antenna Using Gap Loading with Meandering. Fractal Fract. 2023, 7, 841. https://doi.org/10.3390/fractalfract7120841
Kim J, Keun J, Yoo T, Lim S. Miniaturization and Bandwidth Enhancement of Fractal-Structured Two-Arm Sinuous Antenna Using Gap Loading with Meandering. Fractal and Fractional. 2023; 7(12):841. https://doi.org/10.3390/fractalfract7120841
Chicago/Turabian StyleKim, Junghyeon, Jongho Keun, Taehoon Yoo, and Sungjoon Lim. 2023. "Miniaturization and Bandwidth Enhancement of Fractal-Structured Two-Arm Sinuous Antenna Using Gap Loading with Meandering" Fractal and Fractional 7, no. 12: 841. https://doi.org/10.3390/fractalfract7120841
APA StyleKim, J., Keun, J., Yoo, T., & Lim, S. (2023). Miniaturization and Bandwidth Enhancement of Fractal-Structured Two-Arm Sinuous Antenna Using Gap Loading with Meandering. Fractal and Fractional, 7(12), 841. https://doi.org/10.3390/fractalfract7120841








