Hermite Finite Element Method for Time Fractional Order Damping Beam Vibration Problem
Abstract
:1. Introduction
2. Finite Element Approximation
3. Stability of Fully Discrete Finite Element Schemes
4. Error Estimation of Fully Discrete Finite Element Schemes
5. Numerical Experiment
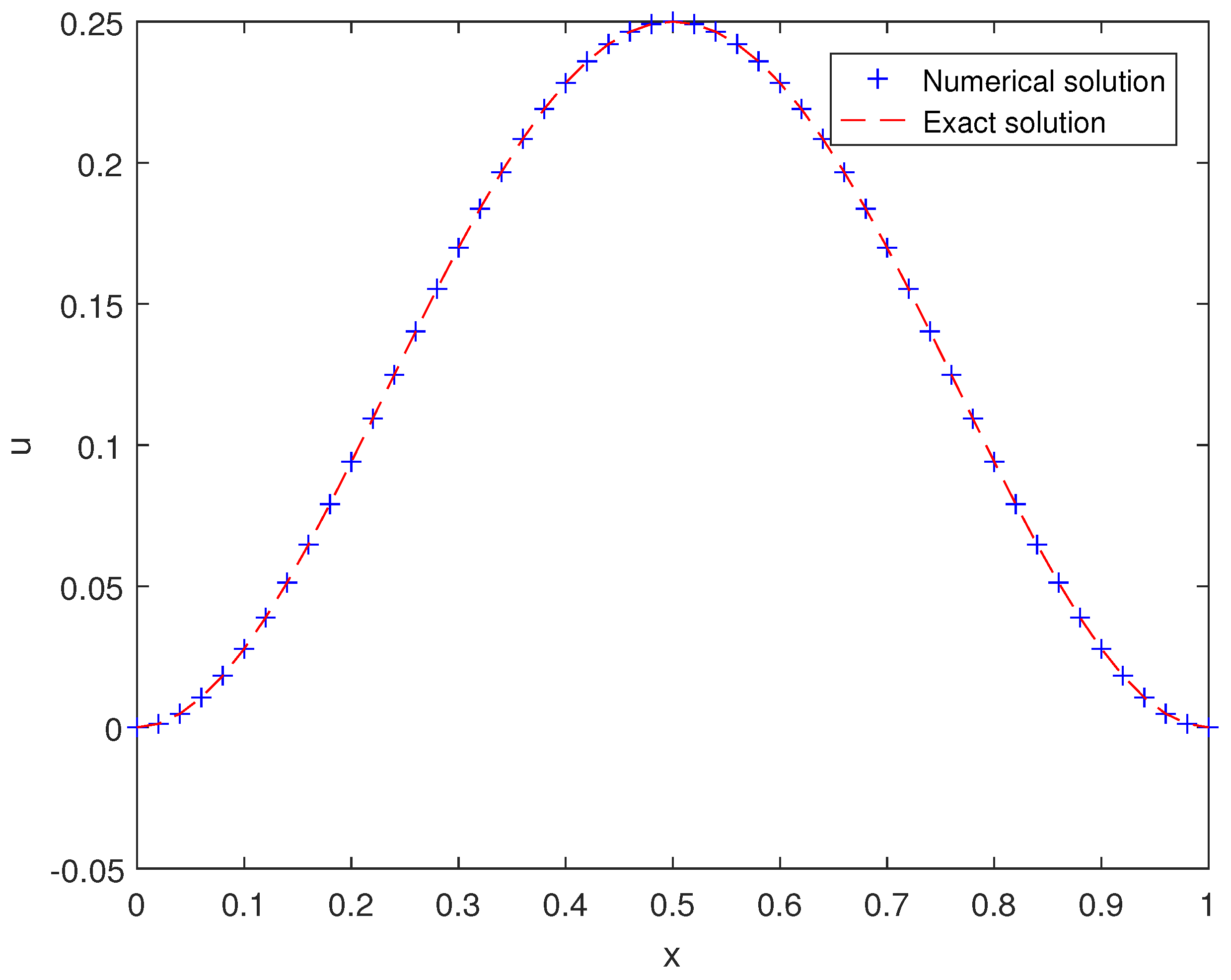
5.1. Example 1
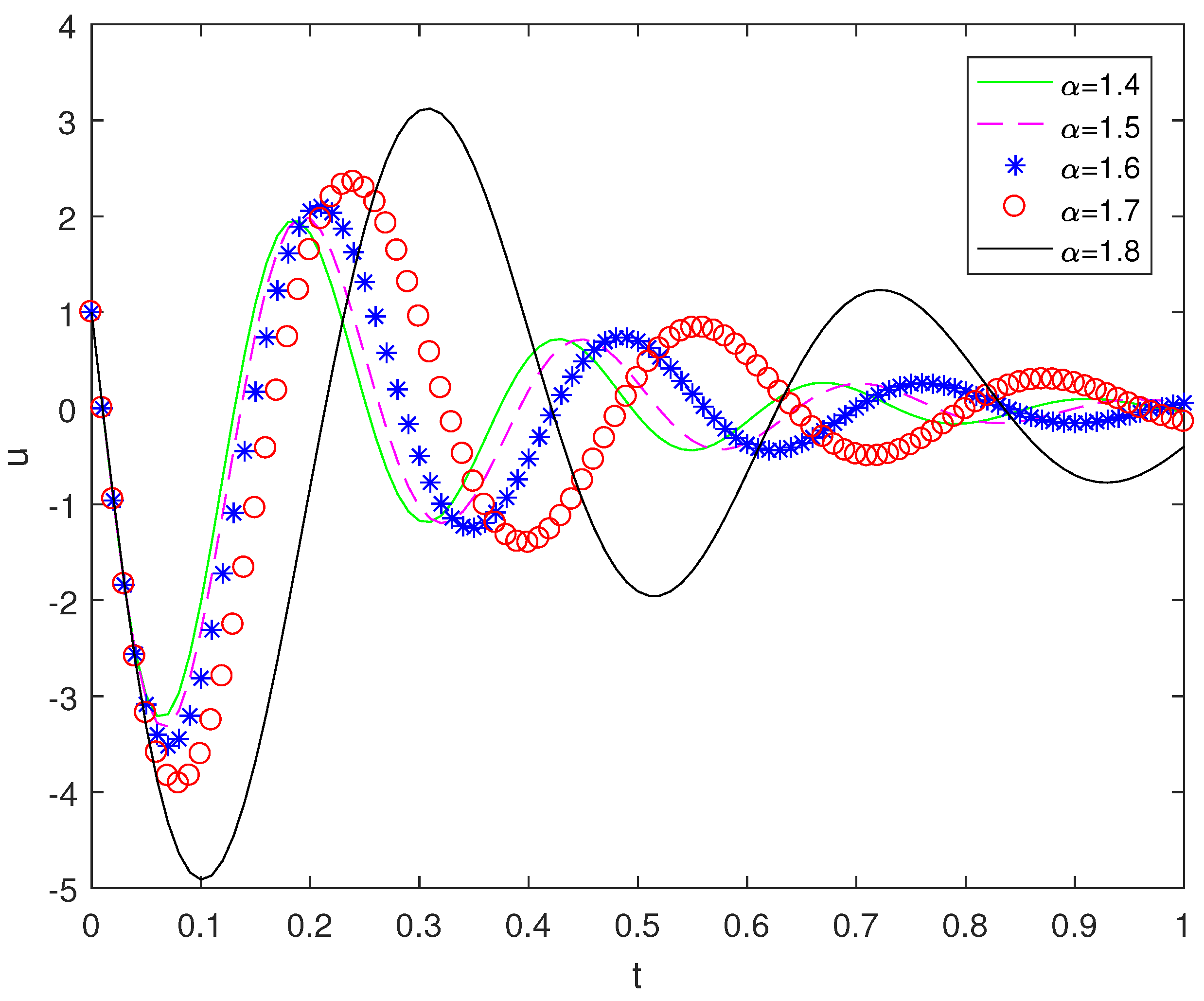
5.2. Example 2
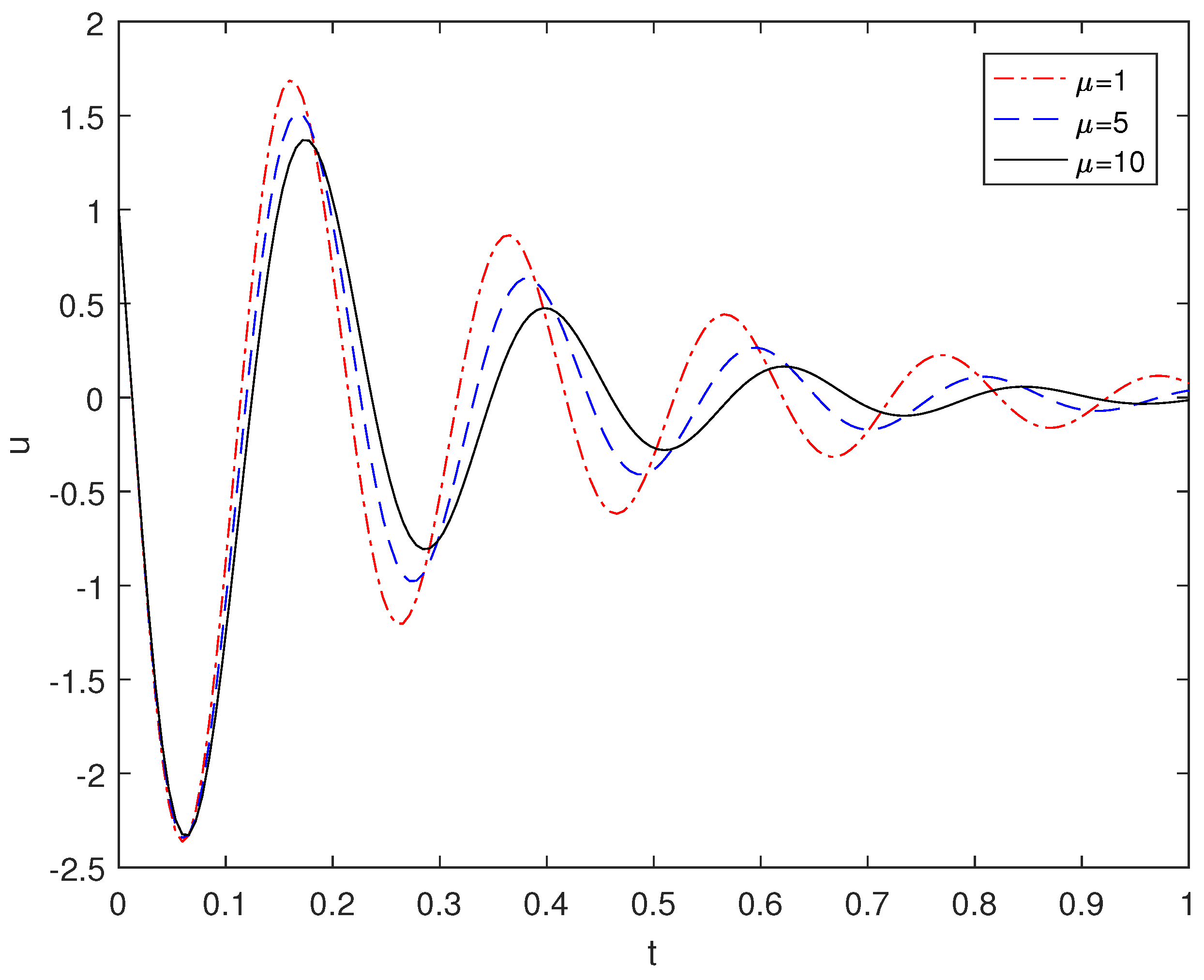
5.3. Example 3
5.4. Example 4
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, H.; Zhang, Y.; Baleanu, D.; Chen, W.; Chen, Y. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear 2018, 64, 213–321. [Google Scholar] [CrossRef]
- Mehdinejadiani, B.; Jafari, H.; Baleanu, D. Derivation of a fractional Boussinesq equation for modelling unconfined groundwater. Eur. Phys. J. Spec. Top. 2013, 222, 1805–1812. [Google Scholar] [CrossRef]
- Elsaka, H.; Ahmed, E. A fractional order network model for ZIKA. BioRxiv 2016, 039917. [Google Scholar] [CrossRef]
- Sun, H.; Chen, W.; Chen, Y. Variable-order fractional differential operators in anomalous diffusion modeling. Phys. A 2009, 388, 4586–4592. [Google Scholar] [CrossRef]
- Nigmatullin, R.R.; Zhang, W. NAFASS in action: How to control randomness? Commun. Nonlinear 2013, 18, 547–558. [Google Scholar] [CrossRef]
- Cai, W.; Chen, W.; Xu, W. Fractional modeling of Pasternak-type viscoelastic foundation. Mech. Time-Depend. Mat. 2017, 21, 119–131. [Google Scholar] [CrossRef]
- Cajić, M.; Karličić, D.; Lazarević, M. Damped vibration of a nonlocal nanobeam resting on viscoelastic foundation: Fractional derivative model with two retardation times and fractional parameters. Meccanica 2017, 52, 363–382. [Google Scholar] [CrossRef]
- Amabili, M. Nonlinear damping in large-amplitude vibrations: Modelling and experiments. Nonlinear Dyn. 2018, 93, 5–18. [Google Scholar] [CrossRef]
- Amabili, M. Derivation of nonlinear damping from viscoelasticity in case of nonlinear vibrations. Nonlinear Dyn. 2019, 97, 1785–1797. [Google Scholar] [CrossRef]
- Galucio, A.C.; Deü, J.F.; Ohayon, R. Finite element formulation of viscoelastic sandwich beams using fractional derivative operators. Comput. Mech. 2004, 33, 282–291. [Google Scholar] [CrossRef]
- Alotta, G.; Failla, G.; Zingales, M. Finite-element formulation of a nonlocal hereditary fractional-order Timoshenko beam. J. Eng. Mech. 2017, 143, D4015001. [Google Scholar] [CrossRef]
- Dönmez Demir, D.; Bildik, N.; Sınır, B.G. Linear dynamical analysis of fractionally damped beams and rods. J. Eng. Math. 2014, 85, 131–147. [Google Scholar] [CrossRef]
- Lewandowski, R.; Wielentejczyk, P. Nonlinear vibration of viscoelastic beams described using fractional order derivatives. J. Sound Vib. 2017, 399, 228–243. [Google Scholar] [CrossRef]
- Loghman, E.; Kamali, A.; Bakhtiari-Nejad, F.; Abbaszadeh, M. Nonlinear free and forced vibrations of fractional modeled viscoelastic FGM micro-beam. Appl. Math. Model. 2021, 92, 297–314. [Google Scholar] [CrossRef]
- Ansari, R.; Oskouie, M.F.; Sadeghi, F.; Bazdid-Vahdati, M. Free vibration of fractional viscoelastic Timoshenko nanobeams using the nonlocal elasticity theory. Phys. E Low Dimens. Syst. Nanostruct. 2015, 74, 318–327. [Google Scholar] [CrossRef]
- Oskouie, M.F.; Ansari, R.; Sadeghi, F. Nonlinear vibration analysis of fractional viscoelastic Euler–Bernoulli nanobeams based on the surface stress theory. AMSS 2017, 30, 416–424. [Google Scholar] [CrossRef]
- Loghman, E.; Bakhtiari-Nejad, F.; Kamali, A.; Abbaszadeh, M.; Amabili, M. Nonlinear vibration of fractional viscoelastic micro-beams. Int. J. Nonlinear Mech. 2021, 137, 103811. [Google Scholar] [CrossRef]
- Bakhtiari-Nejad, F.; Loghman, E.; Pirasteh, M. Nonlinear vibration analysis of a fractional viscoelastic Euler-Bernoulli microbeam. ASME Int. Mech. Eng. Congr. Expo. 2018, 52163, V011T01A017. [Google Scholar]
- Rossikhin, Y.A.; Shitikova, M.V. Application of fractional calculus for analysis of nonlinear damped vibrations of suspension bridges. J. Eng. Mech. 1998, 124, 1029–1036. [Google Scholar] [CrossRef]
- Catania, G.; Fasana, A.; Sorrentino, S. Finite element analysis of vibrating non-homogeneous beams with fractional derivative viscoelastic models. IFAC Proc. Vol. 2006, 39, 280–285. [Google Scholar] [CrossRef]
- Xu, J.; Chen, Y.; Tai, Y.; Xu, X.; Shi, G.; Chen, N. Vibration analysis of complex fractional viscoelastic beam structures by the wave method. Int. J. Mech. Sci. 2020, 167, 105204. [Google Scholar] [CrossRef]
- Yang, A.; Zhang, Q.; Qu, J.; Cui, Y.; Chen, Y. Solving and Numerical Simulations of Fractional-Order Governing Equation for Micro-Beams. Fractal Fract. 2023, 7, 204. [Google Scholar] [CrossRef]
- Cao, J.; Wang, Z.; Wang, Z. A Uniform Accuracy High-Order Finite Difference and FEM for Optimal Problem Governed by Time-Fractional Diffusion Equation. Fractal Fract. 2022, 6, 475. [Google Scholar] [CrossRef]
- Wang, T.; Jiang, Z.; Zhu, A.; Zhe, Y. A Mixed Finite Volume Element Method for Time-Fractional Damping Beam Vibration Problem. Fractal Fract. 2022, 6, 523. [Google Scholar] [CrossRef]
- Liu, Y.; Fang, Z.; Li, H.; He, S. A mixed finite element method for a time-fractional fourth-order partial differential equation. Appl. Math. Comput. 2014, 243, 703–717. [Google Scholar] [CrossRef]
- Liu, Y.; Du, Y.; Li, H.; He, S.; Gao, W. Finite difference/finite element method for a nonlinear time-fractional fourth-order reaction–diffusion problem. Comput. Math. Appl. 2015, 70, 573–591. [Google Scholar] [CrossRef]
- Eldred, L.B.; Baker, W.P.; Palazotto, A.N. Kelvin-Voigt versus fractional derivative model as constitutive relations for viscoelastic materials. AIAA J. 1995, 33, 547–550. [Google Scholar] [CrossRef]
- Di Paola, M.; Pirrotta, A.; Valenza, A. Visco-elastic behavior through fractional calculus: An easier method for best fitting experimental results. Mech. Mater. 2011, 43, 799–806. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus-a different approach to the analysis of viscoelastically damped structures. AIAA J. 1983, 21, 741–748. [Google Scholar] [CrossRef]
- Liu, L.; Yan, Q. Lateral vibration of single pile in viscoelastic soil described by fractional derivative model. Eng. Mech. 2011, 28, 139–145. [Google Scholar]
- Koeller, R. Applications of fractional calculus to the theory of viscoelasticity. J. Appl. Mech. 1984, 51, 299–307. [Google Scholar] [CrossRef]
- Ciarlet, P. The Finite Element Method for Elliptic Problems; North-Holland Publishing Company: Amsterdam, The Netherlands, 1978. [Google Scholar]

| -Error | Order | -Error | Order | ||
|---|---|---|---|---|---|
| = 1.3 | - | - | |||
| 0.9070 | 0.9070 | ||||
| 0.9543 | 0.9542 | ||||
| 0.9774 | 0.9773 | ||||
| = 1.5 | - | - | |||
| 0.9070 | 0.9070 | ||||
| 0.9543 | 0.9542 | ||||
| 0.9774 | 0.9773 | ||||
| = 1.7 | - | - | |||
| 0.9070 | 0.9070 | ||||
| 0.9543 | 0.9542 | ||||
| 0.9774 | 0.9773 |
| -Error | Order | -Error | Order | ||
|---|---|---|---|---|---|
| = 1.3 | - | - | |||
| 3.9125 | 2.9408 | ||||
| 3.9699 | 2.9829 | ||||
| 3.9666 | 2.9914 | ||||
| = 1.5 | - | - | |||
| 3.9093 | 2.9405 | ||||
| 3.9462 | 2.9819 | ||||
| 3.8772 | 2.9887 | ||||
| = 1.7 | - | - | |||
| 3.8969 | 2.9391 | ||||
| 3.8558 | 2.9764 | ||||
| 3.5455 | 2.9695 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, X.; Zhu, A.; Yin, Z.; Ji, P. Hermite Finite Element Method for Time Fractional Order Damping Beam Vibration Problem. Fractal Fract. 2023, 7, 739. https://doi.org/10.3390/fractalfract7100739
Sun X, Zhu A, Yin Z, Ji P. Hermite Finite Element Method for Time Fractional Order Damping Beam Vibration Problem. Fractal and Fractional. 2023; 7(10):739. https://doi.org/10.3390/fractalfract7100739
Chicago/Turabian StyleSun, Xinxin, Ailing Zhu, Zhe Yin, and Pengfei Ji. 2023. "Hermite Finite Element Method for Time Fractional Order Damping Beam Vibration Problem" Fractal and Fractional 7, no. 10: 739. https://doi.org/10.3390/fractalfract7100739
APA StyleSun, X., Zhu, A., Yin, Z., & Ji, P. (2023). Hermite Finite Element Method for Time Fractional Order Damping Beam Vibration Problem. Fractal and Fractional, 7(10), 739. https://doi.org/10.3390/fractalfract7100739






