Fractional View Analysis of Swift–Hohenberg Equations by an Analytical Method and Some Physical Applications
Abstract
:1. Introduction
- Type-I
- Swift–Hohenberg equations:
- Type-II
- Swift–Hohenberg equations:
- Type-III
- Swift–Hohenberg equations:
2. Basic Definitions
3. General Discussion of Method
4. Convergence Analysis
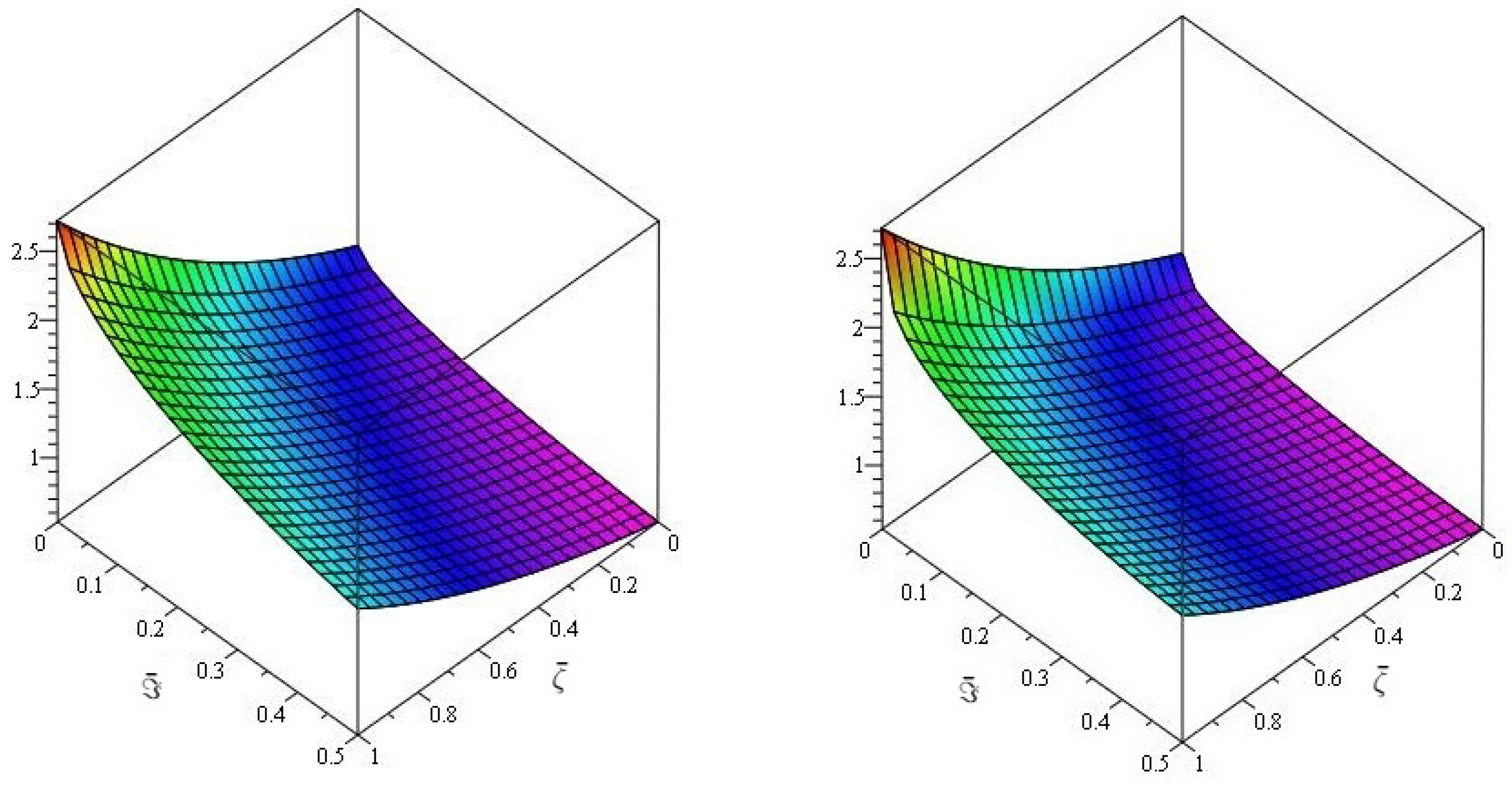
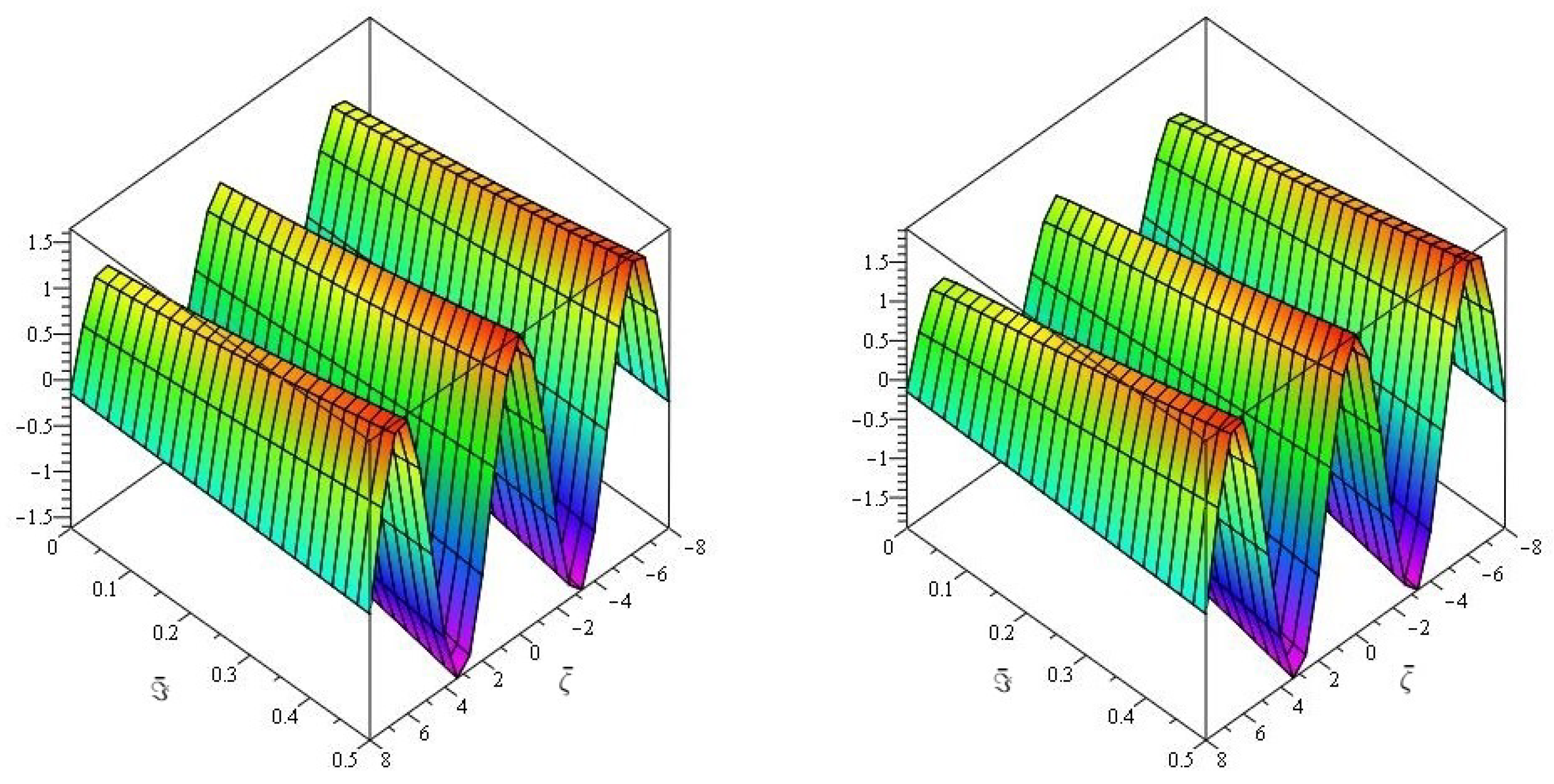
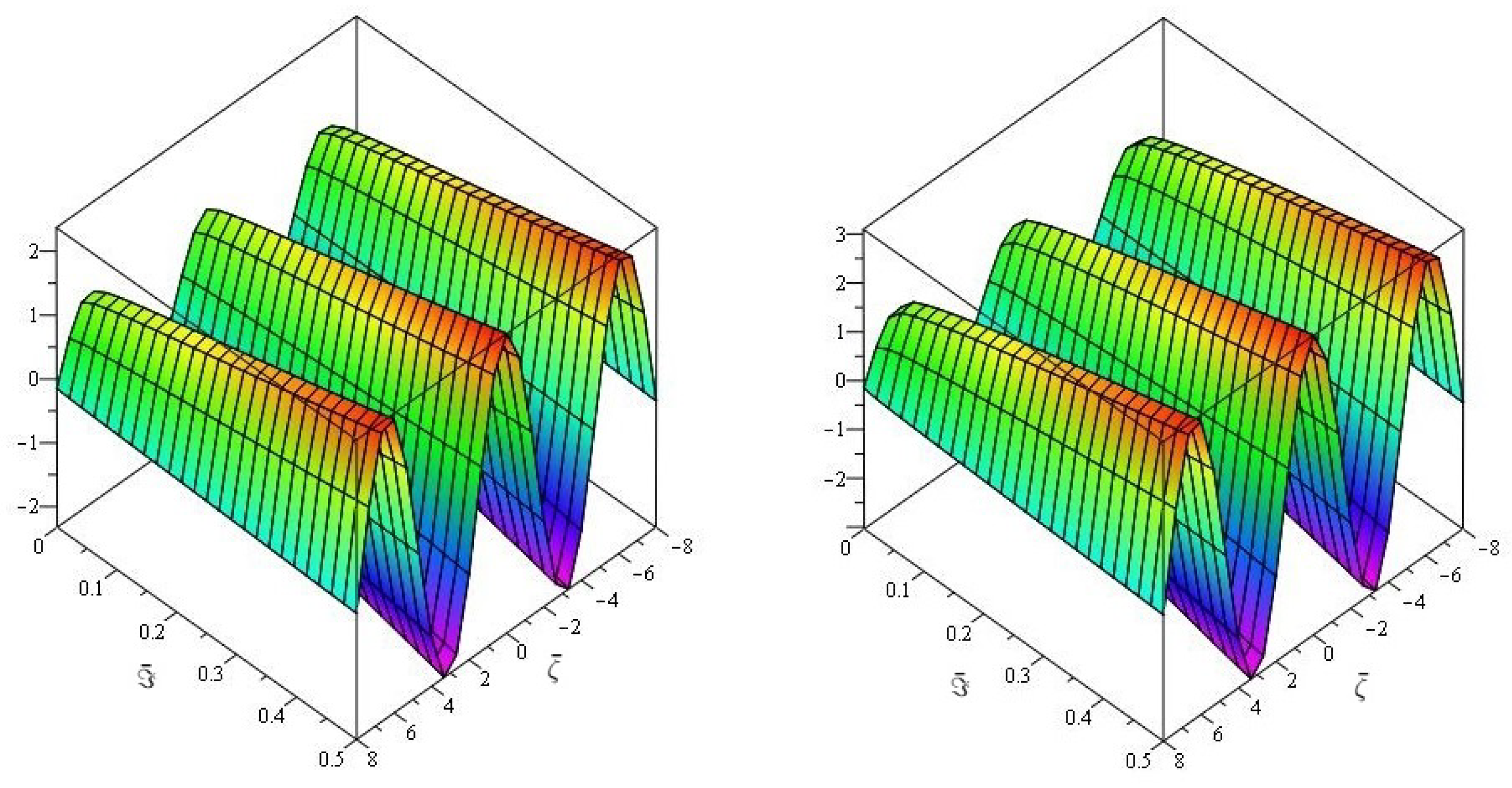
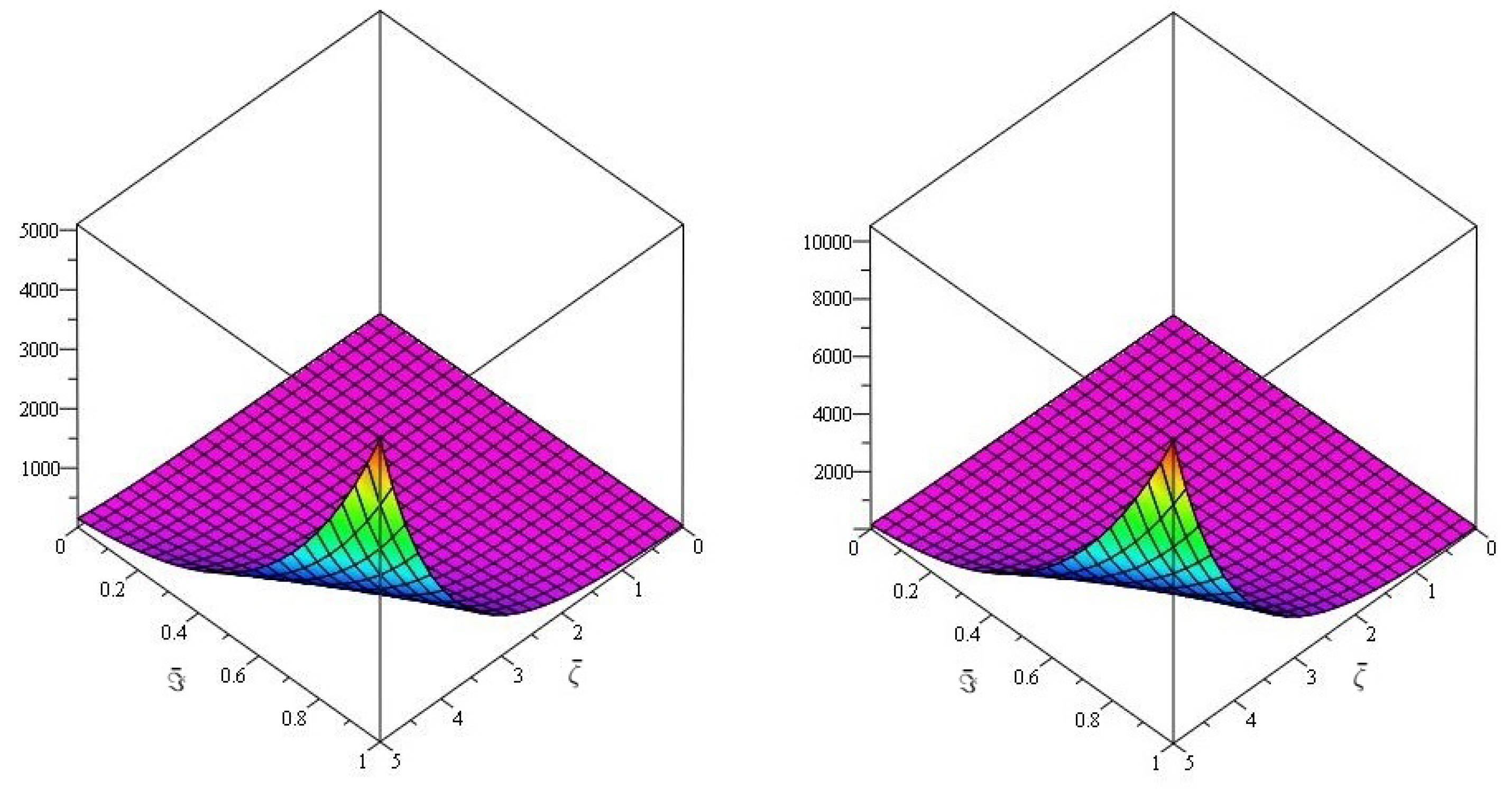
5. Applications
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sabatier, J.A.T.M.J.; Agrawal, O.P.; Machado, J.T. Advances in Fractional Calculus; Springer: Dordrecht, The Netherlands, 2007; Volume 4. [Google Scholar]
- Atangana, A.; Baleanu, D. Caputo–Fabrizio derivative applied to groundwater flow within confined aquifer. J. Eng. Mech. 2017, 143, D4016005. [Google Scholar] [CrossRef]
- Aljahdaly, N.H.; Agarwal, R.P.; Botmart, T. The analysis of the fractional-order system of third-order KdV equation within different operators. Alex. Eng. J. 2022, 61, 11825–11834. [Google Scholar] [CrossRef]
- Mukhtar, S.; Noor, S. The Numerical Investigation of a Fractional-Order Multi-Dimensional Model of Navier-Stokes Equation via Novel Techniques. Symmetry 2022, 14, 1102. [Google Scholar] [CrossRef]
- Morales-Delgado, V.F.; Gómez-Aguilar, J.F.; Yépez-Martínez, H.; Baleanu, D.; Escobar-Jimenez, R.F.; Olivares-Peregrino, V.H. Laplace homotopy analysis method for solving linear partial differential equations using a fractional derivative with and without kernel singular. Adv. Differ. Equ. 2016, 2016, 164. [Google Scholar] [CrossRef]
- Li, Y.; Nohara, B.T.; Liao, S. Series solutions of coupled Van der Pol equation by means of homotopy analysis method. J. Math. Phys. 2010, 51, 063517. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Baleanu, D. Fractional Whitham-Broer-Kaup Equations within Modified Analytical Approaches. Axioms 2019, 8, 125. [Google Scholar] [CrossRef]
- El-Tantawy, S.A.; Shan, S.A.; Mustafa, N.; Alshehri, M.H.; Duraihem, F.Z.; Turki, N.B. Homotopy perturbation and Adomian decomposition methods for modeling the nonplanar structures in a bi-ion ionospheric superthermal plasma. Eur. Phys. J. Plus 2021, 136, 561. [Google Scholar] [CrossRef]
- Kashkari, B.S.; El-Tantawy, S.A.; Salas, A.H.; El-Sherif, L.S. Homotopy perturbation method for studying dissipative nonplanar solitons in an electronegative complex plasma. Chaos Solitons Fractals 2020, 130, 109457. [Google Scholar] [CrossRef]
- Alshehry, A.S.; Imran, M.; Weera, W. Fractional-View Analysis of Fokker-Planck Equations by ZZ Transform with Mittag-Leffler Kernel. Symmetry 2022, 14, 1513. [Google Scholar] [CrossRef]
- Wu, S. Nonlinear information data mining based on time series for fractional differential operators. Chaos Interdiscip. J. Nonlinear Sci. 2019, 29, 013114. [Google Scholar] [CrossRef]
- Lakshmikantham, V.; Vatsala, A.S. Basic theory of fractional differential equations. Nonlinear Anal. Theory Methods Appl. 2008, 69, 2677–2682. [Google Scholar] [CrossRef]
- Prakasha, D.G.; Veeresha, P.; Baskonus, H.M. Residual power series method for fractional Swift–Hohenberg equation. Fractal Fract. 2019, 3, 9. [Google Scholar] [CrossRef]
- Swift, J.; Hohenberg, P.C. Hydrodynamics fluctuations at the convective instability. Phys. Rev. A 1977, 15, 319–328. [Google Scholar] [CrossRef]
- Lega, L.; Moloney, J.V.; Newell, A.C. Swift–Hohenberg equation for lasers. Phys. Rev. Lett. 1994, 73, 2978–2981. [Google Scholar] [CrossRef]
- Pomeau, Y.; Zaleski, S. Dislocation motion in cellular structures. Phys. Rev. A 1983, 27, 2710–2726. [Google Scholar] [CrossRef]
- Peletier, L.A.; Rottschafer, V. Large time behaviour of solutions of the SwiftHohenberg equation. R. Acad. Sci. Paris Ser. I 2003, 336, 225–230. [Google Scholar] [CrossRef]
- Ahmadian, A.; Ismail, F.; Salahshour, S.; Baleanu, D.; Ghaemi, F. Uncertain viscoelastic models with fractional order: A new spectral tau method to study the numerical simulations of the solution. Commun. Nonlinear Sci. Numer. Simul. 2017, 53, 44–64. [Google Scholar] [CrossRef]
- Fife, P.C. Pattern formation in gradient systems. In Handbook of Dynamical Systems; Esevier: Amsterdam, The Netherlands, 2002; Volume 2, pp. 679–719. [Google Scholar]
- Hoyle, R.B. Pattern Formation; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Ryabov, P.N.; Kudryashov, N.A. Nonlinear waves described by the generalized Swift–Hohenberg equation. J. Phys. Conf. Ser. 2017, 788, 012032. [Google Scholar] [CrossRef]
- Vishal, K.; Kumar, S.; Das, S. Application of homotopy analysis method for fractional Swift Hohenberg equation—Revisited. Appl. Math. Model. 2012, 36, 3630–3637. [Google Scholar] [CrossRef]
- Khan, N.A.; Khan, N.U.; Ayaz, M.; Mahmood, A. Analytical methods for solving the time-fractional Swift–Hohenberg (S-H) equation. Comput. Math. Appl. 2011, 61, 2181–2185. [Google Scholar] [CrossRef] [Green Version]
- Li, W.; Pang, Y. An iterative method for time-fractional Swift–Hohenberg equation. Adv. Math. Phys. 2018, 2018, 2405432. [Google Scholar] [CrossRef]
- Vishal, K.; Das, S.; Ong, S.H.; Ghosh, P. On the solutions of fractional Swift Hohenberg equation with dispersion. Appl. Math. Comput. 2013, 219, 5792–5801. [Google Scholar] [CrossRef]
- Rawashdeh, M.S.; Al-Jammal, H. New approximate solutions to fractional nonlinear systems of partial differential equations using the FNDM. Adv. Differ. Equ. 2016, 2016, 235. [Google Scholar] [CrossRef]
- Rawashdeh, M.S.; Al-Jammal, H. Numerical solutions for systems of nonlinear fractional ordinary differential equations using the FNDM. Mediterr. J. Math. 2016, 13, 4661–4677. [Google Scholar] [CrossRef]
- Rawashdeh, M.S. The fractional natural decomposition method: Theories and applications. Math. Methods Appl. Sci. 2017, 40, 2362–2376. [Google Scholar] [CrossRef]
- Aljahdaly, N.H.; Akgul, A.; Shah, R.; Mahariq, I.; Kafle, J. A comparative analysis of the fractional-order coupled Korteweg-De Vries equations with the Mittag-Leffler law. J. Math. 2022, 2022, 8876149. [Google Scholar] [CrossRef]
- Shah, N.A.; Hamed, Y.S.; Abualnaja, K.M.; Chung, J.D.; Shah, R.; Khan, A. A comparative analysis of fractional-order kaup-kupershmidt equation within different operators. Symmetry 2022, 14, 986. [Google Scholar] [CrossRef]
- Zhou, M.X.; Kanth, A.S.V.; Aruna, K.; Raghavendar, K.; Rezazadeh, H.; Inc, M.; Aly, A.A. Numerical solutions of time fractional Zakharov-Kuznetsov equation via natural transform decomposition method with nonsingular kernel derivatives. J. Funct. Spaces 2021, 2021, 9884027. [Google Scholar] [CrossRef]
- El-Tantawy, S.A.; Salas, A.H.; Alharthi, M.R. Novel analytical cnoidal and solitary wave solutions of the Extended Kawahara equation. Chaos Solitons Fractals 2021, 147, 110965. [Google Scholar] [CrossRef]
- El-Tantawy, S.A.; Salas, A.H.; Alharthi, M.R. On the dissipative extended Kawahara solitons and cnoidal waves in a collisional plasma: Novel analytical and numerical solutions. Phys. Fluids 2021, 33, 106101. [Google Scholar] [CrossRef]
- El-Tantawy, S.A.; Salas, A.H.; Alharthi, M.R. Novel anlytical solution to the damped Kawahara equation and its application for modeling the dissipative nonlinear structures in a fluid medium. J. Ocean Eng. Sci. 2021, 33, 106101. [Google Scholar] [CrossRef]







| Exact Solution | NDTM | AE of NDTM | AE of NDTM | AE of NDTM | |
|---|---|---|---|---|---|
| 0 | 0.000000000000 | 0.000000000000 | 0.0000000000 | 0.0000000000 | 0.0000000000 |
| 0.1 | 0.100000900000 | 0.100001000000 | 1.0000000000 | 2.2624000000 | 9.1005000000 |
| 0.2 | 0.200001600000 | 0.200002000000 | 4.0000000000 | 4.7247000000 | 1.8401000000 |
| 0.3 | 0.300002100000 | 0.300003000000 | 9.0000000000 | 7.3870000000 | 2.7901500000 |
| 0.4 | 0.400002400000 | 0.400004000000 | 1.6000000000 | 1.0249300000 | 3.7602000000 |
| 0.5 | 0.500002500000 | 0.500005000000 | 2.5000000000 | 1.3311600000 | 4.7502500000 |
| 0.6 | 0.600002400000 | 0.600006000000 | 3.6000000000 | 1.6574000000 | 5.7603000000 |
| 0.7 | 0.700002100000 | 0.700007000000 | 4.9000000000 | 2.0036200000 | 6.7903500000 |
| 0.8 | 0.800001600000 | 0.800008000000 | 6.4000000000 | 2.3698600000 | 7.8404000000 |
| 0.9 | 0.900000900000 | 0.900009000000 | 8.1000000000 | 2.7561000000 | 8.9104500000 |
| 1.0 | 1.000000000000 | 1.000010000000 | 1.0000000000 | 3.1624000000 | 1.0000500000 |
| 0.2 | 5.2120000000 × 10 | 3.6017600000 × 10 | 2.0943200000 × 10 | 6.4513000000 × 10 | 6.4513000000 × 10 | |
| 0.4 | 1.0384330000 × 10 | 7.1776700000 × 10 | 4.1757400000 × 10 | 1.2893800000 × 10 | 1.2893800000 × 10 | |
| 0.1 | 0.6 | 1.5474640000 × 10 | 1.0698980000 × 10 | 6.2282300000 × 10 | 1.9293900000 × 10 | 1.9293900000 × 10 |
| 0.8 | 2.0443500000 × 10 | 1.4139470000 × 10 | 8.2379300000 × 10 | 2.5631800000 × 10 | 2.5631800000 × 10 | |
| 1 | 2.5253100000 × 10 | 1.7473930000 × 10 | 1.0191440000 × 10 | 3.1886900000 × 10 | 3.1886900000 × 10 | |
| 0.2 | 5.8984400000 × 10 | 4.2773700000 × 10 | 2.7455400000 × 10 | 1.2876600000 × 10 | 1.2876600000 × 10 | |
| 0.4 | 1.1759660000 × 10 | 8.5314400000 × 10 | 5.4809200000 × 10 | 2.5761600000 × 10 | 2.5761600000 × 10 | |
| 0.2 | 0.6 | 1.7533840000 × 10 | 1.2726080000 × 10 | 8.1829600000 × 10 | 3.8562800000 × 10 | 3.8562800000 × 10 |
| 0.8 | 2.3179040000 × 10 | 1.6832640000 × 10 | 1.0835570000 × 10 | 5.1239700000 × 10 | 5.1239700000 × 10 | |
| 1 | 2.8655420000 × 10 | 2.0823970000 × 10 | 1.3423590000 × 10 | 6.3748800000 × 10 | 6.3748800000 × 10 | |
| 0.2 | 6.5642700000 × 10 | 4.9400400000 × 10 | 3.3914500000 × 10 | 1.9276800000 × 10 | 1.9276800000 × 10 | |
| 0.4 | 1.3096920000 × 10 | 9.8624000000 × 10 | 6.7785000000 × 10 | 3.8605500000 × 10 | 3.8605500000 × 10 | |
| 0.3 | 0.6 | 1.9537820000 × 10 | 1.4720680000 × 10 | 1.0127840000 × 10 | 5.7806700000 × 10 | 5.7806700000 × 10 |
| 0.8 | 2.5842940000 × 10 | 1.9484160000 × 10 | 1.3421460000 × 10 | 7.6821500000 × 10 | 7.6821500000 × 10 | |
| 1 | 3.1969960000 × 10 | 2.4123230000 × 10 | 1.6641870000 × 10 | 9.5586800000 × 10 | 9.5586800000 × 10 | |
| 0.2 | 7.2188300000 × 10 | 5.5944200000 × 10 | 4.0328700000 × 10 | 2.5652100000 × 10 | 2.5652100000 × 10 | |
| 0.4 | 1.4414910000 × 10 | 1.1180020000 × 10 | 8.0703200000 × 10 | 5.1423300000 × 10 | 5.1423300000 × 10 | |
| 0.4 | 0.6 | 2.1514870000 × 10 | 1.6697170000 × 10 | 1.2065920000 × 10 | 7.7025600000 × 10 | 7.7025600000 × 10 |
| 0.8 | 2.8472250000 × 10 | 2.2112730000 × 10 | 1.5999330000 × 10 | 1.0237930000 × 10 | 1.0237930000 × 10 | |
| 1 | 3.5242520000 × 10 | 2.7394880000 × 10 | 1.9850950000 × 10 | 1.2740070000 × 10 | 1.2740070000 × 10 | |
| 0.2 | 7.8654200000 × 10 | 6.2423400000 × 10 | 4.6702100000 × 10 | 3.2001400000 × 10 | 3.2001400000 × 10 | |
| 0.4 | 1.5720280000 × 10 | 1.2488040000 × 10 | 9.3572800000 × 10 | 6.4215100000 × 10 | 6.4215100000 × 10 | |
| 0.5 | 0.6 | 2.3474550000 × 10 | 1.8660800000 × 10 | 1.3998180000 × 10 | 9.6219500000 × 10 | 9.6219500000 × 10 |
| 0.8 | 3.1079660000 × 10 | 2.4725350000 × 10 | 1.8570540000 × 10 | 1.2791210000 × 10 | 1.2791210000 × 10 | |
| 1 | 3.8489010000 × 10 | 3.0647790000 × 10 | 2.3052760000 × 10 | 1.5918960000 × 10 | 1.5918960000 × 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almutlak, S.A.; Shah, R.; Weera, W.; El-Tantawy, S.A.; El-Sherif, L.S. Fractional View Analysis of Swift–Hohenberg Equations by an Analytical Method and Some Physical Applications. Fractal Fract. 2022, 6, 524. https://doi.org/10.3390/fractalfract6090524
Almutlak SA, Shah R, Weera W, El-Tantawy SA, El-Sherif LS. Fractional View Analysis of Swift–Hohenberg Equations by an Analytical Method and Some Physical Applications. Fractal and Fractional. 2022; 6(9):524. https://doi.org/10.3390/fractalfract6090524
Chicago/Turabian StyleAlmutlak, Salemah A., Rasool Shah, Wajaree Weera, Samir A. El-Tantawy, and Lamiaa S. El-Sherif. 2022. "Fractional View Analysis of Swift–Hohenberg Equations by an Analytical Method and Some Physical Applications" Fractal and Fractional 6, no. 9: 524. https://doi.org/10.3390/fractalfract6090524
APA StyleAlmutlak, S. A., Shah, R., Weera, W., El-Tantawy, S. A., & El-Sherif, L. S. (2022). Fractional View Analysis of Swift–Hohenberg Equations by an Analytical Method and Some Physical Applications. Fractal and Fractional, 6(9), 524. https://doi.org/10.3390/fractalfract6090524







