On the Physical Fractional Modulations on Langmuir Plasma Structures
Abstract
:1. Introduction
- (i)
- ;
- (ii)
- (iii)
- (vi)
- (v)
- If is differentiable, thus
2. Mathematical Analysis
Solutionsof Equation (1) via a Unified Solver Approach
- The presented solver in this study can be applied for the huge classes of NFPDEs.
- This solver can be easily extended to solve stochastic NFPDEs.
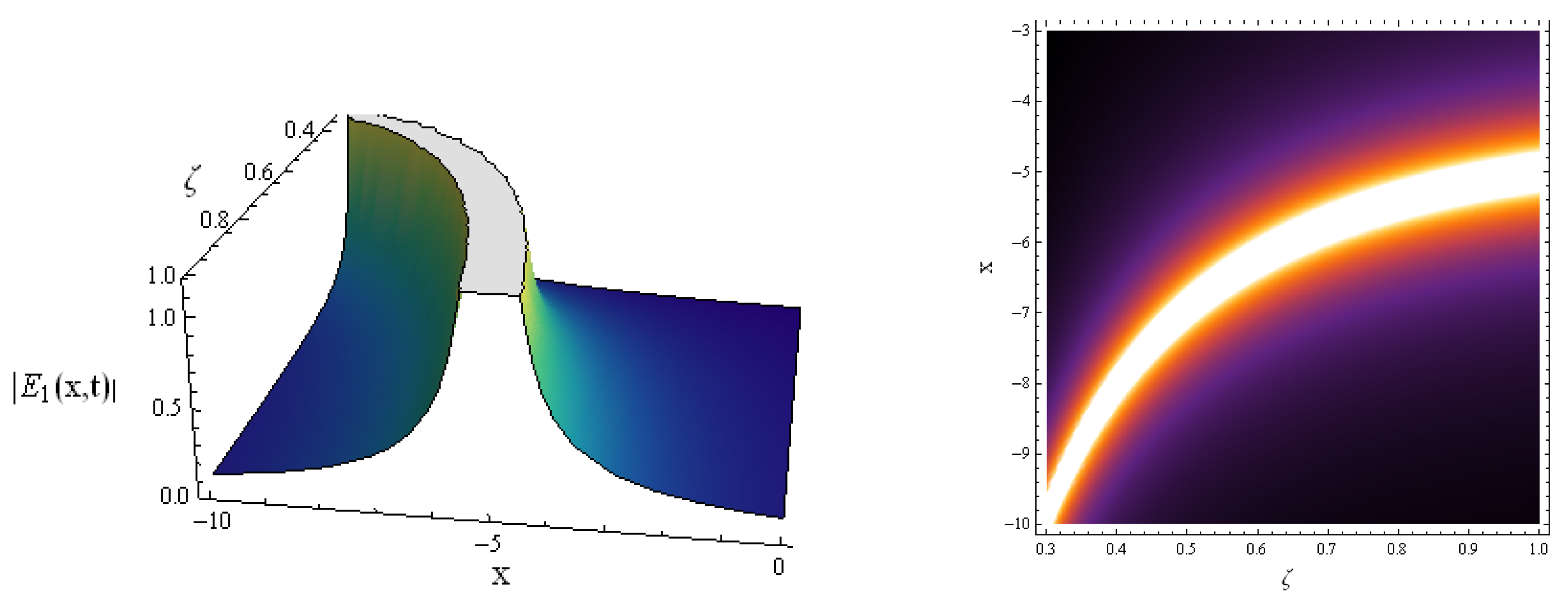
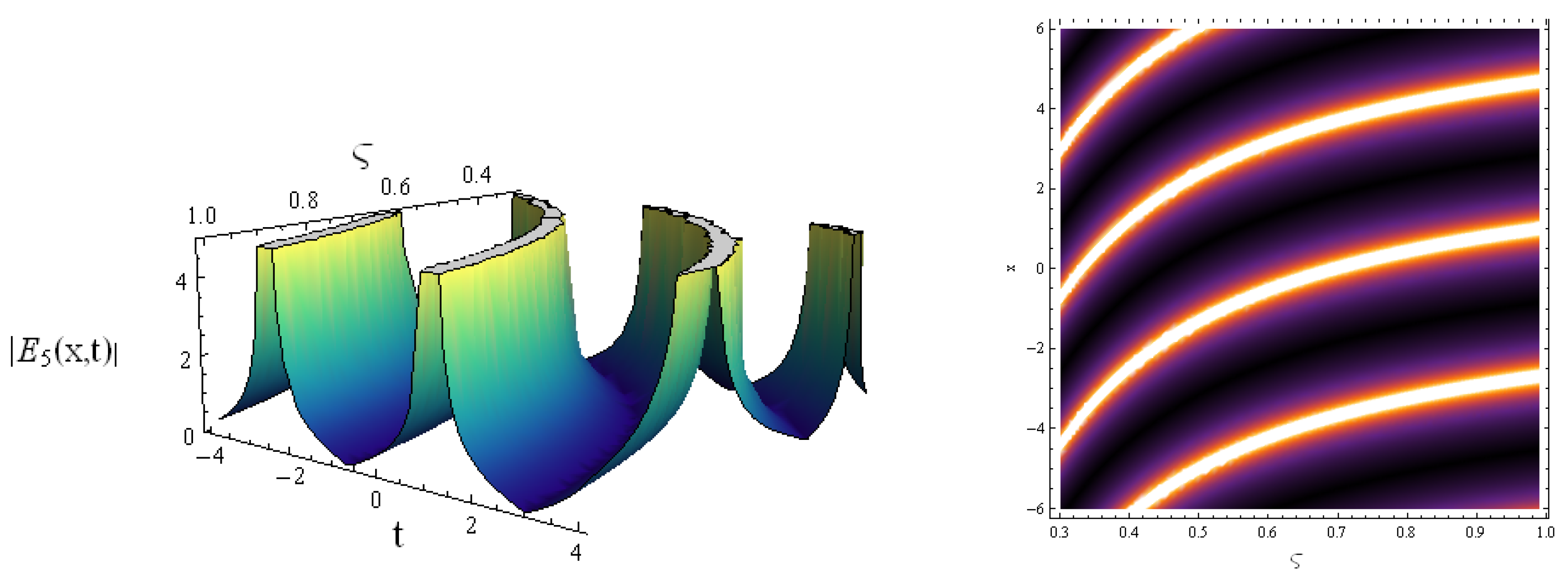
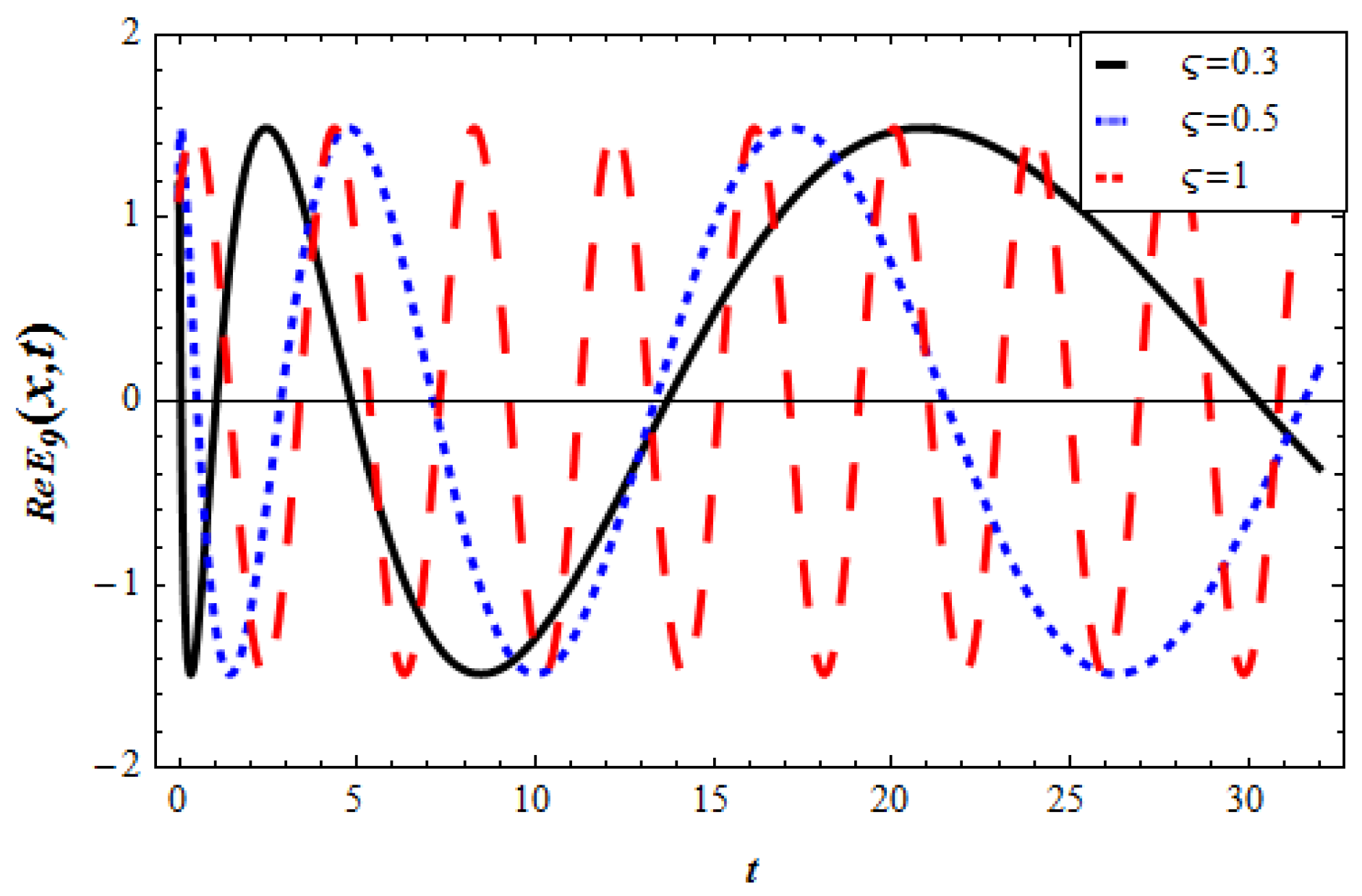
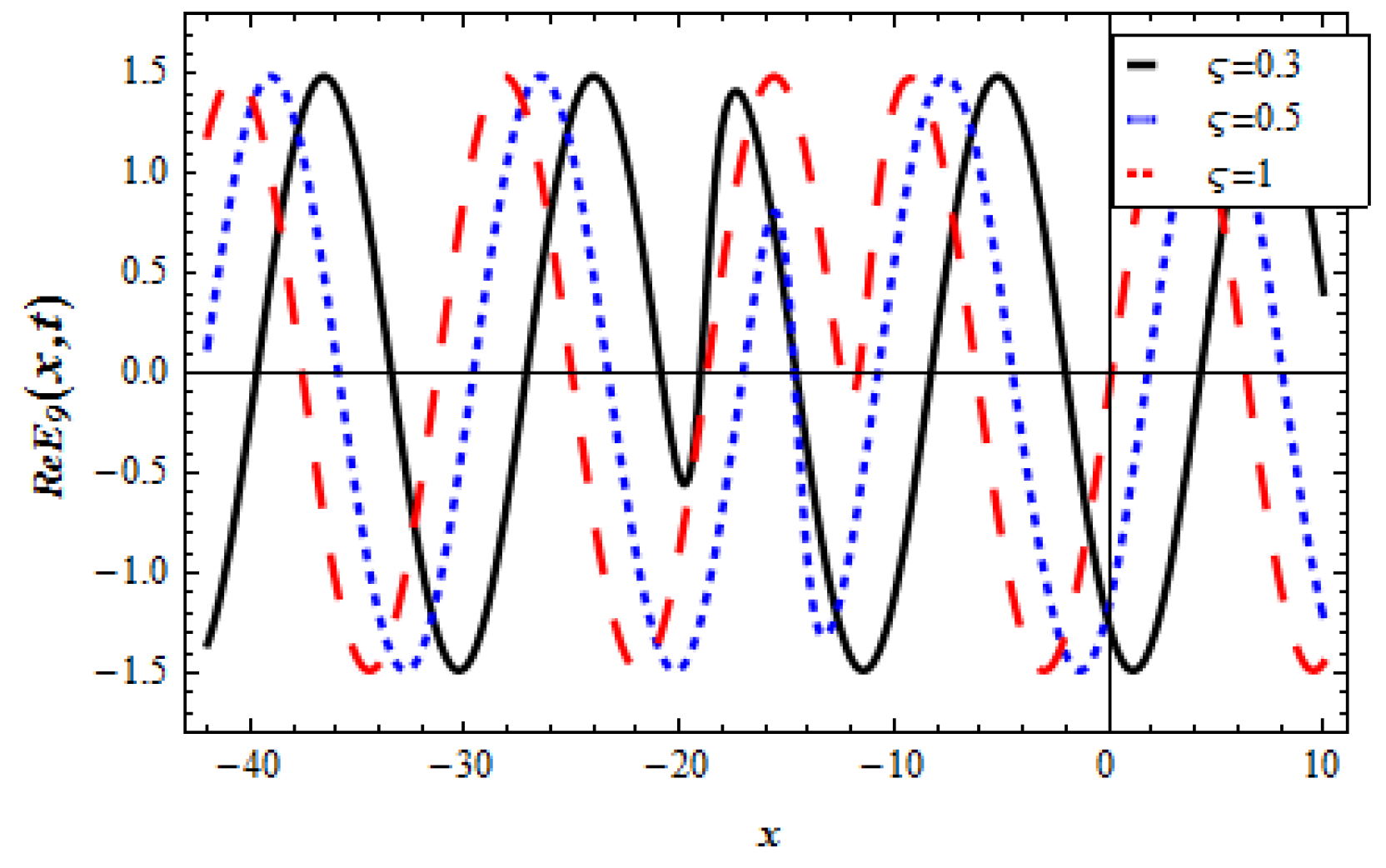
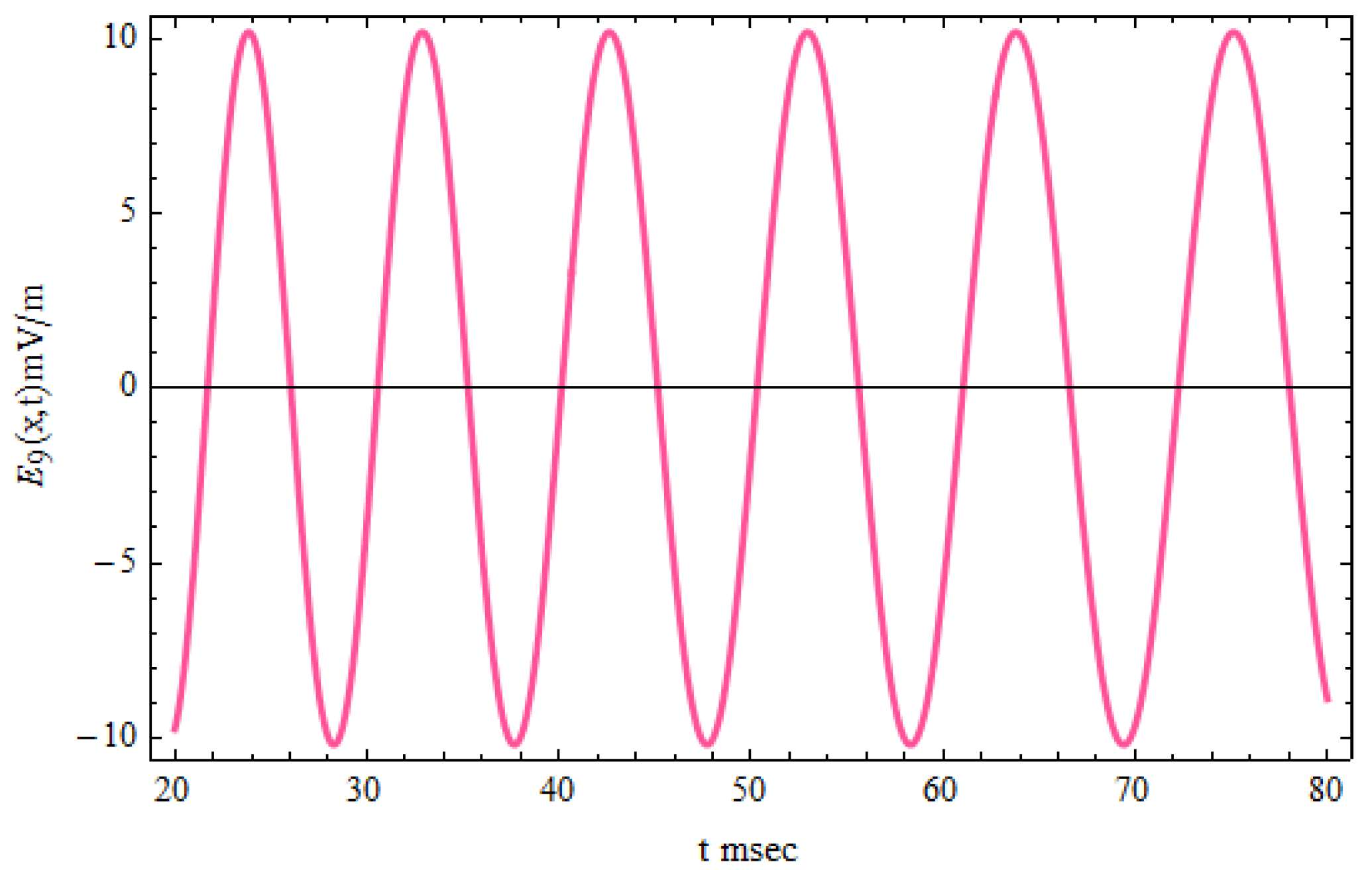
3. Physical Interpretation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kaplan, M.K.; Bekir, A.E. A novel analytical method for time-fractional differential equations. Optik 2016, 127, 8209–8214. [Google Scholar] [CrossRef]
- Tchier, F.; Inc, M.; Korpinar, Z.S.; Baleanu, D. Solution of the time fractional reaction-diffusion equations with residual power series method. Adv. Mech. Eng. 2016, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Zafar, A. Rational exponential solutions of conformable spacetime fractional equal width equations. Nonlinear Eng. 2019, 8, 350–355. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E. A note on Riccati-Bernoulli sub-ODE method combined with complex transform method applied to fractional differential equations. Nonlinear Eng. Model. Appl. 2018, 7, 279–285. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; AlKhidhr, H. Closed-form solutions to the conformable space-time fractional simplified MCH equation and time fractional Phi-4 equation. Results Phys. 2020, 18, 103294. [Google Scholar] [CrossRef]
- Sarwar, S.; Iqbal, S. Stability analysis, dynamical behavior and analytical solutions of nonlinear fractional differential system arising in chemical reaction. Chin. J. Phys. 2018, 56, 374–384. [Google Scholar] [CrossRef]
- Hosseini, K.; Samadani, F.; Kumar, D.; Faridi, M. New optical solitons of cubic-quartic nonlinear Schrzödinger equation. Optik 2018, 157, 1101–1105. [Google Scholar] [CrossRef]
- Foukrach, D. Approximate solution to a Bürgers system with time and space fractional derivatives using Adomian decomposition method. J. Interdiscip. Math. 2018, 21, 111–125. [Google Scholar] [CrossRef]
- Tong, B.; He, Y.; Wei, L.; Zhang, X. A generalized fractional sub-equation method for fractional differential equations with variable coefficients. Phys. Lett. A 2012, 376, 2588–2590. [Google Scholar] [CrossRef]
- Lu, B. The first integral method for some time fractional differential equations. J. Math. Anal. Appl. 2012, 395, 684–693. [Google Scholar] [CrossRef] [Green Version]
- Younis, M.; Rizvi, S.T.R. Dispersive dark optical soliton in (2 + 1)-dimensions by ()-expansion with dual-power law nonlinearity. Optik 2015, 126, 5812–5814. [Google Scholar] [CrossRef]
- Ray, S.S.; Sahoo, S. A novel analytical method with fractional complex transform for new exact solutions of time-fractional fifth-order Sawada-Kotera equation. Rep. Math. Phys. 2015, 75, 63–72. [Google Scholar] [CrossRef]
- Hosseini, K.; Mayeli, P.; Ansari, R. Modified Kudryashov method for solving the conformable time fractional Klein-Gordon equations with quadratic and cubic nonlinearities. Optik 2017, 130, 737–742. [Google Scholar] [CrossRef]
- Faraz, N.; Khan, Y.; Jafari, H.; Yildirim, A.; Madani, M. Fractional variational iteration method via modified Riemann- Liouville derivative. J. King Saud Univ. Sci. 2011, 23, 413–417. [Google Scholar] [CrossRef] [Green Version]
- Zhang, S.; Zong, Q.-A.; Liu, D.; Gao, Q. A generalized exp-function method for fractional riccati differential equations. Commun. Fract. Calc. 2010, 1, 48–51. [Google Scholar]
- Khodadad, F.S.; Nazari, F.; Eslami, M.; Rezazadeh, H. Soliton solutions of the conformable fractional Zakharov–Kuznetsov equation with dual-power law nonlinearity. Opt. Quantum Electron. 2017, 49, 384. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations; Academic Press: San Diego, CA, USA, 1999. [Google Scholar]
- Khalil, R.; Horani, M.A.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Abdeljawad, T. On conformable fractional calculus. J. Comput. Appl. Math. 2015, 279, 57–66. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Tariq, H.; Eslami, M.; Mirzazadeh, M.; Zhou, Q. New exact solutions of nonlinear conformable time-fractional Phi-4 equation. Chin. J. Phys. 2018, 56, 2805–2816. [Google Scholar] [CrossRef]
- Zeliha, K. Some analytical solutions by mapping methods for non-linear conformable time-fractional Phi-4 equation. Therm. Sci. 2019, 23, 1815–1822. [Google Scholar]
- Khader, M.M.; Kumar, S. An accurate numerical method for solving the linear fractional Klein-Gordon equation. Math. Methods Appl. Sci. 2014, 37, 2972–2979. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Hassan, S.Z.; Alomair, R.A.; Alsaleh, D.M. Fundamental solutions for the conformable time fractional Phi-4 and space-time fractional simplified MCH equations. AIMS Math. 2021, 6, 6555–6568. [Google Scholar] [CrossRef]
- Liang, Y.; Ye, A.Q.; Chen, W.; Gatto, R.G.; Colon-Perez, L.; Mareci, T.H.; Magin, R.L. A fractal derivative model for the characterization of anomalous diffusion in magnetic resonance imaging. Commun. Nonlinear Sci. Numer. Simul. 2016, 39, 529–537. [Google Scholar] [CrossRef]
- Wang, K.J.; Li, G.; Liu, J.H.; Wang, G.D. Solitary waves of the fractal regularized long-wave equation traveling along an unsmooth boundary. Fractals 2022, 30, 2250008. [Google Scholar] [CrossRef]
- Wang, K.J.; Wang, G.D.; Shi, F. Abundant exact traveling wave solutions to the local fractional (3 + 1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Fractals 2022, 30, 2250064. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Pushkarev, A.N.; Sagdeev, R.Z.; Soloviev, S.I.; Shapiro, V.D.; Shvets, V.F.; Shevchenko, V.I. Throughout-modelling of the one-dimensional Langmuir turbulence. Sov. Phys. Dokl. 1989, 34, 248–251. [Google Scholar]
- Dyachenko, A.I.; Pushkarev, A.N.; Rubenchik, A.M.; Sagdeevc, R.Z.; Shvetsa, V.F.; Zakharovd, V.E. Computer simulation of Langmuir collapse. Phys. D Nonlinear Phenom. 1991, 52, 78–102. [Google Scholar] [CrossRef]
- Rubenchik, A.M.; Zakharov, V.E. Strong Langmuir turbulence in laser plasma. In Handbook of Plasma Physics; Elsevier Science Publishers: Amsterdam, The Netherlands, 1991; Volume 3, pp. 335–360. [Google Scholar]
- Ratcliffe, H.; Brady, C.S.; Rozenan, M.B.C.; Nakariakov, V.M. A comparison of weak-turbulence and particle-in-cell simulations of weak electron-beam plasma interaction. AIP Phys. Plasmas 2014, 21, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Rezazadeh, H.; Adel, W.; Tala-Tebue, E.; Yao, S.W.; Inc, M. Bright and singular soliton solutions to the Atangana-Baleanu fractional system of equations for the ISALWs. J. King Saud Univ. Sci. 2021, 33, 101420. [Google Scholar] [CrossRef]
- Younas, U.; Seadawy, A.R.; Younis, M.; Rizvi, S.T.R. Construction of analytical wave solutions to the conformable fractional dynamical system of ion sound and Langmuir waves. Waves Random Complex Media 2020, 32, 1–19. [Google Scholar] [CrossRef]
- Durur, H.; Yokus, A.; Abro, K.A. A non-linear analysis and fractionalized dynamics of Langmuir waves and ion sound as an application to acoustic waves. Int. J. Model. Simul. 2022, 21, 1–7. [Google Scholar] [CrossRef]
- Aguirre, J.; Viana, R.L.; Sanjuán, M.A.F. Fractal structures in nonlinear dynamics. Rev. Mod. Phys. 2009, 81, 333–386. [Google Scholar] [CrossRef]
- Viana, R.L.; Da Silva, E.C.; Kroetz, T.; Caldas, I.L.; Roberto, M.; Sanjuán, M.A.F. Fractal structures in nonlinear plasma physics. Phil. Trans. R. Soc. A 2011, 369, 371–395. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- El-Wakil, S.A.; Abulwafa, E.M.; El-Shewy, E.K.; Mahmoud, A.A.; Tawfik, A.M. Effect of space-time fractal order on the ion acoustic waves in electron-positron-ion plasma. Astrophys. Space Sci. 2014, 350, 591–598. [Google Scholar] [CrossRef]
- El-Wakil, S.A.; Abulwafa, E.M.; El-Shewy, E.K.; Mahmoud, A.A. Time-fractional Burgers equation for dust acoustic waves in a two different temperatures dusty plasma. Astrophys. Space Sci. 2013, 2, 383–393. [Google Scholar] [CrossRef]
- Khan, Y. Hausdorff fractal system arising in the field of plasma physics via variational technology. Chin. J. Phys. 2022, 77, 2288–2297. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; Hassan, S.Z.; Alomair, R.A.; Alsaleh, D.M. The new wave structures to the fractional ion sound and Langmuir waves equation in plasma physics. Fractal Fract 2022, 6, 227. [Google Scholar] [CrossRef]
- Abdelrahman, M.A.E.; AlKhidhr, H. A robust and accurate solver for some nonlinear partial differential equations and tow applications. Phys. Scr. 2020, 95, 065212. [Google Scholar] [CrossRef]
- Yang, X.F.; Deng, Z.C.; Wei, Y. A Riccati-Bernoulli sub-ODE method for nonlinear partial differential equations and its application. Adv. Diff. Equa. 2015, 1, 117–133. [Google Scholar] [CrossRef] [Green Version]
- LaBelle, J.; Cairns, I.H.; Kletzing, C.A. Electric field statistics and modulation characteristics of bursty Langmuir waves observed in the cusp. J. Geophys. Res. 2010, 115, 317. [Google Scholar] [CrossRef] [Green Version]
- Ergun, R.E.; Malaspina, D.M.; Cairns, I.H.; Goldman, M.V.; Newman, D.L.; Robinson, P.A.; Eriksson, S.; Bougeret, J.L.; Briand, C.; Bale, S.D.; et al. Eigenmode structure in solar-wind Langmuir waves. Phys. Rev. E 2008, 101, 051101. [Google Scholar] [CrossRef] [PubMed]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelwahed, H.G.; El-Shewy, E.K.; Alghanim, S.; Abdelrahman, M.A.E. On the Physical Fractional Modulations on Langmuir Plasma Structures. Fractal Fract. 2022, 6, 430. https://doi.org/10.3390/fractalfract6080430
Abdelwahed HG, El-Shewy EK, Alghanim S, Abdelrahman MAE. On the Physical Fractional Modulations on Langmuir Plasma Structures. Fractal and Fractional. 2022; 6(8):430. https://doi.org/10.3390/fractalfract6080430
Chicago/Turabian StyleAbdelwahed, H. G., E. K. El-Shewy, S. Alghanim, and Mahmoud A. E. Abdelrahman. 2022. "On the Physical Fractional Modulations on Langmuir Plasma Structures" Fractal and Fractional 6, no. 8: 430. https://doi.org/10.3390/fractalfract6080430
APA StyleAbdelwahed, H. G., El-Shewy, E. K., Alghanim, S., & Abdelrahman, M. A. E. (2022). On the Physical Fractional Modulations on Langmuir Plasma Structures. Fractal and Fractional, 6(8), 430. https://doi.org/10.3390/fractalfract6080430







