Barrier Option Pricing in the Sub-Mixed Fractional Brownian Motion with Jump Environment
Abstract
:1. Introduction
2. Preliminaries
- 1.
- is a central Gaussian process.
- 2.
- When ,
- 3.
- the covariance of and iswhere
- 4.
- , .
3. Asset Pricing Model
- There are two kinds of assets in the financial market: risk-free assets (bonds) and risky assets (stocks).
- The stock price is driven by the sub-mixed fBm with jump:where is the instantaneous expected return rate of the stock; q is the stock dividend rate; and represent the volatility of stock price; is a compensated Poisson process with intensity . , and are independent of each other.
- The return of risk-free assets in time period t iswhere constant r is the risk-free interest rate.
- All assets can be traded freely and continuously without transaction costs and taxes.
- There is no arbitrage opportunity in the market.
- Short selling is not limited.
- The option can be exercised only at the maturity time.
4. Pricing Formula for Barrier Options
5. Numerical Experiment
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dassios, A.; Lim, J.W. Recursive formula for the double-barrier Parisian stopping time. J. Appl. Probab. 2018, 55, 282–301. [Google Scholar] [CrossRef]
- Funahashi, H.; Higuchi, T. An analytical approximation for single barrier options under stochastic volatility models. Ann. Oper. Res. 2018, 266, 129–157. [Google Scholar] [CrossRef]
- Guillaume, T. Closed form valuation of barrier options with stochastic barriers. Ann. Oper. Res. 2021, 1–30. [Google Scholar] [CrossRef]
- Gao, Y.; Jia, L. Pricing formulas of barrier-lookback option in uncertain financial markets. Chaos Solitons Fractals 2021, 147, 110986. [Google Scholar] [CrossRef]
- Shreve, S.E. Stochastic Calculus for Finance II: Continuous-Time Models; Springer: New York, NY, USA, 2004. [Google Scholar]
- Merton, R.C. Theory of rational option pricing. Bell Econ. Manag. Sci. 1973, 4, 141–183. [Google Scholar] [CrossRef] [Green Version]
- Rubinstein, M. Breaking down the barriers. Risk 1991, 4, 28–35. [Google Scholar]
- Black, F.; Scholes, M. The Pricing of Options and Corporate Liabilities. J. Political Econ. 1973, 81, 637–654. [Google Scholar] [CrossRef] [Green Version]
- Ding, Z.; Granger, C.W.; Engle, R.F. A long memory property of stock market returns and a new model. J. Empir. Financ. 1993, 1, 83–106. [Google Scholar] [CrossRef]
- Shiryaev, A.N. Essentials of Stochastic Finance: Facts, Models, Theory; World Scientific: Singapore, 1999. [Google Scholar]
- Necula, C. Option pricing in a fractional Brownian motion environment. Adv. Econ. Financ. Res.-Dofin Work. Pap. Ser. 2008, 2, 259–273. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. Wienersche spiralen und einige andere interessante kurven in hilbertscen raum, cr (doklady). Acad. Sci. URSS (NS) 1940, 26, 115–118. [Google Scholar]
- Chen, Q.; Zhang, Q.; Liu, C. The pricing and numerical analysis of lookback options for mixed fractional Brownian motion. Chaos Solitons Fractals 2019, 128, 123–128. [Google Scholar] [CrossRef]
- Bian, L.; Li, Z. Fuzzy simulation of European option pricing using sub-fractional Brownian motion. Chaos Solitons Fractals 2021, 153, 111442. [Google Scholar] [CrossRef]
- Wang, J.; Yan, Y.; Chen, W.; Shao, W.; Tang, W. Equity-linked securities option pricing by fractional Brownian motion. Chaos Solitons Fractals 2021, 144, 110716. [Google Scholar] [CrossRef]
- Cheridito, P. Arbitrage in fractional Brownian motion models. Financ. Stochastics 2003, 7, 533–553. [Google Scholar] [CrossRef]
- Bender, C.; Elliott, R.J. Arbitrage in a discrete version of the Wick-fractional Black-Scholes market. Math. Oper. Res. 2004, 29, 935–945. [Google Scholar] [CrossRef]
- Björk, T.; Hult, H. A note on Wick products and the fractional Black-Scholes model. Financ. Stochastics 2005, 9, 197–209. [Google Scholar] [CrossRef]
- Bojdecki, T.; Gorostiza, L.G.; Talarczyk, A. Sub-fractional Brownian motion and its relation to occupation times. Stat. Probab. Lett. 2004, 69, 405–419. [Google Scholar] [CrossRef]
- Charles, E.N.; Mounir, Z. On the sub-mixed fractional Brownian motion. Appl.-Math.-J. Chin. Univ. 2015, 30, 27–43. [Google Scholar] [CrossRef] [Green Version]
- Tudor, C. Some properties of the sub-fractional Brownian motion. Stochastics Int. J. Probab. Stoch. Process. 2007, 79, 431–448. [Google Scholar] [CrossRef]
- Xu, F.; Zhou, S. Pricing of perpetual American put option with sub-mixed fractional Brownian motion. Fract. Calc. Appl. Anal. 2019, 22, 1145–1154. [Google Scholar] [CrossRef]
- Merton, R.C. Option pricing when underlying stock returns are discontinuous. J. Financ. Econ. 1976, 3, 125–144. [Google Scholar] [CrossRef] [Green Version]
- Zhou, Q.; Yang, J.J.; Wu, W.X. Pricing vulnerable options with correlated credit risk under jump-diffusion processes when corporate liabilities are random. Acta Math. Appl. Sin. Engl. Ser. 2019, 35, 305–318. [Google Scholar] [CrossRef]
- Sun, W.; Zhao, Y.; MacLean, L. Real Options in a Duopoly with Jump Diffusion Prices. Asia-Pac. J. Oper. Res. 2021, 38, 2150009. [Google Scholar] [CrossRef]
- Zhang, W.G.; Li, Z.; Liu, Y.J.; Zhang, Y. Pricing European option under fuzzy mixed fractional Brownian motion model with jumps. Comput. Econ. 2021, 58, 483–515. [Google Scholar] [CrossRef]
- Liu, M. Two possible types of superfluidity in crystals. Phys. Rev. B 1978, 18, 1165. [Google Scholar] [CrossRef]
- Callen, H.B. Thermodynamics and an Introduction to Thermostatistics; John Wiley & Sons: New York, NY, USA, 1985. [Google Scholar]
- Appel, D.; Grabinski, M. The origin of financial crisis: A wrong definition of value. Port. J. Quant. Methods 2011, 2, 33. [Google Scholar]
- Klinkova, G.; Grabinski, M. Conservation laws derived from systemic approach and symmetry. Int. J. Latest Trends Fin. Ecol. Sci. Vol. 2017, 7, 1307. [Google Scholar]
- Tankov, P. Financial Modelling with Jump Processes; Chapman and Hall/CRC: London, UK, 2003. [Google Scholar]
- Grabinski, M.; Klinkova, G. Wrong use of averages implies wrong results from many heuristic models. Appl. Math. 2019, 10, 605. [Google Scholar] [CrossRef] [Green Version]
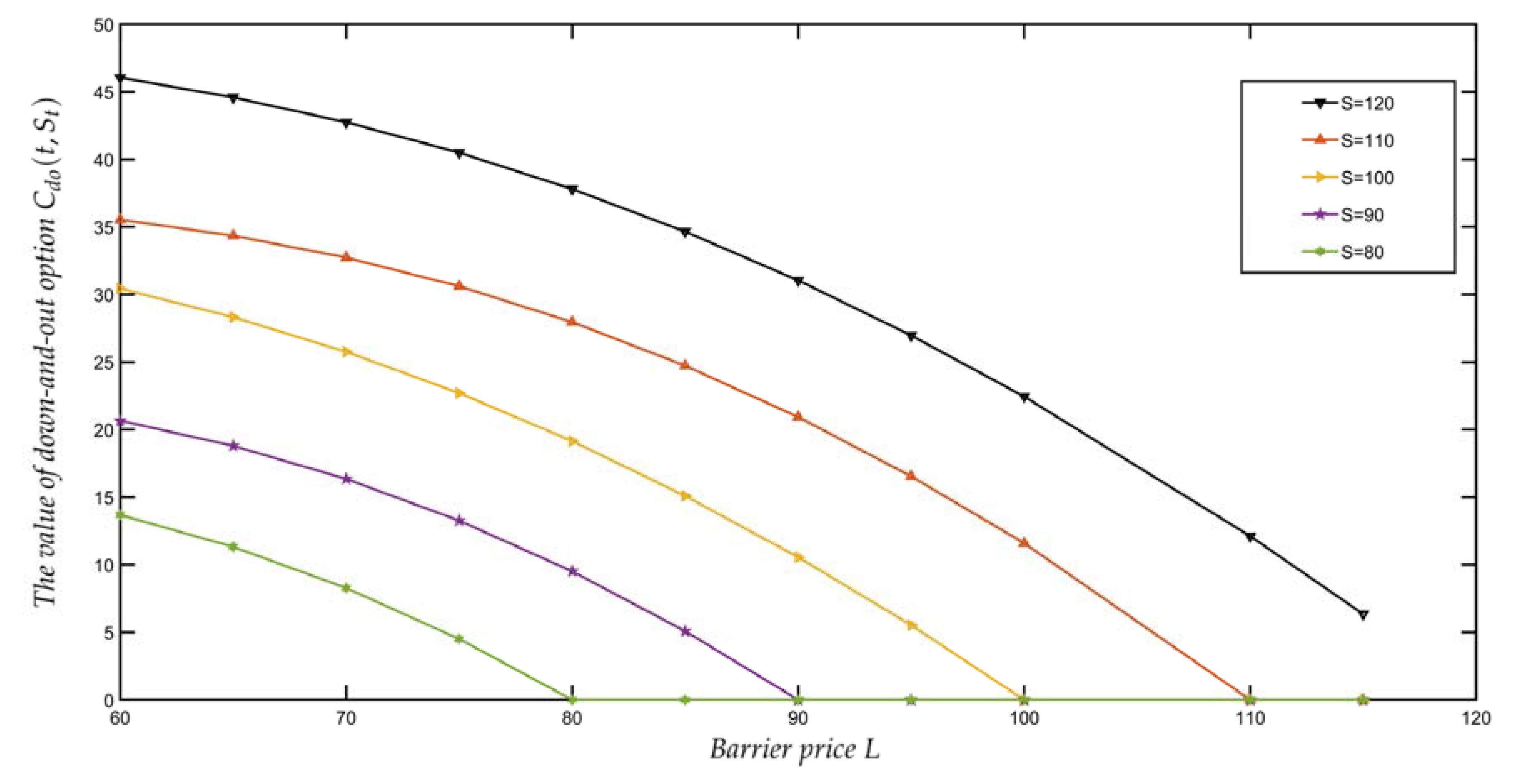

| L | |||||
|---|---|---|---|---|---|
| 115 | 6.350 | – | – | – | – |
| 110 | 12.112 | 0.000 | – | – | – |
| 100 | 22.445 | 11.591 | 0.000 | – | – |
| 95 | 26.969 | 16.541 | 5.534 | – | – |
| 90 | 31.042 | 20.926 | 10.551 | 0.000 | – |
| 85 | 34.654 | 24.731 | 15.095 | 5.086 | – |
| 80 | 37.803 | 27.956 | 19.149 | 9.510 | 0.000 |
| 75 | 40.495 | 30.613 | 22.702 | 13.264 | 4.507 |
| 70 | 42.748 | 32.730 | 25.757 | 16.351 | 8.275 |
| 65 | 44.587 | 34.352 | 28.324 | 18.798 | 11.321 |
| 60 | 46.049 | 35.533 | 30.430 | 20.653 | 13.680 |
| 120 | 31.119 | 36.621 | 40.017 | 29.761 | 35.812 | 39.501 | 28.472 | 35.045 | 39.018 |
| 115 | 27.257 | 32.652 | 35.839 | 25.860 | 31.879 | 35.360 | 24.500 | 31.141 | 34.910 |
| 110 | 23.554 | 28.760 | 31.708 | 22.144 | 28.033 | 31.270 | 20.737 | 27.334 | 30.857 |
| 105 | 20.024 | 24.949 | 27.626 | 18.632 | 24.278 | 27.232 | 17.212 | 23.628 | 26.859 |
| 100 | 16.679 | 21.217 | 23.590 | 15.344 | 20.613 | 23.244 | 13.953 | 20.025 | 22.916 |
| 95 | 13.524 | 17.563 | 19.600 | 12.291 | 17.038 | 19.305 | 10.982 | 16.522 | 19.026 |
| 90 | 10.559 | 13.982 | 15.650 | 9.479 | 13.545 | 15.411 | 8.311 | 13.115 | 15.183 |
| 85 | 7.770 | 10.462 | 11.734 | 6.895 | 10.125 | 11.553 | 5.935 | 9.791 | 11.380 |
| 80 | 5.129 | 6.988 | 7.842 | 4.510 | 6.759 | 7.722 | 3.821 | 6.531 | 7.606 |
| 75 | 2.582 | 3.534 | 3.959 | 2.259 | 3.418 | 3.900 | 1.896 | 3.302 | 3.843 |
(0.1, 0.15, 0.2) | (0.2, 0.25, 0.3) | (0.3, 0.35, 0.4) | (0.4, 0.45, 0.5) | |
|---|---|---|---|---|
| 120 | 25.323 | 28.441 | 32.360 | 36.149 |
| 115 | 20.918 | 24.467 | 28.506 | 32.202 |
| 110 | 16.770 | 20.702 | 24.790 | 28.338 |
| 105 | 12.963 | 17.176 | 21.222 | 24.559 |
| 100 | 9.581 | 13.917 | 17.807 | 20.867 |
| 95 | 6.704 | 10.948 | 14.549 | 17.259 |
| 90 | 4.385 | 8.281 | 11.443 | 13.729 |
| 85 | 2.637 | 5.910 | 8.477 | 10.267 |
| 80 | 1.416 | 3.803 | 5.623 | 6.856 |
| 75 | 0.608 | 1.886 | 2.838 | 3.467 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, B.; Tao, X.; Ji, Y. Barrier Option Pricing in the Sub-Mixed Fractional Brownian Motion with Jump Environment. Fractal Fract. 2022, 6, 244. https://doi.org/10.3390/fractalfract6050244
Ji B, Tao X, Ji Y. Barrier Option Pricing in the Sub-Mixed Fractional Brownian Motion with Jump Environment. Fractal and Fractional. 2022; 6(5):244. https://doi.org/10.3390/fractalfract6050244
Chicago/Turabian StyleJi, Binxin, Xiangxing Tao, and Yanting Ji. 2022. "Barrier Option Pricing in the Sub-Mixed Fractional Brownian Motion with Jump Environment" Fractal and Fractional 6, no. 5: 244. https://doi.org/10.3390/fractalfract6050244
APA StyleJi, B., Tao, X., & Ji, Y. (2022). Barrier Option Pricing in the Sub-Mixed Fractional Brownian Motion with Jump Environment. Fractal and Fractional, 6(5), 244. https://doi.org/10.3390/fractalfract6050244






