Numerical Investigations of the Fractional-Order Mathematical Model Underlying Immune-Chemotherapeutic Treatment for Breast Cancer Using the Neural Networks

Abstract

The aim of this work is to design a stochastic framework to solve the fractional-order differential model based on the breast cancer progression during the immune-chemotherapeutic treatment phase, including certain control parameters such as anti-cancer medications, ketogenic diet and immune boosters. The developed model considers tumor density progression throughout chemotherapy treatment, as well as an immune response during normal cell–tumor cell interaction. This study’s subject seems to be to demonstrate the implications and significance of the fractional-order breast cancer mathematical model. The goal of these studies is to improve accuracy in the breast cancer model by employing fractional derivatives. This study also includes an integer, nonlinear mathematical system with immune-chemotherapeutic treatment impacts. The mathematical system divides the fractional-order breast cancer mathematical model among four manifestations: normal cell population (N), tumor cells (T), immune response class (I), and estrogen compartment (E), i.e., (NTIE). The fractional-order NTIE mathematical system is still not published previously, nor has it ever been addressed employing the stochastic solvers’ strength. To solve a fractional-order NTIE mathematical system, stochastic solvers based on the Levenberg–Marquardt backpropagation scheme (LMBS) and neural networks (NNs), namely, LMBNNs, are been constructed. To solve the fractional-order NTIE mathematical model, three cases with varying values for this same fractional order have been supplied. The statistics used to offer the numerical solutions of the fractional-order NTIE mathematical model are divided as follows: 75% in training, 15% in testing, and 10% in the authorization. The acquired numerical findings were compared using the reference solutions to determine the accuracy of the LMBNNs using Adams–Bashforth–Moulton. The numerical performances employing error histograms (EHs), state transitions (STs), regression, correlation, including mean square error (MSE) have been further supplied to authenticate overall capability, competence, validity, consistency, as well as exactness of such LMBNNs.

1. Introduction

Cells are the fundamental units of a biological organism. When the body demands new cells, cells split and grow to make them. Cells generally expire once they grow extremely aged and hence faulty. Eventually, fresh cells replace them. Cancer develops when genetic mutations disrupt this regular mechanism. Cells begin to expand uncontrollably. These cells can combine to create a growth known as a tumor. A tumor might be cancerous or noncancerous. A malignant tumor can develop and transmit to neighboring regions of the body. A benign tumor can develop but does not transmit.

Cancer harms greater people than TB, AIDS, and malaria combined estimates to the National Cancer Registry. According to statistics, cancer fatalities are expected to reach 13 million by 2030 [1]. As a malignant tumor develops, cancer cells may be transported to neighboring regions of the body via the circulation or lymphatic system. Cancer cells multiply and may evolve into new tumors throughout this phase. That gets frequently characterized as termed metastasis. Lymph nodes are often frequently the first areas of cancer dissemination. Lymph nodes are little coffee bean entities that assist the immune system fight diseases. They develop in clumps over the body, including the neck, groyne, and below the arms. Cancer can spread to other parts of the body via circulation. These organs include the bones, liver, lungs, and brain. Even when the disease spreads, it is still named after the region where it initially emerged. Breast cancer that has progressed to the lungs is referred to as metastatic breast cancer rather than lung cancer.

Despite the fact that breast cancer remains the leading common well-being and obstruction illness among ladies worldwide. Breast cancer seems to be a disease which infects all cells of a breast but rather produces rapid unregulated boundary of malignant cells in the breast tissues [2]. A few of the dangerous characteristics for breast cancer include hormone imbalance, heredity, and environmental factors; nevertheless, there is currently limited evidence on this illness in terms of the major origin of a malignant type [3,4]. Several mathematical as well as analytical techniques, primarily based on integer-order differential equations (IDEs), have now been constructed to investigate the relationship among tumor cells as well as the immune mechanism across therapy phase [5].

Furthermore, it has been demonstrated that fractional-order differential equations (FDEs) may effectively solve many issues in biology as well as various domains including engineering, finance, economics, even neural networks; consider, for example, Refs. [6,7,8,9,10]. The nonlocal feature of FDE models is dependent not only on the present phase but also gives an appropriate description for past ones. Because of the peculiarity of the sequence of differentiation, where even a minor change can result in a significant variation in the outcome, the transition of IDES-regulated models into FDE-regulated models must be precise. The capabilities, characteristic and legacy of the fractional calculus concepts, underlying mathematical foundations and modeling ease are illustrated by computing the solutions dynamics of fractional-order mathematical model underlying immune-chemotherapeutic treatment for breast cancer with the help of both the deterministic as well as stochastic numerical solvers for better insights of super-slow evolution and super-fast transients, which cannot be observed from modeling with traditional integer order calculus [11,12,13,14,15,16,17,18]. Certain events which IDEs unable represent can be modelled by FDEs. As a result, FDEs usually use biological models, despite the fact that these seem important for memorization and inherited traits [19,20,21,22,23,24,25].

A fractional-order breast cancer mathematical model has been partitioned into four phenomena throughout this analysis: normal cell population (N), tumor cells (T), immune reaction class (I), and estrogen compartment (I), and therefore is termed as the NTIE mathematical system. The stochastic numerical performances of the Levenberg–Marquardt backpropagation scheme (LMBS) together with neural networks (NNs), i.e., LMBS-NNs, are addressed.

The remaining portions of the paper are given in regards: We devise a framework of FDEs in the form of NTIE mathematical model is presented in Section 2. The innovative topographies including an overview of stochastic solvers along with important innovative aspects of the LMBNNs for such mathematical fractional-order system employing a breast cancer model are provided in Section 3. The LMBNNs structure is explained in Section 4. The results and simulations obtained employing the planned method for the fractional-order NTIE model are provided in Section 5. Section 6 illustrates the conclusion.

2. Mathematical Model

Over the years, modelling breast cancer is still a significant resource for investigating tumor growth’s dynamic characteristics during the therapy phase. D’Onofrio et al. investigated about use of mathematical visualisation for tumor symptom therapy [26]. Kermack and McKendrick’s [27,28] research, as well as several other studies in [29,30,31,32,33,34,35], have demonstrated that mathematically modeling biological events is a helpful tool for solving epidemic issues. Nevertheless, in these investigations, a nutritious diet (ketogenic diet) was not included in the mathematical model. Oke et al. enhanced Mudufza’s [33] model in [1]. They included control conditions like as a ketogenic meal, immune enhancer, with anti-cancer medication to emphasise the idea that the cells interact as a result of a variation throughout the tumor cell’s DNA. Throughout this research, we develop such FDE system with the following manner: Refs [33,34,35].

$$\left\{\begin{array}{c}{D}^{\alpha }N\left(\tau \right)=N\left(\tau \right)p_1(k_1-{\zeta }_{1}N\left(\tau \right)-{\gamma }_{1}T\left(\tau \right))-(1-\kappa ){\psi }_{1}N\left(\tau \right)E\left(\tau \right),\hfill \\ D^{\alpha }T\left(\tau \right)=T\left(\tau \right)p_2(k_{2}d-{\zeta }_{2}T\left(\tau \right)-{\gamma }_{2}I\left(\tau \right))-\delta T\left(\tau \right)+(1-\kappa ){\psi }_{1}N\left(\tau \right)T\left(\tau \right)E\left(\tau \right),\hfill \\ D^{\alpha }I\left(\tau \right)=\eta \lambda +I\left(\tau \right)p_1(k_3-{\zeta }_3-{\gamma }_{3}T\left(\tau \right))-(1-\kappa ){\psi }_{2}I\left(\tau \right)E\left(\tau \right),\hfill \\ D^{\alpha }E\left(\tau \right)=-{\gamma }_{4}E\left(\tau \right)+(1-\kappa )\nu ,\hfill \end{array}\right.$$

(1)

in which the parameters are positive real values.

The very first equation through system (1) represents a normal cell population $N\left(\tau \right)$. $p_1$ seems to be the rate of population growth, and $k_1$ is the population carrying capacity. ${\zeta }_1$ is logistic rate, as well as ${\gamma }_1$ estimates overall rate of inhibition of $N\left(\tau \right)$. ${\psi }_1$ reflects tumour development rate caused by DNA mutation triggered with the advent of high estrogen, whereas $(1˘\kappa )$ reflects the efficiency of anti-cancer medications.

The luminal type (containing oestrogen receptors, ESR1 + ve) tumour cells are designated using $T\left(\tau \right)$ in the second equation of (1). $k_2$ would be the population’s carrying capacity, and it is affected by that ketogenic diet seems to have a rate of d. The population rate of growth of $T\left(\tau \right)$ is given by $p_2$. Any DNA mutation generated by high oestrogen repopulates tumour cells by ${\psi }_{1}N\left(\tau \right)E\left(\tau \right)$. Rate of logistic of the tumor cell population seems to be represented by ${\zeta }_2$. ${\gamma }_2$ denotes the rate at which human immune system is effective versus tumour cells and is the consequence of the tumor’s food starvation throughout the ketogenic dietary.

The class of immune reaction is shown as $I\left(\tau \right)$ in the third equation in (1), where $\eta$ signifies the source rate of immune reaction completely absorbed in the body daily. When tumor cells overrun immune cells, the immune booster $\lambda$ supports the immune response in activating the immune reaction and fighting the tumor cells. Rate of logistic of the immune cell population is represented by ${\zeta }_3$, whereas the immune suppression rate seems to be represented by ${\psi }_2$. $k_3$ represents the carrying capacity, while ${\gamma }_3$ represents the association rate across $T\left(\tau \right)$ and $I\left(\tau \right)$.

Eventually, the estrogen compartment is indicated by $E\left(\tau \right)$ in the final equation of system (1). It should be noted that a boost in estrogen levels might cause tumor cells to proliferate. $\nu$ represents the process of continually replenishing excessive estrogen. We assume that the majority of cancer cells are estrogen-receptor positive, but only a small percentage of epithelial cells are, which can only be avoided by the anti-cancer drug $(1˘\kappa )$ tamoxifen. cite16,1 ${\gamma }_4$ denotes the rate at which oestrogen is drained out of the body. Based on the parameter characterization,

Table 1. The declaration of parameters in terms of unit day ⁻¹.

Parameter	Symbol	Value
NC’s logistic rate	${\zeta }_1$	[0.05–0.2]
TC’s logistic rate	${\zeta }_2$	[0.5–0.95]
IC’s logistic rate	${\zeta }_2$	[0.05–0.2]
NC’s rate of growth	$p_1$	0.3
The rate of TC growth	$p_2$	0.4
The ketogenic diet’s constant rate	d	0.5
The rate of NC inhibition	${\gamma }_1$	$6\times {10}^{-8}$
TC mortality rate as a result of immune reaction	${\gamma }_2$	$3\times {10}^{-6}$
Rate of interaction coefficient with immune reaction	${\gamma }_3$	1 × 10${}^{-7}$
Estrogen’s natural death rate	${\gamma }_4$	0.97
Estrogen source rate	$\nu$	[0.6–3]
Immune boosting supplement	$\lambda$	0.01
The ketogenic diet’s effect on TC mortality	$\delta$	2
The rate of tumor formation as
a response of estrogen-induced DNA damage	${\psi }_1$	0.2
Excess oestrogen causes immune suppression	${\psi }_2$	0.002
The anti-cancer drug’s efficacy	$\kappa$	0–1
IC source rate	$\eta$	$1.3\times {10}^2$
NC’s carrying capacity	$k_1$	1.232
TC’s carrying capacity	$k_2$	1.75
IC’s carrying capacity	$k_3$	[0.11, 1.17]

includes all parametric quantities as reported in [33,34,35].

Definition 1

([36]). Abdel’s formula gives the fractional integral with order $\alpha >0$ for a function $\Phi \left(\xi \right)$.

$$I_{\alpha }\Phi \left(\xi \right)=\frac{1}{\Gamma \left(\alpha \right)}{\int }_0^{\xi }\Phi \left(\tau \right){(\xi -\tau )}^{\alpha -1}g\left(\tau \right)d\tau ,\xi >0.$$

(2)

Definition 2

([36]). Assume that $\Phi :{\mathbb{R}}^{+}\to \mathbb{R}$ is a continuous function. The Caputo fractional derivative of order $\alpha \in (n˘1,n)$ is described as below, while n is a positive integer.

$$D_0^{\alpha }\Phi \left(\xi \right)=\frac{1}{\Gamma (n-\alpha )}{\int }_0^{\xi }\frac{{\Phi }^{\left(n\right)}\left(\tau \right)}{{(\xi -\tau )}^{\alpha -n+1}}d\tau .$$

(3)

Whenever $\alpha =n$, the derivatives become the standard $n^{th}$ order derivatives.

Definition 3

([36]). One variable’s Mittag-Leffler function is

$$E_{\alpha }(\mu ,z)=E_{\alpha }\left(\mu z^{\alpha }\right)=\sum _{K=0}^{\infty }\frac{{\mu }^K{z}^{\alpha K}}{\Gamma (\alpha K+1)},(\mu \ne 0,z\in \mathbb{C},\mathbb{R}(\alpha )>0).$$

(4)

3. Innovative Topographies Including an Overview of Stochastic Solvers

To solve either the fractional order derivatives of the breast cancer mathematical model based on the NTIE effects, numerical stochastic operators via LMBS-NNs are presented. The performance of local but also global operators using stochastic computer solvers is being used to solve a wide variety of nonlinear, complex, stiff, and singular systems [37]. The nonlinear third sort of singular model [38], fractional order singular models [39], functional order system [40], delayed differential model [41], as well as periodic differential system [42] represent just handful well implementations of such solvers. The goal of this work is to use the stochastic methods of the LMBSNs to generate numerical representations of the fractional-order derivatives of a breast cancer mathematical model founded upon NTIE phenomena. This is discovered how time-fractional order derivatives may be used to specify system conditions in such a variety of ways. The memory function represents the derivative of fractional order, whereas the derivative order structure conveys remembrance. Real and genuine implementations are shown by more such fractional derivatives [43,44,45].

The following seem to be important innovative aspects of the LMBNNs for such mathematical fractional-order system employing a breast cancer model:

• Relying upon that NTIE impacts, a novel design of the fractional-order derivatives of the breast cancer mathematical model is presented.
• Stochastic solvers based measurements/assessments have never been used to solve the fractional order derivatives of a breast cancer mathematical model relying on NTIE impacts
• The numerical studies employing stochastic paradigms are shown effectiveness of using the fractional-order derivatives terms in the breast cancer mathematical model considering on the NTIE effects.
• Artificial intelligence (AI) knacks based LMBNNs is introduced to solve the nonlinear fractional order derivatives of the breast cancer mathematical model relying upon that NTIE impacts.
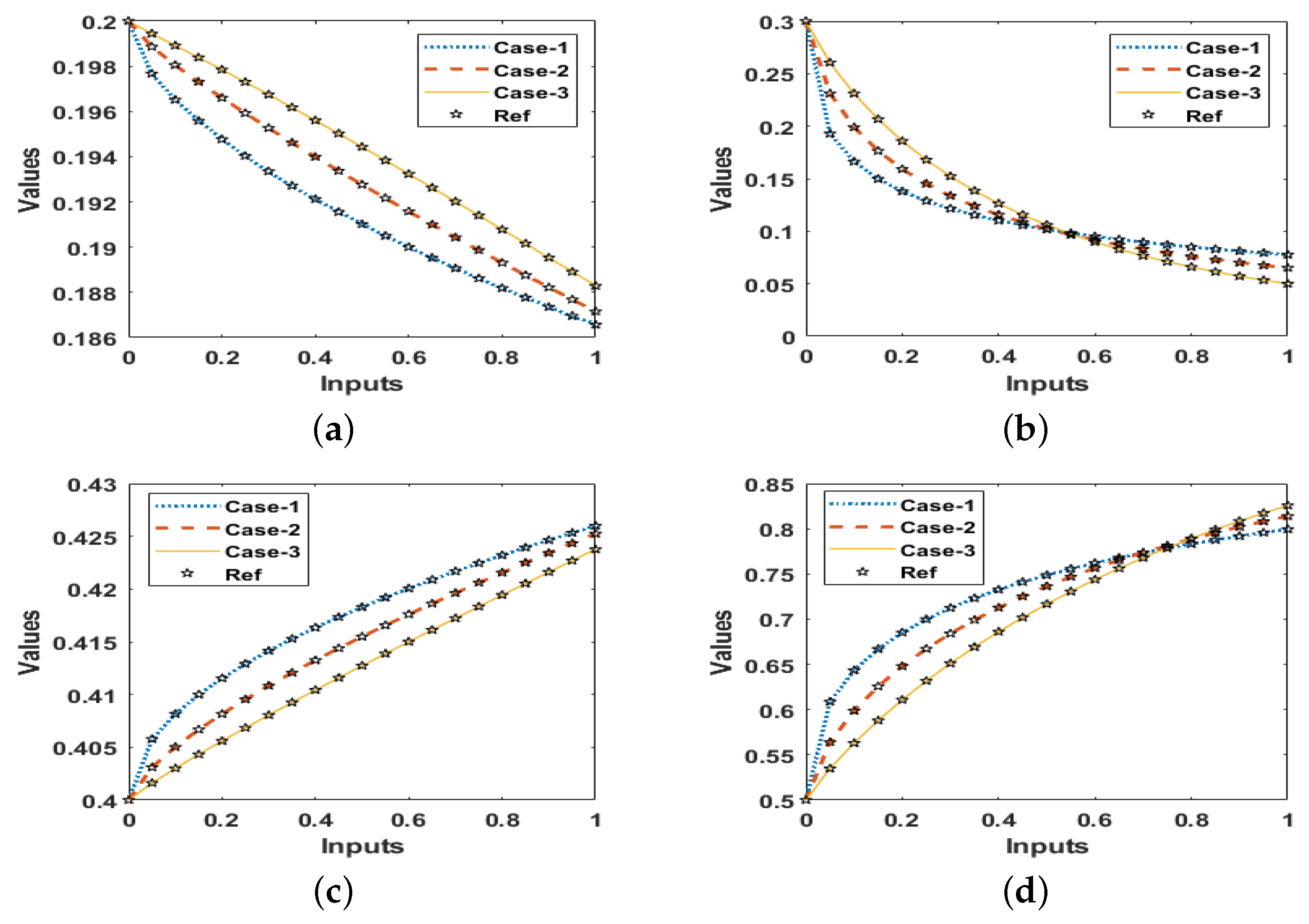
• Three appropriate fractional-order variants depending upon that breast cancer mathematical model have been numerically solved to validate the reliability of proposed LMBNNs.
• The correctness/brilliance of stochastic computing solver-based of LMBNNs is demonstrated by comparing the outcomes of produced and reference solutions Adams–Bashforth–Moulton method in good agreement.
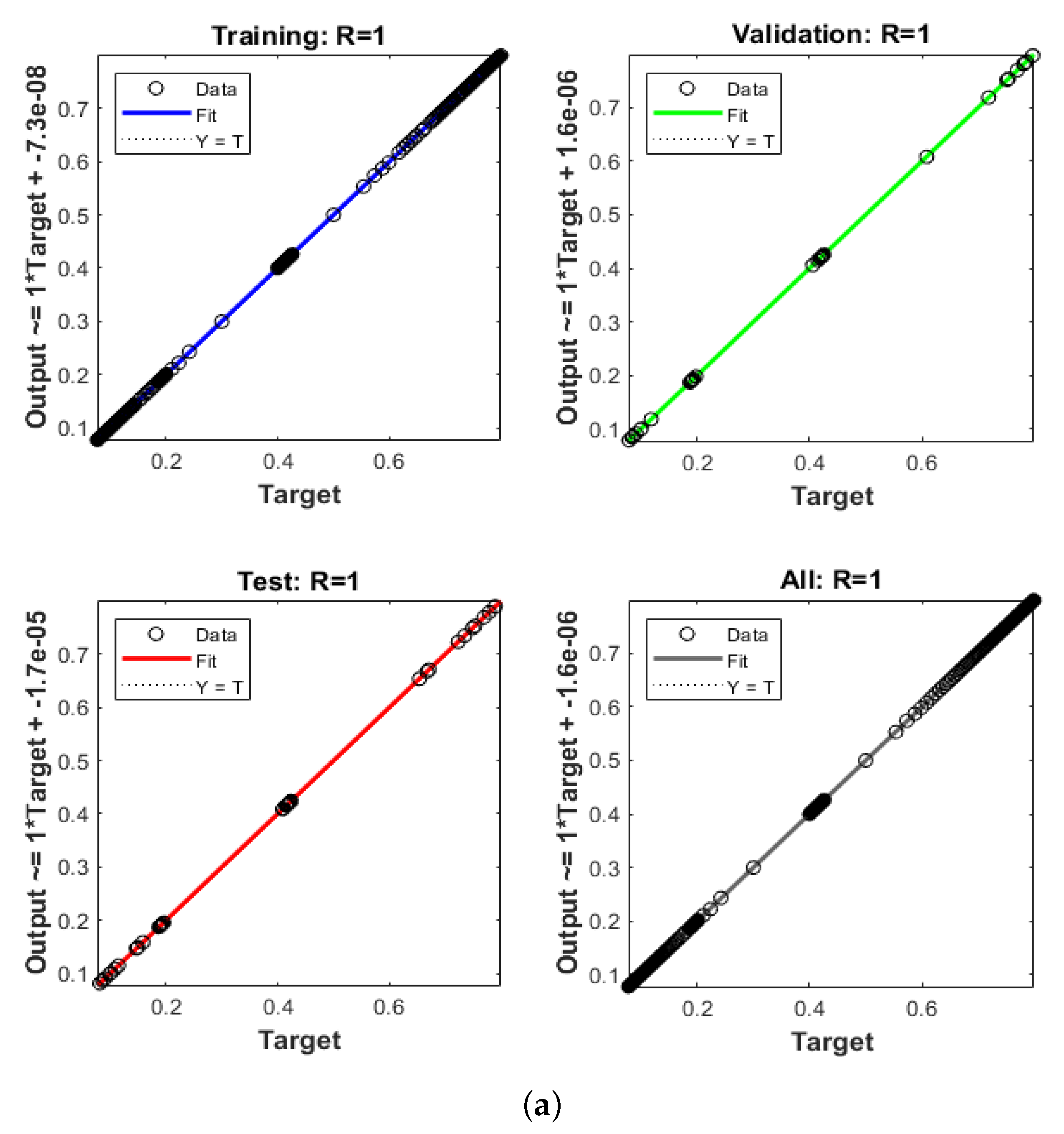
• The regression, STs, MSE, EHs, and correlation performances validate the developed LMBNNs’ dependability and consistency in solving the fractional order breast cancer mathematical model.

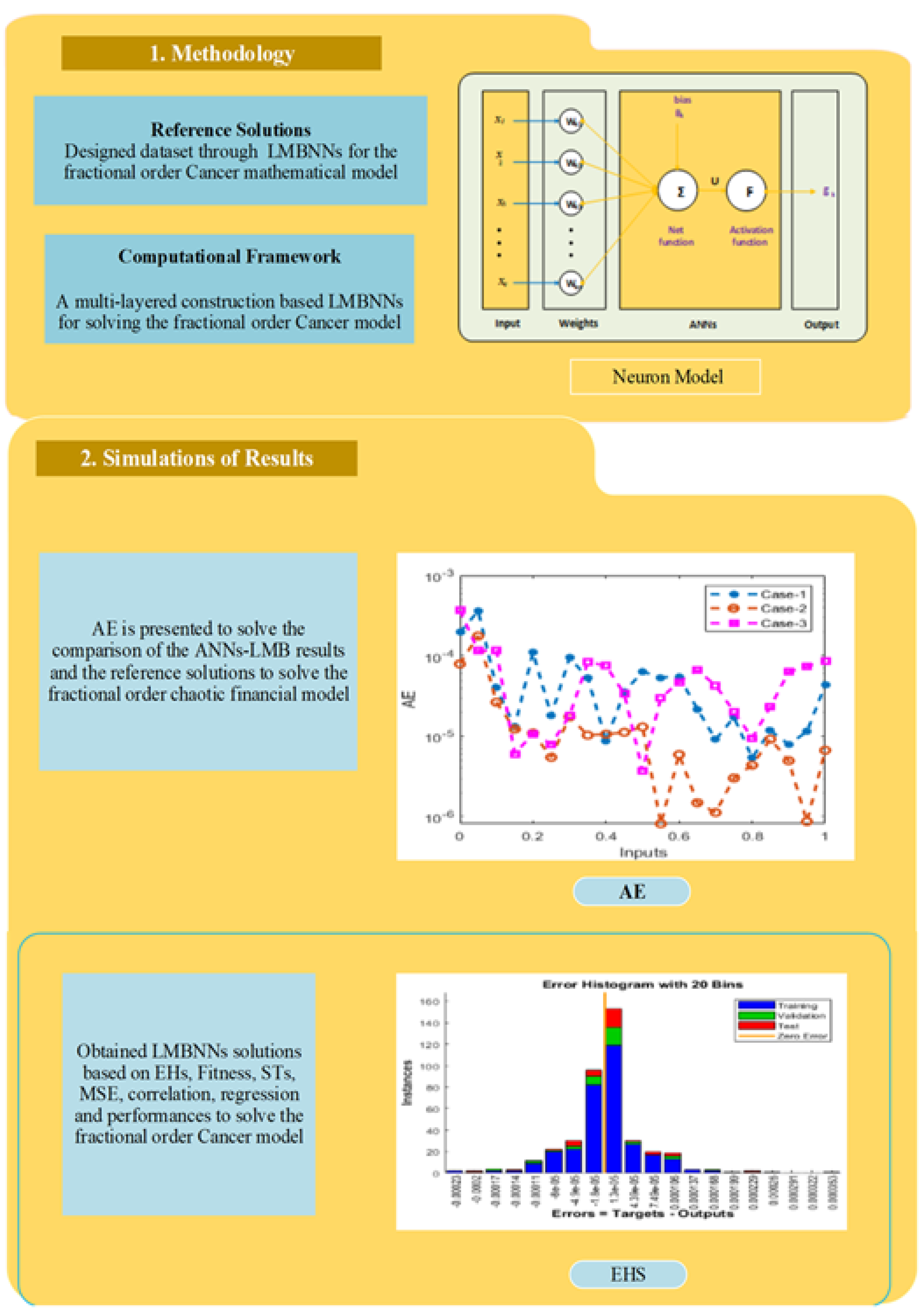
4. Suggested Methodology: LMBNNs

This section explains the suggested LMBNNs structure for solving the fractional order immune-chemotherapeutic treatment treating breast cancer-linked mathematical model. The approach is divided into two sections. First, the fundamental LMBNNs operator performances are presented. Meanwhile, the LMBNNs execution approach is used to solve the fractional-order immune-chemotherapeutic treatment treating breast cancer mathematical model.

Figure 1 depicts the multi-layer optimization procedure employing numerical stochastic LMBNNs, whereas Figure 2 depicts the single-layer neuron layout. The LMBNNs processes are supplied in Matlab using the ’nftool’ command, having data selected like 75% as training, 15% as testing, then 10% for authorization.

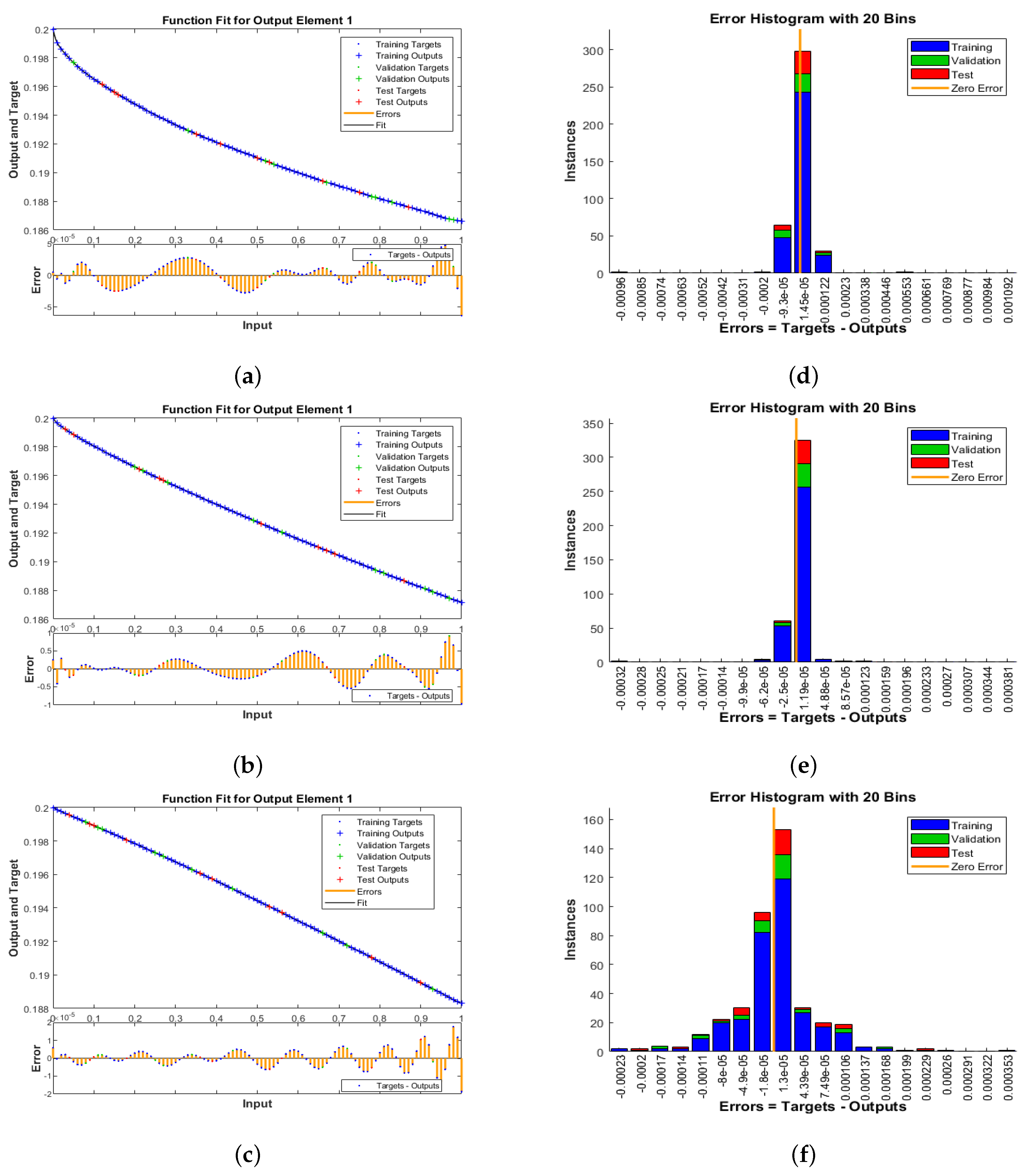
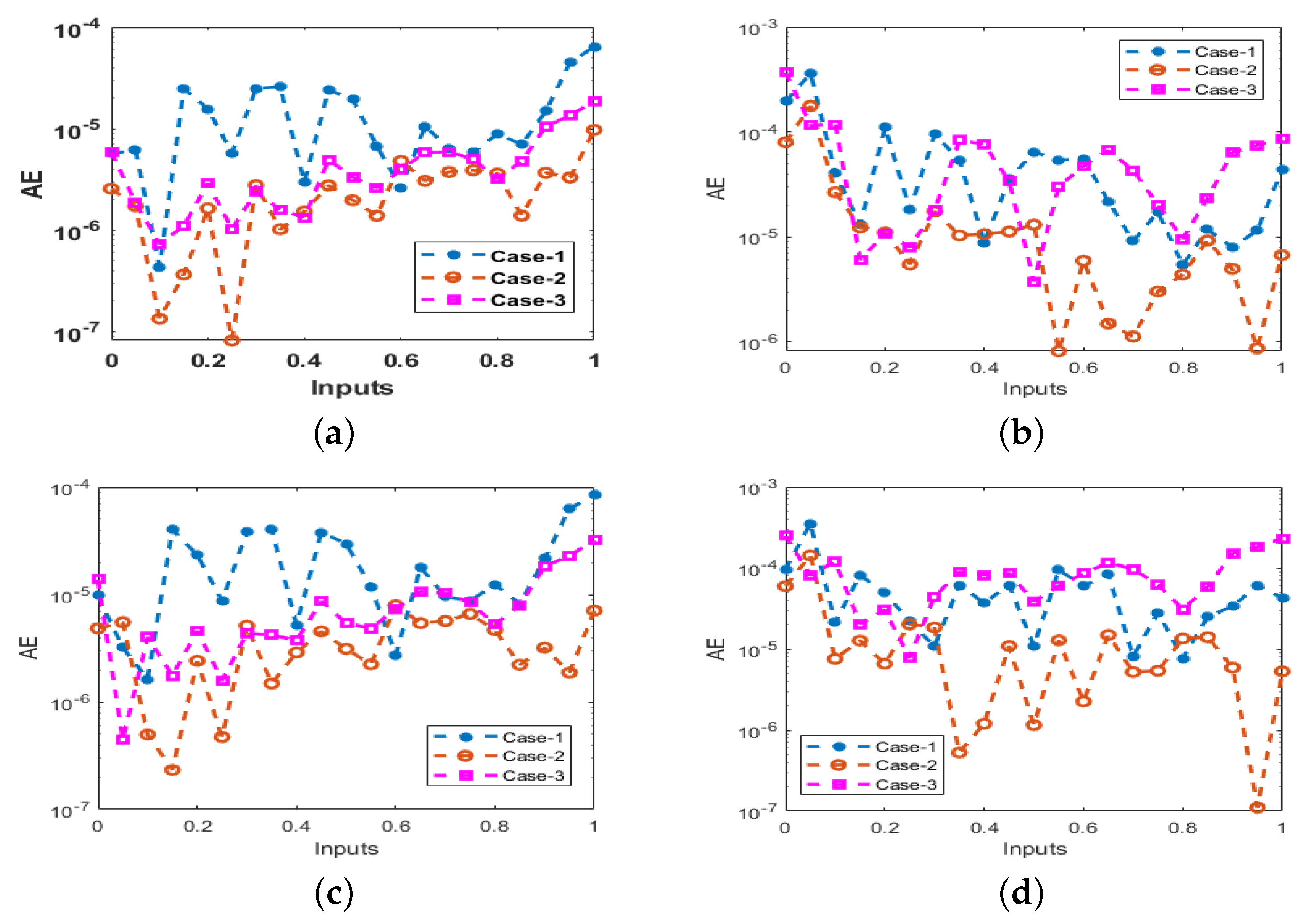
5. Results Obtained Employing the Planned Method

Numerical performances with three possible fractional-order modifications to address the nonlinear immune-chemotherapeutic treatment of breast cancer mathematical system through using suggested LMBNNs are shown in this phase. In the accompanying cases, the mathematical description of each variant is given as follows:

• Case 1:

Adopt the following fractional-order immune-chemotherapeutic treatment of breast cancer-related model with the designated values $\alpha =0.5, {\zeta }_1=0.3, {\zeta }_2=0.7, {\zeta }_3=0.1,$ $p_1=0.3, p_2=0.4, d=0.5, {\gamma }_1=6\times {10}^{-8}, {\gamma }_2=3\times {10}^{-6}, {\gamma }_3=1\times {10}^{-7}, {\gamma }_4=0.97,$ $\nu =2, \lambda =0.01, \delta =2, {\psi }_1=0.2, {\psi }_2=0.02, \kappa =0.5, \eta =0.1, k_1=0.1, k_2=0.2, k_3=0.3$:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\begin{array}{cc}{D}^{0.5}N\left(\tau \right)=0.3N\left(\tau \right)(0.1-0.3N\left(\tau \right)-(6\times {10}^{-8})T\left(\tau \right))-0.1N\left(\tau \right)E\left(\tau \right),\hfill & N_0=0.2,\\ D^{0.5}T\left(\tau \right)=0.4T\left(\tau \right)(0.1-0.7T\left(\tau \right)-(3\times {10}^{-6})I\left(\tau \right))-2T\left(\tau \right)+0.1N\left(\tau \right)T\left(\tau \right)E\left(\tau \right),\hfill & T_0=0.2,\\ D^{0.5}I\left(\tau \right)=0.001+0.3I\left(\tau \right)(0.2-(1\times {10}^{-7})T\left(\tau \right))-0.01I\left(\tau \right)E\left(\tau \right),\hfill & I_0=0.2,\\ D^{0.5}E\left(\tau \right)=-0.97E\left(\tau \right)+1,\hfill & E_0=0.2.\end{array}\hfill \end{array}\right.\end{array}$$

(5)

• Case 2:

Adopt the following fractional-order immune-chemotherapeutic treatment of breast cancer-related model with the designated values $\alpha =0.7, {\zeta }_1=0.3, {\zeta }_2=0.7, {\zeta }_3=0.1,$ $p_1=0.3, p_2=0.4, d=0.5, {\gamma }_1=6\times {10}^{-8}, {\gamma }_2=3\times {10}^{-6}, {\gamma }_3=1\times {10}^{-7}, {\gamma }_4=0.97,$ $\nu =2, \lambda =0.01, \delta =2, {\psi }_1=0.2, {\psi }_2=0.02, \kappa =0.5, \eta =0.1, k_1=0.1, k_2=0.2, k_3=0.3$:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\begin{array}{cc}{D}^{0.7}N\left(\tau \right)=0.3N\left(\tau \right)(0.1-0.3N\left(\tau \right)-(6\times {10}^{-8})T\left(\tau \right))-0.1N\left(\tau \right)E\left(\tau \right),\hfill & N_0=0.2,\\ D^{0.7}T\left(\tau \right)=0.4T\left(\tau \right)(0.1-0.7T\left(\tau \right)-(3\times {10}^{-6})I\left(\tau \right))-2T\left(\tau \right)+0.1N\left(\tau \right)T\left(\tau \right)E\left(\tau \right),\hfill & T_0=0.2,\\ D^{0.7}I\left(\tau \right)=0.001+0.3I\left(\tau \right)(0.2-(1\times {10}^{-7})T\left(\tau \right))-0.01I\left(\tau \right)E\left(\tau \right),\hfill & I_0=0.2,\\ D^{0.7}E\left(\tau \right)=-0.97E\left(\tau \right)+1,\hfill & E_0=0.2.\end{array}\hfill \end{array}\right.\end{array}$$

(6)

• Case 3:

Adopt the following fractional-order immune-chemotherapeutic treatment of breast cancer-related model with the designated values $\alpha =0.9, {\zeta }_1=0.3, {\zeta }_2=0.7, {\zeta }_3=0.1,$ $p_1=0.3, p_2=0.4, d=0.5, {\gamma }_1=6\times {10}^{-8}, {\gamma }_2=3\times {10}^{-6}, {\gamma }_3=1\times {10}^{-7}, {\gamma }_4=0.97,$ $\nu =2, \lambda =0.01, \delta =2, {\psi }_1=0.2, {\psi }_2=0.02, \kappa =0.5, \eta =0.1, k_1=0.1, k_2=0.2, k_3=0.3$:

$$\begin{array}{c}\hfill \left\{\begin{array}{c}\begin{array}{ccc}& D^{0.9}N\left(\tau \right)=0.3N\left(\tau \right)(0.1-0.3N\left(\tau \right)-(6\times {10}^{-8})T\left(\tau \right))-0.1N\left(\tau \right)E\left(\tau \right),\hfill & N_0=0.2,\\ & D^{0.9}T\left(\tau \right)=0.4T\left(\tau \right)(0.1-0.7T\left(\tau \right)-(3\times {10}^{-6})I\left(\tau \right))-2T\left(\tau \right)+0.1N\left(\tau \right)T\left(\tau \right)E\left(\tau \right),\hfill & T_0=0.2,\\ & D^{0.9}I\left(\tau \right)=0.001+0.3I\left(\tau \right)(0.2-(1\times {10}^{-7})T\left(\tau \right))-0.01I\left(\tau \right)E\left(\tau \right),\hfill & I_0=0.2,\\ & D^{0.9}E\left(\tau \right)=-0.97E\left(\tau \right)+1,\hfill & E_0=0.2.\end{array}\hfill \end{array}\right.\end{array}$$

(7)

Numerical presentations of the simulations of fractional order immune-chemotherapeutic treatment of breast cancer-associated model are shown by applying the stochastic LMBNNs processes involving 14 neurons including data selection comprising 75% for training, 15% for testing as well as 10% authorization. Figure 3 depicts the structure of a hidden, output, and input neuron.

Figure 4, Figure 5 and Figure 6 show the graphical visualizations used to analyze the fractional order immune-chemotherapeutic treatment for breast cancer-associated mathematical model employing the LMBNNs processes. The graphical representations in Figure 4 and Figure 5 are presented to examine the best performances with STs. To solve the fractional-order immune-chemotherapeutic treatment of breast cancer-associated mathematical model, the MSE and STs values of training, best curves, as well as authentication are produced in Figure 4. The derived values are $8.8007\times {10}^{-9}$, $8.449\times {10}^{-11}$, and $5.0831\times {10}^{-9}$, respectively, based on the best performances of the fractional-order immune-chemotherapeutic treatment of breast cancer-associated mathematical model at epochs 96, 109, and 18.

In Figure 4, overall gradient measurements are also plotted to solve the fractional-order immune-chemotherapeutic treatment of breast cancer-related mathematical model employing LMBNNs. For cases 1, 2, and 3, these gradient performances were determined to be $9.5876\times {10}^{-8}$, $1.5573\times {10}^{-7}$, and $2.4897\times {10}^{-7}$, respectively. These graphical visualizations illustrate the convergence of suggested LMBNNs to solve the fractional-order immune-chemotherapeutic treatment of breast cancer mathematical model employing LMBNNs. Figure 6, Figure 7 and Figure 8 show the values of the fitting curves used to address every case for the proposed fractional-order immune-chemotherapeutic treatment of breast cancer mathematical model.

Those visualizations compare the performance of the reference and achieved findings. Error plots are representing the substantiation, testing, as well as training to address all scenarios of the fractional-order immune-chemotherapeutic treatment of breast cancer-associated mathematical model. Relying on the fractional-order immune-chemotherapeutic treatment of breast cancer-associated mathematical model, various EHs are displayed through Figure 5d–f, as well as corresponding regression measures, are supplied by Figure 5a–c. For cases 1, 2, and 3, the EHs are estimated as $1.45\times {10}^{-5}$, $1.19\times {10}^{-5}$, and $1.3\times {10}^{-5}$, respectively.

In Figure 6, the correlation has been demonstrated to confirm the regression performance. Such correlation plots for the fractional-order immune-chemotherapeutic treatment of breast cancer-linked mathematical model are calculated as 1. The training, testing, and authentication expressions indicate the correctness of the stochastic LMBNNs procedure for solving the fractional order immune-chemotherapeutic treatment of breast cancer mathematical model.

Table 2. The LMBNNs procedure is adopted to solve the fractional-order immune-chemotherapeutic treatment-based mathematical model of breast cancer.

	MSE
Case	Training	Verification	Testing	Performance	Gradient	Mu	Epoch	Time
1	$1.52\times {10}^{-8}$	$8.80\times {10}^{-9}$	$2.85\times {10}^{-9}$	$1.53\times {10}^{-8}$	$9.59\times {10}^{-8}$	$1.00\times {10}^{-9}$	96	2
2	$1.67\times {10}^{-9}$	$8.44\times {10}^{-11}$	$1.72\times {10}^{-9}$	$1.49\times {10}^{-9}$	$1.57\times {10}^{-7}$	$1.00\times {10}^{-10}$	115	2
3	$3.78\times {10}^{-9}$	$5.08\times {10}^{-9}$	$5.16\times {10}^{-9}$	$2.29\times {10}^{-9}$	$2.49\times {10}^{-7}$	$1.00\times {10}^{-9}$	24	1

displays the convergence of the fractional-order immune-chemotherapeutic treatment of breast cancer-related mathematical model employing MSE, complexity, training, authentication, iterations, testing, as well as backpropagation.

Figure 7 and Figure 8 exhibit the plotting for their result comparisons as well as AE values. To address the fractional-order immune-chemotherapeutic treatment of breast cancer-associated mathematical models employing stochastic LMBNNs, numerical expressions are presented. The overlapping findings of the reference and derived numerical performances are presented in Figure 7. The overlapping result validates the LMBNNs’ exactness in solving the fractional order immune-chemotherapeutic treatment of breast cancer-associated mathematical model.

Figure 8 depicts the AE parameters employed to solve the breast cancer model. Regarding cases 1 to 3, in the dynamics of the normal cell population $N\left(\tau \right)$, the AE values are found around ${10}^{-4}$–${10}^{-6}$, ${10}^{-5}$–${10}^{-7}$, and ${10}^{-5}$–${10}^{-6}$. Regarding cases 1 to 3, the AE values for tumour cells $T\left(\tau \right)$ are estimated in the range of ${10}^{-4}$–${10}^{-5}$, ${10}^{-4}$–${10}^{-7}$, and ${10}^{-4}$–${10}^{-6}$. Regarding cases 1 to 3, the AE for the class of immune reaction $I\left(\tau \right)$ was computed as ${10}^{-4}$–${10}^{-5}$, ${10}^{-5}$–${10}^{-7}$, and ${10}^{-5}$–${10}^{-6}$. Likewise, for cases 1 to 3, the AE for the estrogen compartment $E\left(\tau \right)$ was computed as ${10}^{-4}$–${10}^{-6}$, ${10}^{-5}$–${10}^{-7}$, and ${10}^{-4}$–${10}^{-5}$. Such AE values display the accuracy with which the suggested LMBNNs solved the fractional order immune-chemotherapeutic treatment of breast cancer mathematical model.

6. Conclusions

The numerical representations of the fractional-order breast cancer mathematical model are described throughout this paper. The goal of this research is to give a fractional-order assessment employing a mathematical model focused on the dynamics of breast cancer to offer better accurate system performances. That whole investigation also included an integer, nonlinear mathematical system with immune-chemotherapeutic treatment effects. The fractional-order breast cancer mathematical model is divided into four phenomena: normal cell population, tumor cells, immune reaction class, and estrogen compartment, and is referred to called the NTIE mathematical system. The numerical performances of either the NTIE mathematical model built on fractional-order breast cancer have never been published or solved via stochastic Levenberg–Marquardt backpropagation neural networks. To solve the fractional-order NTIE mathematical model, three cases with varying values of the fractional-order have been supplied. The data used to offer the numerical solutions of the fractional-order NTIE mathematical model is divided as follows: 75% in training, 15% to testing, and 10% for authorization. The numerical performances of the fractional-order breast cancer-based NTIE mathematical system were shown using fourteen neurons. The numerical findings of the fractional-order NTIE mathematical system were compared with the Adams–Bashforth–Moulton mathematical system. The reported numerical findings were produced using LMBNNs to decrease the MSE. The STs, regression, correlation, EHs, as well as MSE are being used to demonstrate the reliability as well as the competency of LMBNNs, as well as their numerical performances. The matching of reference and actual findings demonstrate the accuracy of the LMBNNs based on the fractional-order NTIE mathematical model. The scheme’s performance is validated by the consistency and reliability of the suggested LMBNNs. LMBNNs may be exploited in the upcoming investigation to offer numerical measurement results of the lonngren-wave, as well as fluid dynamics [46,47,48,49].