Fractional Order Distributed Model Predictive Control of Fast and Strong Interacting Systems
Abstract
:1. Introduction
2. Description of Multiple Lighting Control Systems
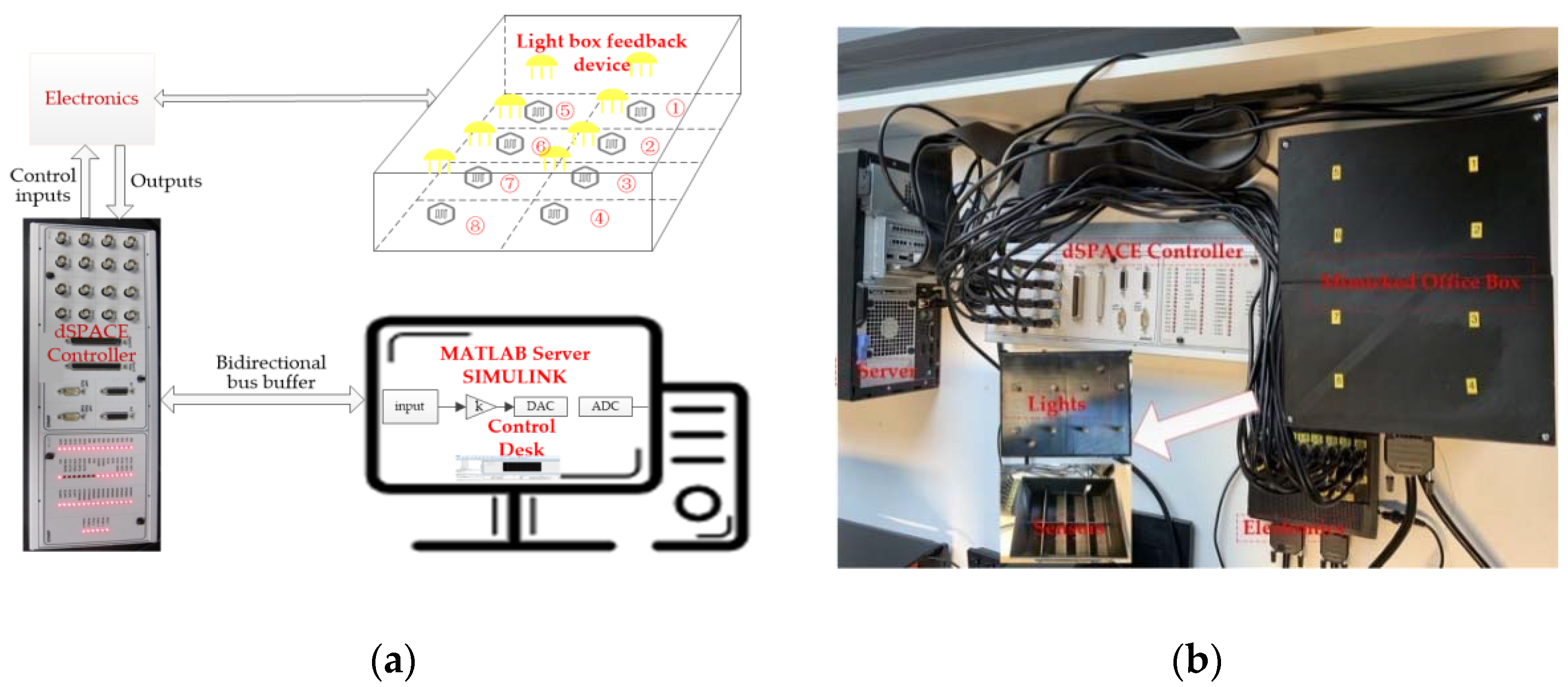
2.1. Multiple Lighting Control System Based on dSPACE
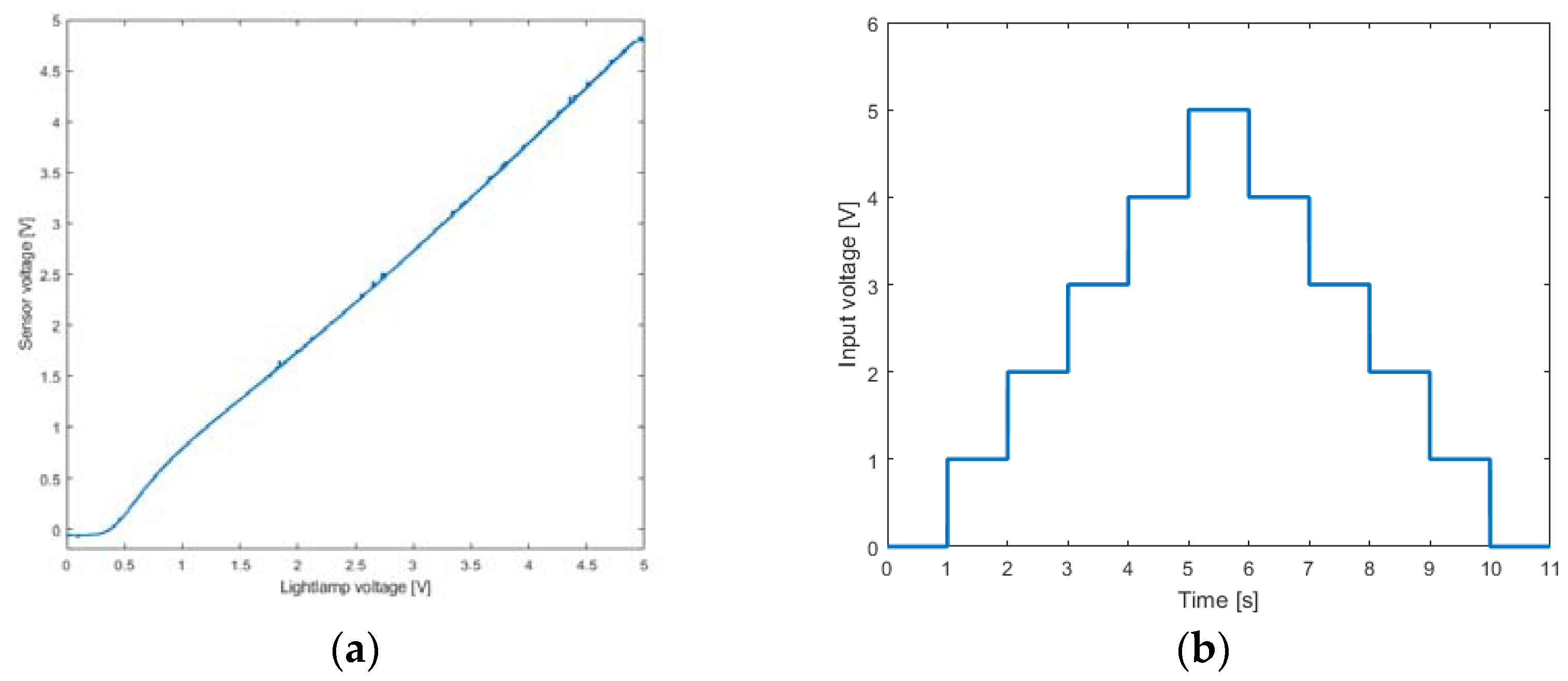
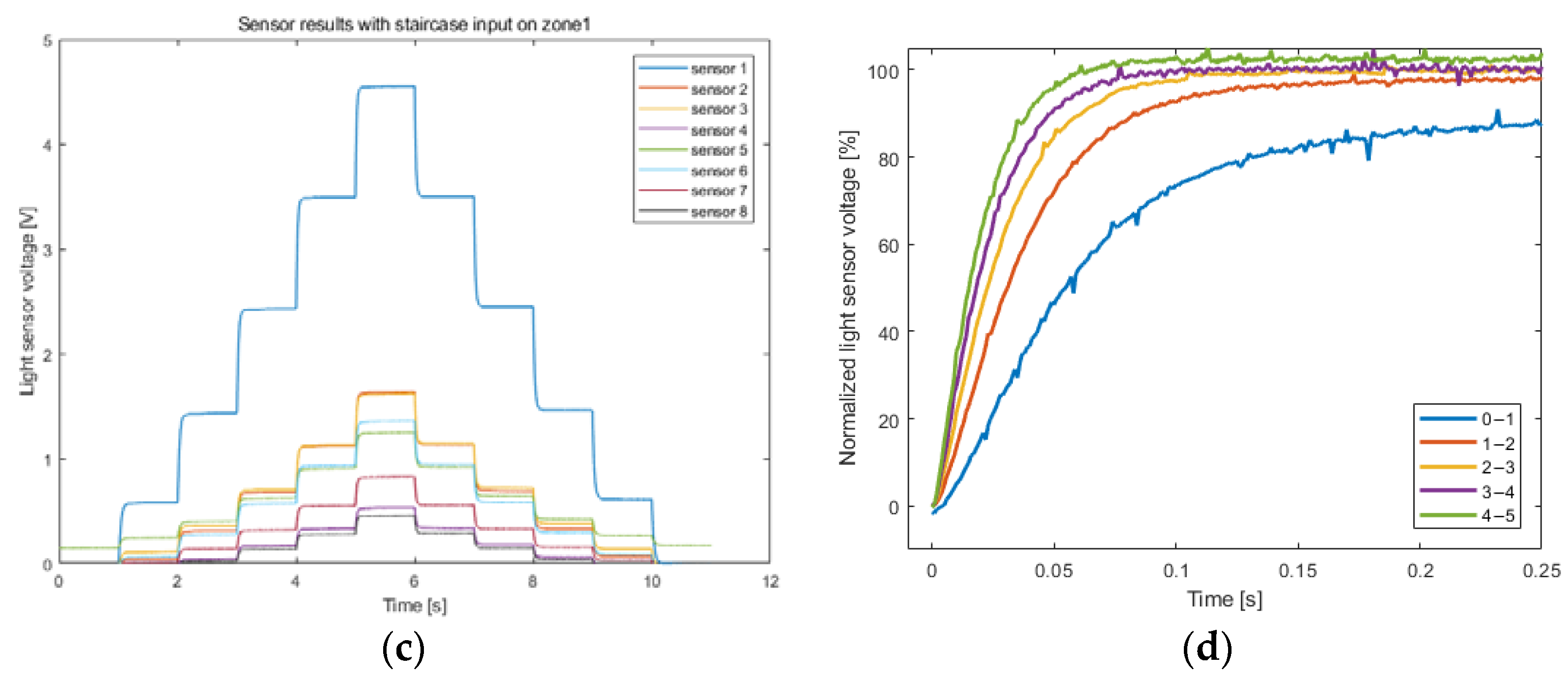
2.2. System Characterization and Calibration
2.3. System Identication
3. Design of Control Scheme Based on Fractional Order Distributed MPC
3.1. Problem Formulation
3.2. Fractional Order Distributed MPC
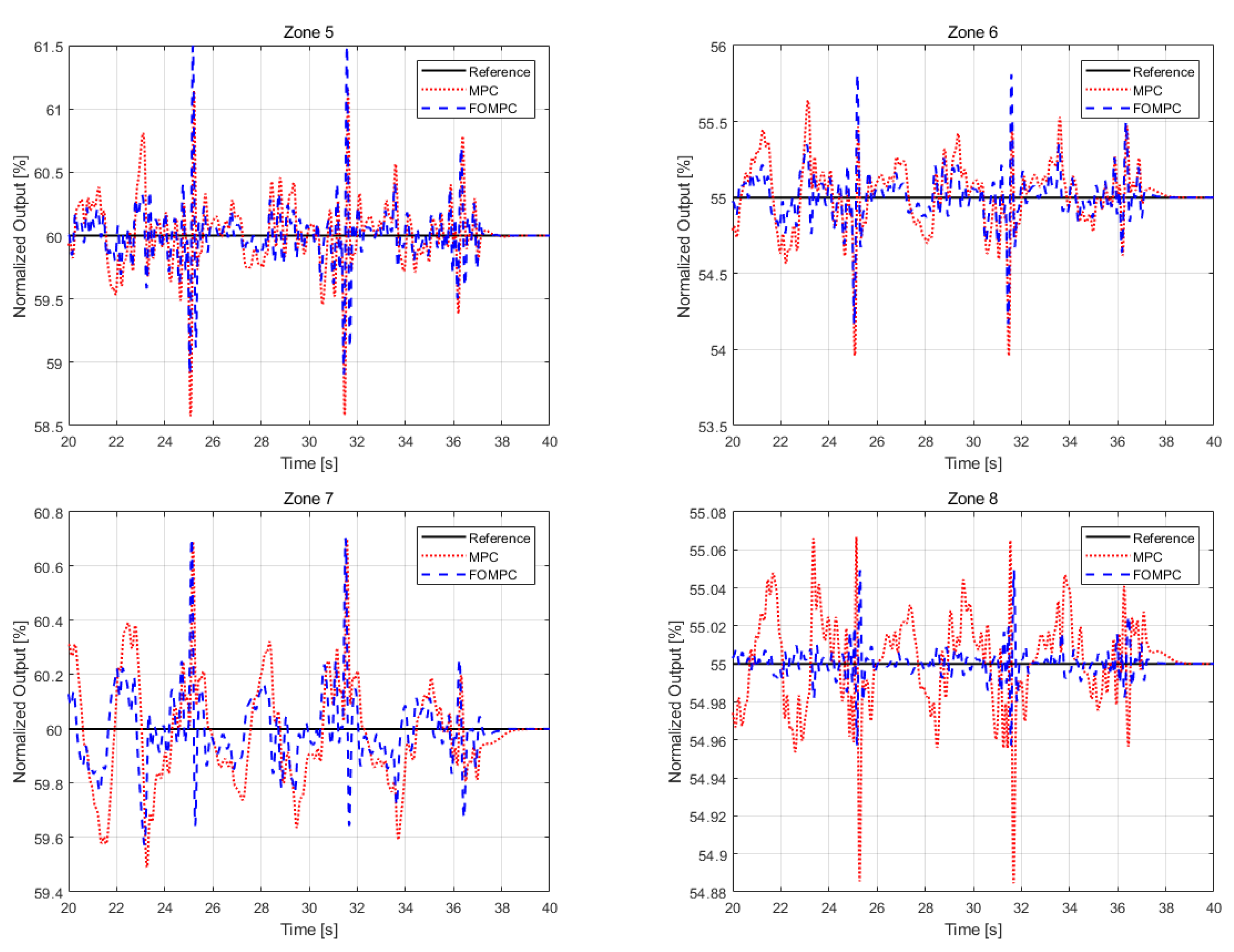
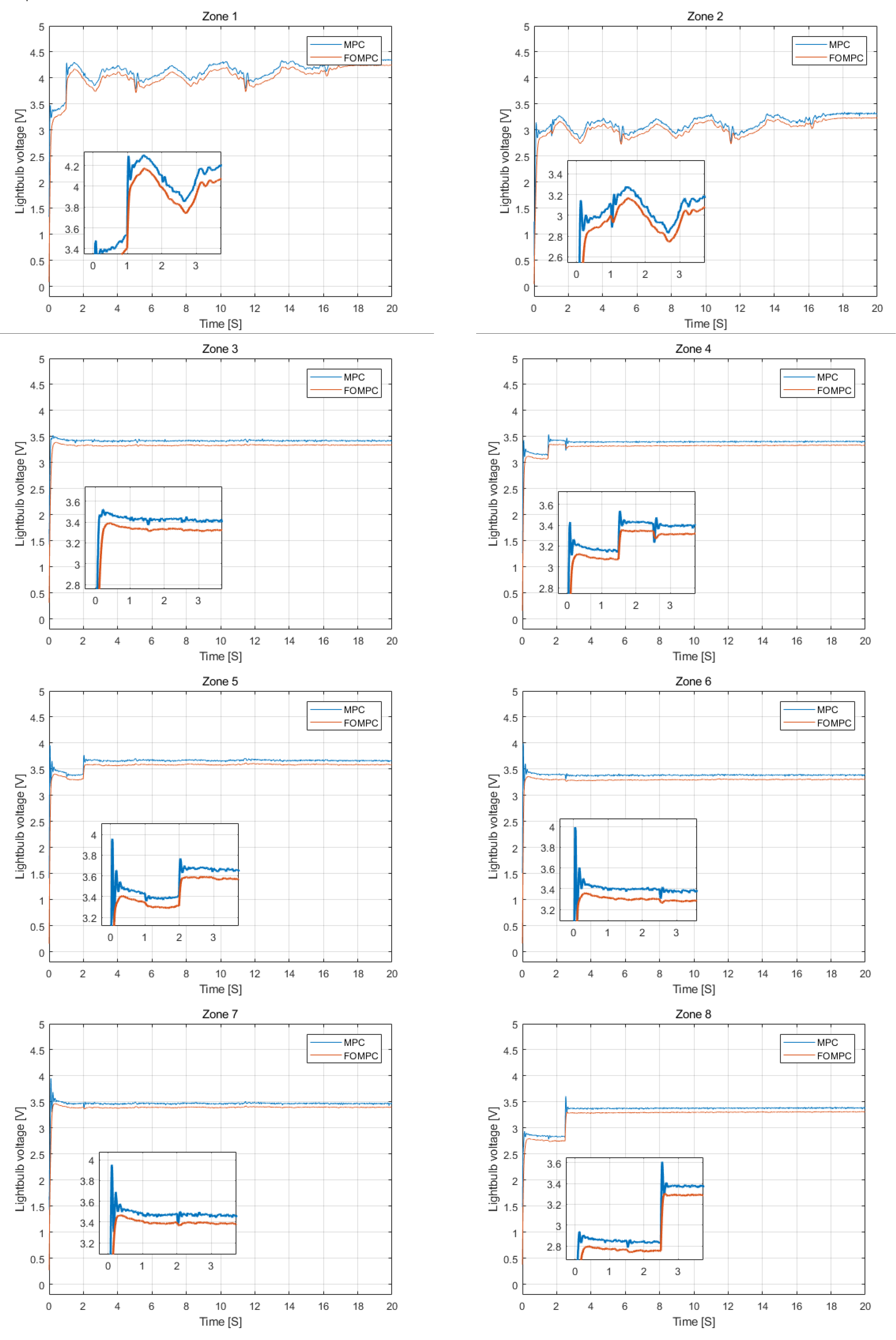
4. Results and Discussion
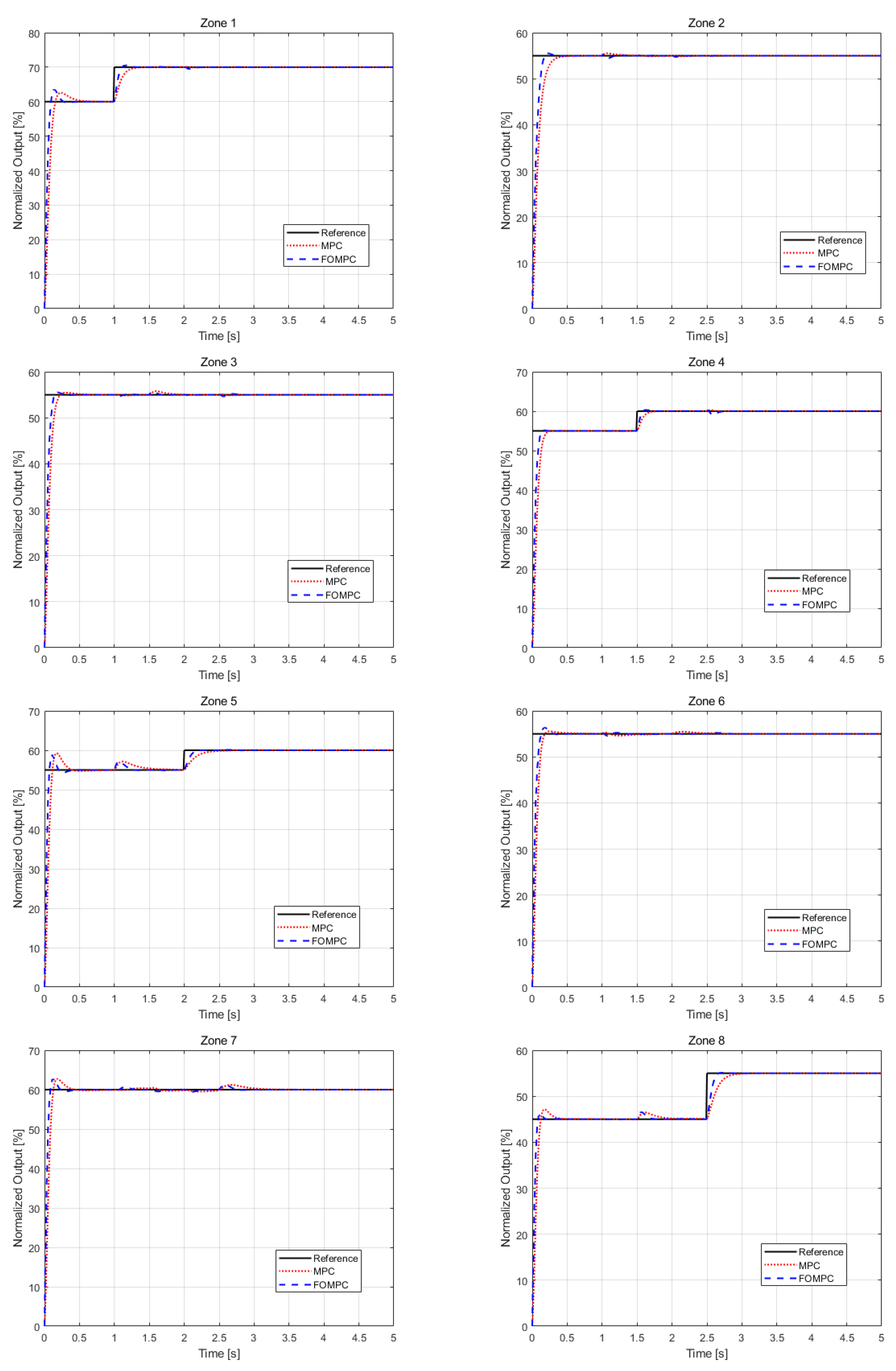
4.1. Numerical Simulation
4.2. Experiment Based on Multiple Lighting Control System
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Cho, J.-H.; Wang, Y.; Chen, I.-R.; Chan, K.S.; Swami, A. A Survey on modeling and optimizing multi-objective systems. IEEE Commun. Surv. Tutorials 2017, 19, 1867–1901. [Google Scholar] [CrossRef]
- Fazlollahi, S.; Becker, G.; Ashouri, A.; Maréchal, F. Multi-objective, multi-period optimization of district energy systems: IV–A case study. Energy 2015, 84, 365–381. [Google Scholar] [CrossRef]
- Khezri, R.; Amin, M. Review on the state-of-the-art multi-objective optimisation of hybrid standalone/grid-connected energy systems. IET Gener. Trans. Distrib. 2020, 14, 4285–4300. [Google Scholar] [CrossRef]
- Lokeshgupta, B.; Sivasubramani, S. Multi-objective home energy management with battery energy storage systems. Sustain. Cities Soc. 2019, 47, 101458. [Google Scholar] [CrossRef]
- Gangwar, P.; Singh, S.N.; Chakrabarti, S. Multi-objective planning model for multi-phase distribution system under uncertainty considering reconfiguration. IET Renew. Power Gener. 2019, 13, 2070–2083. [Google Scholar] [CrossRef]
- Miao, Z.; Yu, J.; Ji, J.; Zhou, J. Multi-objective region reaching control for a swarm of robots. Automatica 2019, 103, 81–87. [Google Scholar] [CrossRef]
- Tan, H.; Wang, Y.; Zhong, H.; Wu, M.; Jiang, Y. Coordination of low-power nonlinear multi-agent systems using cloud computing and a data-driven hybrid predictive control method. Control Eng. Pr. 2021, 108, 104722. [Google Scholar] [CrossRef]
- Pandharipande, A.; Newsham, G. Lighting controls: Evolution and revolution. Light. Res. Technol. 2018, 50, 115–128. [Google Scholar] [CrossRef]
- Wagiman, K.R.; Abdullah, M.N.; Hassan, M.Y.; Radzi, N.H.M. A new optimal light sensor placement method of an indoor lighting control system for improving energy performance and visual comfort. J. Build. Eng. 2020, 30, 101295. [Google Scholar] [CrossRef]
- Pandharipande, A.; Caicedo, D. Smart indoor lighting systems with luminaire-based sensing: A review of lighting control approaches. Energy Build. 2015, 104, 369–377. [Google Scholar] [CrossRef]
- Cimini, G.; Freddi, A.; Ippoliti, G.; Monteriù, A.; Pirro, M. A smart lighting system for visual comfort and energy savings in industrial and domestic use. Electr. Power Components Syst. 2015, 43, 1696–1706. [Google Scholar] [CrossRef]
- Ghita, M.; Diaz, R.A.C.; Birs, I.R.; Copot, D.; Ionescu, C.M. Ergonomic and economic office light level control. Energies 2022, 15, 734. [Google Scholar] [CrossRef]
- Juntunen, E.; Sarjanoja, E.-M.; Eskeli, J.; Pihlajaniemi, H.; Österlund, T. Smart and dynamic route lighting control based on movement tracking. Build. Environ. 2018, 142, 472–483. [Google Scholar] [CrossRef]
- Byun, W.-J.; Jin, Y.-S.; Kim, Y.-W.; Lim, J.-H. Design of Lighting Control System Considering Lighting Uniformity and Discomfort Glare for Indoor Space. In Proceedings of the 2018 International Conference on Platform Technology and Service (PlatCon), Sydney, NSW, Australia, 21–23 November 2018; pp. 1–6. [Google Scholar]
- Liu, G.-P. Coordinated control of networked multiagent systems with communication constraints using a proportional integral predictive control strategy. IEEE Trans. Cybern. 2020, 50, 4735–4743. [Google Scholar] [CrossRef]
- Manzano, J.M.; Limon, D.; de la Peña, D.M.; Calliess, J.-P. Robust learning-based MPC for nonlinear constrained systems. Automatica 2020, 117, 108948. [Google Scholar] [CrossRef]
- Peng, H.; Wang, W.; An, Q.; Xiang, C.; Li, L. Path tracking and direct yaw moment coordinated control based on robust MPC with the finite time horizon for autonomous independent-drive vehicles. IEEE Trans. Veh. Technol. 2020, 69, 6053–6066. [Google Scholar] [CrossRef]
- Christofides, P.D.; Scattolini, R.; de la Peña, D.M.; Liu, J. Distributed model predictive control: A tutorial review and future research directions. Comput. Chem. Eng. 2013, 51, 21–41. [Google Scholar] [CrossRef]
- Halvgaard, R.; Vandenberghe, L.; Poulsen, N.K.; Madsen, H.; Jørgensen, J.B. Distributed model predictive control for smart energy systems. IEEE Trans. Smart Grid 2016, 7, 1675–1682. [Google Scholar] [CrossRef]
- Spudić, V.; Conte, C.; Baotić, M.; Morari, M. Cooperative distributed model predictive control for wind farms. Optim. Control Appl. Methods 2015, 36, 333–352. [Google Scholar] [CrossRef]
- Chen, L.; Hopman, H.; Negenborn, R.R. Distributed model predictive control for vessel train formations of cooperative multi-vessel systems. Transp. Res. Part C Emerg. Technol. 2018, 92, 101–118. [Google Scholar] [CrossRef]
- Wu, Z.; Rincon, D.; Christofides, P.D. Real-time adaptive machine-learning-based predictive control of nonlinear processes. Ind. Eng. Chem. Res. 2020, 59, 2275–2290. [Google Scholar] [CrossRef]
- Domek, S. Fractional-Order Differential Calculus in Model Predictive Control; West Pomeranian University of Technology Academic Press: Szczecin, Poland, 2013. [Google Scholar]
- Nigmatullin, R.; Baleanu, D.; Fernandez, A. Balance equations with generalised memory and the emerging fractional kernels. Nonlinear Dyn. 2021, 104, 4149–4161. [Google Scholar] [CrossRef]
- Domek, S. Mixed logical dynamical modeling of discrete-time hybrid fractional systems. In Fractional Dynamical Systems: Methods, Algorithms and Applications; Springer: Berlin/Heidelberg, Germany, 2022; pp. 77–105. [Google Scholar]
- Zheng, W.; Huang, R.; Luo, Y.; Chen, Y.; Wang, X.; Chen, Y. A look-up table based fractional order composite controller synthesis method for the pmsm speed servo system. Fractal Fract. 2022, 6, 47. [Google Scholar] [CrossRef]
- Zhao, S.; Cajo, R.; De De Keyser, R.; Ionescu, C.-M. The potential of fractional order distributed MPC applied to steam/water loop in large scale ships. Processes 2020, 8, 451. [Google Scholar] [CrossRef] [Green Version]
- Cajo, R.; Zhao, S.; Plaza, D.; Keyser, R.D.; Ionescu, C. A fractional order predictive control for trajectory tracking of the AR. drone quadrotor. In Portuguese Conference on Automatic Control; Springer: Berlin/Heidelberg, Germany, 2020; pp. 528–537. [Google Scholar]
- Juchem, J.; Muresan, C.; De Keyser, R.; Ionescu, C.-M. Robust fractional-order auto-tuning for highly-coupled MIMO systems. Heliyon 2019, 5, e02154. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Domek, S. Switched fractional state-space predictive control methods for non-linear fractional systems. In Conference on Non-Integer Order Calculus and Its Applications; Springer: Berlin/Heidelberg, Germany, 2018; pp. 113–127. [Google Scholar]
- Si, X.; Wang, Z.; Song, Z.; Zhang, Z. Asymptotic stabilization of delayed linear fractional-order systems subject to state and control constraints. Fractal Fract. 2022, 6, 67. [Google Scholar] [CrossRef]
- Ionescu, C.M.; Dulf, E.H.; Ghita, M.; Muresan, C.I. Robust controller design: Recent emerging concepts for control of mechatronic systems. J. Frankl. Inst. 2020, 357, 7818–7844. [Google Scholar] [CrossRef]
- Cajo, R.; Mac, T.T.; Plaza, D.; Copot, C.; De Keyser, R.; Ionescu, C. A survey on fractional order control techniques for unmanned aerial and ground vehicles. IEEE Access 2019, 7, 66864–66878. [Google Scholar] [CrossRef]
- Vilanova, R.; Antonio, V. PID Control in the Third Millennium; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Padula, F.; Visioli, A. Advances in Robust Fractional Control; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- De Keyser, R.; Muresan, C.I.; Ionescu, C.M. An efficient algorithm for low-order direct discrete-time implementation of fractional order transfer functions. ISA Trans. 2018, 74, 229–238. [Google Scholar] [CrossRef]




| Parameters | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | Zone 6 | Zone 7 | Zone 8 |
|---|---|---|---|---|---|---|---|---|
| Step time | 1 | 0 | 0 | 1.5 | 2 | 0 | 0 | 2.5 |
| Initial value | 3 | 2.75 | 2.75 | 2.75 | 2.75 | 2.75 | 3 | 2.25 |
| Final value | 3.5 | 2.75 | 2.75 | 3 | 3 | 2.75 | 3 | 2.75 |
| Index | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | Zone 6 | Zone 7 | Zone 8 | |
|---|---|---|---|---|---|---|---|---|---|
| IARE | MPC | 19.4998 | 29.3360 | 12.3687 | 8.3487 | 17.2006 | 15.1618 | 14.6329 | 10.6225 |
| FOMPC | 11.8894 | 18.0526 | 6.9735 | 5.2766 | 10.4721 | 9.4187 | 8.7458 | 5.4719 |
| Index | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | Zone 6 | Zone 7 | Zone 8 |
|---|---|---|---|---|---|---|---|---|
| RIARE | 1.6400 | 1.6250 | 1.7736 | 1.5822 | 1.6425 | 1.6097 | 1.6731 | 1.9413 |
| Index | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | Zone 6 | Zone 7 | Zone 8 | |
|---|---|---|---|---|---|---|---|---|---|
| IARE | MPC | 19.8122 | 25.5572 | 16.2104 | 14.1862 | 16.0227 | 16.0181 | 14.1150 | 17.1420 |
| FOMPC | 12.5854 | 17.6462 | 12.2813 | 10.9057 | 12.0393 | 12.4272 | 10.7923 | 13.0894 |
| Index | Zone 1 | Zone 2 | Zone 3 | Zone 4 | Zone 5 | Zone 6 | Zone 7 | Zone 8 |
|---|---|---|---|---|---|---|---|---|
| RIARE | 1.5742 | 1.4483 | 1.3199 | 1.3008 | 1.3309 | 1.2890 | 1.3079 | 1.3096 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chi, C.; Cajo, R.; Zhao, S.; Liu, G.-P.; Ionescu, C.-M. Fractional Order Distributed Model Predictive Control of Fast and Strong Interacting Systems. Fractal Fract. 2022, 6, 179. https://doi.org/10.3390/fractalfract6040179
Chi C, Cajo R, Zhao S, Liu G-P, Ionescu C-M. Fractional Order Distributed Model Predictive Control of Fast and Strong Interacting Systems. Fractal and Fractional. 2022; 6(4):179. https://doi.org/10.3390/fractalfract6040179
Chicago/Turabian StyleChi, Chuanguo, Ricardo Cajo, Shiquan Zhao, Guo-Ping Liu, and Clara-Mihaela Ionescu. 2022. "Fractional Order Distributed Model Predictive Control of Fast and Strong Interacting Systems" Fractal and Fractional 6, no. 4: 179. https://doi.org/10.3390/fractalfract6040179
APA StyleChi, C., Cajo, R., Zhao, S., Liu, G.-P., & Ionescu, C.-M. (2022). Fractional Order Distributed Model Predictive Control of Fast and Strong Interacting Systems. Fractal and Fractional, 6(4), 179. https://doi.org/10.3390/fractalfract6040179









