Thermal Performance and Geometric Optimization of Fractal T-Shaped Highly-Conductive Material for Cooling of a Rectangular Chip
Abstract
:1. Introduction
2. Numerical Methods
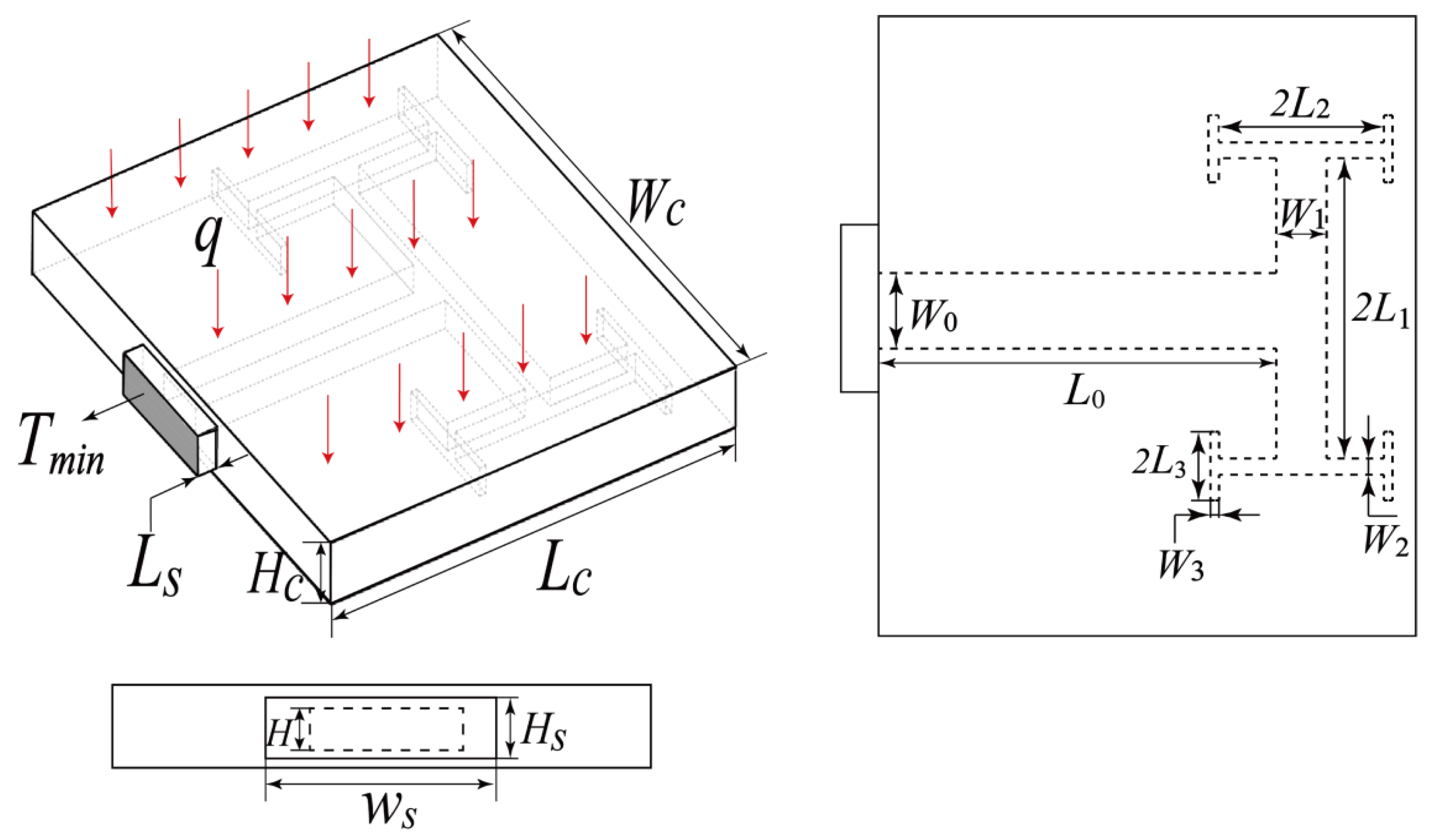
2.1. Description of the Simulation Model
- (1)
- α = Li+1/Li (i = 0,1,2,3,…). The α is the length ratio of branches at two consecutive branching levels.
- (2)
- β = Wi+1/Wi (i = 0,1,2,3,…). The β is the width ratio of branches at two consecutive branching levels.
- (3)
- m. The m is the maximum branching level.
- (4)
- L0. The L0 is the length of the branch at the initial level.
- (5)
- H. The H is the thickness of the HCM.
- (6)
- V. The V is the total volume of the HCM.
2.2. Numerical Method
- (1)
- The heat conduction is steady state.
- (2)
- The physical properties of the solid materials used in the study are not affected by temperature, and the heat exchange and radiation between the chip and the environment are ignored.
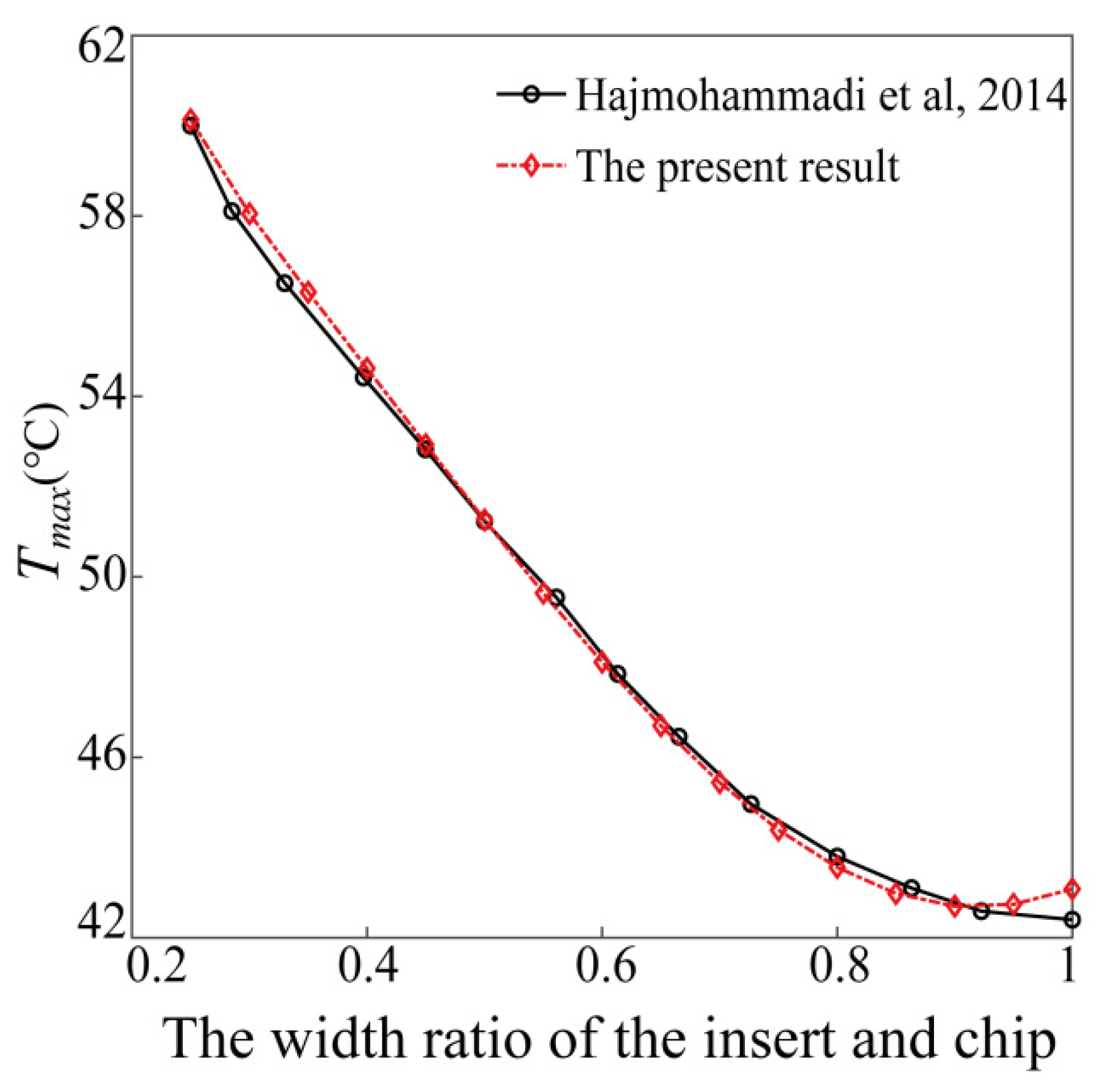
2.3. Mesh Test and Data Validation
3. Results and Discussion
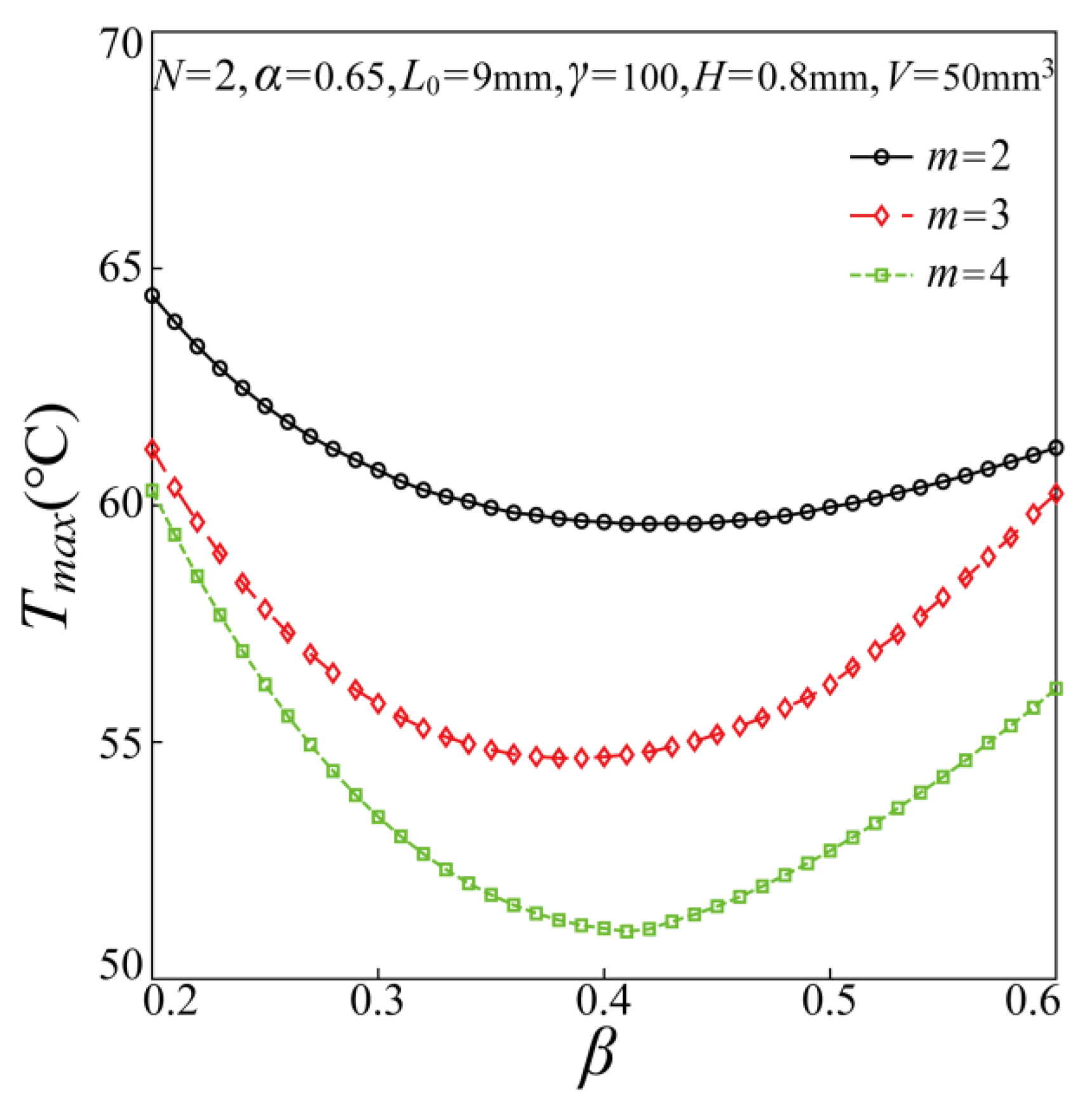
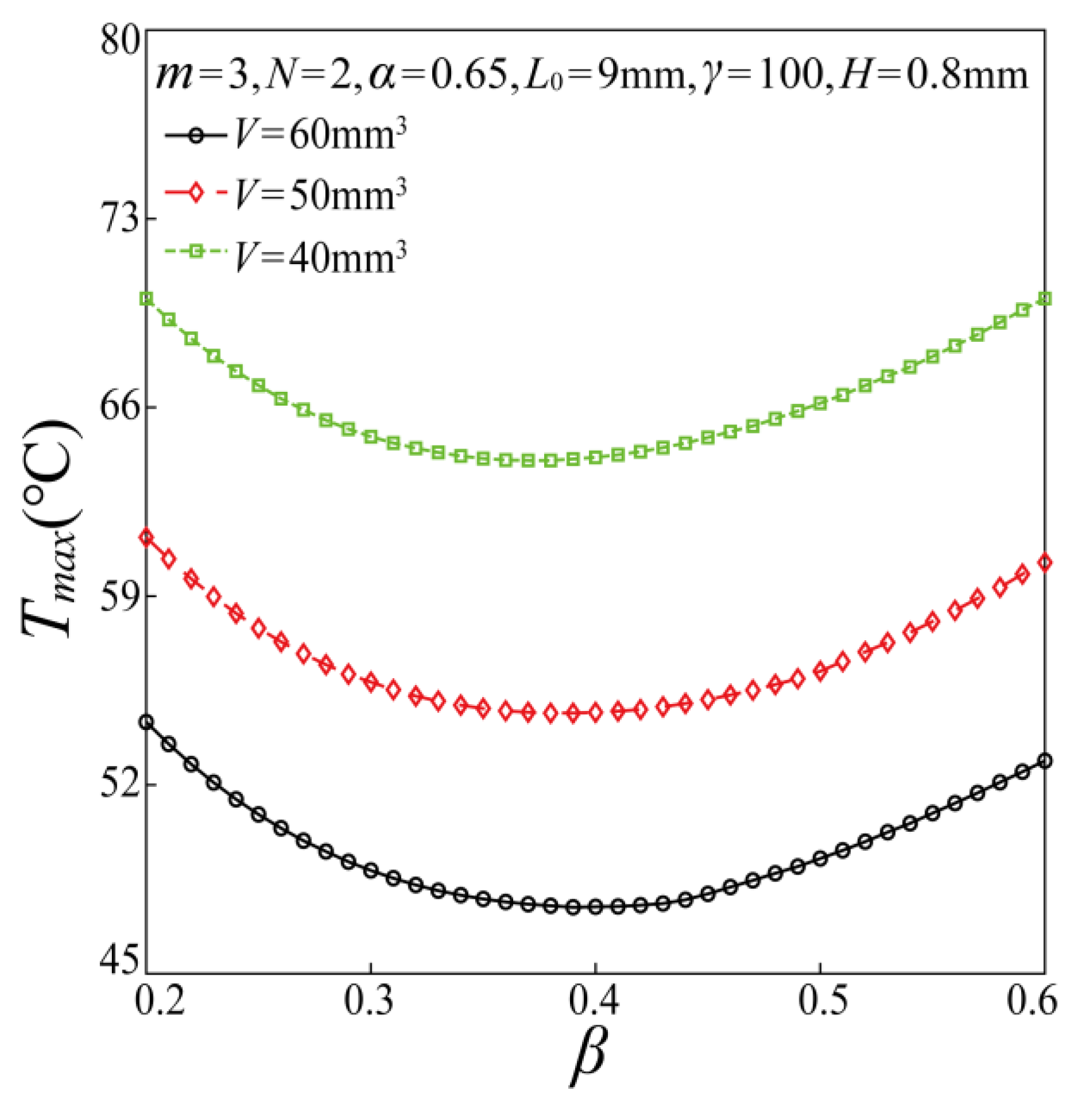
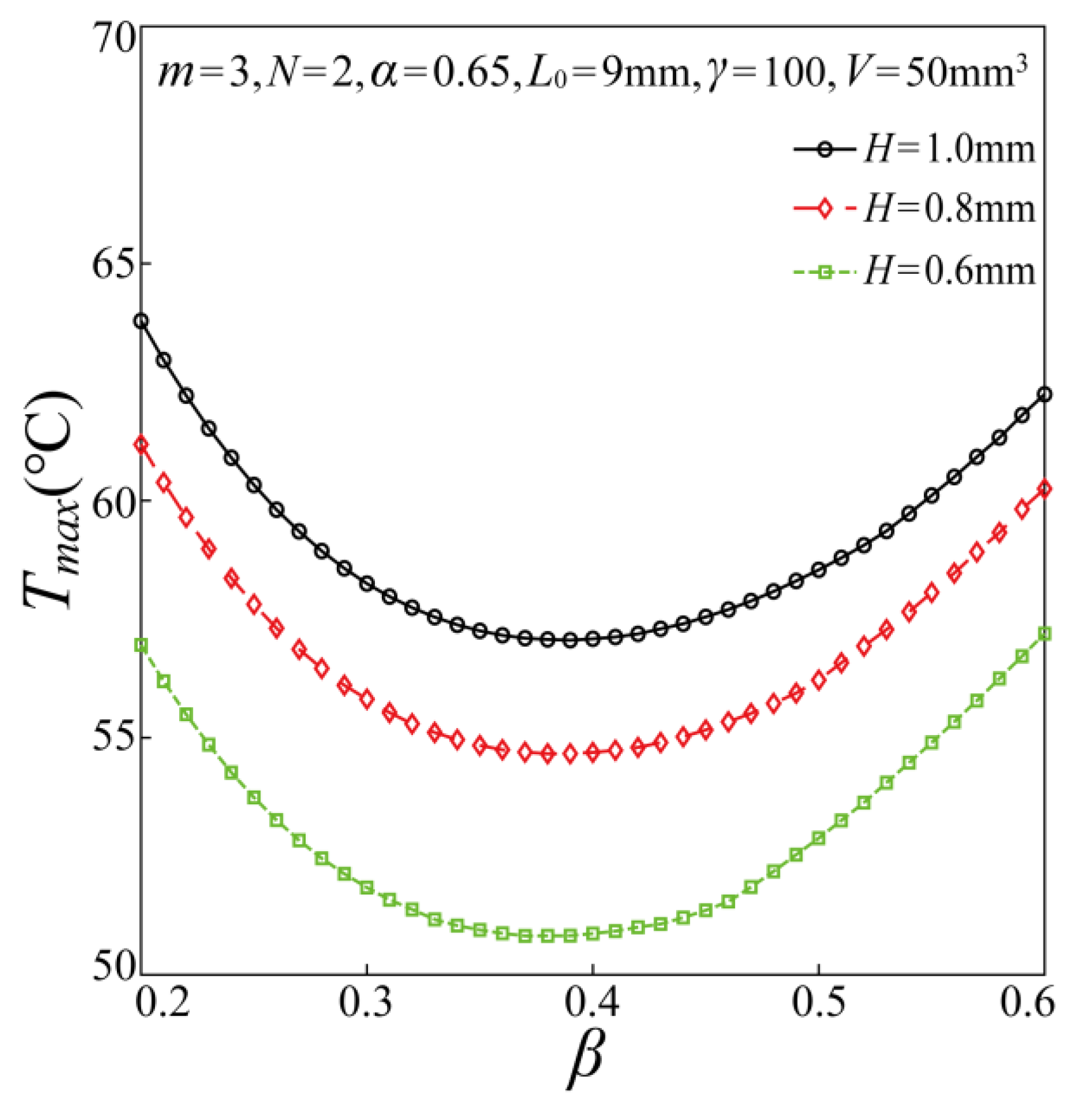
3.1. The Thermal Performances of the Branched HCM
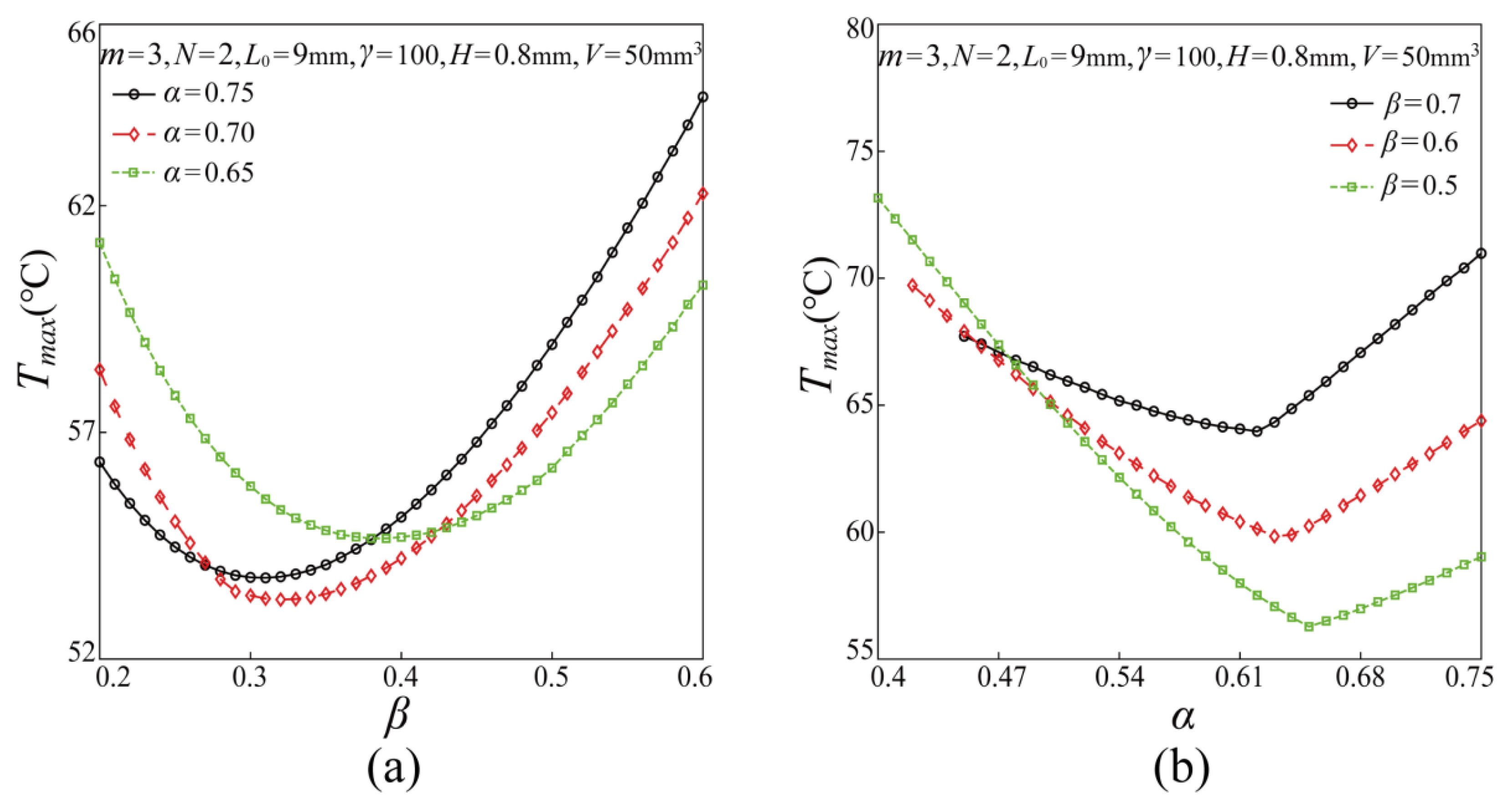
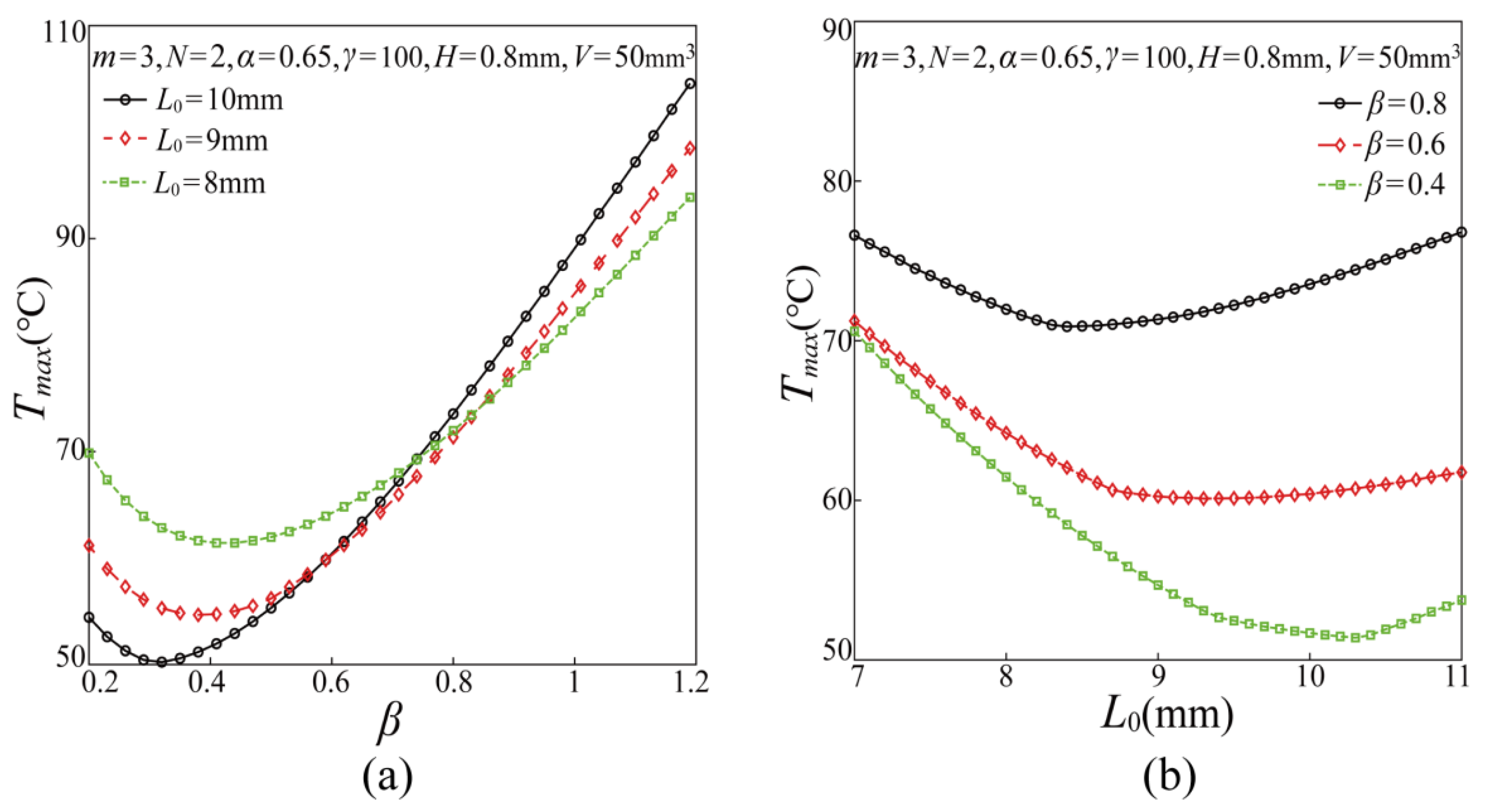
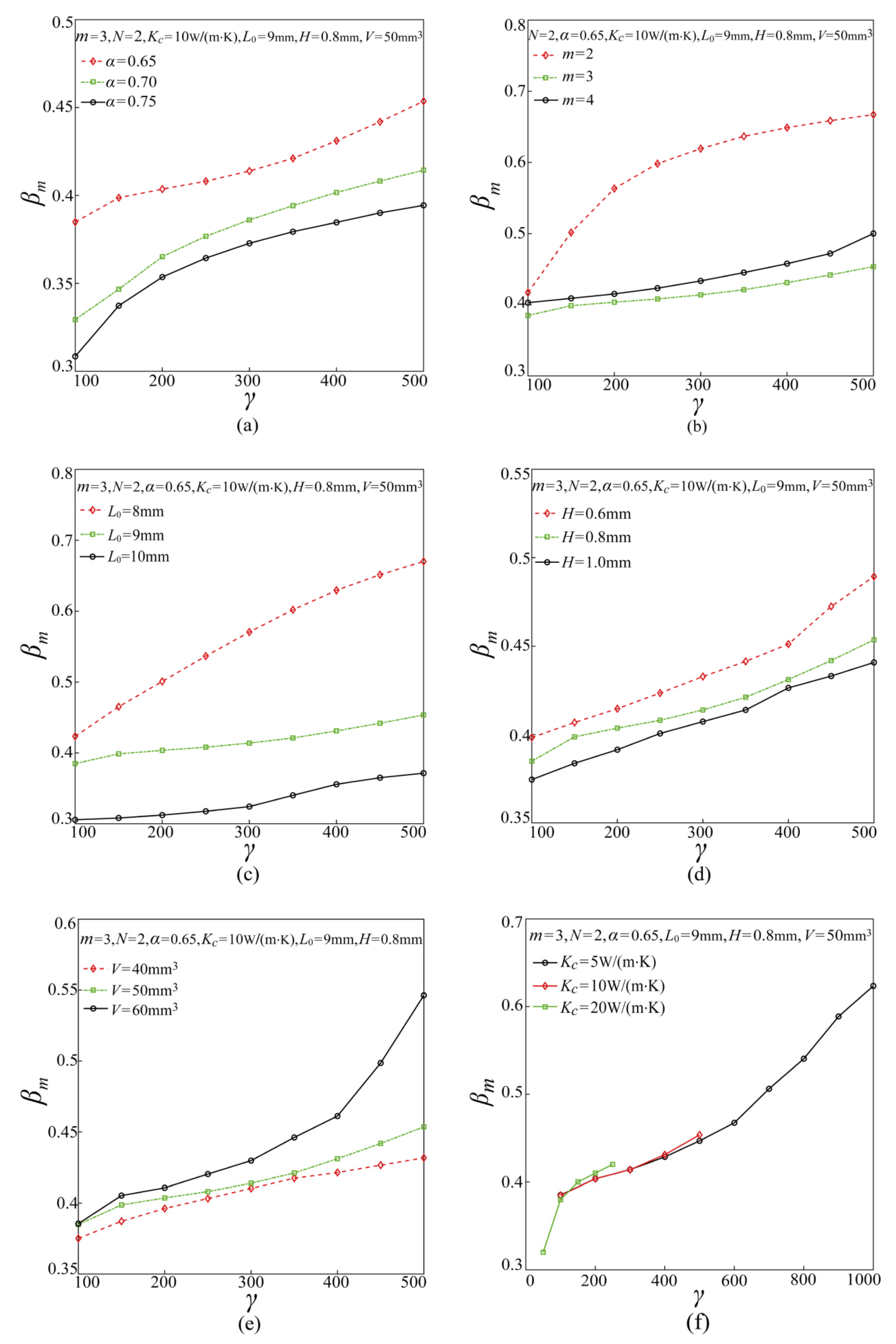
3.2. The Optimal Width Ratio of the Branched HCM
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- He, Z.; Yan, Y.; Zhang, Z.; Lund, H.; Kaiser, M.J. Thermal management and temperature uniformity enhancement of electronic devices by micro heat sinks: A review. Energy 2021, 216, 119223. [Google Scholar] [CrossRef]
- Guo, K.; Qi, W.; Liu, B.; Liu, C.; Huang, Z.; Zhu, G. Optimization of an “area to point” heat conduction problem. Appl. Therm. Eng. 2016, 93, 61–71. [Google Scholar] [CrossRef]
- Bejan, A. Constructal-theory network of conducting paths for cooling a heat generating volume. Int. J. Heat Mass Transf. 1997, 40, 799–816. [Google Scholar] [CrossRef]
- Yu, L.T.; Chen, C.K. Optimization of circular fins with variable thermal parameters. J. Frankl. Inst. 1999, 336, 77–95. [Google Scholar] [CrossRef]
- Lorenzini, G.; Biserni, C.; Rocha, L.A.O. Constructal design of X-shaped conductive pathways for cooling a heat-generating body. Int. J. Heat Mass Transf. 2013, 58, 513–520. [Google Scholar] [CrossRef]
- Fagundes, T.M.; Yaghoobian, N.; Rocha, L.A.O.; Ordonez, J.C. Constructal design of branched conductivity pathways inserted in a trapezoidal body: A numerical investigation of the effect of body shape on optimal pathway structure. Proc. Rom. Acad. Ser. A Math. Phys. Tech. Sci. Inf. Sci. 2018, 19, 297–302. [Google Scholar]
- Yu, C.; Wu, S.; Huang, Y.; Yao, F.; Liu, X. Charging performance optimization of a latent heat storage unit with fractal tree-like fins. J. Energy Storage 2020, 30, 101498. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Rasouli, E.; Elmi, M.A. Geometric optimization of a highly conductive insert intruding an annular fin. Int. J. Heat Mass Transf. 2020, 146, 118910. [Google Scholar] [CrossRef]
- Lorenzini, G.; Barreto, E.X.; Beckel, C.C.; Schneider, P.S.; Rocha, L. Constructal design of I-shaped high conductive pathway for cooling a heat-generating medium considering the thermal contact resistance. Int. J. Heat Mass Transf. 2016, 93, 770–777. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Ahmadian, M.; Nourazar, S.S. Introducing highly conductive materials into a fin for heat transfer enhancement. Int. J. Mech. Sci. 2019, 150, 420–426. [Google Scholar] [CrossRef]
- Lorenzini, G.; Joneydi Shariatzadeh, O.; Hajmohammadi, M.R.; Biserni, C. Evolution in the Design of V-Shaped Highly Conductive Pathways Embedded in a Heat-Generating Piece. J. Heat Transf. 2015, 137, 61001. [Google Scholar]
- Hajmohammadi, M.R.; Abianeh, V.A.; Moezzinajafabadi, M.; Daneshi, M. Fork-shaped highly conductive pathways for maximum cooling in a heat generating piece. Appl. Therm. Eng. 2013, 61, 228–235. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Shariatzadeh, O.J.; Moulod, M.; Nourazar, S.S. Phi and Psi shaped conductive routes for improved cooling in a heat generating piece. Int. J. Therm. Sci. 2014, 77, 66–74. [Google Scholar] [CrossRef]
- Feng, H.; Chen, L.; Xie, Z.; Sun, F. Constructal design for “+” shaped high conductivity pathways over a square body. Int. J. Heat Mass Transf. 2015, 91, 162–169. [Google Scholar] [CrossRef]
- Mazloomi, A.; Sharifi, F.; Salimpour, M.R.; Moosavi, A. Optimization of highly conductive insert architecture for cooling a rectangular chip. Int. Commun. Heat Mass Transf. 2012, 39, 1265–1271. [Google Scholar] [CrossRef]
- Ji, Y.; Ding, X.; Li, H.; Xiong, M. Layout Design of Conductive Heat Channel by Emulating Natural Branch Systems. J. Bionic Eng. 2018, 15, 567–578. [Google Scholar] [CrossRef]
- Yi, P.; Liu, W.; Wang, N.; Tian, Y.; Chen, X. A novel wick structure of vapor chamber based on the fractal architecture of leaf vein. Int. J. Heat Mass Transf. 2013, 63, 120–133. [Google Scholar]
- Ding, X.; Yamazaki, K. Constructal Design of Cooling Channel in Heat Transfer System by Utilizing Optimality of Branch Systems in Nature. J. Heat Transf. 2007, 129, 245–255. [Google Scholar] [CrossRef]
- Hl, A.; Bl, A.; Lz, A.; Xin, L.B. Optimizing heat-absorption efficiency of phase change materials by mimicking leaf vein morphology. Appl. Energy 2020, 269, 114982. [Google Scholar]
- Li, B.; Hong, J.; Ge, L. Constructal design of internal cooling geometries in heat conduction system using the optimality of natural branching structures. Int. J. Therm. Sci. 2017, 115, 16–28. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Parsa, H.; Najafian, J. Proposing an optimal tree-like design of highly conductive material configuration with unequal branches for maximum cooling a heat generating piece. Int. J. Heat Mass Transf. 2019, 142, 118422. [Google Scholar] [CrossRef]
- Xu, P.; Yu, B.; Yun, M.; Zou, M. Heat conduction in fractal tree-like branched networks. Int. J. Heat Mass Transf. 2006, 49, 3746–3751. [Google Scholar] [CrossRef]
- Liu, F.; Jing, D. Optimization of heat conduction for treelike network with arbitrary cross-sectional shape. Fractals 2022, 30, 2150257. [Google Scholar] [CrossRef]
- Hajmohammadi, M.R.; Moulod, M.; Shariatzadeh, O.J.; Nourazar, S.S. Essential reformulations for optimization of highly conductive inserts embedded into a rectangular chip exposed to a uniform heat flux. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2014, 228, 2337–2346. [Google Scholar] [CrossRef]
- Almogbel, M.; Bejan, A. Constructal optimization of nonuniformly distributed tree-shaped flow structures for conduction. Int. J. Heat Mass Transf. 2001, 44, 4185–4194. [Google Scholar] [CrossRef]
- Rocha, L.; Lorente, S.; Bejan, A. Conduction tree networks with loops for cooling a heat generating volume. Int. J. Heat Mass Transf. 2006, 49, 2626–2635. [Google Scholar] [CrossRef]
- Rocha, L.; Lorente, S.; Bejan, A. Constructal design for cooling a disc-shaped area by conduction. Int. J. Heat Mass Transf. 2002, 45, 1643–1652. [Google Scholar] [CrossRef]
- Senn, S.M.; Poulikakos, D. Laminar mixing, heat transfer and pressure drop in tree-like microchannel nets and their application for thermal management in polymer electrolyte fuel cells. J. Power Sources 2004, 130, 178–191. [Google Scholar] [CrossRef]
| m = 3, H = 0.8 mm, V = 50 mm3 | |||||
|---|---|---|---|---|---|
| α | β | L0 (mm) | L1 (mm) | L2 (mm) | L3 (mm) |
| 0.65 | 0.2–0.6 | 9 | 5.85 | 3.80 | 2.47 |
| 0.70 | 9 | 6.30 | 4.41 | 3.09 | |
| 0.75 | 9 | 6.75 | 5.06 | 3.79 | |
| α = 0.65, H = 0.8 mm, V = 50 mm3 | ||||||
|---|---|---|---|---|---|---|
| m | β | L0 (mm) | L1 (mm) | L2 (mm) | L3 (mm) | L4 (mm) |
| 2 | 0.2–0.6 | 9 | 5.85 | 3.80 | / | / |
| 3 | 9 | 5.85 | 3.80 | 2.47 | / | |
| 4 | 9 | 5.85 | 3.80 | 2.47 | 1.61 | |
| α = 0.65, m = 3, H = 0.8 mm, V = 50 mm3 | ||||
|---|---|---|---|---|
| L0 (mm) | β | L1 (mm) | L2 (mm) | L3 (mm) |
| 8 | 0.2–1.2 | 5.20 | 3.38 | 2.19 |
| 9 | 5.85 | 3.80 | 2.47 | |
| 10 | 6.50 | 4.22 | 2.74 | |
| α = 0.65, m = 3, V = 50 mm3 | |||||
|---|---|---|---|---|---|
| H (mm) | β | L0 (mm) | L1 (mm) | L2 (mm) | L3 (mm) |
| 0.6 | 0.2–0.6 | 9 | 5.85 | 3.80 | 2.47 |
| 0.8 | |||||
| 1 | |||||
| α = 0.65, m = 3, H = 0.8 mm | |||||
|---|---|---|---|---|---|
| V (mm3) | β | L0 (mm) | L1 (mm) | L2 (mm) | L3 (mm) |
| 40 | 0.2–0.6 | 9 | 5.85 | 3.80 | 2.47 |
| 50 | |||||
| 60 | |||||
| Test Number | Mesh Number | Tmax [°C] | |
|---|---|---|---|
| 1 | 3809 | 55.7806 | |
| 2 | 10,351 | 56.0943 | 5.6238 × 10−3 |
| 3 | 16,959 | 56.1542 | 1.0678 × 10−3 |
| 4 | 25,931 | 56.2084 | 9.6520 × 10−4 |
| 5 | 45,639 | 56.2407 | 5.7465 × 10−4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, R.; Jing, D. Thermal Performance and Geometric Optimization of Fractal T-Shaped Highly-Conductive Material for Cooling of a Rectangular Chip. Fractal Fract. 2022, 6, 705. https://doi.org/10.3390/fractalfract6120705
Zhu R, Jing D. Thermal Performance and Geometric Optimization of Fractal T-Shaped Highly-Conductive Material for Cooling of a Rectangular Chip. Fractal and Fractional. 2022; 6(12):705. https://doi.org/10.3390/fractalfract6120705
Chicago/Turabian StyleZhu, Rongsheng, and Dalei Jing. 2022. "Thermal Performance and Geometric Optimization of Fractal T-Shaped Highly-Conductive Material for Cooling of a Rectangular Chip" Fractal and Fractional 6, no. 12: 705. https://doi.org/10.3390/fractalfract6120705
APA StyleZhu, R., & Jing, D. (2022). Thermal Performance and Geometric Optimization of Fractal T-Shaped Highly-Conductive Material for Cooling of a Rectangular Chip. Fractal and Fractional, 6(12), 705. https://doi.org/10.3390/fractalfract6120705





