An Entropy Paradox Free Fractional Diffusion Equation

Abstract

A new look at the fractional diffusion equation was done. Using the unified fractional derivative, a new formulation was proposed, and the equation was solved for three different order cases: neutral, dominant time, and dominant space. The solutions were expressed by generalizations of classic formulae used for the stable distributions. The entropy paradox problem was studied and clarified through the Rényi entropy: in the extreme wave regime the entropy is $-\infty$. In passing, Tsallis and Rényi entropies for stable distributions are introduced and exemplified.

1. Introduction

It is no use to refer to the importance of the diffusion equation [1,2,3], which probably one of the most studied in applied sciences. Its fractional versions have attracted the attention of many researchers due to its relation with the alpha stable processes and some new applications [4,5,6,7,8,9,10,11,12,13]. Although such an equation can in general assume different forms with the introduction of non-linearities and using ${\mathbb{R}}^n$ as working space, we considered only the simpler linear case and $n=1$, which is usually expressed as [14]

$${}_x{D}_{\theta }^{\alpha }u(x,t)={}_t{D}_{\ast }^{\beta }u(x,t),x\in \mathbb{R},t\in {\mathbb{R}}^{+}$$

(1)

where ${}_x{D}_{\theta }^{\alpha }$ is the Riesz–Feller space fractional derivative, ${}_t{D}_{\ast }^{\beta }$ is the (Dzherbashian)–Caputo derivative, and the $\alpha ,\beta ,\theta ,$ are real parameters always restricted as follows

$$0<\alpha \le 2,\left|\theta \right|\le min\{\alpha ,2-\alpha \},0<\beta \le 2$$

(2)

A general solution for Equation (1) was discovered by Mainardi et al. [14]. However, such a solution is expressed in terms of the Fox H-function [15,16,17,18,19] that, while attractive from analytical point of view, is very hard to manipulate for obtaining results. This led to separate consideration of the time-fractional case ($\alpha =2$) and the space-fractional case ($\beta =1$) [8,9,16,18,20,21,22].

However, there was a “cataclysm”: Hoffmann et al. [23] discovered that the entropy production rate associated with the diffusion processes had a non-expected variation. When transiting from the dissipative behavior ($\beta =1$) to the reversible wave propagation ($\beta =2$), a decrease in the entropy production was expected, but the reverse was observed. This phenomenon was treated in many studies, trying to find a suitable interpretation and understanding the possible underlying physical reasons [23,24,25,26,27,28].

Here, we reformulate the fractional diffusion equation using the unified fractional derivative [29]. We propose solutions for three regimes: $\beta =\alpha ,$ (neutral case), $\beta >\alpha ,$ (time dominant case), and $\beta <\alpha$ (space dominant case). The solutions here proposed are generalizations of previously known solutions. In particular, the traditional probability density functions of the stable distributions were found as special cases. Attending to the importance of the entropy in this study, we recovered the most important definitions, mainly the Shannon, Tsallis, and Rényi [17,30,31,32,33] definitions. The entropy production paradox was studied, using a Rényi entropy expression in the frequency domain, when possible. In particular, this happens with the stable distributions that are defined through the characteristic function. It is shown that, in such cases, the entropy is “always” $-\infty$, independently of the scale parameter. However, when considering that $\beta \to 2$, the approach to $-\infty$ is smooth. Therefore, there is no paradox.

The article outlines as follows. In Section 2, the unified fractional derivative is recast together with its main properties. The derivatives of power functions are also introduced. The diffusion equation in presented in Section 2.3. The entropy definitions and particularizations are presented in Section 3 and computed in Section 3.2. The diffusion equation is solved in Section 4, starting from the neutral case (Section 4.2) with corresponding entropy computation and continuing with the time dominant case (Section 4.3) and, at the end, the space dominant case (Section 4.4). Finally, a discussion and some conclusions are presented.

Remark 1.

We adopted here the following assumptions:

• We worked on $\mathbb{R}$.
• We used the two-sided Laplace transform (LT):

  $$F\left(s\right)=\mathcal{L}\left[f\left(t\right)\right]=\underset{\mathbb{R}}{\overset{}{\int }}f\left(t\right)e^{-st}\mathrm{d}t,$$

  (3)
  where $f\left(t\right)$ is any function defined on $\mathbb{R}$, and $F\left(s\right)$ is its transform, provided that it has a non-empty region of convergence.
• The Fourier transform (FT), $\mathcal{F}\left[f\left(t\right)\right]$, was obtained from the LT through the substitution $s=i\kappa ,$ with $\kappa \in \mathbb{R}.$
• For two variable functions, $f(x,t)$, we use a capital letter to represent the Fourier or Laplace transforms: $F(\kappa ,t)=\mathcal{F}\left[f(x,t)\right]$ and $F(x,s)=\mathcal{L}\left[f(x,t)\right]$.
• The 2-D transforms are represented by $\overline{F}(\kappa ,s)=\mathcal{L}\mathcal{F}\left[f(x,t)\right].$

2. Derivatives and Diffusion Equation

2.1. Definitions and Main Properties

In [29], a unified fractional derivative (UFD) incorporating most of the useful derivatives was presented and its properties studied. In the following, we describe it.

Definition 1.

Let $\alpha >-1$ if $\theta \ne \pm \alpha$, or $\alpha \in \mathbb{R}$ if $\theta =\pm \alpha .$ We defined a unified fractional derivative GL type derivative by:

$$D_{\theta }^{\alpha }f\left(t\right):=\underset{h\to 0^{+}}{lim}{h}^{-\alpha }\sum _{n=-\infty }^{+\infty }{(-1)}^n·\frac{\mathsf{\Gamma }\left(\alpha +1\right)}{\mathsf{\Gamma }\left(\frac{\alpha +\theta }{2}-n+1\right)\mathsf{\Gamma }\left(\frac{\alpha -\theta }{2}+n+1\right)}f(t-nh).$$

(4)

where α is the derivative order and θ the asymmetry parameter. Suitable choices of these parameters allow us to recover the causal and anti-causal derivatives. The particular, most interesting, cases are

• $\theta =\alpha$—forward GL derivative.
• $\theta =0$— Riesz derivative.
• $\theta =1$—Feller derivative.
• $\alpha =0$—two-sided GL type Hilbert transform.
  With $\theta =1$, we obtained the usual discrete-time formulation of the Hilbert transform [34].

Definition 2.

Let $\alpha >0.$ We defined a general integral formulation for the unified anti-derivative through

$$D_{\theta }^{-\alpha }f\left(t\right)=\frac{1}{sin\left(\alpha \pi \right)\mathsf{\Gamma }\left(\alpha \right)}{\int }_{\mathbb{R}}f(t-\tau )sin\left[\left(\alpha +\theta ·sgn\left(\tau \right)\right)\frac{\pi }{2}\right]{\left|\tau \right|}^{\alpha -1}\mathrm{d}\tau .$$

(5)

where $sgn(.)$ denotes the signum function.

As above, we obtained:

• $\theta =\alpha$—forward Liouville anti-derivative.
• $\theta =0$—Riesz potential.
• $\theta =1$—Feller potental.
• $\alpha =0$—Hilbert transform.
  With $\theta =1$, we obtained the usual formulation [35].

Remark 2.

The integral in (5) can be regularized in order to become valid for positive orders [36].

Some known properties of this derivative can be drawn [36,37,38]:

• Fourier transformation

  $$\mathcal{F}\left[D_{\theta }^{\alpha }f\left(t\right)\right]={\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}F\left(\kappa \right)$$

  (6)
  This property has, as consequence, that

  $$D_{\theta }^{\alpha }f\left(t\right)=cos\left(\theta \frac{\pi }{2}\right)D_0^{\alpha }f\left(t\right)+sin\left(\theta \frac{\pi }{2}\right)D_1^{\alpha }f\left(t\right)$$

  (7)
• Eigenfunctions
  Let $f\left(x\right)=e^{i\kappa x},\kappa ,x\in \mathbb{R}.$ Then

  $$D_{\theta }^{\beta }{e}^{i\kappa x}={\left|\kappa \right|}^{\beta }{e}^{i\frac{\pi }{2}\theta ·sgn\left(\kappa \right)}{e}^{i\kappa x},$$

  (8)

  meaning that the complex sinusoids are the eigenfunctions of the UFD with eigenvalue ${\mathsf{\Psi }}_{\theta }^{\beta }\left(\kappa \right)={\left|\kappa \right|}^{\beta }{e}^{i\frac{\pi }{2}\theta ·sgn\left(\kappa \right)}.$
• Periodicity in $\theta$
  The UFD is periodic in $\theta$ with period 4

  $$D_{\theta }^{\beta }f\left(x\right)={(-1)}^n{D}_{\theta +2n}^{\beta }f\left(x\right),n\in \mathbb{Z},$$

  as we observe from (6).
• Additivity and commutativity of the orders

  $$D_{{\theta }_1}^{{\beta }_1}{D}_{{\theta }_2}^{{\beta }_2}f\left(x\right)=D_{{\theta }_1+{\theta }_2}^{{\beta }_1+{\beta }_2}f\left(x\right).$$

  (9)
• Existence of inverse derivative
  We defined the identity operator

  $$D_0^{0}f\left(x\right)=f\left(x\right).$$

  (10)
  From this definition and (9), the anti-derivative exists when ${\beta }_2=-{\beta }_1$ and ${\theta }_1=-{\theta }_2$. Therefore,

  $$D_{\theta }^{\beta }{D}_{-\theta }^{-\beta }f\left(x\right)=D_{-\theta }^{-\beta }{D}_{\theta }^{\beta }f\left(x\right)=f\left(x\right).$$

  (11)

2.2. Derivatives of Power Functions

The power functions are very important in the theory we present due to the fact that the solutions of our problem are easily expressed in terms of power series. We considered three types of powers defined on $\mathbb{R}$:

• Causal − $t^a\epsilon \left(t\right),$
• Even − ${\left|t\right|}^a,$
• Odd − ${\left|t\right|}^{a}sgn\left(t\right)$

where $a\in \mathbb{R}$ and $\epsilon \left(t\right)$ is the Heaviside unit step. We can show that [36,37,38,39]:

• $$D_{\alpha }^{\alpha }\delta \left(t\right)=\mathcal{L}\left[s^{\alpha }\right]=\frac{t^{-\alpha -1}}{\mathsf{\Gamma }(-\alpha )}\epsilon \left(t\right)$$

  (12)
• $$D_0^{\alpha }\delta \left(x\right)={\mathcal{F}}^{-1}\left[{\left|\kappa \right|}^{\alpha }\right]=\left\{\begin{array}{cc}\begin{array}{cc}\hfill & \frac{1}{2\mathsf{\Gamma }(-\alpha )cos\left(\alpha \frac{\pi }{2}\right)}{\left|x\right|}^{-\alpha -1}=\hfill \\ & -\frac{\mathsf{\Gamma }(\alpha +1)sin(\alpha \pi /2)}{\pi }{\left|x\right|}^{-\alpha -1}\hfill \end{array}\hfill & \alpha \notin \mathbb{N}\hfill \\ -\frac{{(-1)}^N(2N+1)!}{\pi }{\left|x\right|}^{-2N-2}\hfill & \alpha =2N+1,\mathrm{odd}\mathrm{integer}\hfill \\ {(-1)}^N{\delta }^{\left(2N\right)}\left(x\right)\hfill & \alpha =2N,\mathrm{even}\mathrm{integer}\hfill \end{array}\right.,$$

  (13)
• $$D_1^{\alpha }\delta \left(x\right)={\mathcal{F}}^{-1}\left[{i\left|\kappa \right|}^{\alpha }sgn\left(\kappa \right)\right]=\left\{\begin{array}{cc}\begin{array}{cc}\hfill & -\frac{1}{2\mathsf{\Gamma }(-\alpha )sin\left(\alpha \frac{\pi }{2}\right)}{\left|x\right|}^{-\alpha -1}sgn\left(x\right)=\hfill \\ & \frac{\mathsf{\Gamma }(\alpha +1)cos(\alpha \pi /2)}{\pi }{\left|x\right|}^{-\alpha -1}sgn\left(x\right)\hfill \end{array}\hfill & \alpha \notin \mathbb{N}\hfill \\ -\frac{{(-1)}^N\left(2N\right)!}{\pi }{\left|x\right|}^{-2N-1}sgn\left(x\right)\hfill & \alpha =2N,\mathrm{even}\mathrm{integer}\hfill \\ {(-1)}^N{\delta }^{(2N+1)}\left(x\right)\hfill & \alpha =2N+1,\mathrm{odd}\mathrm{integer}.\hfill \end{array}\right.$$

  (14)

Attending to properties (12) to (14) and a suitable parameter change, we obtained derivatives of the power functions. We consider the regular cases (orders not equal to negative integers). The others were obtained from (13) and (14).

• $$D_{\alpha }^{\alpha }{t}^{\mu }\epsilon \left(t\right)=\frac{\mathsf{\Gamma }(\mu +1)}{\mathsf{\Gamma }(\mu -\alpha +1)}{t}^{\mu -\alpha }\epsilon \left(t\right)$$

  (15)
• $$D_0^{\beta }{\left|x\right|}^{\mu }=\frac{\mathsf{\Gamma }(\mu +1)sin(\mu \pi /2)}{\mathsf{\Gamma }(\mu -\beta )sin\left(\right(\mu -\beta )\pi /2)}{\left|x\right|}^{a-\beta }$$

  (16)
• $$D_0^{\beta }{\left|x\right|}^{\mu }sgn\left(x\right)=-\frac{\mathsf{\Gamma }(\mu +1)cos(\mu \pi /2)}{\mathsf{\Gamma }\left(\mu -\beta +1\right)cos((\beta -\mu )\pi /2)}{\left|x\right|}^{\mu -\beta }sgn\left(x\right)$$

  (17)
• $$D_1^{\beta }{\left|x\right|}^{\mu }=\frac{\mathsf{\Gamma }(\mu +1)sin(\mu \pi /2)}{\mathsf{\Gamma }\left(\mu -\beta +1\right)cos((\beta -\mu )\pi /2)}{\left|x\right|}^{a-\beta }sgn\left(x\right)$$

  (18)
• $$D_1^{\beta }{\left|x\right|}^{\mu }sgn\left(x\right)=-\frac{\mathsf{\Gamma }(\mu +1)cos(\mu \pi /2)}{\mathsf{\Gamma }\left(\mu -\beta +1\right)sin((\beta -\mu )\pi /2)}{\left|x\right|}^{a-\beta }$$

  (19)

2.3. Formulation of the Diffusion Equation

The above-defined UFD was used to introduce the linear diffusion equation.

Definition 3.

Let $0\le \alpha \le 2$, $0<\beta \le 2$, and $-2<\theta \le 2$, with $t,x\in \mathbb{R}$. We defined the fractional diffusion equation (also, generalized fractional kinetic equation) by [40]:

$${}_t{D}_{\beta }^{\beta }u(x,t)+C{}_x{D}_{\theta }^{\alpha }u(x,t)=v(x,t)$$

(20)

where $v(x,t)$ is the input, and $u(x,t)$ is the output. Only for simplicity, we consider the $C=1$ case.

Let $U(x,s)=\mathcal{L}\left[u(x,t)\right]$ be the Laplace transform of $u(x,t)$ relatively to t and $U\left(\kappa t\right)=\mathcal{F}\left[u(x,t)\right]$ the Fourier transform relatively to x. The 2-D Laplace–Fourier transform (LT-FT) of $u(x,t)$ is denoted by $\overline{U}(\kappa ,s)=\mathcal{L}\mathcal{F}\left[u(x,t)\right]$ [41,42]. Assume also that we want to compute the output for $t>0$ and that there exists an initial-condition (IC) $u(x,0)=v_0\left(x\right)$ with $V_0\left(\kappa \right)=\mathcal{F}{v}_0\left(x\right)$. Applying both transforms to (20) and attending to the IC (see, [41]) we get:

$$\overline{U}(\kappa ,s)=\frac{s^{\beta -1}}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}{V}_0\left(\kappa \right)+\frac{1}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}\overline{V}(\kappa ,s)$$

(21)

The LT-FT inverse of the first term on the right gives the free response, while the second originates the forced term (particular solution). The function

$$\overline{H}(\kappa ,s)=\frac{1}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}$$

is the transfer function of the system defined by (20). Its LT-FT inverse gives the 2-D green function (impulse response), which we denote by $h_{\theta }^{\alpha ,\beta }(x,t)$ and which, in the zero IC case, allows us to write

$$u(x,t)=h_{\theta }^{\alpha ,\beta }(x,t)\ast \ast v(x,t)$$

(22)

where $\ast \ast$ [42] denotes the 2-D convolution, and $v(x,t)$ is any input function. However, in agreement with (1), we shall be interested in the free therm only that, if $\beta >0$, it is given by the solution of

$${}_x{D}_{\theta }^{\alpha }u(x,t)+{}_t{D}_{\beta }^{\beta }u(x,t)=0$$

(23)

under a suitable IC. If one assumes that $u(x,0)=\delta \left(x\right)$ we obtain also an impulse response, $g_{\theta }^{\alpha ,\beta }(x,t)$ such that

$$u(x,t)=g_{\theta }^{\alpha ,\beta }(x,t)\ast v_0\left(x\right)$$

(24)

that allows to obtain the free therm corresponding to any IC. The function $g_{\theta }^{\alpha ,\beta }(x,t)$ is given by

$$g_{\theta }^{\alpha ,\beta }(x,t)={\mathcal{F}}^{-1}{\mathcal{L}}^{-1}\left[\frac{s^{\beta -1}}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}\right]$$

(25)

or, from (21)

$$G_{\theta }^{\alpha ,\beta }(\kappa ,t)={\mathcal{L}}^{-1}\frac{s^{\beta -1}}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}$$

(26)

As it is well known, from the properties of the Mittag–Leffler function [43],

$$G_{\theta }^{\alpha ,\beta }(\kappa ,t)=\sum _{n=0}^{\infty }{(-1)}^n{\left|\kappa \right|}^{\alpha n}{e}^{in\theta \frac{\pi }{2}sgn\left(\kappa \right)}\frac{t^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}t>0.$$

(27)

The FT invertion of $G_{\theta }^{\alpha ,\beta }(\kappa ,t)$ creates several difficulties that we face later. In the following, we are concerned with the computation of the entropy associated to $g(x,t)=g_{\theta }^{\alpha ,\beta }(x,t)$ (we do not omit the scripts, unless necessary).

Remark 3.

Note that, on assuming that α can be zero, we are including an unsolved case. The $\beta =0$ case corresponds to an eigenvalue problem that is not interesting here.

3. A New Look at Entropy Computations

3.1. Main Entropies

As known, there are several definitions of entropy [30], even fractional entropy [32,33]. However, only a few are suitable for our objectives. Let $P(x,t),x\in \mathbb{R},t\in {\mathbb{R}}^{+}$ be the probability density function and q a real parameter. The most important entropy definitions are

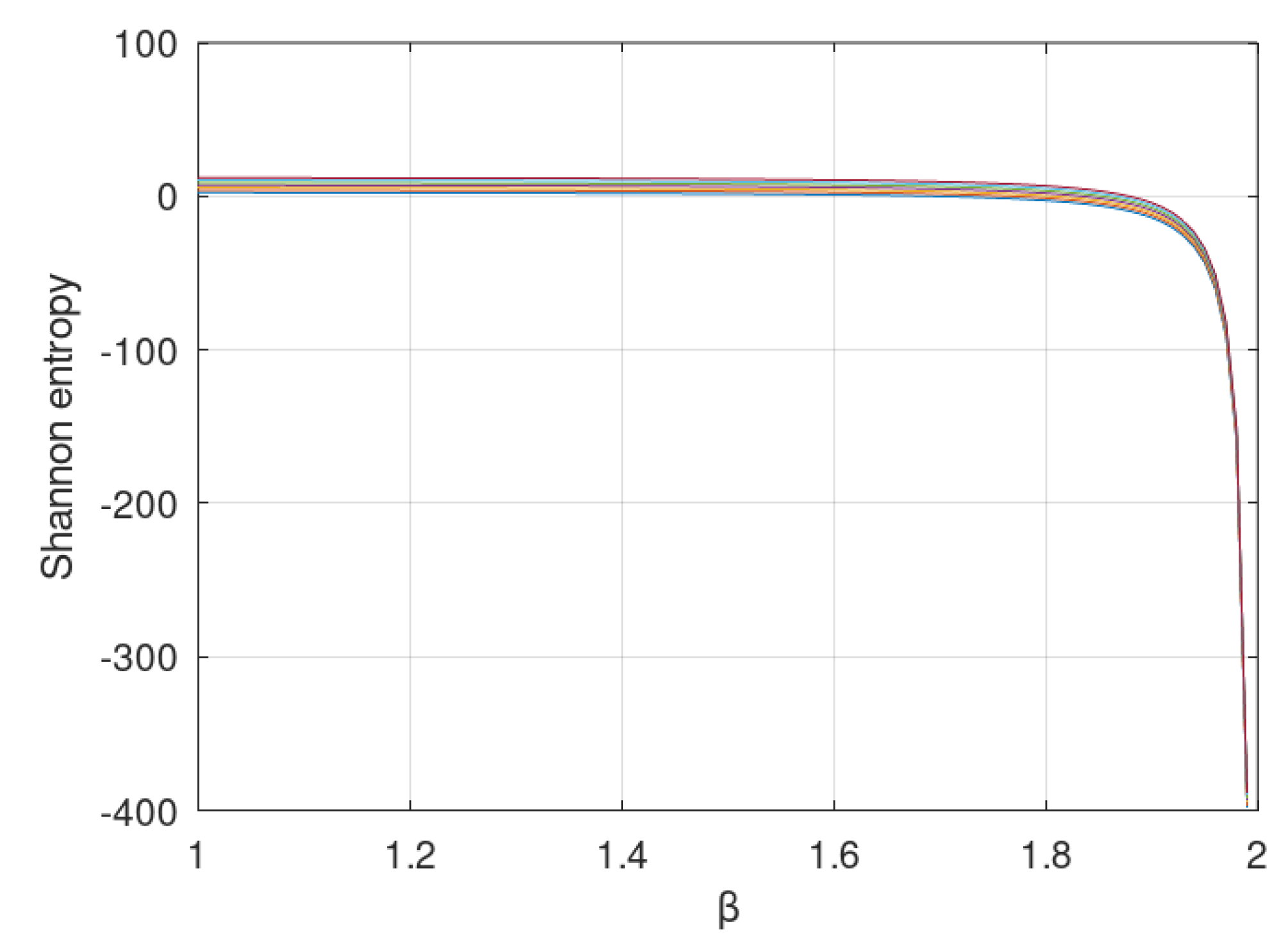
• Shannon’s

  $$S_1\equiv {\int }_{\mathbb{R}}P(x,t)lnP(x,t)\mathrm{d}x$$

  (28)
• Tsallis’

  $$T_q\equiv -\frac{1}{1-q}{\int }_{\mathbb{R}}P(x,t)\left(1-P^{q-1}(x,t)\right)\mathrm{d}x$$

  (29)
  We particularize for $q=2$ giving

  $$T_2={\int }_{\mathbb{R}}P(x,t)\left(1-P(x,t)\right)\mathrm{d}x={\int }_{\mathbb{R}}P(x,t)-P^2(x,t)\mathrm{d}x=1-{\int }_{\mathbb{R}}{P}^2(x,t)\mathrm{d}x$$

  (30)

  where we used the result ${\int }_{\mathbb{R}}P(x,t)\mathrm{d}x=1.$
• Rényi’s

  $$R_q\equiv \frac{1}{1-q}ln\left({\int }_{\mathbb{R}}{P}^q(x,t)\mathrm{d}x\right)$$

  (31)
  Similarly, for $q=2$, we get

  $$R_2=-ln\left({\int }_{\mathbb{R}}{P}^2(x,t)\mathrm{d}x\right)$$

  (32)

Remark 4.

Frequently, the entropies use the base-2 logarithm. For this study, the base was not important. Therefore, we used the one that gives simpler results.

Lemma 1.

Let $f\left(x\right),x\in \mathbb{R}$ be a square-integrable real function with FT, $F\left(\kappa \right)$. The Parseval relation states that [35]

$${\int }_{\mathbb{R}}{f}^2\left(x\right)\mathrm{d}x=\frac{1}{2\pi }{\int }_{\mathbb{R}}F\left(\kappa \right)F(-\kappa )\mathrm{d}\kappa =\frac{1}{2\pi }{\int }_{\mathbb{R}}{\left|F\left(\kappa \right)\right|}^2\mathrm{d}\kappa$$

(33)

since $F^{\ast }\left(\kappa \right)=F(-\kappa ).$

Consequently, we can compute $T_2$ and $R_2$ in the frequency domain respectively by

$$T_2=1-\frac{1}{2\pi }{\int }_{\mathbb{R}}F\left(\kappa \right)F(-\kappa )\mathrm{d}\kappa$$

(34)

and

$$R_2=ln\left(2\pi \right)-ln\left({\int }_{\mathbb{R}}F\left(\kappa \right)F(-\kappa )\mathrm{d}\kappa \right)$$

(35)

Therefore, we only need to compute the “energy” ${\int }_{\mathbb{R}}F\left(\kappa \right)F(-\kappa )\mathrm{d}\kappa .$ Due to the similarity of both $T_2$ and $R_2$ we used only one. We adopted $R_2$ for its resemblance with the Shannon entropy. For application, we set $f\left(x\right)=P(x,t)$.

3.2. The Entropy of Some Special Distributions

3.2.1. The Gaussian

Consider the Gaussian distribution in the form

$$P_G(x,t)=\frac{1}{\sqrt{4\pi t}}{e}^{-\frac{x^2}{4t}}.$$

(36)

where $2t>0$ is the variance. Its Fourier transform is

$$\mathcal{F}{P}_G(x,t)=e^{-t{\kappa }^2}$$

(37)

We took into account the notation used in the expression (27), where we set $\alpha =2$, $\beta =1,$ and $\theta =0.$ The Shannon entropy of a Gaussian distribution is obtained without great difficulty [31]. The Rényi entropy (32) reads

$$R_2=-ln\left(\frac{1}{4\pi t}{\int }_{\mathbb{R}}{e}^{-\frac{x^2}{2t}}\mathrm{d}x\right)=\frac{1}{2}ln\left(8\pi t\right)$$

(38)

which is a very interesting result: the Rényi entropy $R_2$ of the Gaussian distribution depends on the logarithm of the variance. A similar result was obtained with the Shannon entropy [31].

3.2.2. The Extreme Fractional Space

Consider the distribution resulting from (26) with $\beta =2$, $\alpha <2$ and $\theta =0$. It is immediate to see that

$$G_{\theta }^{\alpha ,\beta }(\kappa ,t)={\mathcal{L}}^{-1}\frac{s}{s^2+{\left|\kappa \right|}^{\alpha }}=cos\left({\left|\kappa \right|}^{\alpha /2}t\right)$$

Therefore, the corresponding Rényi entropy is

$$R_2=ln\left(2\pi \right)-ln\left({\int }_{\mathbb{R}}{cos}^2\left({\left|\kappa \right|}^{\alpha /2}t\right)\mathrm{d}\kappa \right)=-\infty$$

(39)

independently of the value of $\alpha \in \left[0,2\right)$. This result suggests that, when approaching the wave limit, $\beta =2$, the entropy decreases without a lower bound.

3.2.3. The Stable Distributions

The above result led us to go ahead and consider again (27), with $\alpha <2,\beta =1$—usually denoted by fractional space. We have

$$G_{\theta }^{\alpha ,1}(\kappa ,t)=\sum _{n=0}^{\infty }{(-1)}^n{\left|\kappa \right|}^{\alpha n}{e}^{in\theta \frac{\pi }{2}sgn\left(\kappa \right)}\frac{t^n}{n!}=e^{-{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}t},$$

(40)

that corresponds to a stable distribution, although not expressed in one of the standard forms [13,44]. We have

$$R_2=ln\left(2\pi \right)-ln\left({\int }_{\mathbb{R}}{e}^{-{2\left|\kappa \right|}^{\alpha }cos\left(\theta \frac{\pi }{2}\right)t}\mathrm{d}\kappa \right)$$

The existence of the integral requires that

$$\left|\theta \right|<1.$$

Under this condition we can compute the integral

$${\int }_{\mathbb{R}}{e}^{-{2\left|\kappa \right|}^{\alpha }cos\theta \frac{\pi }{2}t}\mathrm{d}\kappa =2{\int }_0^{\infty }{e}^{-2{\kappa }^{\alpha }cos\theta \frac{\pi }{2}t}\mathrm{d}\kappa =2\mathsf{\Gamma }(1+1/\alpha ){\left(2t(cos\theta \frac{\pi }{2})\right)}^{-1/\alpha }.$$

Therefore,

$$R_2=ln\left(\pi \right)-ln\left[\mathsf{\Gamma }(1+1/\alpha )\right]+\frac{1}{\alpha }ln\left[2tcos\left(\theta \frac{\pi }{2}\right)\right]$$

(41)

Let $\theta =0$ and $\alpha =2,$ $\mathsf{\Gamma }(1+1/\alpha )=\frac{\sqrt{\pi }}{2}.$ We obtained (38). These results show that the symmetric stable distributions behave similarly to the Gaussian distribution when referring to the variation in t as shown in Figure 1.

It is important to note that for t above some threshold, the entropy for $\alpha <2$ is greater than the entropy of the Gaussian (see Figure 2). This must be contrasted with the well-known property: the Gaussian distribution has the largest entropy among the fixed variance distributions [31]. This fact may have been expected, since the stable distributions have infinite variance. Therefore, it must be important to see how the entropy changes with $\alpha$. It evolutes as illustrated in Figure 3 and shows again that for t above a threshold, the Gaussian distribution has lower entropy than the stable distributions. For $t\to 0$, the entropy decreases without bound (41).

It is important to remark that a $\theta \ne 0$ introduces a negative parcel in (41). Therefore, for the same α and β, the symmetric distributions have greater entropy than the asymmetric.

3.2.4. The Generalised Distributions

The results we obtained led us to consider (27) again but with $0\le \alpha <2,0<\beta \le 2$—usually denoted by fractional time-space. We have

$$G_{\theta }^{\alpha ,\beta }(\kappa ,t)=\sum _{n=0}^{\infty }{(-1)}^n{\left|\kappa \right|}^{\alpha n}{e}^{in\theta \frac{\pi }{2}sgn\left(\kappa \right)}\frac{t^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}$$

(42)

Remark 5.

We do not guarantee that the Fourier inverse $g(x,t)={\mathcal{F}}^{-1}{G}_{\theta }^{\alpha ,\beta }(\kappa ,t)$ as function of x is positive so that it can be considered as a probability density function. Therefore, we assume that for the parameters at hand, $g(x,t)$ is really positive.

The computation of the entropy in this case is not so simple and complete as in the previous case. However, some conclusions can be drawn. As $G_{\theta }^{\alpha ,\beta }(-\kappa ,t)=G_{-\theta }^{\alpha ,\beta }(\kappa ,t),$ we can write

$$\begin{array}{cc}\hfill R_2& =ln\left(2\pi \right)-ln\left({\int }_{\mathbb{R}}{G}_{\theta }^{\alpha ,\beta }(\kappa ,t)G_{-\theta }^{\alpha ,\beta }(\kappa ,t)\mathrm{d}\kappa \right)\hfill \\ & =ln\left(2\pi \right)-ln\left(2{\int }_0^{\infty }{G}_{\theta }^{\alpha ,\beta }(\kappa ,t)G_{-\theta }^{\alpha ,\beta }(\kappa ,t)\mathrm{d}\kappa \right)\hfill \\ & =ln\left(\pi \right)-ln\left({\int }_0^{\infty }{G}_{\theta }^{\alpha ,\beta }(\kappa ,t)G_{-\theta }^{\alpha ,\beta }(\kappa ,t)\mathrm{d}\kappa \right)\hfill \end{array}$$

It is a simple matter to show that

$$G_{\theta }^{\alpha ,\beta }(\kappa ,t)G_{-\theta }^{\alpha ,\beta }(\kappa ,t)=\sum _{n=0}^{\infty }{a}_n{e}^{-i\theta n\frac{\pi }{2}}{\left|\kappa \right|}^{\alpha n}\frac{t^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}$$

where

$$a_n=\sum _{k=0}^n\left(\genfrac{}{}{0pt}{}{\beta n}{\beta k}\right)e^{i\theta k\frac{\pi }{2}}$$

Therefore,

$$\begin{array}{cc}\hfill R_2& =ln\left(\pi \right)-ln\left({\int }_0^{\infty }\left[\sum _{n=0}^{\infty }{a}_n{e}^{-i\theta n\frac{\pi }{2}}{k}^{\alpha n}\frac{t^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}\right]\mathrm{d}\kappa \right)\hfill \\ & =ln\left(\pi \right)-ln\left({\int }_0^{\infty }\left[\sum _{n=0}^{\infty }{a}_n{e}^{-i\theta n\frac{\pi }{2}}\frac{v^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}\right]v^{\beta /\alpha -1}\mathrm{d}v\right)-ln\left(\frac{\beta }{\alpha t^{\frac{\beta }{\alpha }}}\right)\hfill \end{array}$$

and

$$R_2=ln\left(\pi \right)-ln\left(\frac{\beta }{\alpha }{R}_0(\alpha ,\beta )\right)+\frac{\beta }{\alpha }ln\left(t\right)$$

(43)

where $R_0(\alpha ,\beta )={\int }_0^{\infty }\left[{\sum }_{n=0}^{\infty }{a}_n{e}^{-i\theta n\frac{\pi }{2}}\frac{v^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}\right]v^{\beta /\alpha -1}\mathrm{d}v.$ The third term on the right points to an effect of increasing the entropy with the increment of $\beta$.

The results presented above suggest a smooth monotone increase in the entropy for $t>0,$ since the entropy production

$$\frac{d{R}_2}{dt}=\frac{\beta }{\alpha t}$$

(44)

is always positive. It is important to compute the entropy variation with the orders. Concerning $\alpha$, this is not very difficult. It gives

$$\frac{\partial R_2}{\partial \alpha }=\frac{1}{\alpha }-A\frac{\beta }{{\alpha }^2}-ln\left(t\right)\frac{\beta }{{\alpha }^2}$$

(45)

with $A={\int }_0^{\infty }\left[{\sum }_{n=0}^{\infty }{a}_n{e}^{-i\theta n\frac{\pi }{2}}\frac{v^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}\right]v^{\beta /\alpha -1}ln\left(v\right)\mathrm{d}v.$ The derivative relatively to $\beta$ does not give a so-simple expression, which is the reason why we did not compute it. The A integral plays a very important role, but it is not easy to obtain its value. Later, we compute it for the neutral case ($\alpha =\beta$).

4. Equation Solutions

4.1. Some Preliminary Results

Let us introduce the representation [14,36]

$${\mathsf{\Psi }}_{\theta }^{\alpha }={\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}.$$

(46)

Assuming that ${\mathsf{\Psi }}_{\theta }^{\alpha }$ is constant, we can interpret (21) as the LT of a Mittag–Leffler function, as we did above (42). Therefore, we can write:

$$G(\kappa ,t)=\sum _{n=0}^{\infty }{(-1)}^n{\mathsf{\Psi }}_{\theta n}^{\alpha n}\frac{t^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}$$

(47)

As a Fourier transform, this function has to verify

$$\underset{\left|\kappa \right|\to \infty }{lim}\left|G(\kappa ,t)\right|=0.$$

(48)

Let $z=-{\mathsf{\Psi }}_{\theta }^{\alpha }{t}^{\beta }$. Attending to the properties of the Mittag–Leffler function [43,45], we must have

$$Re\left(z^{\frac{1}{\beta }}\right)<0.$$

Since,

$$z=e^{i\pi }{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}{t}^{\beta }$$

So,

$$z^{\frac{1}{\beta }}=e^{i\frac{\pi }{\beta }}{\left|\kappa \right|}^{\frac{\alpha }{\beta }}{e}^{i\frac{\theta }{\beta }\frac{\pi }{2}sgn\left(\kappa \right)}t.$$

Therefore, we must have

$$cos\left[\left(\frac{\pm \theta +2}{\beta }\right)\frac{\pi }{2}\right]<0$$

which implies that

$$\beta -2<\theta <2-\beta$$

(49)

If $\beta >1,$ we can extend the above relation to include the extrema of the interval, since ${lim}_{\left|\kappa \right|\to \infty }\left|G(\kappa ,t)\right|=0$ already, but more slowly, as the decrease is no longer exponential. Therefore, we assumed $\left|\theta \right|\le 2-\beta$.

4.2. The Neutral Case ($\alpha =\beta$)

4.2.1. $\alpha =\beta <2$

We started the solution of the diffusion equation by considering the $\alpha =\beta$ case, which can be treated easily from (47). It can be stated as:

Theorem 1.

Let $\beta =\alpha >0$ derivative orders define the differential Equation (23). The solution of the initial value problem stated by the LT-FT $\overline{G}(\kappa ,s),$ with $\left|\theta \right|\le 2-\beta$, is given by

$$\begin{array}{cc}\hfill g_{\theta }^{\beta }(x,t)& =\frac{1}{\pi \left|x\right|}{\displaystyle \frac{\frac{t^{\beta }}{{\left|x\right|}^{\beta }}sin\left((\beta +\theta )\frac{\pi }{2}\right)}{1+2\frac{t^{\beta }}{{\left|x\right|}^{\beta }}cos\left((\beta +\theta )\frac{\pi }{2}\right)+\frac{t^{2\beta }}{{\left|x\right|}^{2\beta }}}}\hfill \\ \hfill & =\frac{1}{\pi \left|x\right|}{\displaystyle \frac{\frac{{\left|x\right|}^{\beta }}{t^{\beta }}sin\left((\beta +\theta )\frac{\pi }{2}\right)}{1+2\frac{{\left|x\right|}^{\beta }}{t^{\beta }}cos\left((\beta +\theta )\frac{\pi }{2}\right)+\frac{{\left|x\right|}^{2\beta }}{t^{2\beta }}}}\hfill \end{array}$$

(50)

Proof. 

This theorem has been demonstrated earlier [14,46], using the formulation in terms of a Mellin–Barnes integral. Here, we present a proof that arrives directly from the LT of the Mittag–Leffler function.

Consider the relation (47). We intend to compute its inverse FT. For starting, let us reverse the roles of the variables t and $\kappa$

$$G(\kappa ,t)=\sum _{n=0}^{\infty }{(-1)}^n{t}^{\beta n}\frac{{\left|\kappa \right|}^{\beta n}{e}^{i\theta n\frac{\pi }{2}sgn\left(\kappa \right)}}{\mathsf{\Gamma }(\beta n+1)}$$

(51)

Besides, note that

$${\left|\kappa \right|}^{\beta n}{e}^{i\theta n\frac{\pi }{2}sgn\left(\kappa \right)}=\left\{\begin{array}{cc}{\kappa }^{\beta n}{e}^{i\theta n\frac{\pi }{2}}\hfill & \kappa >0\hfill \\ {(-\kappa )}^{\beta n}{e}^{-i\theta n\frac{\pi }{2}}\hfill & \kappa <0\hfill \end{array}\right.$$

and

$$\begin{array}{cc}\hfill g(x,t)=\frac{1}{2\pi }{\int }_{\mathbb{R}}\sum _{n=0}^{\infty }{(-1)}^n{t}^{\beta n}\frac{{\left|\kappa \right|}^{\beta n}{e}^{i\theta n\frac{\pi }{2}sgn\left(\kappa \right)}}{\mathsf{\Gamma }(\beta n+1)}{e}^{i\kappa x}\mathrm{d}\kappa & =\frac{1}{2\pi }{\int }_0^{\infty }\sum _{n=0}^{\infty }{(-1)}^n{e}^{i\theta n\frac{\pi }{2}}{t}^{\beta n}\frac{{\kappa }^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}{e}^{i\kappa x}\mathrm{d}\kappa +\hfill \\ & \frac{1}{2\pi }{\int }_0^{\infty }\sum _{n=0}^{\infty }{(-1)}^n{e}^{-i\theta n\frac{\pi }{2}}{t}^{\beta n}\frac{{\kappa }^{\beta n}}{\mathsf{\Gamma }(\beta n+1)}{e}^{-i\kappa x}\mathrm{d}\kappa \hfill \end{array}$$

Using a well-known property of the Mittag–Leffler function [43], we obtain

$$\begin{array}{cc}\hfill g(x,t)& =\frac{1}{2\pi }{\displaystyle \frac{{(-ix)}^{\beta -1}}{{(-ix)}^{\beta }+e^{i\theta \frac{\pi }{2}}{t}^{\beta }}}+\frac{1}{2\pi }{\displaystyle \frac{{\left(ix\right)}^{\beta -1}}{{\left(ix\right)}^{\beta }+e^{-i\theta \frac{\pi }{2}}{t}^{\beta }}}\hfill \\ & =\frac{1}{\pi }{\displaystyle \frac{{\left|x\right|}^{\beta -1}{t}^{\beta }sin\left((\beta +\theta )\frac{\pi }{2}\right)}{{\left|x\right|}^{2\alpha }+2{\left|x\right|}^{\beta }{t}^{\beta }cos\left((\beta +\theta )\frac{\pi }{2}\right)+t^{2\beta }}}\hfill \end{array}$$

that proves the theorem. This theorem is in agreement with similar result obtained in [40] when solving the generalized fractional kinetic equation. □

For the particular $\theta =0$ case, we get

$$g_0^{\beta }(x,t)=\frac{1}{\pi \left|x\right|}{\displaystyle \frac{\frac{{\left|x\right|}^{\beta }}{t^{\beta }}sin\left(\beta \frac{\pi }{2}\right)}{1+2\frac{{\left|x\right|}^{\beta }}{t^{\beta }}cos\left(\beta \frac{\pi }{2}\right)+\frac{{\left|x\right|}^{2\beta }}{t^{2\beta }}}}$$

(52)

With $\beta =1$, we obtain

$$g(x,t)=\frac{1}{\pi }{\displaystyle \frac{t}{{\left|x\right|}^2+t^2}}$$

(53)

that is the well-known Cauchy kernel.

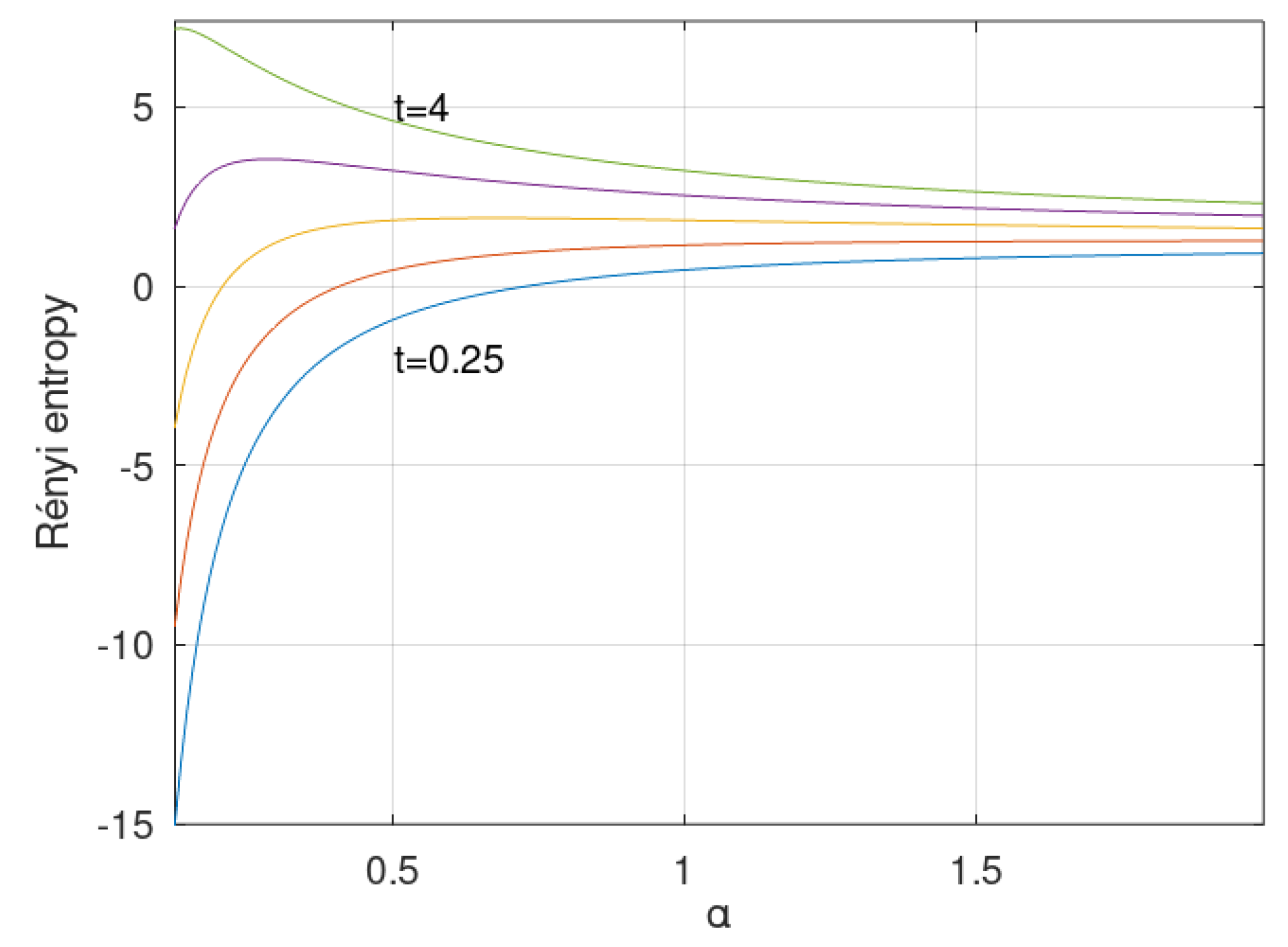
4.2.2. The Entropy of the $\alpha =\beta <2$ Case

For simplicity, let us set

$$q\left(v\right)={\displaystyle \frac{vsin\left((\beta +\theta )\frac{\pi }{2}\right)}{v^2+2vcos\left((\beta +\theta )\frac{\pi }{2}\right)+1}}$$

Now, we are going to return back to (52) and compute the corresponding Rényi entropy:

$$R_2=-ln\left({\int }_{\mathbb{R}}\frac{1}{{\pi }^2{\left|x\right|}^2}{q}^2{\left(\right(\left|x\right|/t)}^{\beta })\mathrm{d}x\right)$$

(54)

With a variable change, $v={\left(\right|x|/t)}^{\beta },$ we obtain:

$$\left({\int }_{\mathbb{R}}\frac{1}{{\pi }^2{\left|x\right|}^2}{q}^2{\left(\right(\left|x\right|/t)}^{\beta })\mathrm{d}x\right)=\frac{2{sin}^2\left((\beta +\theta )\frac{\pi }{2}\right)}{{\pi }^2\beta t}{\int }_0^{\infty }\frac{v^{1-1/\beta }}{{\left(v^2+2vcos\left((\beta +\theta )\frac{\pi }{2}\right)+1\right)}^2}\mathrm{d}x$$

The integral $A\left(\beta \right)={\int }_0^{\infty }\frac{v^{1-1/\beta }}{{\left(v^2+2vcos\left((\beta +\theta )\frac{\pi }{2}\right)+1\right)}^2}\mathrm{d}v$ has different behaviour for $\beta$ less or greater than 1. For the most interesting case, $\beta \ge 1,$ we can use an integration in the complex plane with the help of the residue theorem. We obtain

$$A\left(\beta \right)={\int }_0^{\infty }\frac{v^{1-1/\beta }}{{\left(v^2+2vcos\left((\beta +\theta )\frac{\pi }{2}\right)+1\right)}^2}\mathrm{d}v=-\pi {\displaystyle \frac{cos\left((\beta +\theta )\frac{\pi }{2}\right)}{{sin}^3\left((\beta +\theta )\frac{\pi }{2}\right)sin\left(\frac{\pi }{\beta }\right)}}$$

and then

$$R_2=-ln\left(-{\displaystyle \frac{2cos\left((\beta +\theta )\frac{\pi }{2}\right)}{\pi sin\left((\beta +\theta )\frac{\pi }{2}\right)sin\left(\frac{\pi }{\beta }\right)\beta t}}\right)=-ln\left({\displaystyle \frac{-2cot\left((\beta +\theta )\frac{\pi }{2}\right)}{\pi sin\left(\frac{\pi }{\beta }\right)\beta t}}\right)$$

(55)

so that

$$R_2=-ln\left({\displaystyle \frac{-2cot\left((\beta +\theta )\frac{\pi }{2}\right)}{\pi sin\left(\frac{\pi }{\beta }\right)\beta }}\right)+ln\left(t\right)$$

(56)

For $\beta \ge 1,$ the Rényi entropy increases with $ln\left(t\right)$, which implies that the corresponding entropy production is independent of the derivative order:

$$\frac{d{R}_2}{dt}=\frac{1}{t}$$

In particular, it is important to study the behavior of $R_2$ when $\beta \to 1,$ and when $\theta =0$. We obtained a $0/0$ indeterminacy, which raised gives the value $ln\left(\pi \right)+ln\left(t\right)$.

As we have the expression of the neutral distribution given by (52), we can try to compute the corresponding Shannon entropy. Without loosing generality, having in mind expression (50), we can assume $\theta =0$. Therefore, we can write

$$S_1=-\left(\frac{2}{\pi }{\int }_0^{\infty }\left[\frac{1}{x}q\left({(x/t)}^{\beta }\right)\right]ln\left[\frac{1}{\pi x}q\left({(x/t)}^{\beta }\right)\right]\mathrm{d}x\right)=-\left(\frac{2}{\pi \beta }{\int }_0^{\infty }q\left(v\right)ln\left[\frac{1}{\pi t{v}^{1/\beta }}q\left(v\right)\right]\frac{1}{v}\mathrm{d}v\right)$$

(57)

that can be written as

$$S_1=-\frac{2}{\pi \beta }{\int }_0^{\infty }q\left(v\right)ln\left[q\left(v\right)\right]\frac{1}{v}\mathrm{d}v+\frac{2ln\left(\pi t\right)}{\pi \beta }{\int }_0^{\infty }q\left(v\right)\frac{1}{v}\mathrm{d}v+\frac{2}{\pi {\beta }^2}{\int }_0^{\infty }q\left(v\right)\frac{ln\left(v\right)}{v}\mathrm{d}v.$$

(58)

We can show that

$${\int }_0^{\infty }q\left(v\right)\frac{1}{v}\mathrm{d}v=\beta \frac{\pi }{2}$$

and

$${\int }_0^{\infty }q\left(v\right)\frac{ln\left(v\right)}{v}\mathrm{d}v=0$$

Then

$$S_1=ln\left(\pi t\right)-\frac{2}{\pi \beta }{\int }_0^{\infty }q\left(v\right)ln\left[q\left(v\right)\right]\frac{1}{v}\mathrm{d}v$$

(59)

which shows that the dependence of $S_1$ on $\beta$ is rather complicated, but the entropy production is simple and given by:

$$\frac{d{S}_1}{dt}=\frac{1}{t}$$

that decreases with $t,$ but is independent of $\beta$. This result gave rise to the entropy production paradox.

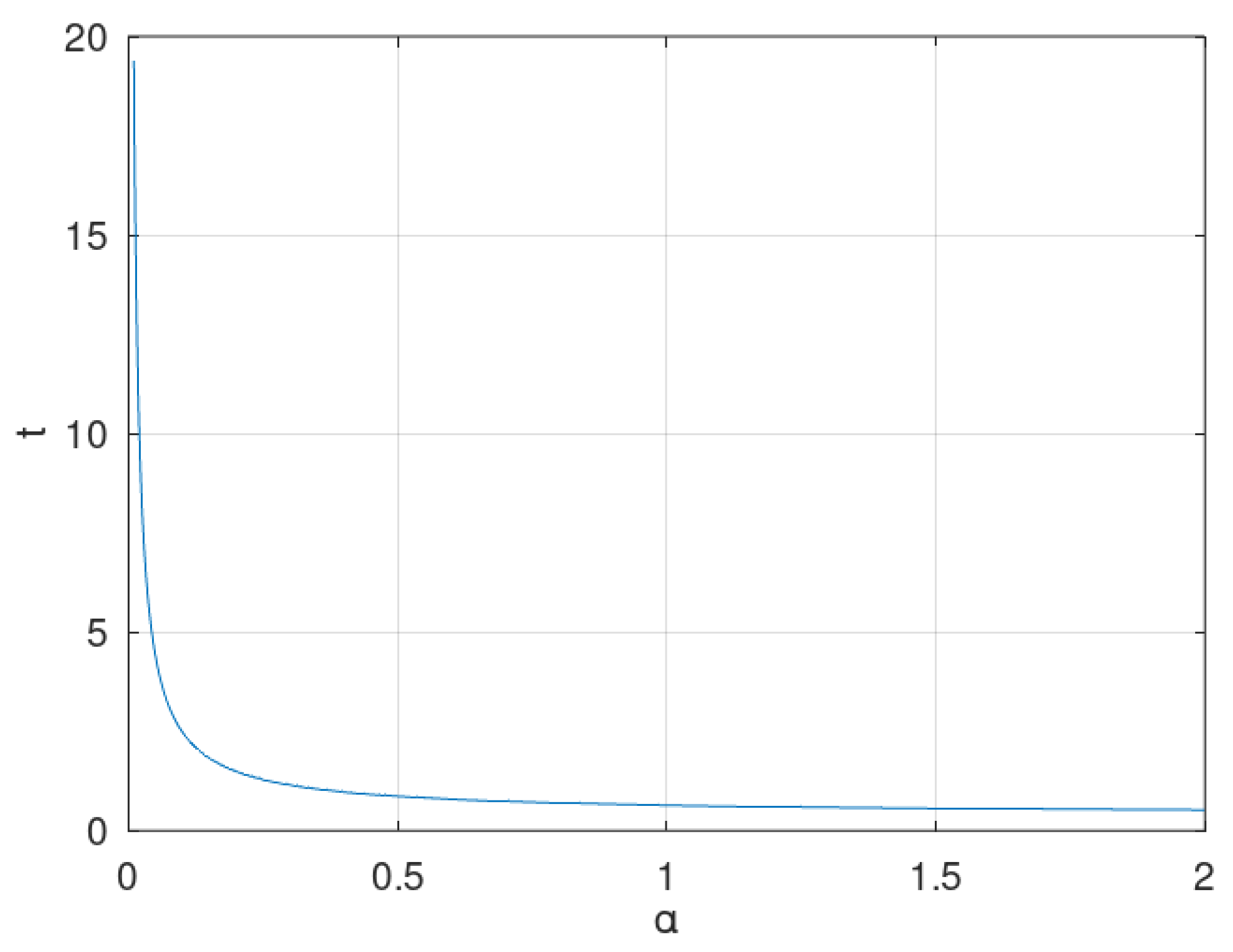
4.2.3. The $\alpha =\beta =2$ Case: There Is No Paradox

The $\alpha =\beta =2,\theta =0$ case corresponds to a singular situation, since [46]

$$\underset{\beta \to 2}{lim}g(x,t)=\frac{1}{2}\left[\delta (x+t)+\delta (x-t)\right],$$

the wave regime. The form of $g(x,t)$, a generalized function, prevents a direct calculation of entropy. Therefore, we can define the Rényi entropy corresponding to this case as a limit when $\beta \to 2$. Then,

$$R_2=\underset{\beta \to 2}{lim}-ln\left({\displaystyle \frac{-2cot\left(\beta \frac{\pi }{2}\right)}{\pi sin\left(\frac{\pi }{\beta }\right)\beta }}\right)+ln\left(t\right)$$

(60)

The Rényi entropy depends directly on $ln\left(t\right)$ implying that the entropy production is independent of the derivative orders. However,

$$R_2=\underset{\beta \to 2}{lim}-ln\left({\displaystyle \frac{-2cot\left(\beta \frac{\pi }{2}\right)}{\pi sin\left(\frac{\pi }{\beta }\right)\beta }}\right)=-\infty$$

(61)

independently of t. Therefore, when the order β approaches 2, the Rényi entropy decreases “smoothly” to $-\infty .$ This is illustrated in Figure 4, which suggests the presence of the generalized function $\delta (\beta -2)$ [46].

Concerning the Shannon entropy, let us return to relation (59). We were unable to compute the integral analytically. In Figure 5, we illustrate the numerically computed entropy. As seen, it suggests that the Shannon entropy goes also to $-\infty$ as the order approaches 2.

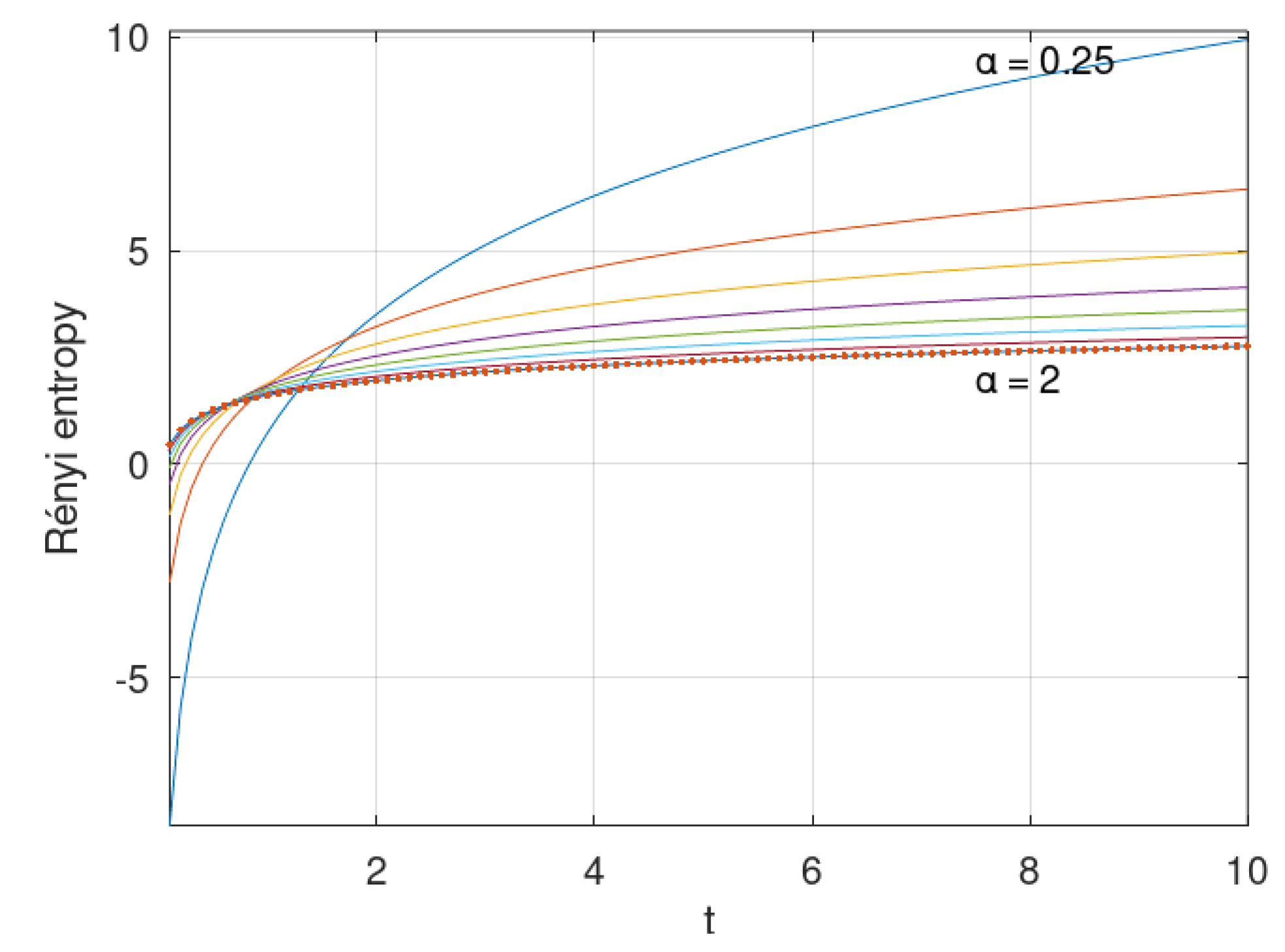
4.3. Time-Dominant Case ($\alpha <\beta$)

We are going ahead to the computation of the inverse FT of $G(\kappa ,t)$ for $\alpha <\beta$. Here, we may have some difficulties in the calculation of ${\mathcal{F}}^{-1}{\mathsf{\Psi }}_{\theta n}^{\alpha n}$ when $\alpha n$ is a positive integer, since Dirac impulses and derivatives will appear in agreement with (13) and (14). However, this may not be considered as a great problem, since they affect only the value at $x=0$ and state only a coherence with initial condition.

As

$${\mathsf{\Psi }}_{\theta n}^{\alpha n}={\left|\kappa \right|}^{\alpha n}{e}^{in\theta \frac{\pi }{2}sgn\left(\kappa \right)}={\left|\kappa \right|}^{\alpha n}\left[cos\left(\theta \frac{\pi }{2}n\right)+isin\left(\theta \frac{\pi }{2}n\right)sgn\left(\kappa \right)\right],$$

using (13) and (14) we can obtain the FT inverse of ${\mathsf{\Psi }}_{\theta n}^{\alpha n}$ as [36]

$${\mathcal{F}}^{-1}{\mathsf{\Psi }}_{\theta n}^{\alpha n}=\frac{sin\left[\right(\alpha +\theta ·sgn\left(x\right))n\pi /2]}{sin\left(\alpha n\pi \right)\mathsf{\Gamma }(-\alpha )}{\left|x\right|}^{-\alpha -1}\left|x\right|>0.$$

(62)

However, using the reflection property of the gamma function, $\frac{sin\left(\alpha n\pi \right)}{\pi }=-\frac{1}{\mathsf{\Gamma }(-\alpha n)\mathsf{\Gamma }(1+\alpha n)}$, we can write:

$${\mathcal{F}}^{-1}{\mathsf{\Psi }}_{\theta n}^{\alpha n}=\frac{sin\left[\right(\alpha +\theta ·sgn\left(x\right))n\pi /2]}{\pi }{\mathsf{\Gamma }(\alpha n+1)\left|x\right|}^{-\alpha n-1}\left|x\right|>0$$

(63)

It is interesting to separate the symmetric and anti-symmetric terms:

$${\mathcal{F}}^{-1}{\mathsf{\Psi }}_{\theta n}^{\alpha n}=\frac{sin(\alpha n\pi /2)cos(\theta n\pi /2)}{\pi }{\mathsf{\Gamma }(\alpha n+1)\left|x\right|}^{-\alpha n-1}+\frac{cos(\alpha n\pi /2)sin(\theta n\pi /2)}{\pi }\mathsf{\Gamma }(\alpha n+1){\left|x\right|}^{-\alpha n-1}sgn\left(x\right)$$

(64)

These results lead us to announce the following theorem.

Theorem 2.

Let $\beta >\alpha$. The inverse Fourier transform of $G(\kappa ,t),$ defined by (47), is given by

$$\begin{array}{cc}\hfill g_{\theta }^{\alpha ,\beta }(x,t)& ={\displaystyle \frac{1}{\pi \left|x\right|}}\sum _{n=0}^{\infty }{(-1)}^{n}sin(\alpha n\pi /2)cos(\theta n\pi /2){\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}{\left|x\right|}^{-\alpha n}{t}^{\beta n}\hfill \\ \hfill & +sgn\left(x\right){\displaystyle \frac{1}{\pi \left|x\right|}}\sum _{n=0}^{\infty }{(-1)}^{n}cos(\alpha n\pi /2)sin(\theta n\pi /2){\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}{\left|x\right|}^{-\alpha n}{t}^{\beta n}\hfill \\ \hfill & ={\displaystyle \frac{1}{\pi \left|x\right|}}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left[(\alpha +\theta sgn(x\left)\right)n\pi /2\right]{\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}{\left|x\right|}^{-\alpha n}{t}^{\beta n}\hfill \end{array}$$

(65)

We must note that the convergence of the series depends mainly on the factor ${\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}$. It is immediate to conclude that

$$\underset{n\to \infty }{lim}{\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}=\left\{\begin{array}{cc}\infty \hfill & \alpha >\beta \hfill \\ 0\hfill & \alpha <\beta \hfill \\ 1\hfill & \alpha =\beta .\hfill \end{array}\right.$$

(66)

Therefore, relation (65) is interesting for $\alpha <\beta .$ It generalizes the results previously obtained for $\beta =1$ in the context of the stable distributions [44].

Example 1.

Let $\beta =2\alpha$. Then

$${\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}=\frac{1}{2}{\displaystyle \frac{\mathsf{\Gamma }\left(\alpha n\right)}{\mathsf{\Gamma }\left(2\alpha n\right)}}$$

As $\mathsf{\Gamma }\left(2z\right)=\mathsf{\Gamma }\left(z\right)\mathsf{\Gamma }(z+1/2)2^{2z-1}/\sqrt{\pi }$ [47],

$${\displaystyle \frac{\mathsf{\Gamma }(\alpha n+1)}{\mathsf{\Gamma }(\beta n+1)}}=\frac{1}{\sqrt{\pi }}{\displaystyle \frac{2^{-2\alpha n}}{\mathsf{\Gamma }(\alpha n+1/2)}}$$

we obtain from (65)

$$g_{\theta }^{\alpha ,\beta }(x,t)={\displaystyle \frac{1}{\sqrt{\pi }\left|x\right|}}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left[(\alpha +\theta sgn(x\left)\right)n\pi /2\right]{\displaystyle \frac{2^{-2\alpha n}}{\mathsf{\Gamma }(\alpha n+1/2)}}{\left|x\right|}^{-\alpha n}{t}^{2\alpha n}$$

(67)

With $\alpha =1/2$ and $\theta =\pm 1/2$, the Lévi–Smirnov distributions emerge.

Remark 6.

The entropy of the $\alpha =1,\beta =2$ case was computed in (39)

4.4. Space-Dominant Case ($\alpha >\beta$)

When $\alpha >\beta$, the approach we followed above is not suitable, since the series becomes divergent. Therefore, we have to find a way where the two orders play reverse roles.

Theorem 3.

Let $\alpha >\beta$ and $\alpha >1$. The Fourier inverse of $G(\kappa ,t),$ (47), is now given by

$$\begin{array}{cc}\hfill g(x,t)& ={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+1)\beta /\alpha )}{\left(2n\right)!}}\frac{sin\left((2n+1)\pi \beta /\alpha \right)}{sin\left((2n+1)\pi /\alpha \right)}{x}^{2n}{t}^{-(2n+1)\beta /\alpha }\hfill \\ \hfill & +{\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left((2n+2)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+2)\beta /\alpha )}{(2n+1)!}}\frac{sin\left((2n+2)\pi \beta /\alpha \right)}{sin\left((2n+2)\pi /\alpha \right)}{x}^{2n+1}{t}^{-(2n+2)\beta /\alpha }\hfill \end{array}$$

(68)

Remark 7.

We must note that (68) is a generalization for any β less than α of the results known for the stable distributions corresponding to $\beta =1$ that emerges as a particular case.

Proof. 

We write the inverse of (21) as

$$g(x,t)={\displaystyle \frac{1}{{\left(2\pi \right)}^{2}i}}{\int }_{\gamma }{\int }_{\mathbb{R}}{\displaystyle \frac{1}{s}}{\displaystyle \frac{s^{\beta }}{s^{\beta }+{\mathsf{\Psi }}_{\theta }^{\alpha }}}{e}^{st}{e}^{i\kappa x}\mathrm{d}s\mathrm{d}\kappa ,$$

(69)

where $\gamma$ is a vertical straight line in the right half complex plane. From it, define a new integration path ${\gamma }_u$ that results from $\gamma$ by the transformation $u=s^{\beta }$ that will be used in the integrand. This path consists of two half straight lines making angles of $\pm \beta \frac{\pi }{2}$ with the real axis. Then, we obtain:

$$g(x,t)={\displaystyle \frac{1}{\beta {\left(2\pi \right)}^{2}i}}{\int }_{{\gamma }_u}{\int }_{\mathbb{R}}{\displaystyle \frac{1}{u+{\mathsf{\Psi }}_{\theta }^{\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{i\kappa x}\mathrm{d}u\mathrm{d}\kappa ,$$

(70)

However,

$${\displaystyle \frac{1}{u+{\mathsf{\Psi }}_{\theta }^{\alpha }}}={\int }_0^{\infty }{e}^{-{\mathsf{\Psi }}_{\theta }^{\alpha }\tau }{e}^{-u\tau }\mathrm{d}\tau ,$$

that allows us to write

$$g(x,t)={\displaystyle \frac{1}{\beta {\left(2\pi \right)}^{2}i}}{\int }_0^{\infty }{\int }_{{\gamma }_{\beta }}{\int }_{\mathbb{R}}{e}^{-{\mathsf{\Psi }}_{\theta }^{\alpha }\tau }{e}^{v^{\frac{1}{\beta }}t}{e}^{i\kappa x}\mathrm{d}v\mathrm{d}\kappa e^{-v\tau }\mathrm{d}\tau ={\displaystyle \frac{1}{\beta 2\pi i}}{\int }_0^{\infty }{\int }_{{\gamma }_{\beta }}I(x,\tau )e^{v^{\frac{1}{\beta }}t}{e}^{-v\tau }\mathrm{d}v\mathrm{d}\tau .$$

(71)

We are going to consider first the inverse FT

$$I(x,\tau )={\displaystyle \frac{1}{2\pi }}{\int }_{\mathbb{R}}{e}^{-{\mathsf{\Psi }}_{\theta }^{\alpha }\tau }{e}^{i\kappa x}\mathrm{d}\kappa$$

If we expand $e^{-{\mathsf{\Psi }}_{\theta }^{\alpha }\tau }$ in Taylor series, we are led to the results obtained in Section 4.3. Therefore, we need to use another method. One possibility is the use of the integration in the complex plane with application of the Cauchy theorem as done in [44] for the stable distribution study. Here, we will follow a different method. We can write

$$I(x,\tau )={\displaystyle \frac{1}{2\pi }}{\int }_0^{\infty }{e}^{-{\kappa }^{\alpha }{e}^{i\theta \frac{\pi }{2}}\tau }{e}^{i\kappa x}\mathrm{d}\kappa +{\displaystyle \frac{1}{2\pi }}{\int }_0^{\infty }{e}^{-{\kappa }^{\alpha }{e}^{-i\theta \frac{\pi }{2}}\tau }{e}^{-i\kappa x}\mathrm{d}\kappa$$

Note that the second integral results from the first with the substitutions $\theta \to -\theta$ and $x\to -x.$ Therefore,

$$I(x,\tau )={\displaystyle \frac{1}{\pi }}Re\left\{{\int }_0^{\infty }{e}^{-{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}}\tau }{e}^{i\kappa x}\mathrm{d}\kappa \right\}$$

If $\alpha =1$,

$$I(x,\tau )={\displaystyle \frac{1}{\pi }}\left[{\displaystyle \frac{1}{e^{i\theta \frac{\pi }{2}}\tau -ix}}+{\displaystyle \frac{1}{e^{-i\theta \frac{\pi }{2}}\tau +ix}}\right]$$

(72)

that must be substituted in (71). We are going to continue with the $\alpha >1$ case. Perform the substitution $v={\kappa }^{\alpha }$ in $I(x,\tau )$ and use the Taylor series of the exponential to obtain

$${\displaystyle \frac{1}{\pi }}{\int }_0^{\infty }{e}^{-{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}}\tau }{e}^{i\kappa x}\mathrm{d}\kappa ={\displaystyle \frac{1}{\alpha \pi }}{\int }_0^{\infty }{e}^{-v{e}^{i\theta \frac{\pi }{2}}\tau }{e}^{i{v}^{1/\alpha }x}{v}^{1/\alpha -1}\mathrm{d}v={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{\displaystyle \frac{x^n}{n!}}{i}^n{\int }_0^{\infty }{e}^{-v{e}^{i\theta \frac{\pi }{2}}\tau }{v}^{(n+1)/\alpha -1}\mathrm{d}v$$

Assume that $\left|\theta \right|<1$. Then, ${\int }_0^{\infty }{e}^{-v{e}^{i\theta \frac{\pi }{2}}\tau }{v}^{(n+1)/\alpha -1}\mathrm{d}v$ is the LT of the function $v^{(n+1)/\alpha -1}$, $(v>0),$ which reads

$${\int }_0^{\infty }{e}^{-v{e}^{i\theta \frac{\pi }{2}}\tau }{v}^{(n+1)/\alpha -1}\mathrm{d}v={\displaystyle \frac{\mathsf{\Gamma }\left(\right(n+1)/\alpha )}{e^{i\left[(n+1)\frac{\pi \theta }{2\alpha }\right]}{\tau }^{(n+1)/\alpha }}}$$

and gives

$${\displaystyle \frac{1}{\pi }}{\int }_0^{\infty }{e}^{-{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}}\tau }{e}^{i\kappa x}\mathrm{d}\kappa ={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{\displaystyle \frac{x^n}{n!}}{i}^n{e}^{-i\left[(n+1)\frac{\pi \theta }{2\alpha }\right]}{\tau }^{-(n+1)/\alpha }$$

As we are only interested in the real terms, we obtain

$$\begin{array}{cc}\hfill I(x,\tau )& ={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+1)/\alpha )}{\left(2n\right)!}}{\displaystyle \frac{x^{2n}}{{\tau }^{(2n+1)/\alpha }}}\hfill \\ \hfill & +{\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left((2n+2)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+2)/\alpha )}{(2n+1)!}}{\displaystyle \frac{x^{2n+1}}{{\tau }^{(2n+2)/\alpha }}}\hfill \end{array}$$

(73)

The first term is an even function, while the second is odd. Now, return back to (71) and insert there the result expressed in (73) to get

$$\begin{array}{cc}\hfill g(x,t)& ={\displaystyle \frac{1}{\alpha \beta \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+1)/\alpha )}{\left(2n\right)!}}{\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{x^{2n}}{{\tau }^{(2n+1)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau \hfill \\ \hfill & +{\displaystyle \frac{1}{\alpha \beta \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left((2n+2)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+2)/\alpha )}{(2n+1)!}}{\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{x^{2n+1}}{{\tau }^{(2n+2)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau \hfill \end{array}$$

(74)

and

$$\begin{array}{cc}\hfill g(x,t)& ={\displaystyle \frac{1}{\alpha \beta \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+1)/\alpha )}{\left(2n\right)!}}{x}^{2n}{\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+1)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau \hfill \\ \hfill & +{\displaystyle \frac{1}{\alpha \beta \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left((2n+2)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\right(2n+2)/\alpha )}{(2n+1)!}}{x}^{2n+1}{\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+2)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau \hfill \end{array}$$

(75)

Consider the LT ${\int }_0^{\infty }{x}^{-a}{e}^{wx}\mathrm{d}x.$ If $a>0$, it is a singular integral. To continue, we adopt Hadamard’s procedure by recovering only the finite part, so that we can make:

$${\int }_0^{\infty }{x}^{-a}{e}^{wx}\mathrm{d}x=w^{a-1}\mathsf{\Gamma }(-a+1)$$

Therefore,

$${\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+1)/\alpha }}}{e}^{-u\tau }\mathrm{d}\tau =u^{(2n+1)/\alpha -1}\mathsf{\Gamma }\left(-\frac{(2n+1)}{\alpha }+1\right)\mathrm{and}{\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+2)/\alpha }}}{e}^{-u\tau }\mathrm{d}\tau =u^{(2n+2)/\alpha -1}\mathsf{\Gamma }\left(-\frac{(2n+2)}{\alpha }+1\right)$$

from which

$${\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+1)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau ={\displaystyle \frac{\mathsf{\Gamma }\left(-\frac{(2n+1)}{\alpha }+1\right)}{2\pi i}}{\int }_{{\gamma }_{\beta }}{u}^{(2n+1)/\alpha -1}{e}^{u^{\frac{1}{\beta }}t}\mathrm{d}u=\beta \frac{\mathsf{\Gamma }\left(-\frac{(2n+1)}{\alpha }+1\right)}{\mathsf{\Gamma }\left(-\frac{(2n+1)\beta }{\alpha }+1\right)}{t}^{-(2n+1)\beta /\alpha }$$

and

$${\displaystyle \frac{1}{2\pi i}}{\int }_{{\gamma }_{\beta }}{\int }_0^{\infty }{\displaystyle \frac{1}{{\tau }^{(2n+2)/\alpha }}}{e}^{u^{\frac{1}{\beta }}t}{e}^{-u\tau }\mathrm{d}u\mathrm{d}\tau ={\displaystyle \frac{\mathsf{\Gamma }\left(-\frac{(2n+2)}{\alpha }+1\right)}{2\pi i}}{\int }_{{\gamma }_{\beta }}{u}^{(2n+2)/\alpha -1}{e}^{u^{\frac{1}{\beta }}t}\mathrm{d}u=\beta \frac{\mathsf{\Gamma }\left(-\frac{(2n+2)}{\alpha }+1\right)}{\mathsf{\Gamma }\left(-\frac{(2n+2)\beta }{\alpha }+1\right)}{t}^{-(2n+2)\beta /\alpha }$$

Finally,

$$\begin{array}{cc}\hfill g(x,t)& ={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\frac{(2n+1)}{\alpha }\right)}{\left(2n\right)!}}\frac{\mathsf{\Gamma }\left(-\frac{(2n+1)}{\alpha }+1\right)}{\mathsf{\Gamma }\left(-\frac{(2n+1)\beta }{\alpha }+1\right)}{x}^{2n}{t}^{-(2n+1)\beta /\alpha }\hfill \\ \hfill & +{\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}sin\left((2n+2)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }\left(\frac{(2n+2)}{\alpha }\right)}{(2n+1)!}}\frac{\mathsf{\Gamma }\left(-\frac{(2n+2)}{\alpha }+1\right)}{\mathsf{\Gamma }\left(-\frac{(2n+2)\beta }{\alpha }+1\right)}{x}^{2n+1}{t}^{-(2n+2)\beta /\alpha }\hfill \end{array}$$

(76)

Using the reflection property of the Gamma function, we can rewrite (76) as shown in (68). □

Example 2.

Let $\theta =0,$ $\alpha =2,$ and $\beta =1$. As $\mathsf{\Gamma }(n+1/2)=\frac{\left(2n\right)!\sqrt{\pi }}{4^{n}n!}$, we obtain the Gaussian introduced in Section 3.2.

Example 3.

Let $\alpha =2\beta$. We have:

$$g(x,t)={\displaystyle \frac{1}{\alpha \pi }}\sum _{n=0}^{\infty }{(-1)}^{n}cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{\mathsf{\Gamma }(n+1/2)}{\left(2n\right)!}}\frac{sin\left((2n+1)\pi /2\right)}{sin\left((2n+1)\pi /\alpha \right)}{x}^{2n}{t}^{-n-1/2}$$

As $\mathsf{\Gamma }(n+1/2)=\frac{\left(2n\right)!\sqrt{\pi }}{4^{n}n!}$, we obtain

$$g(x,t)={\displaystyle \frac{1}{\alpha \sqrt{\pi t}}}\sum _{n=0}^{\infty }cos\left((2n+1)\frac{\pi \theta }{2\alpha }\right){\displaystyle \frac{1}{4^{n}n!}}\frac{1}{sin\left((2n+1)\pi /\alpha \right)}{x}^{2n}{t}^{-n}$$

Now, particularize to $\theta =0$ and $\alpha =\frac{4}{3},$

$$g(x,t)={\displaystyle \frac{1}{\alpha \sqrt{\pi t}}}\sum _{n=0}^{\infty }{\displaystyle \frac{1}{4^{n}n!}}\frac{1}{sin\left((2n+1)\frac{3\pi }{4}\right)}{x}^{2n}{t}^{-n}$$

However,

$$\frac{1}{sin\left((2n+1)\frac{3\pi }{4}\right)},n=0,1,\cdots =\sqrt{2}\left[1,1,-1,-1,1,\cdots \right]=2sin\left((n+1)\frac{3\pi }{4}\right)=-i{e}^{i\left((n+1)\frac{3\pi }{4}\right)}+i{e}^{-i\left((n+1)\frac{3\pi }{4}\right)}$$

and

$$\sum _{n=0}^{\infty }{\displaystyle \frac{e^{i\left((n+1)\frac{4\pi }{3}\right)}}{4^{n}n!}}{x}^{2n}{t}^{-nt}=e^{i\frac{4\pi }{3}}\sum _{n=0}^{\infty }{\displaystyle \frac{\frac{x^2{e}^{i\frac{3\pi }{4}}}{4t}}{n!}}=e^{i\frac{3\pi }{4}}{e}^{\frac{x^2{e}^{i\frac{3\pi }{4}}}{4t}}$$

which leads to

$$g(x,t)=-i{e}^{i\frac{3\pi }{4}}{e}^{\frac{x^2{e}^{i\frac{3\pi }{4}}}{4t}}+i{e}^{-i\frac{3\pi }{4}}{e}^{\frac{x^2{e}^{-i\frac{3\pi }{4}}}{4t}}=\frac{1+i}{\sqrt{2}}{e}^{\frac{x^2(-1+i)}{4\sqrt{2}t}}+\frac{1-i}{\sqrt{2}}{e}^{\frac{x^2(-1-i)}{4\sqrt{2}t}}$$

giving

$$g(x,t)=2e^{-\frac{x^2}{4\sqrt{2}t}}cos\left(\frac{x^2}{4\sqrt{2}t}+\frac{\pi }{4}\right)$$

(77)

Remark 8.

With $\alpha =\frac{4}{5},\frac{4}{7},\frac{4}{9},\cdots ,$ we obtain other solutions similar to (77).

Example 4.

Again with $\alpha =2\beta$ and $\theta =0$, as above, we set $\alpha =\frac{8}{5},$ to obtain

$$g(x,t)={\displaystyle \frac{1}{\alpha \sqrt{\pi t}}}\sum _{n=0}^{\infty }{\displaystyle \frac{1}{4^{n}n!}}\frac{1}{sin\left((2n+1)\frac{5\pi }{8}\right)}{x}^{2n}{t}^{-n}$$

With some work and the help of the relation $sin\frac{\varphi }{2}=\frac{1-cos\varphi }{2}$, we obtain one period of the function $\frac{1}{sin\left((2n+1)\frac{5\pi }{8}\right)}$ that we state as $P=\left[a,-b,-a,b,-a,b,a,-b\right]$ where $a=\sqrt{8-2\sqrt{2}}$ and $b=\sqrt{8+2\sqrt{2}}$, so that

$$P=\left[2.2741,-3.2907,-2.2741,3.2907,-2.2741,3.2907,2.2741,-3.2907\right]$$

Using the discrete Fourier transform [34] we conclude that

$$\frac{1}{sin\left((2n+1)\frac{5\pi }{8}\right)}=A{e}^{i(2\pi \frac{1}{8}n+\varphi )}+A^{\ast }{e}^{-i(2\pi \frac{1}{8}n+\varphi )}+B{e}^{i(2\pi \frac{3}{8}n+\psi )}+B^{\ast }{e}^{-i(2\pi \frac{3}{8}n+\psi )},n=0,1,2,\cdots$$

with $A=6.5830/8$, $B=14.5830/8$, $\varphi =0.7572\pi$, and $\psi =-0.1010\pi .$ With these expressions we obtain a sum of two functions like (77).

Remark 9.

With $\alpha =\frac{8}{7},\frac{8}{9},\cdots ,$ we obtain other similar solutions.

Excepting in particular cases, the entropy computation is not easy to perform as we showed in Section 3.2.4.

5. Discussion and Conclusions

The traditional fractional diffusion equation was based on the Caputo time derivative and on a space pseudo-derivative defined in the frequency domain. The Caputo derivative has the initial-condition drawback [41,42]. The use of a time derivative defined on $\mathbb{R}$ avoids such a problem. Concerning the space derivative, which was defined implicitly before, it was here considered as a particular case of the unified derivative, the Riesz–Feller derivative [36], which was defined both by a GL-type derivative and by a Riesz–Feller integral.

A main point in the diffusion studies concerns the entropy computations and the corresponding entropy paradox. This seems to be a consequence of two facts: the incomplete entropy computations due to the inherent difficulties and a hasty application of scale invariance. To enable the acquisition of tangible results, we considered a particular case of each Tsallis and Rényi entropies, corresponding to setting the parameter that defines them at the value $2.$ This allows us to express the entropy in terms of the “energy” of the probability density function or, using the Parseval relation, its frequency version. With this procedure, we could compute the entropy of the neutral case and of the stable distributions. We showed that really the entropy in the wave regime is $-\infty ,$ and even it increases with time. However, more importantly, the entropy decreases continuously when $\beta$ approaches 2. The scale invariance is questionable. If we take $G(\kappa ,s)$ (26), we can write

$$G(\kappa ,s)={\displaystyle \frac{s^{\beta -1}}{s^{\beta }+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}}}={\displaystyle \frac{1}{s}}{\displaystyle \frac{1}{1+{\left|\kappa \right|}^{\alpha }{e}^{i\theta \frac{\pi }{2}sgn\left(\kappa \right)}{s}^{-\beta }}},$$

which suggests that the ${\displaystyle \frac{1}{s}}$ factor destroys the scale invariance.

The search for solutions of the diffusion equation was done differently from the traditional method. We studied first the neutral case, having obtained the known solution, but using a different easier procedure. For it, we obtained an expression for the Rényi entropy. Then, we considered the dominant time and dominant space cases, where we obtained generalizations of known results obtained before in the study of stable processes. In the dominant space case, we could not solve the equation with all the generality, namely, for low values of the orders. This will remain an open problem. It is important to remark that the scale invariance is different in both regimes. The solutions we obtained assumed the form of series that, while convergent, give rise to numerical difficulties. Perhaps integral solutions can avoid such problems.

Another problem that resulted from examples we presented is the inexistence of a positivity criterion: we found solutions that are not positive and then cannot be considered as probablility density functions. In [48] (p. 265), there is a necessary condition that the Fourier transform of positive functions must verify, but it seems there is no sufficient condition.

Independently of the probabilistic interpretation of the solution, the proposed methodology can be used in other similar equations, as the ones we obtain by joining other terms with different derivative orders [42].