Stability of Parametric Intuitionistic Fuzzy Multi-Objective Fractional Transportation Problem

Abstract

In this study, a parametric intuitionistic fuzzy multi-objective fractional transportation problem (PIF-MOFTP) is proposed. The current PIF-MOFTP has a single-scalar parameter in the objective functions and an intuitionistic fuzzy supply and demand. Based on the $\left(\alpha ,\beta \right)$-cut concept a parametric $\left(\alpha ,\beta \right)$-MOFTP is established. Then, a fuzzy goal programming (FGP) approach is utilized to obtain $\left(\alpha ,\beta \right)$-Pareto optimal solution. We investigated the stability set of the first kind (SSFK) corresponding to the solution by extending the Kuhn-Tucker optimality conditions of multi-objective programming problems. An algorithm to crystalize the progressing SSFK for PIF-MOFTP as well as an illustrative numerical example is presented.

1. Introduction

Transportation issues (TP) have been studied in various writings [1,2,3,4,5,6,7]. These issues and their solution processes postulate a worthy task in logistics and supply chain organization for reducing expenses, further developing service quality, etc. [3,8]. Nonetheless, TP is described by multiple, incommensurable, and clashing objective functions, being known as the multi-objective transportation problem (MO-TP). Accordingly, in MO-TP, the idea of an ideal solution offers spot to the idea of the best compromise solution or the non-dominated solutions. Optimization of the ratio of two functions is called fractional programming (ratio optimization) [7,9]. To be sure, in such circumstances, it is often a question of optimizing a ratio of benefit/cost, stock/deals, specialist/patient, and so on, subject to some constraints [7,9].

One of the significant issues looked at by specialists is that involving the exact values of parameters [7]. In this way, this might involve thinking about vagueness, or specifying the fundamental parameters of the model, which are the coefficients of the objective function and the constrains [4,8]. Accordingly, it might be naturalistic to take the distinct adjectival information on specialists and leaders about the parameters which can be exemplified as fuzzy data [7,10]. Uncertainty may happen because of the accompanying unrestrained factors. In this study the main hypotheses are that the transportation charge has a parametric nature, and the supply and the demand parameters are intuitionistic fuzzy numbers (IFNs). The main hypotheses have not been presented in the literature, and the basic question is how we can get the SSFK for such PIF-MOFTP.

2. Literature Review

The research on MO-TP is improved by fusing the diverse numerical models and procedures. James et al. [11] examined transportation administration quality dependent on data combination. A lot of examination that deals with transportation wellbeing was created by Ergun et al. [12], Sheu and Chen [13]. Recently, MO-TP under different circumstances has been discussed by Roy et al. [14,15], Roy and Mahapatra [16], Roy [17], Maity and Roy [18,19].

Although fuzzy set theory (FST) is novel tool in handling uncertainties, it cannot tackle special kinds of uncertainties, as it is difficult to depict the membership degree using one specific value. To overcome the lack of knowledge of non-membership degrees, intuitionistic fuzzy set (IFS) was presented in 1986 by Atanassov [20] as an extension of FST. In IFS, each element in a set is attached with two grades: membership and non-membership, where the sum of these two grades is restricted to less or equal to one. Moreover, many creators have been utilized IFS for addressing various sorts of TPs [21,22]. The study of MO-TP with vague numbers has been presented by Ammar and Youness [1]. The fuzzy programming strategy was acquainted with tackle MO-TP with various non-linear membership functions [23]. IFS has additionally been utilized by several scientists to tackle different types of TPs [10,24]. One more strategy for thoroughly considering linear MO-TPs with vague nature has been suggested by Gupta and Kumar [25]. Recently, MO-TP under various types of uncertainty has been discussed by Roy and Mahapatra [16], Maity and Roy [26], and Ebrahimnejad and Verdegay [10]. Mahajan and Gupta [27] proposed a fully IF MO-TP utilizing various membership functions. Achievement stability set for parametric linear FGP problems has been introduced by El Sayed and Farahat [28]. The neutrosophic goal programming approach for solving the multi-objective fractional transportation problem was introduced by Veeramani et al., [29]. Pramanik and Banerjee [30] proposed a chance-constrained capacitated MO-TP with two fuzzy goals, and a consensus solution was found. Edalatpanah [31] developed a nonlinear framework for neutrosophic linear programming. Furthermore, Rizk-Allah et al. [32] developed a compromise solution framework for the MO-TP based on the neutrosophic environment. A fuzzy approach using generalized dinkelbach’s algorithm for linear multi-objective fractional transportation problem (MOFTP) has been presented by Cetin and Tiryaki [3]. A fuzzy mathematical programming approach for solving fuzzy linear fractional programming problem has been demonstrated by Veeramani and Sumathi [33]. El Sayed and Abo-Sinna [7] introduced the intuitionistic fuzzy multi-objective fractional transportation problem (IF-MOFTP).

Parametric programming examines the impact of preordained continuous varieties in the objective function coefficients and the right-hand side of the constraints on the ideal solution [34,35,36]. In parametric analysis the objective function and the right-hand side vectors are replaced with the parameterized function $\mathit{c}\left(\vartheta \right)$ and $\mathit{b}\left(\alpha ,\beta \right)$, where $\vartheta \mathrm{and} \alpha ,\beta$ are the parameter of variation. The general idea of parametric analysis is to start with the $\alpha$-Pareto optimal solution at $\vartheta ={\vartheta }^{*}, \alpha ={\alpha }^{*}, \beta ={\beta }^{*}$. Then by applying KKT optimality the SSFK is determined [35,37]. The concept of the stability set of the first kind (SSFK) has been introduced by Osman [35], and extended by Saad [38], Saad and Hughes [39], Osman et al. [36], Saad et al. [40].

In prior examinations, the MO-TP was created with the presumption that the supply, demand, and cost boundaries were known. Nonetheless, applications, every one of the parameters of the TP are not for the most part characterized definitively. It might have IF values. Comparable contemplations might be taken for supply and demand parameters in TP of this paper. Keeping this perspective, the primary commitments are concerned with two unique viewpoints: one is to find a $\left(\alpha ,\beta \right)$-Pareto optimal solution for the PIF-MOFTP, and another is to investigate the SSFK for PIF-MOFTP. First, based on the $\left(\alpha ,\beta \right)$-cut methodology a parametric $\left(\alpha ,\beta \right)$-MOFTP is established. Then, A FGP approach is used to get $\left(\alpha ,\beta \right)$-Pareto optimal solution. Finally, the KKT optimality conditions applied to get the SSFK. An algorithm to clarify the developed SSFK for PIF-MOFTP as well as an illustrative numerical example are given.

The rest of this study is organized as follows: after the introduction and literature review, Section 3 introduces some basic concepts. Modelling of the PIF-MOFTP is presented in Section 4. Section 5 demonstrates the FGP methodology for tackling the PIF-MOFTP. In the next section the SSFK is investigated. An algorithm for obtaining the SSFK for PIF-MOFTP is introduced in Section 6. An illustrative example, discussion and limitations is given in Section 7. This paper ends with some concluding remarks.

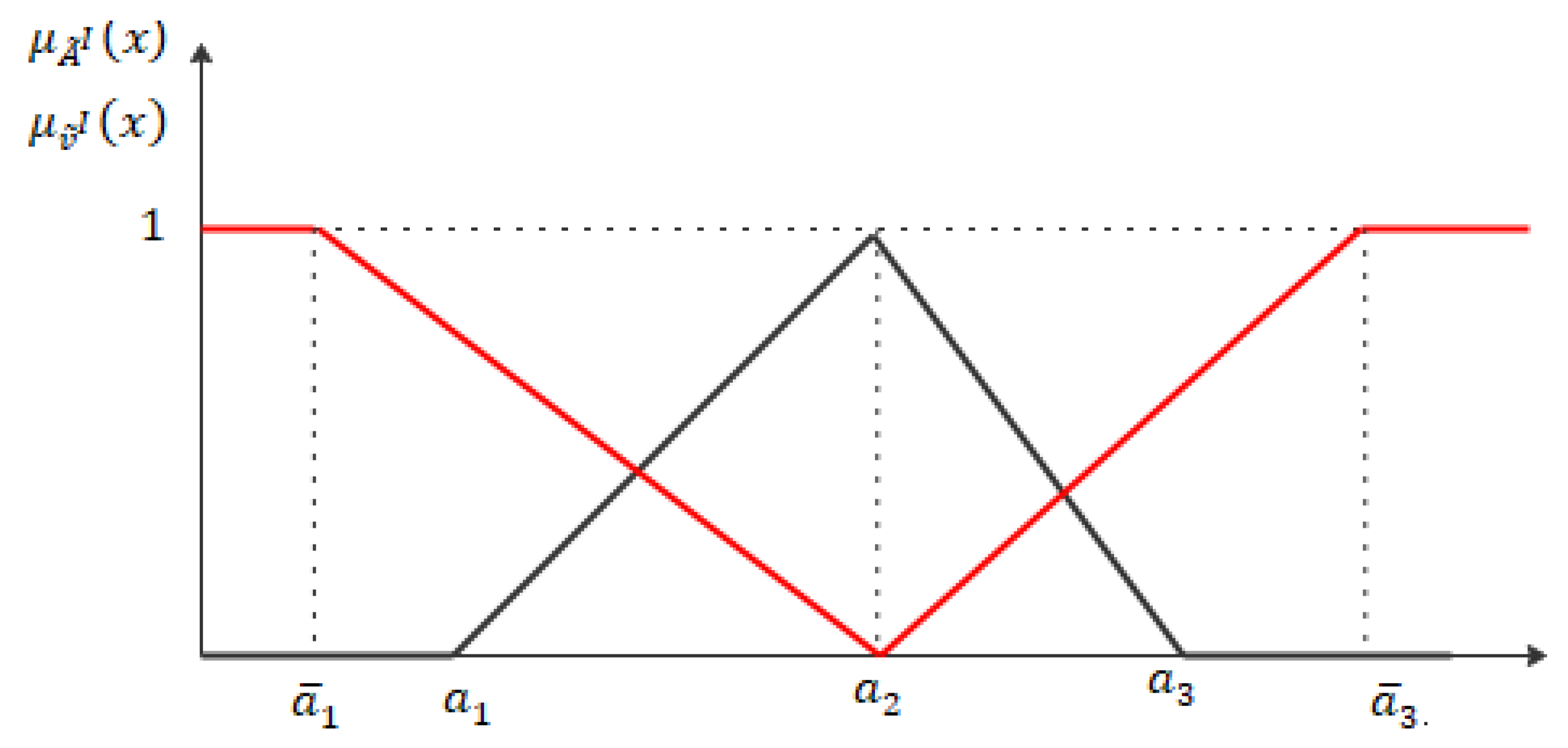
3. Preliminaries

This part presents the concept of IFS [20,21,41,42].

Definition 1.

An IFS ${\tilde{A}}^I$ in $X$ is a set of ordered triples${\tilde{A}}^I=\left\{\left(x,{\mu }_{{\tilde{A}}^I}\left(x\right),v_{{\tilde{A}}^I}\left(x\right)\right)|x\in X\right\}$, where ${\mu }_{{\tilde{A}}^I}\left(x\right),{ v}_{{\tilde{A}}^I}\left(x\right):X\to \left[0,1\right]$ are functions such that $0\le {\mu }_{{\tilde{A}}^I}\left(x\right)+v_{{\tilde{A}}^I}\left(x\right)\le 1, \forall x\in X$. The value of ${\mu }_{{\tilde{A}}^I}\left(x\right)$ acts as the grade of membership and $v_{{\tilde{A}}^I}\left(x\right)$ acts as the grade of non-membership of the element $x\in X$ being in ${\tilde{A}}^I$. $h\left(x\right)=1-{\mu }_{{\tilde{A}}^I}\left(x\right)-v_{{\tilde{A}}^I}\left(x\right)$ represents the grade of hesitation for the element $x$ in ${\tilde{A}}^I$[20,41].

Definition 2.

An IFN of the form ${\tilde{A}}^I=\left(a_1,a_2,a_3;{\stackrel{-}{a}}_1,a_2,{\stackrel{-}{a}}_3\right)$ is said to be triangular IFN (TIFN) with membership and non-membership functions defined as [41,43]:

$${\mu }_{{\tilde{A}}^I}\left(x\right)=\{\begin{array}{c}\frac{x-a_1}{a_2-a_1}, a_1\le x\le a_2,\\ \frac{a_3-x}{a_3-a_2}, a_2\le x\le a_3\\ 0, otherwise\end{array},$$

(1)

$${\nu }_{{\tilde{A}}^I}\left(x\right)=\{\begin{array}{c}\frac{a_2-x}{a_2-{\overline{a}}_1} {\overline{a}}_1\le x\le a_2\\ \frac{x-a_2}{{\overline{a}}_3-a_2} a_2\le x\le {\overline{a}}_3\\ 1 otherwise\end{array},$$

(2)

where $\frac{x-a_1}{a_2-a_1},$ and $\frac{x-a_2}{{\overline{a}}_3-a_2}$ are continuous monotone increasing functions, $\frac{a_3-x}{a_3-a_2}$ and $\frac{a_2-x}{a_2-{\overline{a}}_1}$ are continuous monotone decreasing functions. $\frac{x-a_1}{a_2-a_1},\frac{a_3-x}{a_3-a_2},\frac{a_2-x}{a_2-{\overline{a}}_1} \mathrm{and} \frac{x-a_2}{{\overline{a}}_3-a_2}$ are the left and the right basis functions of the membership function and the non-membership function (see Figure 1), respectively. ${\overline{a}}_1\le a_1\le a_2\le a_3\le {\overline{a}}_3$ and $0\le {\mu }_{{\tilde{A}}^I}\left(x\right)+v_{{\tilde{A}}^I}\left(x\right)\le 1, \forall x\in X$.

Definition 3.

A TIFNs ${\tilde{A}}^I=\left(a_1,a_2,a_3;{\stackrel{-}{a}}_1,a_2,{\stackrel{-}{a}}_3\right)$ is assumed to be a non-negative TIFN iff, ${\stackrel{-}{a}}_1\ge 0$ [41,43].

Definition 4.

Two TIFNs ${\tilde{A}}^I=\left(a_1,a_2,a_3;{\stackrel{-}{a}}_1,a_2,{\stackrel{-}{a}}_3\right)$ and ${\tilde{A}}^I=\left(b_1,b_2,b_3;{\stackrel{-}{b}}_1,b_2,{\stackrel{-}{b}}_3\right)$ are equivalent to one another, ${\tilde{A}}^I={\tilde{B}}^I$ iff, $a_i=b_i$ and ${\stackrel{-}{a}}_i={\stackrel{-}{b}}_i \forall i=1,2,3$ [7,41,43].

Definition 5.

$\left(\alpha ,\beta \right)$-cut of an IFS ${\tilde{A}}^I$ is defined by: ${\tilde{A}}_{\left(\alpha ,\beta \right)}^I=\left\{x:{\mu }_{{\tilde{A}}^I}\left(x\right)\ge \alpha ,{\nu }_{{\tilde{A}}^I}\left(x\right)\le \beta , \alpha +\beta \le 1,x\in X\right\}; where \alpha ,\beta \in \left(0,1\right]$.

Definition 6.

$\left(\alpha ,\beta \right)$-cut of a TIFN ${\tilde{A}}^I=\left(a_1,a_2,a_3;{\stackrel{-}{a}}_1,a_2,{\stackrel{-}{a}}_3\right)$ is the set of all $x$ whose degree of membership is greater than or equal to $\alpha$ and degree of non-membership is less than or equal to $\beta$, i.e., ${\tilde{A}}_{\left(\alpha ,\beta \right)}^I=\left\{x:{\mu }_{{\tilde{A}}^I}\left(x\right)\ge \alpha ,{\nu }_{{\tilde{A}}^I}\left(x\right)\le \beta , \alpha +\beta \le 1,x\in X\right\}$.

The $\left(\alpha ,\beta \right)$-cut of a TIFN is shown in Figure 2, is defined as the crisp set of elements $x$ which belong to ${\tilde{A}}^I$ at least to the degree $\alpha$ and which does belong to ${\tilde{A}}^I$ at most to the degree $\beta$.

Now, ${\mu }_{{\tilde{A}}^I}\left(x\right)\ge \alpha \Rightarrow \frac{x-a_1}{a_2-a_1}\ge \alpha$ and $\frac{a_3-x}{a_3-a_2}\ge \alpha$, or $x\ge a_1+\alpha \left(a_2-a_1\right)$ and $x\le a_3-\alpha \left(a_3-a_2\right)$ again, ${\nu }_{{\tilde{A}}^I}\left(x\right)\le \beta \Rightarrow \frac{a_2-x}{a_2-{\overline{a}}_1}\le \beta$ and $\frac{x-a_2}{{\overline{a}}_3-a_2}\le \beta$, or $x\ge a_2-\beta \left(a_2-{\overline{a}}_1\right)$ and $x\le a_2+\beta \left({\overline{a}}_3-a_2\right)$ [43]. Thus, referring to Figure 2 ${\tilde{A}}_{\left(\alpha ,\beta \right)}^I=\left[A_L,A_U\right]$, where $A_L=max\left\{a_1+\alpha \left(a_2-a_1\right),a_2-\beta \left(a_2-{\overline{a}}_1\right)\right\}$ and $A_U=min\left\{a_3-\alpha \left(a_3-a_2\right),a_2+\beta \left({\overline{a}}_3-a_2\right)\right\}$.

4. Mathematical Formulation

In genuine case TP, during the modeling process, the transportation parameters are not precise on account of insufficient information the variance of the market situation. To deal quantitatively with such unclear information, we deemed parametric IF-MOFTP in which single-scalar parameter $\vartheta \in ℝ$ in the objective functions and an intuitionistic fuzzy supply and demand. Suppose that there are m sources and n destinations. Thus, modelling of the parametric IF-MOFTP can be obtained as [3,7,9]:

$$Max\hspace{1em}{Z}_q\left(x,\vartheta \right)=\frac{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}}{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}},\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(3)

Subject to:

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le {\tilde{a}}_i^I,\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(4)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge {\tilde{b}}_j^I,\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(5)

$$x_{ij}\ge 0,\hspace{1em}\hspace{1em}i=1,2,\dots ,m,\hspace{1em}\hspace{1em}j=1,2,\dots ,n.$$

(6)

where $c_{ij}^{\left(q\right)}={\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}$ denotes the parametric profit gained from shipment of $i^{th}$ source to $j^{th}$ destination. Also, $d_{ij}^{\left(q\right)}$ denotes the expense per unit of shipment from $i^{th}$ source to $j^{th}$ destination. ${\delta }^{\left(q\right)}$, ${\rho }^{\left(q\right)}$ are some constant profit and cost, respectively. $x_{ij}^{\left(q\right)}$ is the quantity shipped from $i^{th}$ source to $j^{th}$ destination. ${\tilde{a}}_i^I=\left(a_i^1,a_i^2,a_i^3;{\overline{a}}_i^1,a_i^2,{\overline{a}}_i^3\right)$ stands for the available intuitionistic fuzzy supply at $i^{th}$ source and ${\tilde{b}}_j^I=\left(b_j^1,b_j^2,b_j^3;{\overline{b}}_j^1,b_j^2,{\overline{b}}_j^3\right)$ alludes to the accessible intuitionistic fuzzy demand at $j^{th}$ destination. Further, we postulate that ${{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}>0, q=1,2,\dots ,Q; {\tilde{a}}_i^I>0^I, {\tilde{b}}_j^I>0^I, \forall j;{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}>0^I, {\delta }^{\left(q\right)},{\rho }^{\left(q\right)}>0$ for all $i, j,$ and the gross supply is greater than or equal the gross demand [3,7].

$${\displaystyle \sum }_{i=1}^m{\left({\tilde{a}}_i^I\right)}_{\left(\alpha ,\beta \right)}\ge {\displaystyle \sum }_{j=1}^n{\left({\tilde{b}}_j^I\right)}_{\left(\alpha ,\beta \right)}.$$

(7)

The disparity (7) is considered as a necessary and sufficient condition for the existence of a feasible solution to PIF-MOFTP.

For a certain degree of $\left(\alpha ,\beta \right)$-cut the PIF-MOFTP could be transformed into parametric $\left(\alpha ,\beta \right)$-MOFTP as:

$$Max\hspace{1em}{Z}_q\left(x,\vartheta \right)=\frac{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}}{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}},\hspace{1em}\hspace{1em}\hspace{1em}q=12,\dots ,Q,$$

(8)

Subject to:

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le {\left(a_i\right)}_{\left(\alpha ,\beta \right)}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(9)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge {\left(b_j\right)}_{\left(\alpha ,\beta \right)}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(10)

$$x_{ij}\ge 0,\hspace{1em}\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(11)

$$a_i^L\le {\left(a_i\right)}_{\left(\alpha ,\beta \right)}\le a_i^U,\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(12)

$$b_j^L\le {\left(b_j\right)}_{\left(\alpha ,\beta \right)}\le b_j^U,\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n.$$

(13)

Based on the concept of a convex linear combination method proposed in [40] parametric $\left(\alpha ,\beta \right)$-MOFTP can be rewritten as:

$$Max\hspace{1em}{Z}_q\left(x,\vartheta \right)=\frac{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}}{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}},\hspace{1em}\hspace{1em}q=12,\dots ,Q,$$

(14)

Subject to:

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le \lambda a_i^L+\left(1-\lambda \right)a_i^U\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(15)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge \lambda b_j^L+\left(1-\lambda \right)b_j^U\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(16)

$$x_{ij}\ge 0,\hspace{1em}\lambda \in \left[0,1\right],\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(17)

Let $M_{\left(\alpha ,\beta \right)}$ denote the set of constraints in Equations (15)–(17), the parametric $\left(\alpha ,\beta \right)$-MOFTP has an $\left(\alpha ,\beta \right)$-Pareto optimal solution $x_{ij}^{*}$ at ${\vartheta }^{*}$.

Definition 7.

$\left(\alpha ,\beta \right)$-Pareto optimal solution. $x_{\mathrm{ij}}^{*}\in M_{\left(\alpha ,\beta \right)}$ is said to be an $\left(\alpha ,\beta \right)$-Pareto optimal solution to $\left(\alpha ,\beta \right)$-MOFTP if and only if there does not exist another $x_{ij}^{°}\in M_{\left(\alpha ,\beta \right)}$ $a_i\in {\left(a_i\right)}_{\left(\alpha ,\beta \right)},{ b}_j\in {\left(b_j\right)}_{\left(\alpha ,\beta \right)}$, such that $Z_q\left(x_{ij}^{°},{\vartheta }^{*}\right)\ge Z_q\left(x_{ij}^{*},{\vartheta }^{*}\right)$ with at least one strict inequality hold for $q \left(q=1,2,\dots ,Q\right)$.

5. FGP Methodology for PIF-MOFTP

In this section the FGP approach is applied to obtain the compromise solution of the parametric $\left(\alpha ,\beta \right)$-MOFTP. The objective functions are modeled as fuzzy goals characterized by its’ membership function ${\mu }_{\left(z_q\left(x,{\vartheta }^{*}\right)\right)}$ [36,44,45,46]. The model formulation and solution process are carried out at ϑ = ϑ^*. The membership functions of the q^th fuzzy goals [36,44], is defined as:

$${\mu }_{\left(z_q\left(x,{\vartheta }^{*}\right)\right)}=\{\begin{array}{cc}1,& if Z_q\left(\mathit{x},{\vartheta }^{*}\right)\ge u_q{}^{*},\\ \frac{Z_q\left(\mathit{x},{\vartheta }^{*}\right)-g_q{}^{*}}{u_q{}^{*}-g_q{}^{*}},& if g_q{}^{*}\le Z_q\left(\mathit{x},{\vartheta }^{*}\right)\le u_q{}^{*},\\ 0,& if Z_q\left(\mathit{x},{\vartheta }^{*}\right)\le g_q{}^{*},\end{array} q=1,2,\dots ,Q$$

(18)

where $u_q{}^{*} = max Z_q\left(\mathit{x},{\vartheta }^{*}\right)$, $g_q{}^{*} = min Z_q\left(\mathit{x},{\vartheta }^{*}\right)$, and denotes the upper and lower tolerance limit for the membership function of q^th objective, respectively. In the FGP approach, the most extensive level of membership is unity. So, the membership goals having the aspired level unity follows as [44]:

$${\mu }_q\left(Z_q\left(\mathit{x},{\vartheta }^{*}\right)\right)+d_q^{-}-d_q^{+}=1,\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(19)

where $d_q^{-},d_q^{+}\ge 0,$ with $d_q^{-}\times d_q^{+}=0,$ denote the under- and over-deviations, respectively, from the aspired levels [36,44]. The final FGP model of the parametric $\left(\alpha ,\beta \right)$-MOFTP can be obtained as:

$$Min\hspace{1em}AF={\displaystyle \sum }_{q=1}^Q{w}_q^{-} d_q^{-},$$

(20)

Subject to:

$$\frac{Z_q\left(\mathit{x},{\vartheta }^{*}\right)-g_q{}^{*}}{u_q{}^{*}-g_q{}^{*}}+d_q^{-}-d_q^{+}=1,\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(21)

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le \lambda a_i^L+\left(1-\lambda \right)a_i^U\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(22)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge \lambda b_j^L+\left(1-\lambda \right)b_j^U\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(23)

$$x_{ij}\ge 0,\hspace{1em}\lambda \in \left[0,1\right],\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(24)

$$d_q^{-}\times d_q^{+}=0, \mathrm{and} d_q^{-}, d_q^{+}\ge 0,\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(25)

where $w_q^{-}$ represents the relative importance of achieving the aspired levels of the respective fuzzy goals which given by [44,47]:

$$w_q^{-}=\frac{1}{u_q{}^{*}-g_q{}^{*}},\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}q=1,2,\dots ,Q$$

(26)

Extension of Pal’s Method to Linearize the Membership Goals

It can be easily realized that the parametric membership goals in Equation (19) are non-linear fractional in nature. To avoid such problem, Pal et al. [45] method is extended here to linearize the $q^{th}$ membership goals with single-scalar parameter $\vartheta ={\vartheta }^{*}$ as:

$${\mu }_q\left(Z_q\left(\mathit{x},{\vartheta }^{*}\right)\right)+d_q^{-}-d_q^{+}=1,\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(27)

$$L_q\left(Z_q\left(\mathit{x},{\vartheta }^{*}\right)\right)-L_q{g}_q{}^{*}+d_q^{-}-d_q^{+}=1;\hspace{1em}\hspace{1em}{L}_q=\frac{1}{u_q{}^{*}-g_{ij}{}^{*}},$$

(28)

$$Z_q\left(\mathit{x},{\vartheta }^{*}\right)=\frac{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}}{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}},\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(29)

Substituting from Equation (29) in Equation (28), we obtain:

$$L_q\frac{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}}{{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}}-L_q{g}_q{}^{*}+d_q^{-}-d_q^{+}=1,$$

(30)

$$\begin{array}{c}{L}_q\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}\right]-L_q{g}_q{}^{*}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] +d_q^{-}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]\\ - d_q^{+}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]=\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right],\end{array}$$

(31)

$$\left(\begin{array}{c}{L}_q\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}\right]\\ +d_q^{-}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] \\ -d_q^{+}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] \end{array}\right)=\left(1+L_q{g}_q{}^{*}\right)\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right],$$

(32)

$$\left(\begin{array}{c}{L}_q\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\delta }^{\left(q\right)}\right]\\ +d_q^{-}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] \\ -d_q^{+}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] \end{array}\right)=L_q^{°}\left[{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]; L_q^{\mathbf{0}}=\left(1+L_q{g}_q{}^{*}\right)$$

(33)

$$\begin{array}{l}\left[L_q{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}\right]x_{ij}^{\left(q\right)}+d_q^{-}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]-d_q^{+}\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}=\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right],\end{array}$$

(34)

$${\mathit{C}}_{\mathit{i}\mathit{j}}^{\left(\mathit{q}\right)}{x}_{ij}^{\left(q\right)}+d_q^{-}\left[{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]-d_q^{+}\left[{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]={\mathit{G}}_{\mathit{q}};$$

(35)

where

$${\mathit{C}}_{\mathit{i}\mathit{j}}^{\left(\mathit{q}\right)}=\left[L_q{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}\right],$$

(36)

$${\mathit{G}}_{\mathit{q}}=\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right],$$

(37)

Considering Pal et al. [45], the goal expression in Equation (35) can be linearized as follows. Letting $D_q^{-}=d_q^{-}\left[{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right] \mathrm{and}$ $D_q^{+}=d_q^{+}\left[{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right],$ then the linear form of expression in Equation (32) is obtained as:

$${\mathit{C}}_{\mathit{i}\mathit{j}}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}-D_q^{+}={\mathit{G}}_{\mathit{q}},$$

(38)

with $D_q^{-}, D_q^{+}\ge 0; and D_q^{-}\times D_q^{+}=0,$ since $d_q^{-}, d_q^{+}\ge 0,$ and ${{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}>0$. So, minimization of $d_q^{-}$ means minimization of $D_q^{-}=d_q^{-}\left[{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]$ which is also non-linear. So, involvement of $d_q^{-}\le 1,$ in the solution leads to impose the following constraint in the model:

$$\frac{D_q^{-}}{\left[{{\displaystyle \sum }}_{i=1}^m{{\displaystyle \sum }}_{j=1}^n{d}_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+{\rho }^{\left(q\right)}\right]}\le 1.$$

(39)

Now, the final FGP model of the parametric $\left(\alpha ,\beta \right)$-MOFTP in model (20)–(25) becomes:

$$Min\hspace{1em}AF={\displaystyle \sum }_{q=1}^Q{w}_q^{-} d_q^{-},$$

(40)

Subject to:

$$\left[L_q{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{\left(c_{ij}+{\vartheta }^{*}{\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}\right]x_{ij}^{\left(q\right)}+D_q^{-}-D_q^{+}=\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right],$$

(41)

$${\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n-d_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}\le {\rho }^{\left(q\right)},\hspace{1em}q=1,2,\dots ,Q,\hspace{1em}\forall i,j,$$

(42)

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le \lambda a_i^L+\left(1-\lambda \right)a_i^U,\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(43)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge \lambda b_j^L+\left(1-\lambda \right)b_j^U\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(44)

$$x_{ij}\ge 0,\hspace{1em}\lambda \in \left[0,1\right],\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(45)

$$D_q^{-}\times D_q^{+}=0, \mathrm{and} D_q^{-}, D_q^{+}\ge 0,\hspace{1em}q=1,2,\dots ,Q.$$

(46)

Thus, the above FGP model provides the satisfactory solution $x_{ij}^{*}$ for the parametric $\left(\alpha ,\beta \right)$-MOFTP.

6. The SSFK for Parametric $\mathbf{\left(}\mathit{\alpha },\mathit{\beta }\mathbf{\right)}$-MOFTP

The main area of inquiry is as follows: having solved the parametric $\left(\alpha ,\beta \right)$-MOFTP, to what extent can its data with respect to $\alpha ,\beta \mathrm{and} \vartheta$ be changed without invalidating the efficiency of its $\left(\alpha ,\beta \right)$-Pareto optimal solution? The set of feasible parameters, the solvability set, and the SSFK for parametric $\left(\alpha ,\beta \right)$-MOFTP are defined as:

Definition 8.

The set of feasible parameters for the parametric $\left(\alpha ,\beta \right)$-MOFTP is defined by:

$$ℱ=\left\{\begin{array}{c}a\in R^m,\\ b\in R^n\end{array}|\begin{array}{c}{a}_i\in L_{\alpha ,\beta }\left({\tilde{a}}_i^I\right), i=1,2,\dots m; b_j\in L_{\alpha ,\beta }\left({\tilde{b}}_j^I\right), j=1,2,\dots ,n;\\ \alpha ,\beta \in \left[0,1\right]; and M_{\left(\alpha ,\beta \right)}\left(x_{ij},a,b\right)\ne \varnothing \end{array}\right\}.$$

Definition 9.

The solvability set $ℳ$ of the parametric $\left(\alpha ,\beta \right)$-MOFTP is defined by:

$$ℳ=\left\{\left(\vartheta ,a,b\right)\in R\times R^m\times R^n|\begin{array}{c}parametric\left(\alpha ,\beta \right)-MOFTP has\\ an \left(\alpha ,\beta \right)-Pareto optimal solution.\end{array}\right\}.$$

Definition 10.

Suppose that $x_{ij}^{*}$ be an $\left(\alpha ,\beta \right)$-Pareto optimal solution of the parametric  $\left(\alpha ,\beta \right)$-MOFTP, then the SSFK ${\mathit{S}}_{\mathbf{1}}\left(x_{ij}^{*},\alpha ,\beta \right)$ corresponding to $x_{ij}^{*}$ is defined by:

$${\mathit{S}}_{\mathbf{1}}\left(x_{ij}^{*},\alpha ,\beta \right)=\left\{\left(\vartheta ,a,b\right)\in R\times R^m\times R^n|\begin{array}{c}{x}_{ij}^{*} is an \left(\alpha ,\beta \right)-Pareto optimal solution of \\ parametric \left(\alpha ,\beta \right)-MOFTP \end{array}\right\}.$$

The SSFK of the parametric $\left(\alpha ,\beta \right)$-MOFTP is the set of all parameters corresponding to one $\left(\alpha ,\beta \right)$-Pareto optimal solution [35,36]. It is easy to see that the stability of the parametric $\left(\alpha ,\beta \right)$-MOFTP model (14)–(17) implies the stability of the parametric FGP model which is defined as follows:

$$Min\hspace{1em}AF={\displaystyle \sum }_{q=1}^Q{w}_q^{-} d_q^{-}{,}_q$$

(47)

Subject to:

$$\left[L_q{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}\right]x_{ij}^{\left(q\right)}+D_q^{-}-D_q^{+}=\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right],$$

(48)

$${\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n-d_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}\le {\rho }^{\left(q\right)},\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,\hspace{1em}\forall i,j$$

(49)

$${\displaystyle \sum }_{j=1}^n{x}_{ij}\le \lambda a_i^L+\left(1-\lambda \right)a_i^U,\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(50)

$${\displaystyle \sum }_{i=1}^m{x}_{ij}\ge \lambda b_j^L+\left(1-\lambda \right)b_j^U,\hspace{1em}\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(51)

$$x_{ij}\ge 0,\hspace{1em}\lambda \in \left[0,1\right],\hspace{1em}\vartheta \in R,\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(52)

$$D_q^{-}\times D_q^{+}=0, \mathrm{and} D_q^{-}, D_q^{+}\ge 0,\hspace{1em}\hspace{1em}q=1,2,\dots ,Q.$$

(53)

6.1. KKT Optimality Conditions for Parametric FGP Model

The Lagrangian function of parametric FGP model (47)–(53) follows as [36,37]:

$$\begin{array}{ll}\mathit{L}=\left[{\displaystyle \sum _{q=1}^Q}{w}_q^{-} D_q^{-}\right]& + {\xi }_q\left[\left[L_q{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}{d}_{ij}^{\left(q\right)}\right]x_{ij}^{\left(q\right)}+D_q^{-}-D_q^{+}-\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right]\right]\\ & + {\upsilon }_q\left[{\displaystyle \sum _{i=1}^m}{\displaystyle \sum _{j=1}^n}-d_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}-{\rho }^{\left(q\right)}\right]+{\tau }_i\left[{\displaystyle \sum _{j=1}^n}{x}_{ij}-\left(\lambda a_i^L+\left(1-\lambda \right)a_i^U\right)\right]\\ & + {\eta }_j\left[-{\displaystyle \sum _{i=1}^m}{x}_{ij}+\left(\lambda b_j^L+\left(1-\lambda \right)b_j^U\right)\right]+{\phi }_{ij}\left[-x_{ij}\right]+{\psi }_i\left[-a_i^L\right]+{\varphi }_j\left[-b_j^L\right]+{\varpi }_i\left[-a_i^U\right]+{ϵ}_j\left[-b_j^U\right]\\ & + {\zeta }_q\left[-D_q^{-}\right]+{\pi }_q\left[-D_q^{+}\right],\end{array}$$

(54)

where $\xi ,\upsilon ,\tau ,\eta , \phi ,\psi ,\varphi ,\varpi , ϵ,\zeta \mathrm{and} \pi$ are the Lagrange multipliers. Thus, KKT optimality conditions [28,36,37,39] have the following form:

$$\begin{gathered} \frac{\partial L}{\partial x_{ij}}={\xi }_q\left[L_q{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}\right]+{\upsilon }_q\left[{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n-d_{ij}^{\left(q\right)}\right]+{\tau }_i-{\eta }_j-{\phi }_{ij}=0, \\ i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n, \end{gathered}$$

(55)

$$\frac{\partial L}{\partial a_i^L}=-\lambda {\tau }_i-{\psi }_i=0,\hspace{1em}i=1,2,\dots m,$$

(56)

$$\frac{\partial L}{\partial a_i^U}=-\left(1-\lambda \right){\tau }_i-{\varpi }_i=0,\hspace{1em}i=1,2,\dots m,$$

(57)

$$\frac{\partial L}{\partial b_j^L}=\lambda {\eta }_j-{\varphi }_j=0,\hspace{1em}\hspace{1em}\hspace{1em}i=1,2,\dots m,$$

(58)

$$\frac{\partial L}{\partial b_j^U}=\left(1-\lambda \right){\eta }_j-{ϵ}_j=0,\hspace{1em}i=1,2,\dots m,$$

(59)

$$\frac{\partial L}{\partial D_q^{-}}={\displaystyle \sum }_{q=1}^Q{w}_q^{-}+{\xi }_q+{\upsilon }_q-{\zeta }_q=0,\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(60)

$$\frac{\partial L}{\partial D_q^{+}}=-{\xi }_q-{\pi }_q=0,\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(61)

$$\left[L_q{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}-L_q^0{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n{d}_{ij}^{\left(q\right)}\right]x_{ij}^{\left(q\right)}+D_q^{-}-D_q^{+}-\left[L_q^0{\rho }^{\left(q\right)}-L_q{\delta }^{\left(q\right)}\right]=0,$$

(62)

$${\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n-d_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}-{\rho }^{\left(q\right)}\le 0,\hspace{1em}q=1,2,\dots ,Q,\hspace{1em}\forall i,j$$

(63)

$${\displaystyle \sum }_{j=1}^n{x}_{ij}-\left[\lambda a_i^L+\left(1-\lambda \right)a_i^U\right]\le 0,\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(64)

$$\left[\lambda b_j^L+\left(1-\lambda \right)b_j^U\right]-{\displaystyle \sum }_{i=1}^m{x}_{ij}\le 0,\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(65)

$$x_{ij}\ge 0,\hspace{1em}i=1,2,\dots ,m,\hspace{1em}j=1,2,\dots ,n,$$

(66)

$$D_{ij}^{-}, D_{ij}^{+}\ge 0,\hspace{1em}\hspace{1em}q=1,2,\dots ,Q,$$

(67)

$${\upsilon }_q\left[{\displaystyle \sum }_{i=1}^m{\displaystyle \sum }_{j=1}^n-d_{ij}^{\left(q\right)}{x}_{ij}^{\left(q\right)}+D_q^{-}-{\rho }^{\left(q\right)}\right]=0,\hspace{1em}q=1,2,\dots ,Q,\hspace{1em}\forall i,j$$

(68)

$${\tau }_i\left[{\displaystyle \sum }_{j=1}^n{x}_{ij}-\left(\lambda a_i^L+\left(1-\lambda \right)a_i^U\right)\right]=0,\hspace{1em}\hspace{1em}i=1,2,\dots ,m,$$

(69)

$${\eta }_j\left[-{\displaystyle \sum }_{i=1}^m{x}_{ij}+\left(\lambda b_j^L+\left(1-\lambda \right)b_j^U\right)\right]=0,\hspace{1em}\hspace{1em}j=1,2,\dots ,n,$$

(70)

$${\phi }_{ij}\left[x_{ij}\right]=0,$$

(71)

$${\psi }_i\left[a_i^L\right]=0,$$

(72)

$${\varphi }_j\left[b_j^L\right]=0,$$

(73)

$${\varpi }_i\left[a_i^U\right]=0,$$

(74)

$${ϵ}_j\left[b_j^U\right]=0,$$

(75)

$${\zeta }_q\left[D_q^{-}\right]=0,$$

(76)

$${\pi }_q\left[D_q^{+}\right]=0,$$

(77)

$$\upsilon ,\tau ,\eta , \phi ,\psi ,\varphi ,\varpi , ϵ,\zeta ,\pi \ge 0, \mathrm{and} \vartheta ,\xi \in R;$$

(78)

where the KKT conditions (55)–(78) are evaluated at $x_{ij}^{*}$. Solving the system of Equations (55)–(78), the SSFK ${\mathit{S}}_{\mathbf{1}}\left(x_{ij}^{*},\alpha ,\beta \right)$ for parametric IF-MOFTP is obtained.

6.2. Algorithm for Determination of the SSFK S₁ $(x_{ij}^{*},a,b)$

Following the above discussion, the algorithm for obtaining the SSFK ${\mathit{S}}_{\mathbf{1}}\left(x_{ij}^{*},\alpha ,\beta \right)$ for parametric $\left(\alpha ,\beta \right)$-MOFTP van be described as follows (Algorithms 1 and 2):

Algorithm 1 Phase I: Obtain an $\left(\alpha ,\beta \right)$-Pareto Optimal Solution of the Problem

1: Set the value of $\alpha ,$ and $\beta$. 2: Presume that $\vartheta ={\vartheta }^{*}$. 3: Calculate the sole maximum and minimum values of $Z_q\left(\mathit{x},{\vartheta }^{*}\right), q=1,2,\dots ,Q$. 4: Set the goals and the upper tolerance limits for $Z_q\left(\mathit{x},{\vartheta }^{*}\right), q=1,2,\dots ,Q.$ 5: Formulate ${\mu }_{\left(z_q\left(x,{\vartheta }^{*}\right)\right)}, q=1,2,\dots ,Q$ as in Equation (18). 6: Evaluate the weights $w_{ij}^{-}$ as defined in Equation (26). 7: Do the linearization procedures at $\vartheta ={\vartheta }^{*}$ for each parametric membership goal according to Equations (35)–(38). 8: Formulate and solve the FGP model (Equations (40)–(46)) to get $\left(\alpha ,\beta \right)$-Pareto optimal solution $x_{ij}^{*}$.

Algorithms 2 Phase II: Determination of the SSFK ${\mathit{S}}_{\mathbf{1}}(x_{ij}^{*},\alpha ,\beta )$

1: Formulate the parametric FGP model (Equations (47)–(53)). 2: Obtain the Lagrangian function, for the final FGP model, as in Equation (54). 3: Apply the KKT optimality conditions to find the SSFK (Equations (55)–(78)). 4: Reduce and solve the system of Equations (55)–(78), to obtain ${\mathit{S}}_{\mathbf{1}}(x_{ij}^{*},\alpha ,\beta )$ and stop.

7. Numerical Example

To demonstrate the proposed algorithm for finding the SSFK, consider the following parametric IF-MOFTP:

$$Max \left(\begin{array}{c}{Z}_1\left(\mathit{x},\vartheta \right)=\frac{\vartheta x_{11} + \left(2 + \vartheta \right)x_{12} + \left(3 + 2\vartheta \right)x_{21} + 6x_{22} + 4}{x_{11} + 3x_{12} + x_{21} + 2x_{22} + 2}, \\ Z_2\left(\mathit{x},\vartheta \right)=\frac{2x_{11} + \left(3 + \vartheta \right)x_{12} + \left(4 + 2\vartheta \right)x_{21} + \left(5 + \vartheta \right)x_{22} + 6}{x_{11} + 2x_{12} + 3x_{21} + x_{22}+ 4}\end{array}\right),$$

Subject to:

Supply constraints:

$$\begin{gathered} x_{11}+x_{12}\le {\tilde{a}}_1^I, \\ {x}_{21}+x_{22}\le {\tilde{a}}_2^I, \end{gathered}$$

Demand constraints:

$$\begin{gathered} x_{11}+x_{21}\ge {\tilde{b}}_1^I, \\ {x}_{12}+x_{22}\ge {\tilde{b}}_2^I, \end{gathered}$$

where the membership functions ${\mu }_{{\tilde{a}}_1^I}\left(x\right),{\mu }_{{\tilde{a}}_2^I}\left(x\right),{\mu }_{{\tilde{b}}_1^I}\left(x\right), {\mu }_{{\tilde{b}}_2^I}\left(x\right)$ and the non-membership functions ${\gamma }_{{\tilde{a}}_1^I}\left(x\right), {\gamma }_{{\tilde{a}}_2^I}\left(x\right), {\gamma }_{{\tilde{b}}_2^I}\left(x\right), {\gamma }_{{\tilde{b}}_2^I}\left(x\right)$ of the supplies and demands are described as follows:

$${\mu }_{{\tilde{a}}_1^I}\left(x\right)=\{\begin{array}{cc}\frac{x-140}{20}& if 140\le x\le 160,\\ \frac{180-x}{20}& if 160\le x\le 180,\\ 0& otherwise,\end{array} {\gamma }_{{\tilde{a}}_1^I}\left(x\right)=\{\begin{array}{cc}\frac{160-x}{30}& if 130\le x\le 160,\\ \frac{x-160}{40}& if 160\le x\le 200,\\ 1& otherwise,\end{array}$$

$${\mu }_{{\tilde{a}}_2^I}\left(x\right)=\{\begin{array}{cc}\frac{x-220}{20}& if 220\le x\le 240,\\ \frac{250-x}{10}& if 240\le x\le 250,\\ 0& otherwise,\end{array} {\gamma }_{{\tilde{a}}_2^I}\left(x\right)=\{\begin{array}{cc}\frac{240-x}{20}& if 210\le x\le 240,\\ \frac{x-240}{30}& if 240\le x\le 270,\\ 1& otherwise,\end{array}$$

$${\mu }_{{\tilde{b}}_1^I}\left(x\right)=\{\begin{array}{cc}\frac{x-40}{10}& if 40\le x\le 50,\\ \frac{60-x}{10}& if 50\le x\le 60,\\ 0& otherwise,\end{array} {\gamma }_{{\tilde{b}}_1^I}\left(x\right)=\{\begin{array}{cc}\frac{50-x}{20}& if 30\le x\le 50,\\ \frac{x-50}{30}& if 50\le x\le 80,\\ 1& otherwise,\end{array}$$

$${\mu }_{{\tilde{b}}_2^I}\left(x\right)=\{\begin{array}{cc}\frac{x-310}{10}& if 310\le x\le 320,\\ \frac{350-x}{30}& if 320\le x\le 350,\\ 0& otherwise,\end{array} {\gamma }_{{\tilde{b}}_2^I}\left(x\right)=\{\begin{array}{cc}\frac{320-x}{20}& if 300\le x\le 320,\\ \frac{x-320}{60}& if 320\le x\le 380,\\ 1& otherwise,\end{array}$$

Phase I: Finding an $\left(\alpha ,\beta \right)$-Pareto optimal solution of the parametric IF-MOFTP.

For a desired values of α = 0.6, and $\beta =0.2$, then applying the concept of $\left(\alpha ,\beta \right)$-cut of the IFN we formulate the $\left(\alpha ,\beta \right)$-MOFTP at $\vartheta ={\vartheta }^{*}=3$.

$$Max \left(\begin{array}{c}{Z}_1\left(\mathit{x}\right)=\frac{3x_{11}+ 5x_{12}+ 9x_{21}+ 6x_{22}+ 8}{x_{11}+ 3x_{12}+ x_{21}+ 2x_{22}+ 2}, \\ Z_2\left(\mathit{x}\right)=\frac{2x_{11}+ 6x_{12}+ 10x_{21}+ 8x_{22}+ 6}{x_{11}+ 2x_{12}+ 3x_{21}+ x_{22}+ 4}\end{array}\right),$$

Subject to:

Supply constraints:

$$x_{11}+x_{12}\le \left[154, 168\right],x_{21}+x_{22}\le \left[234, 244\right].$$

Demand constraints:

$$x_{11}+x_{21}\ge \left[46, 54\right],x_{12}+x_{22}\ge \left[316, 332\right].$$

Based on the concept of convex linear combination on the constraints, then we obtain the MOFTP:

$$Max \left(\begin{array}{c}{Z}_1\left(\mathit{x}\right)=\frac{3x_{11}+ 5x_{12}+ 9x_{21}+ 6x_{22}+ 8}{x_{11}+ 3x_{12}+ x_{21}+2x_{22}+ 2}, \\ Z_2\left(\mathit{x}\right)=\frac{2x_{11}+ 6x_{12}+ 10x_{21}+ 8x_{22}+ 6}{x_{11}+ 2x_{12}+ 3x_{21}+ x_{22}+ 4}\end{array}\right),$$

Subject to:

$$x_{11}+x_{12}\le 165.2, x_{21}+x_{22}\le 240, x_{11}+x_{21}\ge 51.6, x_{12}+x_{22}\ge 328.8.$$

A FGP approach is utilized to solve the MOFTP according to the model of Equations (40)–(46). Firstly, the coefficients of the linearized membership goals are obtained in

Table 1. The coefficient of the linearized membership goals ${\left({\mathit{c}}^{ij}\right)}^T$ and G_ij.

	Z1(x)	Z2(x)
${\left(c_{ij}^q\right)}^T$	${\left(\begin{array}{c}0.682\\ -10.22\\ 19.081\\ 1.364\end{array}\right)}^T$	${\left(\begin{array}{c}-2.8628\\ -4.048\\ -5.234\\ 2.1688\end{array}\right)}^T$
Gij	−7.497	13.128

.

$$Min AF=3.0665D_1^{-}+0.8386D_2^{-},$$

Subject to:

$$0.682x_{11}-10.22x_{12}+19.081x_{21}+1.364x_{22}+D_1^{-}-D_1^{+}=-7.497,$$

$$-2.8628x_{11}-4.048x_{12}-5.234x_{21}+2.169x_{22}+D_2^{-}-D_2^{+}=13.128,$$

$$-x_{11}-3x_{12}-x_{21}-2x_{22}+D_1^{-}\le 2,$$

$$-x_{11}-2x_{12}-3x_{21}-x_{22}+D_2^{-}\le 4,$$

$$x_{11}+x_{12}\le 165.2,$$

$$x_{21}+x_{22}\le 240,$$

$$x_{11}+x_{21}\ge 51.6,$$

$$x_{12}+x_{22}\ge 328.8,$$

$$x_{11},x_{12},x_{21},x_{22}, D_1^{-}, D_1^{+},D_2^{-}, D_2^{+}\ge 0.$$

Using Lingo programming, the $\left(\alpha ,\beta \right)$-Pareto optimal solution of the parametric IF-MOFTP is obtained at $\left(x_{11}^{*},x_{12}^{*},x_{21}^{*},x_{22}^{*}, D_1^{-}, D_1^{+},D_2^{-}, D_2^{+}\right)=\left(0,165.88, 76.39, 163.61, 0, 0, 726.78, 0\right)$.

Phase II: determination of the SSFK ${\mathit{S}}_{\mathbf{1}}\left({\mathit{x}}^{*},\alpha ,\beta \right)$.

To determine the SSFK ${\mathit{S}}_{\mathbf{1}}\left({\mathit{x}}^{*},a,b\right)$ of the parametric IF-MOFTP, the coefficients of the linearized membership goals in the parametric form are recalculated in

Table 2. The coefficients of the linearized membership goals ${\left[{\left(c_{ij}+\vartheta {\omega }_{ij}\right)}^{\left(q\right)}\right]}^T and {\mathit{G}}_{\mathit{i}\mathit{j}}$.

	${\mathit{Z}}_{\mathbf{1}}\left(\mathit{x},\mathit{\vartheta }\right)$	${\mathit{Z}}_{\mathbf{2}}\left(\mathit{x},\mathit{\vartheta }\right)$
${\left[{\left({\mathit{c}}_{\mathit{i}\mathit{j}}+\mathit{\vartheta }{\mathit{\omega }}_{\mathit{i}\mathit{j}}\right)}^{\left(\mathit{q}\right)}\right]}^{\mathit{T}}$	${\left(\begin{array}{c}-\mathbf{8.518}+\mathbf{3.067}\mathit{\vartheta }\\ -\mathbf{19.42}+\mathbf{3.067}\mathit{\vartheta }\\ \mathbf{0.682}+\mathbf{6.133}\mathit{\vartheta }\\ \mathbf{1.364}\end{array}\right)}^{\mathit{T}}$	${\left(\begin{array}{c}-\mathbf{2.863}\\ -\mathbf{6.564}+\mathbf{0.839}\mathit{\vartheta }\\ -\mathbf{10.266}+\mathbf{1.677}\mathit{\vartheta }\\ -\mathbf{0.347}+\mathbf{0.839}\mathit{\vartheta }\end{array}\right)}^{\mathit{T}}$
Gij	−7.497	13.128

.

Therefore, the stability of parametric IF-MOFTP implies the stability of the parametric FGP model which is defined as:

$$Min AF=3.067D_1^{-}+0.839D_2^{-},$$

Subject to:

$$\left(-8.518+3.067\vartheta \right)x_{11}+\left(-19.42+3.067\vartheta \right)x_{12}+\left(0.682+6.133\vartheta \right)x_{21}+1.364x_{22}$$

$$+D_1^{-}-D_1^{+}=-7.497,$$

$$-2.8628x_{11}+\left(-6.564+0.839\vartheta \right)x_{12}+\left(-10.266+1.677\vartheta \right)x_{21}$$

$$+\left(-0.347+0.839\vartheta \right)x_{22}+D_2^{-}-D_2^{+}=13.128,$$

$$-x_{11}-3x_{12}-x_{21}-2x_{22}+D_1^{-}\le 2,$$

$$-x_{11}-2x_{12}-3x_{21}-x_{22}+D_2^{-}\le 4,$$

$$x_{11}+x_{12}\le 0.2a_1^L+0.8a_1^U,$$

$$x_{21}+x_{22}\le 0.4a_2^L+0.6a_2^U,$$

$$x_{11}+x_{21}\ge 0.3b_1^L+0.7b_1^U,$$

$$x_{12}+x_{22}\ge 0.2b_2^L+0.8b_2^U,$$

$$x_{11},x_{12},x_{21},x_{22}, a_1^L,a_1^U,a_2^L,a_2^U, b_1^L,b_1^U,b_2^L,b_2^U\ge 0,$$

$$D_1^{-}, D_1^{+},D_2^{-}, D_2^{+}\ge 0; \vartheta \in R$$

The Lagrangean function of the above parametric FGP model follows as:

$$\begin{array}{l}\mathit{L}\\ = 3.067D_1^{-}+0.839D_2^{-}+{\xi }_1\left[\begin{array}{c}\left(-8.518+3.067\vartheta \right)x_{11}+\left(-19.42+3.067\vartheta \right)x_{12} \\ +\left(0.682+6.133\vartheta \right)x_{21}+1.364x_{22}+D_1^{-}-D_1^{+}+7.497\end{array}\right]\\ + {\xi }_2\left[\begin{array}{c}-2.8628x_{11}+\left(-6.564+0.839\vartheta \right)x_{12}+\left(-10.266+1.677\vartheta \right)x_{21}\\ +\left(-0.347+0.839\vartheta \right)x_{22}+D_2^{-}-D_2^{+}-13.128 \end{array}\right]\\ + {\vartheta }_1\left[-x_{11}-3x_{12}-x_{21}-2x_{22}+D_1^{-}-2\right]+{\vartheta }_2\left[-x_{11}-2x_{12}-3x_{21}-x_{22}+D_2^{-}-4\right]\\ + {\tau }_1\left[x_{11}+x_{12}-0.2a_1^L-0.8a_1^U\right]+{\tau }_2\left[x_{21}+x_{22}-0.4a_2^L-0.6a_2^U\right]\\ + {\eta }_1\left[-x_{11}-x_{21}+0.3b_1^L+0.7b_1^U\right]+{\eta }_2\left[-x_{12}-x_{22}+0.2b_2^L+0.8b_2^U\right]+{\phi }_1\left[-x_{11}\right]\\ + {\phi }_2\left[-x_{12}\right]+{\phi }_3\left[-x_{21}\right]+{\phi }_4\left[-x_{22}\right]+{\psi }_1\left[-a_1^L\right]+{\psi }_2\left[-a_2^L\right]+{\varphi }_1\left[-b_1^L\right]+{\varphi }_2\left[-b_2^L\right]\\ + {\varpi }_1\left[-a_1^U\right]+{\varpi }_2\left[-b_2^U\right]+{ϵ}_1\left[-b_1^U\right]+{ϵ}_2\left[-b_2^U\right]+{\zeta }_1\left[-D_1^{-}\right]+{\zeta }_2\left[-D_2^{-}\right]+{\pi }_1\left[-D_1^{+}\right]\\ + {\pi }_2\left[-D_2^{+}\right]\end{array}$$

where $\vartheta ,{\xi }_1,{\xi }_2\in R,$ and ${\upsilon }_1,{\upsilon }_2,{\tau }_1,{\tau }_2,{\eta }_1,{\eta }_2,{\phi }_1,{\phi }_2,{\phi }_3,{\phi }_4,{\psi }_1,{\psi }_2,{\varphi }_1,{\varphi }_2,{\varpi }_1,{\varpi }_2,{ϵ}_1,{ϵ}_2\ge 0,$ and ${\zeta }_1,{\zeta }_2,{\pi }_1,{\pi }_2\ge 0$, are the Lagrange multipliers. Therefore, KKT optimality conditions follows as:

$$\frac{\partial \mathit{L}}{\partial x_{11}}=\left(-8.518+3.067\vartheta \right){\xi }_1-2.863{\xi }_2-{\upsilon }_1-{\upsilon }_2+{\tau }_1-{\eta }_1-{\phi }_1=0$$

$$\frac{\partial \mathit{L}}{\partial x_{12}}=\left(-19.42+3.067\vartheta \right){\xi }_1+\left(-6.564+0.839\vartheta \right){\xi }_2-3{\upsilon }_1-2{\upsilon }_2+{\tau }_1-{\eta }_2-{\phi }_2=0,$$

$$\frac{\partial \mathit{L}}{\partial x_{21}}=\left(0.682+6.133\vartheta \right){\xi }_1+\left(-10.266+1.677\vartheta \right){\xi }_2-{\upsilon }_1-3{\upsilon }_2+{\tau }_2-{\eta }_1-{\phi }_3=0,$$

$$\frac{\partial \mathit{L}}{\partial x_{22}}=1.364{\xi }_1+\left(-0.347+0.839\vartheta \right){\xi }_2-2{\upsilon }_1-{\upsilon }_2+{\tau }_2-{\eta }_2-{\phi }_4=0,$$

$$\frac{\partial \mathit{L}}{\partial a_1^L}=-0.2{\tau }_1-{\psi }_1=0,$$

$$\frac{\partial \mathit{L}}{\partial a_1^U}=-0.8{\tau }_1-{\varpi }_1=0,$$

$$\frac{\partial \mathit{L}}{\partial a_2^L}=-0.4{\tau }_2-{\psi }_2=0,$$

$$\frac{\partial \mathit{L}}{\partial a_2^U}=-0.6{\tau }_2-{\varpi }_2=0,$$

$$\frac{\partial \mathit{L}}{\partial b_1^L}=0.3{\eta }_1-{\varphi }_1=0,$$

$$\frac{\partial \mathit{L}}{\partial b_1^U}=0.7{\eta }_1-{ϵ}_1=0,$$

$$\frac{\partial \mathit{L}}{\partial b_2^L}=0.2{\eta }_2-{\varphi }_2=0,$$

$$\frac{\partial \mathit{L}}{\partial b_2^U}=0.8{\eta }_2-{ϵ}_2=0,$$

$$\frac{\partial \mathit{L}}{\partial D_1^{-}}=3.067+{\xi }_1+v_1-{\zeta }_1=0,$$

$$\frac{\partial \mathit{L}}{\partial D_1^{+}}=-{\xi }_1-{\pi }_1=0,$$

$$\frac{\partial \mathit{L}}{\partial D_2^{-}}=0.839+{\xi }_2+{\upsilon }_2-{\zeta }_2=0,$$

$$\frac{\partial \mathit{L}}{\partial D_2^{+}}=-{\xi }_2-{\pi }_2=0,$$

$${\upsilon }_1\left[-x_{11}-3x_{12}-x_{21}-2x_{22}+D_1^{-}-2\right]=0, \text{i.e.}, {\upsilon }_1=0,$$

$${\upsilon }_2\left[-x_{11}-2x_{12}-3x_{21}-x_{22}+D_2^{-}-4\right]=0, \text{i.e.}, {\upsilon }_2=0,$$

$${\tau }_1\left[x_{11}+x_{12}-0.2a_1^L-0.8a_1^U\right]=0, \text{i.e.}, {\tau }_1=0,$$

$${\tau }_2\left[x_{21}+x_{22}-0.4a_2^L-0.6a_2^U\right]=0, \text{i.e.}, {\tau }_2\ge 0,$$

$${\eta }_1\left[-x_{11}-x_{21}+0.3b_1^L+0.7b_1^U\right]=0, \text{i.e.}, {\eta }_1=0,$$

$${\eta }_2\left[-x_{12}-x_{22}+0.2b_2^L+0.8b_2^U\right]=0, \text{i.e.}, {\eta }_2=0,$$

$${\phi }_1\left[-x_{11}\right]=0, \text{i.e.}, {\phi }_1\ge 0,$$

$${\phi }_2\left[-x_{12}\right]=0, \text{i.e.}, {\phi }_2=0,$$

$${\phi }_3\left[-x_{21}\right]=0, \text{i.e.}, {\phi }_3=0,$$

$${\phi }_4\left[-x_{22}\right]=0, \text{i.e.}, {\phi }_4=0,$$

$${\psi }_1\left[-a_1^L\right]=0, \text{i.e.}, {\psi }_1=0,$$

$${\psi }_2\left[-a_2^L\right]=0, \text{i.e.}, {\psi }_2=0,$$

$${\varphi }_1\left[-b_1^L\right]=0, \text{i.e.}, {\varphi }_1=0,$$

$${\varphi }_2\left[-b_2^L\right]=0, \text{i.e.}, {\varphi }_2=0,$$

$${\varpi }_1\left[-a_1^U\right]=0, \text{i.e.}, {\varpi }_1=0,$$

$${\varpi }_2\left[-a_2^U\right]=0, \text{i.e.}, {\varpi }_2=0,$$

$${ϵ}_1\left[-b_1^U\right]=0, \text{i.e.}, {ϵ}_1=0,$$

$${ϵ}_2\left[-b_2^U\right]=0, \text{i.e.}, {ϵ}_2=0,$$

$${\zeta }_1\left[-D_1^{-}\right]=0, \text{i.e.},{\zeta }_1\ge 0,$$

$${\zeta }_2\left[-D_2^{-}\right]=0, \text{i.e.}, {\zeta }_2=0,$$

$${\pi }_1\left[-D_1^{+}\right]=0, \text{i.e.}, {\pi }_1\ge 0,$$

$${\pi }_2\left[-D_2^{+}\right]=0, \text{i.e.}, {\pi }_2\ge 0,$$

$$-x_{11}-3x_{12}-x_{21}-2x_{22}+D_1^{-}\le 2,$$

$$-x_{11}-2x_{12}-3x_{21}-x_{22}+D_2^{-}\le 4,$$

$$x_{11}+x_{12}\le 0.2a_1^L+0.8a_1^U,$$

$$x_{21}+x_{22}\le 0.4a_2^L+0.6a_2^U,$$

$$x_{11}+x_{21}\ge 0.3b_1^L+0.7b_1^U,$$

$$x_{12}+x_{22}\ge 0.2b_2^L+0.8b_2^U,$$

$$x_{11},x_{12},x_{21},x_{22}, a_1^L,a_1^U,a_2^L,a_2^U, b_1^L,b_1^U,b_2^L,b_2^U,D_1^{-}, D_1^{+},D_2^{-}, D_2^{+}\ge 0; \vartheta \in R$$

Solving the above system of Equation. we get: ${\upsilon }_1={\upsilon }_2={\tau }_1={\tau }_2={\eta }_1={\eta }_2={\phi }_2={\phi }_3={\phi }_4={\psi }_1={\psi }_2={\varphi }_1={\varphi }_2={\varpi }_1={\varpi }_2={ϵ}_1={ϵ}_2={\zeta }_2=0,$ and ${\phi }_1,{\zeta }_1,{\pi }_1,{\pi }_2\ge 0$. Also, ${\xi }_2=-{\pi }_2=-0.839, {\xi }_1=-{\pi }_1$. The above system of Equation is reduced to the following:

$$\left(-8.518+3.067\vartheta \right){\xi }_1-2.863{\xi }_2-{\phi }_1=0,$$

$$\left(-19.42+3.067\vartheta \right){\xi }_1+\left(-6.564+0.839\vartheta \right){\xi }_2=0,$$

$$\left(0.682+6.133\vartheta \right){\xi }_1+\left(-10.266+1.677\vartheta \right){\xi }_2=0,$$

$$1.364{\xi }_1+\left(-0.347+0.839\vartheta \right){\xi }_2=0,$$

Therefore, the SSFK for the parametric IF-MOFTP is given by:

$$\begin{array}{l}{\mathit{S}}_{\mathbf{1}}\left(0,165.88, 76.39, 163.61, 0, 0, 726.78, 0\right)\\ \hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em}\hspace{1em} =\left\{\begin{array}{c}\vartheta \in R, \\ \alpha ,\beta \in \left[0,1\right]\end{array}|\begin{array}{c}12.948 {\xi }_1+\left[-1.41+6.133{\xi }_1\right]\vartheta +5.799-{\phi }_1=0,\\ {\xi }_1={\zeta }_1-3.67; {\xi }_1=-{\pi }_1; {\xi }_2=-{\pi }_2=-0.839,\\ {\zeta }_1, {\phi }_1,{\pi }_1,{\pi }_2\ge 0; {\xi }_1,{\xi }_2\in R\end{array}\right\}\end{array}$$

After applying the KKT optimality conditions we obtain a large system of algebraic equations. By reducing and solving the algebraic system of equations the SSFK is obtained. The SSFK introduces the values and relations between different parameters which generate the same solution of the PIF-MOFTP as indicated by set ${\mathit{S}}_1$. To test the obtained results of the SSFK, different values of $\alpha ,\beta \in \left[0,1\right]$ will be taken and the solution will remain the same.

8. Conclusions

The SSFK for the PIF-MOFTP was investigated in this study. Also, we characterized definitions of the set of feasible parameters and the solvability set for PIF-MOFTP. First, the concept of $\left(\alpha ,\beta \right)$-cut methodology was applied to get the parametric model. Moreover, the FGP approach was applied to find a $\left(\alpha ,\beta \right)$-Pareto optimal solution for PIF-MOFTP which has not been published in the literature to date. To obtain the SSFK for the novel model of PIF-MOFTP, the KKT necessary optimality conditions are applied. After applying the KKT optimality conditions, we obtained a large system of algebraic equations. By reducing and solving the algebraic system of equations, the SSFK was obtained. A detailed procedure that determines the SSFK for the PIF-MOFTP was exhibited. A numerical example was given to ensure the applicability and efficiency of the proposed PIF-MOFTP.

The major limitation of the proposed PIF-MOFTP is that a specific $\left(\alpha ,\beta \right)$-level is adopted in the proposed methods to represent the confidence level on DMs’ subjective uncertainty to specify parameter values in the PIF-MOFTP. For simplification, the $\left(\alpha ,\beta \right)$-level for all parameters of the supply and demand in the solution process are assumed to be the same. However, these may be limitations in practical applications. The determination of $\left(\alpha ,\beta \right)$-levels for various DMs’ subjective uncertainties could be different in the real world due to DMs’ different consideration of the real transportation data. Thus, this will be addressed in future studies.

Several remaining areas of research in the topic of parametric MOFTP include the following:

• The parametric study of multi-choice MOTP should be addressed.
• Real-world PIF-MOFTP is a vital field in the future research.
• Rough parametric MOFTP is a vital topic to be investigated.