Chirped Periodic and Solitary Waves for Improved Perturbed Nonlinear Schrödinger Equation with Cubic Quadratic Nonlinearity

Abstract

In this paper, we study the improved perturbed nonlinear Schrödinger equation with cubic quadratic nonlinearity (IPNLSE-CQN) to describe the propagation properties of nonlinear periodic waves (PW) in fiber optics. We obtain the chirped periodic waves (CPW) with some Jacobi elliptic functions (JEF) and also obtain some solitary waves (SW) such as dark, bright, hyperbolic, singular and periodic solitons. The nonlinear chirp associated with each of these optical solitons was observed to be dependent on the pulse intensity. The graphical behavior of these waves will also be displayed.

1. Introduction

A chirp is a signal whose frequency changes over time. The chirping sound generated by birds is the source of the term chirp. For example, ultrashort pulses in optical transmission systems may show chirp, which interacts with the dispersion characteristics of the materials through which they propagate to increase or reduce total pulse dispersion. The propagation of chirped soliton pulses in fiber optics is getting popular due to a wide range of applications in amplification and pulse compression. As a result, these objects are especially useful in the design of optical pulse compressors, fiber-optic amplifiers, and soliton-based communications links [1,2]. The NLSE is used to describe these pulses, and it only includes the effects of group velocity dispersion (GVD) and self-phase modulation that are valid in the picosecond region. Higher-order effects such as self-steeping (SS), self-frequency (SF) shift, and quintic nonlinearity can occur when optical pulses are short (in the femtosecond region). Models with higher-order terms governing most practical cases, unlike the NLSE, are not entirely integrable and cannot be solved correctly by the well-known inverse scattering transform [3,4,5,6,7,8,9,10,11,12,13,14,15,16,17].

Recently, many researchers have studied the chirped soliton-like solutions for various NLSE, such as Alka et al. who studied chirped solitons for NLSE with SS and SF shift effect along with quintic nonlinearity [18]. Vysa et al. studied the NLSE with SS and SF shift effect in order to obtain the chirped chiral solitons [19]. For a higher-order NLSE with competing cubic-quintic-septic nonlinearities, non-Kerr quintic nonlinearity, SS, and SF shift, Bouzida et al. obtained families of chirped soliton-like solutions. Hmurcik and Kaup used numerical methods to investigate a pulse with a linear chirp and a hyperbolic-secant-amplitude profile [20]. It was also demonstrated that there are exact chirped soliton solutions for the generalised NLSE with polynomial nonlinearity and non-Kerr terms of arbitrary order [21]. In addition, the chirped solitary pulses for a nonic NLSE were studied on a continuous-wave background [5,22,23,24,25,26,27,28,29,30,31]. In this paper, we studied the IPNLSE-CQN in order to obtain some chirped periodic and soliton waves. We also show that the resultant chirp associated with each of these optical solitons, which includes both linear and nonlinear terms, where nonlinear terms is directly and inversely proportional to the wave’s intensity.

This paper is organized as follows; In Section 2, we will investigate the mathematical analysis of our governing model. We will present CPW in Section 3. In Section 4, we will present the SW solution. In Section 5, we will discuss our results. In the end, we will conclude our results in Section 6.

2. Mathematical Analysis

The IPNLSE-CQN dimensionless form is given by [32,33,34,35]:

$$i{{\rm Y}}_t+a{{\rm Y}}_{tx}+b{{\rm Y}}_{xx}+(c_1\mid {\rm Y}\mid +c_2{\mid {\rm Y}\mid }^2){\rm Y}=i\{\alpha {{\rm Y}}_x+\lambda {({\mid {\rm Y}\mid }^{2p}{\rm Y})}_x+\vartheta {({\mid {\rm Y}\mid }^{2p})}_x{\rm Y}\},$$

(1)

Here a, b, $\alpha$, $\lambda$ and $\upsilon$ stand for spatio-temporal dispersion (STD), GVD, inter-modal dispersion, SS perturbation term and nonlinear dispersion respectively. Where $c_1$ and $c_2$ are constant. The parameter p is the full nonlinearity parameter. By putting the $p=1$, so that Equation (1) reduce to:

$$i{{\rm Y}}_t+a{{\rm Y}}_{tx}+b{{\rm Y}}_{xx}+(c_1\mid {\rm Y}\mid +c_2{\mid {\rm Y}\mid }^2){\rm Y}=i\{\alpha {{\rm Y}}_x+\lambda {({\mid {\rm Y}\mid }^2{\rm Y})}_x+\vartheta {({\mid {\rm Y}\mid }^2)}_x{\rm Y}\},$$

(2)

In order to obtain the PW solution of Equation (2), we consider the following transformation:

$${\rm Y}(x,t)=J\left(\gamma \right)exp\left[i\right(K\left(\gamma \right)-\Omega t\left)\right],$$

(3)

where $\gamma =x-vt$ and $J\left(\gamma \right)$ and $K\left(\gamma \right)$ are real functions of $\gamma$, while w is the wave number constant $(w>0)$. The chirping that corresponds to this waveform can be expressed as:

$$\delta {\rm Y}(x,t)=-\frac{\partial }{\partial x}[K\left(\gamma \right)-\Omega t]=-K^{\prime }\left(\gamma \right),$$

(4)

Now insert Equation (3) into Equation (2). Then, we get the real and imaginary parts that can be written as:

$$(b-av)J^{″}+(av-b)J{K}^{\prime 2}+c_1{J}^2+c_2{J}^3+(v+a\Omega +\alpha +\lambda J^2)J{K}^{\prime }+J\Omega =0,$$

(5)

and

$$(b-av)J{K}^{″}+2(b-av)J^{\prime }{K}^{\prime }-(3\lambda +2\vartheta )J^2{J}^{\prime }-(v+a\Omega +\alpha )J^{\prime }=0,$$

(6)

Now multiply Equation (6) with $J\left(\gamma \right)$ and integration yield,

$$K^{\prime }=\frac{A_1}{(b-av)J^2}+\frac{(3\lambda +2\vartheta )J^2}{4(b-av)}+\frac{v+a\Omega +\alpha }{2(b-av)},$$

(7)

where $A_1$ is the integration constant. Hence, the resultant chirping can be expressed as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)J^2}-\frac{(3\lambda +2\vartheta )J^2}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(8)

by substitution of Equation (7) into Equation (5), as the required differential equation can be shown as:

$$(b-av)J^{″}+\frac{(3\lambda +2\vartheta )(\lambda -2\vartheta )J^5}{16(b-av)}+\frac{(2c_2(b-av)+(v+a\Omega +\alpha )\lambda )J^3}{2(b-av)}+c_1{J}^2$$

(9)

$$+\frac{(4\Omega (b-av)-2A_1(\lambda +2\vartheta )+{(v+a\Omega +\alpha )}^2)J}{4(b-av)}-\frac{{A_1}^2}{(b-av)J^3}=0,$$

(10)

divide by $(b-av)$ on both side of the Equations (9) and (10), as:

$$J^{″}+\frac{(3\lambda +2\vartheta )(\lambda -2\vartheta )J^5}{16{(b-av)}^2}+\frac{(2c_2(b-av)+(v+a\Omega +\alpha )\lambda )J^3}{2{(b-av)}^2}+\frac{c_1{J}^2}{b-av}$$

(11)

$$+\frac{(4\Omega (b-av)-2A_1(\lambda +2\vartheta )+{(v+a\Omega +\alpha )}^2)J}{4{(b-av)}^2}-\frac{{A_1}^2}{{(b-av)}^2{J}^3}=0,$$

(12)

multiply the Equations (11) and (12) by $2J^{\prime }d\gamma$ and integration;

$${\left(J^{\prime }\right)}^2+\frac{(3\lambda +2\vartheta )(\lambda -2\vartheta )J^6}{48{(b-av)}^2}+\frac{(2c_2(b-av)+(v+a\Omega +\alpha )\lambda )J^4}{4{(b-av)}^2}+\frac{2c_1{J}^3}{3(b-av)}$$

(13)

$$+\frac{(4\Omega (b-av)-2A_1(\lambda +2\vartheta )+{(v+a\Omega +\alpha )}^2)J^2}{4{(b-av)}^2}-\frac{{A_1}^2}{{(b-av)}^2{J}^2}+2A_2=0,$$

(14)

where $A_2$ is an another integration constant. Now after substituting $\Psi =J^2$ into Equations (13) and (14) we get the equation:

$${\left({\Psi }^{\prime }\right)}^2+V(\Psi )=0,$$

(15)

where $V(\Psi )$ is expressed as:

$$V(\Psi )=\eta {\Psi }^4+\mu {\Psi }^3+\sigma {\Psi }^{\frac{5}{2}}+\xi {\Psi }^2+\epsilon \Psi +H,$$

(16)

with the coefficients:

$$\eta =\frac{(3\lambda +2(\lambda \vartheta -2\vartheta \left)\right)}{12{(b-av)}^2}, \mu =\frac{2c_2(b-av)+(v+a\Omega +\alpha )\lambda }{{(b-av)}^2)},$$

(17)

$$\sigma =\frac{2c_2}{3(b-av)}, \xi =\frac{4\Omega (b-av)-2A_1(\lambda +2\vartheta )-{(v+a\Omega +\alpha )}^2}{{(b-av)}^2},$$

(18)

$$\epsilon =8A_2, H=\frac{{4A_1}^2}{{(b-av)}^2},$$

(19)

Equation (15) shows the dynamics of partial with energy and potentials. The general wave solution of Equation (2) is:

$$\begin{array}{c}\hfill {\rm Y}(x,t)=\sqrt{\Psi \left(\gamma \right)}exp\left[i(K\left(\gamma \right)-\Omega t)\right],\end{array}$$

(20)

where $\Psi \left(\gamma \right)$ satisfies the Equation (15) and $K\left(\gamma \right)$ can be obtained with the help of Equation (7). Now using these relations into Equation (8) we can obtained chirping function $\delta {\rm Y}(x,t)$ as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Psi \left(\gamma \right)}-\frac{(3\lambda +2\vartheta )\Psi \left(\gamma \right)}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(21)

Hence, it is clear that the structure of the Equation (21) is nontrivial, which contains the first and second term with intensity dependent terms while the last term is linear. In Equation (21), it is clear that the first and second term represent the nonlinear chirp that is inversely and directly proportional to the intensity and the last term is linear chirp. Now, we have to obtain the chirped solutions for Equation (21) with the condition that $\Psi \left(\gamma \right)\ne 0$ along with $A_1\ne 0$ and under some constrained conditions.

3. CPW Solution

To investigate the different forms of elliptic ordinary differential equations in Equation (15), we have to obtain the exact CPW solutions of Equation (2) by using the transformation Equation (20). We also express our result in terms of JEF. The more results shows in Figure 1, Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13, Figure 14, Figure 15, Figure 16, Figure 17, Figure 18, Figure 19 and Figure 20.

3.1. cn-Form

We can obtain the chirped periodic solution of Equation (15) for $cn$-form that are represented as [36]:

$$\begin{array}{c}\hfill \Psi \left(\gamma \right)=D\{1\pm Lcn[\varphi (\gamma -{\gamma }_o),w]\},\end{array}$$

(22)

where ${\gamma }_o$ is the constant and $cn(x,w)$ is JEF with modulus w containing values $0<w<1$. The variables D and L are shown below:

$$D=-\frac{\mu }{4\eta }, L=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(23)

We can also find the following values:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(24)

Periodic solutions of $cn$-form for Equation (2) obtained by substituting the equations Equations (20) and (22):

$${\rm Y}(x,t)={\left\{D[1\pm Lcn(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(25)

where $ϵ=x-vt-{\gamma }_o$. By equating the Equations (19) and (24), we can get integration constant $A_1$ and $A_2$:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(26)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(27)

The resultant chirping can be written as:

$$\begin{array}{c}\hfill \delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)D\{1\pm Lcn[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )D\{1\pm Lcn[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},\end{array}$$

(28)

3.2. dc-Form

Another periodic solution of Equation (15) can be obtained in this way [36]:

$$\Psi \left(\gamma \right)=Z\{1\pm Ydc[\varphi (\gamma -{\gamma }_o),w]\},$$

(29)

where ${\gamma }_o$ is the constant and $dc(x,w)$ is JEF with modulus w taking values $0<w<1$. The values of V and Y are expressed as:

$$V=-\frac{\mu }{4\eta }, Y=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(30)

After solving the above, we also obtained the following values:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(31)

by putting the equations Equation (29) in Equation (20), as we get the periodic solutions of $dc$-form for Equation (2) as:

$${\rm Y}(x,t)={\left\{V[1\pm Ydc(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(32)

where $ϵ=x-vt-{\gamma }_o$. By inserting the Equation (19) into Equation (31), we can obtain the integration constant $A_1$ and $A_2$:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(33)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(34)

By putting Equation (29) in Equation (21), we can get the resultant chirping as follow:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)V\{1\pm Ydc[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )V\{1\pm Ydc[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(35)

3.3. dn-Type

We obtain the periodic solution of $dn$-form [36]:

$$\Psi \left(\gamma \right)=R\{1\pm Fdn[\varphi (\gamma -{\gamma }_o),w]\},$$

(36)

where ${\gamma }_o$ is the constant and $dn(x,w)$ is JEF with modulus w having values $0<w<1$. The parameters R and F are defined as:

$$R=-\frac{\mu }{4\eta }, F=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(37)

we can also find $\epsilon$ and H:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(38)

Insert the equation Equation (36) in Equation (20), so we can find the periodic solutions of $dn$-form for Equation (2) as:

$${\rm Y}(x,t)={\left\{R[1\pm Fdn(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(39)

where $ϵ=x-vt-{\gamma }_o$. We can also find the integration constant $A_1$ and $A_2$:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(40)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(41)

Insert the Equation (36) in Equation (21), so we can obtain the resultant chirping takes form:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)R\{1\pm Fdn[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )R\{1\pm Fdn[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(42)

3.4. ds-Type

The PW solution of $ds$-form are shown below [36]:

$$\Psi \left(\gamma \right)=E\{1\pm Mds[\varphi (\gamma -{\gamma }_o),w]\},$$

(43)

where ${\gamma }_o$ is the constant and $ds(x,w)$ is JEF with modulus w having values $0<w<1$. The parameters E and M are represented as:

$$E=-\frac{\mu }{4\eta }, M=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(44)

we can also get the $\epsilon$ and H:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(45)

We can get the periodic solutions of $ds$-form for Equation (2) by putting the Equation (43) in Equation (20):

$${\rm Y}(x,t)={\left\{E[1\pm Mds(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(46)

where $ϵ=x-vt-{\gamma }_o$. The integration constant $A_1$ and $A_2$ are represented as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(47)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(48)

The chirping that associated with this exact PW can be written as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)E\{1\pm Mds[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )E\{1\pm Mds[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(49)

3.5. sc-Type

Equation (15) can be solved for the periodic solution of $sc$-form that can be present as [36]:

$$\Psi \left(\gamma \right)=G\{1\pm Bsc[\varphi (\gamma -{\gamma }_o),w]\},$$

(50)

where ${\gamma }_o$ is the constant and $sc(x,w)$ is JEF with modulus w having values $0<w<1$. The parameters G and B are present as:

$$E=-\frac{\mu }{4\eta }, M=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(51)

we can also find the following parameters:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(52)

We can find the periodic solutions of $sc$-form for Equation (2) by combining the solution of the Equation (50) in Equation (20):

$${\rm Y}(x,t)={\left\{G[1\pm Bsc(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(53)

where $ϵ=x-vt-{\gamma }_o$. The constant $A_1$ and $A_2$ are as follow:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(54)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(55)

By combining the solution of Equations (21) and (50), since the corresponding chirping are shown below:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)G\{1\pm Bsc[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )G\{1\pm Bsc[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(56)

3.6. sn-Type

Obtain another periodic solution of Equation (15) that are as follow [36]:

$$\Psi \left(\gamma \right)=P\{1\pm Usn[\varphi (\gamma -{\gamma }_o),w]\},$$

(57)

where ${\gamma }_o$ is the constant and $sn(x,w)$ is JEF with modulus w containing values $0<w<1$. Obtain the P and U to solve the above solution that are present as:

$$P=-\frac{\mu }{4\eta }, U=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(58)

we can also get the following parameters by solving above equations:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(59)

Periodic solutions of $sn$-form for Equation (2) are represented as:

$${\rm Y}(x,t)={\left\{P[1\pm Usn(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(60)

where $ϵ=x-vt-{\gamma }_o$. The constant $A_1$ and $A_2$ are written as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(61)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(62)

By putting the solution of Equation (57) in Equation (21), so we get the following chirping that are as follow:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)P\{1\pm Usn[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )P\{1\pm Usn[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(63)

3.7. $ns\pm ds$-Type

Periodic solution for Equation (15) are obtained as follow [36]:

$$\Psi \left(\gamma \right)=\chi \{1\pm o(ns\pm ds)[\varphi (\gamma -{\gamma }_o),w]\},$$

(64)

where ${\gamma }_o$ is the constant and $ns\pm ds(x,w)$ is JEF with modulus w having values $0<w<1$. By solving above solution, we can obtain following parameters:

$$\chi =-\frac{\mu }{4\eta }, o=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(65)

The value of the following parameters are represented as:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(66)

Obtain the periodic solutions for Equation (2) by putting the solution of the Equation (64) in Equation (20):

$${\rm Y}(x,t)={\left\{\chi [1\pm o(ns\pm ds)(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(67)

where $ϵ=x-vt-{\gamma }_o$. The constant of integration may be written as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(68)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(69)

Insert the solution of Equation (64) and Equation (21), since the corresponding chirping are expressed as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\chi \{1\pm o(ns\pm ds\left)\right[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )\chi \{1\pm o(ns\pm ds\left)\right[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(70)

3.8. $wsn\pm idn$-Type

The PW solution for Equation (15) are written as [36]:

$$\Psi \left(\gamma \right)=\Lambda \{1\pm t(wsn\pm idn)[\varphi (\gamma -{\gamma }_o),w]\},$$

(71)

where ${\gamma }_o$ is the constant and $wsn\pm idn(x,w)$ is JEF with modulus w having values $0<w<1$. By solving above solution, we can find following parameters:

$$\Lambda =-\frac{\mu }{4\eta }, t=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(72)

The value of following parameters that are expressed as:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(73)

Obtain the periodic solutions for Equation (2) by insert the solution of the Equation (71) in Equation (20):

$${\rm Y}(x,t)={\{\Lambda [1\pm t(wsn\pm idn)(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(74)

where $ϵ=x-vt-{\gamma }_o$. The constant of integration can be expressed as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(75)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(76)

The corresponding chirping are as follow:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Lambda \{1\pm t(wsn\pm idn\left)\right[\varphi ϵ,w\left]\right\}}-\frac{(3\lambda +2\vartheta )\Lambda \{1\pm t(wsn\pm idn\left)\right[\varphi ϵ,w\left]\right\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(77)

3.9. $\sqrt{1-w^2}sc\pm dc$-Type

We can find the periodic solution for Equation (15) that are written as [36]:

$$\Psi \left(\gamma \right)=\rho \{1\pm h(\sqrt{1-w^2}sc\pm dc)[\varphi (\gamma -{\gamma }_o),w]\},$$

(78)

where ${\gamma }_o$ is the constant and $\sqrt{1-w^2}sc\pm dc(x,w)$ is JEF with modulus w having values $0<w<1$. By solving above solution, we can get following parameters:

$$\rho =-\frac{\mu }{4\eta }, h=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(79)

The value of following parameters that are shown below:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(80)

Obtain the periodic solutions for Equation (2) by equating the solution of the Equation (78) in Equation (20):

$${\rm Y}(x,t)={\left\{\rho [1\pm h(\sqrt{1-w^2}sc\pm dc)(\varphi ϵ,w)]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(81)

where $ϵ=x-vt-{\gamma }_o$. The constant of integration are represented as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(82)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(83)

The corresponding chirping are represented as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\rho \{1\pm h(\sqrt{1-w^2}sc\pm dc)[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\rho \{1\pm h(\sqrt{1-w^2}sc\pm dc)[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(84)

3.10. $\frac{sn}{1\pm dn}$-Type

Equation (15) provides another periodic solution that are shown below [36]:

$$\Psi \left(\gamma \right)=\varrho \{1\pm r(\frac{sn}{1\pm dn})[\varphi (\gamma -{\gamma }_o),w]\},$$

(85)

where ${\gamma }_o$ is the constant and $\frac{sn}{1\pm dn}(x,w)$ is JEF with modulus w having values $0<w<1$. By solving above solution, we can get following parameters:

$$\varrho =-\frac{\mu }{4\eta }, r=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(86)

The following parameters are obtained as:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(87)

Obtain the periodic solutions for Equation (2) that are represented as:

$${\rm Y}(x,t)={\{\varrho [1\pm r(\frac{sn}{1\pm dn})(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(88)

where $ϵ=x-vt-{\gamma }_o$. The integration constants are shown below:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(89)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(90)

The following chirping are shown below:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varrho \{1\pm r(\frac{sn}{1\pm dn})[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\varrho \{1\pm r(\frac{sn}{1\pm dn})[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(91)

3.11. $\frac{sndn}{cn}$-Type

Equation (15) gives another periodic solution that are expressed as [36]:

$$\Psi \left(\gamma \right)=\Delta \{1\pm Z(\frac{sndn}{cn})[\varphi (\gamma -{\gamma }_o),w]\},$$

(92)

where ${\gamma }_o$ is the constant and $\frac{sndn}{cn}(x,w)$ is JEF with modulus w having values $0<w<1$. In order to solve above solution, we find following parameters:

$$\Delta =-\frac{\mu }{4\eta }, Z=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(93)

we can find the values of $\epsilon$ and H that are present as:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(94)

Obtain the periodic bounded solutions for Equation (2) that are as follow:

$${\rm Y}(x,t)={\{\Delta [1\pm Z(\frac{sndn}{cn})(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(95)

where $ϵ=x-vt-{\gamma }_o$. The integration constants are written as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(96)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(97)

The accompanying chirping are shown below:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Delta \{1\pm Z(\frac{sndn}{cn})[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\Delta \{1\pm Z(\frac{sndn}{cn})[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(98)

3.12. $\frac{dncn}{A\left(1sn\right)(1+wsn)}$-Type

Equation (15) shows another periodic solution that are takes form [36]:

$$\Psi \left(\gamma \right)=\varsigma \{1\pm g(\frac{dncn}{A(1+sn)(1+wsn)})[\varphi (\gamma -{\gamma }_o),w]\},$$

(99)

where ${\gamma }_o$ is the constant and $\frac{dncn}{A(1+sn)(1+wsn)}(x,w)$ is JEF with modulus w having values $0<w<1$. The value of $\varsigma$ and g are given by:

$$\varsigma =-\frac{\mu }{4\eta }, g=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(100)

The following parameters are as follow:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(101)

Periodic bounded solutions for Equation (2) takes form:

$${\rm Y}(x,t)={\{\varsigma [1\pm g(\frac{dncn}{A(1+sn)(1+wsn)})(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(102)

where $ϵ=x-vt-{\gamma }_o$. The integration constants takes form:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(103)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(104)

By substituting the Equations (99) and (21), since the chirping can be given as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varsigma \{1\pm g(\frac{dncn}{A(1+sn)(1+wsn)})[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\varsigma \{1\pm g(\frac{dncn}{A(1+sn)(1+wsn)})[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(105)

3.13. $\frac{wcndn}{ws{n}^2+1}$-Type

Another PW solution of Equation (15) are obtain as [36]:

$$\Psi \left(\gamma \right)=\varpi \{1\pm y(\frac{wcndn}{ws{n}^2+1})[\varphi (\gamma -{\gamma }_o),w]\},$$

(106)

where ${\gamma }_o$ is the constant and $\frac{wcndn}{ws{n}^2+1}(x,w)$ is JEF with modulus w having values $0<w<1$. The parameters $\varpi$ and y are defined as:

$$\varpi =-\frac{\mu }{4\eta }, y=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(107)

The following parameters are also defined as:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(108)

The chirped periodic solution of Equation (2) may be written as:

$${\rm Y}(x,t)={\{\varpi [1\pm y(\frac{wcndn}{ws{n}^2+1})(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(109)

where $ϵ=x-vt-{\gamma }_o$. The value of integration constants can be given as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(110)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(111)

By equating the Equations (21) and (106), so that the following chirping takes form:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varpi \{1\pm y(\frac{wcndn}{ws{n}^2+1})[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\varpi \{1\pm y(\frac{wcndn}{ws{n}^2+1})[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(112)

3.14. $\frac{sn}{1\pm cn}$-Type

The bounded periodic solution of Equation (15) is as follows [36]:

$$\Psi \left(\gamma \right)=\Sigma \{1\pm e(\frac{sn}{1\pm cn})[\varphi (\gamma -{\gamma }_o),w]\},$$

(113)

where ${\gamma }_o$ is the constant and $\frac{sn}{1\pm cn}(x,w)$ is JEF with modulus w having values $0<w<1$. The values of $\Sigma$ and e are defined as:

$$\Sigma =-\frac{\mu }{4\eta }, e=\frac{4w\varphi \sqrt{\eta }}{\mu },$$

(114)

we can get the parameters $\epsilon$ and H by solving above relations:

$$\epsilon =\frac{\mu (4\eta \xi -{\mu }^2)}{8{\eta }^2}, H=\frac{5{\mu }^2(10\eta \xi -{\mu }^2)}{256{\eta }^3},$$

(115)

Obtain the bounded periodic solutions for Equation (2) that are given as:

$${\rm Y}(x,t)={\{\Sigma [1\pm e(\frac{sn}{1\pm cn})(\varphi ϵ,w)]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(116)

where $ϵ=x-vt-{\gamma }_o$. The value of following constants are defined as:

$${A_1}^2=\frac{5{\mu }^2{(b-av)}^2(10\xi \eta -{\mu }^2)}{1024{\eta }^3},$$

(117)

$$A_2=\frac{\mu (4\xi \eta -{\mu }^2))}{64{\eta }^2},$$

(118)

The chirping that accompanies with this PW is defined by:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Sigma \{1\pm e\left(\frac{sn}{1\pm cn}\right)[\varphi ϵ,w]\}}-\frac{(3\lambda +2\vartheta )\Sigma \{1\pm e\left(\frac{sn}{1\pm cn}\right)[\varphi ϵ,w]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(119)

4. The SW Limit

Here, we can find the chirped SW solutions of Equation (2). The JEF convert into trigonometric functions at the long-wave limit, which correspond to $w\to 1$ and $w\to 0$. The nonlinear chirp of each of these optical pulses is also calculated.

4.1. Bright SW

In the limiting case $w\to 1$, the function $cn(ϵ,w)\to sech\left(ϵ\right)$ and Equation (25) gives the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\left\{D[1\pm Lsech\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(120)

The values of D and L are defined as:

$$D=-\frac{\mu }{4\eta }, L=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(121)

The chirping associated with the nonlinearly chirped SW is easy to accomplish:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)D\{1\pm Lsech\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )D\{1\pm Lsech\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(122)

4.2. Dark Wave

In the limiting case $w\to 1$, the function $sn(ϵ,w)\to tanh\left(ϵ\right)$ and Equation (60) present the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\left\{P[1\pm Utanh\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(123)

where the variables are defined as:

$$P=-\frac{\mu }{4\eta }, U=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(124)

The nonlinearly chirped SW’s chirping may be represented as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)P\{1\pm Utanh\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )P\{1\pm Utanh\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(125)

4.3. Singular-I Wave

In the limiting case $w\to 1$, the function $ds(ϵ,w)\to csch\left(ϵ\right)$, the CPW solution of Equation (46) converts to the SW solution of Equation (2):

$${\rm Y}(x,t)={\left\{E[1\pm Mcsch\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(126)

where the parameters are defined as:

$$E=-\frac{\mu }{4\eta }, M=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(127)

The corresponding chirping associated with the SW solution are represented as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)E\{1\pm Mcsch\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )E\{1\pm Mcsch\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(128)

4.4. Hyperbolic-I Wave

In the limiting case $w\to 1$, the function $sc(ϵ,w)\to sinh\left(ϵ\right)$ and Equation (53) shows the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\left\{G[1\pm Bsinh\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(129)

where the variables are present as:

$$G=-\frac{\mu }{4\eta }, B=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(130)

The chirping that belongs to SW solution can be written as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)G\{1\pm Bsinh\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )G\{1\pm Bsinh\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(131)

4.5. Periodic-I Wave

In the limiting case $w\to 0$, the function $cn(ϵ,w)\to cos\left(ϵ\right)$ and Equation (25) express the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\left\{D[1\pm Lcos\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(132)

The values of D and L are defined as:

$$D=-\frac{\mu }{4\eta },$$

(133)

The chirping associated with the nonlinearly chirped SW is expressed as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)D\{1\pm Lcos\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )D\{1\pm Lcos\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(134)

4.6. Periodic-II Wave

In the limiting case $w\to 0$, the function $ds(ϵ,w)\to csc\left(ϵ\right)$, the CPW solution of Equation (46) converts to the SW solution of Equation (2):

$${\rm Y}(x,t)={\left\{E[1\pm Mcsc\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(135)

where the parameter are express as:

$$E=-\frac{\mu }{4\eta },$$

(136)

The corresponding chirping associated with the SW solution are shown below:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)E\{1\pm Mcsc\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )E\{1\pm Mcsc\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(137)

4.7. Periodic-III Wave

In the limiting case $w\to 0$, the function $sn(ϵ,w)\to sin\left(ϵ\right)$ and Equation (60) shows the SW solution of Equation (2) that are shown below:

$${\rm Y}(x,t)={\left\{P[1\pm Usin\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(138)

where the variable takes form:

$$P=-\frac{\mu }{4\eta },$$

(139)

The nonlinearly chirped SW’s chirping can be expressed as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)P\{1\pm Usin\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )P\{1\pm Usin\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(140)

4.8. Kink Type

In the limiting case $w\to 0$, the function $sc(ϵ,w)\to tan\left(ϵ\right)$ and Equation (53) express the SW solution of Equation (2) that can be written as:

$${\rm Y}(x,t)={\left\{G[1\pm Btan\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(141)

where the variables are present as:

$$G=-\frac{\mu }{4\eta },$$

(142)

The chirping that belongs to CSW solution can be present as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)G\{1\pm Btan\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )G\{1\pm Btan\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(143)

4.9. Periodic Type

In the limiting case $w\to 0$, the function $dc(ϵ,w)\to sec\left(ϵ\right)$ and Equation (32) shows the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\left\{Z[1\pm Ysec\left[{\varphi }_o(x-vt-w_o)\right]]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(144)

where the variable can be expressed as:

$$Z=-\frac{\mu }{4\eta },$$

(145)

The chirping that belongs to SW solution takes form:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)Z\{1\pm Ysec\left[{\varphi }_o(x-vt-w_o)\right]\}}-\frac{(3\lambda +2\vartheta )Z\{1\pm Ysec\left[{\varphi }_o(x-vt-w_o)\right]\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(146)

4.10. Dipole Soliton

In the limiting case $w\to 1$, the function $wsn\pm idn(ϵ,w)\to tanh\left(ϵ\right)\pm isech\left(ϵ\right)$ and Equation (74) gives the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\{\Lambda [1\pm t(tanh\left[{\varphi }_o(x-vt-w_o)\right]\pm isech\left[{\varphi }_o(x-vt-w_o)\right])]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(147)

where the parameters takes form:

$$\Lambda =-\frac{\mu }{4\eta }, t=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(148)

The chirping that belongs to SW can be written as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Lambda \{1\pm t(tanh\left[{\varphi }_o(x-vt-w_o)\right]\pm isech\left[{\varphi }_o(x-vt-w_o)\right])\}}-\frac{(3\lambda +2\vartheta )\Lambda \{1\pm t(tanh\left[{\varphi }_o(x-vt-w_o)\right]\pm isech\left[{\varphi }_o(x-vt-w_o)\right])\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(149)

4.11. $\frac{tanh\left(ϵ\right)}{1\pm sech\left(ϵ\right)}$ SW

In the limiting case $w\to 1$, the function $\frac{sn}{1\pm dn}(ϵ,w)\to \frac{tanh\left(ϵ\right)}{1\pm sech\left(ϵ\right)}$ and the chirped solution of Equation (88) gives the SW solution of Equation (2) takes form:

$${\rm Y}(x,t)={\{\varrho [1\pm r(\frac{tanh\left[{\varphi }_o(x-vt-w_o)\right]}{1\pm sech\left[{\varphi }_o(x-vt-w_o)\right]})]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(150)

where the parameters are given as:

$$\varrho =-\frac{\mu }{4\eta }, r=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(151)

The following chirping as follow:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varrho \{1\pm r(\frac{tanh\left[{\varphi }_o(x-vt-w_o)\right]}{1\pm sech\left[{\varphi }_o(x-vt-w_o)\right]})\}}-\frac{(3\lambda +2\vartheta )\varrho \{1\pm r(\frac{tanh\left[{\varphi }_o(x-vt-w_o)\right]}{1\pm sech\left[{\varphi }_o(x-vt-w_o)\right]})\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(152)

4.12. $\frac{{sech}^2\left(ϵ\right)}{1+{tanh}^2\left(ϵ\right)}$ SW

In the limiting case $w\to 1$, the function $\frac{mcndn}{ws{n}^2+1}(ϵ,w)\to \frac{{sech}^2\left(ϵ\right)}{1+{tanh}^2\left(ϵ\right)}$ and the chirped solution of Equation (109) express the SW solution of Equation (2) shown below:

$${\rm Y}(x,t)={\{\varpi [1\pm y(\frac{{sech}^2\left[{\varphi }_o(x-vt-w_o)\right]}{1+{tanh}^2\left[{\varphi }_o(x-vt-w_o)\right]})]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(153)

where the value of $\varpi$ and y are shown below:

$$\varpi =-\frac{\mu }{4\eta }, y=\frac{4\varphi \sqrt{\eta }}{\mu },$$

(154)

The following chirping are expressed as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varpi \{1\pm y(\frac{{sech}^2\left[{\varphi }_o(x-vt-w_o)\right]}{1+{tanh}^{[}{\varphi }_o(x-vt-w_o)]2})\}}-\frac{(3\lambda +2\vartheta )\varpi \{1\pm y(\frac{{sech}^2\left[{\varphi }_o(x-vt-w_o)\right]}{1+{tanh}^2\left[{\varphi }_o(x-vt-w_o)\right]})\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(155)

4.13. $isech\left(ϵ\right)$ SW

In the limiting case $w\to 0$, the function $wsn\pm idn(ϵ,w)\to isech\left(ϵ\right)$ and Equation (74) gives the SW solution of Equation (2) as follows:

$${\rm Y}(x,t)={\{\Lambda [1\pm t(isech\left[{\varphi }_o(x-vt-w_o)\right])]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(156)

where the parameter takes form:

$$\Lambda =-\frac{\mu }{4\eta },$$

(157)

The chirping that belongs to SW can be written as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Lambda \{1\pm t(isech\left[{\varphi }_o(x-vt-w_o)\right])\}}-\frac{(3\lambda +2\vartheta )\Lambda \{1\pm t(isech\left[{\varphi }_o(x-vt-w_o)\right])\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(158)

4.14. $sec\left(ϵ\right)-tan\left(ϵ\right)$ SW

In the limiting case $w\to 0$, the function $\frac{dncn}{A(1+sn)(1+wsn)}(ϵ,w)\to sec\left(ϵ\right)-tan\left(ϵ\right)$ and Equation (102) gives the SW solution of Equation (2) can be written as:

$${\rm Y}(x,t)={\left\{\varsigma [1\pm g(sec\left[{\varphi }_o(x-vt-w_o)\right]-tan\left[{\varphi }_o(x-vt-w_o)\right])]\right\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(159)

where the parameter can be express as:

$$\varsigma =-\frac{\mu }{4\eta },$$

(160)

The resultant chirping shown below:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\varsigma \{1\pm g(sec\left[{\varphi }_o(x-vt-w_o)\right]-tan\left[{\varphi }_o(x-vt-w_o)\right])\}}$$

(161)

$$-\frac{(3\lambda +2\vartheta )\varsigma \{1\pm g(sec\left[{\varphi }_o(x-vt-w_o)\right]-tan\left[{\varphi }_o(x-vt-w_o)\right])\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(162)

4.15. $csc\left(ϵ\right)\pm cot\left(ϵ\right)$ SW

In the limiting case $w\to 0$, the function $\frac{sn}{1\pm cn)}(ϵ,w)\to csc\left(ϵ\right)\pm cot\left(ϵ\right)$ and Equation (116) gives the SW solution of Equation (2) can be represent as:

$${\rm Y}(x,t)={\{\Sigma [1\pm e(csc\left[{\varphi }_o(x-vt-w_o)\right]\pm cot\left[{\varphi }_o(x-vt-w_o)\right])]\}}^{\frac{1}{2}}exp\left[i(K\left(\gamma \right)-\Omega t)\right],$$

(163)

where the parameter can be present as:

$$\Sigma =-\frac{\mu }{4\eta },$$

(164)

The resultant chirping present as:

$$\delta {\rm Y}(x,t)=-\frac{A_1}{(b-av)\Sigma \{1\pm e(csc\left[{\varphi }_o(x-vt-w_o)\right]\pm cot\left[{\varphi }_o(x-vt-w_o)\right])\}}$$

(165)

$$-\frac{(3\lambda +2\vartheta )\Sigma \{1\pm e(csc\left[{\varphi }_o(x-vt-w_o)\right]\pm cot\left[{\varphi }_o(x-vt-w_o)\right])\}}{4(b-av)}-\frac{v+a\Omega +\alpha }{2(b-av)},$$

(166)

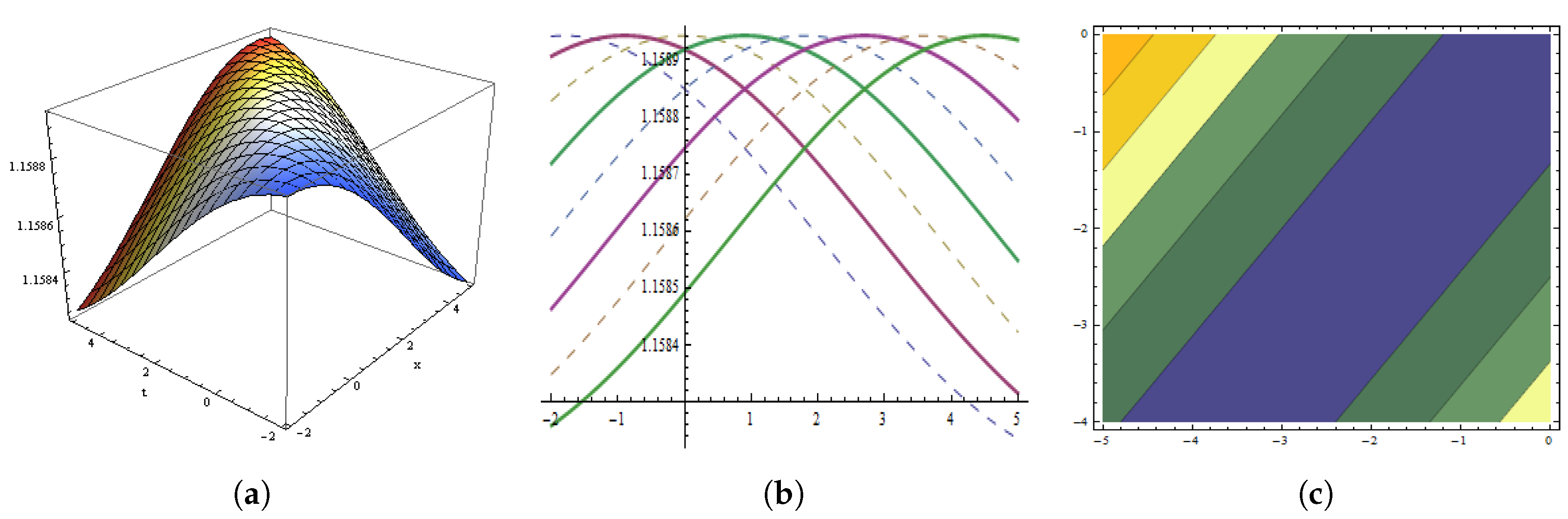
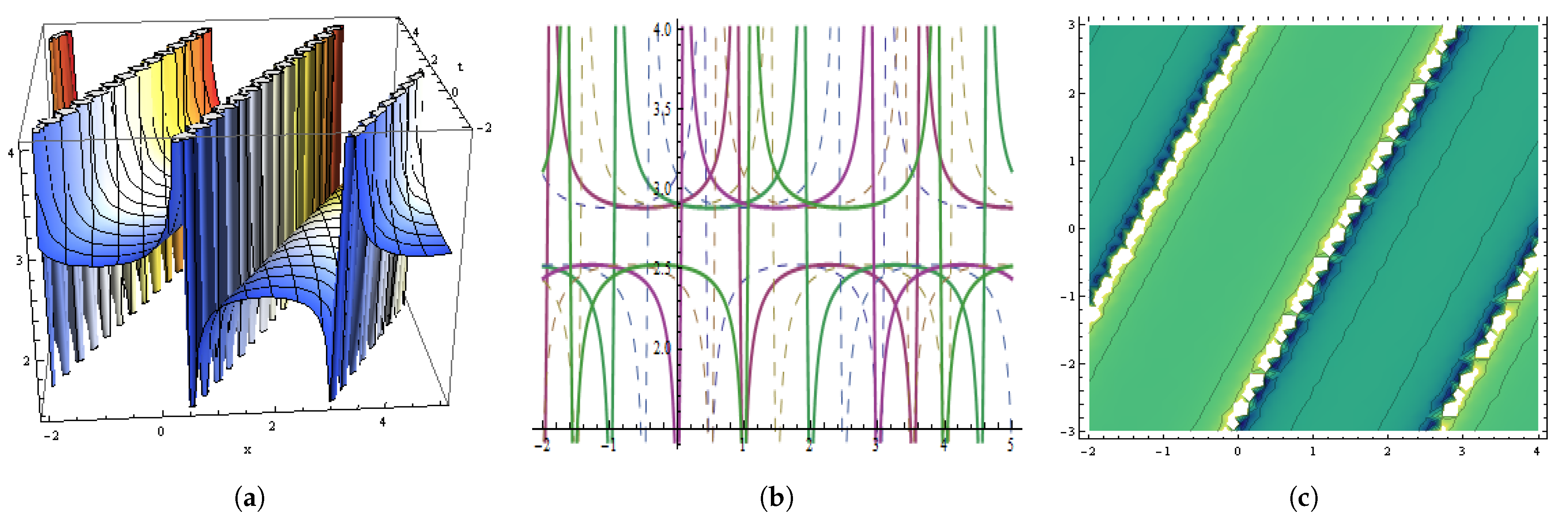
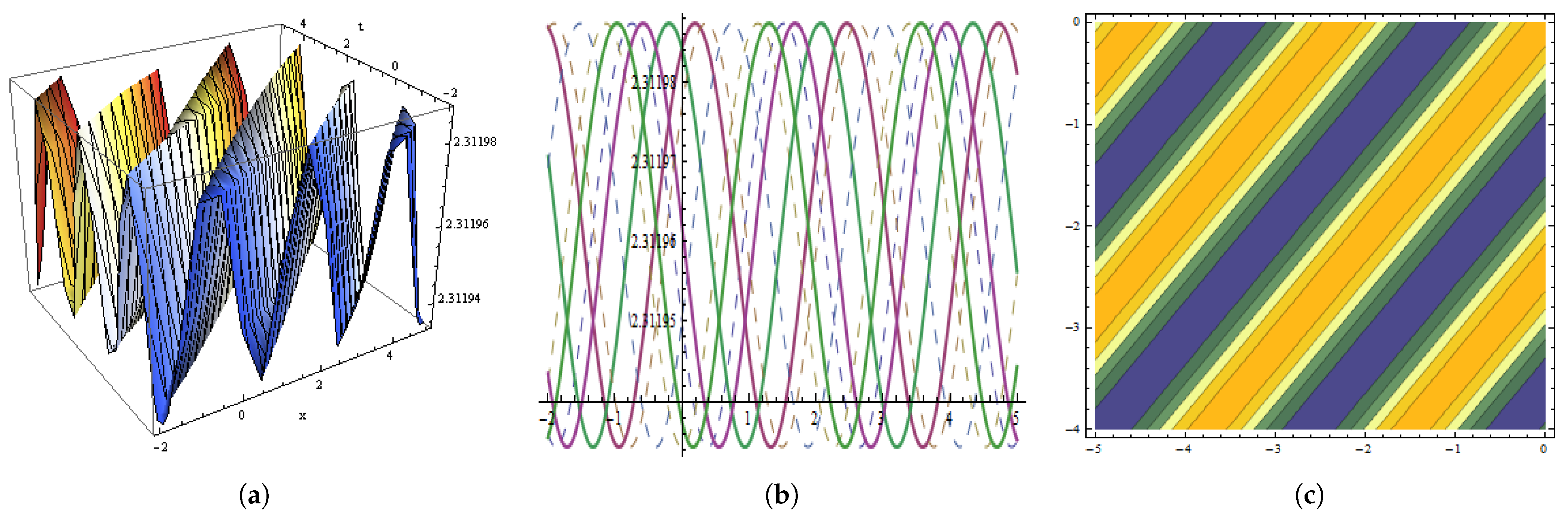
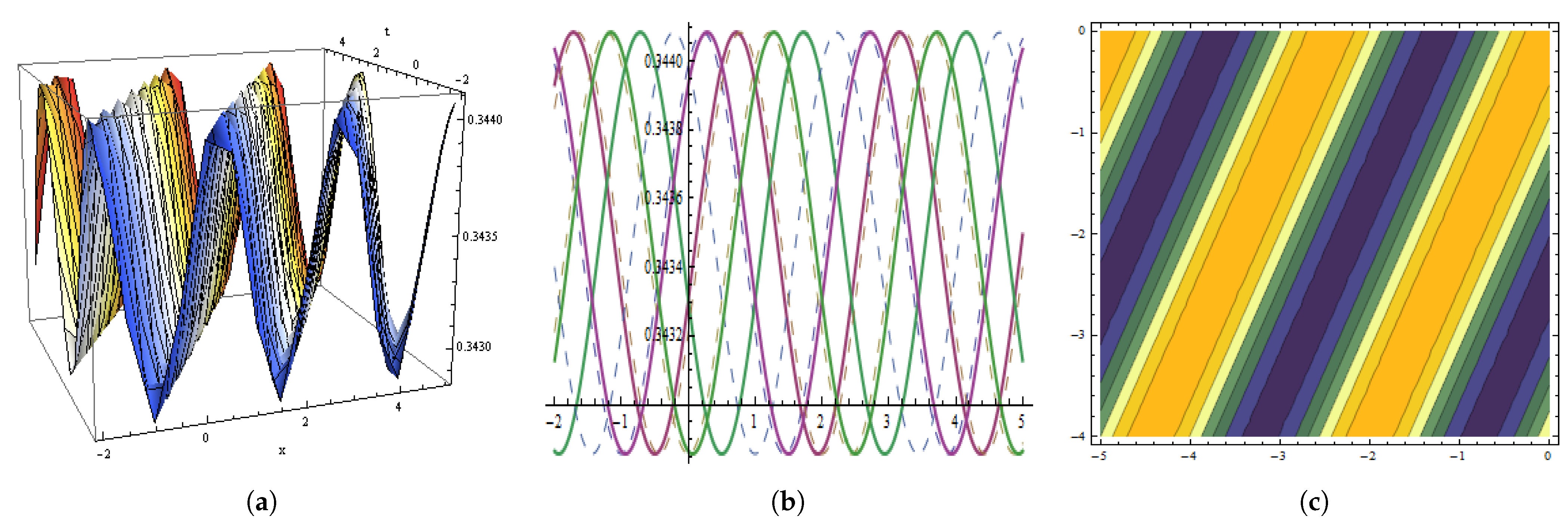
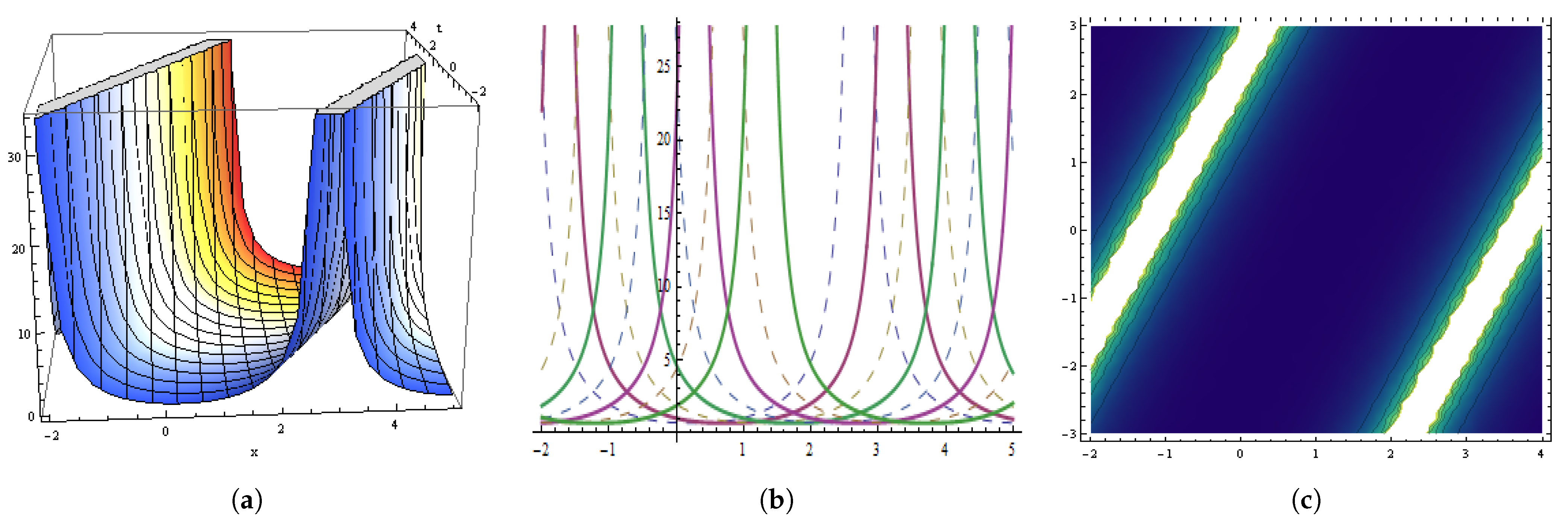
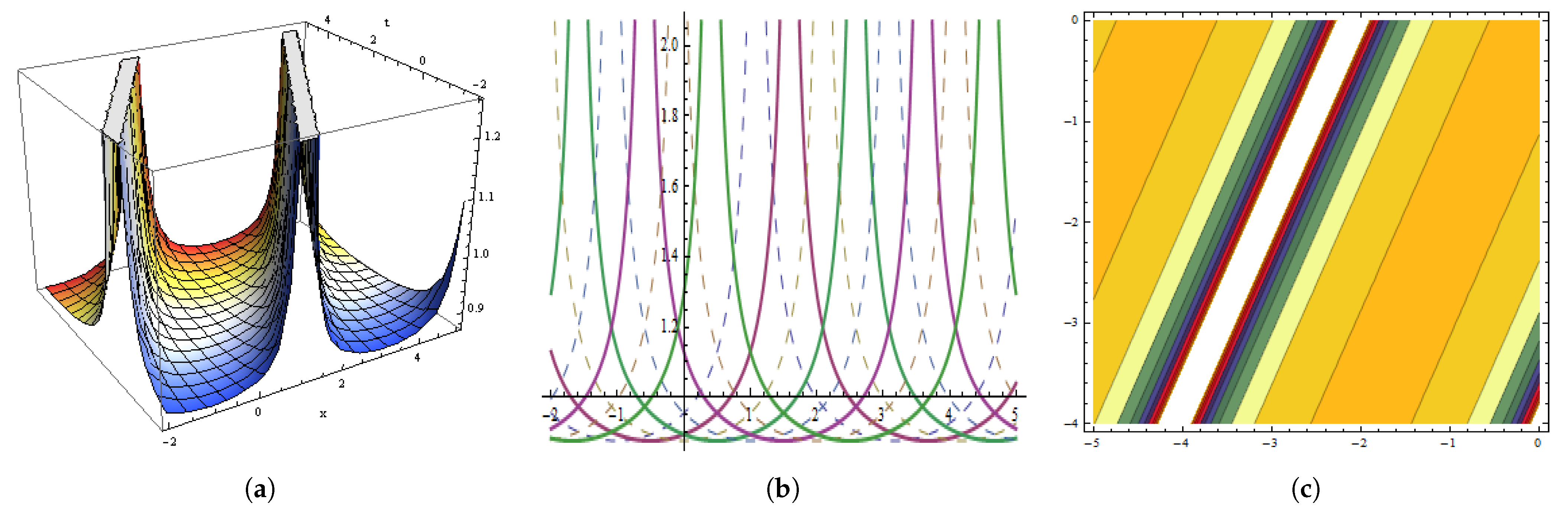
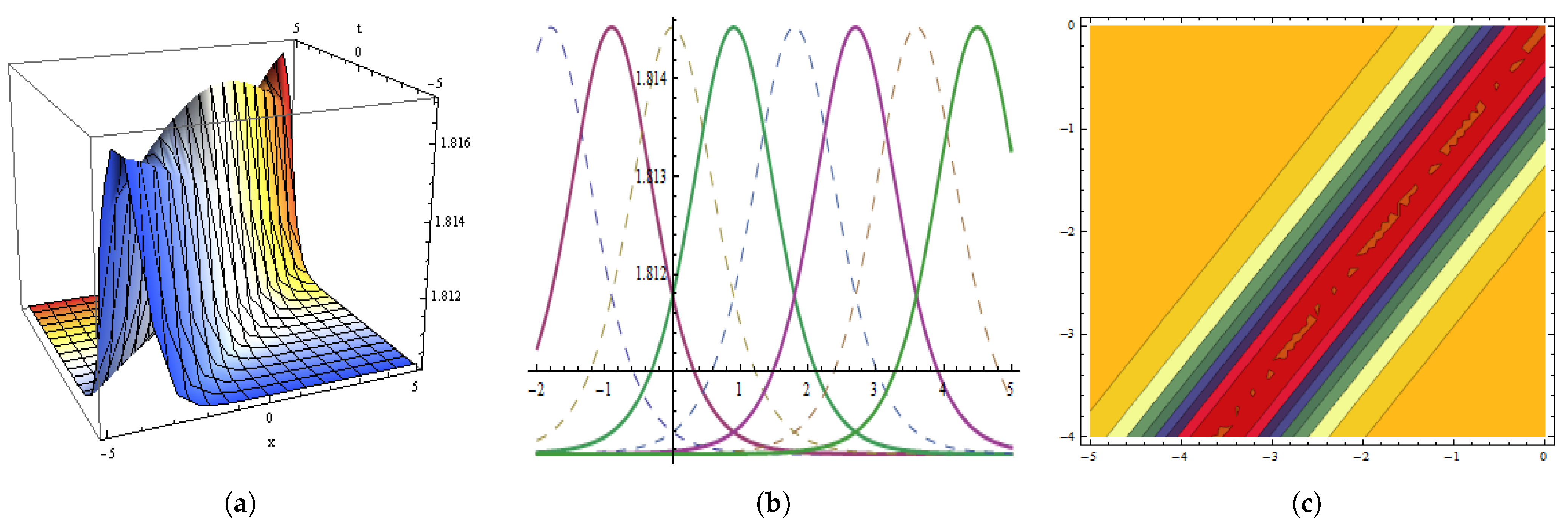
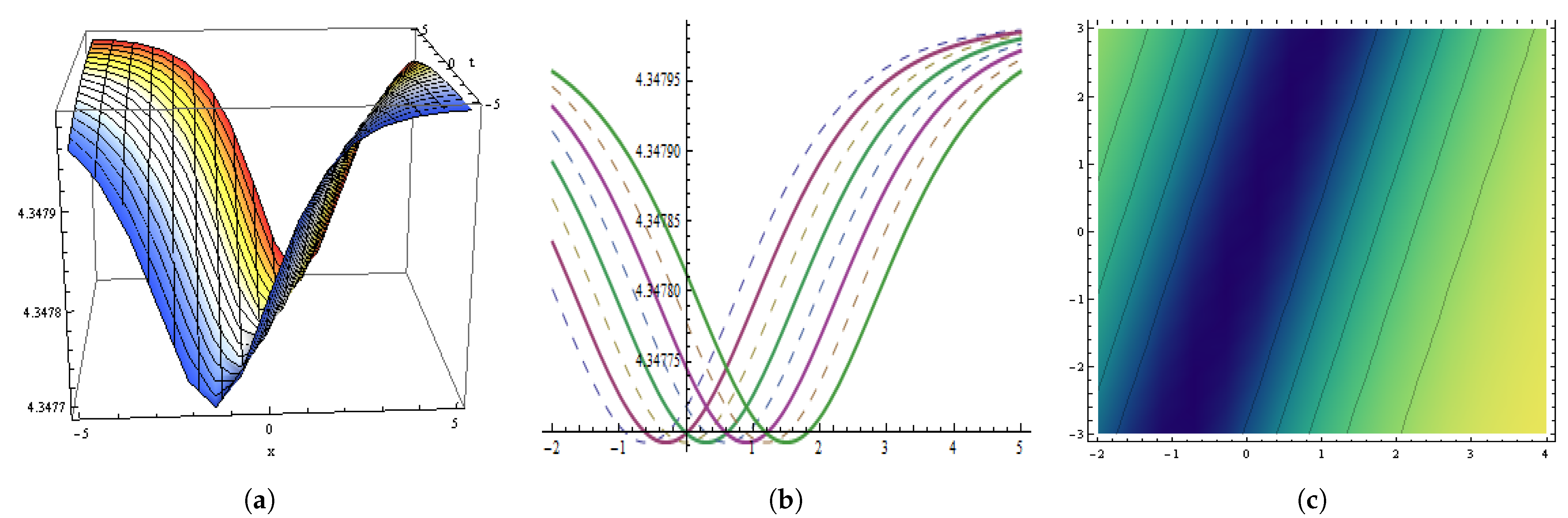
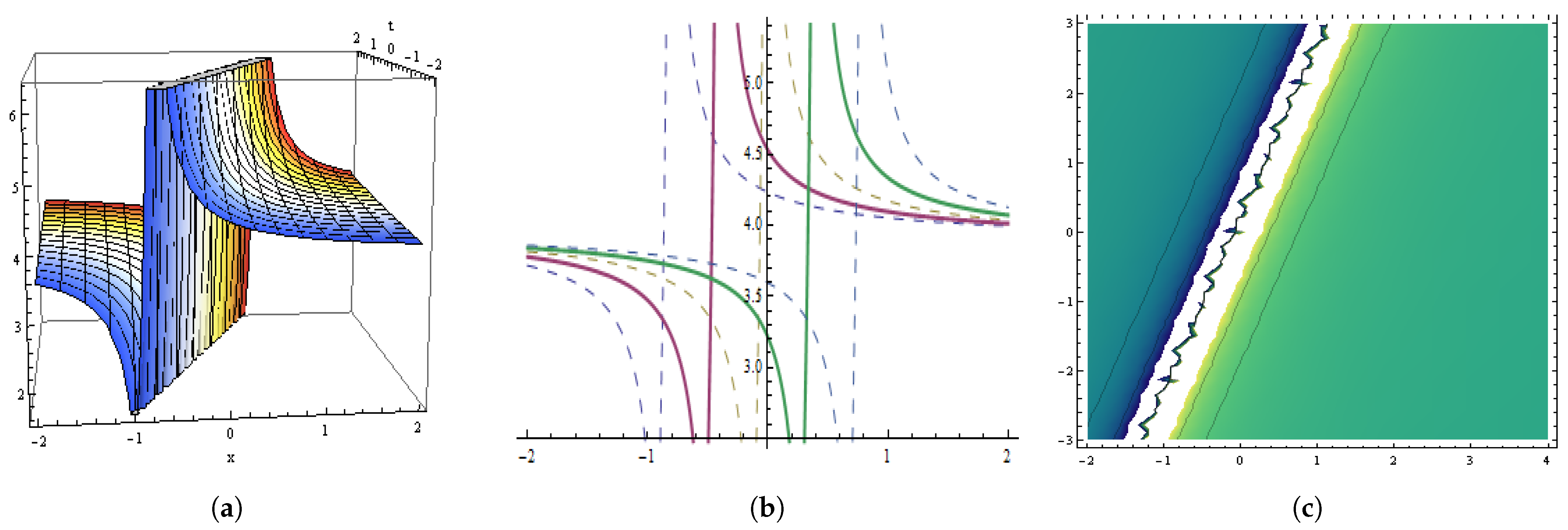
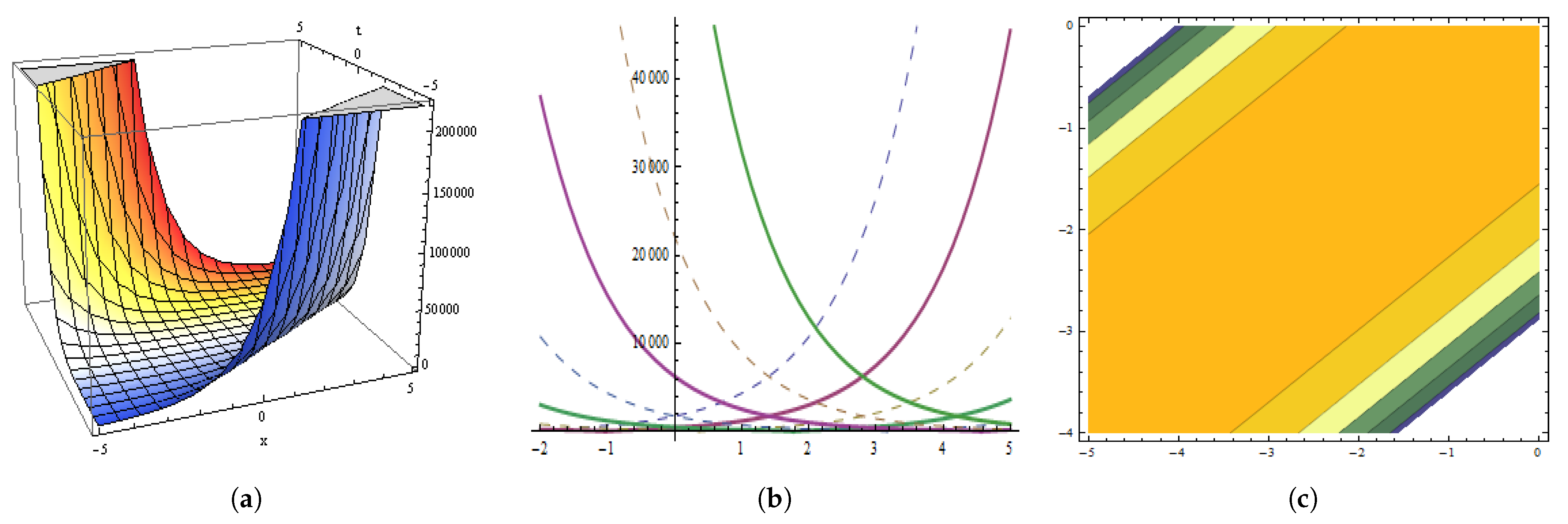
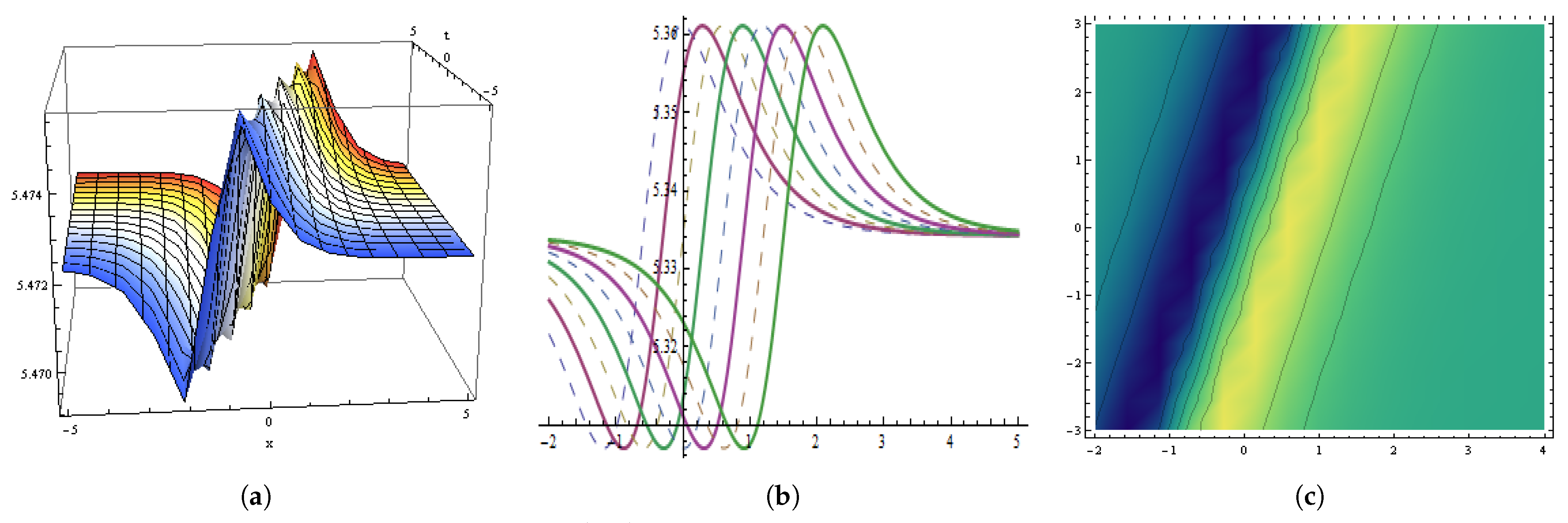
5. Results and Discussion

In this section, we will discuss and compare our results with other authors results. Biswas et al. used the extended trial function technique to find solitons for the perturbed NLSE with 10 types of fibre nonlinearity. In the presence of a few Hamiltonian perturbation terms, Bouzida et al. [32] provide a large range of chirped soliton solutions for the improved NLSE with dual-power law nonlinearity. Savescu et al. [33] investigated the perturbed NLSE with full nonlinearity in order to discuss the dynamic of solitons in nano fibre optics. Biswas et al. [34] also studied the perturbed NLSE in order to obtain the optical solitons solutions by trial equation method.

In this manuscript, we display the different forms of CPW and SW solutions. Equation (25) gives the $cn$-form periodic solution while in Equation (120) it changed into a bright SW when $w\to 1$ and in Equation (132) it changed into periodic-I SW when $w\to 0$. Equation (32) present the periodic $dc$-form solution while in Equation (144) it changed into Bell SW when $w\to 0$. Equation (46) represent the periodic $ds$-form solution while in Equation (126) it changed into singular-I SW when $w\to 1$ and in Equation (135) it changed into periodic-II SW when $w\to 0$. Equation (53) present the $sc$-form periodic solution while in Equation (129) it changed into hyperbolic-I SW when $w\to 1$ and in Equation (141) it convert into kink SW when $w\to 0$. Equation (60) shows the periodic $sn$-form solution while in Equation (123) it changed into dark SW when $w\to 1$ and in Equation (138) it changed into periodic-III SW when $w\to 0$. Equation (67) shows the $ns\pm ds$-form periodic solution. Equation (74) present the periodic $wsn\pm idn$-form solution while in Equation (147) it changed into $tanh\left(ϵ\right)+isech\left(ϵ\right)$ Sw when $w\to 1$ and in Equation (156) it changed into $isech\left(ϵ\right)$ SW when $w\to 0$. Equation (81) express the $\sqrt{1-w^2}sc\pm dc$-form periodic solution. Equation (88) gives $\frac{sn}{1\pm dn}$-form periodic solution while in Equation (150) it changed into $\frac{tanh\left(ϵ\right)}{1\pm sech\left(ϵ\right)}$ SW when $w\to 1$. Equation (95) shows the $\frac{sndn}{cn}$-form periodic solution. Equation (102) present the $\frac{dncn}{A(1+sn)(1+msn)}$-form periodic solution while in Equation (159) it changed into $sec\left(ϵ\right)-tan\left(ϵ\right)$ SW when $w\to 0$. Equation (109) express the periodic $\frac{mcndn}{ms{n}^2+1}$-form solution while in Equation (153) it changed into $\frac{{sech}^2\left(ϵ\right)}{1+{tanh}^2\left(ϵ\right)}$ SW when $w\to 1$. Equation (116) gives the periodic $\frac{sn}{1\pm cn}$-form solution while in Equation (163) it changed into $csc\left(ϵ\right)\pm cot\left(ϵ\right)$ SW when $w\to 0$.

6. Conclusions

In this paper, we have investigated IPNLSE-CQN in order to obtain some chirped periodic solution based on JEF and also obtain SW solution. There are a number of exact solutions with nontrivial phase chirping that varies as a function of intensity. Localized solutions of bright, dark, singular and other type of solutions are among them. For each of these optical solitons, the corresponding chirp has been produced. The graphical description of obtained chirped solitons have also been shown.