Diffusion in Heterogenous Media and Sorption—Desorption Processes
Abstract
:1. Introduction
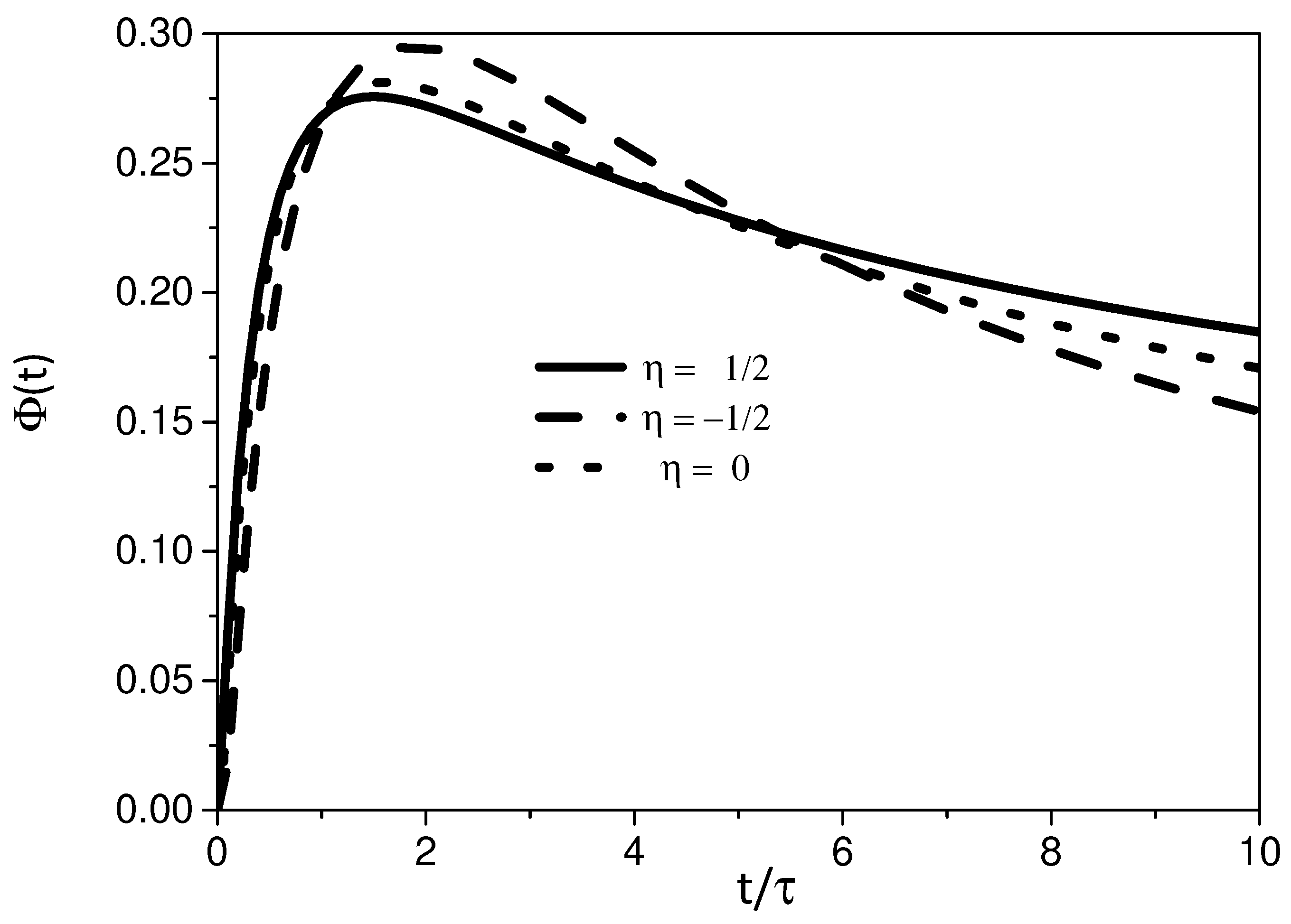
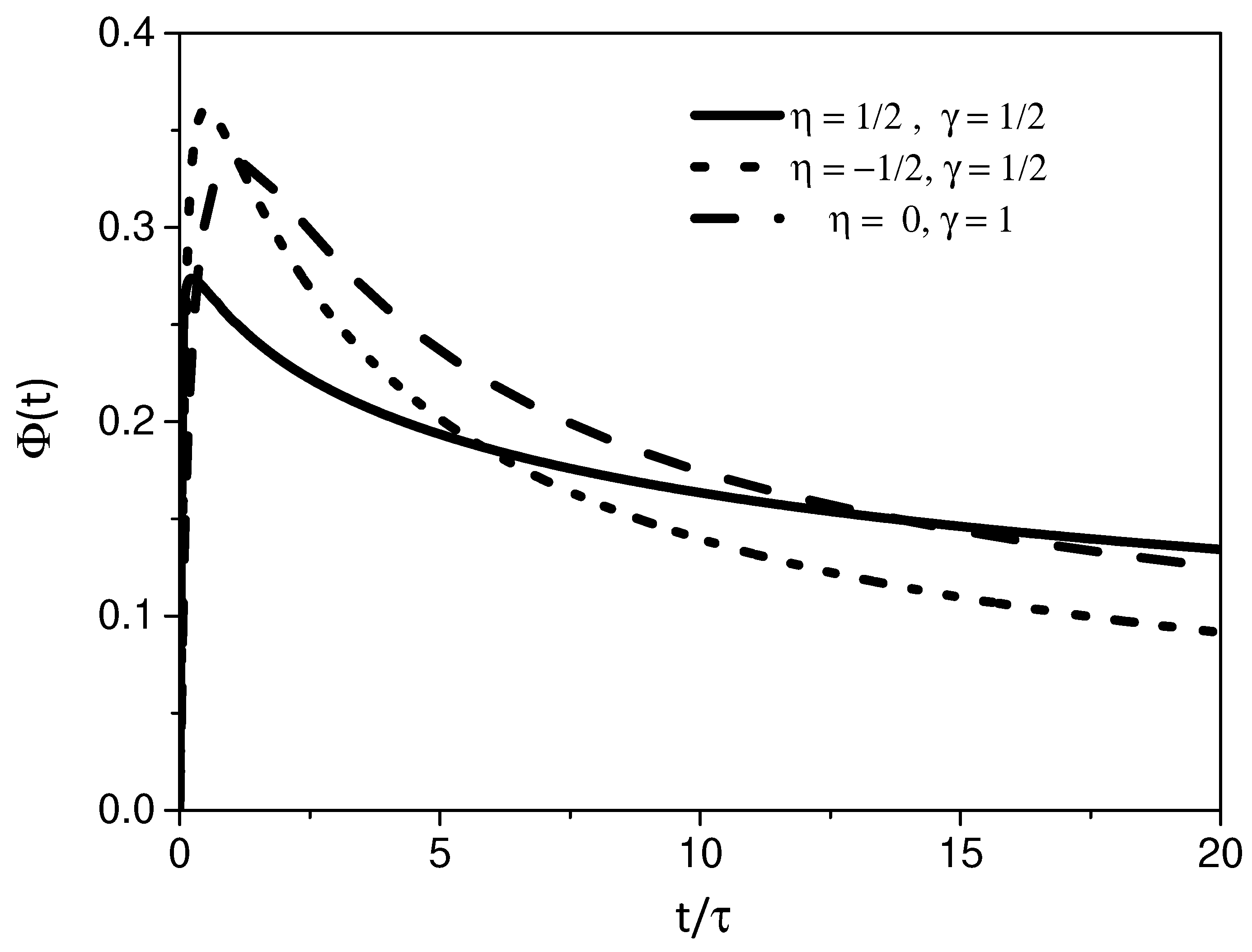
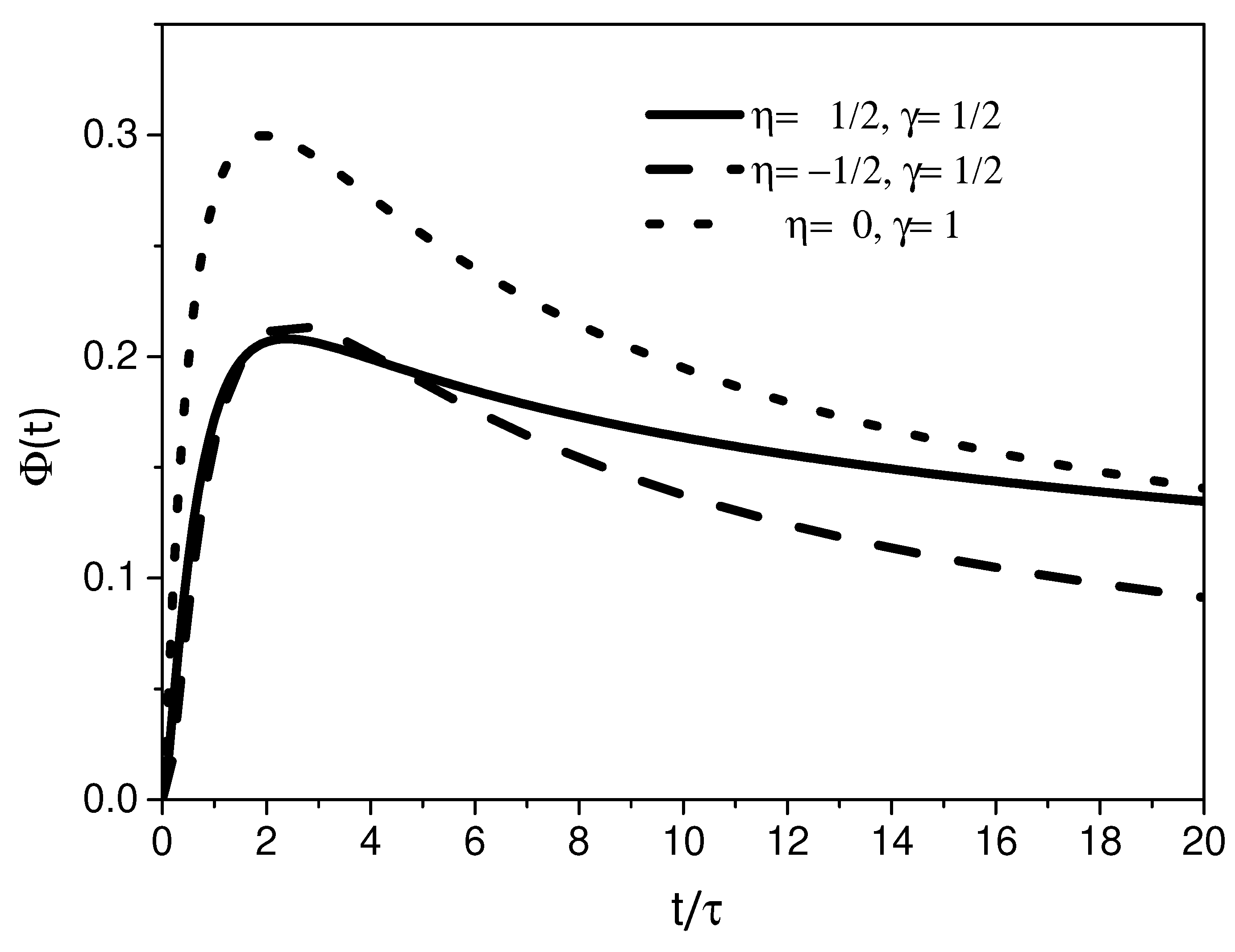
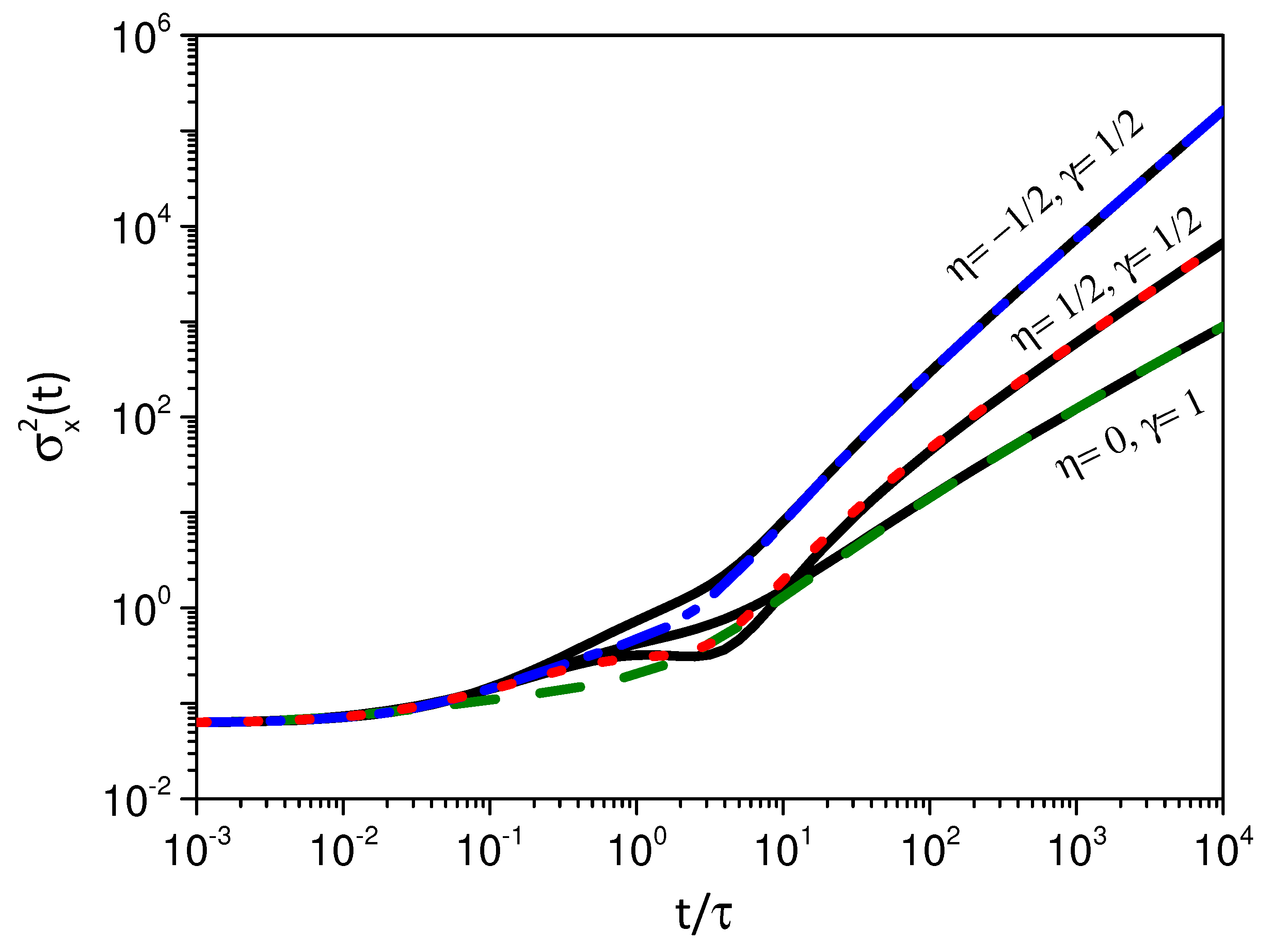
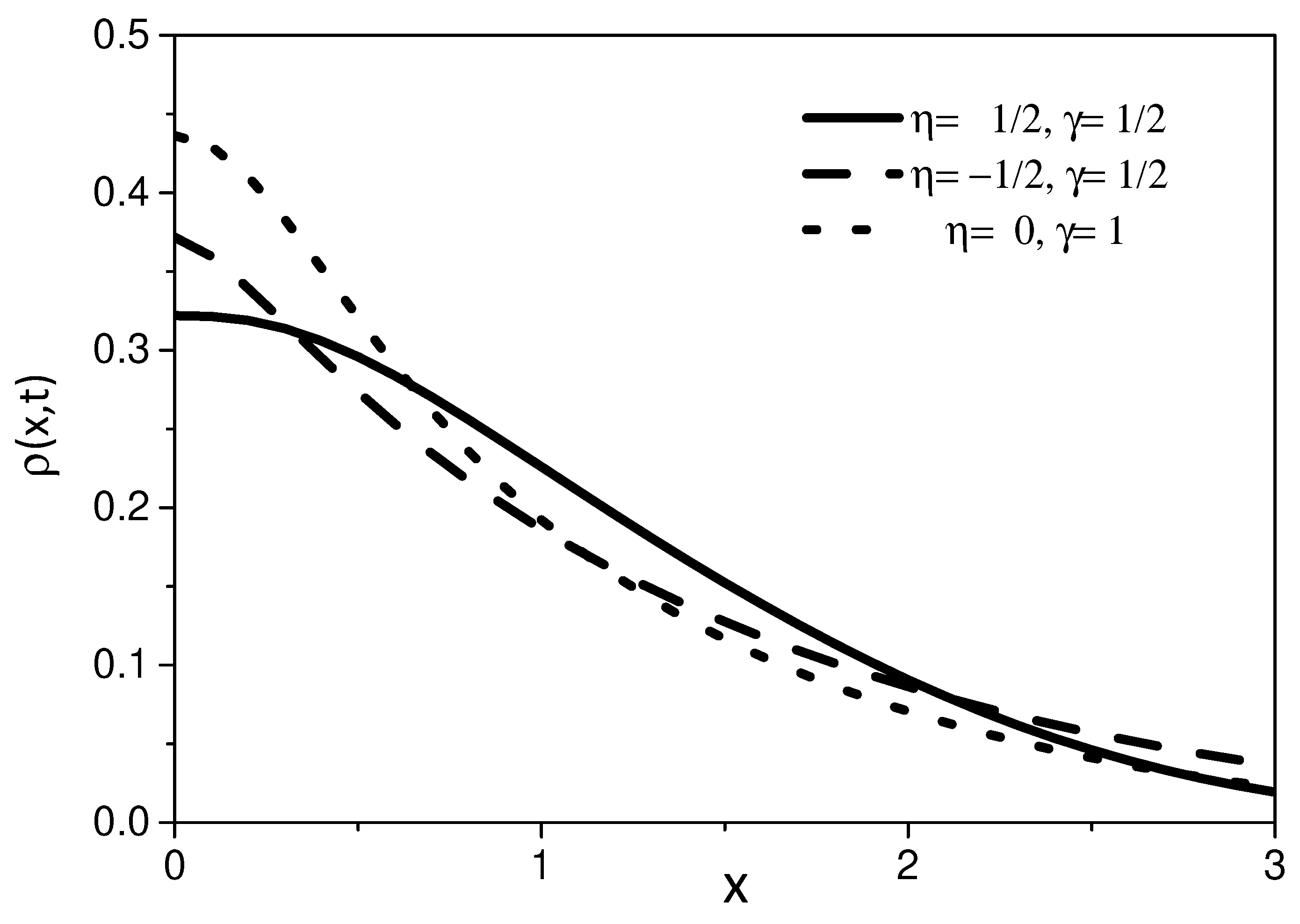
2. Diffusion Equation and Solutions
3. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, R. Gas Separation by Adsorption Processes; Elsevier Science: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Council, N.; Sciences, D.; Board, N.; Systems, C.; Assessments, C.; Recycling, P. Separation Technologies for the Industries of the Future; Publication NMAB, National Academies Press: Washington, DC, USA, 1999. [Google Scholar]
- Grandison, A.; Grandison, A.; Lewis, M. Separation Processes in the Food and Biotechnology Industries: Principles and Applications; Woodhead Publishing Series in Food Science, Technology and Nutrition; Elsevier Science: Amsterdam, The Netherlands, 1996. [Google Scholar]
- Worch, E. Adsorption Technology in Water Treatment: Fundamentals, Processes, and Modeling; De Gruyter: Berlin, Germany, 2012. [Google Scholar]
- Yiacoumi, S.; Tien, C. Kinetics of Metal Ion Adsorption from Aqueous Solutions: Models, Algorithms, and Applications; Springer: New York, NY, USA, 2013. [Google Scholar]
- Adamson, A.; Gast, A. Physical Chemistry of Surfaces; Wiley: New York, NY, USA, 1997. [Google Scholar]
- Barbero, G.; Evangelista, L. Adsorption Phenomena and Anchoring Energy in Nematic Liquid Crystals; Liquid Crystals Book Series; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Crank, J. The Mathematics of Diffusion; Oxford Science Publications; Clarendon Press: Oxford, UK, 1979. [Google Scholar]
- Weiss, M.; Hashimoto, H.; Nilsson, T. Anomalous Protein Diffusion in Living Cells as Seen by Fluorescence Correlation Spectroscopy. Biophys. J. 2003, 84, P4043–P4052. [Google Scholar] [CrossRef] [Green Version]
- Gal, N.; Weihs, D. Experimental evidence of strong anomalous diffusion in living cells. Phys. Rev. E 2010, 81, 020903. [Google Scholar] [CrossRef]
- Evangelista, L.R.; Lenzi, E.K. Fractional Diffusion Equations and Anomalous Diffusion; Cambridge University Press: Cambridge, UK, 2018; p. 395. [Google Scholar]
- Gatenby, R.A.; Gawlinski, E.T. A Reaction-Diffusion Model of Cancer Invasion. Cancer Res. 1996, 56, 5745–5753. [Google Scholar]
- Cohen, D.S.; Murray, J.D. A generalized diffusion model for growth and dispersal in a population. J. Math. Biol. 1981, 12, 237–249. [Google Scholar] [CrossRef]
- Cantrell, R.S.; Cosner, C. Diffusion models for population dynamics incorporating individual behavior at boundaries: Applications to refuge design. Theor. Popul. Biol. 1999, 55, 189–207. [Google Scholar] [CrossRef] [PubMed]
- Sonetaka, N.; Fan, H.J.; Kobayashi, S.; Chang, H.N.; Furuya, E. Simultaneous determination of intraparticle diffusivity and liquid film mass transfer coefficient from a single-component adsorption uptake curve. J. Hazard. Mater. 2009, 164, 1447–1451. [Google Scholar] [CrossRef] [PubMed]
- Bouzid, M.; Torkia, Y.B.; Wjihi, S.; Lamine, A.B. Kinetic adsorption modeling of ethanol molecules onto three types of activated carbons: Microscopic interpretation of adsorption and diffusion parameters. J. Mol. Liq. 2017, 242, 98–108. [Google Scholar] [CrossRef]
- Fontana, K.B.; Lenzi, G.G.; Watanabe, E.R.; Lenzi, E.K.; Pietrobelli, J.A.; Chaves, E.S. Biosorption and Diffusion Modeling of Pb(II) by Malt Bagasse. Int. J. Chem. Eng. 2016, 2016. [Google Scholar] [CrossRef]
- Song, J.J.; Bhattacharya, R.; Kim, H.; Chang, J.; Tang, T.Y.; Guo, H.; Ghosh, S.K.; Yang, Y.; Jiang, Z.; Kim, H.; et al. One-Dimensional Anomalous Diffusion of Gold Nanoparticles in a Polymer Melt. Phys. Rev. Lett. 2019, 122, 107802. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.; Berland, K.M. Propagators and Time-Dependent Diffusion Coefficients for Anomalous Diffusion. Biophys. J. 2008, 95, 2049–2052. [Google Scholar] [CrossRef] [Green Version]
- Alcázar-Cano, N.; Delgado-Buscalioni, R. A general phenomenological relation for the subdiffusive exponent of anomalous diffusion in disordered media. Soft Matter 2018, 14, 9937–9949. [Google Scholar] [CrossRef]
- Yurchenko, I.; Basso, J.M.V.; Syrotenko, V.S.; Staii, C. One-Dimensional Anomalous Diffusion of Gold Nanoparticles in a Polymer Melt. PLoS ONE 2019, 14, e021618. [Google Scholar]
- Naumov, S.; Valiullin, R.; Monson, P.A.; Kärger, J. Probing Memory Effects in Confined Fluids via Diffusion Measurements. Langmuir 2008, 24, 6429–6432. [Google Scholar] [CrossRef]
- Murase, K.; Fujiwara, T.; Umemura, Y.; Suzuki, K.; Iino, R.; Yamashita, H.; Saito, M.; Murakoshi, H.; Ritchie, K.; Kusumi, A. Ultrafine Membrane Compartments for Molecular Diffusion as Revealed by Single Molecule Techniques. Biophys. J. 2004, 86, 4075–4093. [Google Scholar] [CrossRef] [Green Version]
- Berrod, Q.; Hanot, S.; Guillermo, A.; Mossa, S.; Lyonnard, S. Water sub-diffusion in membranes for fuel cells. Sci. Rep. 2017, 7, 8326. [Google Scholar] [CrossRef] [Green Version]
- Dudko, O.K.; Berezhkovskii, A.M.; Weiss, G.H. Time-Dependent Diffusion Coefficients in Periodic Porous Materials. J. Phys. Chem. B 2005, 109, 21296–21299. [Google Scholar] [CrossRef] [PubMed]
- Gerber, G.; Bensouda, M.; Weitz, D.A.; Coussot, P. Self-Limited Accumulation of Colloids in Porous Media. Phys. Rev. Lett. 2019, 123, 158005. [Google Scholar] [CrossRef] [PubMed]
- Sandev, T.; Metzler, R.; Chechkin, A. From continuous time random walks to the generalized diffusion equation. Fract. Calc. Appl. Anal. 2018, 21, 10–28. [Google Scholar] [CrossRef] [Green Version]
- Sandev, T.; Tomovski, Z. Fractional Equations and Models; Springer International Publishing: Zurich, Switwerland, 2019. [Google Scholar]
- Liang, Y.; Wang, S.; Chen, W.; Zhou, Z.; Magin, R.L. A Survey of Models of Ultraslow Diffusion in Heterogeneous Materials. Appl. Mech. Rev. 2019, 71, 040802. [Google Scholar] [CrossRef]
- Yang, Q.H.; Yang, X.; Luo, M.B. Adsorption of polymer chains on heterogeneous surfaces with random adsorption sites. Polymer 2019, 180, 121677. [Google Scholar] [CrossRef]
- Morrin, G.T.; Schwartz, D.K. Three Regimes of Polymer Surface Dynamics under Crowded Conditions. Macromolecule 2018, 51, 1207–1214. [Google Scholar] [CrossRef]
- Dumazer, G.; Flekkøy, E.; Renard, F.M.C.; Angheluta, L. Transient anomalous diffusion regimes in reversible adsorbing systems. Phys. Rev. E 2017, 96, 042106. [Google Scholar] [CrossRef]
- Recanello, M.; Lenzi, E.K.; Martins, A.; Li, Q.; Zola, R. Extended adsorbing surface reach and memory effects on the diffusive behavior of particles in confined systems. Int. J. Heat Mass Transf. 2020, 151, 119433. [Google Scholar] [CrossRef]
- Guimarães, V.G.; Ribeiro, H.V.; Li, Q.; Evangelista, L.R.; Lenzi, E.K.; Zola, R.S. Unusual diffusing regimes caused by different adsorbing surfaces. Soft Matter 2015, 11, 1658–1666. [Google Scholar] [CrossRef]
- Höfling, F.; Franosch, T. Anomalous transport in the crowded world of biological cells. Rep. Prog. Phys. 2013, 76, 046602. [Google Scholar] [CrossRef] [Green Version]
- Saxton, M.J. A Biological Interpretation of Transient Anomalous Subdiffusion. I. Qualitative Model. Biophys. J. 2007, 92, 1178–1191. [Google Scholar] [CrossRef] [Green Version]
- Banks, D.S.; Fradin, C. Anomalous Diffusion of Proteins Due to Molecular Crowding. Biophys. J. 2005, 89, 2960–2971. [Google Scholar] [CrossRef] [Green Version]
- Fermandes, M.; Lenzi, E.K.; Evangelista, L.R.; Li, Q.; Zola, R.S.; de Souza, R. Diffusion and adsorption-deserntion phenomena in confined systems with periodically varying medium. Chem. Eng. Sci. 2021, 233, 116386. [Google Scholar] [CrossRef]
- Ribeiro, H.V.; Tateishi, A.A.; Alves, L.G.A.; Zola, R.S.; Lenzi, E.K. Investigating the interplay between mechanisms of anomalous diffusion via fractional Brownian walks on a comb-like structure. New J. Phys. 2014, 16, 093050. [Google Scholar] [CrossRef] [Green Version]
- Torquato, S. Diffusion and reaction among traps: Some theoretical and simulation results. J. Stat. Phys. 1991, 65, 1173–1206. [Google Scholar] [CrossRef]
- Lenzi, E.K.; Yednak, C.A.R.; Evangelista, L.R. Non-Markovian diffusion and the adsorption-desorption process. Phys. Rev. E 2010, 81, 011116. [Google Scholar] [CrossRef] [Green Version]
- Lenzi, E.K.; Evangelista, L.R.; Barbero, G.; Mantegazza, F. Anomalous diffusion and the adsorption-desorption process in anisotropic media. EPL (Europhys. Lett.) 2009, 85, 28004. [Google Scholar] [CrossRef]
- de Paula, J.L.; Santoro, P.A.; Zola, R.S.; Lenzi, E.K.; Evangelista, L.R.; Ciuchi, F.; Mazzulla, A.; Scaramuzza, N. Non-Debye relaxation in the dielectric response of nematic liquid crystals: Surface and memory effects in the adsorption-desorption process of ionic impurities. Phys. Rev. E 2012, 86, 051705. [Google Scholar] [CrossRef] [PubMed]
- Lenzi, E.K.; Lenzi, M.K.; Zola, R.; Ribeiro, H.; Zola, F.; Evangelista, L.; Gonçalves, G. Reaction on a solid surface supplied by an anomalous mass transfer source. Phys. A 2014, 410, 399–406. [Google Scholar] [CrossRef]
- Zola, R.S.; Lenzi, E.K.; Evangelista, L.R.; Barbero, G. Memory effect in the adsorption phenomena of neutral particles. Phys. Rev. E 2007, 75, 042601. [Google Scholar] [CrossRef] [PubMed]
- O’Shaughnessy, B.; Procaccia, I. Diffusion on fractals. Phys. Rev. A 1985, 32, 3073–3083. [Google Scholar] [CrossRef] [PubMed]
- O’Shaughnessy, B.; Procaccia, I. Analytical Solutions for Diffusion on Fractal Objects. Phys. Rev. Lett. 1985, 54, 455–458. [Google Scholar] [CrossRef] [PubMed]
- Richardson, L.F. Atmospheric diffusion shown on a distance-neighbour graph. Proc. Math. Phys. Eng. Sci. 1926, 110, 709–737. [Google Scholar]
- Boffetta, G.; Sokolov, I.M. Relative Dispersion in Fully Developed Turbulence: The Richardson’s Law and Intermittency Corrections. Phys. Rev. Lett. 2002, 88, 094501. [Google Scholar] [CrossRef] [Green Version]
- Daniel ben Avraham, S.H. Diffusion and Reactions in Fractals and Disordered Systems; CUP: Cambridge, UK, 2000. [Google Scholar]
- Su, N.; Sander, G.; Liu, F.; Anh, V.; Barry, D. Similarity solutions for solute transport in fractal porous media using a time- and scale-dependent dispersivity. App. Math. Model. 2005, 29, 852–870. [Google Scholar] [CrossRef] [Green Version]
- Brault, P.; Josserand, C.; Bauchire, J.M.; Caillard, A.; Charles, C.; Boswell, R.W. Anomalous Diffusion Mediated by Atom Deposition into a Porous Substrate. Phys. Rev. Lett. 2009, 102, 045901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wyld, H.W. Mathematical Methods for Physics, Advanced Book Program; Perseus Books: Reading, MA, USA, 1976. [Google Scholar]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Academic Press: Washington, DC, USA, 2007. [Google Scholar]
- Duffy, D.G. Transform Methods for Solving Partial Differential Equations; Chapman & Hall/CRC: Boca Raton, FL, USA, 2004. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koltun, A.P.S.; Lenzi, E.K.; Lenzi, M.K.; Zola, R.S. Diffusion in Heterogenous Media and Sorption—Desorption Processes. Fractal Fract. 2021, 5, 183. https://doi.org/10.3390/fractalfract5040183
Koltun APS, Lenzi EK, Lenzi MK, Zola RS. Diffusion in Heterogenous Media and Sorption—Desorption Processes. Fractal and Fractional. 2021; 5(4):183. https://doi.org/10.3390/fractalfract5040183
Chicago/Turabian StyleKoltun, Ana Paula S., Ervin Kaminski Lenzi, Marcelo Kaminski Lenzi, and Rafael Soares Zola. 2021. "Diffusion in Heterogenous Media and Sorption—Desorption Processes" Fractal and Fractional 5, no. 4: 183. https://doi.org/10.3390/fractalfract5040183
APA StyleKoltun, A. P. S., Lenzi, E. K., Lenzi, M. K., & Zola, R. S. (2021). Diffusion in Heterogenous Media and Sorption—Desorption Processes. Fractal and Fractional, 5(4), 183. https://doi.org/10.3390/fractalfract5040183








