Numerical Solution of Fractional Elliptic Problems with Inhomogeneous Boundary Conditions
Abstract
1. Introduction
2. Materials and Methods
3. Results
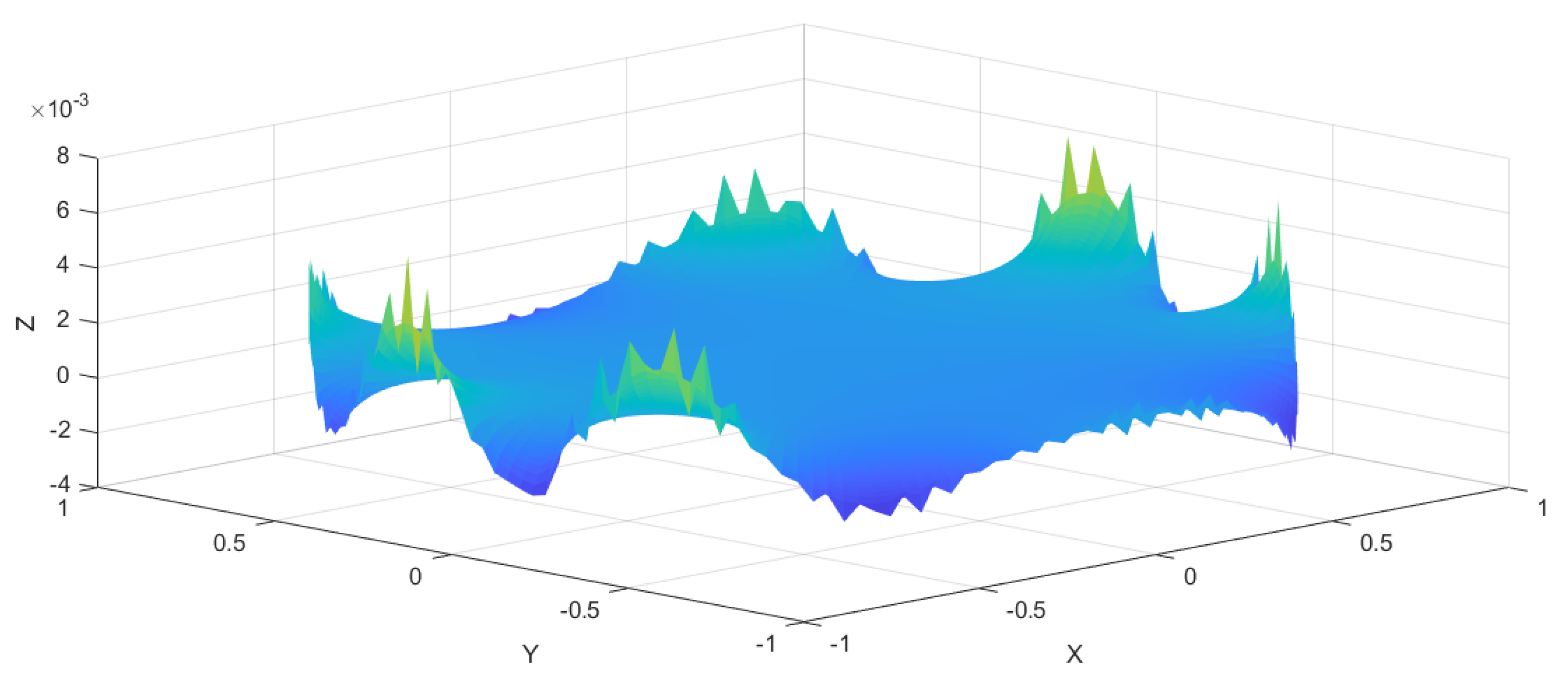
3.1. Error Analysis
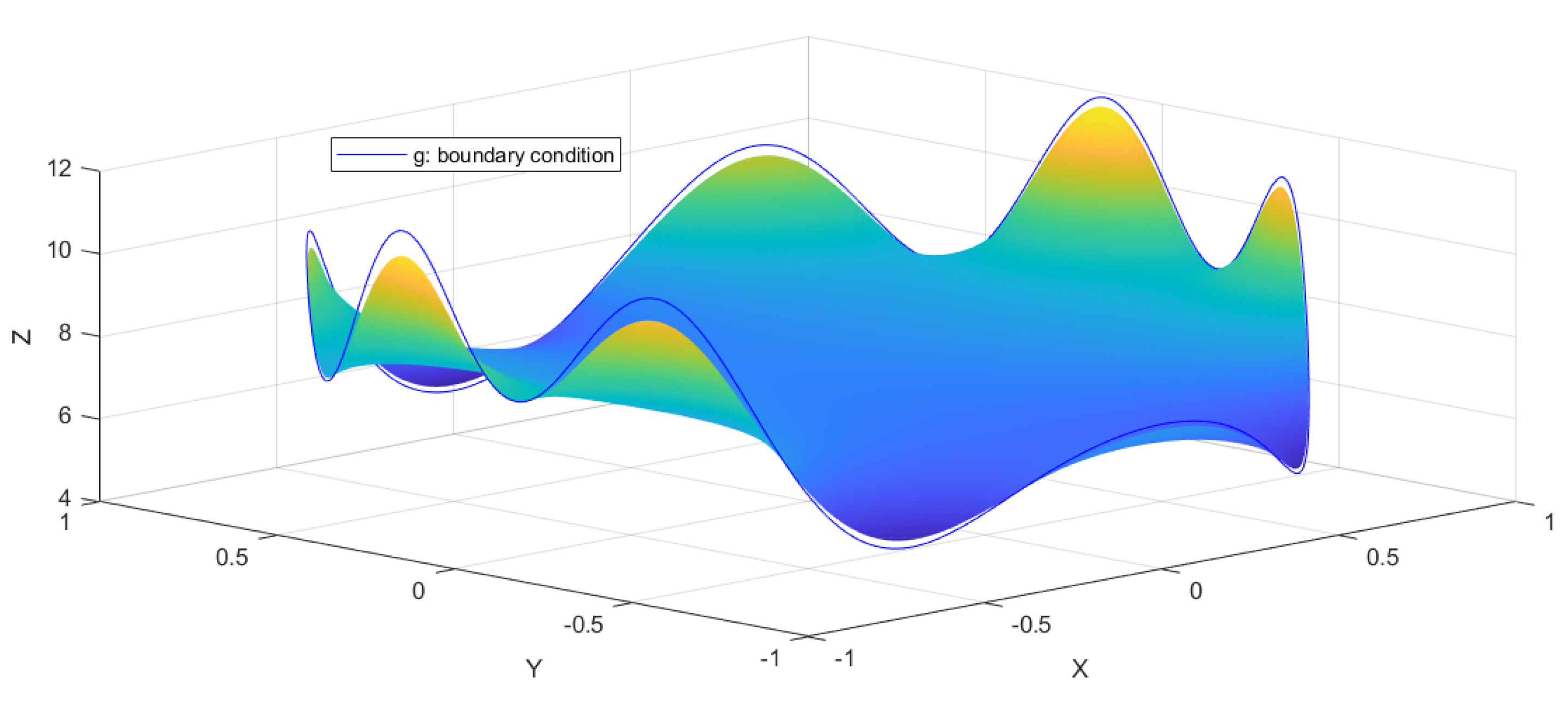
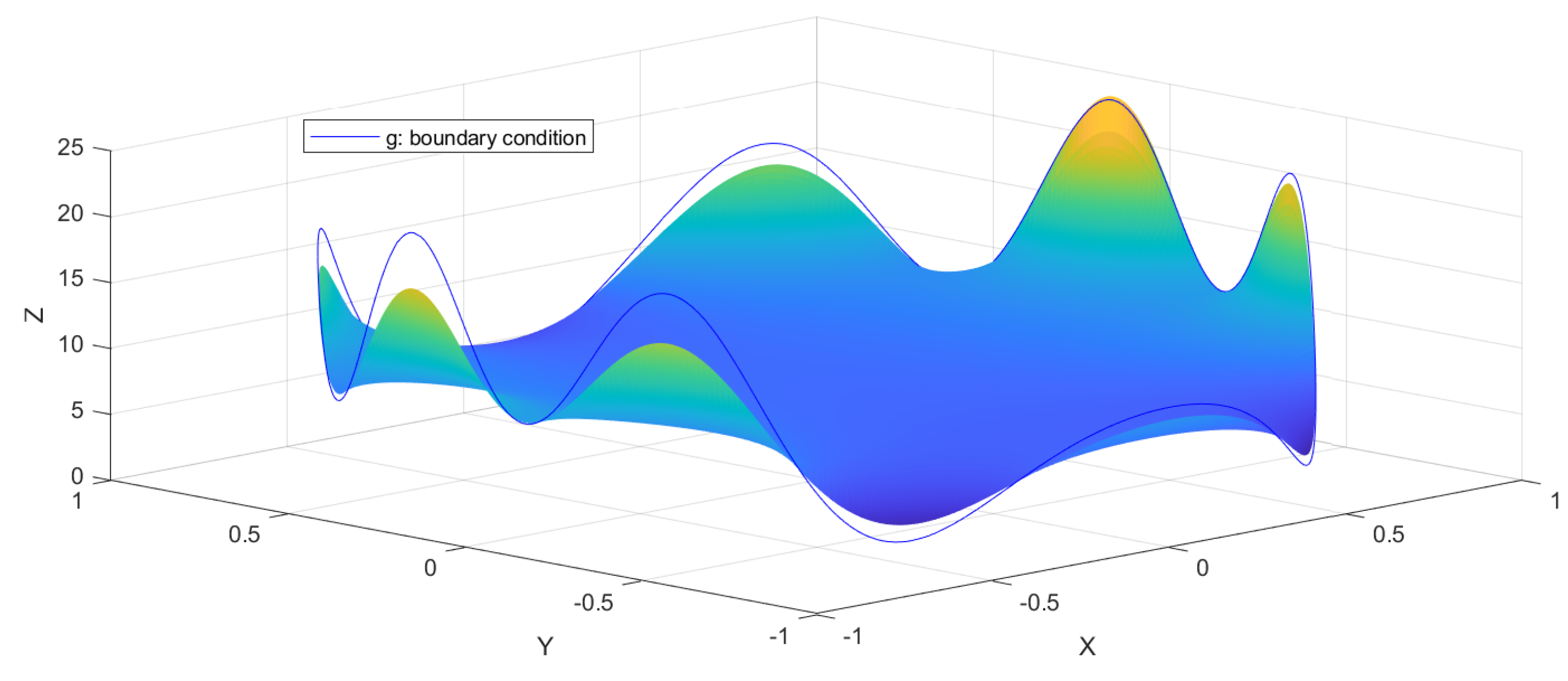
3.2. Numerical Experiments
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Schumer, R.; Benson, D.A.; Meerschaert, M.M.; Wheatcraft, S.W. Eulerian derivation of the fractional advection-dispersion equation. J. Contam. Hydrol. 2001, 48, 69–88. [Google Scholar] [CrossRef]
- Banks, D.S.; Fradin, C. Anomalous Diffusion of Proteins Due to Molecular Crowding. Biophys. J. 2005, 89, 2960–2971. [Google Scholar] [CrossRef] [PubMed]
- Glusa, C.; Antil, H.; D’Elia, M.; Waanders, B.v.; Weiss, C.J. A Fast Solver for the Fractional Helmholtz Equation. SIAM J. Sci. Comput. 2021, 43, 1362–1388. [Google Scholar] [CrossRef]
- Hapca, S.; Crawford, J.W.; Young, I.M. Anomalous diffusion of heterogeneous populations characterized by normal diffusion at the individual level. J. R. Soc. Interface 2009, 30, 111–122. [Google Scholar] [CrossRef] [PubMed]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection-dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef]
- Woringer, M.; Darzacq, X. Protein motion in the nucleus: From anomalous diffusion to weak interactions. Biochem. Soc. Trans. 2018, 46, 945–956. [Google Scholar] [CrossRef] [PubMed]
- Kwaśnicki, M. Ten equivalent definitions of the fractional Laplace operator. Fract. Calc. Appl. Anal. 2017, 20, 7–51. [Google Scholar] [CrossRef]
- Servadei, R.; Valdinoci, E. On the spectrum of two different fractional operators. Proc. Roy. Soc. Edinburgh Sect. A 2014, 144, 831–855. [Google Scholar] [CrossRef]
- Izsák, F.; Szekeres, B.J. Models of space-fractional diffusion: A critical review. Appl. Math. Lett. 2017, 71, 38–43. [Google Scholar] [CrossRef]
- Harizanov, S.; Lazarov, R.; Margenov, S.; Marinov, P. Numerical solution of fractional diffusion–reaction problems based on BURA. Comput. Math. Appl. 2020, 80, 316–331. [Google Scholar] [CrossRef]
- Izsák, F.; Szekeres, B.J. Efficient computation of matrix power-vector products: Application for space-fractional diffusion problems. Appl. Math. Lett. 2018, 86, 70–76. [Google Scholar] [CrossRef]
- Vabishchevich, P.N. Numerical solution of time-dependent problems with fractional power elliptic operator. Comput. Method Appl. Math. 2018, 18, 111–128. [Google Scholar] [CrossRef]
- Acosta, G.; Bersetche, F.M.; Borthagaray, J.P. A short FE implementation for a 2d homogeneous Dirichlet problem of a fractional Laplacian. Comput. Math. Appl. 2017, 74, 784–816. [Google Scholar] [CrossRef]
- D’Elia, M.; Du, Q.; Glusa, C.; Gunzburger, M.; Tian, X.; Zhou, Z. Numerical methods for nonlocal and fractional models. Acta Numer. 2020, 29, 1–12. [Google Scholar] [CrossRef]
- Abatangelo, N.; Dupaigne, L. Nonhomogeneous boundary conditions for the spectral fractional Laplacian. Ann. Inst. H. Poincaré Anal. Non Linéaire 2017, 34, 439–467. [Google Scholar] [CrossRef]
- Lischke, A.; Pang, G.; Gulian, M.; Song, F.; Glusa, C.; Zheng, X.; Mao, Z.; Cai, W.; Meerschaert, M.M.; Ainsworth, M.; et al. What is the fractional Laplacian? A comparative review with new results. J. Comput. Phys. 2020, 404, 109009. [Google Scholar] [CrossRef]
- Harizanov, S.; Margenov, S.; Popivanov, N. Spectral Fractional Laplacian with Inhomogeneous Dirichlet Data: Questions, Problems, Solutions. In Advanced Computing in Industrial Mathematics; Georgiev, I., Kostadinov, H., Lilkova, E., Eds.; Springer: Berlin/Heidelberg, Germany, 2018; Volume 961. [Google Scholar] [CrossRef]
- Chang, T. Boundary integral operator for the fractional Laplace equation in a bounded Lipschitz domain. Integr. Equat. Oper. Th. 2012, 72, 345–361. [Google Scholar] [CrossRef][Green Version]
- Izsák, F.; Maros, G. Fractional order elliptic problems with inhomogeneous Dirichlet boundary conditions. Fract. Calc. Appl. Anal. 2020, 23, 378–389. [Google Scholar] [CrossRef]
- Adams, R.; Fournier, J. Sobolev Spaces; Academic Press: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Steinbach, O. Numerical Approximation Methods for Elliptic Boundary Value Problems. Finite and Boundary Elements; Springer: New York, NY, USA, 2008. [Google Scholar]
- McLean, W. Strongly Elliptic Systems and Boundary Integral Equations; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Mathon, R.; Johnston, R.L. The approximate solution of elliptic boundary-value problems by fundamental solutions. SIAM J. Numer. Anal. 1977, 14, 638–650. [Google Scholar] [CrossRef]

| h | |||||||
| error | |||||||
| rate | − |
| h | |||||||
| error | |||||||
| rate | − |
| h | |||||||
| error | |||||||
| rate | − |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maros, G.; Izsák, F. Numerical Solution of Fractional Elliptic Problems with Inhomogeneous Boundary Conditions. Fractal Fract. 2021, 5, 75. https://doi.org/10.3390/fractalfract5030075
Maros G, Izsák F. Numerical Solution of Fractional Elliptic Problems with Inhomogeneous Boundary Conditions. Fractal and Fractional. 2021; 5(3):75. https://doi.org/10.3390/fractalfract5030075
Chicago/Turabian StyleMaros, Gábor, and Ferenc Izsák. 2021. "Numerical Solution of Fractional Elliptic Problems with Inhomogeneous Boundary Conditions" Fractal and Fractional 5, no. 3: 75. https://doi.org/10.3390/fractalfract5030075
APA StyleMaros, G., & Izsák, F. (2021). Numerical Solution of Fractional Elliptic Problems with Inhomogeneous Boundary Conditions. Fractal and Fractional, 5(3), 75. https://doi.org/10.3390/fractalfract5030075







