1. Introduction
Fractional calculus has undergone rapid developments in its theory, methods, and applications in recent decades due to its capability of modeling memory phenomena and hereditary properties. Its application range includes viscoelastic mechanics [
1,
2,
3], anomalous diffusion-related problems [
2,
3], control theory [
4,
5], vibration theory [
6], signal processing [
7], bioengineering [
8], data fitting [
9], and so on. Interpretations that use physics and geometry for fractional calculus have been developed [
10,
11]. Torbati and Hammond [
10] applied fractional calculus to research on fractals to model self-similarity, hereditary effects, and partial dissipation. Podlubny [
11] introduced two kinds of time—the equably flowing homogeneous individual time and the cosmic inhomogeneous time—to show that the fractional derivative describes the relationship between the real distance and the individual speed of a moving object.
Fractional calculus has been applied to viscoelasticity theory since the middle of the last century. Its wide uses and the importance of viscoelastic materials have promoted the theoretical development of fractional calculus. Scott-Blair [
1] suggested a fractional constitutive relation for viscoelastic bodies with a fractional derivative of order
, which represents the mechanical properties between elastic solids and viscous fluids. The fractional model is referred to as the Scott-Blair model [
2] and represents a “spring-pot" element [
12], which is homologous with the dashpot element. In [
2], fractional constitutive models describing viscoelastic behavior, including the fractional Kelvin–Voigt, Maxwell, and Zener models, were considered. In [
13], a fractional Maxwell relation was used to model the flow of a superpolymer. In [
14], a fractional constitutive relation with five parameters was investigated for high-frequency polymeric materials. In addition, a six-parameter fractional constitutive model was proposed and analyzed [
15].
Fractional oscillation or vibration problems have attracted the interest of many scholars, such as Caputo [
16], Bagley and Torvik [
17], Beyer and Kempfle [
18], and more [
19,
20,
21,
22]. Li et al. [
20] considered impulse response and stability for fractional oscillation. In [
21], equivalent integer-order equations were presented for three types of fractional oscillators. In [
22], fractional vibration and Brownian motion were compared. In [
23], steady-state responses to harmonic and periodic excitations in a fractional vibration system were given. Shen et al. [
24], Li et al. [
25], and Wang and Hu [
26] explored the dynamics and stability of a fractional-order system.
In research on dissipation mechanisms, Caputo [
16,
27] generalized fractional derivatives to a situation where the fractional-order derivatives are integrated with respect to the order of differentiation, which is currently called the distributed-order derivative. In [
28], the distributed-order derivative was used in the constitutive relation of dielectric media with model diffusion. In [
29], solutions of distributed-order differential equations were considered. In [
30], mechanical models of a viscoelastic body with distributed-order derivatives were analyzed. In [
31], the properties of the solutions for a distributed-order oscillator system were investigated. In [
32], the steady-state periodic responses of a distributed-order vibration system were considered.
For a piecewise continuous function
on
, the Riemann–Liouville fractional integral is defined as
and
where
is the Gamma function,
There exist different definitions for fractional derivatives. The
th-order Riemann–Liouville fractional derivative is defined, when it exists, as
The
th-order Caputo fractional derivative is defined as
In this article, we employ fractional integrals and derivatives with support in the real set
. That is, the lower limit in the fractional integral is taken as
. In this case, the two fractional derivatives in Equations (
2) and (
3) are consistent [
4]. We denote them uniformly as
. Such cases are also called the Liouville–Weyl fractional integral and derivative [
2]. With such definitions, the steady-state solutions of a vibration system can be directly obtained. We note that the steady-state solutions are more significant than the transient solution in the vibration mechanics. Moreover, the solution can be considered within the field of elementary functions.
If
is a non-negative integer,
will be used to represent the integer-order derivative
. We notice the convenient formula [
2,
4]:
For comparison, we review the classical vibration system with the harmonic excitation
where i is the imaginary unit and
are all positive constants. The steady-state response is
where
is the phase difference between the excitation and the response within the range
, and it satisfies
The phase difference may be explicitly given in the following equation:
where
is the principal value of the inverse tangent function with the range
.
In fractional constitutive relations and their simulations of the oscillations of viscoelastic bodies, the order of the fractional derivative is usually taken between 0 and 1 to characterize both the viscosity and the elasticity (e.g., in [
32,
33]). In [
34], a fractional vibration equation was considered, and it was found that if the order satisfies
, the fractional derivative contributes to both the viscosity and the inertia, i.e., the viscoinertia, corresponding to the terminology of viscoelasticity. We will continue this research on the subject of the fractional calculus and the viscoinertia and will include both the case of the fractional order and the case of the distributed order in this paper.
We note that there is a mechanical element, the inerter [
35,
36,
37]—analogous to the spring or dashpot—which has two endpoints, one of which can shift relative to the other end. However, the magnitude of the external force depends on the relative acceleration of the two ends. Inerters have been applied to vehicle suspension, motorcycle steering damping systems, and other structures [
38,
39]. In [
40], effects of inerter-based isolators were shown to have some advantages over the effects of traditional dynamic absorbers if the same mass ratio was applied. In [
41], multichannel inertial absorbers were distributed on a panel to implement vibration control in a wide frequency range. In [
42], Wang et al. presented a hydraulic inerter with an external helical tube and found that in high-frequency stimulation, the hydraulic inerter could be treated as a component with a damper and an inerter in parallel.
In this article, a fractional derivative model of these dampers with inertia is presented. We focus on the effects of the fractional derivative and the distributed-order derivative on the viscosity and the inertia in a vibration system. Both the fractional and distributed-order derivatives play the role of a damper and an inerter in parallel. In the next section, we consider a forced harmonic vibration system with the fractional-order derivative , where . We derive the equivalent integer-order vibration system and discuss the contribution of damping and that of mass, as well as the frequency–amplitude response and frequency-phase response.
In
Section 3, we further investigate the harmonic vibration system with the distributed-order derivative
, where
is a weight function of the order
. The distributed-order derivative enhances the viscosity and inertia of the system, contributes to the damping and mass, and thus represents an “inerter-pot” element. The equivalent damping, the equivalent mass, and the steady-state response are expressed with the general weight function
. Compared with the fractional case, the weight function
influences the viscosity and inertia of the system instead of the order
in
Section 2.
In
Section 4, we explore a distributed-order vibration model where the weight function is taken as an exponential function involving the parameter
p,
. Detailed analyses of the weight function, the contribution of damping, and the contribution of mass are given. Frequency–amplitude curves and the frequency-phase curves are plotted for various coefficients and parameters for comparison with the fractional case in
Section 2.
Section 5 presents our conclusions.
2. Fractional-Order Vibration Characterizing Viscoinertia
Consider a fractional-order vibration system with a complex harmonic excitation
where
are positive real constants, i is the imaginary unit, the order
is restricted on the interval
, and
is the system response to be determined.
Similarly to the case of integer orders, the form of the response is assumed to be
where
X is independent of
t and is called the complex amplitude. Substituting the integer-order derivatives and the fractional derivative into Equation (
9) and eliminating the nonzero factor
, we solve for the amplitude
X as
Using the equality
, we rewrite the complex amplitude as
Since
,
and the equality in the first expression holds only for
, while the equality in the second expression holds only for
. Thus, the two terms generated from the fractional derivative enhance the viscosity and inertia, i.e., they contribute to the damping and mass, respectively. In view of this, we introduce the following equivalent damping and equivalent mass as
Using the equivalent damping and equivalent mass, Equation (
12) has the form
Further, we denote the denominator as the exponential form
where the phase difference
between the excitation and the response is determined as
Thus, substituting Equation (
15) into Equation (
10), we obtain the response of the system to complex harmonic excitation:
As regards the steady-state response, the fractional-order vibration system (
9) is equivalent to the integer-order system
where the coefficients
and
are frequency-dependent. We refer to
as the damping contribution and the mass contribution, respectively. In
Figure 1 and
Figure 2, the curves of the damping contribution and the mass contribution versus the order
are shown for
and different values of
.
At the two endpoints of
, the equivalent damping and equivalent mass satisfy the properties
From the derivatives
we obtain the following conclusions.
Proposition 1. (i) When the damping contribution is a strictly decreasing function of λ; when , reaches a peak within the interval . (ii) When the mass contribution reaches a peak within the interval ; when , is a strictly increasing function of λ.
From Equation (
17), the magnification factor of the amplitude is derived as a function of
,
where
denotes the static elongation of the spring for the applied force
. Equations (
24) and (
16) are also called the frequency–amplitude response and frequency-phase response, respectively. They completely determine the response features of the system.
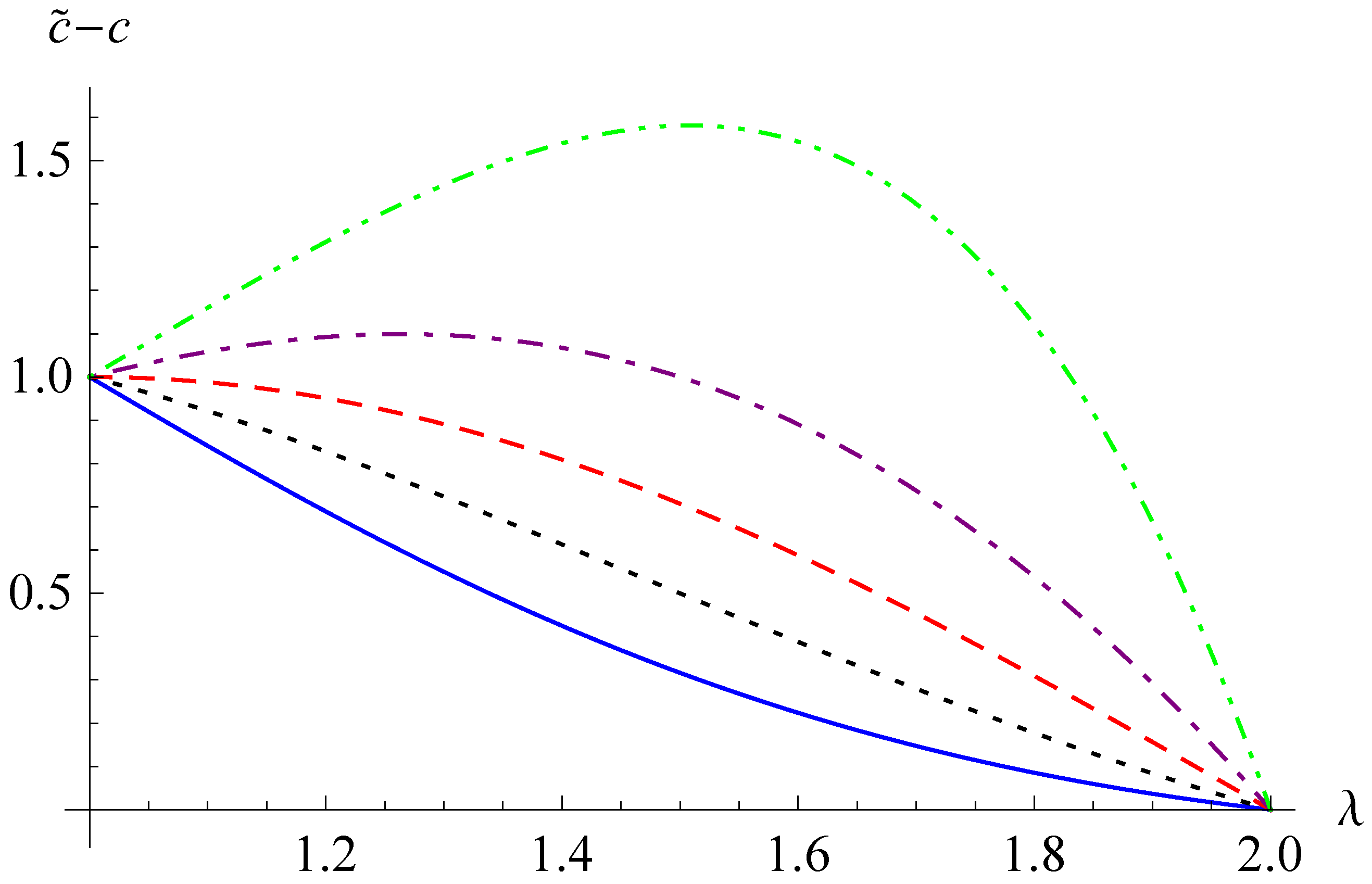
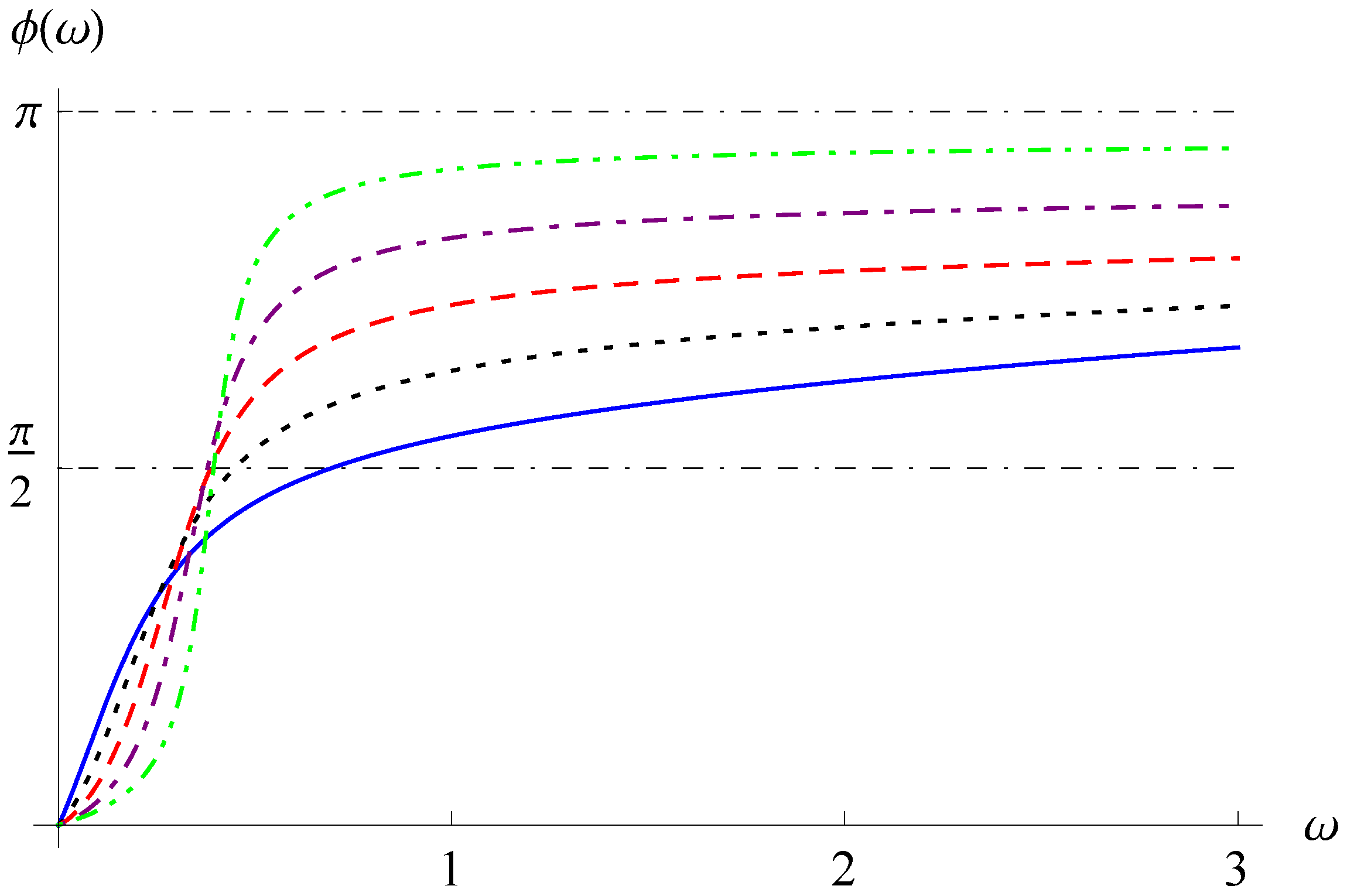
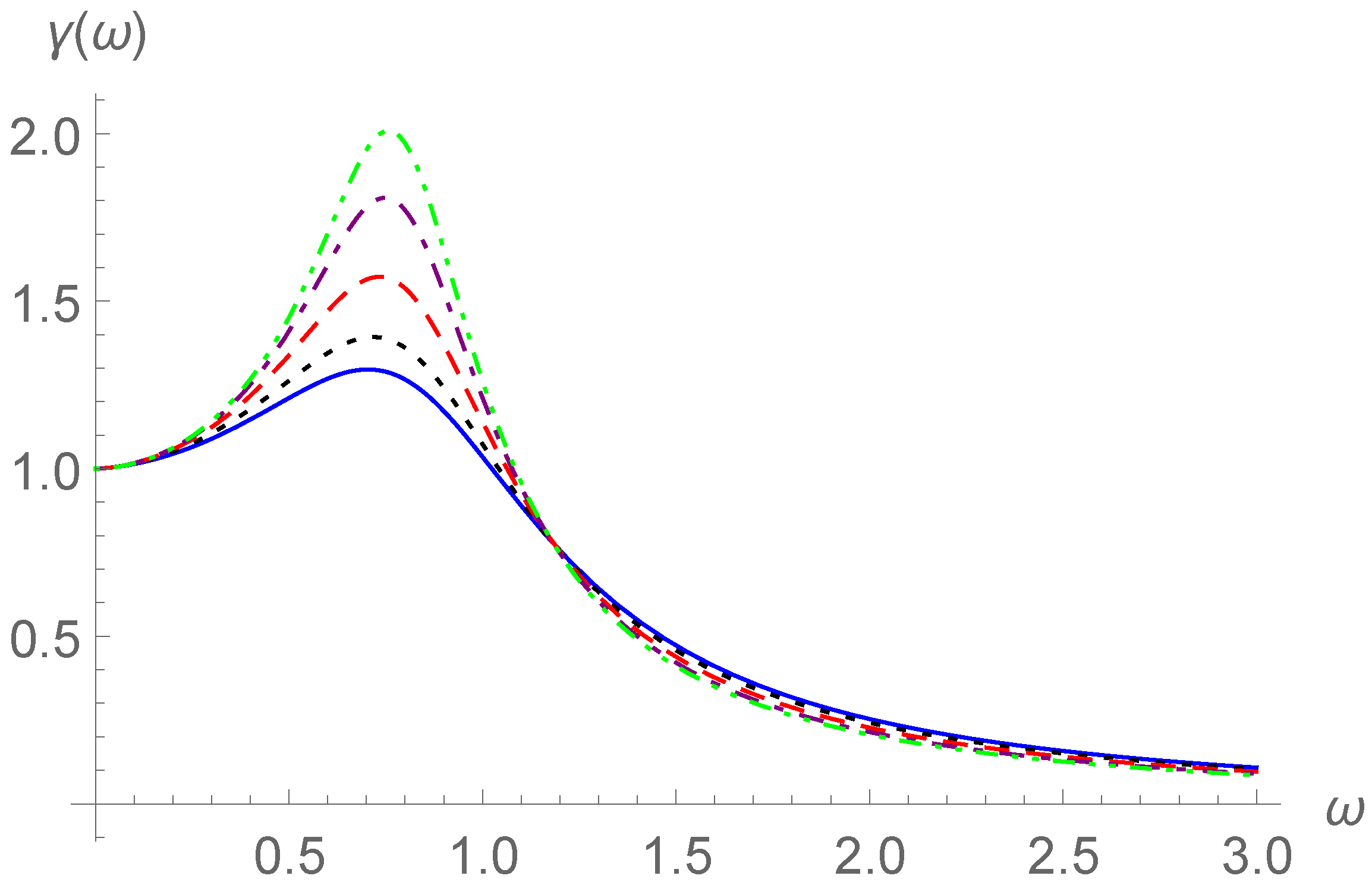
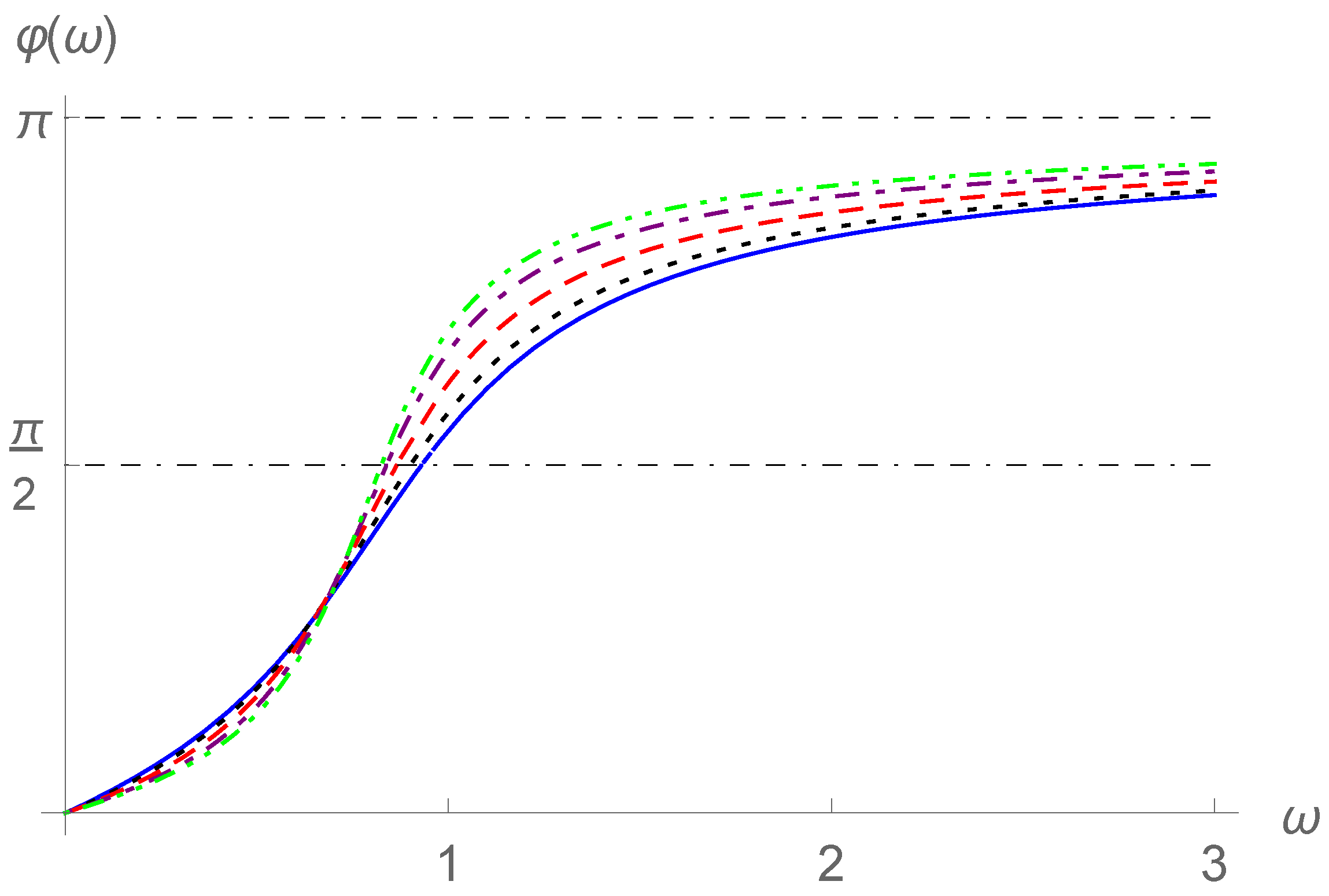
We take
,
, and
and 5 to explore the frequency–amplitude response and the frequency-phase response. In
Figure 3 and
Figure 4, the frequency–amplitude curves are shown for
and 5, respectively, and for different values of
. In
Figure 5 and
Figure 6, the frequency-phase curves are displayed for
and 5, respectively, and for different values of
. From
Figure 3,
Figure 4,
Figure 5 and
Figure 6, the increase in the order
enhances the values of the resonance humps and makes the changes in the phase differences following
more sensitive. In addition, the resonance humps in
Figure 4 are shifted to the left compared with those in
Figure 3, and the growth spurts of the phase differences appear for smaller values of
in
Figure 6 than in
Figure 5.
3. Distributed-Order Vibration Characterizing Viscoinertia
Consider a forced harmonic vibration system equipped with the distributed-order derivative:
where
are positive real constants, and
is the weight function of the order
that satisfies
We note that the constraint (27) is not a prerequisite for a distributed-order derivative. Here,
is regarded as a weight function of the order
. It includes the special case of the Dirac
-function
In this case, Equation (
25) degenerates to the fractional case in the last section.
Similarly to the last section, we suppose that the response is
Substituting it into Equation (
25) and eliminating the common factor
, we obtain the complex amplitude as
The integration in Equation (
28) may be decomposed into
where the imaginary part and the real part satisfy
Therefore, the distributed-order derivative enhances the viscosity and inertia and contributes to the damping and mass as well. We introduce the equivalent damping and equivalent mass as
That is,
and
are the damping contribution and mass contribution, respectively. Then, Equation (
28) has the equivalent expression
and the equivalent integer-order system of (
25) is
Therefore, we obtain the system response to complex harmonic excitation:
where the phase difference between the excitation and the response satisfies
From (
35), the magnification factor of the amplitude is
In next section, the weight function is specified to analyze these results.
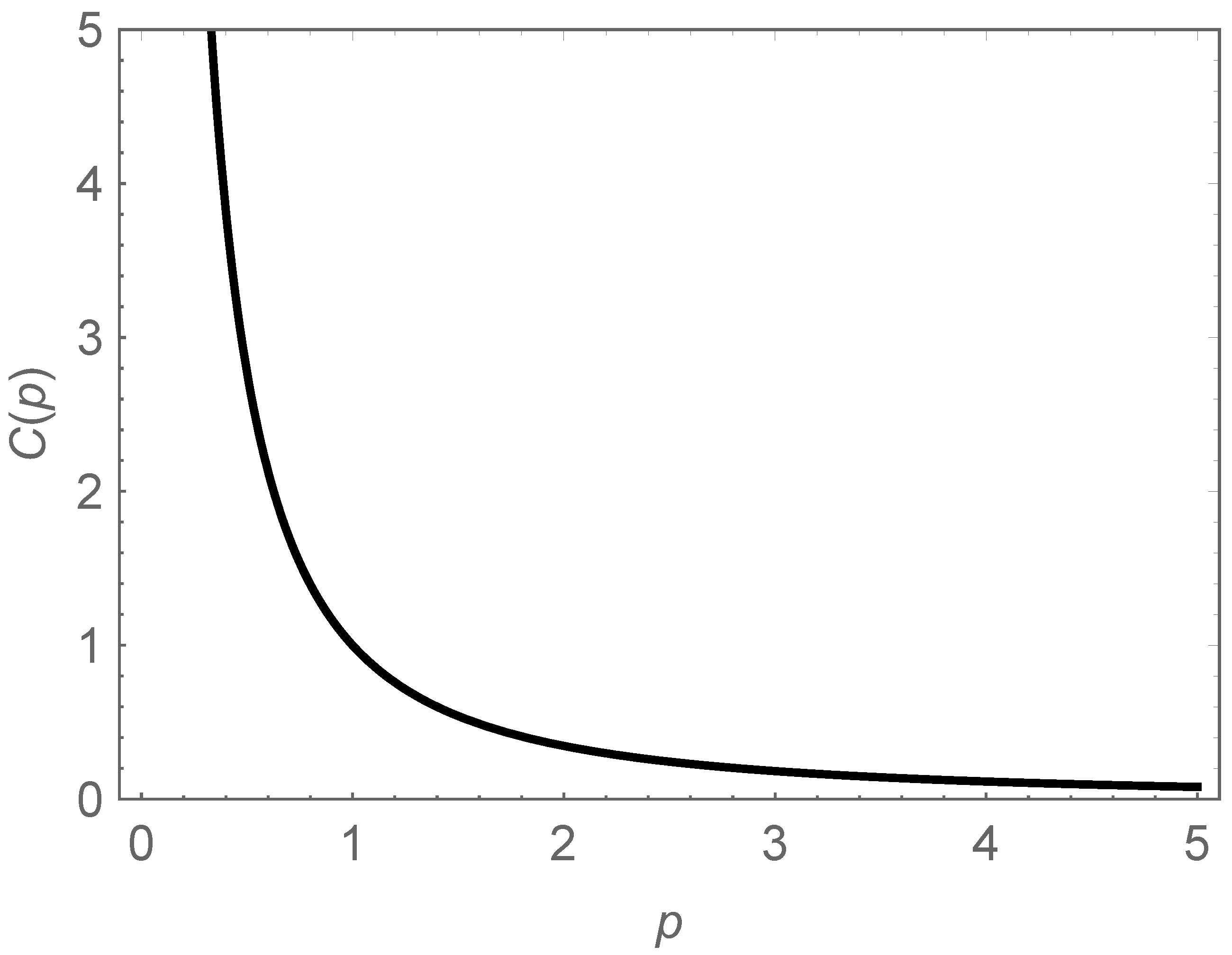
4. Results for the Weight Function in the Form of a Parametrized Exponential Function
The weight function
can be specified in infinitely many ways. In this section, we consider the weight function in the form of an exponential involving the parameter
p as
where the coefficient is
It is easy to verify that
satisfies the conditions for a weight function in Equations (
26) and (27). Choosing such a weight function can adequately express the shift of the order on the interval
. Meanwhile, this weight function is easy to deal with in the following calculations. In
Figure 7, we plot the curve of
versus
p on the interval
. In
Figure 8, we display the curves of
versus
on the interval
for the values
0.01, 0.1, 1, 10, and 100. For
, the weight function
is the constant unity function.
In order to gain further understanding of the coefficient and the parametrized weight , we give the following two propositions.
Proposition 2. is positive, monotonically decreasing, and differentiable on the interval
Proof. It is direct that
whenever
and
is continuous on the interval
. By calculating the first-order derivative, we have
By the L’Hospital’s rule, we obtain the limitation Thus, we have , so is differentiable on the interval
In order to show that
is monotonically decreasing, we let
, the numerator in Equation (
40). Then,
It is clear that
has a unique zero at
, and
reaches the minimum at
as
Thus, we have
for
. Therefore, we derive that
for all
This means that
is monotonically decreasing. □
Proposition 3. (i) The curves of versus λ and versus λ are symmetrical about the vertical line .
(ii) For , decreases monotonically on , while for , increases monotonically on .
(iii) decreases monotonically on , while increases monotonically on .
Proof . (i) The relation
may be verified via direct substitution. (ii) From the sign of the derivative
, we can obtain the monotonicity of the weight function
on the interval
. (iii) First, from
we calculate the derivative as
It is easy to verify that for and . Thus, ensures that is monotonically decreasing on . The monotonic increase in follows from the symmetry property in (i). The proof is complete. □
Considering the symmetry property stated in Proposition 3 (i) and shown in
Figure 8, we will use the logarithmic scale of the parameter
p whenever plotting the
p axis. Substituting the weight function in Equation (
38) into (
31) and (32) and calculating the integrals, we obtain the damping contribution and the mass contribution for the case of the distributed-order derivative:
It is easy to verify that the equivalent damping and equivalent mass satisfy the following initial value and final value with respect to the parameter
p:
In
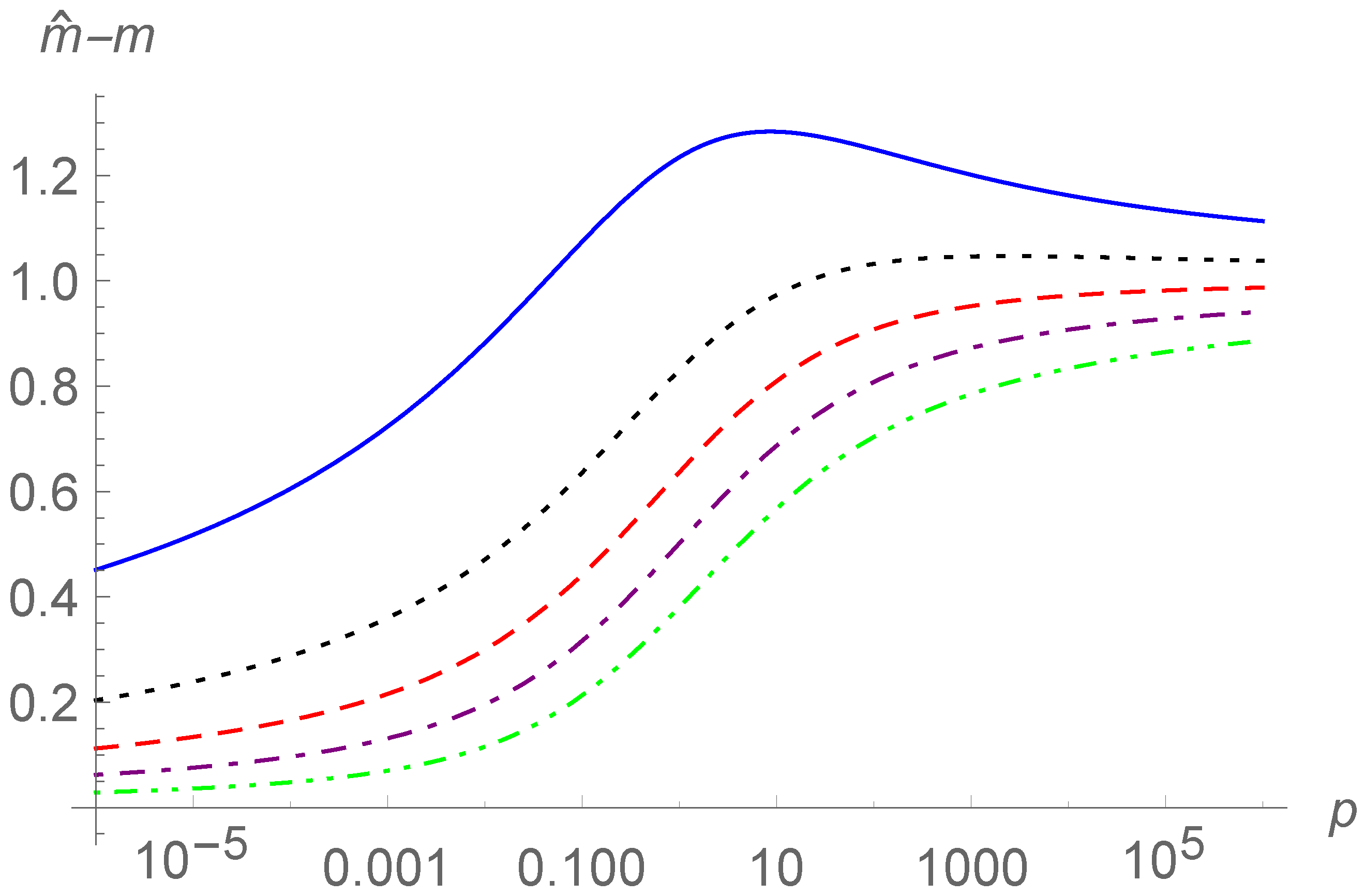
Figure 9, the curves of the damping contribution
versus
p are depicted for
and different values of
. In
Figure 10, the curves of the mass contribution
versus
p for
and different values of
are shown. Here, logarithmic scales are used for the
p axes with the range
Compared with the single fractional case in
Section 2, there are similar properties between the fractional order and the distributed order as far as the damping contribution and mass contribution.
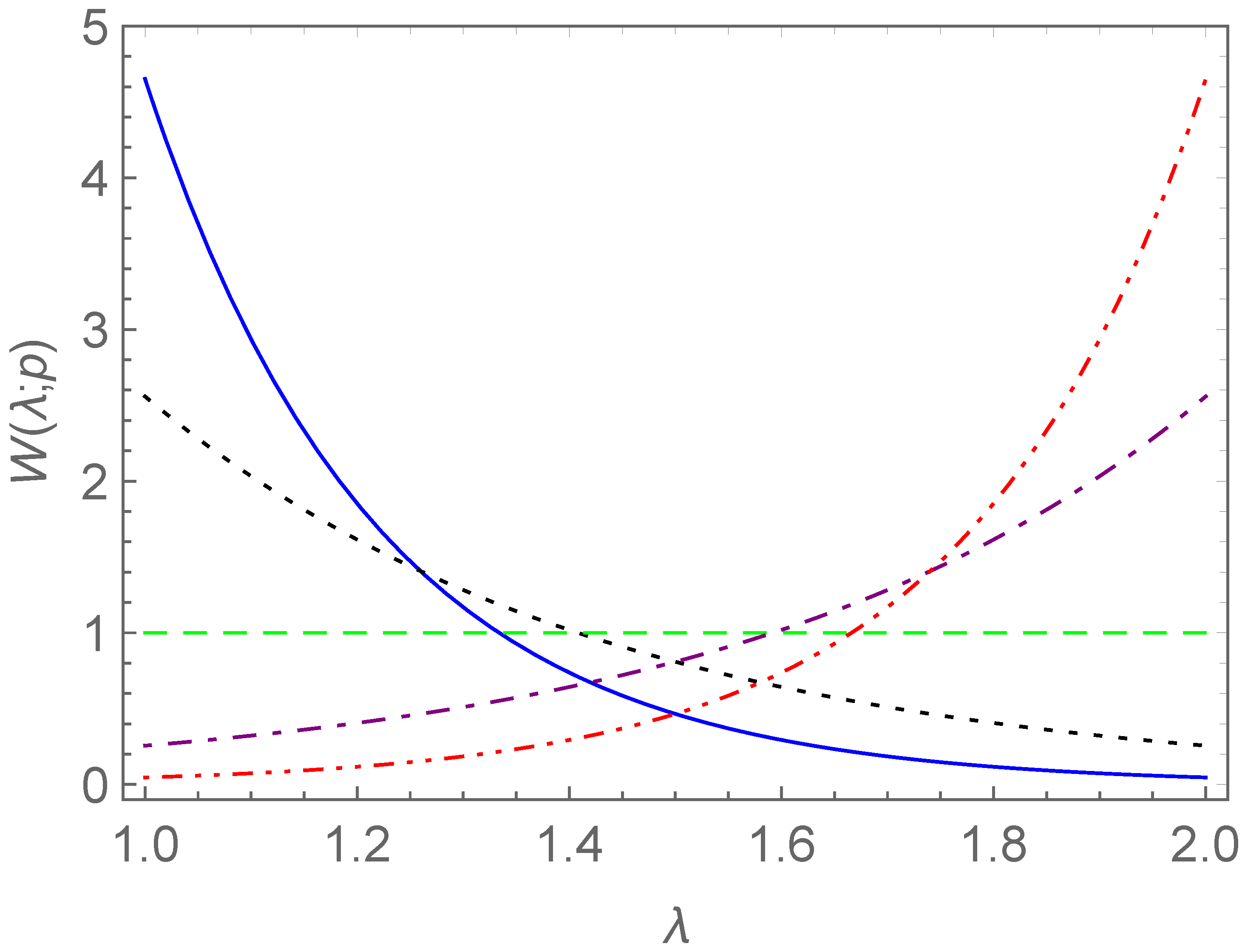
By using the equivalent damping
and the equivalent mass
in Equations (
43) and (44), the system response
, the frequency–amplitude response
, and the frequency-phase response
can be calculated from Equations (
35)–(
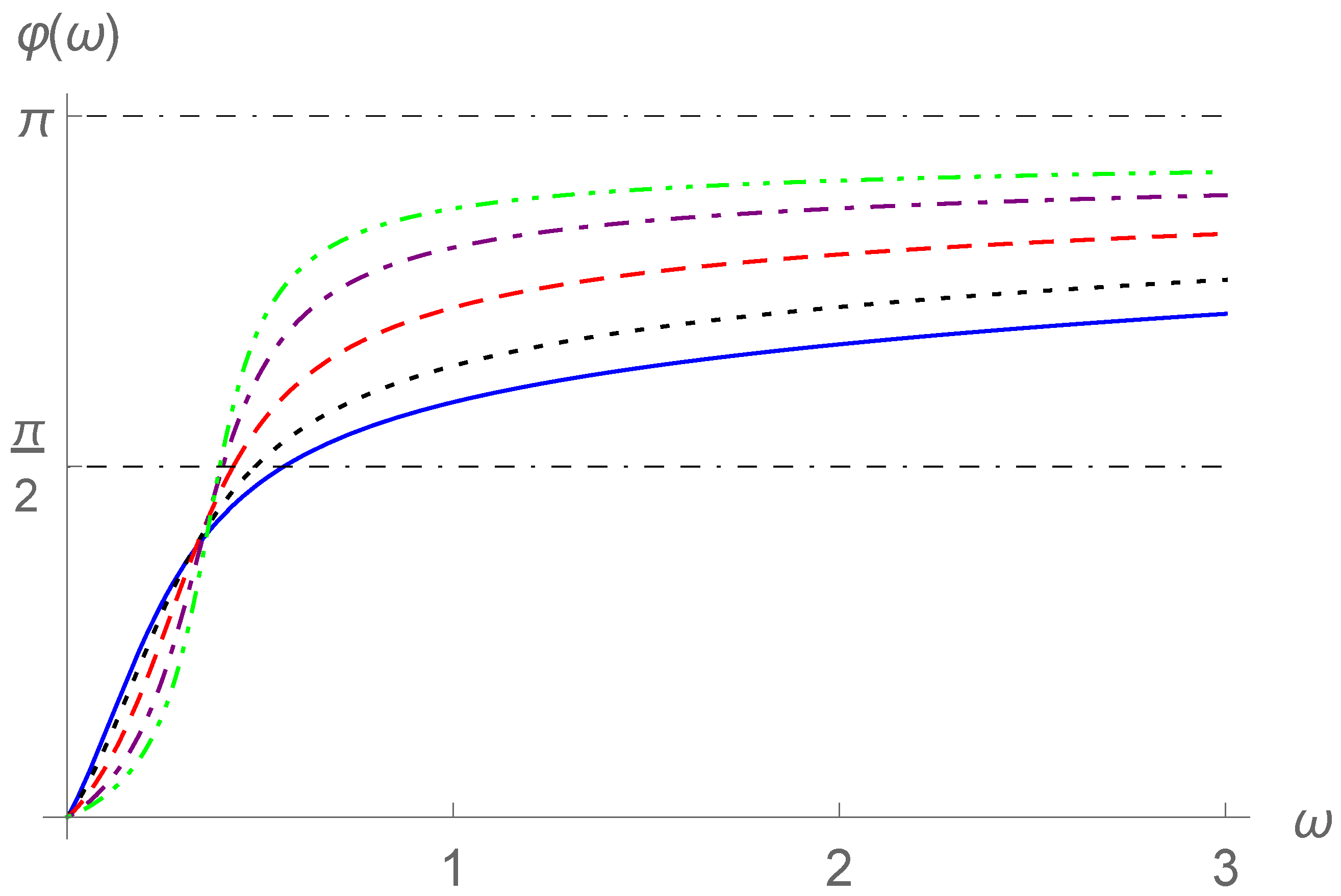
37). We take
,
, and
and 5 to examine the frequency–amplitude response and frequency-phase response for different values of the parameter
p. In
Figure 11 and
Figure 12, the frequency–amplitude curves are shown for
and 5, respectively. In
Figure 13 and
Figure 14, the frequency-phase curves are displayed for
and 5, respectively.
5. Conclusions
We considered forced harmonic vibration systems with a fractional-order derivative where and a distributed-order derivative where is a weight function of the order . Both of the two types of derivatives enhance the viscosity and inertia of the system, contribute to damping and mass, and play the role of a damper and an inerter in parallel. Hence, such types of derivatives characterize the viscoinertia and represent an “inerter-pot”, corresponding to a “spring-pot" in viscoelasticity.
In the distributed-order vibration system, the weight function of the order defined by Equations (
26) and (27) can be given in infinitely many ways. Instead of the order
in the fractional-order vibration model, we discuss the effects of the weight function
on the system. The distributed-order vibration model is more self-directed and flexible than the fractional vibration system. In particular, the fractional vibration system is a special case of the distributed-order vibration if the weight function is the Dirac
-function
,
.
For the fractional-order and the distributed-order vibration systems characterizing viscoinertia, we derived the equivalent damping and equivalent mass and gave the equivalent integer-order vibration systems. Further, we considered the damping contribution and the mass contribution of the fractional-order derivative and the distributed-order derivative, the frequency–amplitude response, and the frequency-phase response. Particularly, for the distributed-order vibration model where the weight function was taken as an exponential function involving the parameter p, , we gave detailed analyses for the weight function, the damping contribution, and the mass contribution. The frequency–amplitude curves and the frequency-phase curves were plotted for the two types of vibration models, and the various model coefficients and parameters were used for the comparison of the two vibration models.