Orthonormal Ultraspherical Operational Matrix Algorithm for Fractal–Fractional Riccati Equation with Generalized Caputo Derivative

Abstract

Herein, we developed and analyzed a new fractal–fractional (FF) operational matrix for orthonormal normalized ultraspherical polynomials. We used this matrix to handle the FF Riccati differential equation with the new generalized Caputo FF derivative. Based on the developed operational matrix and the spectral Tau method, the nonlinear differential problem was reduced to a system of algebraic equations in the unknown expansion coefficients. Accordingly, the resulting system was solved by Newton’s solver with a small initial guess. The efficiency, accuracy, and applicability of the developed numerical method were checked by exhibiting various test problems. The obtained results were also compared with other recent methods, based on the available literature.

1. Introduction

Since a few years ago and to this day, some researchers have started focusing on old stable methodologies such as finite difference and finite element [1,2,3,4,5]. The main drawback of these methods is their limited accuracy, which has motivated some researchers to dedicated significant effort to develop new numerical techniques which can reduce their difficulties, such as the time consumed and the convergence rate and obtain reasonably high accuracy. These advantages can be achieved via using spectral methods [6,7,8,9,10,11].

Spectral algorithms are a category of schemes used in mathematics and physics to handle numerically specific types of differential equations. The main idea behind these algorithms is to expand the exact unknown solution of the differential equation as a truncated series of basis functions—most probably orthogonal functions—for example: the Fourier basis, Chebyshev polynomials, Jacobi polynomials or others, and then to choose the expansion coefficients in the sum to satisfy both the differential equation as well as its initial and/or boundary conditions [12,13].

Gegenbauer (ultraspherical) $C_n^{\left(\lambda \right)}\left(x\right)$ polynomials have generated significant attention due to their rudimentary properties as well as their heavy use in mathematics and physics. The presence of the parameter $\lambda$ enriches their implementation in spectral and pseudo-spectral methods to solve many types of differential problems. Gegenbauer polynomials provide a convenient basis functions for polynomial approximations of many models involving differential, integral and integro-differential equations [14,15,16,17,18].

The fractional calculus theory is a study of the differential/integral operators of arbitrary order, as the fractional calculus was initially considered a purely mathematical idea with no applications; nevertheless, in recent decades, a noteworthy evolution has been achieved from both theoretical and applied points of views. Most of the fractional differential problems are difficult—sometimes impossible—to solve analytically; for this reason, there is a brawny need to handle them numerically [19,20,21].

The omnipresence of fractional derivative/integral operators inspired by the value of the parameters included in the definition of the operator, studying the effect of these parameters on the nature of the resulted function is one of the trends in the fractional calculation. The generalized Caputo type fractional derivative [22] has the same properties as the Caputo derivative. It also dispenses a useful method for superintending and constructing fractional measured mathematical models.

In this research, we developed the operational matrix of the new generalized Caputo FF derivative for the orthonormal normalized ultraspherical polynomials, we built an efficient Tau algorithm to handle the nonlinear FF Riccati equation, and we used the linearization formula of the product of two ultraspherical polynomials to deal with the quadratic term, resulting in a system of nonlinear algebraic equations. We then used Newton’s iterative method to solve this system and finally obtain the needed approximate solution.

The manuscript is organized as follows. In Section 2, we report the essential definitions of the generalized as well as the new generalized Caputo derivatives and the relevant properties of the orthonormal normalized ultraspherical polynomials; in Section 3, we build the operational matrices of the FF derivative of the ultraspherical vector; in Section 4, we construct a spectral Tau algorithm for the FF Riccati equation; in Section 5, we report the truncation error estimate of the approximate solution; in Section 6, we exhibit some numerical test problems; and some concluding remarks are reported in Section 7.

2. Preliminaries

In this section, we report the essential definitions of the generalized as well as the new generalized Caputo derivatives and some relevant properties of the orthonormal normalized ultraspherical polynomials, which will subsequently be of important use.

2.1. New Generalized Caputo FF Derivative

Definition 1

([23]). Let ρ be a positive constant, the generalized fractional integral of a continuous function $\xi \left(t\right)$ of order $\alpha >0$ is defined by

$${\displaystyle I^{\alpha ,\rho }\xi \left(t\right)=\frac{{\rho }^{1-\alpha }}{\mathsf{\Gamma }\left(\alpha \right)}{\int }_0^t{s}^{\rho -1}{(t^{\rho }-s^{\rho })}^{\alpha -1}\xi \left(s\right)ds.}$$

Definition 2

([23]). Let $\rho >0, n-1<\alpha <n$, and $n=⌈\alpha ⌉$, the generalized Caputo fractional derivative of order α of a function $\xi \left(t\right)\in C^n[0,1]$ is defined as

$${\displaystyle D^{\alpha ,\rho }\xi \left(t\right)=\frac{{\rho }^{\alpha -n-1}}{\mathsf{\Gamma }(n-\alpha )}{\int }_0^t{s}^{\rho -1}{(t^{\rho }-s^{\rho })}^{n-\alpha -1}{\left(s^{1-\rho }\frac{d}{ds}\right)}^n\xi \left(s\right)ds.}$$

The generalized Caputo fractional derivative satisfies:

$$D^{\alpha ,\rho }\mathrm{const}.=0,$$

if $\alpha \in (n-1,n),k>n-1$, then

$$D^{\alpha ,\rho }{t}^{k\rho }=\left\{\begin{array}{c}\begin{array}{cc}{\rho }^k\frac{\mathsf{\Gamma }(k+1)}{\mathsf{\Gamma }(k+1-\alpha )}{t}^{\rho (k-\alpha )},& k\in {\mathbb{N}}_0,k\ge ⌈\alpha ⌉,\\ 0,& k\in {\mathbb{N}}_0,k<⌈\alpha ⌉.\end{array}\hfill \end{array}\right.$$

Definition 3

([19]). Let $\xi \left(t\right)$ be continuous and fractal differentiable of order β on $(0,1)$, and as the new generalized Caputo FF derivative of order $\alpha , \beta$ is defined as

$${\displaystyle D^{\alpha ,\beta ,\rho }\xi \left(t\right)=\frac{{\rho }^{\alpha -n-1}}{\mathsf{\Gamma }(n-\alpha )}{\int }_0^t{s}^{\rho -1}{(t^{\rho }-s^{\rho })}^{n-\alpha -1}{\left(s^{1-\rho }\frac{d}{d{s}^{\beta }}\right)}^n\xi \left(s\right)ds,}$$

where, $\rho >0, n-1<\alpha , \beta \le n=⌈\alpha ⌉$ and ${\displaystyle \frac{d\xi }{d{t}^{\beta }}=\underset{z\to t}{lim}\frac{\xi \left(z\right)-\xi \left(t\right)}{z^{\beta }-t^{\beta }}}$.

It should be noted here that, in Definition 3, if we set $\rho =\beta =1$, we directly obtain the usual Caputo derivative.

2.2. Orthonormal Normalized Shifted Ultraspherical Polynomials

The analytic form of the orthonormal normalized shifted ultraspherical polynomials [24], $U_k^{\left(\sigma \right)}\left(t\right), t\in [0,1]$, of degree k, is given by

$${\displaystyle U_k^{\left(\sigma \right)}\left(t\right)=\sum _{r=0}^k\frac{{(-1)}^{k-r}k!\mathsf{\Gamma }(\sigma +\frac{1}{2})\mathsf{\Gamma }(k+r+2\sigma )\sqrt{h_k}}{r!(k-r)!\mathsf{\Gamma }(r+\sigma +\frac{1}{2})\mathsf{\Gamma }(k+2\sigma )}{t}^r,}$$

(1)

where:

$$h_k=\frac{2(k+\sigma )\mathsf{\Gamma }(k+2\sigma )}{k!{\left(\mathsf{\Gamma }(\sigma +\frac{1}{2})\right)}^2},$$

the following special values are important:

$$U_k^{\left(\sigma \right)}\left(0\right)={(-1)}^k\sqrt{h_k},U_k^{\left(\sigma \right)}\left(1\right)=\sqrt{h_k},$$

(2)

$U_k^{\left(\sigma \right)}\left(t\right)$ satisfy the following orthonormality relation:

$${\displaystyle {\int }_0^{1}w{U}_i^{\left(\sigma \right)}\left(t\right)U_j^{\left(\sigma \right)}\left(t\right)dt={\delta }_{ij},}$$

where $w={(t-t^2)}^{\sigma -\frac{1}{2}}$, and ${\delta }_{ij}$ is the well-known Kronecker delta symbol.

The following linearization formula [25] is needed:

$${\displaystyle U_m^{\left(\sigma \right)}\left(t\right)U_n^{\left(\sigma \right)}\left(t\right)=V_{m,n}^{\left(\sigma \right)}\left(t\right)=\sum _{s=0}^{min(m,n)}{L}_{m,n,s}^{\left(\sigma \right)}{U}_{m+n-2s}^{\left(\sigma \right)}\left(t\right),}$$

(3)

where:

$$L_{m,n,s}^{\left(\sigma \right)}=\frac{2^{2\sigma -1}m!n!\sqrt{h_m{h}_n}\mathsf{\Gamma }\left(\sigma +\frac{1}{2}\right)\mathsf{\Gamma }(s+\sigma )\mathsf{\Gamma }(m-s+\sigma )\mathsf{\Gamma }(n-s+\sigma )\mathsf{\Gamma }(m+n-s+2\sigma )(m+n-2s+\sigma )}{\sqrt{\pi h_{m+n-2s}}s!\mathsf{\Gamma }\left(\sigma \right)(m-s)!(n-s)!\mathsf{\Gamma }(m+2\sigma )\mathsf{\Gamma }(n+2\sigma )\mathsf{\Gamma }(m+n-s+\sigma +1)}.$$

2.3. Function Approximation

If $\xi \left(t\right)\in L^2(0,1)$, then $\xi \left(t\right)$ can be expanded as a series of $U_i^{\left(\sigma \right)}\left(t\right)$ as

$${\displaystyle \xi \left(t\right)=\sum _{i=0}^{\infty }{u}_i{U}_i^{\left(\sigma \right)}\left(t\right),}$$

where:

$${\displaystyle u_i={\int }_0^{1}w{U}_i^{\left(\sigma \right)}\left(t\right)\xi \left(t\right)dt,i=0,1\cdots .}$$

If we truncate the above series and only keep the first $(n+1)$-terms, we have:

$${\displaystyle \xi \left(t\right)\approx {\xi }_n\left(t\right)=\sum _{i=0}^n{u}_i{U}_i^{\left(\sigma \right)}\left(t\right)={\mathbf{C}}^T\mathbf{U}\left(t\right),}$$

(4)

where, ${\mathbf{C}}^T$ and $\mathbf{U}\left(t\right)$ are given by

$${\mathbf{C}}^T=[u_0,u_1,\cdots u_n],\mathbf{U}\left(t\right)={[U_0^{\left(\sigma \right)}\left(t\right),U_1^{\left(\sigma \right)}\left(t\right),\cdots ,U_n^{\left(\sigma \right)}\left(t\right)]}^T.$$

3. Different Derivatives of the Orthonormal Normalized Ultraspherical Vector

The first derivative of $\mathbf{U}\left(t\right)$ can be expressed as

$$\frac{d\mathbf{U}\left(t\right)}{dt}={\mathcal{D}}_1\mathbf{U}\left(t\right),$$

where ${\mathcal{D}}_1$ is the operational matrix of the first-order derivative [26], with entries:

$${\mathcal{D}}_1={\left(d_{ij}\right)}_{0\le i,j\le n};d_{ij}=\left\{\begin{array}{c}\begin{array}{cc}\frac{4i!(j+\lambda )\mathsf{\Gamma }(j+2\lambda )}{j!\mathsf{\Gamma }(i+2\lambda )}\sqrt{\frac{h_i}{h_j}},& i>j,(i+j)\mathrm{odd},\\ 0,& \mathrm{otherwise}.\end{array}\hfill \end{array}\right.$$

We also have:

$$\frac{d^m\mathbf{U}\left(t\right)}{d{t}^m}={\mathcal{D}}_m\mathbf{U}\left(t\right);{\mathcal{D}}_m={\left({\mathcal{D}}_1\right)}^m.$$

Lemma 1.

For $\alpha ,\beta \in (0,1),\rho >0$ and $k\in {\mathbb{N}}_0$, we have:

$$D^{\alpha ,\beta ,\rho }{t}^k=\frac{{\rho }^{\alpha }\mathsf{\Gamma }\left(\frac{k}{\rho }+1\right)}{\beta \mathsf{\Gamma }\left(\frac{k}{\rho }-\alpha +1\right)}{t}^{\frac{k}{\rho }-\alpha +1}.$$

Theorem 1.

The new generalized Caputo FF derivative of $\mathit{U}\left(t\right)$ is given by

$$D^{\alpha ,\beta ,\rho }\mathit{U}\left(t\right)\approx {\mathit{F}}_{\alpha ,\beta ,\rho }\mathit{U}\left(t\right),$$

where:

$${\mathit{F}}_{\alpha ,\beta ,\rho }=\left(\begin{array}{ccccc}0& 0& 0& \cdots & 0\\ ⋮& ⋮& ⋮& & ⋮\\ 0& 0& 0& \cdots & 0\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Omega }}_{⌈\alpha ⌉,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Omega }}_{⌈\alpha ⌉,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Omega }}_{⌈\alpha ⌉,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Omega }}_{⌈\alpha ⌉,n,k}}\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Omega }}_{i,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Omega }}_{i,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Omega }}_{i,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Omega }}_{i,n,k}}\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Omega }}_{n,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Omega }}_{n,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Omega }}_{n,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Omega }}_{n,n,k}}\end{array}\right),$$

where ${\mathsf{\Omega }}_{i,j,k}$ is given by

$${\displaystyle {\mathsf{\Omega }}_{i,j,k}=\sqrt{h_j}\sum _{l=0}^j\frac{{(-1)}^{j-l}j!\mathsf{\Gamma }{\left(\sigma +\frac{1}{2}\right)}^2\mathsf{\Gamma }(j+l+2\sigma )\mathsf{\Gamma }\left(\frac{k}{\rho }+l-\beta -\alpha +\sigma +\frac{3}{2}\right)}{l!(j-l)!\mathsf{\Gamma }(j+2\sigma )\mathsf{\Gamma }\left(l+\sigma +\frac{1}{2}\right)\mathsf{\Gamma }\left(\frac{k}{\rho }+l-\beta -\alpha +2\sigma +2\right)}.}$$

Proof. 

From (1), we have:

$${\displaystyle D^{\alpha ,\beta ,\rho }{U}_k^{\left(\sigma \right)}\left(t\right)=\sum _{r=0}^k\frac{{(-1)}^{k-r}k!\mathsf{\Gamma }(\sigma +\frac{1}{2})\mathsf{\Gamma }(k+r+2\sigma )\sqrt{h_k}}{r!(k-r)!\mathsf{\Gamma }(r+\sigma +\frac{1}{2})\mathsf{\Gamma }(k+2\sigma )}\frac{{\rho }^{\alpha }\mathsf{\Gamma }\left(\frac{r}{\rho }+1\right)}{\beta \mathsf{\Gamma }\left(\frac{r}{\rho }-\alpha +1\right)}{t}^{\frac{r}{\rho }-\alpha -\beta +1}.}$$

Now, we can approximate $t^{\frac{r}{\rho }-\alpha -\beta +1}$ as

$${\displaystyle t^{\frac{r}{\rho }-\alpha -\beta +1}\approx \sum _{j=0}^n{T}_{r,j}{U}_j^{\left(\sigma \right)}\left(t\right),}$$

and:

$${\displaystyle T_{r,j}={\int }_0^1{t}^{\frac{r}{\rho }-\alpha -\beta +1}{U}_j^{\left(\sigma \right)}wdt,}$$

again with the aid of (1), we have:

$${\displaystyle D^{\alpha ,\beta ,\rho }{U}_k^{\left(\sigma \right)}\left(t\right)=\sum _{j=0}^n\sum _{r=⌈\alpha ⌉}^k{\mathsf{\Omega }}_{k,j,r}{U}_j^{\left(\sigma \right)}\left(t\right);k=⌈\alpha ⌉,⌈\alpha ⌉+1,\dots ,n,}$$

which completes the proof of the theorem. □

As a direct special case of Theorem 1, we have the following theorem.

Theorem 2.

The generalized Caputo FF derivative of $\mathit{U}\left(t\right)$ is given by

$$D^{\alpha ,\rho }\mathit{U}\left(t\right)\approx {\mathit{F}}_{\alpha ,\rho }\mathit{U}\left(t\right),$$

where:

$${\mathit{F}}_{\alpha ,\rho }=\left(\begin{array}{ccccc}0& 0& 0& \cdots & 0\\ ⋮& ⋮& ⋮& & ⋮\\ 0& 0& 0& \cdots & 0\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Theta }}_{⌈\alpha ⌉,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Theta }}_{⌈\alpha ⌉,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Theta }}_{⌈\alpha ⌉,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^{⌈\alpha ⌉}{\mathsf{\Theta }}_{⌈\alpha ⌉,n,k}}\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Theta }}_{i,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Theta }}_{i,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Theta }}_{i,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^i{\mathsf{\Theta }}_{i,n,k}}\\ ⋮& ⋮& ⋮& & ⋮\\ {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Theta }}_{n,0,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Theta }}_{n,1,k}}& {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Theta }}_{n,2,k}}& \cdots & {\displaystyle \sum _{k=⌈\alpha ⌉}^n{\mathsf{\Theta }}_{n,n,k}}\end{array}\right),$$

where ${\mathsf{\Theta }}_{i,j,k}$ is given by

$${\displaystyle {\mathsf{\Theta }}_{i,j,k}=\sqrt{h_j}\sum _{l=0}^j\frac{{(-1)}^{j-l}j!\mathsf{\Gamma }{\left(\sigma +\frac{1}{2}\right)}^2\mathsf{\Gamma }(j+l+2\sigma )\mathsf{\Gamma }\left(\frac{k}{\rho }+l-\alpha +\sigma +\frac{1}{2}\right)}{l!(j-l)!\mathsf{\Gamma }(j+2\sigma )\mathsf{\Gamma }\left(l+\sigma +\frac{1}{2}\right)\mathsf{\Gamma }\left(\frac{k}{\rho }+l-\alpha +1\sigma +2\right)}.}$$

4. Tau Algorithm for FF Riccati Equation

Usually, the Tau method was used for linear problems, whereas in this section, we adopt it to handle the following nonlinear Riccati equation:

$$D^{\alpha ,\beta ,\rho }\psi \left(t\right)=\chi \left(t\right)+\mu \psi \left(t\right)+\lambda {\psi }^2\left(t\right);t\in (0,1),$$

(5)

subject to the initial condition:

$$\psi \left(0\right)={\psi }_0,$$

(6)

where $\mu ,\lambda ,{\psi }_0$ are known constants, and $\chi \left(t\right)$ is a known continuous source term.

We start with the following approximation:

$${\displaystyle \psi \left(t\right)\approx {\psi }_n\left(t\right)=\sum _{i=0}^n{u}_i{U}_i^{\left(\sigma \right)}\left(t\right)={\mathbf{C}}^T\mathbf{U}\left(t\right),}$$

by Theorem 1, we have:

$$D^{\alpha ,\beta ,\rho }\psi \left(t\right)\approx {\mathbf{C}}^T{\mathbf{F}}_{\alpha ,\beta ,\rho }\mathbf{U}\left(t\right),$$

and we have:

$${\psi }^2\left(t\right)\approx {\mathbf{C}}^T\mathbf{U}\left(t\right){\mathbf{U}}^T\left(t\right)\mathbf{C}={\mathbf{C}}^T\mathbf{V}\left(t\right)\mathbf{C},$$

where:

$$\mathbf{V}\left(t\right)=\left(\begin{array}{ccccc}{\left(U_0^{\left(\sigma \right)}\right)}^2& U_0^{\left(\sigma \right)}{U}_1^{\left(\sigma \right)}& U_0^{\left(\sigma \right)}{U}_2^{\left(\sigma \right)}& \cdots & U_0^{\left(\sigma \right)}{U}_n^{\left(\sigma \right)}\\ U_1^{\left(\sigma \right)}{U}_0^{\left(\sigma \right)}& {\left(U_1^{\left(\sigma \right)}\right)}^2& U_1^{\left(\sigma \right)}{U}_2^{\left(\sigma \right)}& \cdots & U_1^{\left(\sigma \right)}{U}_n^{\left(\sigma \right)}\\ ⋮& ⋮& ⋮& & ⋮\\ U_n^{\left(\sigma \right)}{U}_0^{\left(\sigma \right)}& U_n^{\left(\sigma \right)}{U}_1^{\left(\sigma \right)}& U_n^{\left(\sigma \right)}{U}_2^{\left(\sigma \right)}& \cdots & {\left(U_n^{\left(\sigma \right)}\right)}^2\end{array}\right),$$

thanks to the linearization formula (3), $\mathbf{V}\left(t\right)$ can be simplified as

$$\mathbf{V}\left(t\right)=\left(\begin{array}{ccccc}{V}_{0,0}^{\left(\sigma \right)}& V_{0,1}^{\left(\sigma \right)}& V_{0,2}^{\left(\sigma \right)}& \cdots & V_{0,n}^{\left(\sigma \right)}\\ V_{1,0}^{\left(\sigma \right)}& V_{1,1}^{\left(\sigma \right)}& V_{0,2}^{\left(\sigma \right)}& \cdots & V_{1,n}^{\left(\sigma \right)}\\ ⋮& ⋮& ⋮& & ⋮\\ V_{n,0}^{\left(\sigma \right)}& V_{n,1}^{\left(\sigma \right)}& V_{n,2}^{\left(\sigma \right)}& \cdots & V_{n,n}^{\left(\sigma \right)}\end{array}\right),$$

where $V_{i,j}^{\left(\sigma \right)}$ is defined in (3), and the source term $\chi \left(t\right)$ can be approximated as

$${\displaystyle \chi \left(t\right)\approx \sum _{i=0}^n{\chi }_i{U}_i^{\left(\sigma \right)}\left(t\right)={\chi }^T\mathbf{U}\left(t\right),}$$

where ${\chi }^T$ is given by

$${\chi }^T=[{\chi }_0,{\chi }_1,\cdots {\chi }_n],$$

and:

$${\displaystyle {\chi }_i={\int }_0^{1}w{U}_i^{\left(\sigma \right)}\left(t\right)\chi \left(t\right)dt,i=0,1\cdots n,}$$

now the residual of Equation (5) is given by

$$R\left(t\right)=D^{\alpha ,\beta ,\rho }{\psi }_n\left(t\right)-\lambda {\psi }_n^2\left(t\right)-\mu {\psi }_n\left(t\right)-\chi \left(t\right),$$

in other words:

$$R\left(t\right)={\mathbf{C}}^T{\mathbf{F}}_{\alpha ,\beta ,\rho }\mathbf{U}\left(t\right)-\lambda {\mathbf{C}}^T\mathbf{V}\left(t\right)\mathbf{C}-\mu {\mathbf{C}}^T\mathbf{U}\left(t\right)-{\chi }^T\mathbf{U}\left(t\right),$$

the application of the Tau method:

$${\displaystyle {\int }_0^{1}R\left(t\right)U_j^{\left(\sigma \right)}\left(t\right)wdt=0;j=0,1,\dots ,n-1,}$$

(7)

the use of the initial condition (6), and thanks to (2), we have

$${\mathbf{C}}^T\mathbf{U}\left(0\right)={\psi }_0.$$

(8)

Finally, Equations (7) and (8) generate a system of $(n+1)$ algebraic equations in the $(n+1)$ unknown expansion coefficients, with quadratic nonlinearity, which can be perfectly solved by Newton’s iterative procedure, with the vanishing initial guess of the form $u_i=e^{-i}$, and consequently, we obtain the desired approximate solution.

5. Truncation Error Estimate

In this section, we report an upper estimate for the truncation error of the suggested approximate solution. Based on the detailed analysis in [16], we have the following theorem.

Theorem 3.

If $\xi \left(t\right)\in C^p(0,1)$, for some $p>2$, then the expansion coefficients in (4) satisfy the following estimate

$$|u_i|⪅i^{1-p-\sigma },$$

where ⪅ means that there exists a generic constant d such that $|u_i|\le d/i^{p+\sigma -1}$.

Based on the result of Theorem 3, we the following truncation error estimate.

Theorem 4.

If $\xi \left(t\right)$ satisfies the hypothesis of Theorem 3 is approximated by ${\xi }_n\left(t\right)$, and then the following truncation error estimate is valid:

$$|\xi \left(t\right)-{\xi }_n\left(t\right)|⪅n^{2-p}.$$

Proof. 

We start with:

$${\displaystyle \xi \left(t\right)=\sum _{i=0}^{\infty }{u}_i{U}_i^{\left(\sigma \right)}\left(t\right),{\xi }_n\left(t\right)=\sum _{i=0}^n{u}_i{U}_i^{\left(\sigma \right)}\left(t\right),}$$

hence:

$${\displaystyle |\xi \left(t\right)-{\xi }_n\left(t\right)|\le \sum _{i=n+1}^{\infty }\left|u_i{U}_i^{\left(\sigma \right)}\left(t\right)\right|,}$$

now, by the application of Theorem 3 and noting that $|U_i^{\left(\sigma \right)}\left(t\right)|\le \sqrt{h_i}$, we have

$$|\xi \left(t\right)-{\xi }_n\left(t\right)|⪅\sum _{i=n+1}^{\infty }{i}^{1-p}=O\left(n^{2-p}\right),$$

which completes the proof of the theorem. □

6. Test Problems

In this section, we handle three Riccati problems with the new generalized FF derivative.

Example 1.

Consider the following FF Riccati initial value problem (see [19,22,27,28,29]):

$$D^{\alpha ,\beta ,\rho }\varphi \left(t\right)=1-{\varphi }^2\left(t\right);t\in (0,1),$$

subject to the homogeneous initial condition:

$$\varphi \left(0\right)=0.$$

The exact smooth solution for the classical model (when $\alpha =\beta =\rho =1$) is $\varphi \left(t\right)=tanht$.In

Table 1. Comparison between our method [19,22], methods for $\varphi \left(t\right)$ of Example 1, with $n=15, \alpha =0.9, \beta =1$ and $\rho =1.01$.

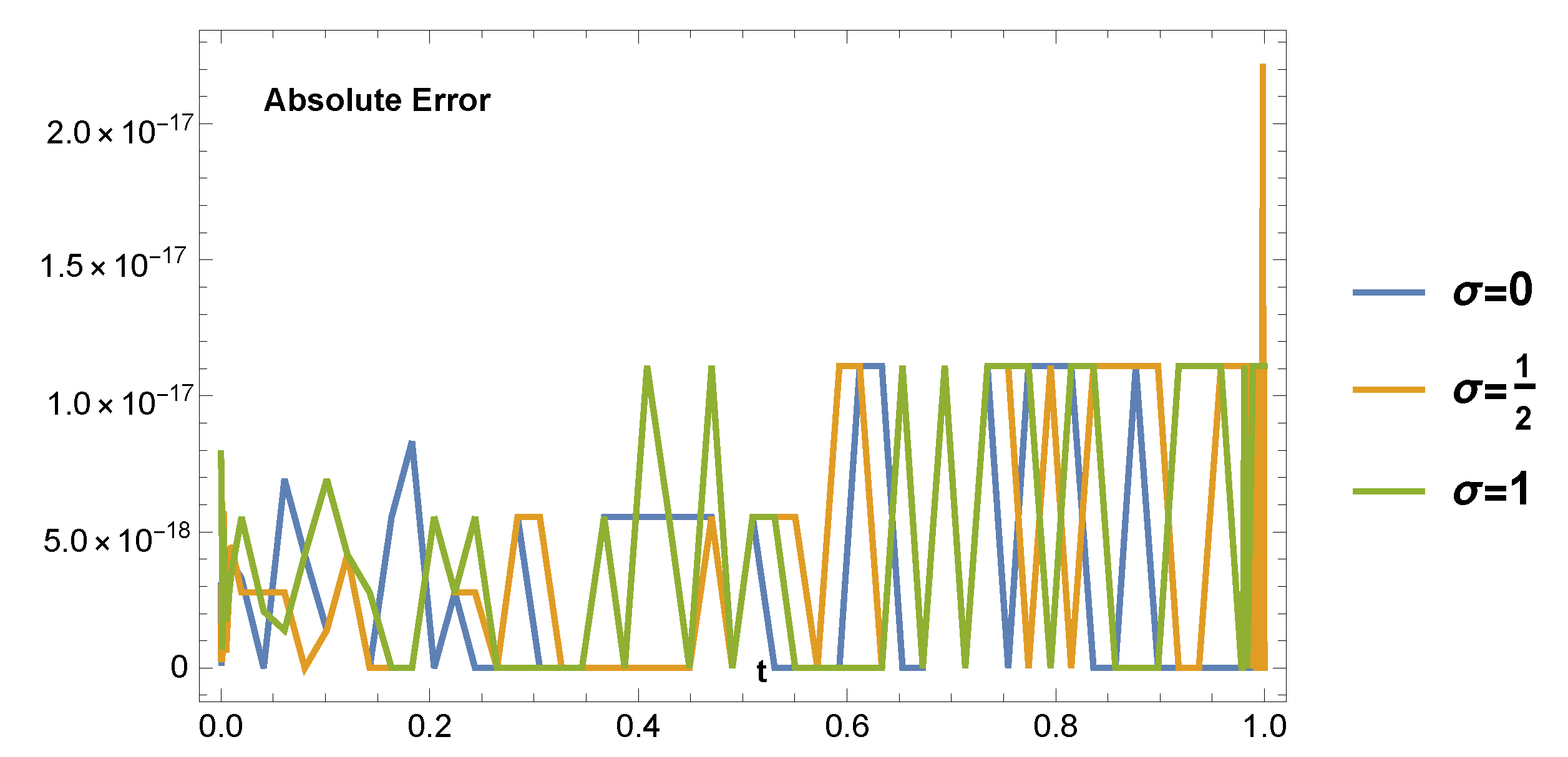
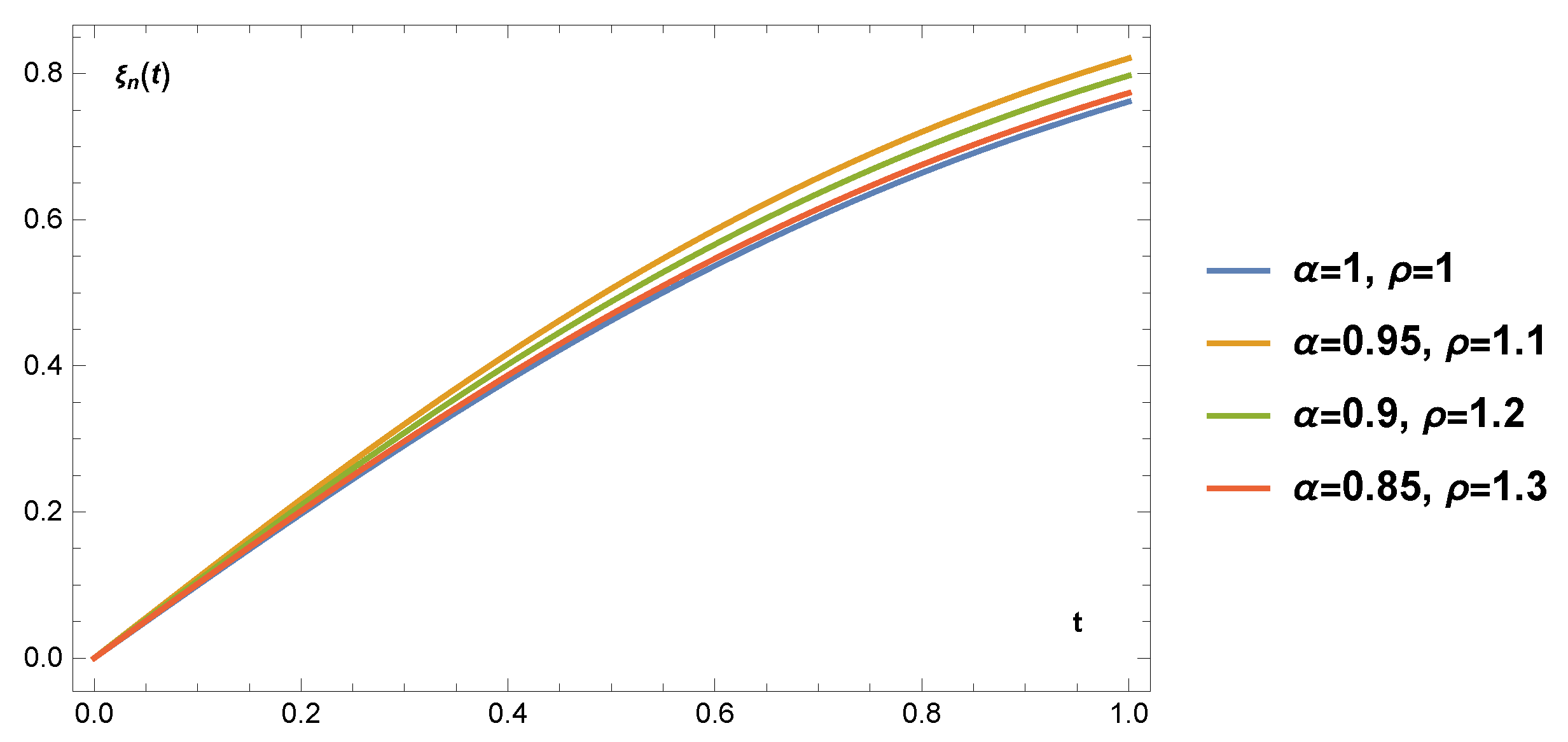
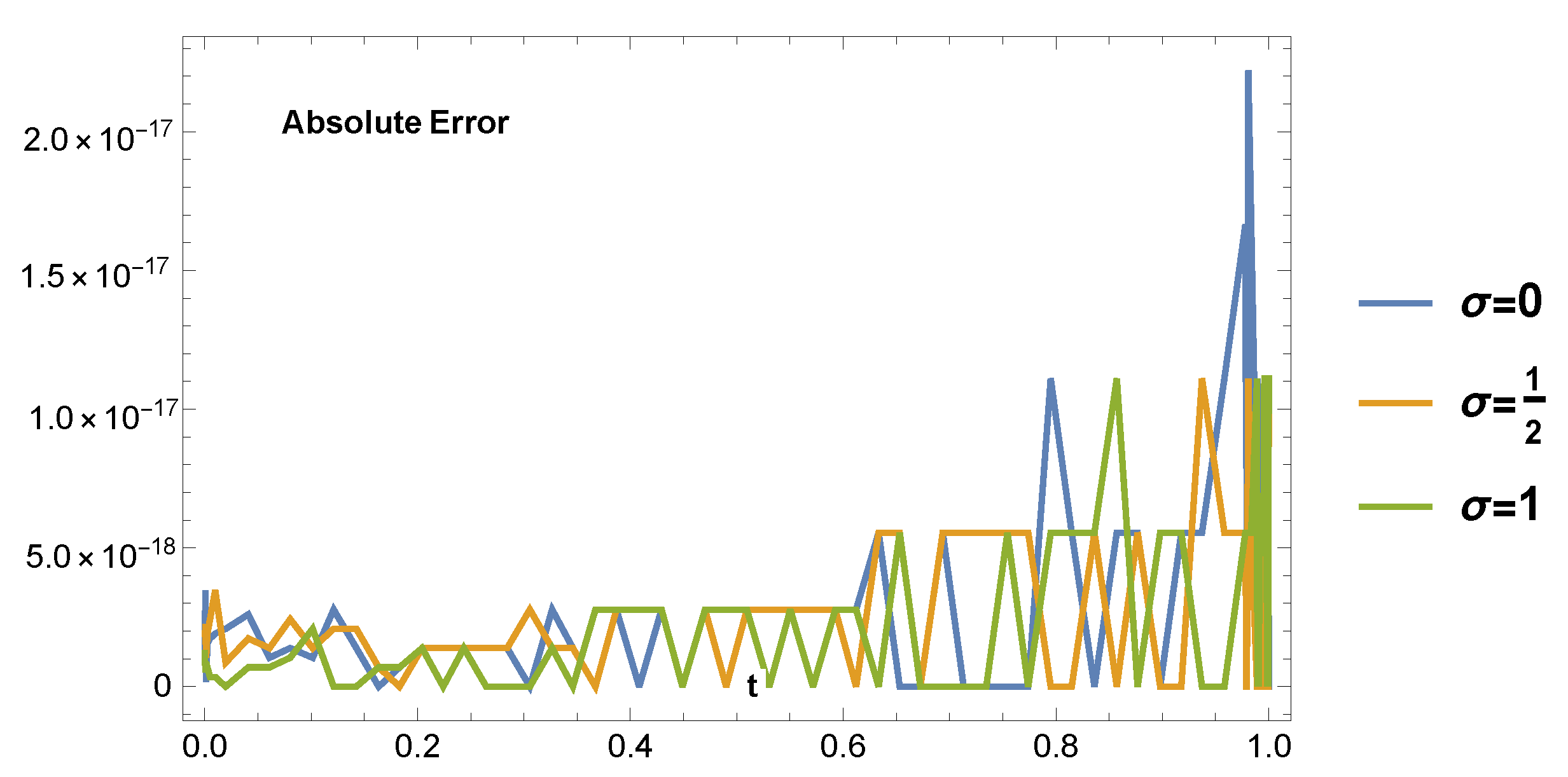
t	$\mathit{\sigma }=0$	$\mathit{\sigma }=\frac{1}{2}$	$\mathit{\sigma }=1$	[19]	[22]	Exact
0.0	0.0	0.0	0.0	0.0	0.0	0.0
0.2	0.2342826045	0.2342826056	0.2342826071	0.2342826056	0.260941	0.1973753203
0.4	0.4181001627	0.4181001633	0.4181001623	0.4181001633	0.442638	0.3799489622
0.6	0.5505899501	0.5505899500	0.5505899500	0.5505899500	0.577781	0.5370495670
0.8	0.6624900000	0.6624900000	0.6624900000	0.6624900000	0.676930	0.6640367702
CPU Time	$3.412$	$1.247$	$3.267$	-	-	-

and

Table 2. Comparison between our method [19,27,28,29], methods for $\varphi \left(t\right)$ of Example 1, with $n=15, \alpha =\beta =\rho =1$.

t	$\mathit{\sigma }=0$	$\mathit{\sigma }=\frac{1}{2}$	Exact	[19]	[28]	[29]	[27]
0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0
0.2	0.1973753203	0.1973753203	0.1973753203	0.1973753203	0.197375	0.1973753092	0.1973753160
0.4	0.3799489622	0.3799489622	0.3799489622	0.3799489624	0.379944	0.3799435784	0.3799469862
0.6	0.5370495670	0.5370495670	0.5370495670	0.5370495668	0.536857	0.5368572343	0.5369833784
0.8	0.6640367702	0.6640367702	0.6640367702	0.6640367701	0.661706	0.6617060368	0.6633009217
1.0	0.7615941558	0.7615941557	0.7615941557	0.7615941550	0.746032	0.746031746	0.7571662667

, we compare our results for $\alpha =0.9, 1, 0.75, \beta =1$ and $\rho =1.01, 1$ with:

• Legendre operational matrix method [19];
• Variation of parameters method [27];
• Modified homotopy perturbation method [28];
• Trigonometric basic functions [22];
• Fractional generalized homotopy analysis method [29].

In Figure 1, we depict the best absolute errors for Example 1, with $\alpha =\beta =\rho =1$ and $n=18$.

Based on the CPU time reported in seconds in Table 1, it is worth mentioning here that we obtained the numerical approximate solutions in a few seconds, which emphasizes the effectiveness of the method.

In Figure 2, we study the effect of changing $\rho$ and $\alpha$ on the approximate solution for a fixed value of $\beta =1$.

Example 2.

Consider the following FF Riccati initial value problem (see [22,28,30,31]):

$$D^{\alpha ,\beta ,\rho }\varphi \left(t\right)=1+2\varphi \left(t\right)-{\varphi }^2\left(t\right);t\in (0,1),$$

subject to the homogeneous initial condition:

$$\varphi \left(0\right)=0.$$

The exact smooth solution for the classical model is:

$$\varphi \left(t\right)=1+\sqrt{2}tanh\left(\sqrt{2}t-{coth}^{-1}\sqrt{2}\right).$$

In

Table 3. Comparison of absolute errors for some methods for Example 2, with $\alpha =\beta =\rho =1$.

t	$\mathit{\sigma }=0$	$\mathit{\sigma }=\frac{1}{2}$	$\mathit{\sigma }=1$	[30]	[31]	[28]	[22]
0.0	0.0	0.0	0.0	0.0	0.0	0.0	0.0
0.2	2.36 × ${10}^{-13}$	2.10 × ${10}^{-13}$	1.24 × ${10}^{-13}$	1.03 × ${10}^{-6}$	2.90 × ${10}^{-4}$	1.20 × ${10}^{-5}$	9.23 × ${10}^{-5}$
0.4	2.24 × ${10}^{-13}$	2.22 × ${10}^{-13}$	2.21 × ${10}^{-13}$	3.33 × ${10}^{-5}$	2.50 × ${10}^{-4}$	3.03 × ${10}^{-4}$	7.35 × ${10}^{-5}$
0.6	4.37 × ${10}^{-13}$	5.37 × ${10}^{-13}$	3.27 × ${10}^{-13}$	9.98 × ${10}^{-5}$	5.50 × ${10}^{-4}$	4.69 × ${10}^{-3}$	7.56 × ${10}^{-5}$
0.8	5.63 × ${10}^{-13}$	4.69 × ${10}^{-13}$	5.91 × ${10}^{-13}$	1.54 × ${10}^{-5}$	3.80 × ${10}^{-3}$	1.88 × ${10}^{-2}$	3.94 × ${10}^{-5}$
1.0	2.35 × ${10}^{-12}$	3.62 × ${10}^{-12}$	6.28 × ${10}^{-12}$	3.47 × ${10}^{-3}$	3.40 × ${10}^{-3}$	3.43 × ${10}^{-2}$	7.12 × ${10}^{-5}$

, we compare our results for $\alpha =\beta =\rho =1$, we take $n=10$ with:

• Modified homotopy perturbation method [28];
• Trigonometric basic functions [22];
• Variational iteration method [30];
• Optimal homotopy asympytotic method [31].

In

Table 4. MAR for Example 2, with $\alpha =0.9, \beta =0.7, \rho =1.5$.

n	$\mathit{\sigma }=0$	$\mathit{\sigma }=\frac{1}{2}$	$\mathit{\sigma }=1$
6	3.28 × ${10}^{-8}$	6.97 × ${10}^{-8}$	3.57 × ${10}^{-8}$
10	4.42 × ${10}^{-12}$	6.38 × ${10}^{-12}$	7.29 × ${10}^{-12}$
14	3.69 × ${10}^{-15}$	3.57 × ${10}^{-15}$	6.82 × ${10}^{-15}$
18	2.22 × ${10}^{-17}$	2.22 × ${10}^{-17}$	2.22 × ${10}^{-17}$

, we list the maximum absolute residual error MAR for this problem for the case $\alpha =0.9, \beta =0.7, \rho =1.5$, where $MAR$ is defined by

$$MAR=\left|D^{\alpha ,\beta ,\rho }{\varphi }_n\left(t\right)-1-2{\varphi }_n\left(t\right)+{\varphi }_n^2\left(t\right)\right|.$$

In Figure 3, we depict the absolute error graph of Example 2, for $\alpha =\beta =\rho =1$ and $n=18$.

Example 3.

Consider the FF simple Riccati equation:

$$D^{\frac{1}{2},\frac{1}{2},\frac{3}{2}}\varphi \left(t\right)=\frac{16}{9}\sqrt{\frac{2}{3\pi }}{t}^{3/4}-t^2+{\varphi }^2\left(t\right);t\in (0,1),$$

subject to the homogeneous initial condition:

$$\varphi \left(0\right)=0.$$

The exact smooth solution for this model is $\varphi \left(t\right)=t$.

Application of the proposed method for $\sigma =n=1$ will yield the following system of equations:

$$-0.100849-1.59577u_0^2+1.89373u_1-1.59577u_1^2=0,\mathrm{and}1.59577u_0-3.19154u_1=0,$$

thanks to Newton’s method, we obtain:

$$u_0=0.31332853432887514,u_1=0.15666426716443757,$$

and consequently:

$${\varphi }_1\left(t\right)=2\sqrt{\frac{2}{\pi }}\left(0.31332853432887514\right)+4\sqrt{\frac{2}{\pi }}(2t-1)\left(0.15666426716443757\right)=t,$$

which is the exact solution.

7. Conclusions

In this research, the spectral Tau method was adopted for numerically solving the nonlinear Riccati initial value problem with a new generalized Caputo FF derivative. The FF operational matrix of the orthonormal normalized ultraspherical polynomials was constructed and then used to discretize the problem to a system of algebraic equations with quadratic nonlinearity. We then applied Newton’s method with the vanishing initial guess to obtain the desired approximate solution. The Tau method is characterized by its efficiency, accuracy, and fine applicability. The validity of the presented method was verified through three numerical simulations.