Control and Robust Stabilization at Unstable Equilibrium by Fractional Controller for Magnetic Levitation Systems
Abstract
:1. Introduction
- To design and investigate the roles of the FOFPID controller in a Maglev system;
- To use the GWO–PSO algorithm in designing process of the FOFPID controller considering its optimization for the first time in the literature and due to short computation time of the algorithm;
- To illustrate the advantage of GWO–PSO-based FOFPID over FOPID and FOSMC tuned by the GWO–PSO algorithm for the Maglev system;
- To validate the superiority of the presented fractional order controllers compared to the integer order counterparts proposed in the literature like PID and SMC for the above stated system;
- To scrutinize the results based on dynamic transient responses of the fractional order controllers tuned according to the IAE, ISE, ITAE, and ITSE;
- To carry out sensitivity analysis for assessing the robustness of the designed fractional order controllers in the presence of parameter uncertainty, external disturbance, and different trajectory tracking.
2. Mathematical Model of the Maglev System
3. Controllers’ Design
3.1. Conventional and Fractional Order PID Controller
3.2. Integer Order and Fractional Order Sliding Mode Control
3.3. Conventional and Fractional Order Fuzzy-PID Control
3.4. Design of Fractional Order Operator
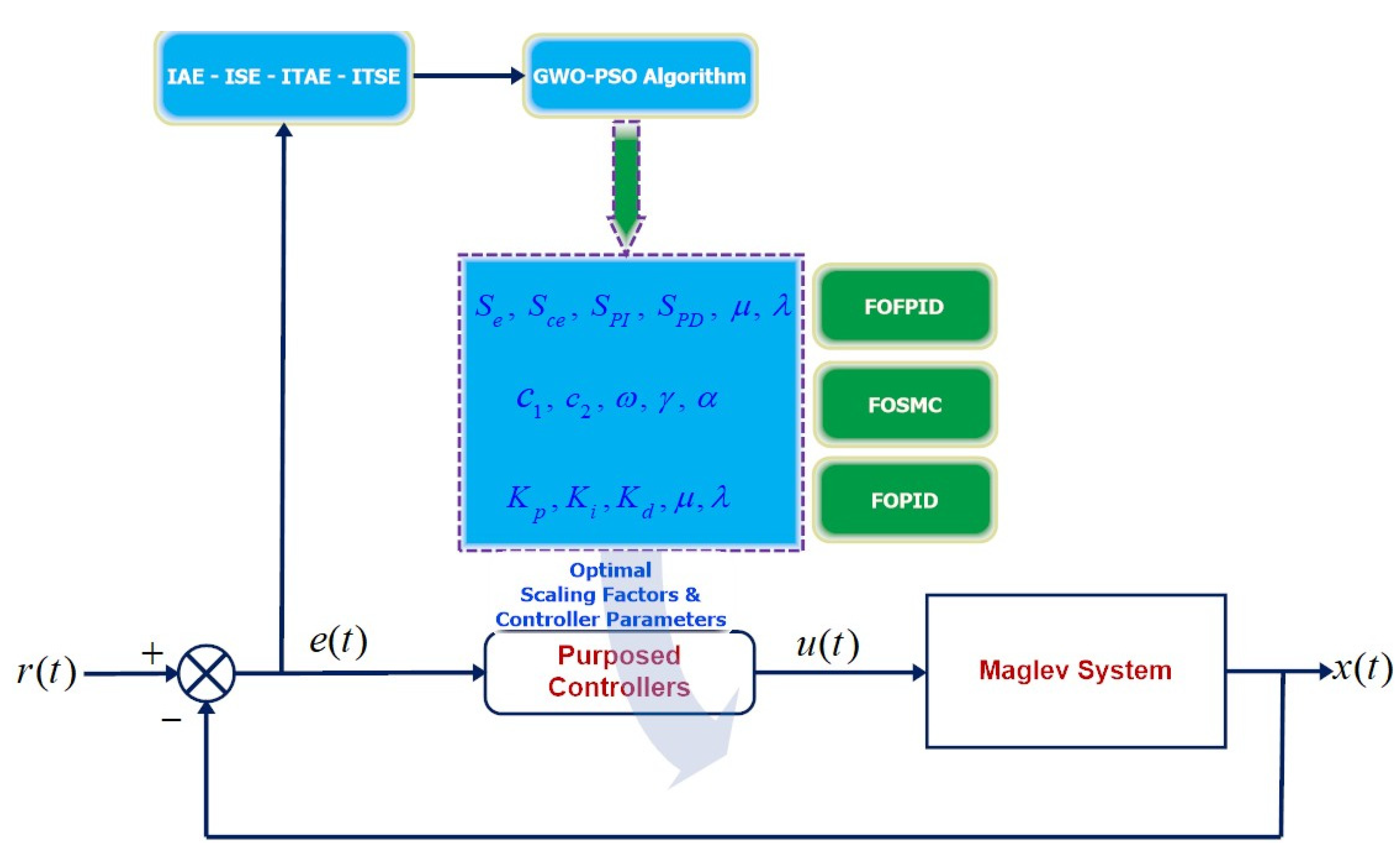
4. Controller Parameters Optimization
4.1. Optimization Algorithm
4.1.1. PSO Algorithm
4.1.2. GWO Algorithm
4.1.3. GWO–PSO Algorithm
- Step 1. Initialization of the positions of wolves in the population and that of particles in the swarm.
- Step 2. Updating of each wolf location by using the GWO algorithm.
- Step 3. Determination of the three best ones among all search agents.
- Step 4. Running PSO by using the best values, found by GWO, as initial positions of the swarm.
- Step 5. Returning the positions modified by PSO back to the GWO algorithm.
- Step 6. Repeating these steps until the maximum iteration number is reached.
- for the FOPID controller
- for the FOSMC controller
- for the FOFPID controller
4.2. Objective Functions
4.3. Proposed Optimization Framework
5. Simulation Results and Discussion
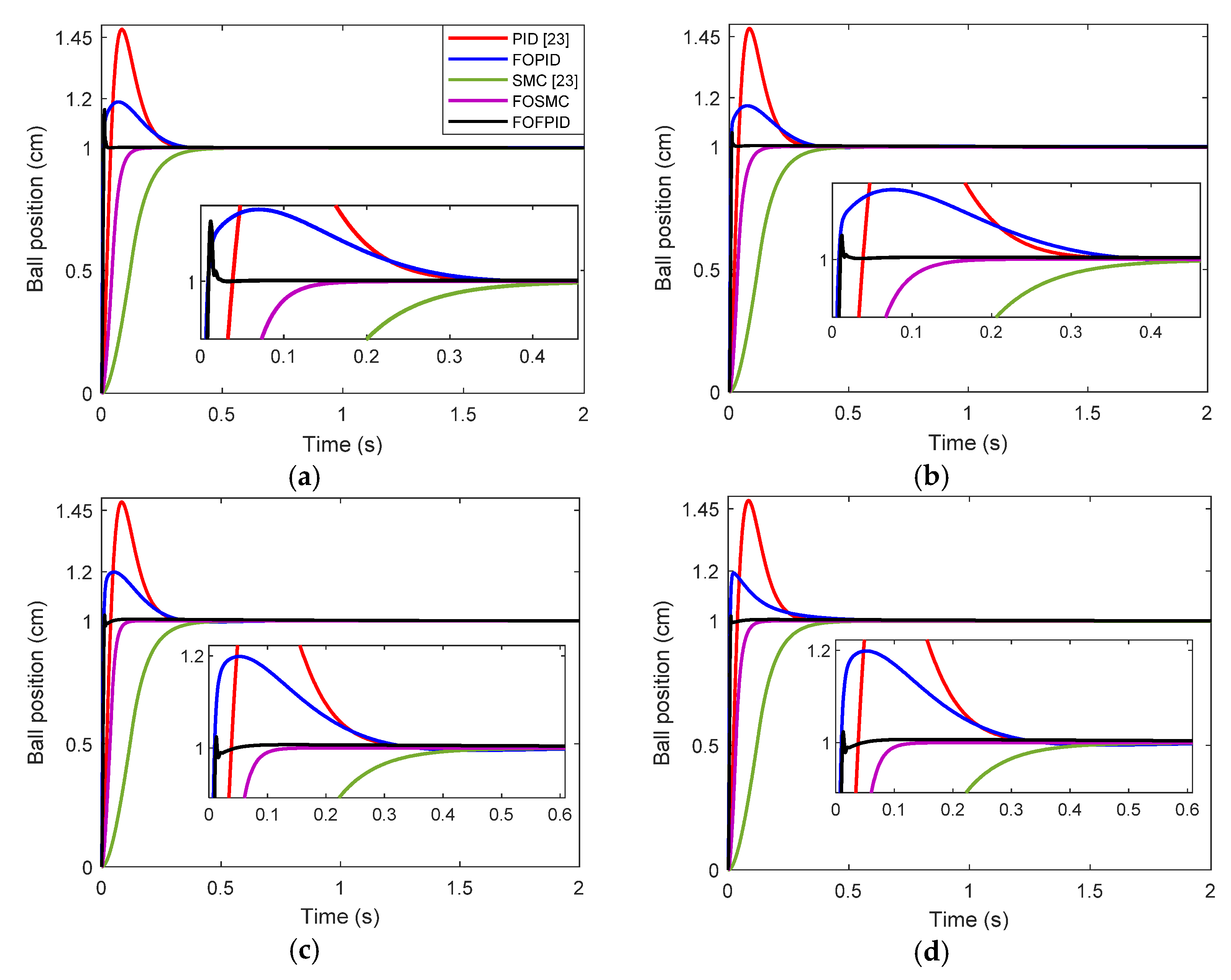
5.1. Dynamic Performance Analysis
5.2. Controller Performance Analysis under Parametric Variations
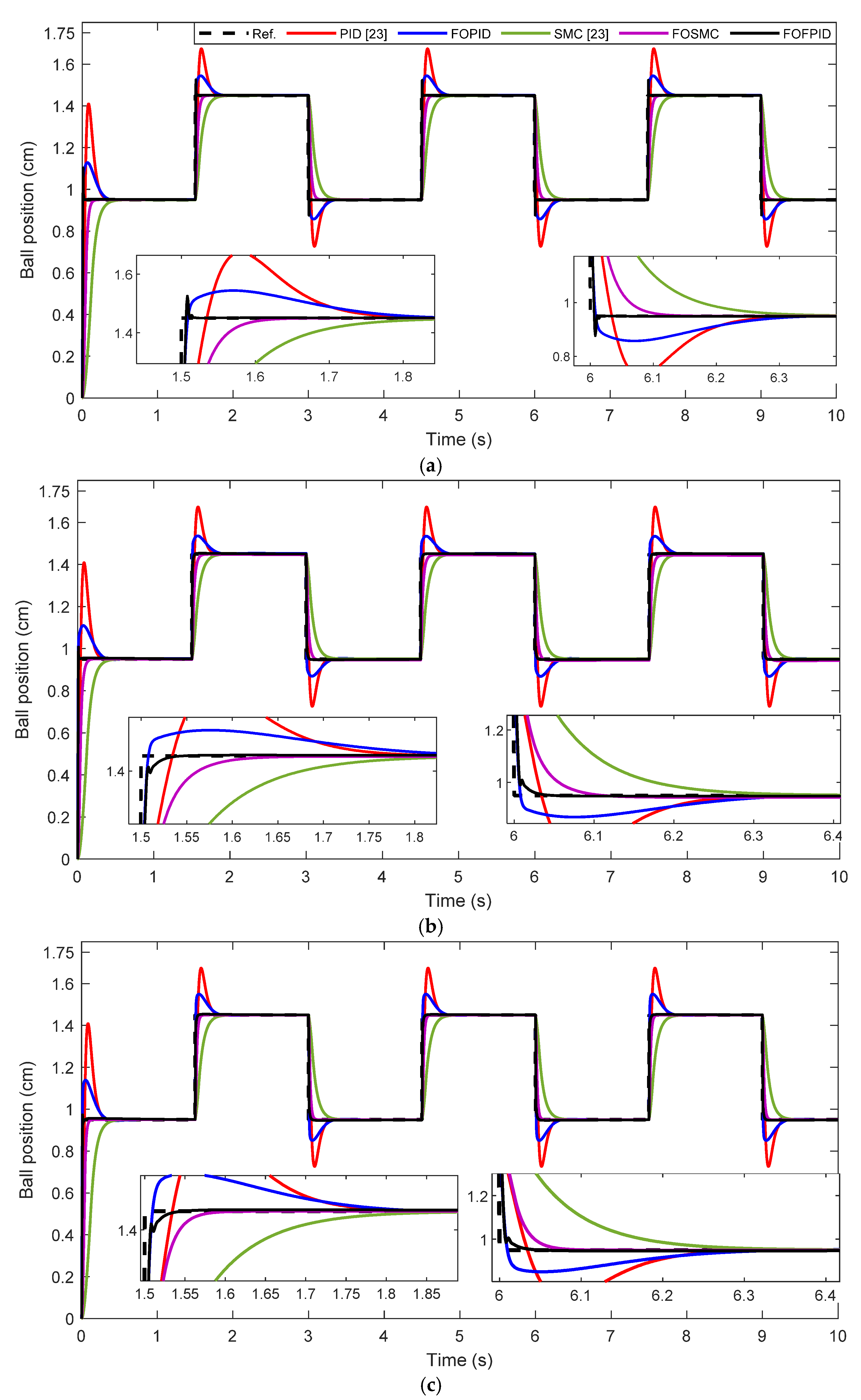
5.3. Controller Performance Analysis under Different Trajectory Tracking
5.4. Controller Performance Analysis under Disturbance
- The proposed fractional order PID and SMC approaches outperform the integer order PID and SMC developed in [23] in terms of overshoot, rise time, settling time, and all objective function values in the presence of internal and external disturbances;
- While consuming more control energy of the closed-loop control system with the FOFPID controller, as compared to the others except for the FOPID, the beauty of the proposed FOFPID controller is able to efficiently reduce the adverse effects of the parameter variation, different trajectory tracking, and external disturbance.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Schweitzer, G.; Maslen, E. Magnetic Bearings: Theory, Design and Application to Rotating Machinery; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- He, Y.; Wu, J.; Xie, G.; Hong, X.; Zhang, Y. Data-driven relative position detection technology for high-speed maglev train. Measurement 2021, 180, 109468. [Google Scholar] [CrossRef]
- Chaban, A.; Lukasik, Z.; Lis, M.; Szafraniec, A. Mathematical Modeling of Transient Processes in Magnetic Suspension of Maglev Trains. Energies 2020, 13, 6642. [Google Scholar] [CrossRef]
- Tsuda, M.; Tamashiro, K.; Sasaki, S.; Yagai, T.; Hamajima, T.; Yamada, T. Vibration transmission characteristics against vibration in magnetic levitation type HTS seismic/vibration isolation device. IEEE Trans. Appl. Supercond. 2009, 19, 2249–2252. [Google Scholar] [CrossRef]
- Rohacs, D.; Rohacs, J. Magnetic levitation assisted aircraft take-off and landing (feasibility study—GABRIEL concept). Prog. Aerosp. Sci. 2016, 85, 33–50. [Google Scholar] [CrossRef]
- Xie, J.; Zhao, P.; Zhang, C.; Hao, Y.; Xia, N.; Fu, J. A feasible, portable and convenient density measurement method for minerals via magnetic levitation. Measurement 2019, 136, 564–572. [Google Scholar] [CrossRef]
- Lockett, M.; Mirica, K.A.; Mace, C.R.; Blackledge, R.D.; Whitesides, G.M. Analyzing Forensic Evidence Based on Density with Magnetic Levitation. J. Forensic Sci. 2012, 58, 40–45. [Google Scholar] [CrossRef]
- Tang, D.; Zhao, P.; Shen, Y.; Zhou, H.; Xie, J.; Fu, J. Detecting shrinkage voids in plastic gears using magnetic levitation. Polym. Test. 2020, 91, 106820. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, W.; Chen, W. Simulation analysis and experimental study of the diamagnetically levitated electrostatic micromotor. J. Magn. Magn. Mater. 2019, 492, 165634. [Google Scholar] [CrossRef]
- Ashkarran, A.A.; Mahmoudi, M. Magnetic Levitation Systems for Disease Diagnostics. Trends Biotechnol. 2021, 39, 311–321. [Google Scholar] [CrossRef]
- Yaseen, M.H. A comparative study of stabilizing control of a planer electromagnetic levitation using PID and LQR controllers. Results Phys. 2017, 7, 4379–4387. [Google Scholar] [CrossRef]
- Zhu, H.; Pang, C.K.; Teo, T.J. Analysis and control of a 6 DOF maglev positioning system with characteristics of end-effects and eddy current damping. Mechatronics 2017, 47, 183–194. [Google Scholar] [CrossRef]
- Ghosh, A.; Krishnan, R.; Tejaswy, P.; Mandal, A.; Pradhan, J.K.; Ranasingh, S. Design and implementation of a 2-DOF PID compensation for magnetic levitation systems. ISA Trans. 2014, 53, 1216–1222. [Google Scholar] [CrossRef] [PubMed]
- Acharya, D.S.; Swain, S.K.; Mishra, S.K. Real-Time Implementation of a Stable 2 DOF PID Controller for Unstable Second-Order Magnetic Levitation System with Time Delay. Arab. J. Sci. Eng. 2020, 45, 6311–6329. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional-Order Systems and Fractional-Order Controllers; Institute of Experimental Physics, Slovak Academy of Sciences: Kosice, Watsonova, The Slovak Republic, 1994. [Google Scholar]
- Demirören, A.; Ekinci, S.; Hekimoğlu, B.; Izci, D. Opposition-based artificial electric field algorithm and its application to FOPID controller design for unstable magnetic ball suspension system. Eng. Sci. Technol. Int. J. 2021, 24, 469–479. [Google Scholar] [CrossRef]
- Mughees, A.; Mohsin, S.A. Design and Control of Magnetic Levitation System by Optimizing Fractional Order PID Controller Using Ant Colony Optimization Algorithm. IEEE Access 2020, 8, 116704–116723. [Google Scholar] [CrossRef]
- Bauer, W.; Baranowski, J. Fractional PI^λ D Controller Design for a Magnetic Levitation System. Electronics 2020, 9, 2135. [Google Scholar] [CrossRef]
- Swain, S.; Sain, D.; Mishra, S.K.; Ghosh, S. Real time implementation of fractional order PID controllers for a magnetic levitation plant. AEU Int. J. Electron. Commun. 2017, 78, 141–156. [Google Scholar] [CrossRef]
- Acharya, D.S.; Mishra, S. A multi-agent based symbiotic organisms search algorithm for tuning fractional order PID controller. Measurement 2020, 155, 107559. [Google Scholar] [CrossRef]
- Pandey, S.; Dwivedi, P.; Junghare, A.S. A novel 2-DOF fractional-order PIλ-Dμ controller with inherent anti-windup capability for a magnetic levitation system. AEU Int. J. Electron. Commun. 2017, 79, 158–171. [Google Scholar] [CrossRef]
- Karahan, O.; Ataşlar-Ayyıldız, B. Optimized PID Based Controllers for Improving Transient and Steady State Response of Maglev System. In Advances in Engineering Research; Nova Science Publishers: New York, NY, USA, 2020; pp. 149–185. [Google Scholar]
- Starbino, A.V.; Sathiyavathi, S. Design of sliding mode controller for magnetic levitation system. Comput. Electr. Eng. 2019, 78, 184–203. [Google Scholar]
- Shieh, H.-J.; Siao, J.-H.; Liu, Y.-C. A robust optimal sliding-mode control approach for magnetic levitation systems. Asian J. Control. 2010, 12, 480–487. [Google Scholar] [CrossRef]
- Lin, F.-J.; Teng, L.-T.; Shieh, P.-H. Intelligent Sliding-Mode Control Using RBFN for Magnetic Levitation System. IEEE Trans. Ind. Electron. 2007, 54, 1752–1762. [Google Scholar] [CrossRef]
- Chen, S.; Kuo, C. ARNISMC for MLS with global positioning tracking control. IET Electr. Power Appl. 2018, 12, 518–526. [Google Scholar] [CrossRef]
- Boonsatit, N.; Pukdeboon, C. Adaptive Fast Terminal Sliding Mode Control of Magnetic Levitation System. J. Control. Autom. Electr. Syst. 2016, 27, 359–367. [Google Scholar] [CrossRef]
- Roy, P.; Roy, B.K. Sliding Mode Control Versus Fractional-Order Sliding Mode Control: Applied to a Magnetic Levitation System. J. Control. Autom. Electr. Syst. 2020, 31, 597–606. [Google Scholar] [CrossRef]
- Pandey, S.; Dourla, V.; Dwivedi, P.; Junghare, A. Introduction and realization of four fractional-order sliding mode controllers for nonlinear open-loop unstable system: A magnetic levitation study case. Nonlinear Dyn. 2019, 98, 601–621. [Google Scholar] [CrossRef]
- Wang, J.; Shao, C.; Chen, Y.-Q. Fractional order sliding mode control via disturbance observer for a class of fractional order systems with mismatched disturbance. Mechatronics 2018, 53, 8–19. [Google Scholar] [CrossRef]
- Zhang, C.-L.; Wu, X.-Z.; Xu, J. Particle Swarm Sliding Mode-Fuzzy PID Control Based on Maglev System. IEEE Access 2021, 9, 96337–96344. [Google Scholar] [CrossRef]
- Lin, C.-M.; Lin, M.-H.; Chen, C.-W. SoPC-based adaptive PID control system design for magnetic levitation system. IEEE Syst. J. 2011, 5, 278–287. [Google Scholar] [CrossRef]
- Abdel-Hady, F.; Abuelenin, S. Design and simulation of a fuzzy-supervised PID controller for a magnetic levitation system. Stud. Inform. Control. 2008, 17, 315–328. [Google Scholar]
- Luat, T.H.; Cho, J.-H.; Kim, Y.-T. Fuzzy-Tuning PID Controller for Nonlinear Electromagnetic Levitation System. Adv. Intell. Syst. Comput. 2014, 272, 17–28. [Google Scholar] [CrossRef]
- Sahoo, A.K.; Mishra, S.K.; Majhi, B.; Panda, G.; Satapathy, S.C. Real-Time Identification of Fuzzy PID-Controlled Maglev System using TLBO-Based Functional Link Artificial Neural Network. Arab. J. Sci. Eng. 2021, 46, 4103–4118. [Google Scholar] [CrossRef]
- Burakov, M. Fuzzy PID Controller for Magnetic Levitation System. In Proceedings of the Second International Conference on Intelligent Transportation; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 2019; Volume 154, pp. 655–663. [Google Scholar]
- Ataşlar-Ayyıldız, B.; Karahan, O. Design of a MAGLEV System with PID Based Fuzzy Control Using CS Algorithm. Cybern. Inf. Technol. 2020, 20, 5–19. [Google Scholar] [CrossRef]
- Sain, D.; Mohan, B.M. Modelling of a Nonlinear Fuzzy Three-Input PID Controller and Its Simulation and Experimental Realization. IETE Tech. Rev. 2020, 1–20. [Google Scholar] [CrossRef]
- Sain, D.; Mohan, B. A simple approach to mathematical modelling of integer order and fractional order fuzzy PID controllers using one-dimensional input space and their experimental realization. J. Frankl. Inst. 2021, 358, 3726–3756. [Google Scholar] [CrossRef]
- Podlubny, I.; Dorcak, L.; Kostial, I. On fractional derivatives fractional-order dynamic systems and Pi/sup/spl lambda//D/sup/spl mu//-controllers. In Proceedings of the 36th IEEE Conference on Decision and Control, San Diego, CA, USA, 12 December 1997; Volume 5, pp. 4985–4990. [Google Scholar]
- Dastjerdi, A.A.; Saikumar, N.; HosseinNia, S.H. Tuning guidelines for fractional order PID controllers: Rules of thumb. Mechatronics 2018, 56, 26–36. [Google Scholar] [CrossRef]
- Behera, P.K.; Mendi, B.; Sarangi, S.K.; Pattnaik, M. Robust wind turbine emulator design using sliding mode controller. Renew. Energy Focus 2021, 36, 79–88. [Google Scholar] [CrossRef]
- Kumar, G.E.; Arunshankar, J. Control of nonlinear two-tank hybrid system using sliding mode controller with fractional-order PI-D sliding surface. Comput. Electr. Eng. 2018, 71, 953–965. [Google Scholar] [CrossRef]
- Woo, Z.-W.; Chung, H.-Y.; Lin, J.-J. A PID type fuzzy controller with self-tuning scaling factors. Fuzzy Sets Syst. 2000, 115, 321–326. [Google Scholar] [CrossRef]
- Yesil, E.; Güzelkaya, M.; Eksin, I. Self tuning fuzzy PID type load and frequency controller. Energy Convers. Manag. 2004, 45, 377–390. [Google Scholar] [CrossRef]
- MacVicar-Whelan, P. Fuzzy sets for man-machine interaction. Int. J. Man Mach. Stud. 1976, 8, 687–697. [Google Scholar] [CrossRef]
- Oustaloup, A.; Levron, F.; Mathieu, B.; Nanot, F.M. Frequency-band complex noninteger differentiator: Characterization and synthesis. IEEE Trans. Circuits Syst. I 2000, 47, 25–39. [Google Scholar] [CrossRef]
- Bauer, W.; Baranowski, J.; Tutaj, A.; Piatek, P.; Bertsias, P.; Kapoulea, S.; Psychalinos, C. Implementing Fractional PID Control for MagLev with SoftFRAC. In Proceedings of the 2020 43rd International Conference on Telecommunications and Signal Processing (TSP), Milan, Italy, 7–9 July 2020; pp. 435–438. [Google Scholar]
- Kennedy, J.; Eberhart, R. Particle Swarm Optimization. In Proceedings of the ICNN’95—International Conference on Neural Networks, Perth, WA, Australia, 27 November–1 December 1995; Volume 4, pp. 1942–1948. [Google Scholar] [CrossRef]
- Mirjalili, S.; Mirjalili, S.M.; Lewis, A. Grey Wolf Optimizer. Adv. Eng. Softw. 2014, 69, 46–61. [Google Scholar] [CrossRef] [Green Version]
- Shaheen, M.A.; Hasanien, H.M.; Alkuhayli, A. A novel hybrid GWO-PSO optimization technique for optimal reactive power dispatch problem solution. Ain Shams Eng. J. 2021, 12, 621–630. [Google Scholar] [CrossRef]
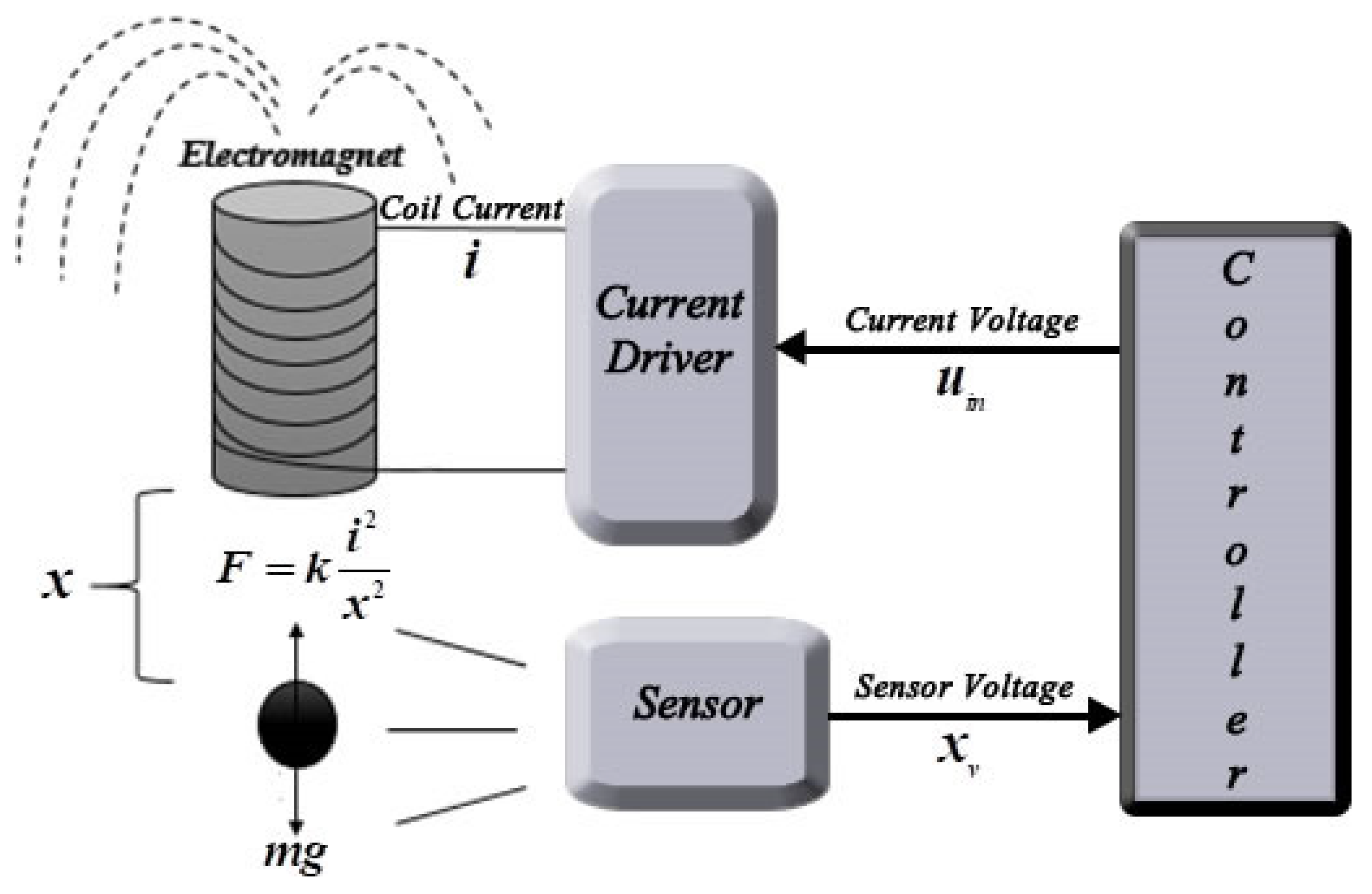
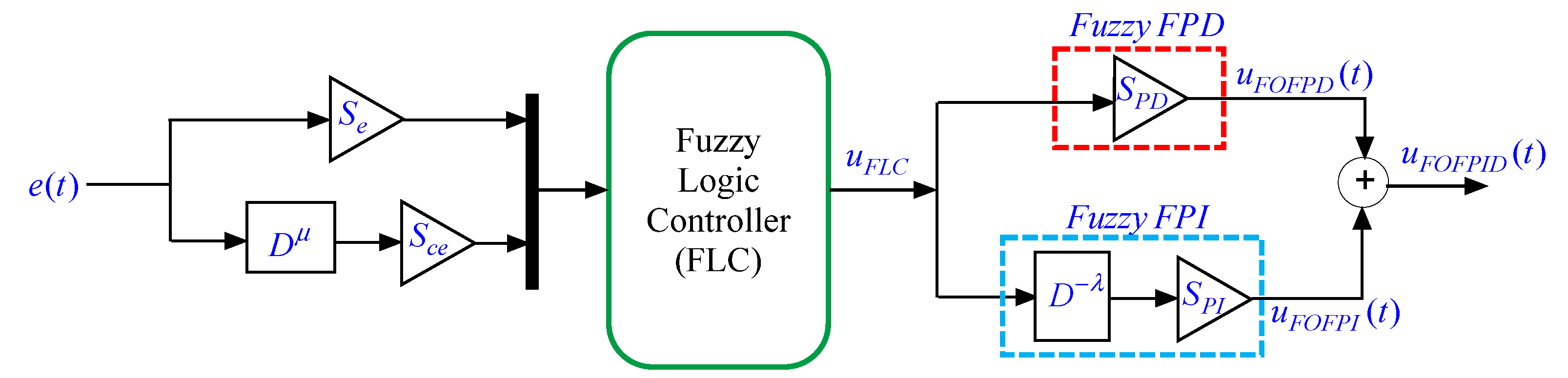
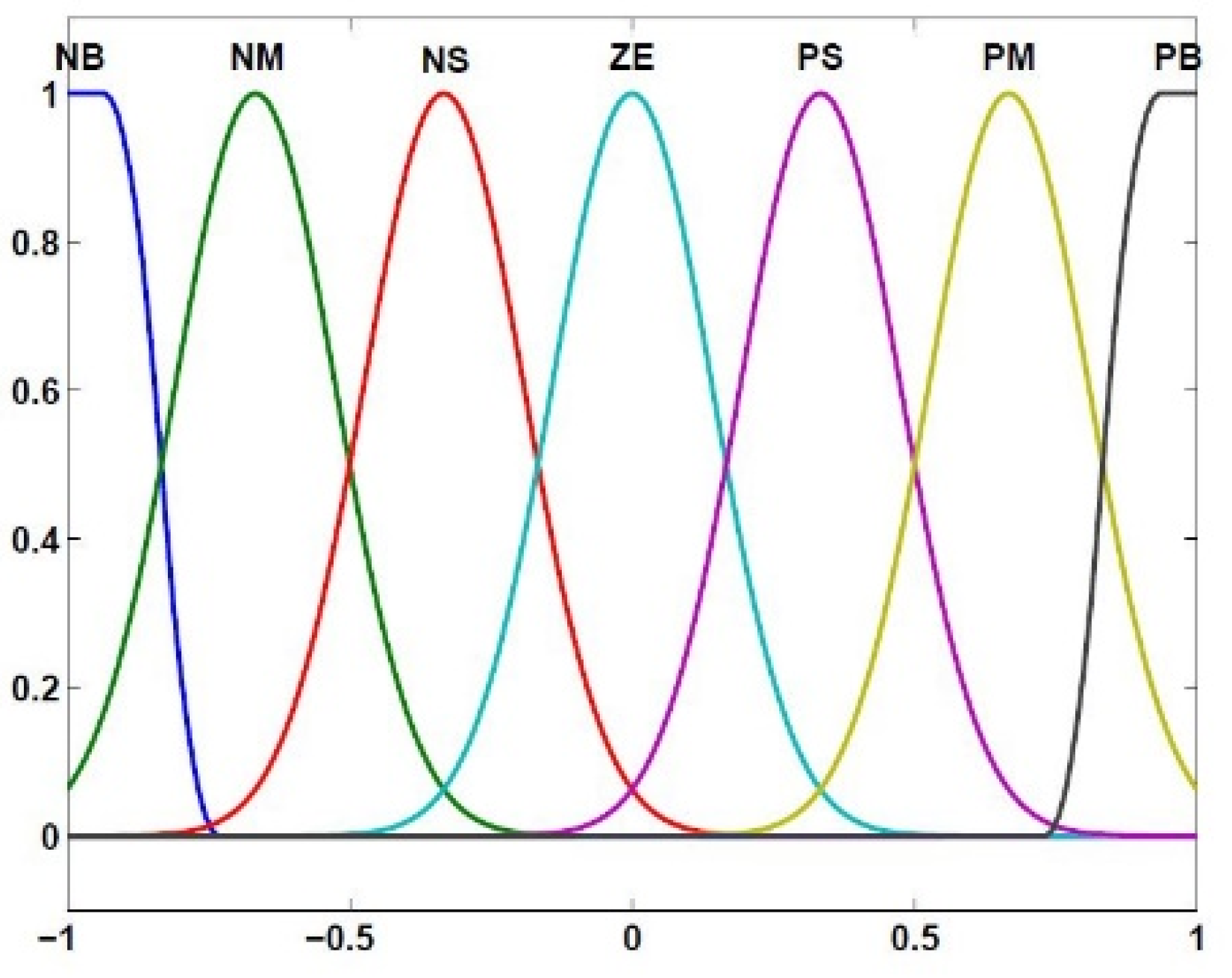
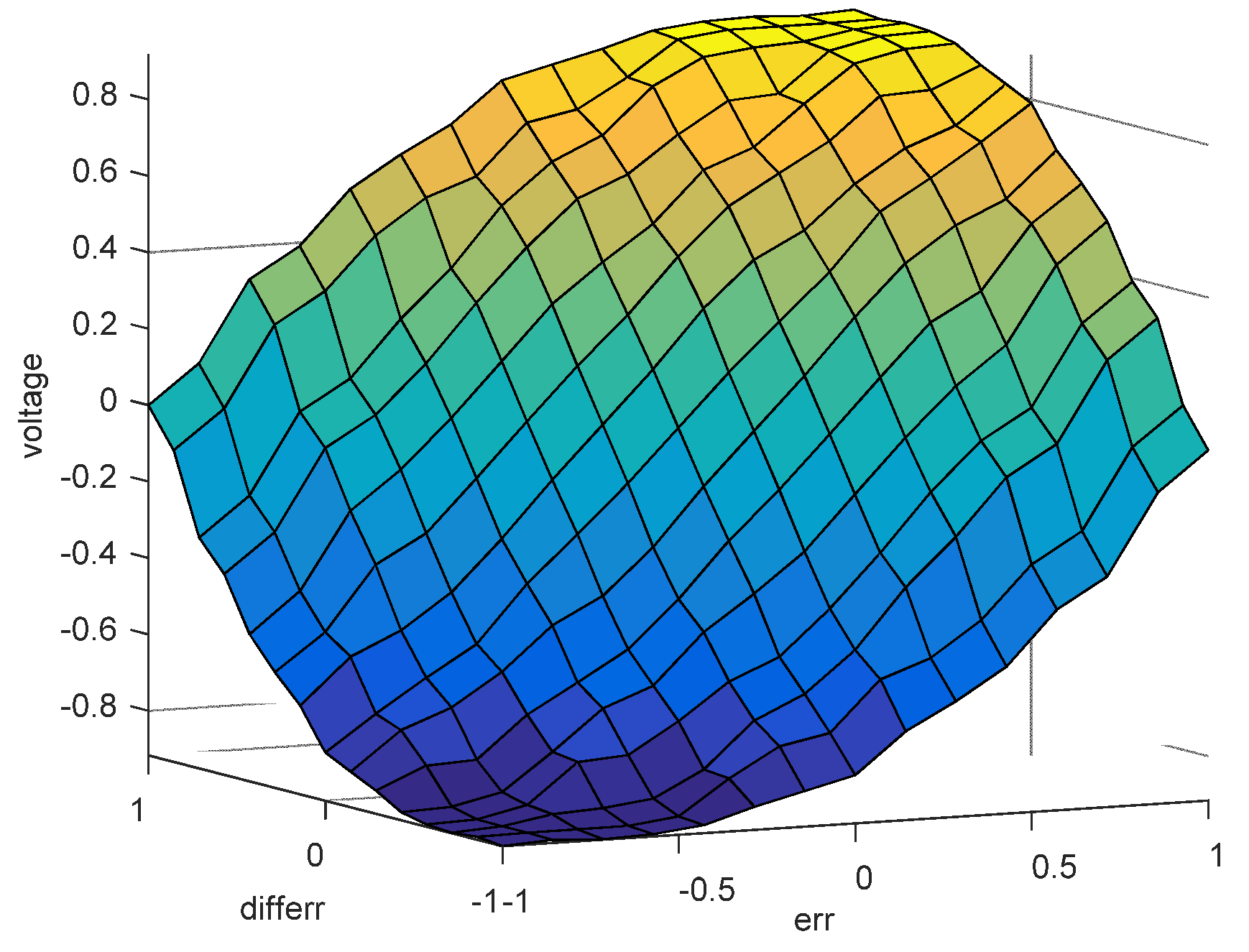







| Parameter | Notation | Value |
|---|---|---|
| Mass of the ball (gr.) | 22 | |
| Equilibrium value of current (A.) | 0.6105 | |
| Equilibrium value of position (mm.) | 20 | |
| Sensor gain | 458.7157 | |
| The gain of the amplifier of the driving circuit | 5.8929 |
| NB | NM | NS | ZE | PS | PM | PB | |
| NB | NB | NB | NB | NB | NM | NS | ZE |
| NM | NB | NB | NB | NM | NS | ZE | PS |
| NS | NB | NB | NM | NS | ZE | PS | PM |
| ZE | NB | NM | NS | ZE | PS | PM | PB |
| PS | NM | NS | ZE | PS | PM | PB | PB |
| PM | NS | ZE | PS | PM | PB | PB | PB |
| PB | ZE | PS | PM | PB | PB | PB | PB |
| Controller | Parameters | Objective Functions | |||
|---|---|---|---|---|---|
| FOPID | 1.9095 | 1.9936 | 2.0325 | 2.5475 | |
| 13.9014 | 13.8985 | 14.8587 | 16.7852 | ||
| 0.1072 | 0.1197 | 0.0992 | 0.1331 | ||
| 0.9715 | 0.9703 | 0.9524 | 0.8924 | ||
| 0.9561 | 0.9364 | 1.0030 | 0.9236 | ||
| FOSMC | 0.0169 | 0.0135 | 0.0102 | 0.0102 | |
| 0.7354 | 0.4753 | 0.6839 | 0.4995 | ||
| 15.7659 | 16.0584 | 15.9247 | 15.9825 | ||
| 0.0216 | 0.0192 | 0.0113 | 0.0137 | ||
| 0.9992 | 0.9460 | 0.9911 | 0.9779 | ||
| FOFPID | 8.5982 | 3.7212 | 3.1247 | 3.2948 | |
| 0.0577 | 0.0454 | 0.0468 | 0.0904 | ||
| 17.9569 | 17.2330 | 17.9871 | 16.6151 | ||
| 18.0998 | 18.1233 | 15.1292 | 18.7125 | ||
| 0.9080 | 0.9622 | 0.9257 | 0.9808 | ||
| 1.2908 | 1.3407 | 1.1802 | 1.3571 | ||
| Transient Response and Steady State Characteristics | ||||||||
|---|---|---|---|---|---|---|---|---|
| Objective (J) | Controller | J IAE ISE ITAE ITSE | ||||||
| FOPID | 18.7081 | 0.0050 | 0.2850 | 0.000518 | 0.0369 | |||
| FOSMC | 0.0004 | 0.0670 | 0.1210 | 0.000034 | 0.0477 | |||
| FOFPID | 15.5977 | 0.0060 | 0.0190 | 0.000020 | 0.0079 | |||
| FOPID | 16.7848 | 0.0050 | 0.3070 | 0.001823 | 0.0064 | |||
| FOSMC | 0.0696 | 0.0610 | 0.1190 | 0.002506 | 0.0274 | |||
| FOFPID | 5.7631 | 0.0060 | 0.0130 | 0.000119 | 0.0041 | |||
| FOPID | 19.8640 | 0.0060 | 0.2770 | 0.000004 | 0.0041 | |||
| FOSMC | 0 | 0.0500 | 0.0880 | 0.000124 | 0.0010 | |||
| FOFPID | 2.3659 | 0.0070 | 0.0160 | 0.000031 | 0.0032 | |||
| FOPID | 19.1968 | 0.0060 | 0.3210 | 0.000742 | 0.0002 | |||
| FOSMC | 0.0064 | 0.0520 | 0.0990 | 0.000515 | 0.0004 | |||
| FOFPID | 1.9090 | 0.0060 | 0.0100 | 0.000001 | 0.00001 | |||
| , | PID [23] | 48.2852 | 0.0270 | 0.2690 | 0.000001 | 0.0741 | 0.0334 | |
| SMC [23] | 0 | 0.1820 | 0.3360 | 0.000638 | 0.1314 | 0.0897 | ||
| Objective Function | Controller | J IAE ISE ITAE ITSE | |
|---|---|---|---|
| PID [23] | 4.5698 | 0.2678 | |
| FOPID | 25.5283 | 0.1456 | |
| SMC [23] | 2.2707 | 0.3830 | |
| FOSMC | 2.7272 | 0.1388 | |
| FOFPID | 15.9137 | 0.0247 | |
| PID [23] | 4.5698 | 0.0702 | |
| FOPID | 31.5188 | 0.0152 | |
| SMC [23] | 2.2707 | 0.1552 | |
| FOSMC | 2.5674 | 0.0497 | |
| FOFPID | 11.9206 | 0.0080 | |
| PID [23] | 4.5698 | 1.0595 | |
| FOPID | 18.0171 | 0.6007 | |
| SMC [23] | 2.2707 | 1.4038 | |
| FOSMC | 3.4337 | 0.3610 | |
| FOFPID | 9.5196 | 0.1706 | |
| PID [23] | 4.5698 | 0.2146 | |
| FOPID | 15.8200 | 0.0453 | |
| SMC [23] | 2.2707 | 0.4092 | |
| FOSMC | 2.8977 | 0.1151 | |
| FOFPID | 13.0436 | 0.0252 |
| Controller | ||||||||
|---|---|---|---|---|---|---|---|---|
| PID [23] | 0.1800 | 2.9785 | 0.0583 | 2.9785 | 0.4359 | 2.9785 | 0.0423 | 2.9785 |
| FOPID | 0.1029 | 19.4858 | 0.0111 | 23.8734 | 0.2891 | 13.9850 | 0.0076 | 12.3772 |
| SMC [23] | 0.5276 | 2.3861 | 0.5163 | 2.3861 | 1.5122 | 2.3861 | 1.5617 | 2.3861 |
| FOSMC | 0.0863 | 2.5273 | 0.0492 | 2.4813 | 0.0802 | 2.6897 | 0.0183 | 2.5716 |
| FOFPID | 0.0170 | 8.4440 | 0.0076 | 6.8566 | 0.0703 | 5.6572 | 0.0042 | 7.2778 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ataşlar-Ayyıldız, B.; Karahan, O.; Yılmaz, S. Control and Robust Stabilization at Unstable Equilibrium by Fractional Controller for Magnetic Levitation Systems. Fractal Fract. 2021, 5, 101. https://doi.org/10.3390/fractalfract5030101
Ataşlar-Ayyıldız B, Karahan O, Yılmaz S. Control and Robust Stabilization at Unstable Equilibrium by Fractional Controller for Magnetic Levitation Systems. Fractal and Fractional. 2021; 5(3):101. https://doi.org/10.3390/fractalfract5030101
Chicago/Turabian StyleAtaşlar-Ayyıldız, Banu, Oğuzhan Karahan, and Serhat Yılmaz. 2021. "Control and Robust Stabilization at Unstable Equilibrium by Fractional Controller for Magnetic Levitation Systems" Fractal and Fractional 5, no. 3: 101. https://doi.org/10.3390/fractalfract5030101
APA StyleAtaşlar-Ayyıldız, B., Karahan, O., & Yılmaz, S. (2021). Control and Robust Stabilization at Unstable Equilibrium by Fractional Controller for Magnetic Levitation Systems. Fractal and Fractional, 5(3), 101. https://doi.org/10.3390/fractalfract5030101






