Nonlinear Vibration of a Nonlocal Nanobeam Resting on Fractional-Order Viscoelastic Pasternak Foundations
Abstract
:1. Introduction
2. Preliminaries
2.1. Fractional Order Viscoelasticity
- The left Riemann–Liouville fractional derivative of order is of the form:
- The right Riemann–Liouville fractional derivative of order is of the form:
2.2. Nonlocal Theory
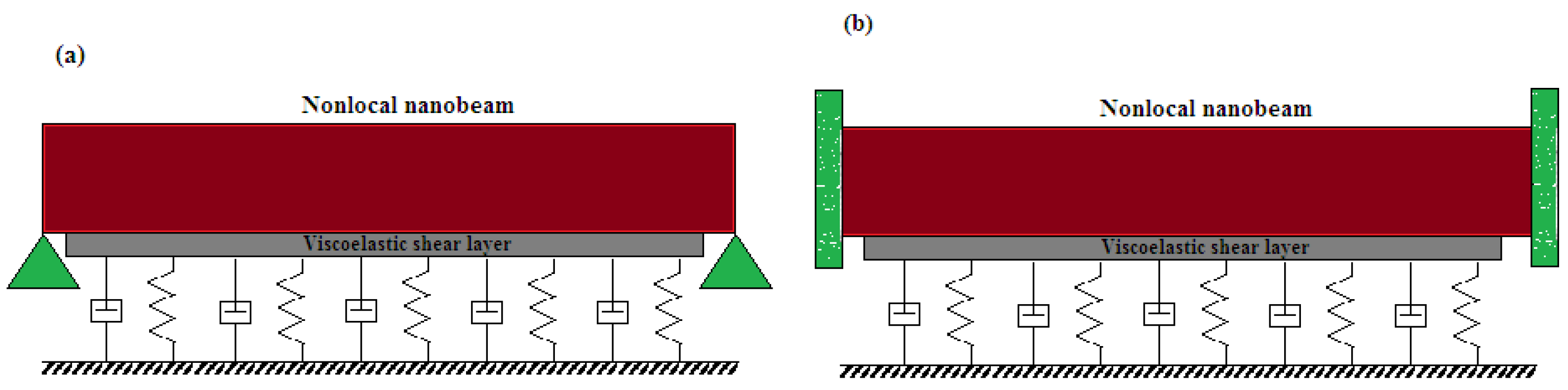
3. Governing Equation of the Nanobeam Resting on the Fractional Order Viscoelastic Foundation
Solution of the Governing Equation
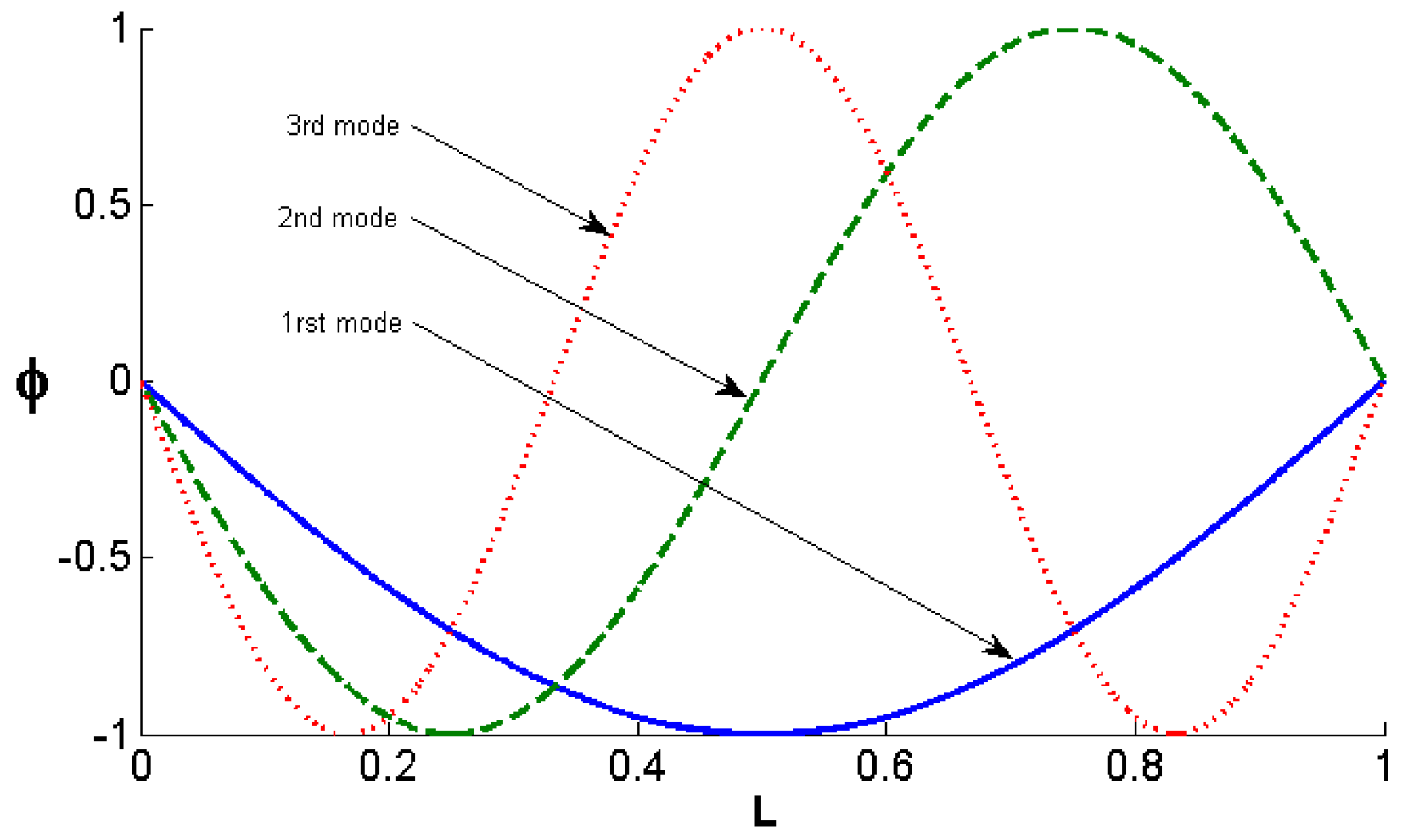
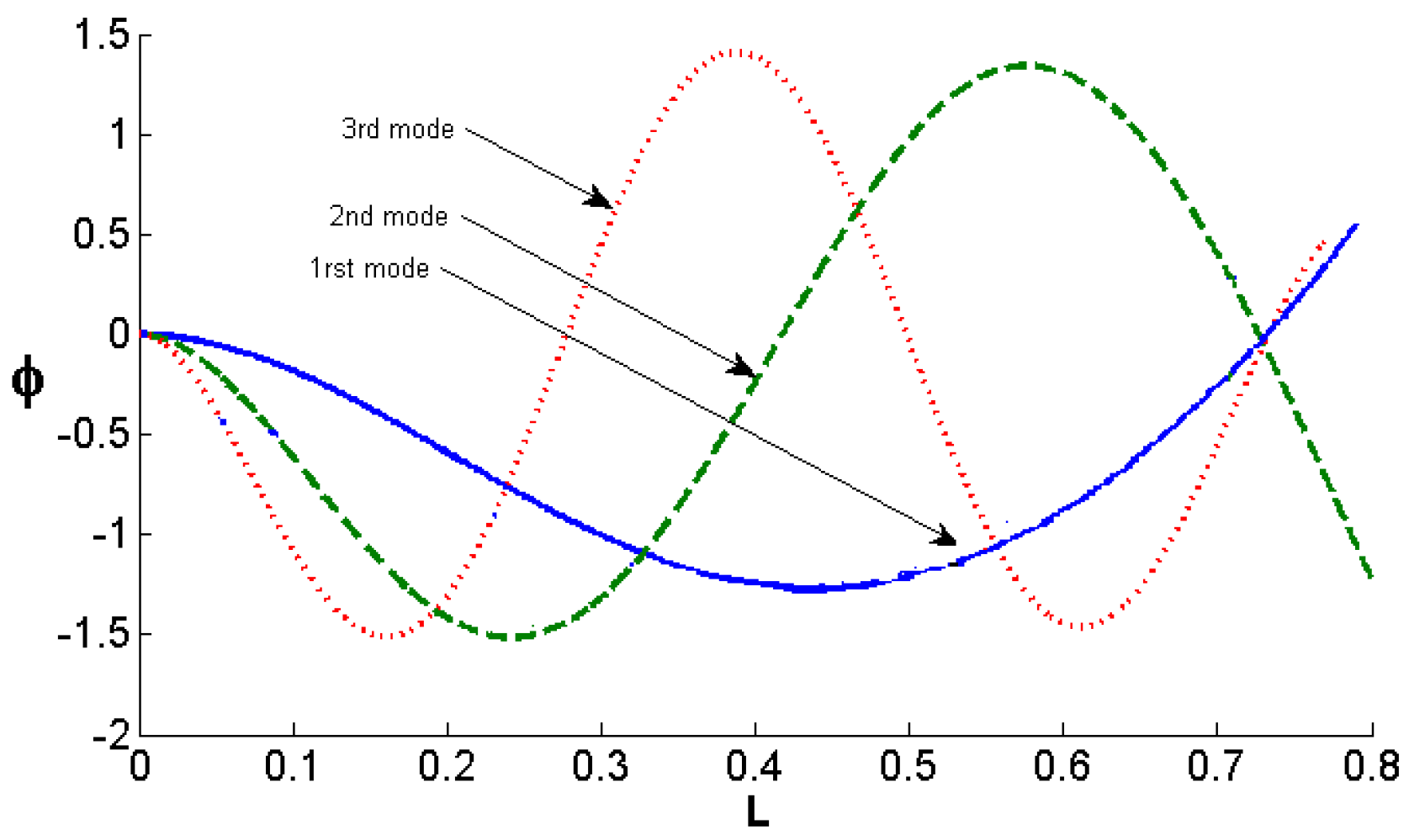
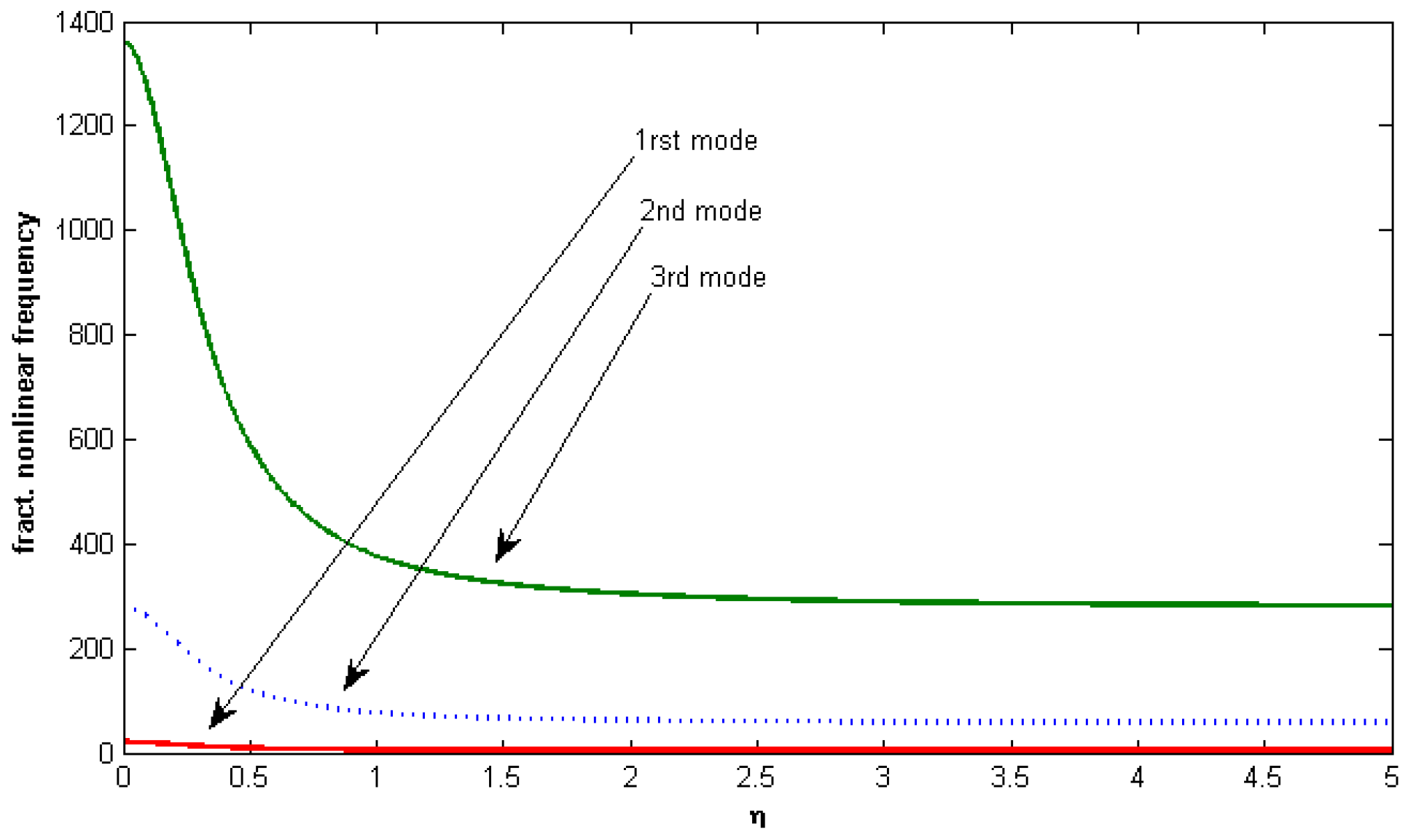
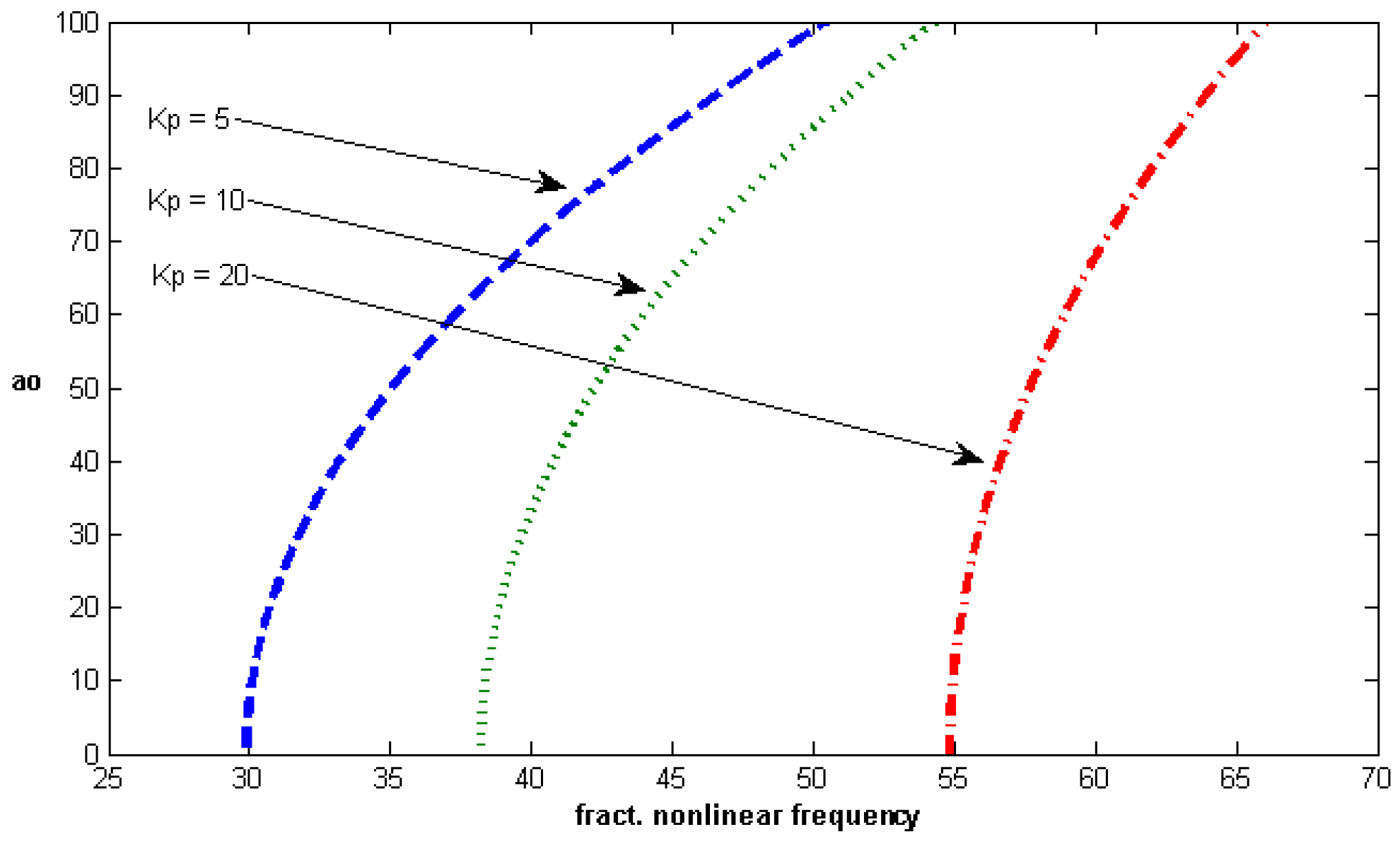
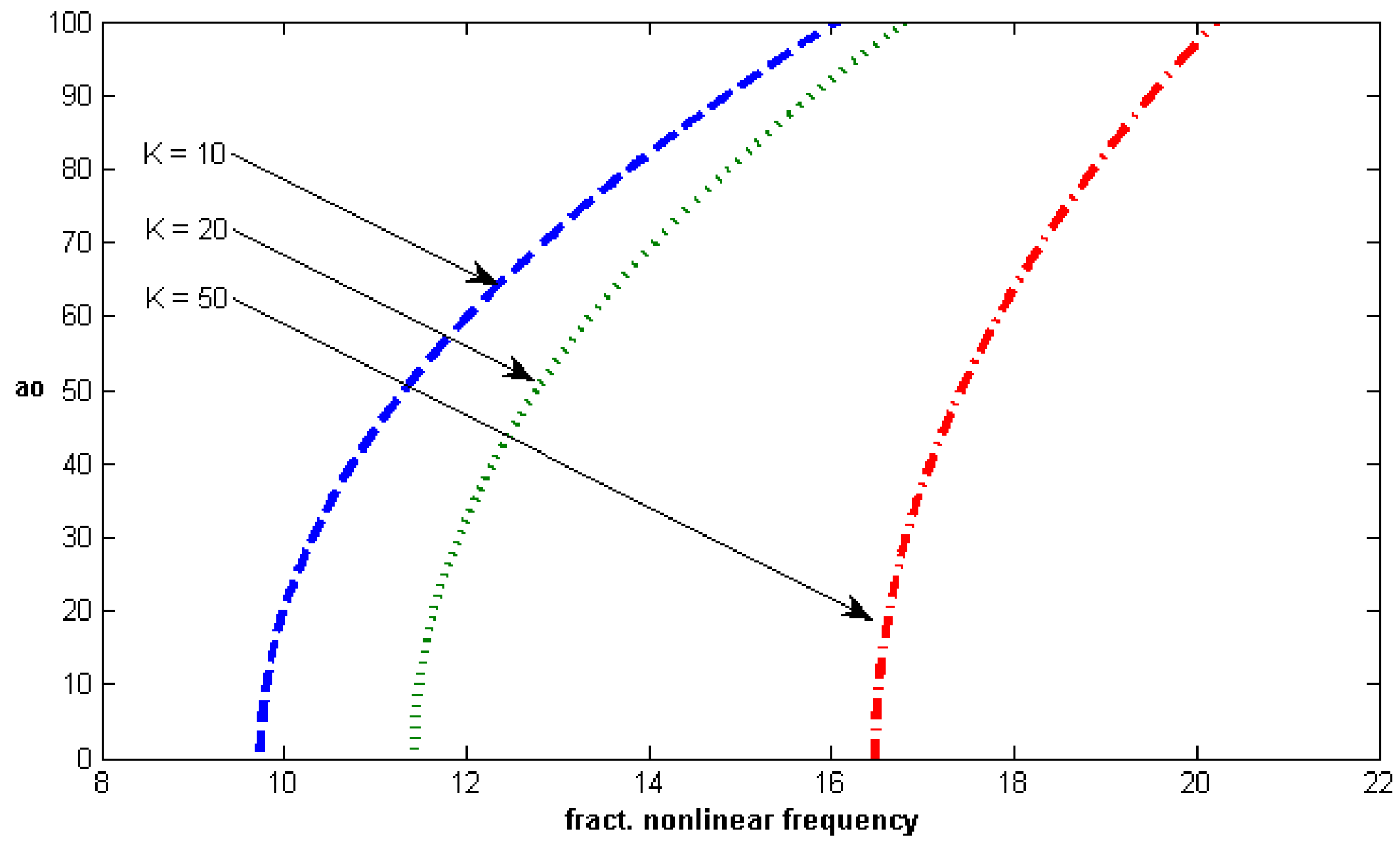
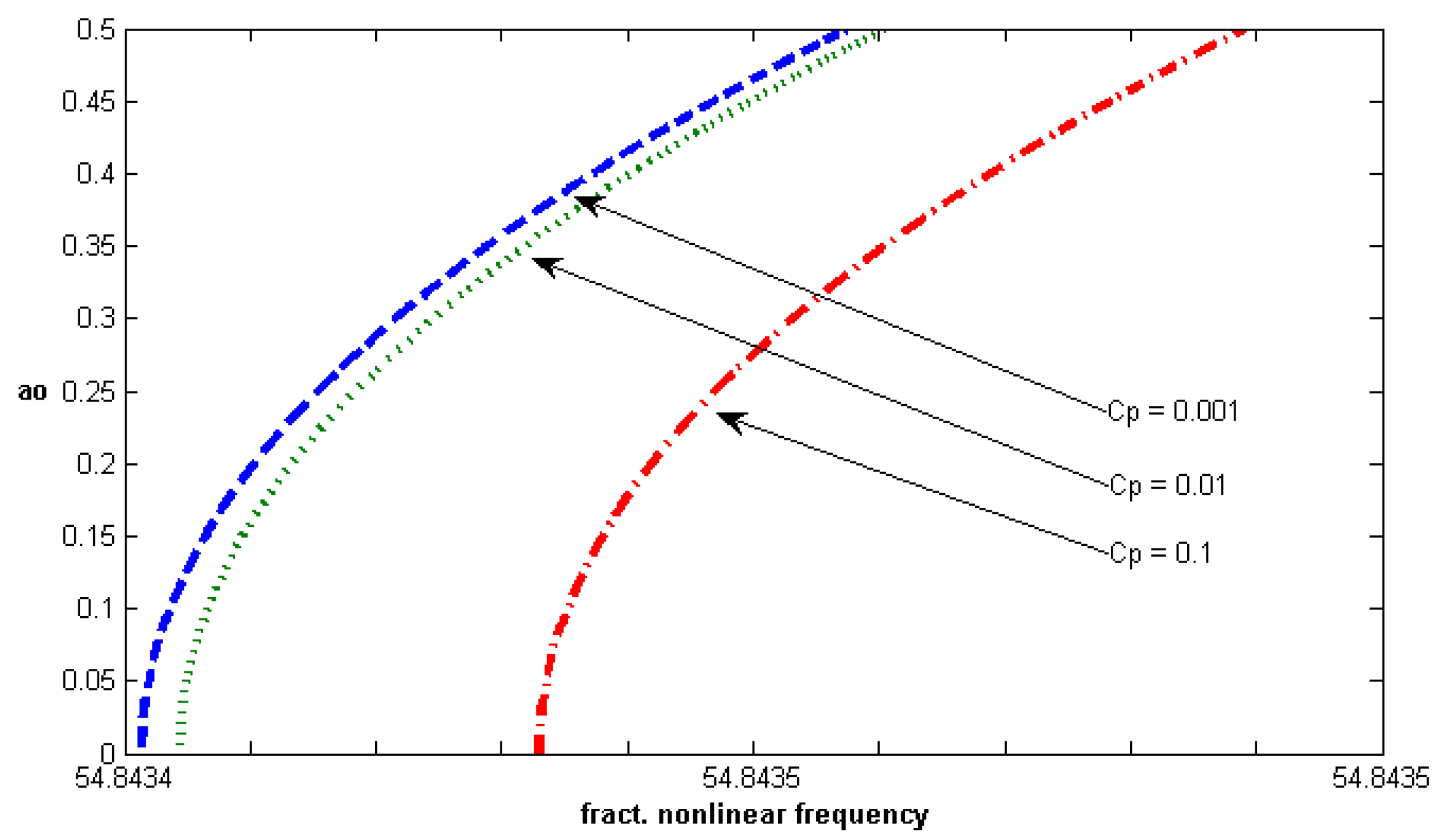
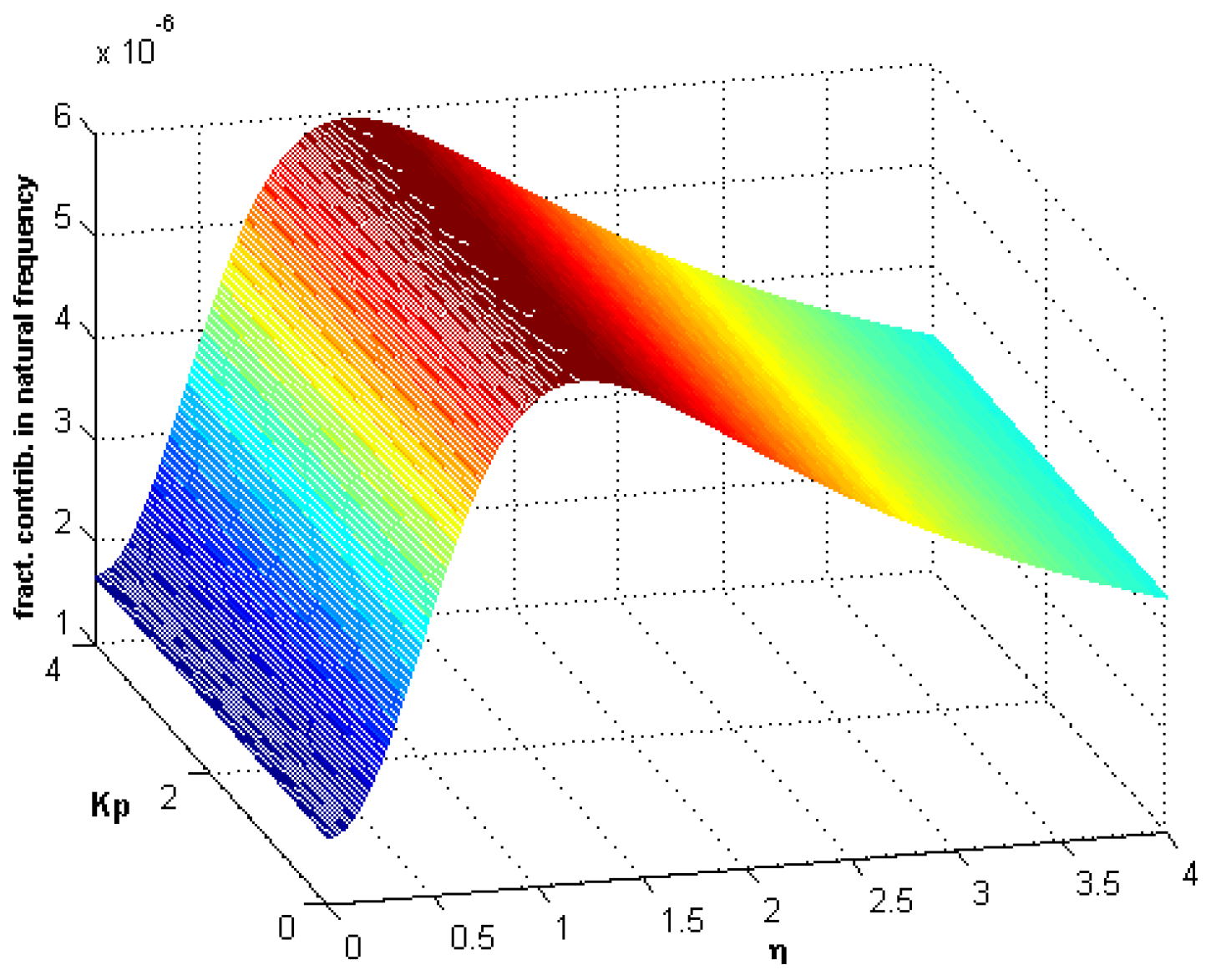
4. Numerical Results
Validation Study
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CNT | Carbon nanotube |
| SDCNT | Single-walled carbon nanotube |
| DWCNT | Double-walled carbon nanotube |
References
- Eringen, A.C. On differential equations of nonlocal elasticity and solution of screw dislocation and surface waves. J. Appl. Phys. 1983, 54, 4703–4710. [Google Scholar] [CrossRef]
- Eringen, A.C. Nonlocal Continuum Fields Theories; Springer: New York, NY, USA, 2002. [Google Scholar]
- Kargonovin, M.H.; Younesian, D.; Thompson, D.J.; Jones, C.J.C. Response of beams on the nonlinear viscoelastic foundations to harmonic moving loads. Comput. Struct. 2005, 83, 1865–1877. [Google Scholar] [CrossRef]
- Sapountzakis, E.J.; Kampitsis, A. Nonlinear response of shear deformable beams on tensionless nonlinear viscoelastic foundation under moving loads. J. Sound Vib. 2011, 330, 5410–5426. [Google Scholar] [CrossRef]
- Hryniewicz, Z. Dynamics of rayleigh beam on nonlinear foundation due to moving load using adomian decomposition and coiflet expansion. Soil Dyn. Earthq. Eng. 2011, 31, 1123–1131. [Google Scholar] [CrossRef]
- Ding, H.; Chen, Q.L.; Yang, S.P. Convergence of garlekin truncation for dynamic response of finite beams on nonlinear foundations under a moving load. J. Sound Vib. 2012, 331, 2426–2442. [Google Scholar] [CrossRef]
- Mathews, P.M. Vibrations of a beam on elastic foundation. J. Appl. Math. Mech. 1958, 38, 105–115. [Google Scholar] [CrossRef]
- Haitao, Y.; Yuan, Y. Analytical solution for an infinite euler-bernoulli beam on a visco-elastic foundation subjected to arbitrary dynamic loads. J. Eng. Mech. 2014, 140, 542–551. [Google Scholar]
- Achenbach, J.D.; Sun, C. Moving load on a flexibly supported timochenko beam. Int. J. Solids Struct. 1965, 1, 353–370. [Google Scholar] [CrossRef]
- Anague Tabejieu, L.M.; Nana Nbendjo, B.R.; Woafo, P. On the dynamics of rayleigh beams resting on fractional-order viscoelastic Pasternak foundations subjected to moving loads. Chaos Solitons Fract. 2016, 93, 39–47. [Google Scholar] [CrossRef]
- Niknam, H.; Aghdam, M.M. A semi analytical approach for large amplitude free vibration and buckling of nonlocal FG beams resting on elastic foundation. Compos. Struct. 2015, 119, 452–462. [Google Scholar] [CrossRef]
- Kiani, K. A meshless approach for free transverse vibration of embedded single walled nanotubes with arbitrary boundary conditions accounting for nonlocal effect. Int. J. Mech. Sci. 2010, 52, 1343–1356. [Google Scholar] [CrossRef]
- Murmu, T.; Pradhan, S.C. Buckling analysis of a single-walled carbon nanotube embedded in an elastic medium based on nonlocal elasticity and Timoshenko beam theory and using DQM. Physica E 2009, 41, 1232–1239. [Google Scholar] [CrossRef]
- Pradhan, S.C.; Reddy, G.K. Buckling analysis of single walled carbon nanotube on Winkler foundation using nonlocal elasticity theory and DTM. Comput. Mater. Sci. 2011, 50, 1052–1056. [Google Scholar] [CrossRef]
- Kazemi-Lari, M.A.; Fazelzadeh, S.A.; Ghavanloo, E. Non-conservative instability of cantilever carbon nanotubes resting on viscoelastic foundation. Physica E 2012, 44, 1623–1630. [Google Scholar] [CrossRef]
- Mikhasev, G. On localized modes of free vibrations of single-walled carbon nanotubes embedded in nonhomogeneous elastic medium. Z. Angew. Math. Mech. 2014, 94, 130–141. [Google Scholar] [CrossRef]
- Mustapha, K.B.; Zhong, Z.W. Free transverse vibration of an axially loaded non-prismatic single-walled carbon nanotube embedded in a two parameter elastic medium. Comput. Mater. Sci. 2010, 50, 742–751. [Google Scholar] [CrossRef]
- Lee, H.L.; Chang, W.J. Vibration analysis of a viscous-fluid-conveying single-walled carbon nanotube embedded in an elastic medium. Physica E 2009, 41, 529–532. [Google Scholar] [CrossRef]
- Kiani, K. Vibration analysis of elastically restrained double-walled carbon nanotubes on elastic foundation subject to axial load using nonlocal shear deformable beam theories. Int. J. Mech. Sci. 2013, 68, 16–34. [Google Scholar] [CrossRef]
- Kiani, K. Nonlinear vibrations of a single-walled carbon nanotube for delivering of nanoparticles. Nonlinear Dyn. 2014, 76, 1885–1903. [Google Scholar] [CrossRef]
- Ghavanloo, E.; Daneshmand, F.; Rafiei, M. Vibration and instability analysis of carbon nanotubes conveying fluid and resting on a linear viscoelastic Winkler foundation. Physica E 2010, 42, 2218–2224. [Google Scholar] [CrossRef]
- Yas, M.H.; Samadi, N. Free vibrations and buckling analysis of carbon nanotube-reinforced composite Timoshenko beams on elastic foundation. Int. J. Press Vessels Piping 2012, 98, 119–128. [Google Scholar] [CrossRef]
- Rafiei, M.; Mohebpour, S.R.; Daneshmand, F. Small-scale effect on the vibration of non-uniform carbon nanotubes conveying fluid and embedded in viscoelastic medium. Physica E 2012, 44, 1372–1379. [Google Scholar] [CrossRef]
- Aydogdu, M. Axial vibration analysis of nanorods (carbon nanotubes) embedded in an elastic medium using nonlocal elasticity. Mech. Res. Commun. 2012, 43, 34–40. [Google Scholar] [CrossRef]
- Wang, B.L.; Wang, K.F. Vibration analysis of embedded nanotubes using nonlocal continuum theory. Composites Part B Eng. 2013, 47, 96–101. [Google Scholar] [CrossRef]
- Mehdipour, I.; Barari, A.; Kimiaeifar, A.; Domairry, G. Vibrational analysis of curved single-walled carbon nanotube on a Pasternak elastic foundation. Adv. Eng. Softw. 2012, 48, 1–5. [Google Scholar] [CrossRef]
- Aydogdu, M.; Arda, M. Torsional vibration analysis of double walled carbon nanotubes using nonlocal elasticity. Int. J. Mech. Mater. Des. 2016, 12, 71–84. [Google Scholar] [CrossRef]
- Togun, N.; Bagdatli, S.M. Nonlinear vibration of a nanobeam on Pasternak elastic foundation based on nonlocal euler-bernoulli beam theory. Math. Comput. Appl. 2016, 21, 1–19. [Google Scholar]
- Ozturk, B.; Coskun, S.B. The homotopy perturbation method for free vibration analysis of beams on elastic foundation. Struct. Eng. Mech. 2011, 37, 415–425. [Google Scholar] [CrossRef]
- Yan, Y.; Wang, W.; Zhang, L. Applied multiscale method to analysis of nonlinear vibration for double-walled carbon nanotubes. Appl. Math. Model. 2011, 35, 2279–2289. [Google Scholar] [CrossRef]
- Wang, Y.Z.; Li, F.M. Nonlinear free vibration of nanotube with small scale effects embedded in viscous matrix. Mech. Res. Commun. 2014, 60, 45–51. [Google Scholar] [CrossRef]
- Bagdatli, S.M. Nonlinear vibration of nanobeams with various boundary condition based on nonlocal elasticity theory. Composites Part B Eng. 2015, 80, 43–52. [Google Scholar] [CrossRef]
- Bagdatli, S.M. Non-linear transverse vibrations of tensioned nanobeams using nonlocal beam theory. Struct. Eng. Mech. 2015, 55, 281–298. [Google Scholar] [CrossRef]
- Simsek, M. Large amplitude free vibration of nanobeams with various boundary conditions based on the nonlocal elasticity theory. Composites Part B Eng. 2014, 56, 621–628. [Google Scholar] [CrossRef]
- Simsek, M. Nonlinear static and free vibration analysis of microbeams based on the nonlinear elastic foundation using modified couple stress theory and He’s variational method. Compos. Struct. 2014, 112, 264–272. [Google Scholar] [CrossRef]
- Fallah, A.; Aghdam, M.M. Nonlinear free vibration and post-buckling analysis of functionally graded beams on nonlinear elastic foundation. Eur. J. Mech. A Solids 2011, 30, 571–583. [Google Scholar] [CrossRef]
- Fallah, A.; Aghdam, M.M. Thermo-mechanical buckling and nonlinear free vibration analysis of functionally graded beams on nonlinear elastic foundation. Composites Part B Eng. 2012, 43, 1523–1530. [Google Scholar] [CrossRef]
- Ke, L.L.; Xiang, Y.; Yang, J.; Kitipornchai, S. Nonlinear free vibration of embedded double-walled carbon nanotubes based on nonlocal Timoshenko beam theory. Comput. Mater. Sci. 2009, 47, 409–417. [Google Scholar] [CrossRef]
- Pritz, T. Analysis of four parameter fractional derivative model of real solid materials. J. Sound Vib. 1996, 195, 103–115. [Google Scholar] [CrossRef]
- Koeller, R.C. Application of fractional calculus to the theory of viscoelasticity. ASME J. Appl. Mech. 1984, 51, 299–307. [Google Scholar] [CrossRef]
- Nayfeh, A.H. Introduction to Pertubation Techniques; John Wiley: New York, NY, USA, 1981. [Google Scholar]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; John Wiley: New York, NY, USA, 1979. [Google Scholar]
- Yokoyama, T. Vibrations and transient responses of timoshenko beams resting on elastic foundations. Arch. Appl. Mech. 1987, 57, 81–90. [Google Scholar] [CrossRef]





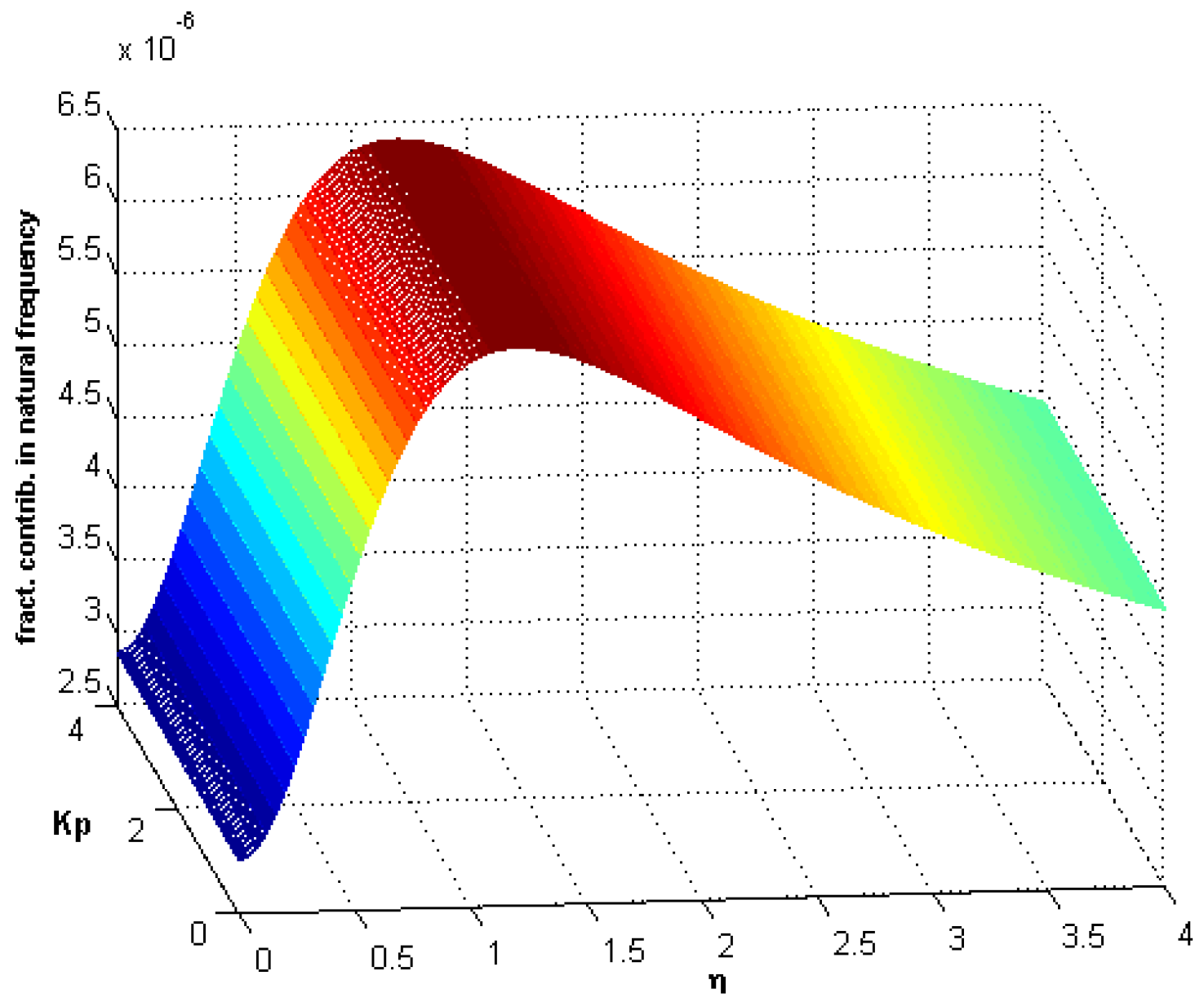
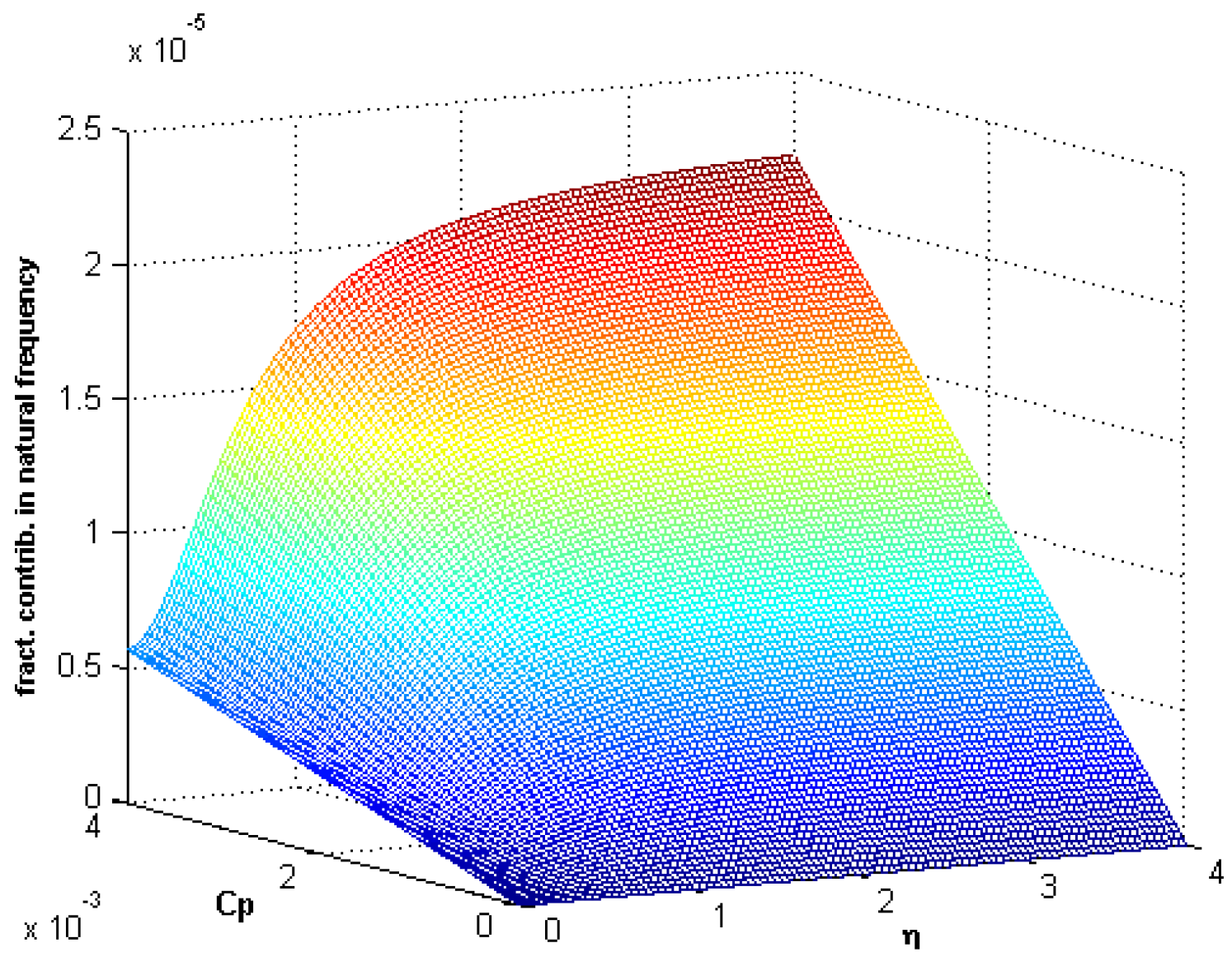
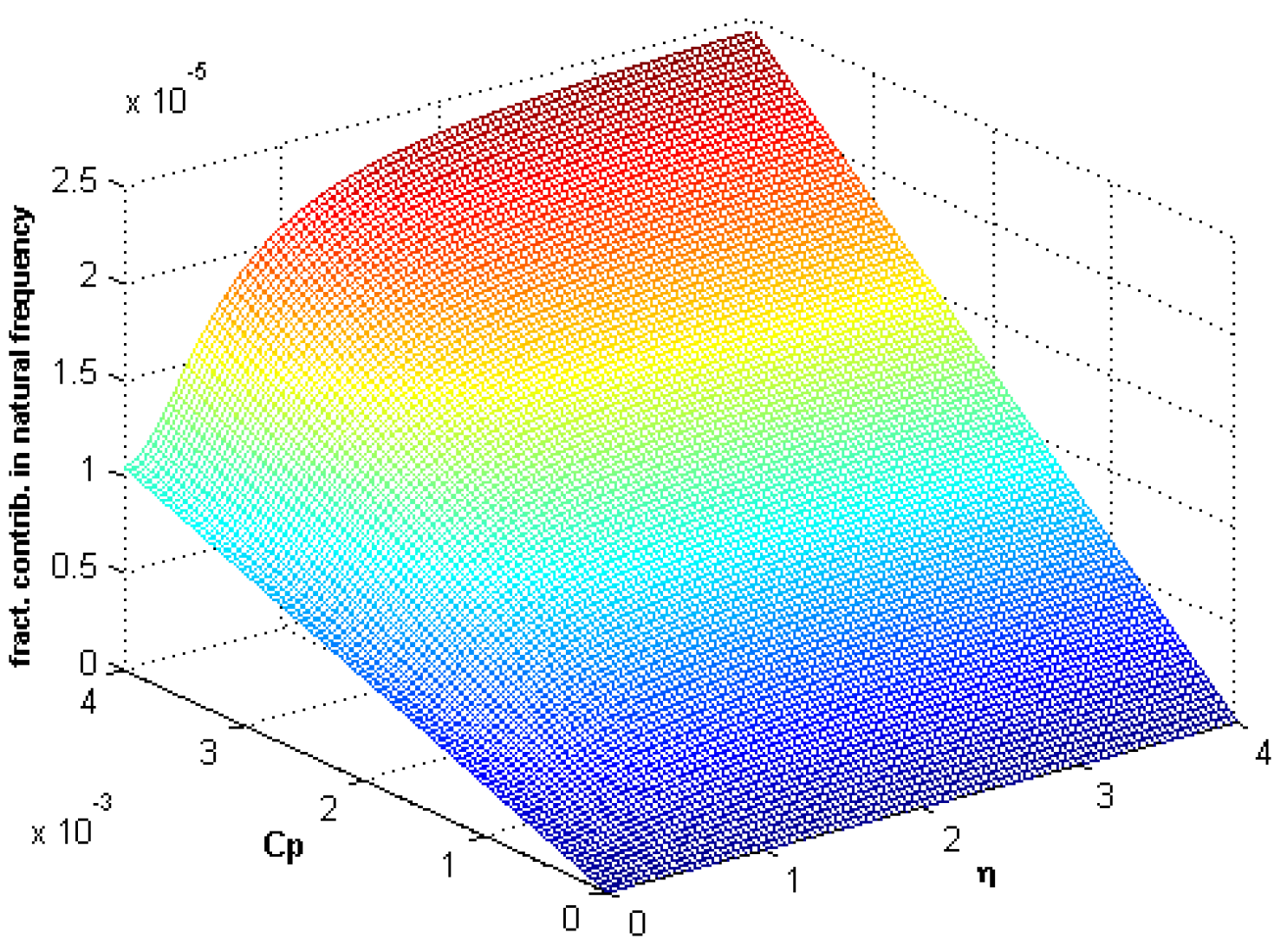
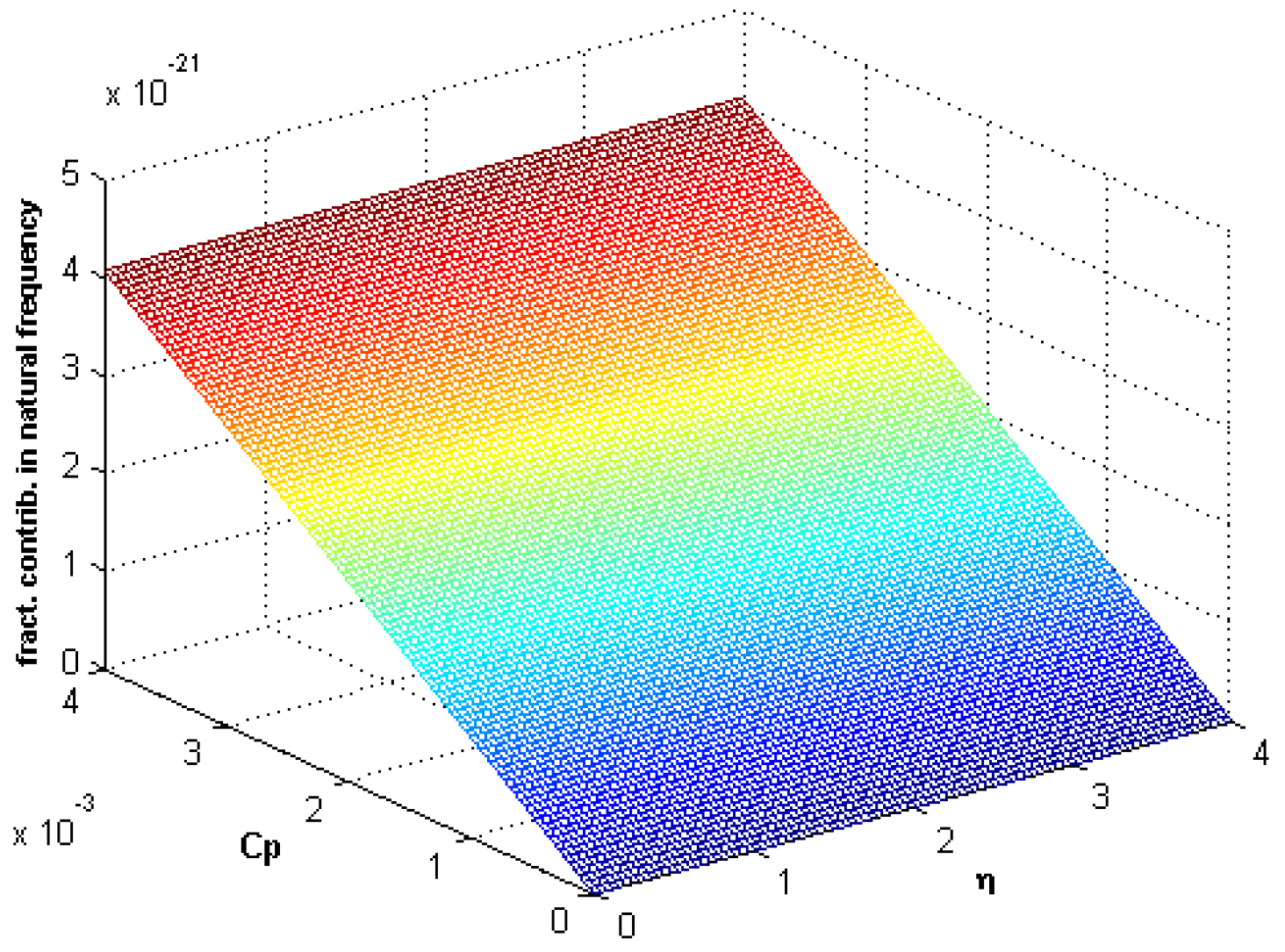
| Mode | Present | Ref. [28] | Ref. [17] | Ref. [43] |
|---|---|---|---|---|
| 1 | 19.2133 | 19.2133 | 19.2178 | 19.21 |
| 2 | 50.7002 | 50.7002 | 50.7804 | 50.71 |
| 3 | 100.6767 | 100.677 | - | - |
| 4 | 170.0281 | 170.028 | - | - |
| 5 | 258.9868 | 258.987 | - | - |
| Mode | Present | Ref. [28] | Ref. [17] | Ref. [43] |
|---|---|---|---|---|
| 1 | 22.1069 | 22.1069 | 22.1112 | - |
| 2 | 54.9160 | 54.916 | 55.1873 | - |
| 3 | 105.4698 | 105.47 | - | - |
| 4 | 175.0932 | 175.093 | - | - |
| 5 | 264.1956 | 264.196 | - | - |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eyebe, G.J.; Betchewe, G.; Mohamadou, A.; Kofane, T.C. Nonlinear Vibration of a Nonlocal Nanobeam Resting on Fractional-Order Viscoelastic Pasternak Foundations. Fractal Fract. 2018, 2, 21. https://doi.org/10.3390/fractalfract2030021
Eyebe GJ, Betchewe G, Mohamadou A, Kofane TC. Nonlinear Vibration of a Nonlocal Nanobeam Resting on Fractional-Order Viscoelastic Pasternak Foundations. Fractal and Fractional. 2018; 2(3):21. https://doi.org/10.3390/fractalfract2030021
Chicago/Turabian StyleEyebe, Guy Joseph, Gambo Betchewe, Alidou Mohamadou, and Timoleon Crepin Kofane. 2018. "Nonlinear Vibration of a Nonlocal Nanobeam Resting on Fractional-Order Viscoelastic Pasternak Foundations" Fractal and Fractional 2, no. 3: 21. https://doi.org/10.3390/fractalfract2030021
APA StyleEyebe, G. J., Betchewe, G., Mohamadou, A., & Kofane, T. C. (2018). Nonlinear Vibration of a Nonlocal Nanobeam Resting on Fractional-Order Viscoelastic Pasternak Foundations. Fractal and Fractional, 2(3), 21. https://doi.org/10.3390/fractalfract2030021




