Aeroelastic Response in an Oscillating Transonic Compressor Cascade—An Experimental and Numerical Approach †
Abstract
1. Introduction
2. Materials and Methodology
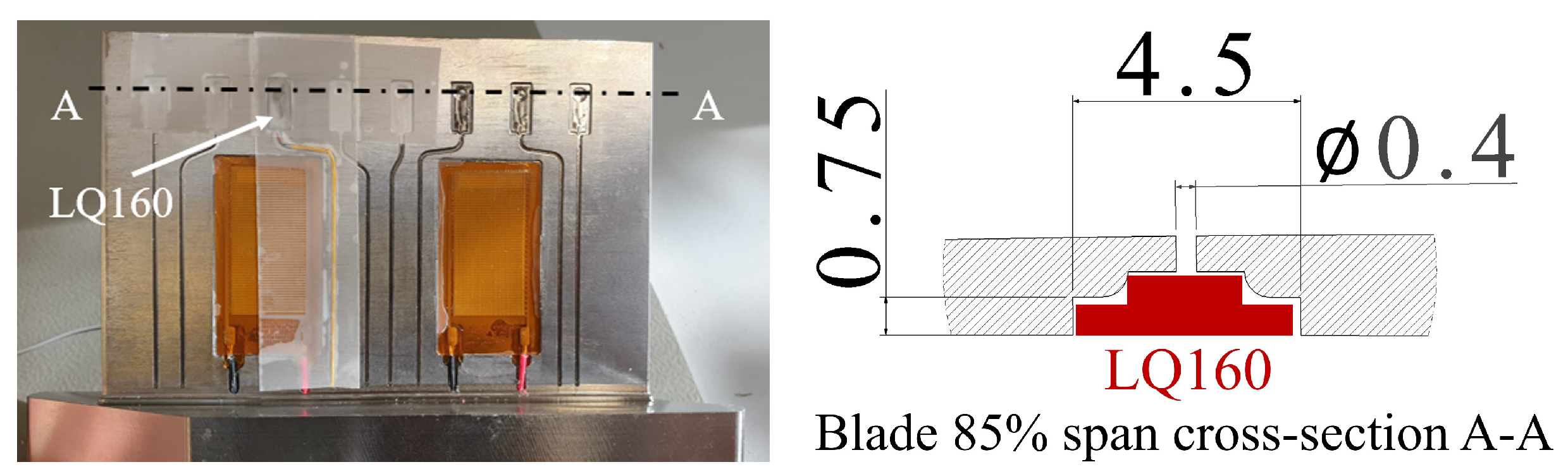
2.1. Test Case
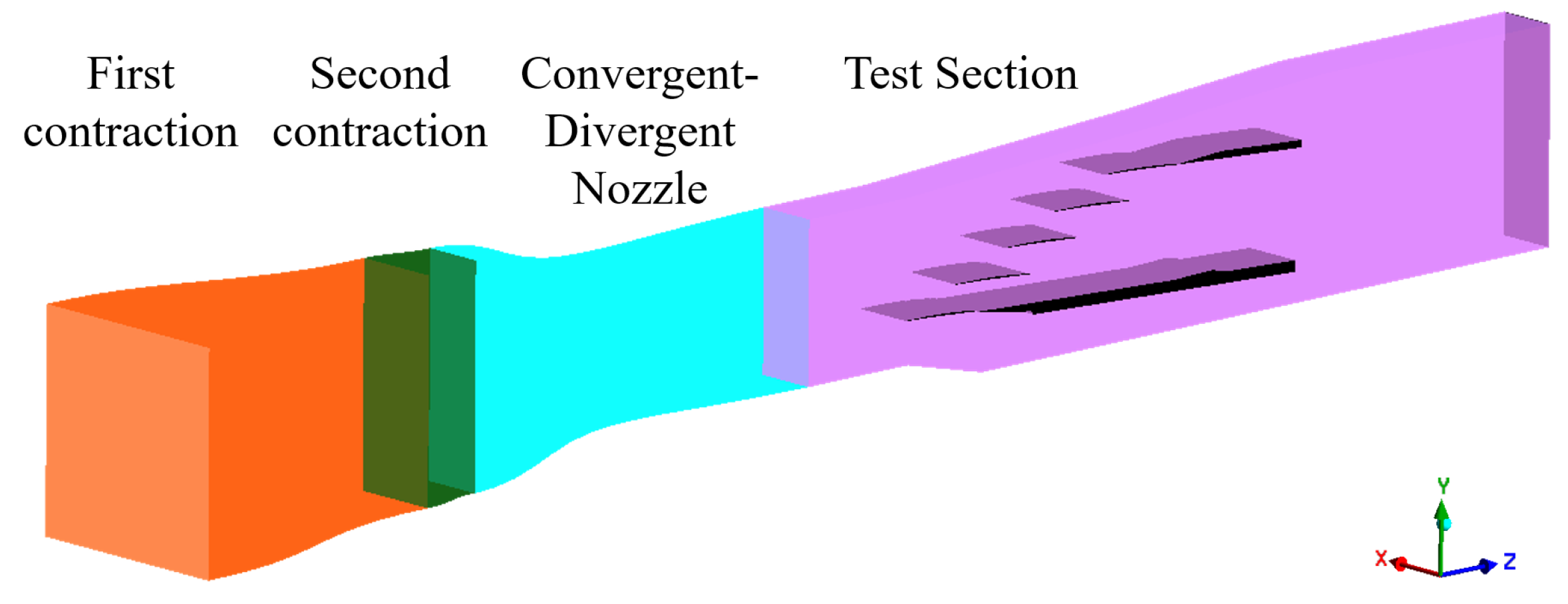
2.2. Experimental Setup
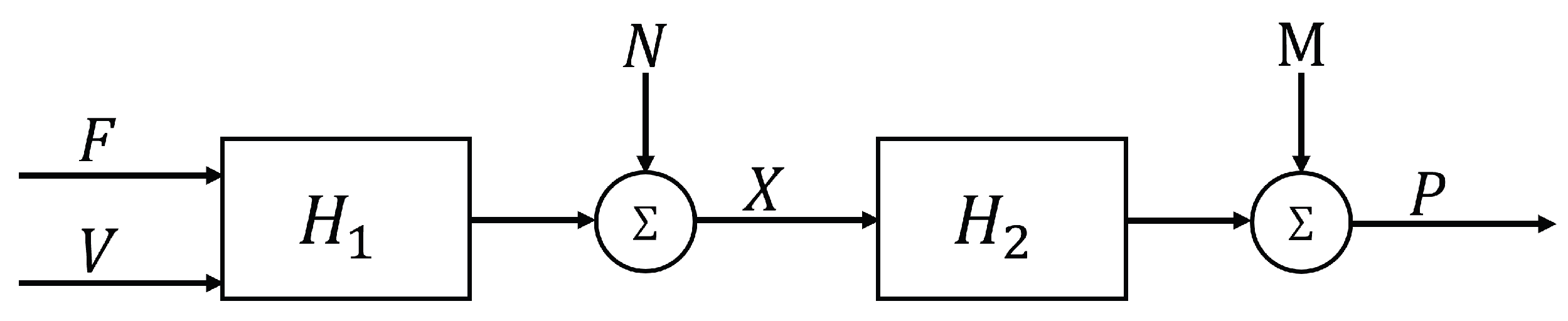
2.3. Numerical Setup
3. Results and Discussion
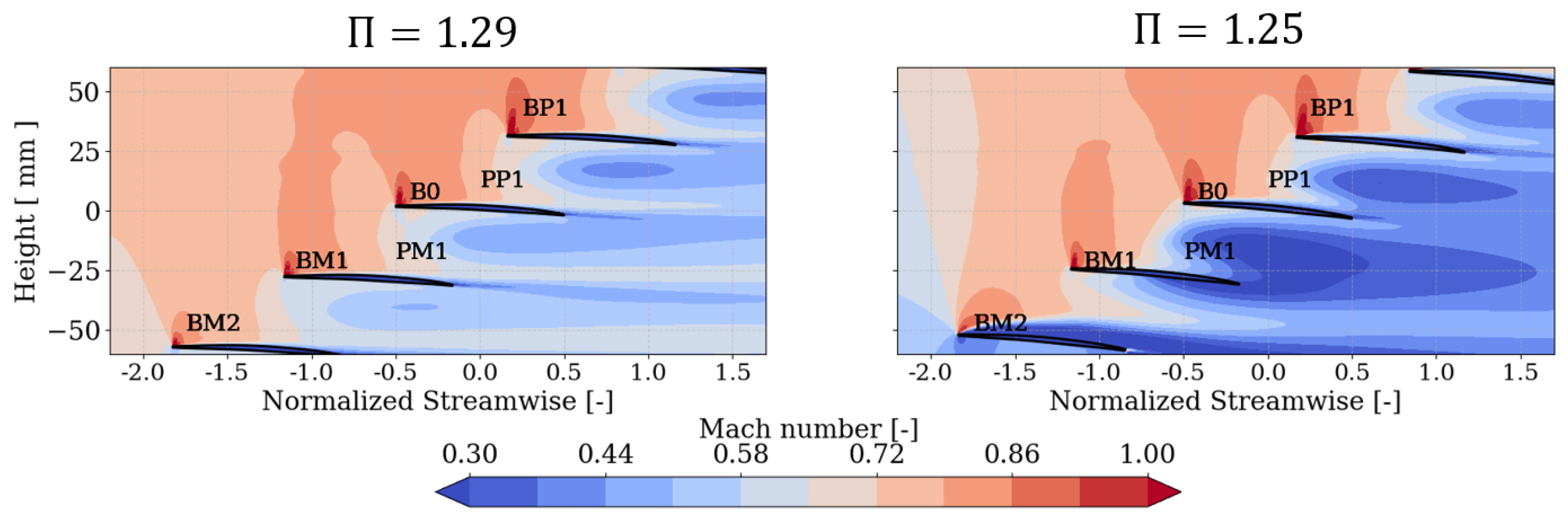
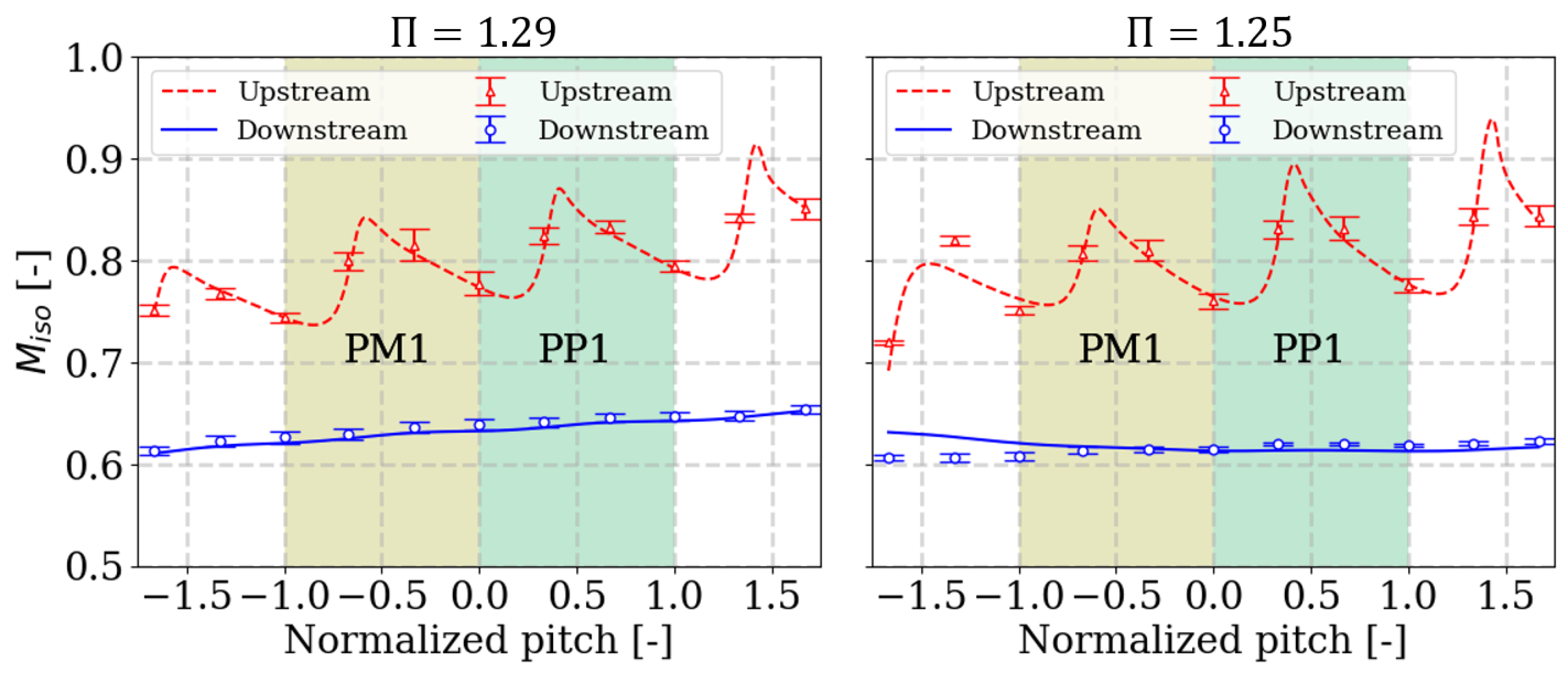
3.1. Steady-State Aerodynamics
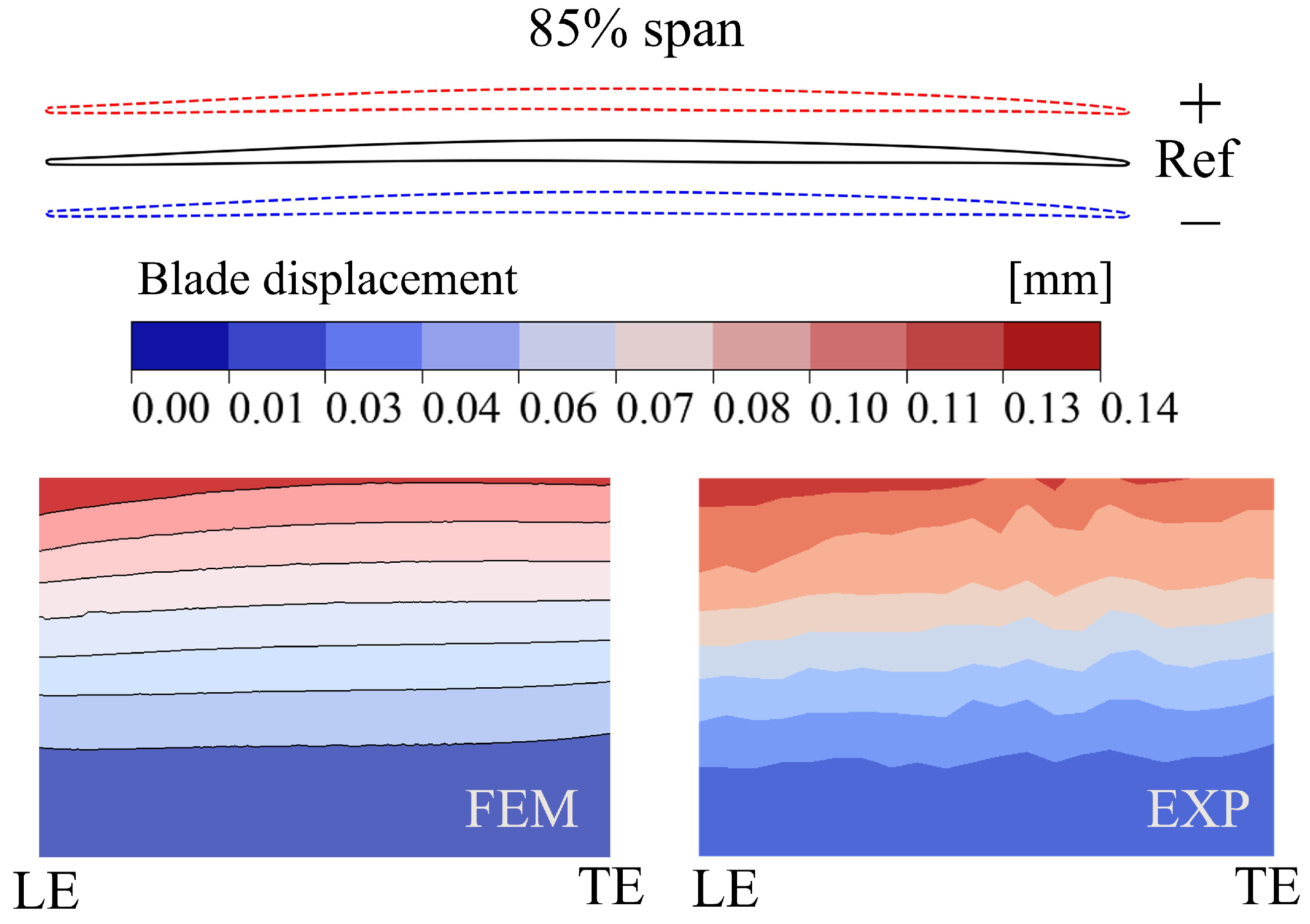
3.2. Aeroelastic Response
4. Conclusions
- The central (B0) and adjacent blades (BP1 and BM1) are subjected to slightly different upstream conditions that modify the blade loadings at each operating point. However, the numerical model can predict such differences with a quantitative agreement.
- A small separation bubble was confirmed experimentally. At an 85% span, near-wall effects are visible, and reattachment length measurements are carried out at 50% to avoid three-dimensional influence. The numerical reattachment length is within a 0.7 mm standard deviation from experimental data for both operating points.
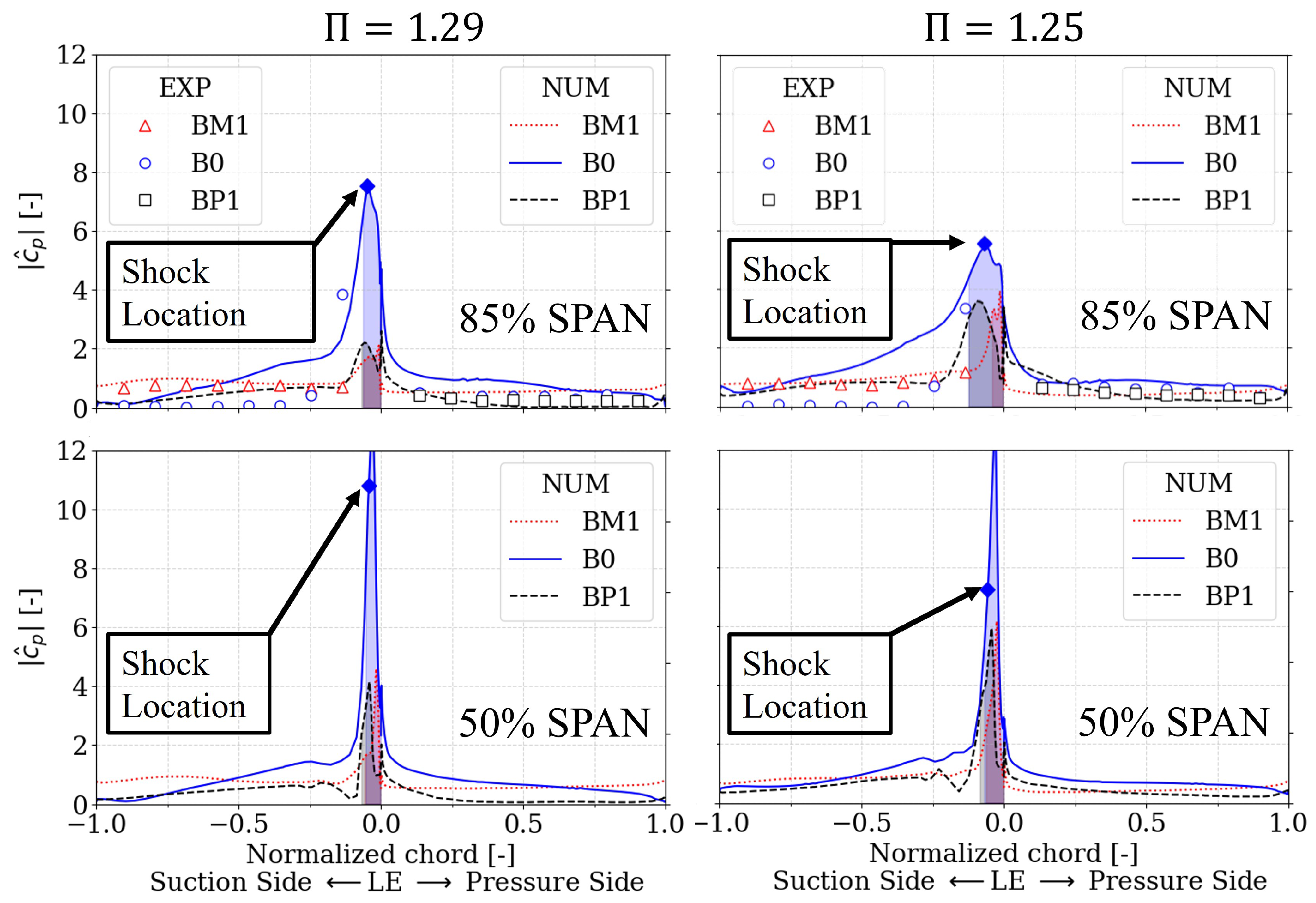
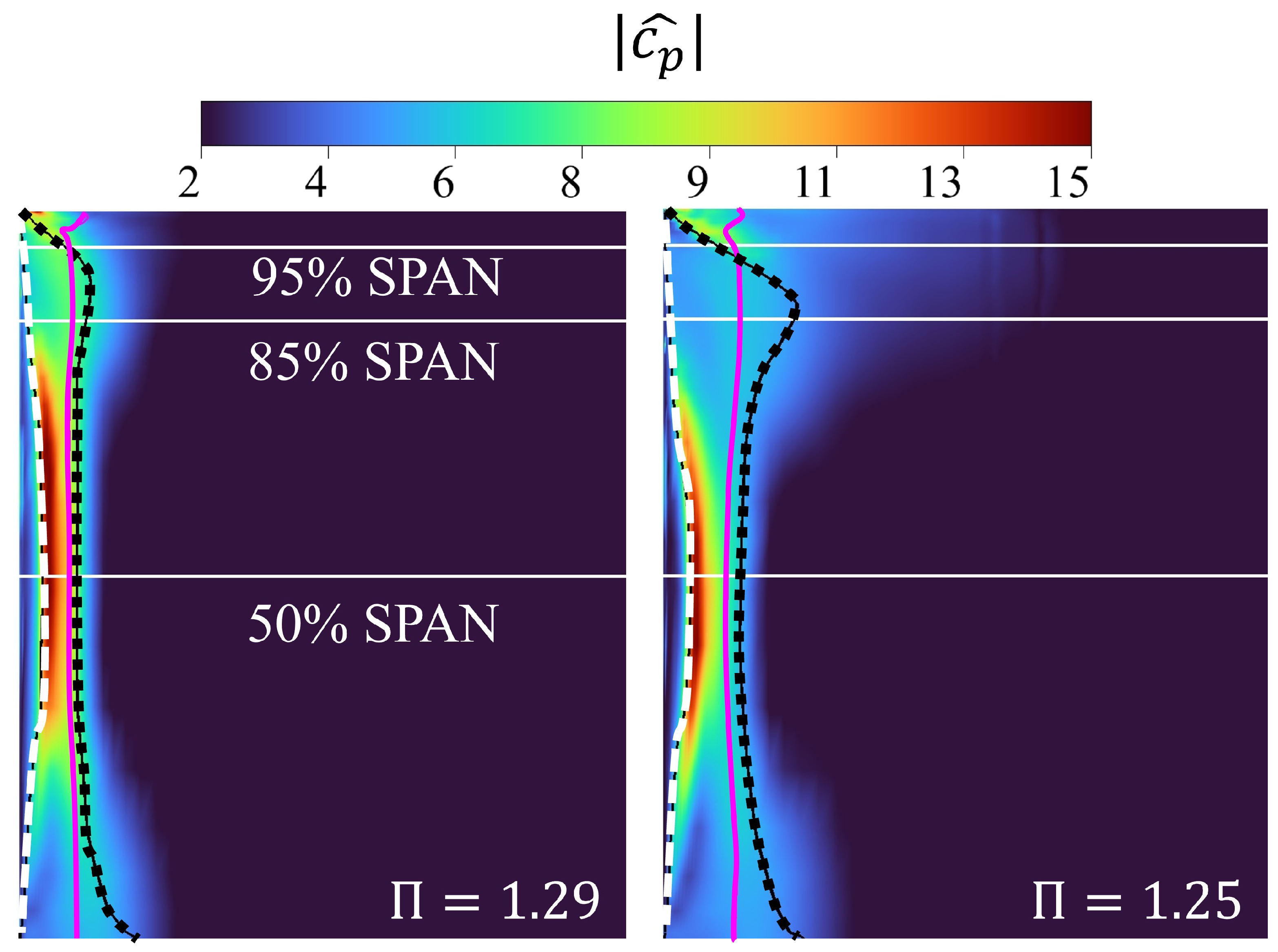
- At both operating points, the numerical harmonic pressure amplitude coefficient at midspan shows that the shock is the main contributor. The shock interacts with the separation onset, where its maximum amplitude is located. The amplitude from this interaction decreases between and , as the shock location is further downstream from the separation line at .
- At both operating points, the numerical harmonic pressure amplitude is affected by near-wall effects. At the measurement plane, the shock dominates the response with an agreement between the maximum amplitude and the shock location.
- Experimental and numerical data at an 85% span display a qualitative agreement in the harmonic pressure at the adjacent blades BM1 and BP1. However, on the suction side of the oscillating blade, there is a qualitative difference between both methods downstream from an ≈30% chord, where CFD appears to be overpredicting the harmonic amplitude with no clear root cause. The phase showed larger qualitative variations on the Blade Plus 1 pressure side, which was attributed to a low signal amplitude.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Abbreviations | |
| A | Amplitude of vibration |
| BM1 | Blade Minus 1 |
| BM2 | Blade Minus 2 |
| BP1 | Blade Plus 1 |
| BP2 | Blade Plus 2 |
| B0 | Blade Zero |
| LE | Leading edge |
| PS | Pressure side |
| PSD | Power-spectral density |
| RM | Reattachment modification |
| PM1 | Passage Minus 1 |
| PP1 | Passage Plus 1 |
| SS | Suction side |
| TE | Trailing edge |
| TLC | Transonic linear cascade |
| VINK | Virtual Integrated Compressor |
| Subscripts | |
| iso | Isentropic |
| ref | Reference value |
| rms | Root-mean-squared |
| tot | Total flow property at stagnation chamber |
| 0 | Total local flow property |
| vacuum | Vacuum conditions |
| Latin | |
| M | Mach number |
| Complex unsteady pressure coefficient | |
| P | Pressure |
| T | Temperature |
| V | Voltage |
| Geek | |
| Total-to-static pressure ratio | |
| Eigen-frequency | |
| Phase lag between blade motion and pressure |
References
- Biollo, R.; Benini, E. Recent advances in transonic axial compressor aerodynamics. Prog. Aerosp. Sci. 2013, 56, 1–18. [Google Scholar] [CrossRef]
- Thermann, H.; Niehuis, R. Unsteady Navier-Stokes Simulation of a Transonic Flutter Cascade Near-Stall Conditions Applying Algebraic Transition Models. J. Turbomach. 2005, 128, 474–483. [Google Scholar] [CrossRef]
- Srinivasan, A.V. Flutter and Resonant Vibration Characteristics of Engine Blades. J. Eng. Gas Turbines Power 1997, 119, 742–775. [Google Scholar] [CrossRef]
- Holzinger, F.; Wartzek, F.; Schiffer, H.P.; Leichtfuss, S.; Nestle, M. Self-Excited Blade Vibration Experimentally Investigated in Transonic Compressors: Acoustic Resonance. J. Turbomach. 2015, 138, 041001. [Google Scholar] [CrossRef]
- Bölcs, A.; Fransson, T.H. Aeroelasticity in Turbomachines. Comparison of Theoretical and Experimental Cascade Results; Technical Report; Ecole Polytechnique Federale de Lausanne (Switzerland) lab de Thermique: Lausanne, Switzerland, 1986. [Google Scholar]
- Hanamura, Y.; Tanaka, H.; Yamaguchi, K. A Simplified Method to Measure Unsteady Forces Acting on the Vibrating Blades in Cascade. Bull. JSME 1980, 23, 880–887. [Google Scholar] [CrossRef]
- Ellenberger, K.; Gallus, H.E. Experimental Investigations of Stall Flutter in a Transonic Cascade. In Turbo Expo: Power for Land, Sea, and Air; American Society of Mechanical Engineers: New York, NY, USA, 1999; p. V004T03A047. [Google Scholar] [CrossRef]
- Buffum, D.H.; Capece, V.R.; King, A.J.; EL-Aini, Y.M. Oscillating Cascade Aerodynamics at Large Mean Incidence. J. Turbomach. 1998, 120, 122–130. [Google Scholar] [CrossRef]
- Yang, H.; He, L. Experimental Study on Linear Compressor Cascade with Three-Dimensional Blade Oscillation. J. Propuls. Power 2004, 20, 180–188. [Google Scholar] [CrossRef]
- Watanabe, T.; Aotsuka, M. Unsteady Aerodynamic Characteristics of Oscillating Cascade With Separation Bubble in High Subsonic Flow. In Volume 4: Turbo Expo 2005; American Society of Mechanical Engineers: New York, NY, USA, 2005; pp. 625–633. [Google Scholar] [CrossRef]
- Vogt, D.; Fransson, T. A Technique for Using Recessed-Mounted Pressure Transducers to Measure Unsteady Pressure. In Proceedings of the 17th Symposium on Measuring Techniques in Transonic and Supersonic Flows in Cascades and Turbo machines, Stockholm, Sweden, 9–10 September 2004. [Google Scholar]
- Lepicovsky, J.; McFarland, E.; Capece, V.; Jett, T.; Senyitko, R. Methodology of Blade Unsteady Pressure Measurement in the NASA Transonic Flutter Cascade; Technical Report No. NASA/TM-2002-211894; NASA Center for Aerospace Information: Hanover, MD, USA, 2002.
- Fransson, T.H.; Jöcker, M.; Bölcs, A.; Ott, P. Viscous and Inviscid Linear/Nonlinear Calculations Versus Quasi-Three-Dimensional Experimental Cascade Data for a New Aeroelastic Turbine Standard Configuration. J. Turbomach. 1999, 121, 717–725. [Google Scholar] [CrossRef]
- Lejon, M.; Grönstedt, T.; Glodic, N.; Petrie-Repar, P.; Genrup, M.; Mann, A. Multidisciplinary Design of a Three Stage High Speed Booster. In Turbo Expo: Power for Land, Sea, and Air. Volume 2B: Turbomachinery; American Society of Mechanical Engineers: New York, NY, USA, 2017; p. V02BT41A037. [Google Scholar] [CrossRef]
- Tian, S.; Petrie-Repar, P.; Glodic, N.; Sun, T. CFD-Aided Design of a Transonic Aeroelastic Compressor Rig. J. Turbomach. 2019, 141, 101003. [Google Scholar] [CrossRef]
- Tavera Guerrero, C.; Glodic, N.; Groth, P. Validation of Steady-State Aerodynamics in a Transonic Linear Cascade at Near Stall Conditions. In Turbo Expo: Power for Land, Sea, and Air. Volume 10A: Turbomachinery—Axial Flow Fan and Compressor Aerodynamics; American Society of Mechanical Engineers: New York, NY, USA, 2022; p. V10AT29A013. [Google Scholar] [CrossRef]
- Tavera Guerrero, C.; Glodic, N.; Mårtensson, H. Steady-state aerodynamics tip gap influence in a transonic linear cascade at near stall. In Proceedings of the International Council of the Aeronautical Sciences (ICAS), Stockholm, Sweden, 4–9 September 2022. [Google Scholar]
- Glodic, N.; Guerrero, C.T.; Salas, M.G. Blade oscillation mechanism for aerodynamic damping measurements at high reduced frequencies. E3S Web Conf. 2022, 345, 03002. [Google Scholar]
- Solomon, O.M., Jr. PSD Computations Using Welch’s Method. [Power Spectral Density (PSD)]; USDOE: Washington, DC, USA, 1991. [CrossRef]
- Ansys. CFX-Solver Solver Theory Guide Section: 2.2.2.4.4; R2; Ansys: Canonsburg, PA, USA, 2021. [Google Scholar]
- Glodic, N.; Tavera Guerrero, C.; Gutierrez, M. Aeroelastic Response of a Transonic Compressor Cascade at High Reduced Fequencies. In Proceedings of the ISUAAAT16, Toledo, Spain, 19–23 September 2022. [Google Scholar]










| VINK OP | [°] | [-] | Re [-] | |
|---|---|---|---|---|
| Peak efficiency | ≈5.6 | 0.81 | 1.29 | |
| Near stall | ≈7.1 | 0.81 | 1.25 |
| Blade | EXP [mm] | CFD [mm] | EXP [mm] | CFD [mm] |
|---|---|---|---|---|
| BM1 | 7.1 | 6.2 | 7.8 | 6.8 |
| B0 | 5.8 | 5.6 | 7.1 | 7.5 |
| BP1 | 7.3 | 6.9 | 8.5 | 8.8 |
| Case | [Hz] | [mm] |
|---|---|---|
| Without flow | 279 | 0.74 |
| With flow | 281 | 0.14 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Published by MDPI on behalf of the EUROTURBO. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Tavera Guerrero, C.A.; Glodic, N.; Gutierrez Salas, M.; Mårtensson, H. Aeroelastic Response in an Oscillating Transonic Compressor Cascade—An Experimental and Numerical Approach. Int. J. Turbomach. Propuls. Power 2025, 10, 7. https://doi.org/10.3390/ijtpp10020007
Tavera Guerrero CA, Glodic N, Gutierrez Salas M, Mårtensson H. Aeroelastic Response in an Oscillating Transonic Compressor Cascade—An Experimental and Numerical Approach. International Journal of Turbomachinery, Propulsion and Power. 2025; 10(2):7. https://doi.org/10.3390/ijtpp10020007
Chicago/Turabian StyleTavera Guerrero, Carlos Alberto, Nenad Glodic, Mauricio Gutierrez Salas, and Hans Mårtensson. 2025. "Aeroelastic Response in an Oscillating Transonic Compressor Cascade—An Experimental and Numerical Approach" International Journal of Turbomachinery, Propulsion and Power 10, no. 2: 7. https://doi.org/10.3390/ijtpp10020007
APA StyleTavera Guerrero, C. A., Glodic, N., Gutierrez Salas, M., & Mårtensson, H. (2025). Aeroelastic Response in an Oscillating Transonic Compressor Cascade—An Experimental and Numerical Approach. International Journal of Turbomachinery, Propulsion and Power, 10(2), 7. https://doi.org/10.3390/ijtpp10020007






