Modeling Sustained Transmission of Wolbachia among Anopheles Mosquitoes: Implications for Malaria Control in Haiti
Abstract
1. Introduction
2. Materials and Methods
2.1. Model Description
2.1.1. Male Adult Mosquitoes ( and )
2.1.2. Female Adult Mosquitoes ( and )
2.1.3. Egg Stages ( and )
2.1.4. Larvae/Pupae Stages ( and )
| Description | Value | Reference | |
|---|---|---|---|
| Specific toAnopheles spp. | |||
| Hatching rate for eggs (=) | 1/3 | [26] | |
| Emergence rate for larvae (=) | 1/18 | [6] | |
| Death rate for uninfected females (=) | 1/13 | [6,26] | |
| Death rate for infected females (=) | 1/15 | [6,26] | |
| Death rate for uninfected males (=) | 1/7 | [6,26] | |
| Death rate for infected males (=) | 1/7 | [6,26] | |
| Death rate for uninfected eggs | 0.12 | [6] | |
| Death rate for infected eggs | 0.33 | [6] | |
| Death rate for larvae | 0.01 | [6] | |
| Per capita egg laying rate for wild females | 3.8 | [6] | |
| Per capita egg laying rate for infected females | 3.3 | [6] | |
| wAlbB maternal transmission fraction | 1 | [7] | |
| wAlbB CI fraction | 1 | ||
| Not specific to Anopheles spp. | |||
| Fraction of larvae emerging as females | 0.5 | [27] | |
| Fraction of larvae emerging as males | 0.5 | [27] | |
| Carrying capacity of larvae/pupae stages | Assume |
2.2. Model Analysis
2.2.1. Next-Generation Numbers
2.2.2. Equilibria and Basic Reproductive Number
Disease-Free Equilibrium (DFE)
Complete-Infection Equilibrium (CIE)
Basic Reproductive Number
Endemic Equilibrium (EE)
2.2.3. Stability and Bifurcation Analysis
2.3. Parameter Estimations
2.3.1. Maternal Transmission
2.3.2. Mosquito Lifespan
2.3.3. Egg-laying Rates
2.3.4. Egg-hatching Rate and Death Rates
2.3.5. Larvae/Pupae Emergence Rate and Death Rate
3. Results
3.1. Sensitivity Analysis
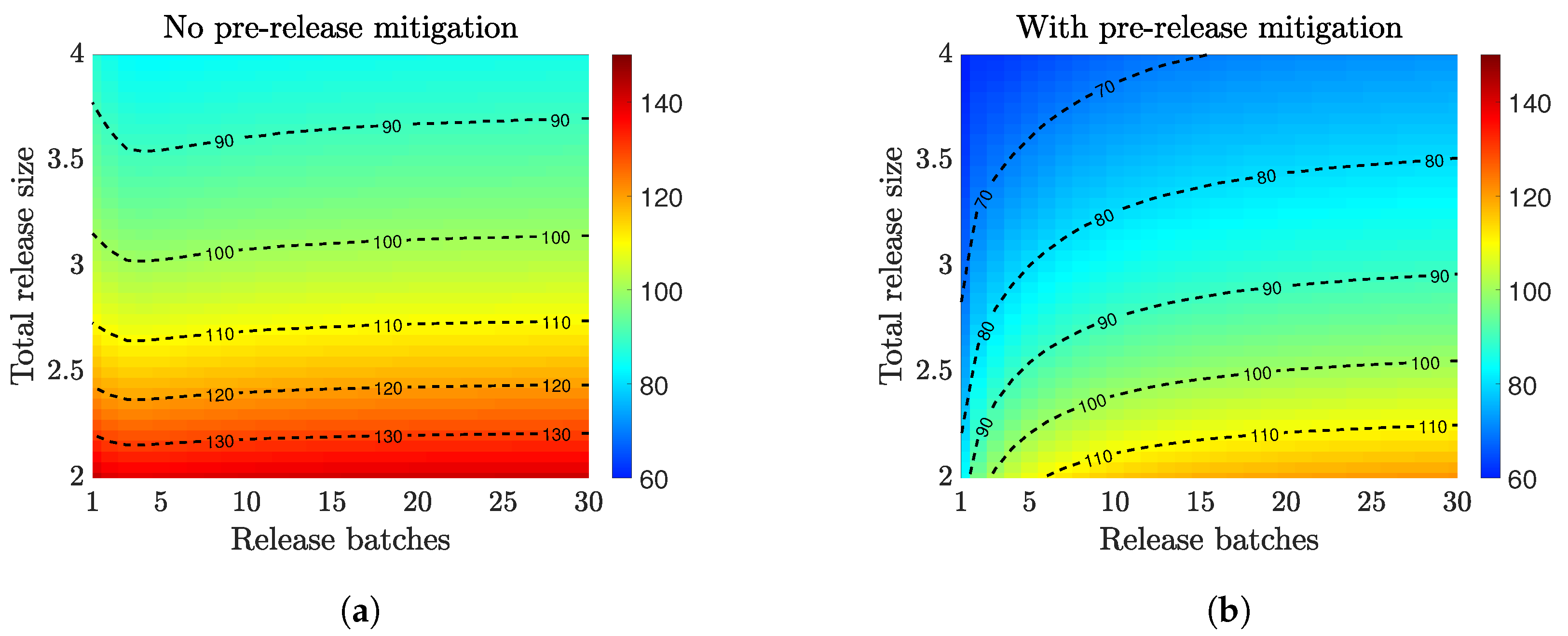
3.2. Compare Prerelease Mitigation Strategies
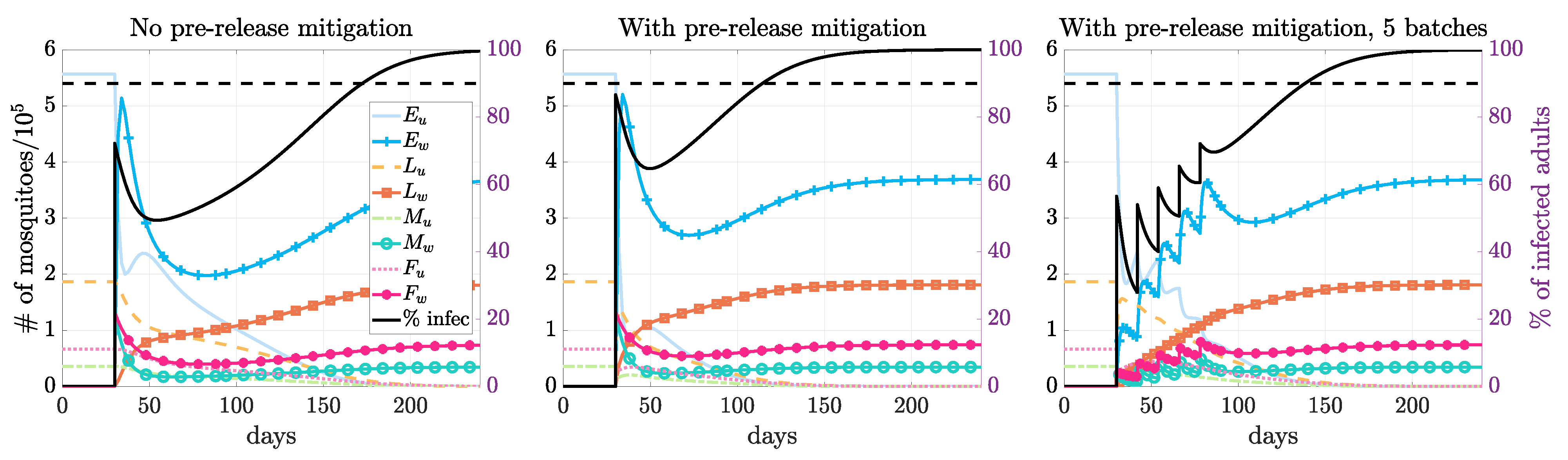
3.3. Multiple Releases
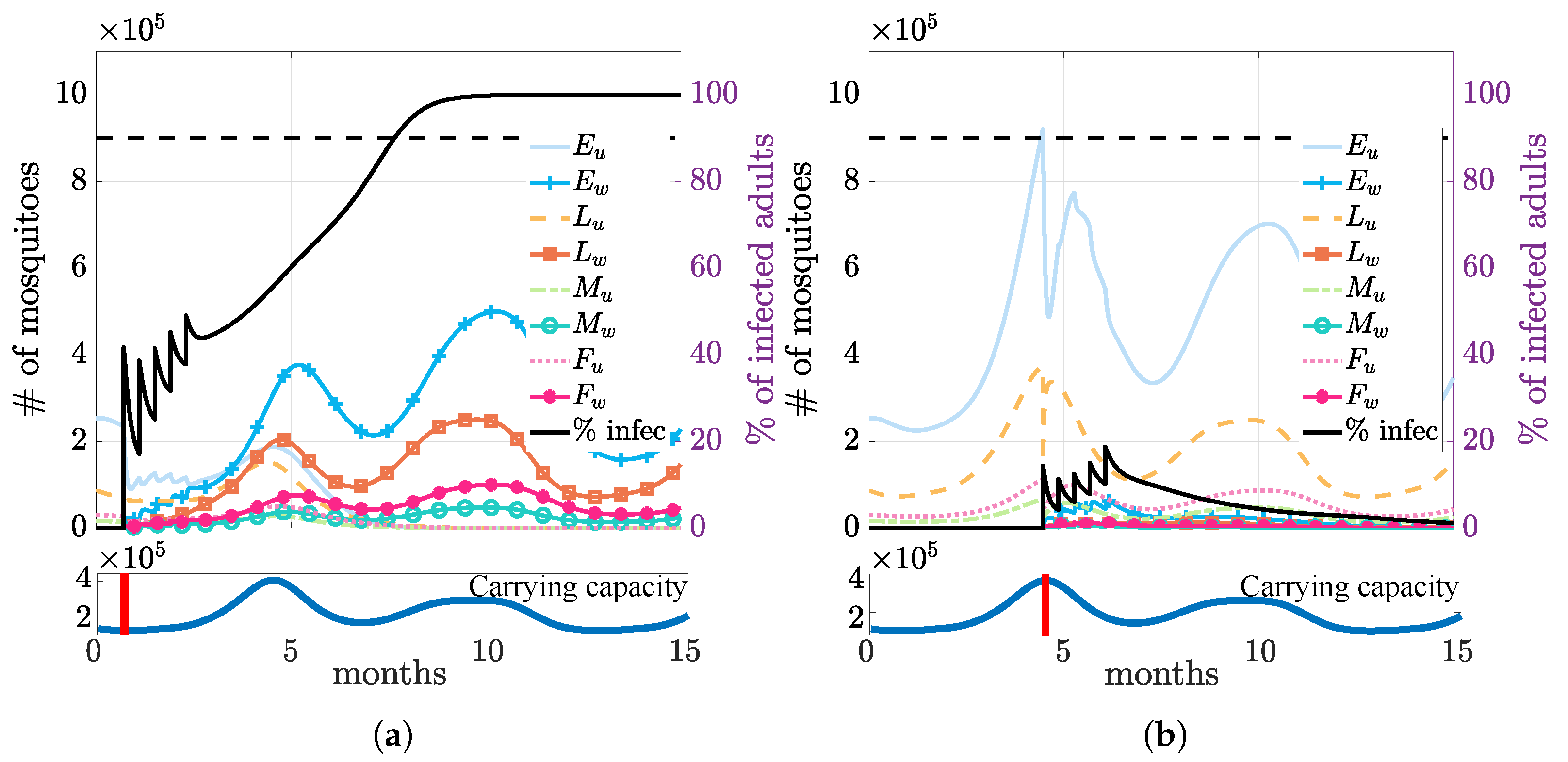
3.4. Seasonality
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Proof of Theorem 1 (Stability of DFE)
Appendix B. Proof of Theorem 2 (Stability of CIE)
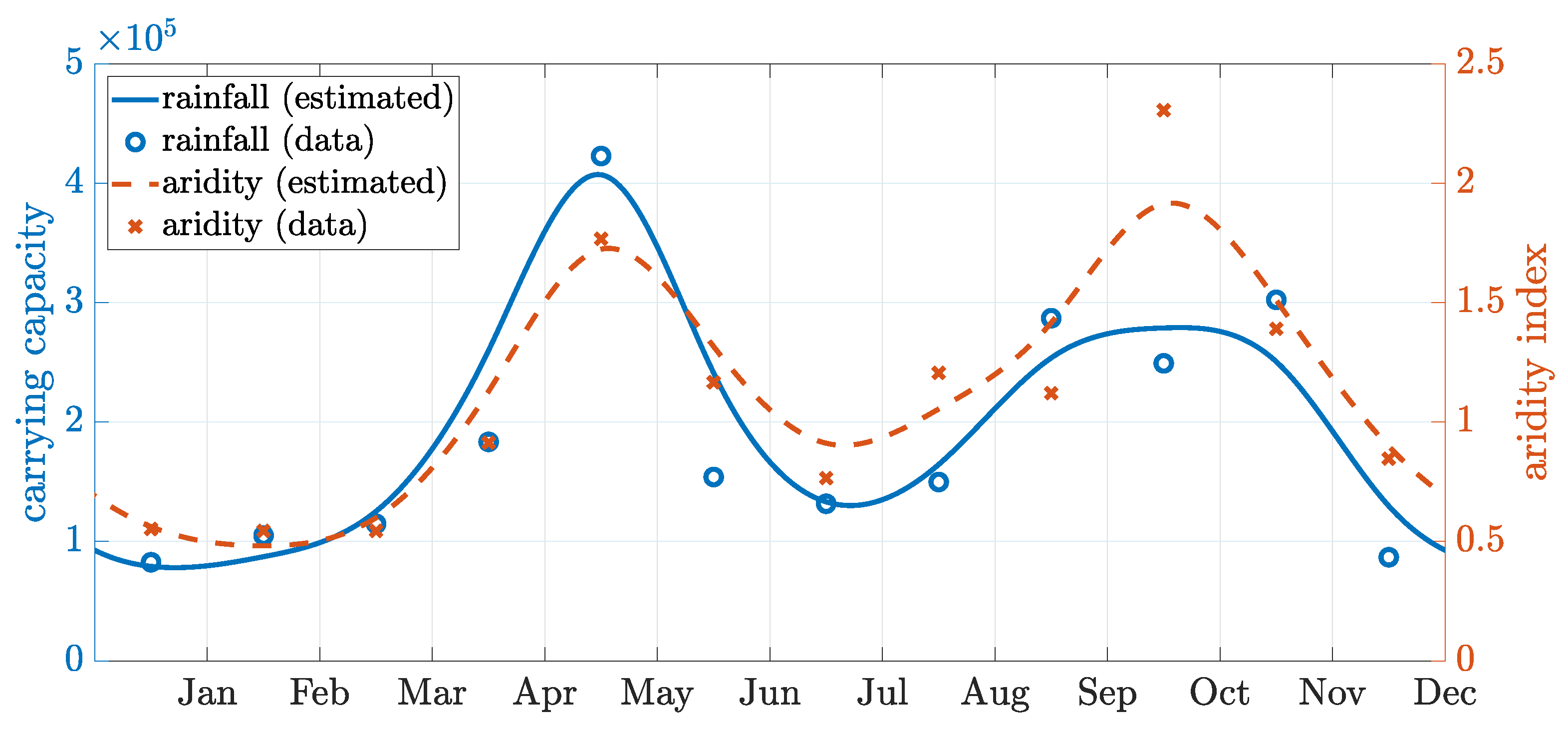
Appendix C. Seasonality Fitting
| Jan | Feb | Mar | Apr | May | Jun | Jul | Aug | Sep | Oct | Nov | Dec | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Rainfall | 59 | 75 | 82 | 131 | 302 | 110 | 94 | 107 | 205 | 178 | 216 | 62 |
| Aridity | 0.6 | 0.5 | 0.5 | 0.9 | 1.8 | 1.2 | 0.8 | 1.2 | 1.1 | 2.3 | 1.4 | 0.8 |
| Temp. | 25.7 | 27.6 | 28.9 | 29.8 | 29.8 | 29.6 | 29.2 | 29.7 | 29.3 | 27.8 | 26.4 | 25.7 |
References
- World Health Organization. Malaria: Fact Sheet; Technical Report; World Health Organization, Regional Office for the Eastern Mediterranean: Cairo, Egypt, 2014.
- Rich, S.M.; Leendertz, F.H.; Xu, G.; LeBreton, M.; Djoko, C.F.; Aminake, M.N.; Takang, E.E.; Diffo, J.L.D.; Pike, B.L.; Rosenthal, B.M.; et al. The Origin of Malignant Malaria. Proc. Natl. Acad. Sci. USA 2009, 106, 14902–14907. [Google Scholar] [CrossRef] [PubMed]
- World Health Organization. Guidelines for Malaria Vector Control; World Health Organization: Geneva, Switzerland, 2019.
- Pan, X.; Thiem, S.; Xi, Z. Wolbachia-Mediated Immunity Induction in Mosquito Vectors. In Arthropod Vector: Controller of Disease Transmission, Volume 1; Elsevier: Amsterdam, The Netherlands, 2017; pp. 35–58. [Google Scholar]
- Mustafa, M.; Rastogi, V.; Gupta, R.; Jain, S.; Singh, P.; Gupta, A. Wolbachia: The selfish Trojan Horse in dengue control. Med. J. Armed Forces India 2016, 72, 373–376. [Google Scholar] [CrossRef]
- Joshi, D.; McFadden, M.J.; Bevins, D.; Zhang, F.; Xi, Z. Wolbachia Strain W AlbB Confers Both Fit. Costs Benefit Anopheles Stephensi. Parasites Vectors 2014, 7, 336. [Google Scholar] [CrossRef] [PubMed]
- Bian, G.; Joshi, D.; Dong, Y.; Lu, P.; Zhou, G.; Pan, X.; Xu, Y.; Dimopoulos, G.; Xi, Z. Wolbachia Invades Anopheles Stephensi Popul. Induces Refract. Plasmodium Infect. Science 2013, 340, 748–751. [Google Scholar]
- Gomes, F.M.; Barillas-Mury, C. Infection of Anopheline mosquitoes with Wolbachia: Implic. Malaria Control. PLoS Pathog. 2018, 14, e1007333. [Google Scholar] [CrossRef]
- Zheng, X.; Zhang, D.; Li, Y.; Yang, C.; Wu, Y.; Liang, X.; Liang, Y.; Pan, X.; Hu, L.; Sun, Q.; et al. Incompatible and Sterile Insect Techniques Combined Eliminate Mosquitoes. Nature 2019, 572, 56–61. [Google Scholar] [CrossRef]
- Zhang, D.; Zheng, X.; Xi, Z.; Bourtzis, K.; Gilles, J.R. Combining the sterile insect technique with the incompatible insect technique: I-impact of Wolbachia infection on the fitness of triple-and double-infected strains of Aedes albopictus. PLoS ONE 2015, 10, e0121126. [Google Scholar] [CrossRef]
- Walker, T.; Johnson, P.H.; Moreira, L.A.; Iturbe-Ormaetxe, I.; Frentiu, F.D.; McMeniman, C.J.; Leong, Y.S.; Dong, Y.; Axford, J.; Kriesner, P.; et al. The wMel Wolbachia Strain Blocks Dengue and Invades Caged Aedes Aegypti Populations. Nature 2011, 476, 450–453. [Google Scholar] [CrossRef]
- Hoffmann, A.A.; Montgomery, B.L.; Popovici, J.; Iturbe-Ormaetxe, I.; Johnson, P.H.; Muzzi, F.; Greenfield, M.; Durkan, M.; Leong, Y.S.; Dong, Y.; et al. Successful Establishment of Wolbachia in Aedes Populations to Suppress Dengue Transmission. Nature 2011, 476, 454–457. [Google Scholar] [CrossRef]
- Joshi, D.; Pan, X.; McFadden, M.J.; Bevins, D.; Liang, X.; Lu, P.; Thiem, S.; Xi, Z. The maternally inheritable Wolbachia wAlbB induces refractoriness to Plasmodium berghei in Anopheles Stephensi. Front. Microbiol. 2017, 8, 366. [Google Scholar] [CrossRef]
- Hoffmann, A.A.; Iturbe-Ormaetxe, I.; Callahan, A.G.; Phillips, B.L.; Billington, K.; Axford, J.K.; Montgomery, B.; Turley, A.P.; O’Neill, S.L. Stability of the wMel Wolbachia Infection Following Invasion into Aedes Aegypti Populations. PLoS Negl. Trop. Dis. 2014, 8, e3115. [Google Scholar] [CrossRef] [PubMed]
- O’Neill, S.L. The Use of Wolbachia by the World Mosquito Program to Interrupt Transmission of Aedes Aegypti Transmitted Viruses. In Dengue and Zika: Control and Antiviral Treatment Strategies; Hilgenfeld, R., Vasudevan, S.G., Eds.; Springer: Singapore, 2018; Volume 1062, pp. 355–360. [Google Scholar] [CrossRef]
- Shaw, W.R.; Marcenac, P.; Childs, L.M.; Buckee, C.O.; Baldini, F.; Sawadogo, S.P.; Dabiré, R.K.; Diabaté, A.; Catteruccia, F. Wolbachia infections in natural Anopheles populations affect egg laying and negatively correlate with Plasmodium development. Nat. Commun. 2016, 7, 11772. [Google Scholar] [CrossRef] [PubMed]
- Childs, L.M.; Hughes, R.; Blackwood, J.C. The role of increased gonotrophic cycles in the establishment of Wolbachia in Anopheles populations. Theor. Ecol. 2020, 13, 349–369. [Google Scholar] [CrossRef]
- Sicard, M.; Bonneau, M.; Weill, M. Wolbachia prevalence, diversity, and ability to induce cytoplasmic incompatibility in mosquitoes. Curr. Opin. Insect Sci. 2019, 34, 12–20. [Google Scholar] [CrossRef]
- Gomes, F.; Hixson, B.; Tyner, M.; Ramirez, J.; Canepa, G.; Silva, T.L.; Molina-Cruz, A.; Kéita, M.; Kane, F.; Traoré, B.; et al. Effect of naturally occurring Wolbachia Anopheles Gamb. S.l. Mosquitoes Mali Plasmodium Falciparum Malar. Transm. Proc. Natl. Acad. Sci. USA 2017, 114, 201716181. [Google Scholar] [CrossRef]
- Adams, K.L.; Abernathy, D.G.; Willett, B.C.; Selland, E.K.; Itoe, M.A.; Catteruccia, F. Wolbachia cifB Induces Cytoplasmic Incompatibility in the Malaria Mosquito Vector. Nat. Microbiol. 2021, 6, 1575–1582. [Google Scholar] [CrossRef] [PubMed]
- Hughes, G.L.; Koga, R.; Xue, P.; Fukatsu, T.; Rasgon, J.L. Wolbachia infections are virulent and inhibit the human malaria parasite Plasmodium falciparum in Anopheles gambiae. PLoS Pathog. 2011, 7, e1002043. [Google Scholar] [CrossRef]
- Xue, L.; Fang, X.; Hyman, J.M. Comparing the effectiveness of different strains of Wolbachia Control. Chikungunya, Dengue Fever, Zika. PLoS Negl. Trop. Dis. 2018, 12, e0006666. [Google Scholar] [CrossRef]
- Qu, Z.; Xue, L.; Hyman, J. Modeling the Transmission of Wolbachia Mosquitoes for Controlling Mosquito-Borne Diseases. SIAM J. Appl. Math. 2018, 78, 826–852. [Google Scholar] [CrossRef]
- Qu, Z.; Hyman, J. Generating a Hierarchy of Reduced Models for a System of Differential Equations Modeling the Spread of Wolbachia in Mosquitoes. SIAM J. Appl. Math. 2019, 79, 1675–1699. [Google Scholar] [CrossRef]
- Xue, L.; Manore, C.; Thongsripong, P.; Hyman, J. Two-sex mosquito model for the persistence of Wolbachia. J. Biol. Dyn. 2015, 11, 216–237. [Google Scholar] [CrossRef] [PubMed]
- Centers for Disease Control and Prevention. Anopheles Mosquitoes. 2022. Available online: https://www.cdc.gov/malaria/about/biology/index.html (accessed on 2 February 2022).
- Tun-Lin, W.; Burkot, T.; Kay, B. Effects of temperature and larval diet on development rates and survival of the Dengue vector Aedes Aegypti North Queensland, Australia. Med Vet. Entomol. 2000, 14, 31–37. [Google Scholar] [CrossRef] [PubMed]
- Derua, Y.A.; Kweka, E.J.; Kisinza, W.N.; Githeko, A.K.; Mosha, F.W. Bacterial larvicides used for malaria vector control in sub-Saharan Africa: Review of their effectiveness and operational feasibility. Parasites Vectors 2019, 12, 426. [Google Scholar] [CrossRef] [PubMed]
- World Health Organization. Space Spray Application of Insecticides for Vector and Public Health Pest Control: A Practitioner’s Guide; Technical Report; World Health Organization: Geneva, Switzerland, 2003.
- Pryce, J.; Choi, L.; Richardson, M.; Malone, D. Insecticide space spraying for preventing malaria transmission. Cochrane Database Syst. Rev. 2018, CD012689. [Google Scholar] [CrossRef] [PubMed]
- Funk, C.; Peterson, P.; Landsfeld, M.; Pedreros, D.; Verdin, J.; Shukla, S.; Husak, G.; Rowland, J.; Harrison, L.; Hoell, A.; et al. The climate hazards infrared precipitation with stations—A new environmental record for monitoring extremes. Sci. Data 2015, 2, 150066. [Google Scholar] [CrossRef]
- Christiansen-Jucht, C.; Parham, P.E.; Saddler, A.; Koella, J.C.; Basáñez, M.G. Temperature during larval development and adult maintenance influences the survival of Anopheles gambiae ss. Parasites Vectors 2014, 7, 489. [Google Scholar] [CrossRef]
- Beck-Johnson, L.M.; Nelson, W.A.; Paaijmans, K.P.; Read, A.F.; Thomas, M.B.; Bjørnstad, O.N. The effect of temperature on Anopheles mosquito population dynamics and the potential for malaria transmission. PLoS ONE 2013, 8, e79276. [Google Scholar] [CrossRef]
- Koiller, J.; Da Silva, M.; Souza, M.; Codeço, C.; Iggidr, A.; Sallet, G. Aedes, Wolbachia and Dengue; Research Report RR-8462; Inria Nancy-Grand Est: Villers-lès-Nancy, France, 2014. [Google Scholar]
- Taghikhani, R.; Sharomi, O.; Gumel, A.B. Dynamics of a Two-Sex Model for the Population Ecology of Dengue Mosquitoes in the Presence of Wolbachia. Math. Biosci. 2020, 328, 108426. [Google Scholar] [CrossRef]
- Gumel, A. Causes of Backward Bifurcations in Some Epidemiological Models. J. Math. Anal. Appl. 2012, 395, 355–365. [Google Scholar] [CrossRef]
- Axford, J.K.; Ross, P.A.; Yeap, H.L.; Callahan, A.G.; Hoffmann, A.A. Fitness of wAlbB Wolbachia Infection in Aedes Aegypti: Parameter Estimates in an Outcrossed Background and Potential for Population Invasion. Am. J. Trop. Med. Hyg. 2016, 94, 507–516. [Google Scholar] [CrossRef]
- Adrien, P.; Jacques Boncy, P.; Frantz Lemoine, J.; Existe, A.; Juin, S.; Amouzou, S.; Thimoté, G.; Liverdieu Andrécy, L.; Marseille, S.; Dély, P.; et al. Malaria Elimination in Haiti: Challenges, Progress and Solutions. Clin. Microbiol. Infect. Dis. 2016, 1. [Google Scholar] [CrossRef]
- Steinhardt, L.C.; St Jean, Y.; Impoinvil, D.; Mace, K.E.; Wiegand, R.; Huber, C.S.; Alexandre, J.S.F.; Frederick, J.; Nkurunziza, E.; Jean, S.; et al. Effectiveness of insecticide-treated bednets in Malaria prevention in Haiti: A case-control study. Lancet Glob. Health 2017, 5, e96–e103. [Google Scholar] [CrossRef] [PubMed]
- Pagendam, D.; Elfekih, S.; Nassar, M.S.; Nelson, S.; Almalik, A.M.; Tawfik, E.A.; Al-Fageeh, M.B.; Hoffmann, A.A. Spatio-Temporal Modelling Informing Wolbachia Replacement Releases in a Low Rainfall Climate. Insects 2022, 13, 949. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, T.L.; Barton, N.H.; Rašić, G.; Turley, A.P.; Montgomery, B.L.; Iturbe-Ormaetxe, I.; Cook, P.E.; Ryan, P.A.; Ritchie, S.A.; Hoffmann, A.A.; et al. Local Introduction and Heterogeneous Spatial Spread of Dengue-Suppressing Wolbachia through an Urban Population of Aedes Aegypti. PLoS Biol. 2017, 15, e2001894. [Google Scholar] [CrossRef]
- Qu, Z.; Wu, T.; Hyman, J.M. Modeling Spatial Waves of Wolbachia Invasion for Controlling Mosquito-Borne Diseases. SIAM J. Appl. Math. 2022, 82, 1903–1929. [Google Scholar] [CrossRef]
- Kamgang, J.C.; Sallet, G. Computation of Threshold Conditions for Epidemiological Models and Global Stability of the Disease-Free Equilibrium (DFE). Math. Biosci. 2008, 213, 1–12. [Google Scholar] [CrossRef] [PubMed]


| Population Replacement | Population Suppression | |
|---|---|---|
| Goal | Replace wild mosquito population with Wolbachia-infected mosquitoes that have significantly lower competence and cannot transmit parasite as efficiently | Introduce male mosquitoes that cannot produce viable offspring, which limits the ability of the mosquito to reproduce and reduces mosquito population [8] |
| Role of CI | Infected females can mate successfully with infected males providing them with an evolutionary advantage over uninfected females | The sperm of the infected male is unable to form viable offspring during the egg fertilization process, and as a result, eggs do not hatch |
| Release | Release infected males and females | Release infected males only |
| Wolbachia Strain | Anopheles Species | Impact on Vector | Impact on P. falciparum | Reference |
|---|---|---|---|---|
| coluzzii | No CI, increases egg laying rate | Reduces sporozoite prevalence | [16,17,18,19] | |
| funestus | No CI | Unknown | [18] | |
| gambiae | No CI | Unknown | [18] | |
| arabiensis | No CI | Unknown | [18] | |
| stephensi | Almost complete CI, reduces egg hatching rate, perfect maternal transmission, no impact on female lifespan | Reduces sporozoite and oocyst levels | [6,7] | |
| gambiae | CI, reduces egg development rate | Unknown | [20] | |
| gambiae | No effect on lifespan | Significantly reduces oocyst level | [21] |
| 1 | 1 | −1 | −1 | 1 | −0.5 | 0.26 | 0.23 | 0 | 0 | 0 | 0 | −2.4 × 10−13 | |
| Threshold | −3.5 | −2.1 | 2.1 | 1.4 | −1.4 | 1 | −0.55 | −0.48 | 0 | 0 | 0 | 0 | 4 × 10−13 |
| Time | −6.7 | −1.1 | 1.1 | 0.88 | −0.6 | 0.51 | −0.32 | −0.32 | −0.6 | −0.69 | 0.6 | −0.14 | 0.14 |
| Prerelease Mitigation | Larvae Mitigation Efficacy | Adults Mitigation Efficacy | Threshold Release Factor | Release Factor to Reach by Two Months |
|---|---|---|---|---|
| No mitigation (DFE) | 0 | 0 | 1.13 | 9.9 |
| Thermal fogging | 0 | 0.2 | 1.03 | 9.2 |
| 0 | 0.4 | 0.93 | 9.2 | |
| 0 | 0.6 | 0.82 | 7.9 | |
| Larviciding | 0.2 | 0 | 1.04 | 8.0 |
| 0.4 | 0 | 0.96 | 6.5 | |
| 0.6 | 0 | 0.88 | 5.3 | |
| Thermal fogging + larviciding | 0.6 | 0.2 | 0.79 | 4.8 |
| 0.6 | 0.4 | 0.69 | 4.4 | |
| 0.6 | 0.6 | 0.60 | 3.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Florez, D.; Young, A.J.; Bernabé, K.J.; Hyman, J.M.; Qu, Z. Modeling Sustained Transmission of Wolbachia among Anopheles Mosquitoes: Implications for Malaria Control in Haiti. Trop. Med. Infect. Dis. 2023, 8, 162. https://doi.org/10.3390/tropicalmed8030162
Florez D, Young AJ, Bernabé KJ, Hyman JM, Qu Z. Modeling Sustained Transmission of Wolbachia among Anopheles Mosquitoes: Implications for Malaria Control in Haiti. Tropical Medicine and Infectious Disease. 2023; 8(3):162. https://doi.org/10.3390/tropicalmed8030162
Chicago/Turabian StyleFlorez, Daniela, Alyssa J. Young, Kerlly J. Bernabé, James M. Hyman, and Zhuolin Qu. 2023. "Modeling Sustained Transmission of Wolbachia among Anopheles Mosquitoes: Implications for Malaria Control in Haiti" Tropical Medicine and Infectious Disease 8, no. 3: 162. https://doi.org/10.3390/tropicalmed8030162
APA StyleFlorez, D., Young, A. J., Bernabé, K. J., Hyman, J. M., & Qu, Z. (2023). Modeling Sustained Transmission of Wolbachia among Anopheles Mosquitoes: Implications for Malaria Control in Haiti. Tropical Medicine and Infectious Disease, 8(3), 162. https://doi.org/10.3390/tropicalmed8030162







