Neutron Stress Measurement of W/Ti Composite in Cryogenic Temperatures Using Time-of-Flight Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Preparation of Fiber Reinforced Material
2.2. In Situ Thermal Stress Measurement
3. Results
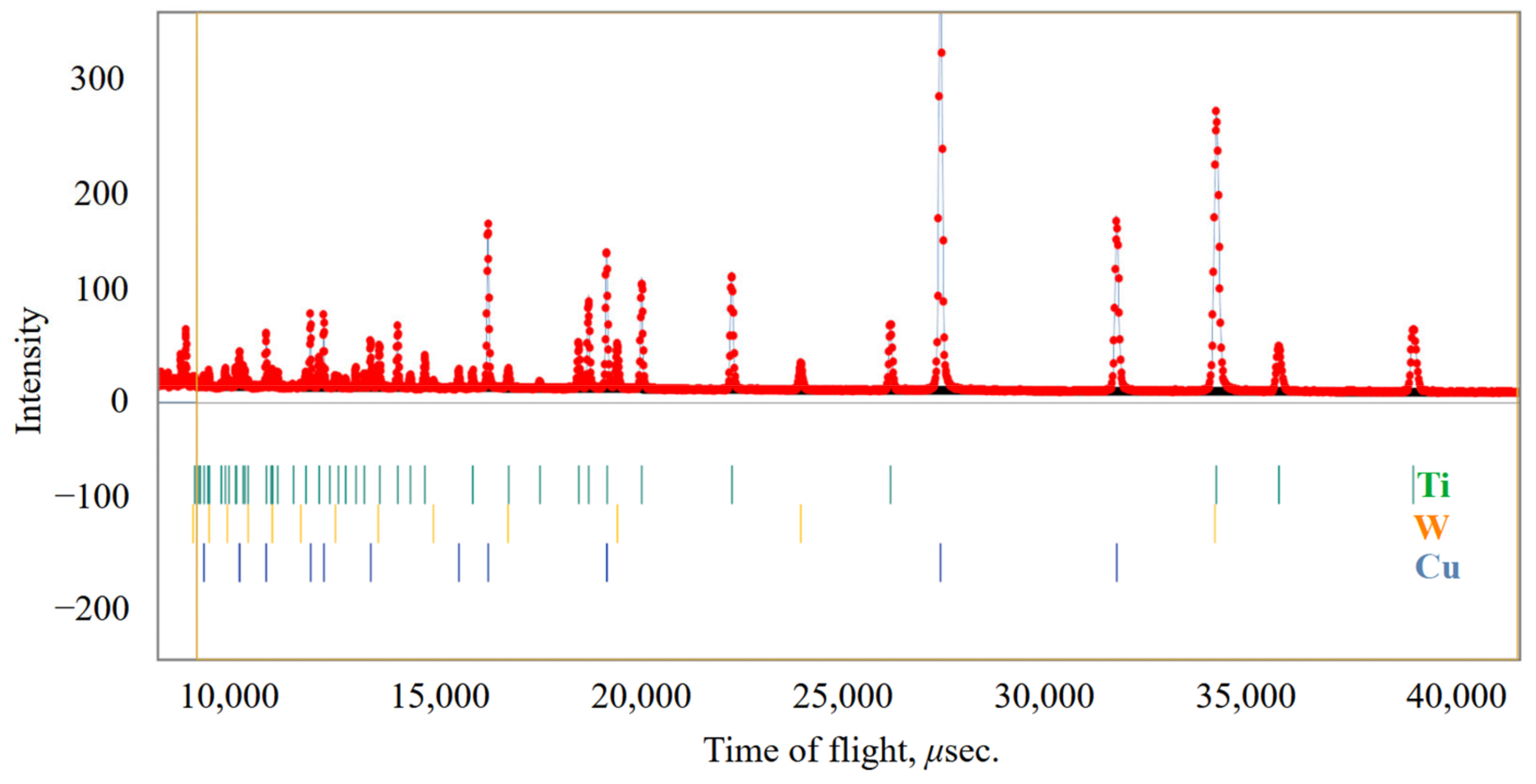
3.1. Diffraction Profile by the Time-of-Flight Method
3.2. Strain Calculation by the TOF Method
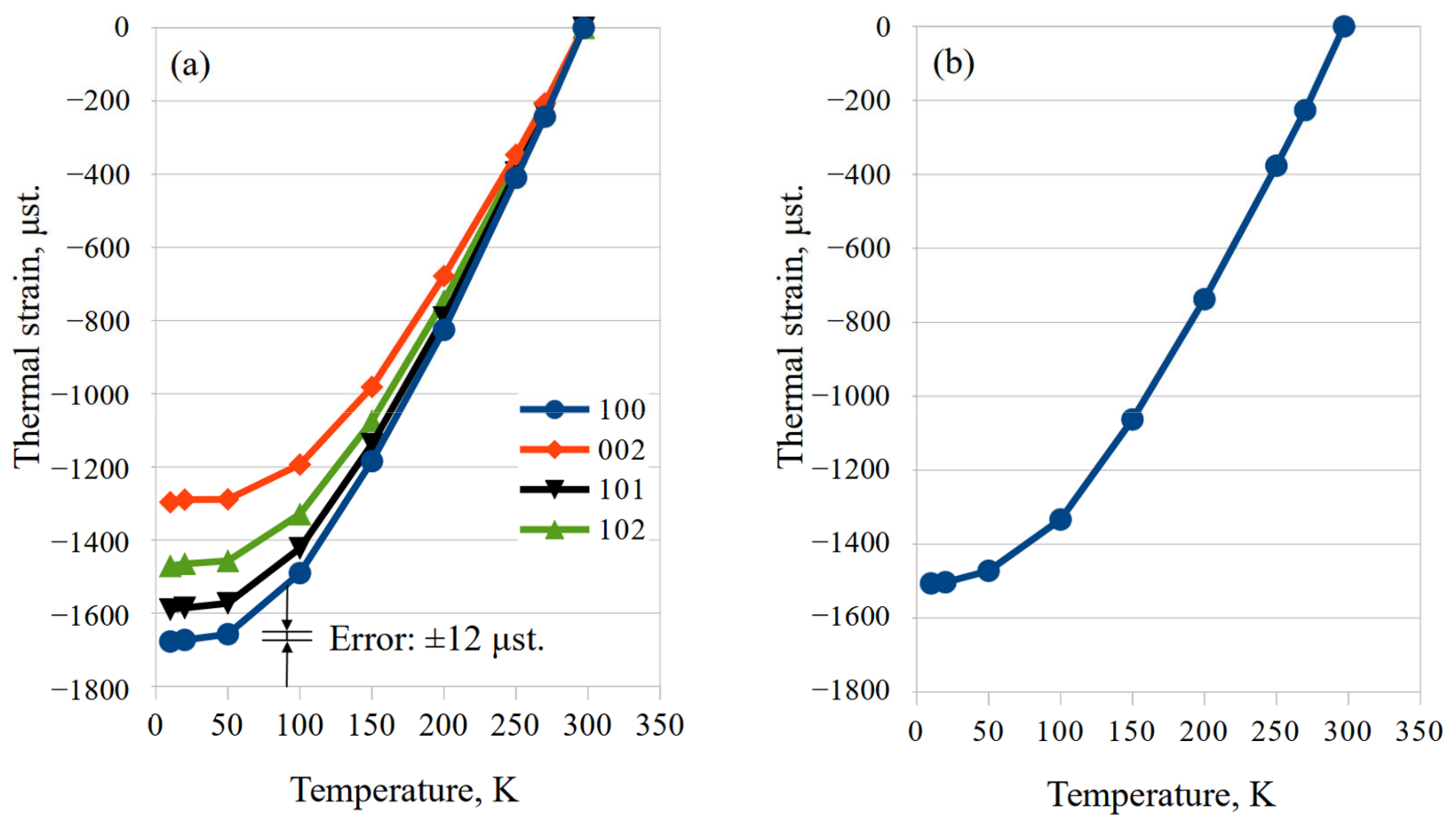
3.3. Results of Thermal Strains
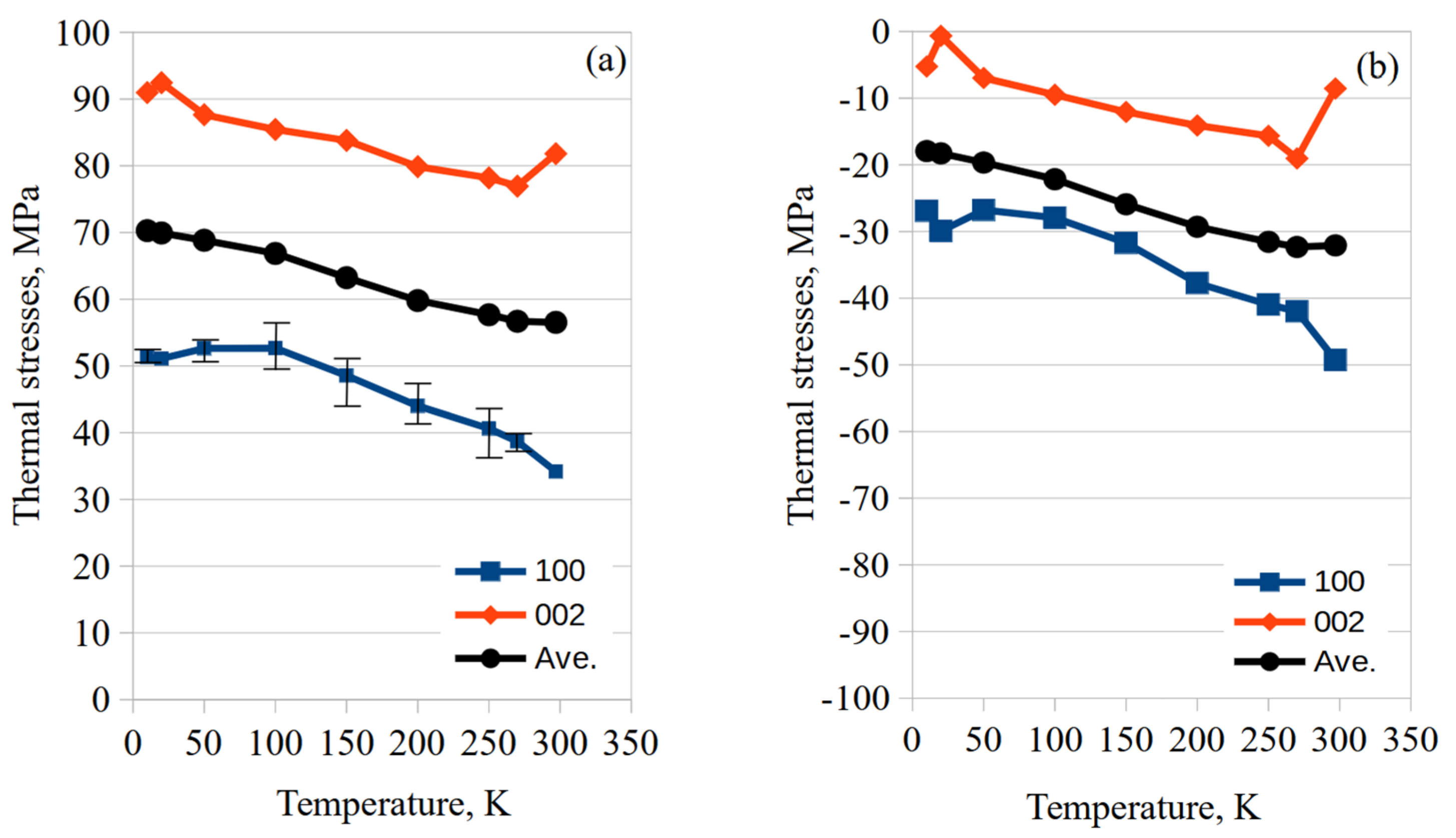
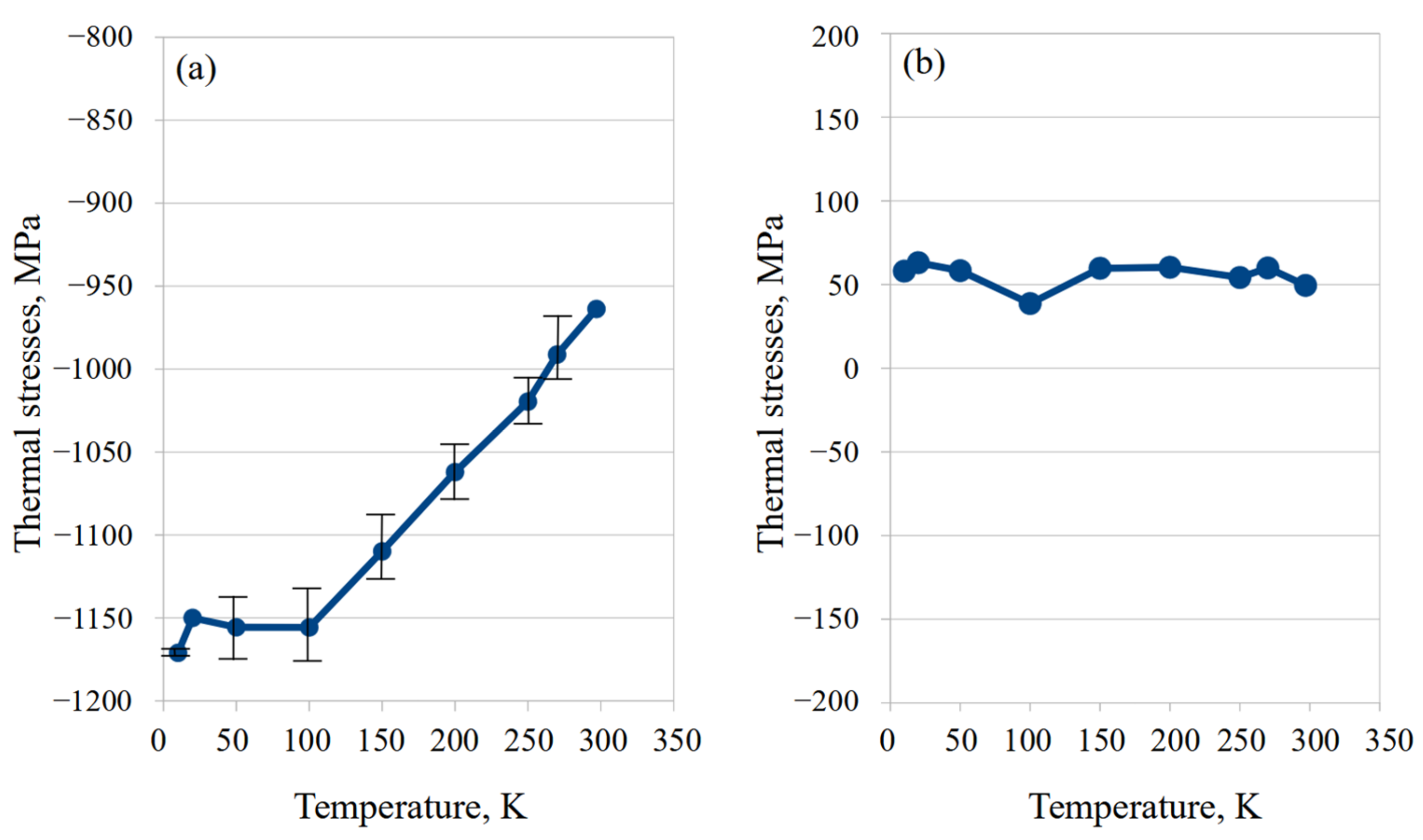
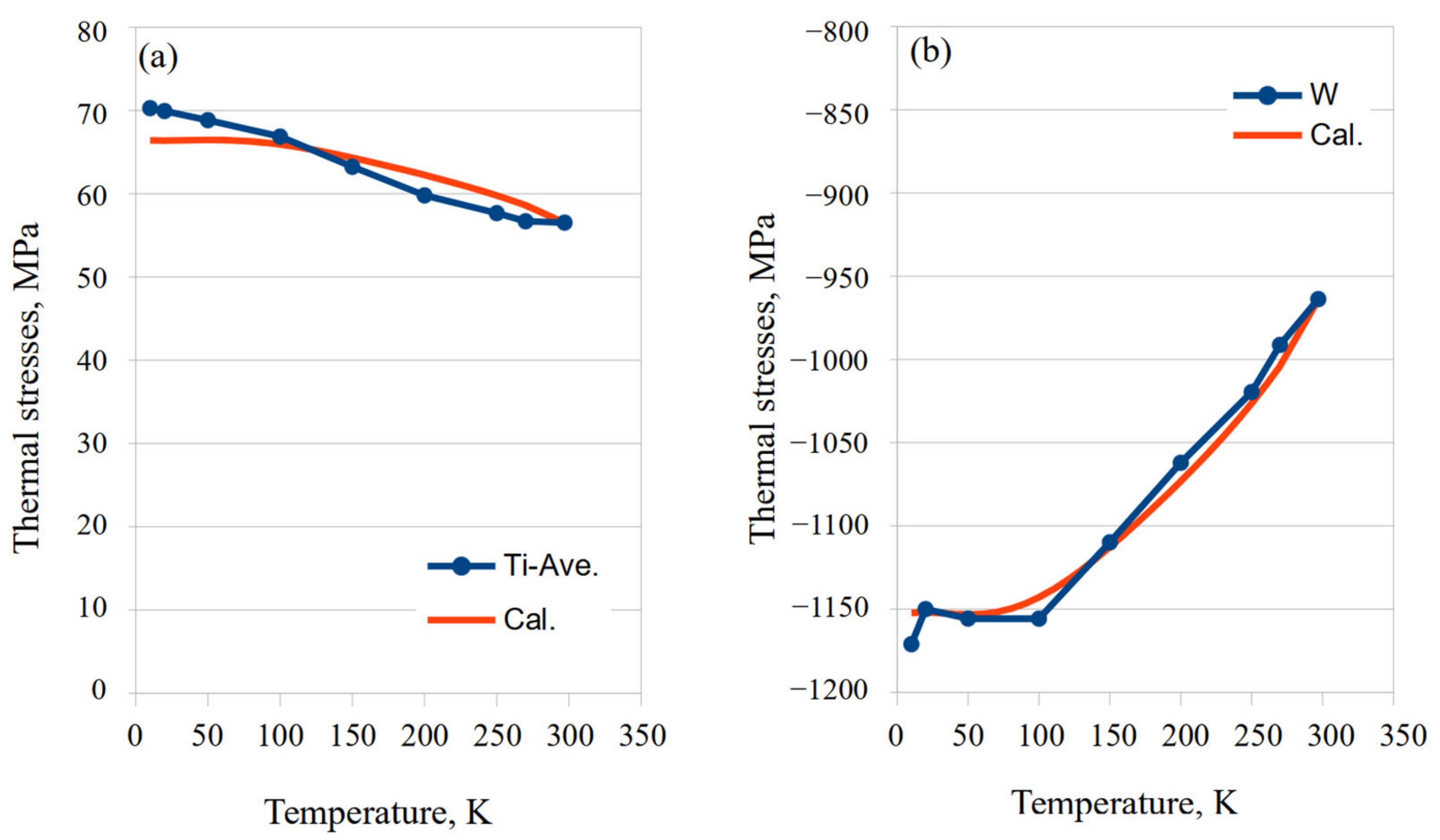
3.4. Results of Thermal Stress Alterations
4. Discussion
5. Conclusions
- (1)
- Regarding the initial residual stress in the fiber longitudinal direction, compressive stresses existed in tungsten fibers, and tensile stresses existed in the titanium matrix.
- (2)
- Thermal residual stresses in tungsten fibers and the titanium matrix were changed to other states depending on temperature changes.
- (3)
- The main factor of thermal stress alterations was the difference in thermal expansion between tungsten fiber and titanium matrix, and the effect in the longitudinal direction of the fibers was dominant.
- (4)
- The alterations in thermal stresses followed the same path during temperature rise and temperature drop in the thermal cycle changes.
- (5)
- The simple elastic calculations and the measured results showed very good agreement, confirming the high measurement accuracy of the time-of-flight method using TAKUMI.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ogasawara, T.; Arai, N.; Fukumoto, R.; Ogawa, T.; Yokozeki, T.; Yoshimura, A. Titanium alloy foil-inserted carbon fiber/epoxy composites for cryogenic propellant tank application. Adv. Compos. Mater. 2013, 23, 129–149. [Google Scholar] [CrossRef]
- El Etri, H.; Korkmaz, M.E.; Gupta, M.K.; Gunay, M.; Xu, J. A state-of-the-art review on mechanical characteristics of different fiber metal laminates for aerospace and structural applications. Int. J. Adv. Manuf. Technol. 2022, 123, 2965–2991. [Google Scholar] [CrossRef]
- Smit, T.C.; Nobre, J.P.; Reid, R.G.; Wu, T.; Niendorf, T.; Marais, D.; Venter, A.M. Assessment and Validation of Incremental Hole-Drilling Calculation Methods for Residual Stress Determination in Fiber-Metal Laminates. Exp. Mech. 2022, 62, 1289–1304. [Google Scholar] [CrossRef]
- Ramesh, K.C.; Sagar, R. Fabrication of Metal Matrix Composite Automotive Parts. Int. J. Adv. Manuf. Technol. 1999, 15, 114–118. [Google Scholar] [CrossRef]
- Okamoto, S.; Taki, M.; Hagihara, T.; Fuyama, N. Material Properties on Metal Matrix Composite Used as Reinforcement for Pistons of Engines in Automobiles. Trans. Jpn. Soc. Mech. Eng. Ser. A 2007, 73, 368–371. [Google Scholar] [CrossRef]
- Asanuma, H.; Kato, K.; Mochizakl, T.; Hakoda, G.; Kurihira, H. Development of active materials based on fiber-reinforced metals. J. Adv. Sci. 2006, 18, 16–19. [Google Scholar] [CrossRef]
- Emamian, S.S.; Awang, M.; Yusof, F.; Sheikholeslam, M.; Mehrpouya, M. Improving the friction stir welding tool life for joining the metal matrix composites. Int. J. Adv. Manuf. Technol. 2020, 106, 3217–3227. [Google Scholar] [CrossRef]
- Hua, M.; Guo, W.; Law, H.W.; Ho, J.K.L. Half-transient liquid phase diffusion welding: An approach for diffusion welding of SiCp/A356 with Cu interlayer. Int. J. Adv. Manuf. Technol. 2007, 37, 504–512. [Google Scholar] [CrossRef]
- Ekin, J.W. Experimental Techniques for Low Temperature Measurements; Oxford University Press: Oxford, UK, 2008; pp. 50–52, 234–235. [Google Scholar]
- Network Database System for Thermophysical Property Data, The National Institute of Advanced Industrial Science and Technology (AIST). Available online: https://tpds.db.aist.go.jp/index_en.html (accessed on 5 January 2023).
- Nishida, M.; Haneoka, M.; Matsue, T.; Jing, T.; Hanabusa, T. Thermal Stress Estimation of Tungsten Fiber Reinforced Titanium Composite by In-situ X-ray Diffraction Method. Mat. Sci. Forum 2014, 768–769, 335–342. [Google Scholar]
- Ikeuchi, Y.; Matsue, T.; Hanabusa, T. X-ray Triaxial Evaluation of Thermal Residual Stresses in Continuous Alumina Fiber Reinforced Aluminum. In Proceedings of the 5th International Conference on Residual Stresses, Linköping, Sweden, 16–18 June 1997; Volume 2, pp. 958–963. [Google Scholar]
- Ikeuchi, Y.; Iga, K.; Hanabusa, T. X-ray Triaxial Evaluation of Thermal Residual Stress in Continuous Alumina Fiber Reinforced Aluminum Composite. J. Soc. Mat. Sci. Jpn. 1994, 43, 792–798. (In Japanese) [Google Scholar] [CrossRef]
- Nishida, M.; Muslih, M.R.; Ikeuchi, Y.; Minakawa, N.; Hanabusa, T. Low temperature in-situ stress measurement of w/cu composite by neutron diffraction. Int. J. Mod. Phys. B 2006, 20, 3668–3673. [Google Scholar] [CrossRef]
- Masayuki, N.; Muslih, M.; Ikeuchi, Y.; Minakawa, N.; Takao, H. Internal Stress Measurement of Fiber Reinforced Composite by Neutron Diffraction with In Situ Low Temperature Stress Measurement System. Mater. Sci. Forum 2005, 490–491, 239–244. [Google Scholar] [CrossRef]
- Clausen, B.; Brown, D.W.; Noyan, I.C. Engineering Applications of Time-of-Flight Neutron Diffraction. JOM 2012, 64, 117–126. [Google Scholar] [CrossRef]
- X-ray Elastic Constants by Krouner Model by The Committee on X-ray Study on Mechanical Behavior of Materials, Japan. Available online: https://x-ray.jsms.jp/x_database/index.html (accessed on 5 January 2023).
- Oishi, R.; Yonemura, M.; Nishimaki, Y.; Torii, S.; Hoshikawa, A.; Ishigaki, T.; Morishima, T.; Mori, K.; Kamiyama, T. Rietveld analysis software for J-PARC. Nucl. Instrum. Methods Phys. Res. Sect. A: Accel. Spectrometers Detect. Assoc. Equip. 2008, 600, 94–96. [Google Scholar] [CrossRef]
- Harjo, S.; Moriai, A.; Torii, S.; Suzuki, H.; Suzuya, K.; Morii, Y.; Arai, M.; Tomota, Y.; Akita, K.; Akiniwa, Y. Design of Engineering Diffractometer at J-PARC. Materi. Sci. Forum 2006, 524, 199–204. [Google Scholar] [CrossRef]
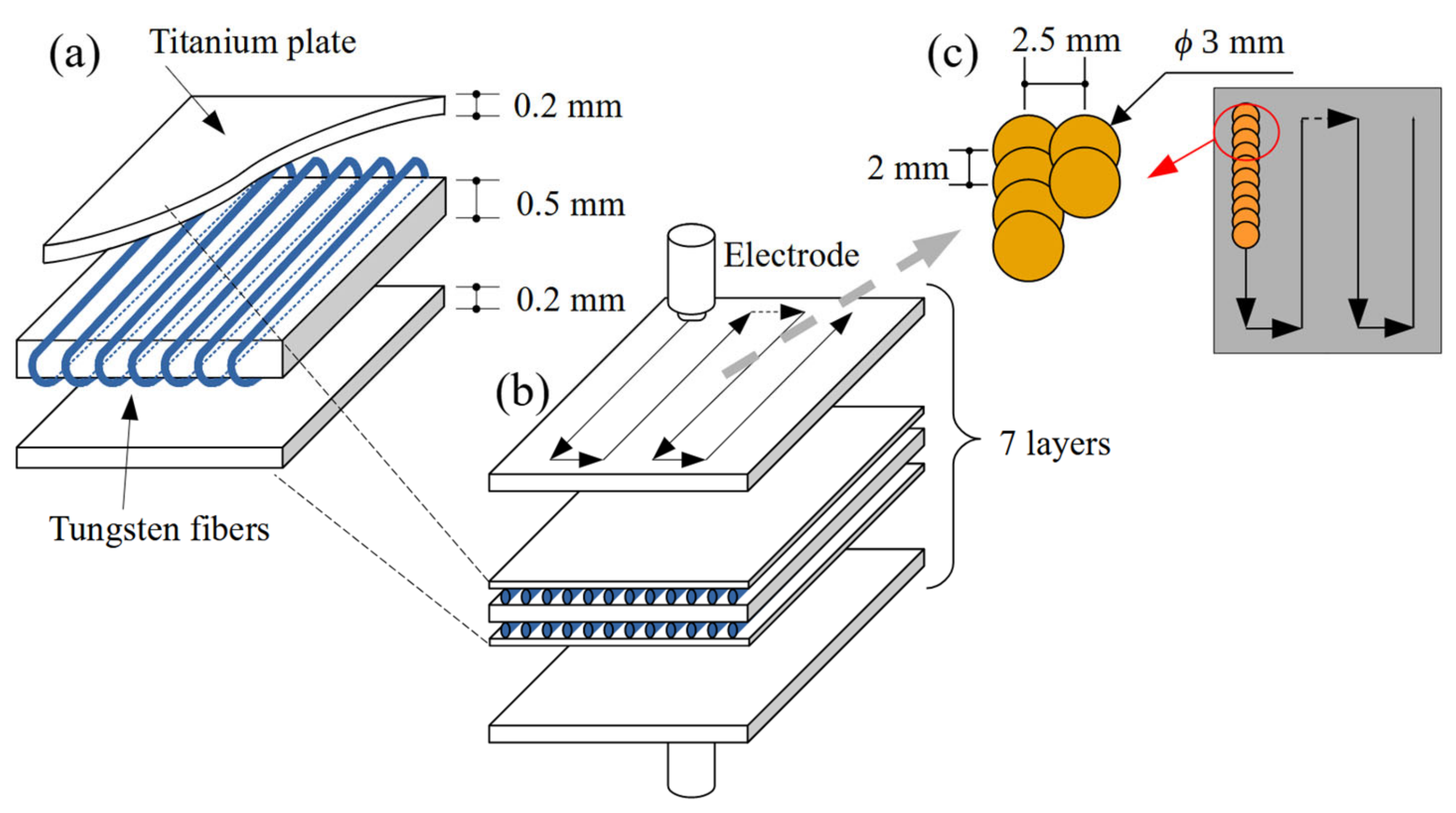
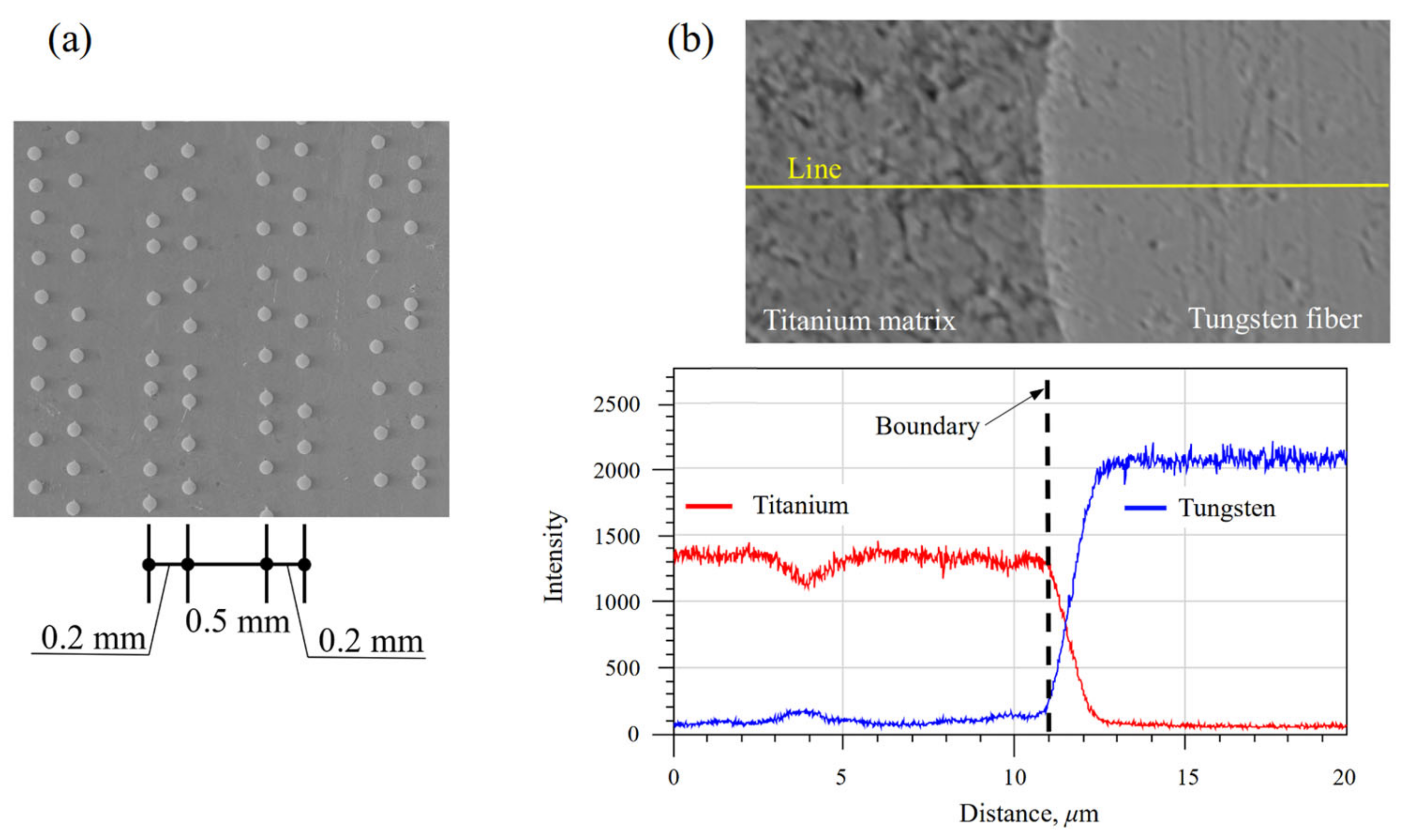
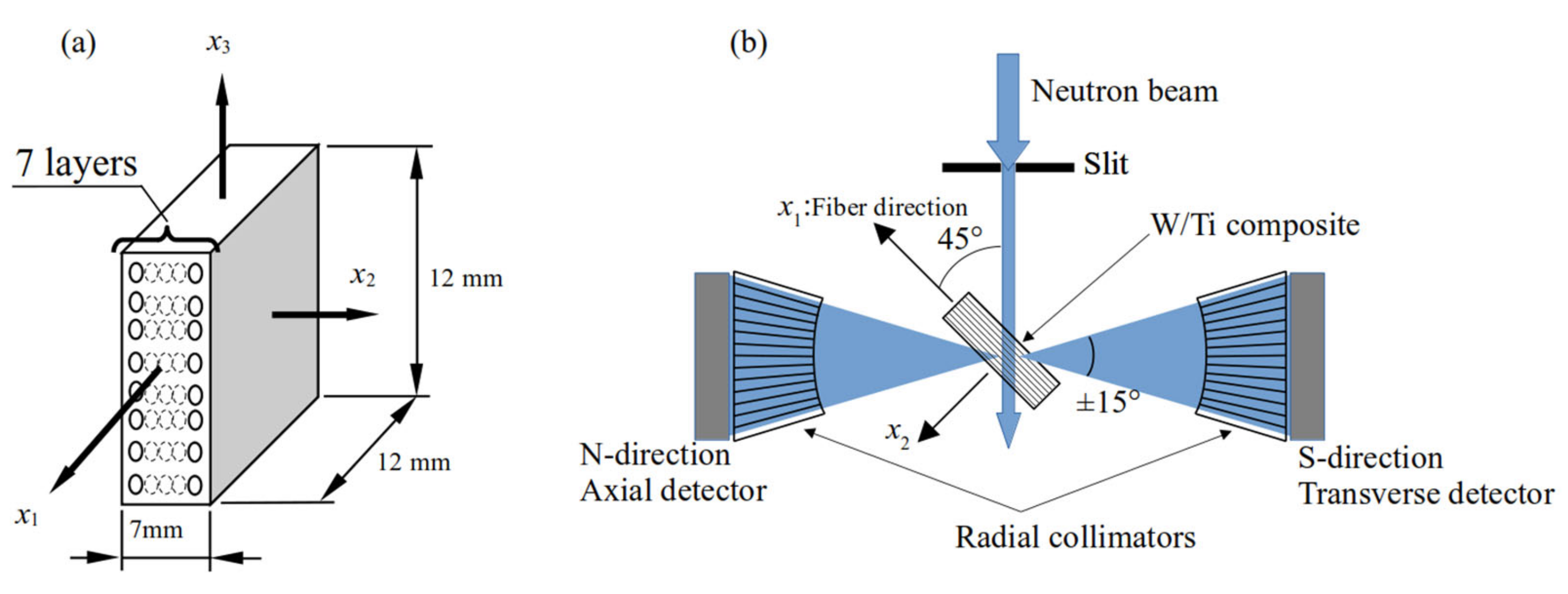
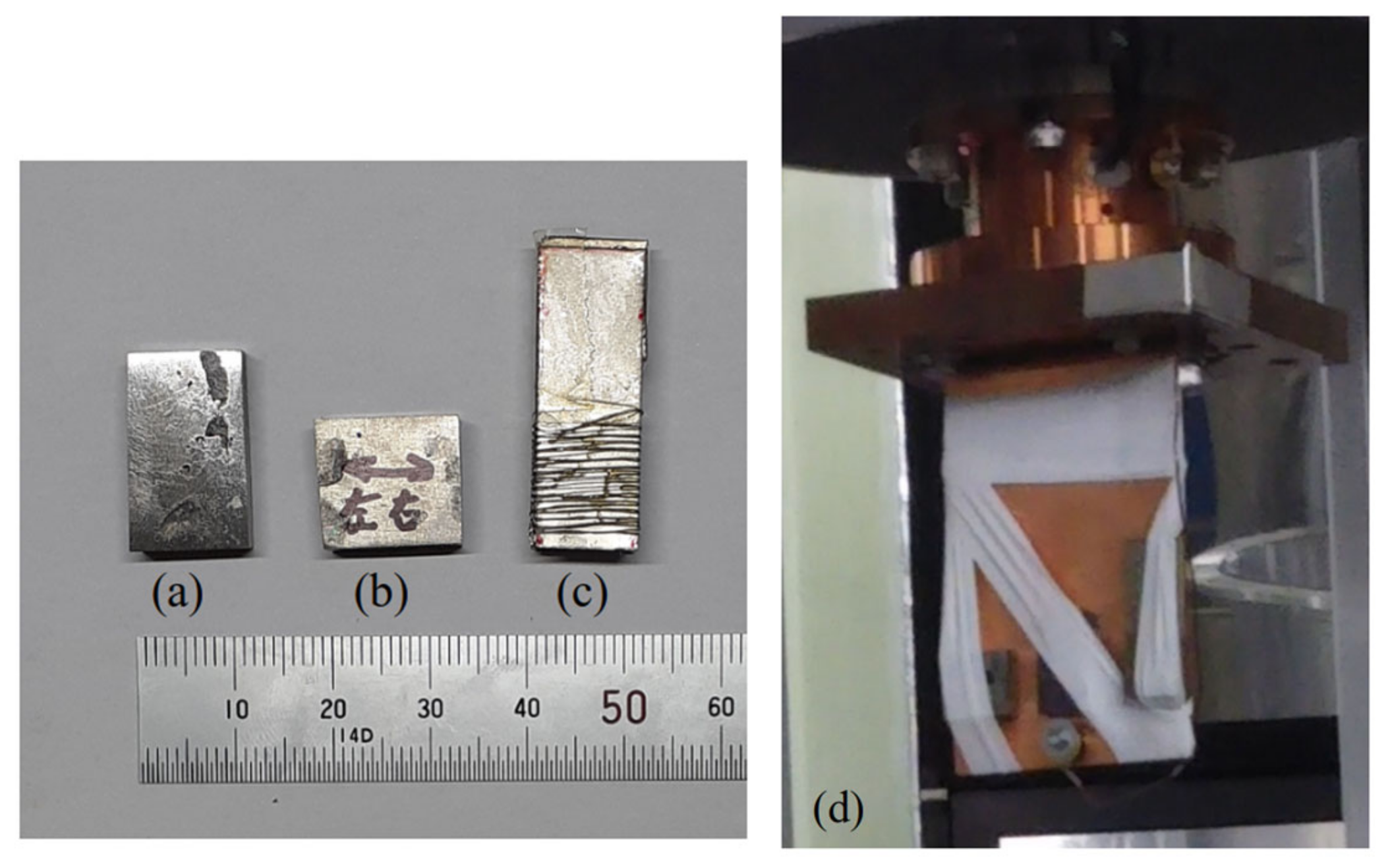


| Welding voltage (V) & current (A) | 200, 8.5 |
| Welding pressure (kN) | 1.9 |
| Holding time (msec.) | 200 |
| Diameter of electrode (mm) | 11 |
| MLF beam power | 600 kW | ||
| Measurement material | W/Ti composite | ||
| Slit system | Incident slit: 5 × 10 mm Detected 90° A, B banks | ||
| Measurement method | Time of Flight (TOF) | ||
| Measurement time | 600 sec./profile | ||
| Tungsten: | |||
| E: 388.69, ν: 0.2833 | |||
| Young’s modulus E (GPa) Poisson’s ratio ν | Titanium: | ||
| hkl, | E, | ν | |
| 100, | 110.86, | 0.3290 | |
| 002, | 128.35, | 0.2976 | |
| 101, | 123.41, | 0.3061 | |
| 102, | 126.83, | 0.3002 | |
| Macro, | 114.70, | 0.3217 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nishida, M.; Harjo, S.; Kawasaki, T.; Yamashita, T.; Gong, W. Neutron Stress Measurement of W/Ti Composite in Cryogenic Temperatures Using Time-of-Flight Method. Quantum Beam Sci. 2023, 7, 8. https://doi.org/10.3390/qubs7010008
Nishida M, Harjo S, Kawasaki T, Yamashita T, Gong W. Neutron Stress Measurement of W/Ti Composite in Cryogenic Temperatures Using Time-of-Flight Method. Quantum Beam Science. 2023; 7(1):8. https://doi.org/10.3390/qubs7010008
Chicago/Turabian StyleNishida, Masayuki, Stefanus Harjo, Takuro Kawasaki, Takayuki Yamashita, and Wu Gong. 2023. "Neutron Stress Measurement of W/Ti Composite in Cryogenic Temperatures Using Time-of-Flight Method" Quantum Beam Science 7, no. 1: 8. https://doi.org/10.3390/qubs7010008
APA StyleNishida, M., Harjo, S., Kawasaki, T., Yamashita, T., & Gong, W. (2023). Neutron Stress Measurement of W/Ti Composite in Cryogenic Temperatures Using Time-of-Flight Method. Quantum Beam Science, 7(1), 8. https://doi.org/10.3390/qubs7010008






