Ovine Bone Morphology and Deformation Analysis Using Synchrotron X-ray Imaging and Scattering
Abstract
1. Introduction
- (a)
- Macrostructure (cortical and trabecular bone tissues, of extent of many mm);
- (b)
- Meso-structure (osteons, 500–1 mm);
- (c)
- Microstructure (Haversian canals, 10–500 μm);
- (d)
- Sub-microstructure (lamellae, 1–10 μm);
- (e)
- Nanostructure (collagen fibrils, less than 1 μm).
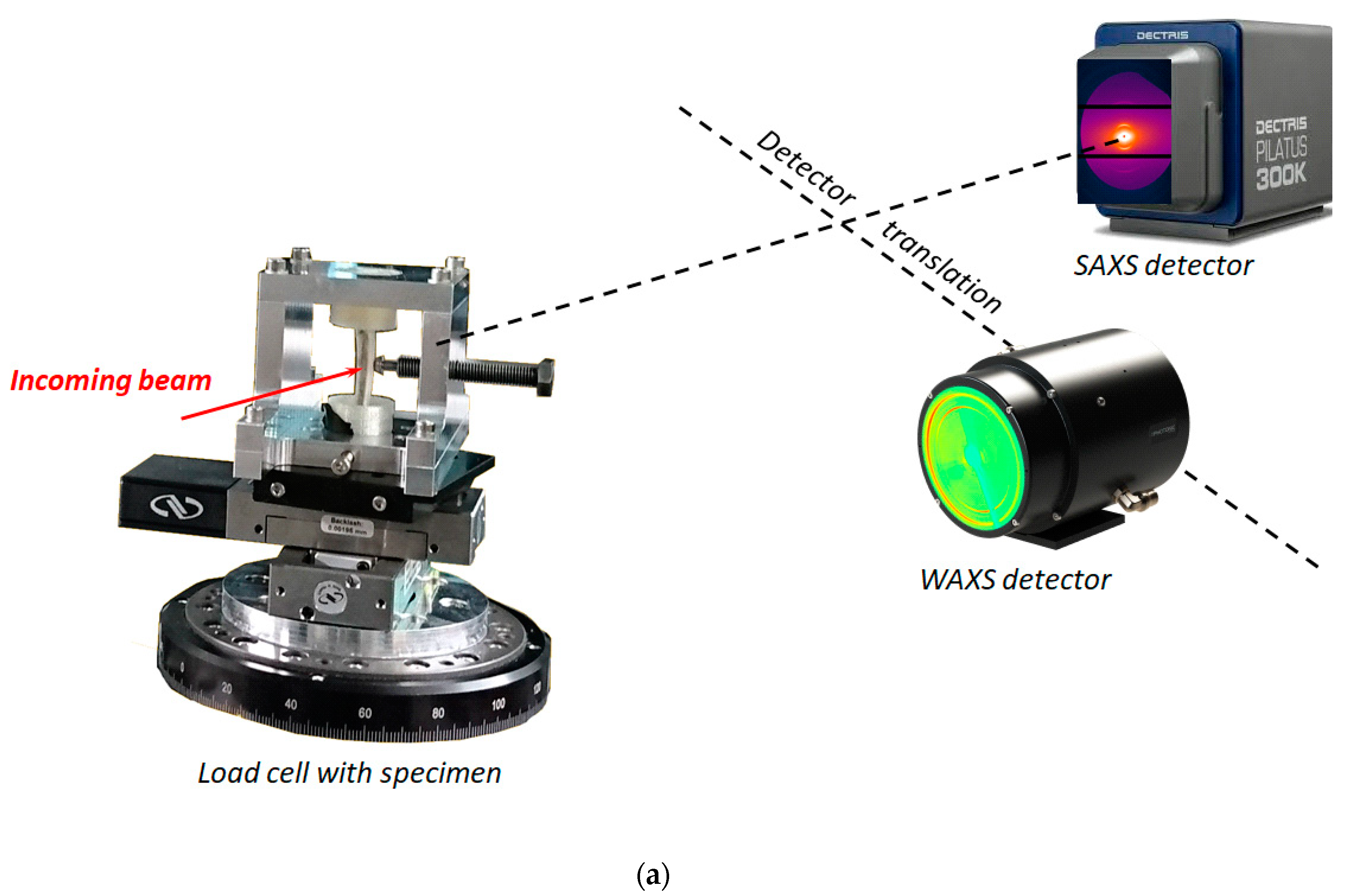
2. Materials and Methods
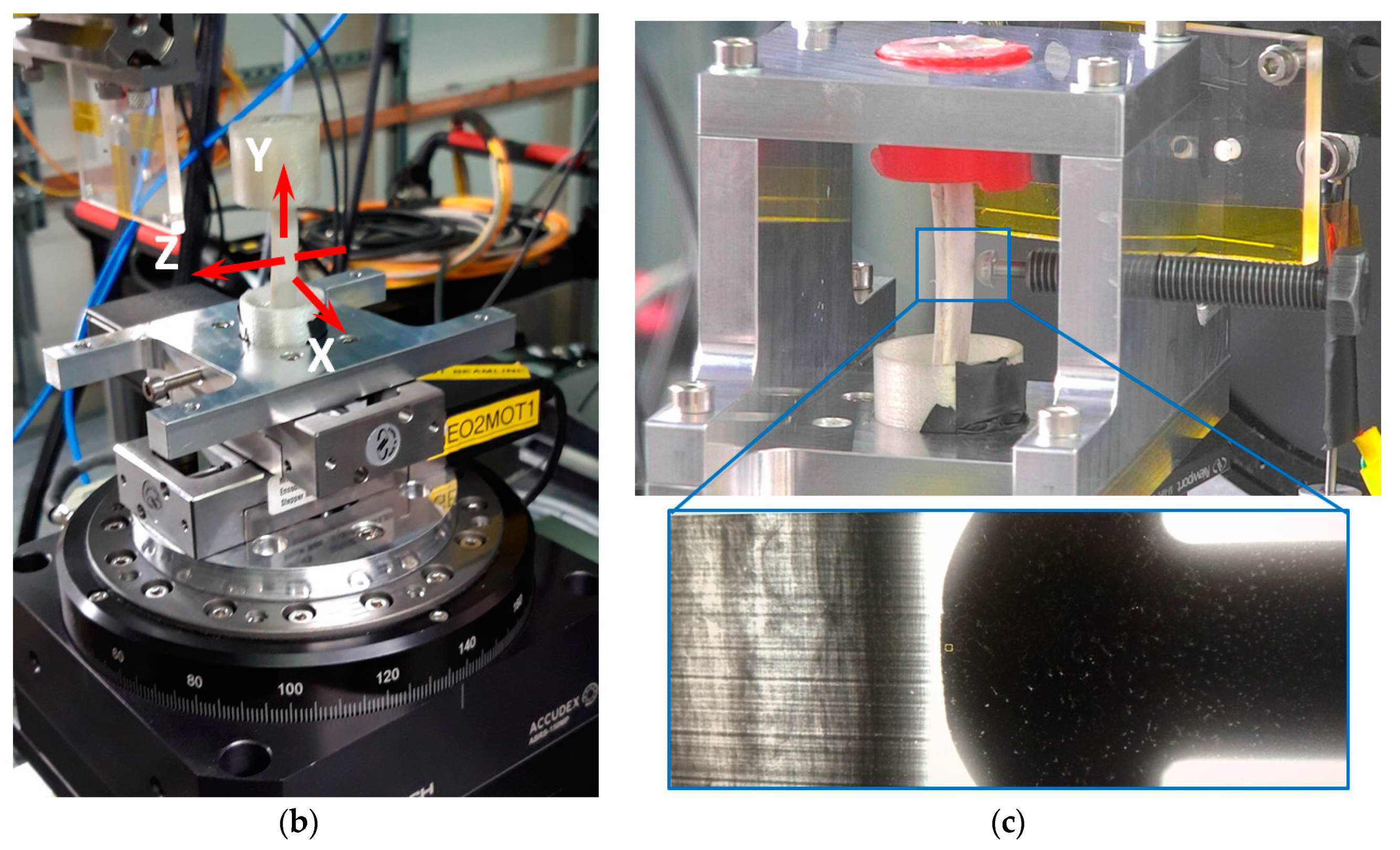
2.1. Sample Preparation
2.2. Synchrotron X-ray Multiple Projection Imaging for Tomography Analysis
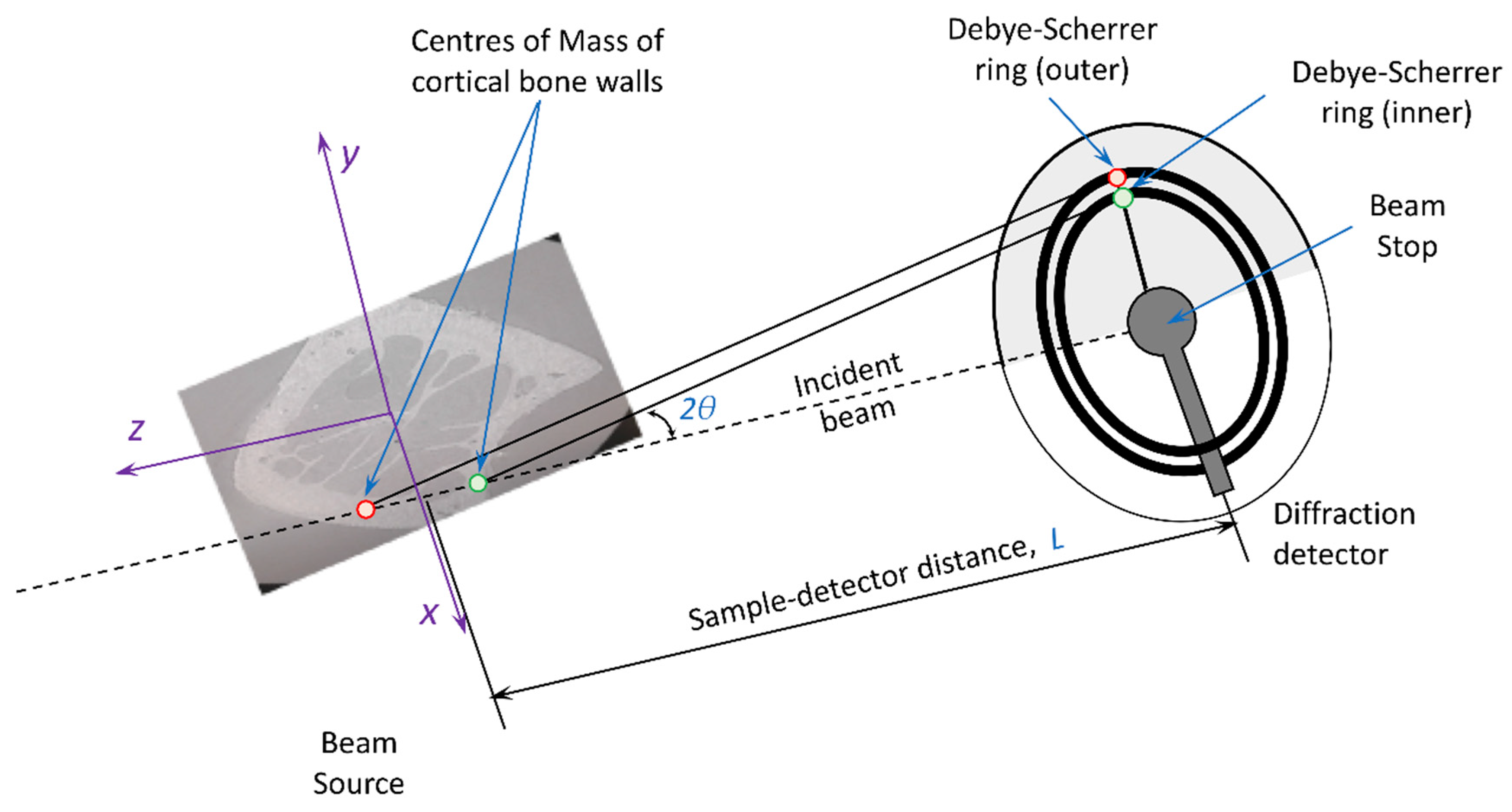
2.3. Synchrotron X-ray Scattering
2.4. Internal Strain Evaluation
3. Results
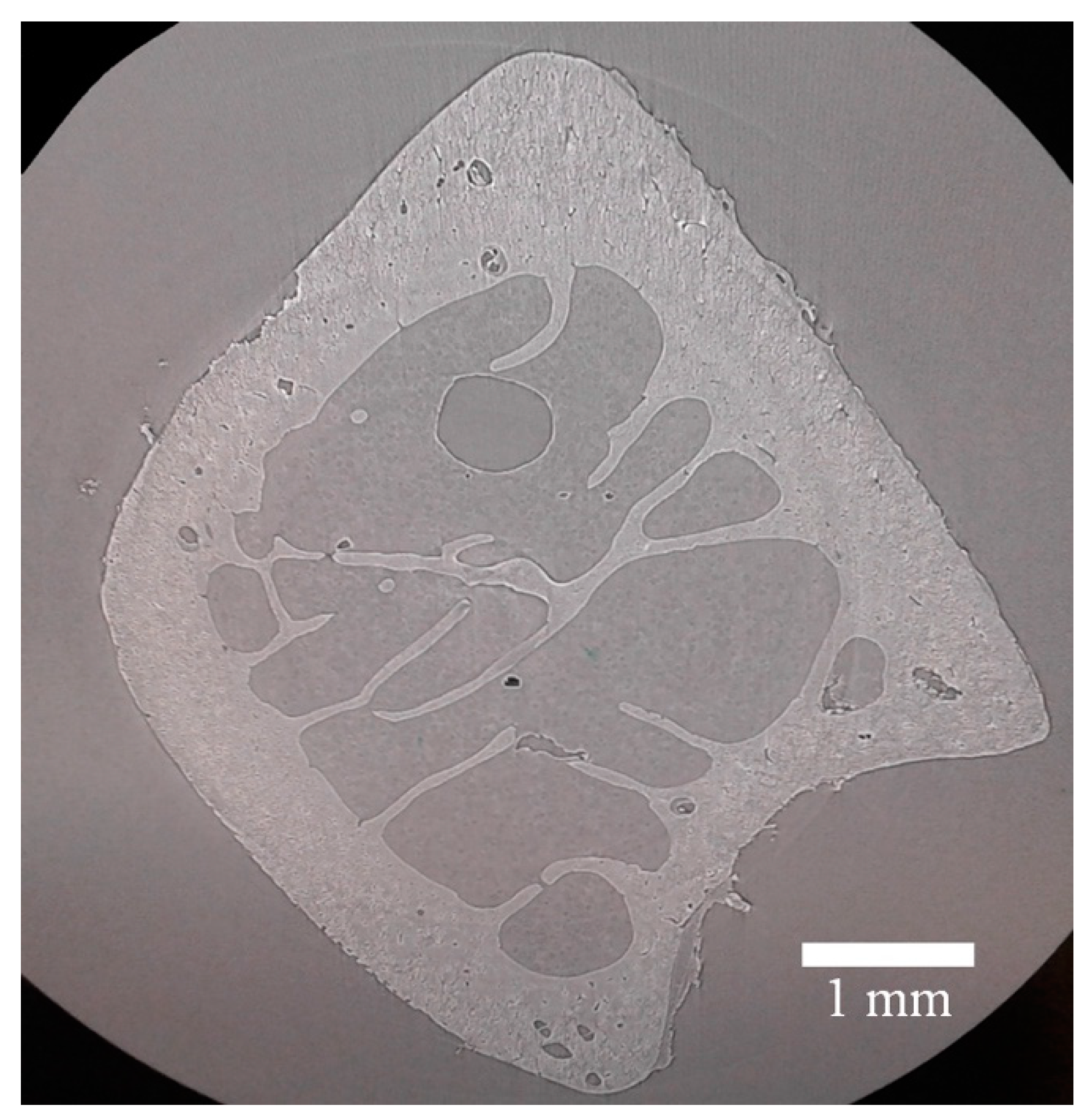
3.1. Tomography, Reconstruction and Segmentation
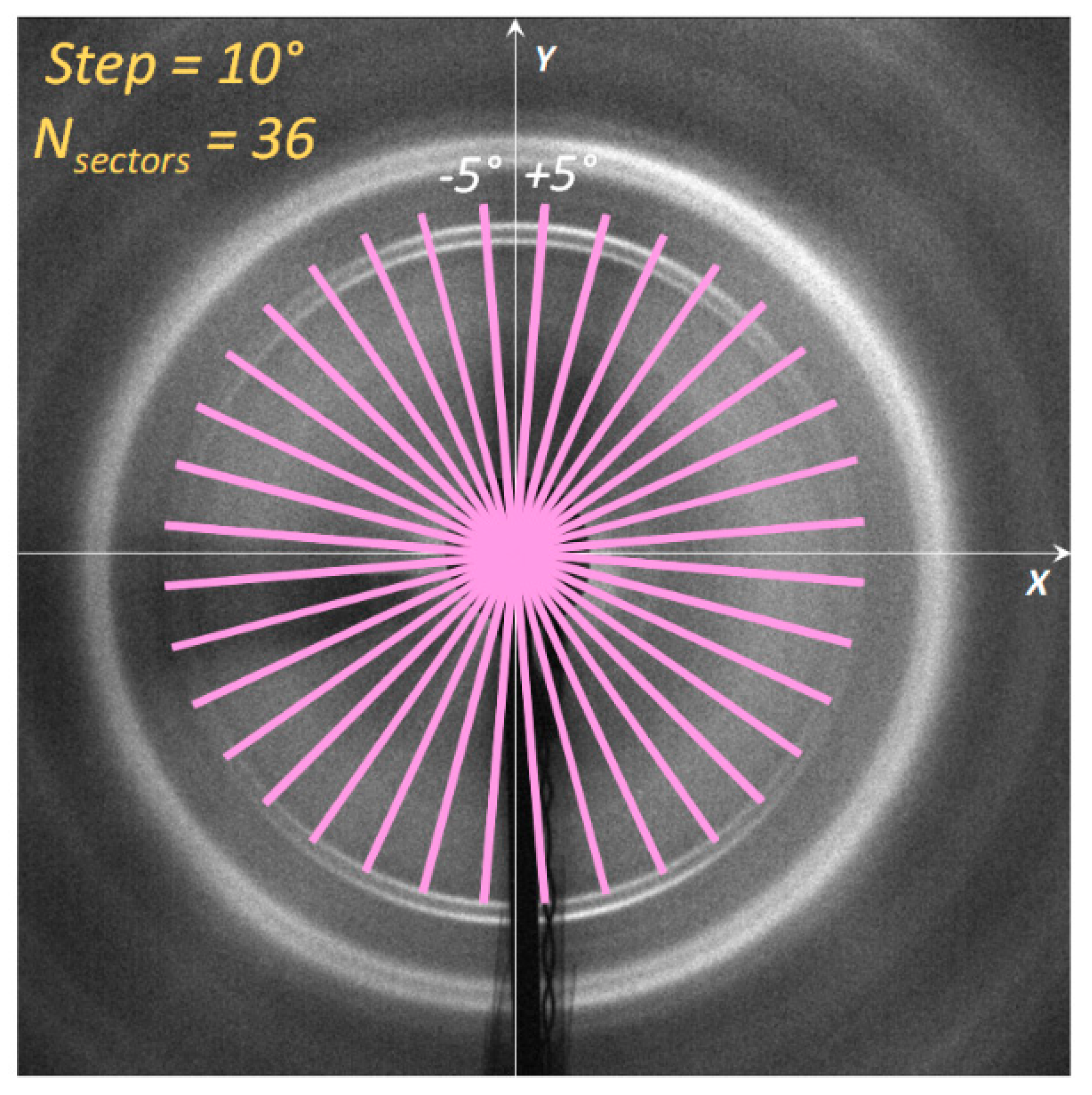
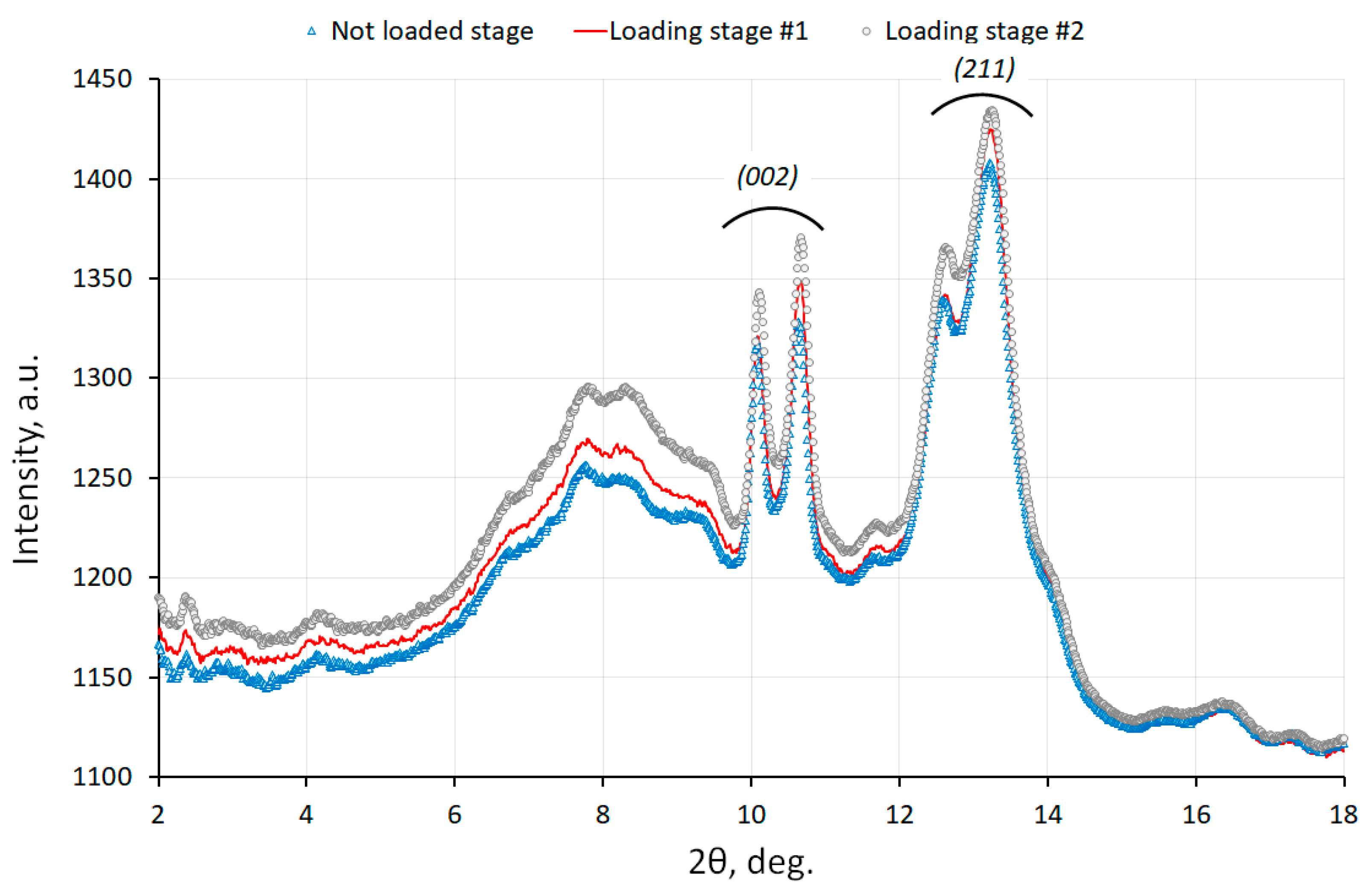
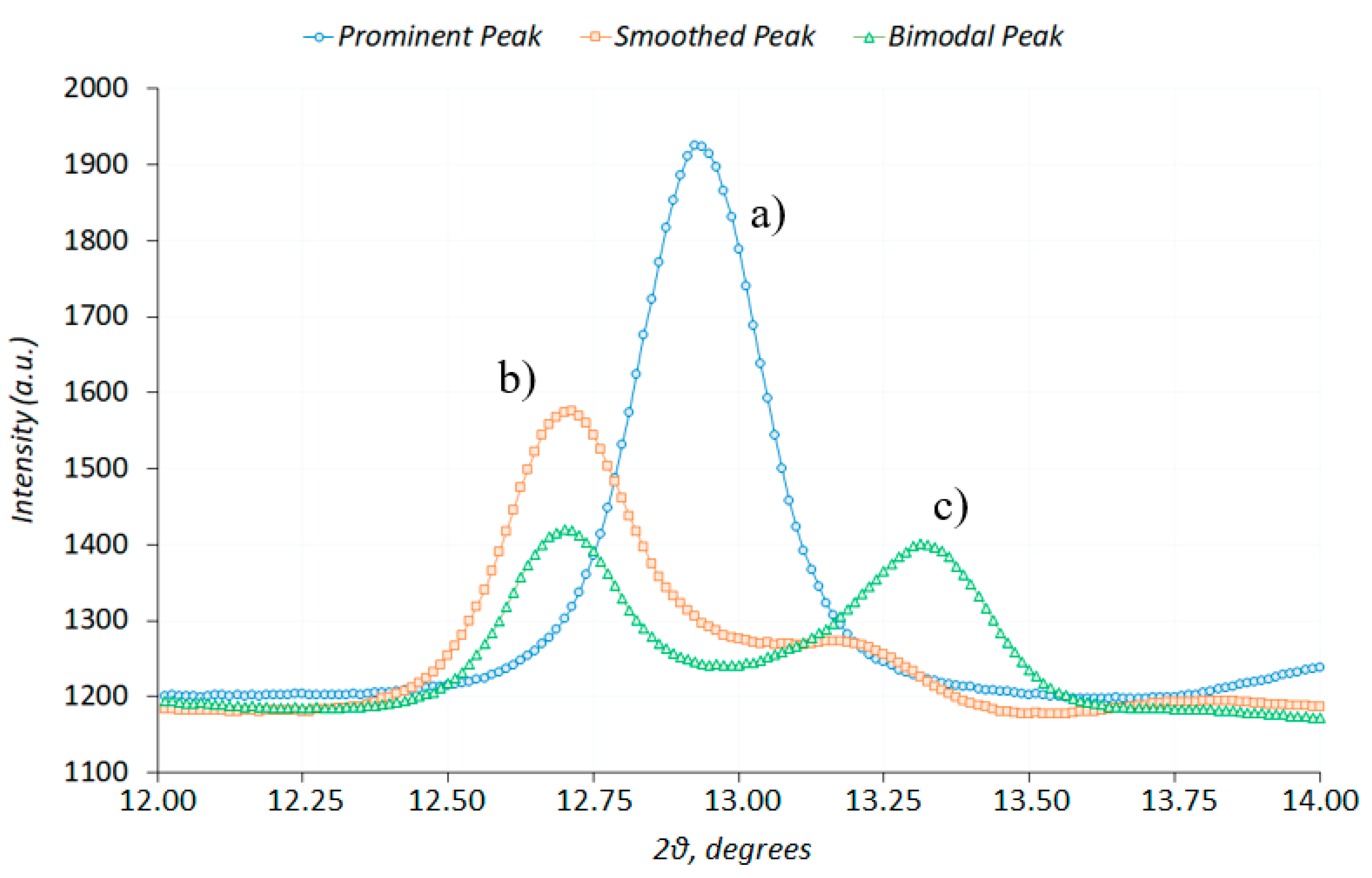
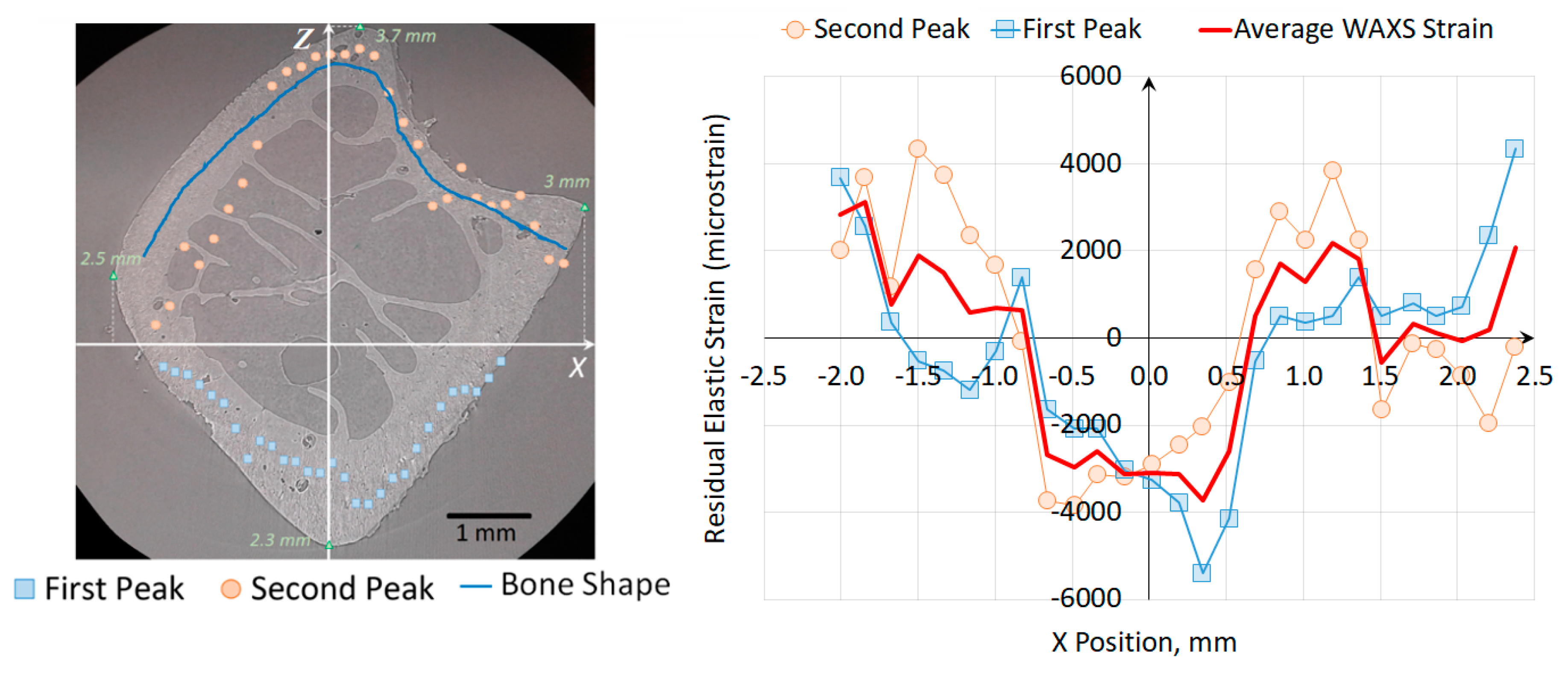
3.2. WAXS Analysis
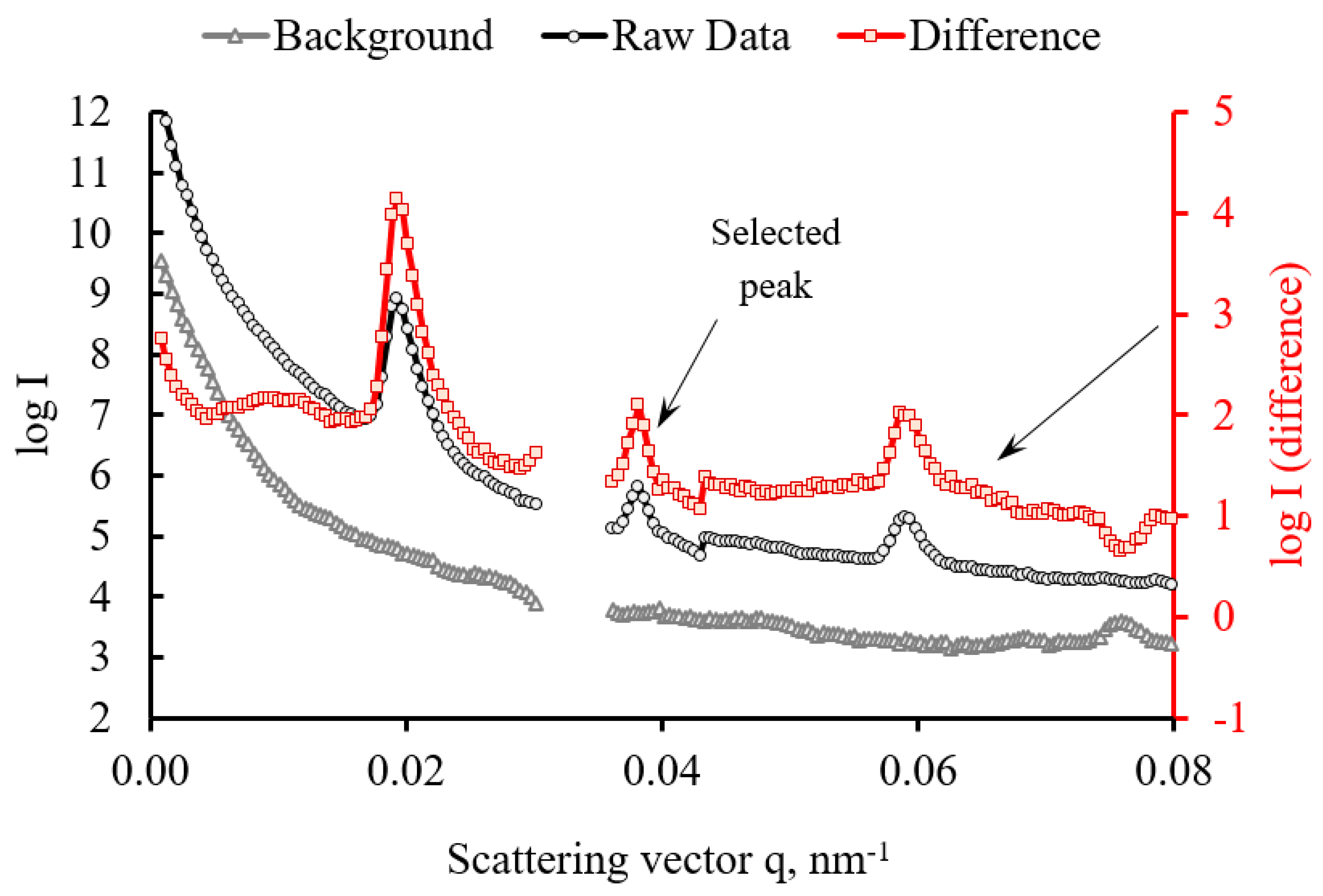
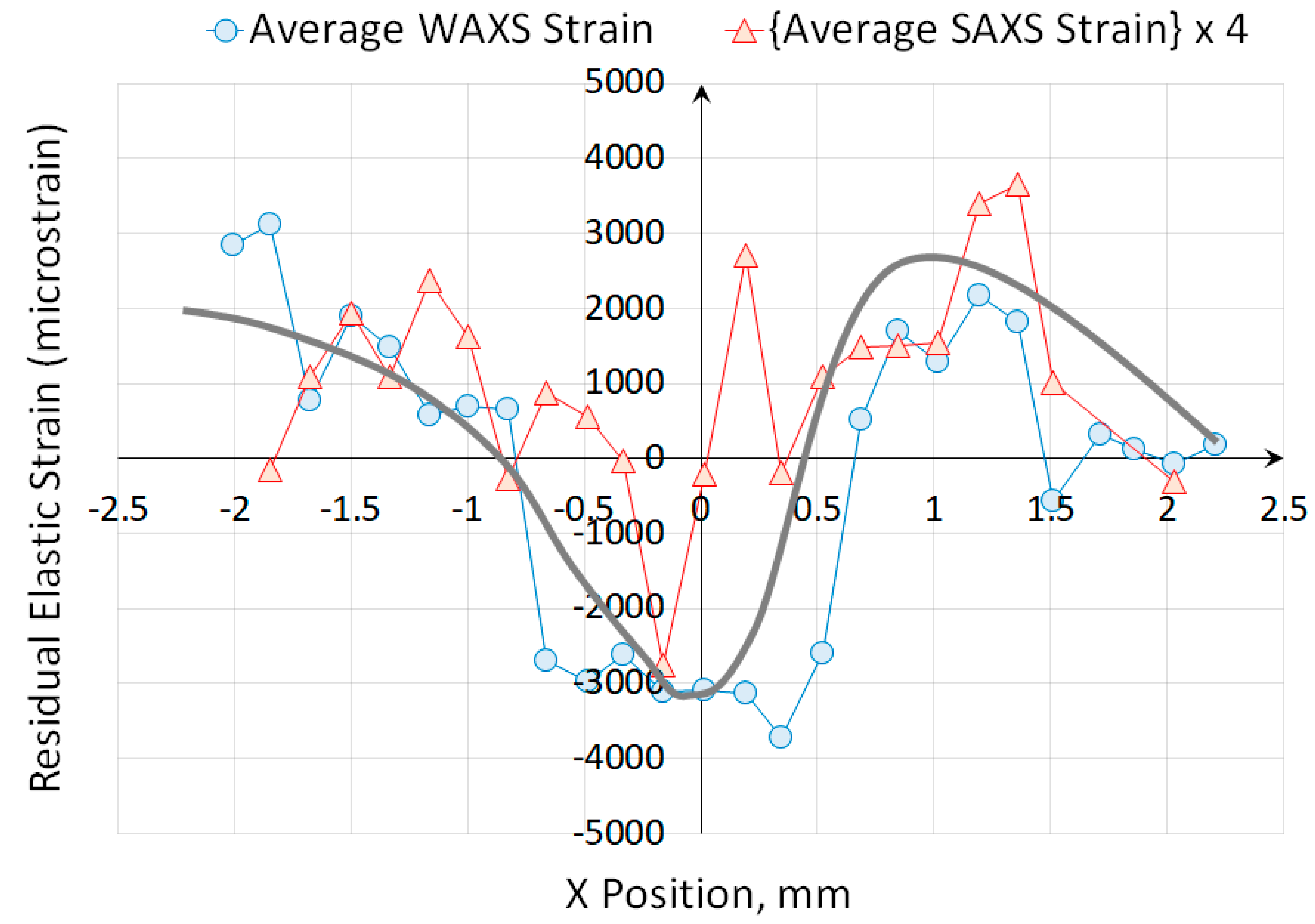
3.3. SAXS Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- International Osteoporosis Foundation. Facts and Statistics. Available online: https://www.iofbonehealth.org/facts-statistics (accessed on 20 April 2020).
- Hooke, R. Micrographia, or Some Physiological Descriptions of Minute Bodies Made by Magnifying Glasses, with Observations and Inquiries Thereupon, 1st ed.; Royal Society: London, UK, 1665; pp. 141–142. [Google Scholar]
- Tadano, S.; Yamada, S. How is residual stress/strain detected in bone tissue? Mech. Eng. Rev. 2016, 3, 1–13. [Google Scholar] [CrossRef]
- Frost, H.M. A 2003 update of bone physiology and Wolff’s law for clinicians. Angle Orthod. 2004, 74, 3–15. [Google Scholar] [PubMed]
- Spiegelberg, B.; Parratt, T.; Dheerendra, S.K.; Khan, W.S.; Jennings, R.; Marsh, D.R. Ilizarov principles of deformity correction. RCS Ann. 2010, 92, 101–105. [Google Scholar] [CrossRef] [PubMed]
- Sui, T.; Lunt, A.J.G.; Baimpas, N.; Sandholzer, M.A.; Li, T.; Zeng, K.; Landini, G.; Korsunsky, A.M. Understanding nature’s residual strain engineering at the human dentine–enamel junction interface. Acta Biomater. 2016, 32, 256–263. [Google Scholar] [CrossRef] [PubMed]
- Barthelat, F.; Yin, Z.; Buehler, M.J. Structure and mechanics of interfaces in biological materials. Nat. Rev. Mater. 2016, 1, 1–16. [Google Scholar] [CrossRef]
- Tadano, S.; Giri, B. X-ray diffraction as a promising tool to characterize bone nanocomposites. Sci. Technol. Adv. Mater. 2001, 12, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Sasaki, N.; Sudoh, Y. X-ray pole figure analysis of apatite crystals and collagen molecules in bone. Calcif. Tissue Int. 1997, 60, 361–367. [Google Scholar] [CrossRef]
- Almer, J.D.; Stock, S.R. Internal strains and stresses measured in cortical bone via high-energy X-ray diffraction. J. Struct. Biol. 2005, 152, 14–27. [Google Scholar] [CrossRef]
- Yamada, S.; Tadano, S.; Todoh, M.; Fujisaki, K. Residual stress distribution in the bovine femoral diaphysis measured by synchrotron. J. Biomech. Sci. Eng. 2011, 6, 114–124. [Google Scholar] [CrossRef][Green Version]
- Yamada, S.; Tadano, S. Effects of growth on residual stress distribution along the radial depth of cortical cylinders from bovine femurs. J. Biomech. 2013, 46, 2130–2136. [Google Scholar] [CrossRef][Green Version]
- Yamada, S.; Tadano, S.; Onuma, M. X-ray diffraction technique with imaging plate for detecting surface distribution of residual stress in diaphysis of bovine femurs. Exp. Mech. 2014, 54, 633–640. [Google Scholar] [CrossRef][Green Version]
- Tadano, S.; Okoshi, T. Residual stress in bone structure and tissue of rabbit’s tibiofibular. Bio-Med Mater. Eng. 2006, 16, 11–21. [Google Scholar]
- Todoh, M.; Tadano, S.; Shibano, J.; Ukai, T. Polychromatic X-ray measurements of anisotropic residual stress in bovine femoral bone. JSME Int. J. Ser. C Mech. Syst. Mach. Elem. Manuf. 2000, 43, 795–801. [Google Scholar] [CrossRef][Green Version]
- Al Nazer, R.; Lanovaz, J.; Kawalilak, C.; Johnston, J.D.; Kontulainen, S. Direct in vivo measurements in human bone–a systematic literature review. J. Biomech. 2012, 45, 27–40. [Google Scholar] [CrossRef]
- Rho, J.Y.; Zioupos, P.; Currey, J.D.; Pharr, G.M. Variations in the individual thick lamellar properties within osteons by nanoindentation. Bone 1999, 25, 295–300. [Google Scholar] [CrossRef]
- Fujisaki, K.; Tadano, S.; Sasaki, N. A method on strain measurement of HAp in cortical bone from diffusive profile of X-ray diffraction. J. Biomech. 2006, 39, 579–586. [Google Scholar] [CrossRef][Green Version]
- Fujisaki, K.; Tadano, S. Relationship between bone tissue strain and lattice strain of HAp crystals in bovine cortical under tensile loading. J. Biomech. 2007, 40, 1832–1838. [Google Scholar] [CrossRef]
- Grady, M.E. Mechanics of Biological Systems and Materials & Micro-and Nanomechanics, 1st ed.; Springer: Cham, Switzerland, 2020; pp. 11–18. [Google Scholar]
- Gustafsson, A.; Mathavan, N.; Turunen, M.J.; Engqvist, J.; Khayyeri, H.; Hall, S.A.; Isaksson, H. Linking multiscale deformation to microstructure in cortical bone using in situ loading, digital image correlation and synchrotron X-ray scattering. Acta Biomater. 2018, 15, 323–331. [Google Scholar] [CrossRef]
- Fernandez, M.P.; Barber, A.H.; Blunn, G.W.; Tozzi, G. Optimization of digital volume correlation computation in SR-microCT images of trabecular bone and bone-biomaterial systems. J. Microsc. 2018, 272, 213–228. [Google Scholar] [CrossRef]
- Mastrogiacomo, M.; Campi, G.; Cancedda, R.; Cedola, A. Synchrotron radiation techniques boost the research in bone tissue engineering. Acta Biomater. 2019, 15, 33–46. [Google Scholar] [CrossRef]
- Ma, S.; Boughton, O.; Jin, A.; Cobb, J.; Hansen, U.; Abel, R. Synchrotron imaging assessment of bone quality. Clin. Rev. Bone Miner. Metab. 2016, 14, 150–160. [Google Scholar] [CrossRef] [PubMed]
- Zaouali, A.; Girault, B.; Gloaguen, D.; Jordana, F.; Moya, M.J.; Dubos, P.A.; Geoffroy, V.; Schwartzkopf, M.; Snow, T.; Gupta, H.; et al. In situ mechanical behavior of regenerating rat calvaria bones under tensile load via synchrotron diffraction characterization. Mater. Res. Proc. 2018, 6, 117–122. [Google Scholar]
- Larrue, A.; Rattner, A.; Peter, Z.A.; Olivier, C.; Laroche, N.; Vico, L.; Peyrin, F. Synchrotron radiation Micro-CT at the micrometer scale for the analysis of the three-dimensional morphology of microcracks in human trabecular bone. PLoS ONE 2011, 6, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Koester, K.J.; Ager, J.W.; Ritchie, R.O. The true toughness of human cortical bone measured with realistically short cracks. Nat. Mater. 2008, 7, 672–677. [Google Scholar] [CrossRef]
- Salvati, E.; Sui, T.; Zhang, H.; Lunt, A.J.G.; Fong, K.S.; Song, X.; Korsunsky, A.M. Elucidating the mechanism of fatigue crack acceleration following the occurrence of an underload. Adv. Eng. Mater. 2016, 18, 2076–2087. [Google Scholar] [CrossRef]
- Zhou, T.; Wang, H.; Connolley, T.; Scott, S.; Baker, N.; Sawhney, K. Development of an X-ray imaging system to prevent scintillator degradation for white synchrotron radiation. J. Synchrotron Rad. 2018, 25, 801–807. [Google Scholar] [CrossRef]
- Sawhney, K.J.S.; Dolbnya, I.P.; Tiwari, M.K.; Alianelli, L.; Scott, S.M.; Preece, G.M.; Pedersen, U.K.; Walton, R.D. A test beamline on diamond light source. AIP Conf. Proc. 2010, 1234, 387–390. [Google Scholar]
- Filik, J.; Ashton, A.W.; Chang, P.C.Y.; Chater, P.A.; Day, S.J.; Drakopoulos, M.; Gerring, M.W.; Hart, M.L.; Magdysyuk, O.V.; Michalik, S.; et al. Processing Two-Dimensional X-ray diffraction and small-angle scattering data in DAWN 2. J. Appl. Cryst. 2017, 50, 959–966. [Google Scholar] [CrossRef]
- Statnik, E.S.; Uzun, F.; Salimon, A.I.; Korsunsky, A.M. Communications in Computer and Information Science, 1st ed.; Springer Link: New York, NY, USA, 2019; pp. 282–288. [Google Scholar]
- Korsunsky, A.M.; Baimpas, N.; Song, X.; Belnoue, J.; Hofmann, F.; Abbey, B.; Xie, M.; Andrieux, J.; Buslaps, T.; Neo, T.K. Strain tomography of polycrystalline zirconia dental prostheses by synchrotron X-ray diffraction. Acta Mater. 2011, 59, 2501–2513. [Google Scholar] [CrossRef]
- Sui, T.; Baimpas, N.; Dolbnya, I.P.; Prisacariu, C.; Korsunsky, A.M. Multiple-length-scale deformation analysis in a thermoplastic polyurethane. Nat. Commun. 2015, 6, 65–83. [Google Scholar] [CrossRef]
- Kinney, J.H.; Pople, J.A.; Marshall, G.W.; Marshall, S.J. Collagen orientation and crystallite size in guman dentin: A small angle X-ray scattering study. Calcif. Tissue Int. 2001, 69, 31–37. [Google Scholar] [CrossRef] [PubMed]
- Korsunsky, A.M. Variational eigenstrain analysis of synchrotron diffraction measurements of residual elastic strain in a bent titanium alloy bar. J. Mech. Mat. Struct. 2006, 1, 259–277. [Google Scholar] [CrossRef][Green Version]
- Ritchie, R.O. How does human bone resist fracture? Ann. N. Y. Acad. Sci. 2010, 1192, 72–80. [Google Scholar] [CrossRef] [PubMed]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Statnik, E.S.; Salimon, A.I.; Besnard, C.; Chen, J.; Wang, Z.; Moxham, T.; Dolbnya, I.P.; Korsunsky, A.M. Ovine Bone Morphology and Deformation Analysis Using Synchrotron X-ray Imaging and Scattering. Quantum Beam Sci. 2020, 4, 29. https://doi.org/10.3390/qubs4030029
Statnik ES, Salimon AI, Besnard C, Chen J, Wang Z, Moxham T, Dolbnya IP, Korsunsky AM. Ovine Bone Morphology and Deformation Analysis Using Synchrotron X-ray Imaging and Scattering. Quantum Beam Science. 2020; 4(3):29. https://doi.org/10.3390/qubs4030029
Chicago/Turabian StyleStatnik, Eugene S., Alexey I. Salimon, Cyril Besnard, Jingwei Chen, Zifan Wang, Thomas Moxham, Igor P. Dolbnya, and Alexander M. Korsunsky. 2020. "Ovine Bone Morphology and Deformation Analysis Using Synchrotron X-ray Imaging and Scattering" Quantum Beam Science 4, no. 3: 29. https://doi.org/10.3390/qubs4030029
APA StyleStatnik, E. S., Salimon, A. I., Besnard, C., Chen, J., Wang, Z., Moxham, T., Dolbnya, I. P., & Korsunsky, A. M. (2020). Ovine Bone Morphology and Deformation Analysis Using Synchrotron X-ray Imaging and Scattering. Quantum Beam Science, 4(3), 29. https://doi.org/10.3390/qubs4030029








