In Situ Analysis of the Phase Transformation Kinetics in the β-Water-Quenched Ti-5Al-5Mo-5V-3Cr-1Zr Alloy during Ageing after Fast Heating
Abstract
1. Introduction
2. Materials and Methods
3. Results
3.1. Initial Condition
3.2. In Situ Measurements
3.2.1. Influence of Heating Up to 500 °C
3.2.2. Phase Evolution during Ageing at 500 °C
3.2.3. Microhardness
4. Discussion
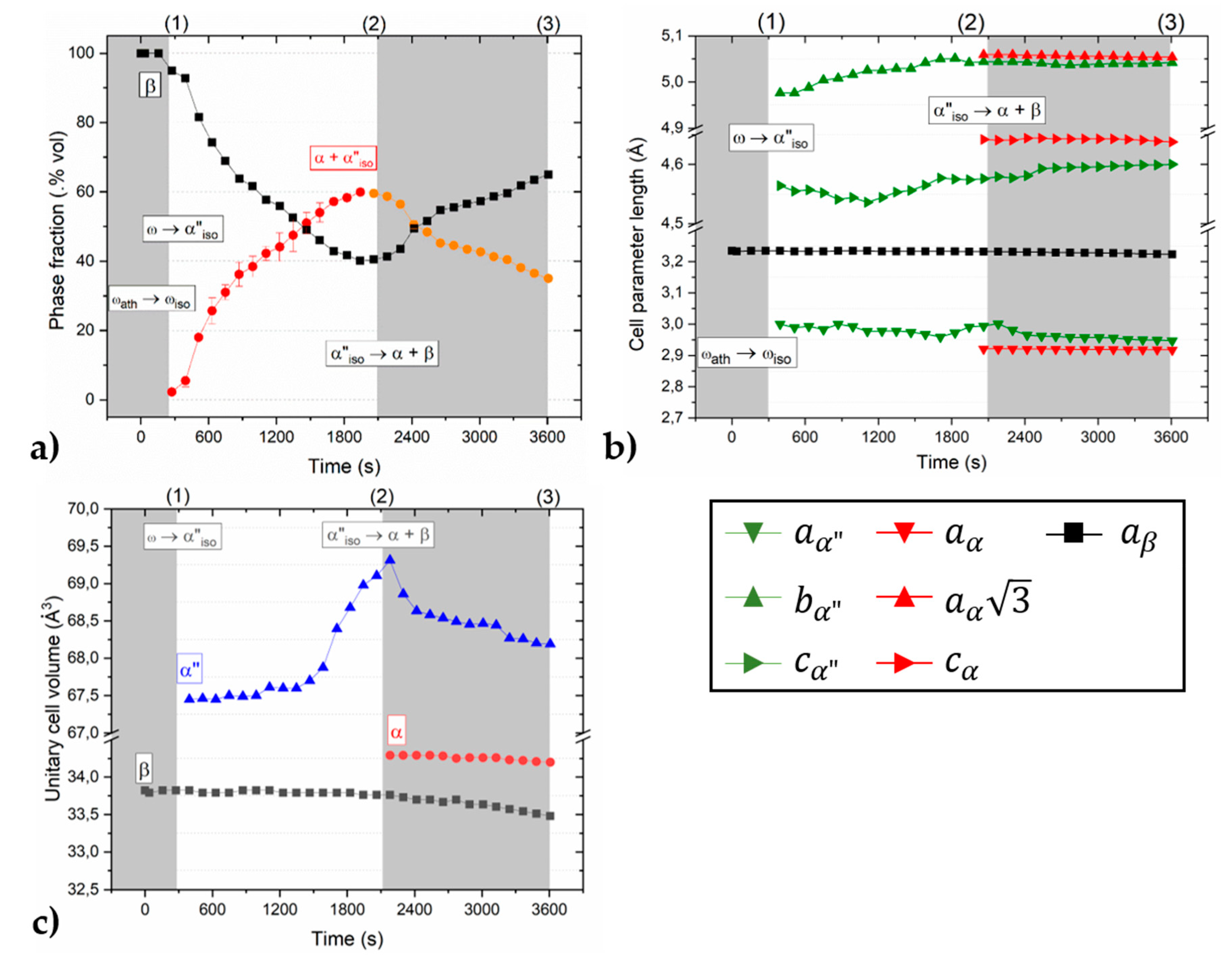
4.1. Quantitative Analysis of Phase Transformation during Isothermal Treatment
- From t = 0 s β was considered;
- From t = 300 s α and α”iso volume fraction were considered together. The deconvolution of both phases during refinement was not possible due to presented difficulties; thus, it is not possible to assure that the fraction provided by the refinement for each one of these phases individually is correct, so the summed values were used. The α”iso lattice parameter was taken into account from t = 300 s;
- From t = 2080 s, the α phase lattice parameter evolution started to be evaluated following the increment of the intensity of {101}α reflections from {111}α” and {021}α”.
4.2. Behavior of β during Ageing
4.3. Precipitation and Decomposition of α”iso
4.4. Precipitation and Morphology of α
4.5. Hardness
5. Conclusions
- The use of fast heating suppresses any change in the microstructure, restricting the phase transformations to the ageing period.
- During the ageing treatment, the phase transformation follows the sequence β + ω → β + ωiso + ω → α + α”iso+ β. The presence of the diffuse streaks is indicative of a transient state.
- The presence of ω phase is confirmed by hardness measurements. The ω phase reflections are not observed in this work and this might be related to the low volume fraction and the superposition of broader and intense β peaks over the ω peaks.
- The isothermal α” (α”iso) phase may precipitate from the ω phase and mainly from the domains of spinodal decomposition of the β phase, given its role in the homogenization of these domains. This phase is considered to regulate the transformation throughout the studied ageing.
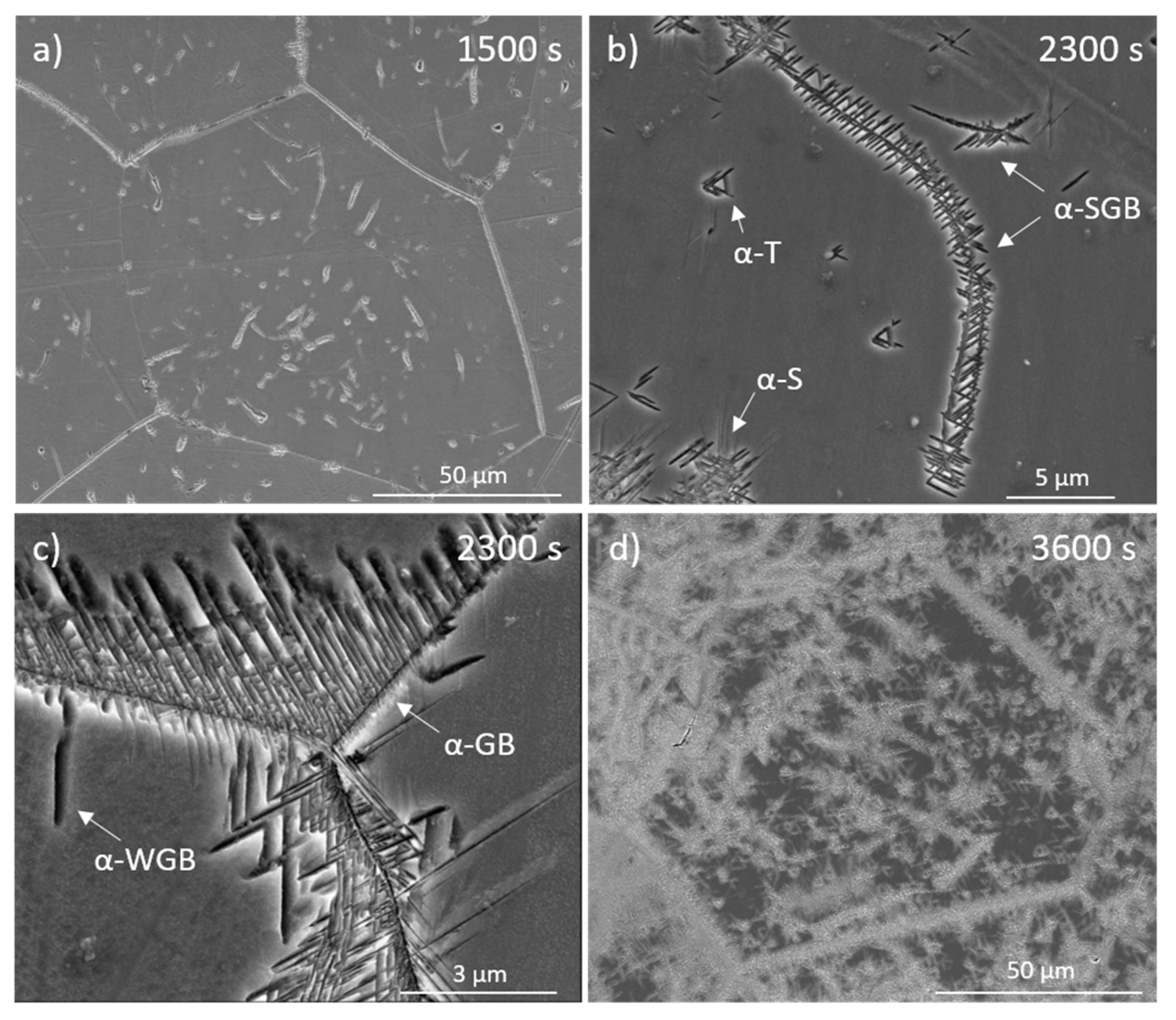
- Based on the evolution of the {111}α”/{021}α”reflection to {101}α, the α phase may nucleate from α”iso, ω phase and β phases. SEM analysis allows the observation of some characteristic morphologies of sympathetic nucleation (α-S) and groups with a tendency to choose preferred directions (α-T), in addition to the preferential precipitation of α along grain boundaries (α-GB), and the Widmanstätten α (α-WGB) formed near to α-GB.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lütjering, G.; Williams, J.C. Titanium, 2nd ed.; Springer-Verlag Berlin and Heidelberg GmbH & Co. K: Berlin, Germany, 2007. [Google Scholar]
- Moiseyev, V.N. Titanium Alloys: Russian Aircraft and Aerospace Applications; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Barriobero-Vila, P.; Requena, G.; Schwarz, S.; Warchomicka, F.; Buslaps, T. Influence of phase transformation kinetics on the formation of α in a β-quenched Ti–5Al–5Mo–5V–3Cr–1Zr alloy. Acta Mater. 2015, 95, 90–101. [Google Scholar] [CrossRef]
- Jones, N.G.; Dashwood, R.J.; Jackson, M.; Dye, D. β Phase decomposition in Ti-5Al-5Mo-5V-3Cr. Acta Mater. 2009, 57, 3830–3839. [Google Scholar] [CrossRef]
- Aeby-Gautier, E.; Settefrati, A.; Bruneseaux, F.; Appolaire, B.; Denand, B.; Dehmas, M.; Geandier, G.; Boulet, P. Isothermal α″ formation in β metastable titanium alloys. J. Alloys Compd. 2013, 577, S439–S443. [Google Scholar] [CrossRef]
- Ivasishin, O.; Markovsky, P.; Semiatin, S.; Ward, C. Aging response of coarse- and fine-grained β titanium alloys. Mater. Sci. Eng. A 2005, 405, 296–305. [Google Scholar] [CrossRef]
- Ivasishin, O.M.; Markovsky, P.E.; Matviychuk, Y.V.; Semiatin, S.L. Precipitation and recrystallization behavior of beta titanium alloys during continuous heat treatment. Met. Mater. Trans. A 2003, 34, 147–158. [Google Scholar] [CrossRef]
- Settefrati, A.; Aeby-Gautier, E.; Dehmas, M.; Geandier, G.; Appolaire, B.; Audion, S.; Delfosse, J. Precipitation in a near Beta Titanium Alloy on Ageing: Influence of Heating Rate and Chemical Composition of the Beta-Metastable Phase. Solid State Phenom. 2011, 172, 760–765. [Google Scholar] [CrossRef]
- Barriobero-Vila, P.; Requena, G.; Warchomicka, F.; Stark, A.; Schell, N.; Buslaps, T. Phase transformation kinetics during continuous heating of a β-quenched Ti–10V–2Fe–3Al alloy. J. Mater. Sci. 2015, 50, 1412–1426. [Google Scholar] [CrossRef]
- Aeby-Gautier, E.; Bruneseaux, F.; Teixeira, J.D.C.; Appolaire, B.; Geandier, G.; Denis, S. Microstructural formation in Ti alloys: In-situ characterization of phase transformation kinetics. JOM 2007, 59, 54–58. [Google Scholar] [CrossRef]
- Liss, K.-D.; Bartels, A.; Schreyer, A.; Clemens, H. High-Energy X-Rays: A tool for Advanced Bulk Investigations in Materials Science and Physics. Textures Microstruct. 2003, 35, 219–252. [Google Scholar] [CrossRef]
- Liss, K.-D.; Yan, K. Thermo-mechanical processing in a synchrotron beam. Mater. Sci. Eng. A 2010, 528, 11–27. [Google Scholar] [CrossRef]
- Liss, K.D. Thermo-Mechanical Processing in a Synchrotron Beam—From Simple Metals To Multiphase Alloys and Intermetallics. World J. Eng. 2010, 7, 438. [Google Scholar]
- Warchomicka, F.; Yubero, C.-; Zehetner, E.; Requena, G.; Stark, A.; Canelo-Yubero, D.; Poletti, C. In-Situ Synchrotron X-Ray Diffraction of Ti-6Al-4V during Thermomechanical Treatment in the Beta Field. Metals 2019, 9, 862. [Google Scholar] [CrossRef]
- Liss, K.-D.; Whitfield, R.E.; Xu, W.; Buslaps, T.; Yeoh, L.A.; Wu, X.; Zhang, D.; Xia, K. In situsynchrotron high-energy X-ray diffraction analysis on phase transformations in Ti–Al alloys processed by equal-channel angular pressing. J. Synchrotron Radiat. 2009, 16, 825–834. [Google Scholar] [CrossRef] [PubMed]
- Bania, P.J. Beta titanium alloys and their role in the titanium industry. JOM 1994, 46, 16–19. [Google Scholar] [CrossRef]
- Nag, S.; Zheng, Y.; Williams, R.; Devaraj, A.; Boyne, A.; Wang, Y.; Collins, P.; Viswanathan, G.; Tiley, J.; Muddle, B.; et al. Non-classical homogeneous precipitation mediated by compositional fluctuations in titanium alloys. Acta Mater. 2012, 60, 6247–6256. [Google Scholar] [CrossRef]
- The FIT2D Home Page. Available online: http://www.esrf.eu/computing/scientific/FIT2D/ (accessed on 20 October 2019).
- ImageJ. Available online: https://imagej.nih.gov/ij/ (accessed on 20 October 2019).
- MAUD—Material Analys Using Diffraction. Available online: http://maud.radiographema.eu/ (accessed on 20 October 2019).
- Banerjee, S.; Mukhopadhyay, P. Phase Transformations: Examples from Titanium and Zirconium Alloys; Elsevier: Amsterdam, The Netherlands, 2007; Volume 1. [Google Scholar]
- Basak, C.B.; Neogy, S.; Srivastava, D.; Dey, G.; Banerjee, S. Disordered bcc γ-phase to δ-phase transformation in Zr-rich U-Zr alloy. Philos. Mag. 2011, 91, 3290–3306. [Google Scholar] [CrossRef]
- Obbard, E.G.; Hao, Y.L.; Talling, R.J.; Li, S.J.; Zhang, Y.W.; Dye, D.; Yang, R. The effect of oxygen on α″ martensite and superelasticity in Ti-24Nb-4Zr-8Sn. Acta Mater. 2011, 59, 112–125. [Google Scholar] [CrossRef]
- Li, T.; Kent, D.; Sha, G.; Stephenson, L.T.; Ceguerra, A.V.; Ringer, S.P.; Dargusch, M.S.; Cairney, J.M. New insights into the phase transformations to isothermal ω and ω-assisted α in near β-Ti alloys. Acta Mater. 2016, 106, 353–366. [Google Scholar] [CrossRef]
- Liss, K.-D.; Stark, A.; Bartels, A.; Clemens, H.; Buslaps, T.; Phelan, D.; Yeoh, L.A. Directional Atomic Rearrangements During Transformations Between the α- and γ-Phases in Titanium Aluminides. Adv. Eng. Mater. 2008, 10, 389–392. [Google Scholar] [CrossRef]
- Settefrati, A.; Dehmas, M.; Geandier, G.; Denand, B.; Aeby-Gautier, E.; Appolaire, B.; Khelifati, G.; Delfosse, J. Precipitation sequences in beta metastable phase of Ti-5553 alloy during ageing. Ti-2011; Science Press Beijing: Beijing, China, 2011; pp. 468–472. [Google Scholar]
- Dehghan-Manshadi, A.; Dippenaar, R.J. Development of α-phase morphologies during low temperature isothermal heat treatment of a Ti–5Al–5Mo–5V–3Cr alloy. Mater. Sci. Eng. A 2011, 528, 1833–1839. [Google Scholar] [CrossRef]
- Porter, D.A.; Easterling, K.E.; Sherif, M. Phase Transformations in Metals and Alloys; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar]
- Zheng, Y.; Sosa, J.M.; Fraser, H.L. On the Influence of Athermal ω and α Phase Instabilities on the Scale of Precipitation of the α Phase in Metastable β-Ti Alloys. JOM 2016, 68, 1343–1349. [Google Scholar] [CrossRef]
- Contrepois, Q.; Carton, M.; Lecomte-Beckers, J. Characterization of the β Phase Decomposition in Ti-5Al-5Mo-5V-3Cr at Slow Heating Rates. Open J. Met. 2011, 1, 1–11. [Google Scholar] [CrossRef]
- Ramsteiner, I.; Shchyglo, O.; Mezger, M.; Udyansky, A.; Bugaev, V.; Schöder, S.; Reichert, H.; Dosch, H. Omega-like diffuse X-ray scattering in Ti–V caused by static lattice distortions. Acta Mater. 2008, 56, 1298–1305. [Google Scholar] [CrossRef]
- Banerjee, S.; Tewari, R.; Dey, G.K. Omega phase transformation-morphologies and mechanisms. Int. J. Mater. Res. 2006, 97, 963–977. [Google Scholar] [CrossRef]
- Mittemeijer, E.J.; Scardi, P. Diffraction Analysis of the Microstructure of Materials; Springer: New York, NY, USA, 2013. [Google Scholar]
- Settefrati, A.; Aeby-Gautier, E.; Appolaire, B.; Dehmas, M.; Geandier, G.; Khelifati, G. Low temperature transformation in the β-metastable Ti 5553 alloy. Mater. Sci. Forum 2013, 738–739, 97–102. [Google Scholar] [CrossRef]
- Sabeena, M.; Murugesan, S.; Mythili, R.; Sinha, A.K.; Singh, M.N.; Vijayalakshmi, M.; Deb, S.K. Studies on ω Phase Formation in Ti-Mo Alloys Using Synchrotron XRD. Trans. Indian Inst. Met. 2014, 68, 1–6. [Google Scholar] [CrossRef]
- Šmilauerová, J.; Harcuba, P.; Kriegner, D.; Janecek, M.; Holý, V. Growth kinetics of ω particles in β-Ti matrix studied by in-situ small-angle x-ray scattering. Acta Mater. 2015, 100, 126–134. [Google Scholar] [CrossRef]
- Nejezchlebová, J.; Janovská, M.; Seiner, H.; Sedlák, P.; Landa, M.; Šmilauerová, J.; Stráský, J.; Harcuba, P.; Janeček, M. The effect of athermal and isothermal ω phase particles on elasticity of β-Ti single crystals. Acta Mater. 2016, 110, 185–191. [Google Scholar] [CrossRef]
- Williams, J.C.; Hickman, B.S.; Marcus, H.L. The effect of omega phase on the mechanical properties of titanium alloys. Metall. Trans. 1971, 2, 1913–1919. [Google Scholar] [CrossRef]
- Laughlin, D.E.; Cahn, J.W. Spinodal decomposition in age hardening copper-titanium alloys. Acta Met. 1975, 23, 329–339. [Google Scholar] [CrossRef]
- Geandier, G.; Aeby-Gautier, E.; Settefrati, A.; Dehmas, M.; Appolaire, B. Study of diffusive transformations by high energy X-ray diffraction. Comptes Rendus Phys. 2012, 13, 257–267. [Google Scholar] [CrossRef]
- Barriobero-Vila, P.; Requena, G.; Buslaps, T.; Alfeld, M.; Boesenberg, U. Role of element partitioning on the α–β phase transformation kinetics of a bi-modal Ti–6Al–6V–2Sn alloy during continuous heating. J. Alloy. Compd. 2015, 626, 330–339. [Google Scholar] [CrossRef]
- Chen, F.; Xu, G.; Zhou, K.; Zhang, X. Exploring the Phase Transformation in β-Quenched Ti-55531 Alloy During Continuous Heating via Dilatometric Measurement, Microstructure Characterization, and Diffusion Analysis. Met. Mater. Trans. A 2016, 47, 5383–5394. [Google Scholar] [CrossRef]
- Elmer, J.; Palmer, T.; Babu, S.; Specht, E. In situ observations of lattice expansion and transformation rates of α and β phases in Ti–6Al–4V. Mater. Sci. Eng. A 2005, 391, 104–113. [Google Scholar] [CrossRef]
- Mantani, Y.; Tajima, M. Phase transformation of quenched α″ martensite by aging in Ti-Nb alloys. Mater. Sci. Eng. A 2006, 438–440, 315–319. [Google Scholar] [CrossRef]
- Mantani, Y.; Takemoto, Y.; Hida, M.; Sakakibara, A.; Tajima, M. Phase Transformation of α″ Martensite Structure by Aging in Ti-8 mass%Mo Alloy. Mater. Trans. 2004, 45, 1629–1634. [Google Scholar] [CrossRef]
- Banumathy, S.; Mandal, R.K.; Singh, A.K. Structure of orthorhombic martensitic phase in binary Ti–Nb alloys. J. Appl. Phys. 2009, 106, 093518. [Google Scholar] [CrossRef]
- Ohmori, Y.; Ogo, T.; Nakai, K.; Kobayashi, S. Effects of ω-phase precipitation on β → α, α″ transformations in a metastable β titanium alloy. Mater. Sci. Eng. A 2001, 312, 182–188. [Google Scholar] [CrossRef]
- Tang, B.; Kou, H.C.; Wang, Y.H.; Zhu, Z.S.; Zhang, F.S.; Li, J.S. Kinetics of orthorhombic martensite decomposition in TC21 alloy under isothermal conditions. J. Mater. Sci. 2012, 47, 521–529. [Google Scholar] [CrossRef]
- Guimarães, R.P.M. Estudo in situ da solubilização e do envelhecimento da liga β-metaestável Ti-5Al-5Mo-5V-3Cr-1Zr usando difração de raio-X com luz síncrotron de alta energia. Master’s Dissertation, Escola de Engenharia de São Carlos, University of São Paulo, São Carlos, Brazil, 2018. [Google Scholar] [CrossRef]
- Balachandran, S.; Kashiwar, A.; Choudhury, A.; Banerjee, D.; Shi, R.; Wang, Y. On variant distribution and coarsening behavior of the α phase in a metastable β titanium alloy. Acta Mater. 2016, 106, 374–387. [Google Scholar] [CrossRef]
- Aaronson, H.; Spanos, G.; Masamura, R.; Vardiman, R.; Moon, D.; Menon, E.; Hall, M. Sympathetic nucleation: An overview. Mater. Sci. Eng. B 1995, 32, 107–123. [Google Scholar] [CrossRef]
- Dong, R.; Li, J.; Kou, H.; Fan, J.; Tang, B.; Sun, M. Precipitation behavior of α phase during aging treatment in a β-quenched Ti-7333. Mater. Charact. 2018, 140, 275–280. [Google Scholar] [CrossRef]
- Manda, P.; Singh, V.; Chakkingal, U.; Singh, A. Development of α precipitates in metastable Ti-5Al-5Mo-5V-3Cr and similar alloys. Mater. Charact. 2016, 120, 220–228. [Google Scholar] [CrossRef]
- Salib, M.; Teixeira, J.; Germain, L.; Lamielle, E.; Gey, N.; Aeby-Gautier, E. Influence of transformation temperature on microtexture formation associated with α precipitation at β grain boundaries in a β metastable titanium alloy. Acta Mater. 2013, 61, 3758–3768. [Google Scholar] [CrossRef]
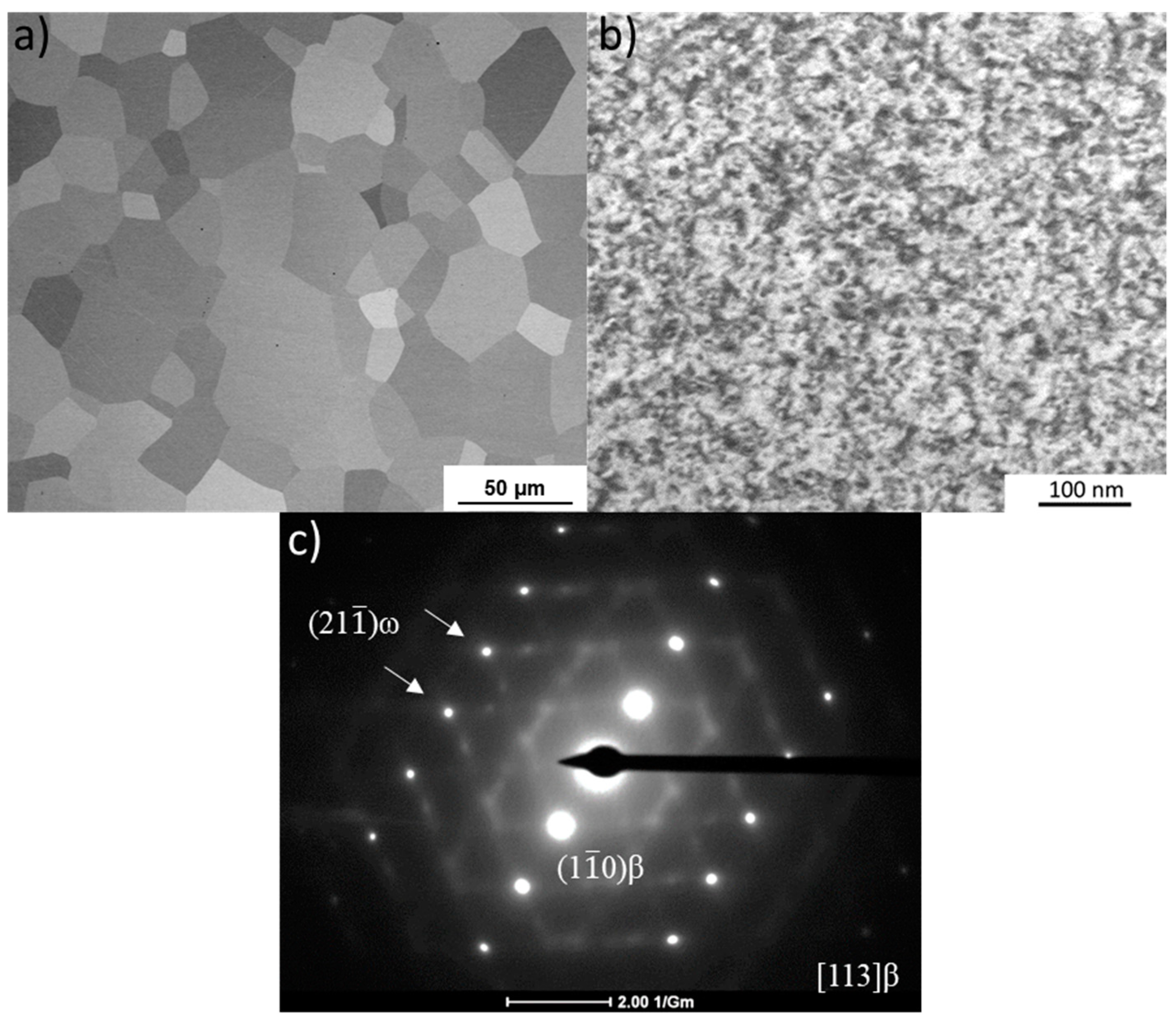
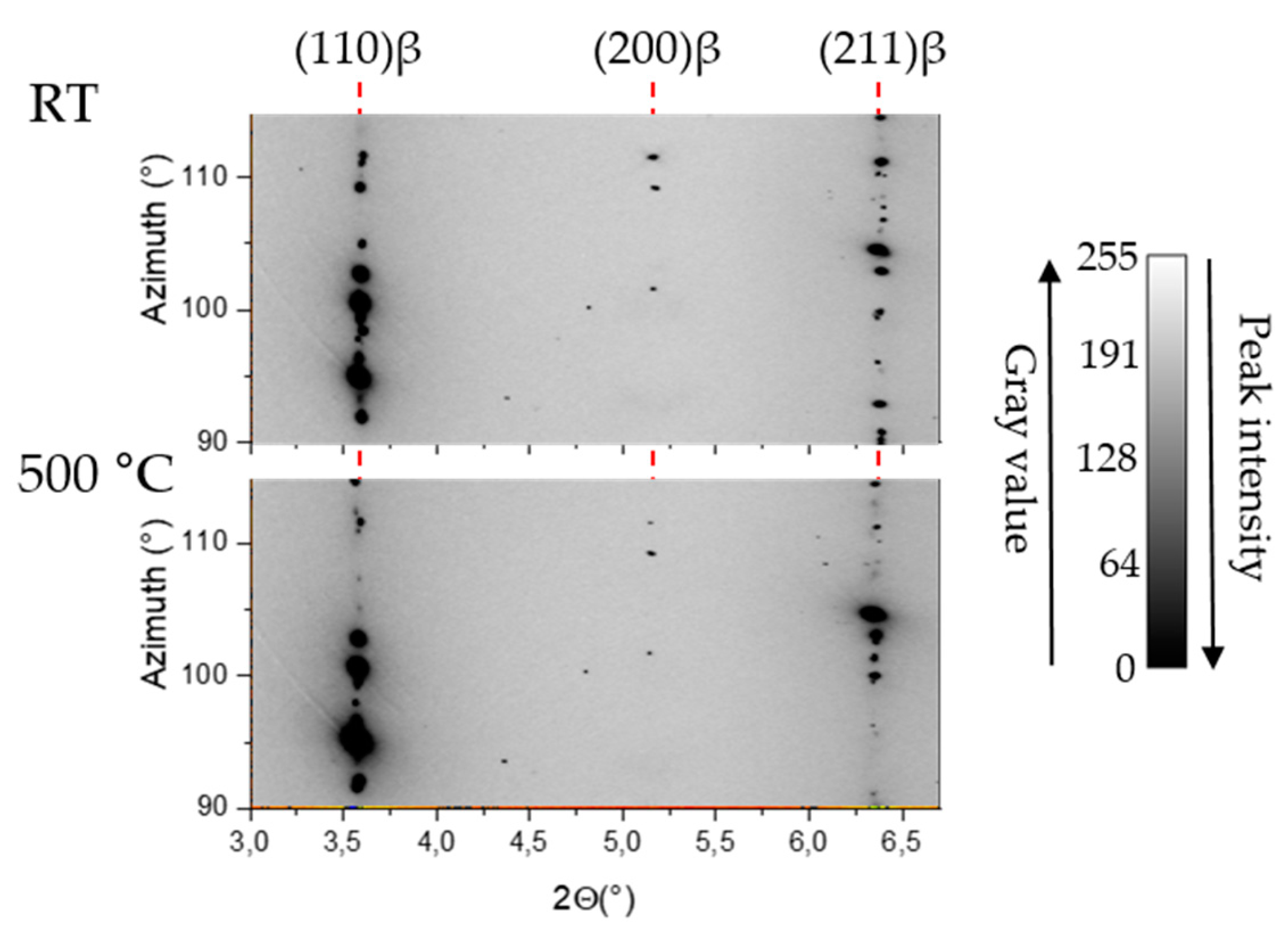
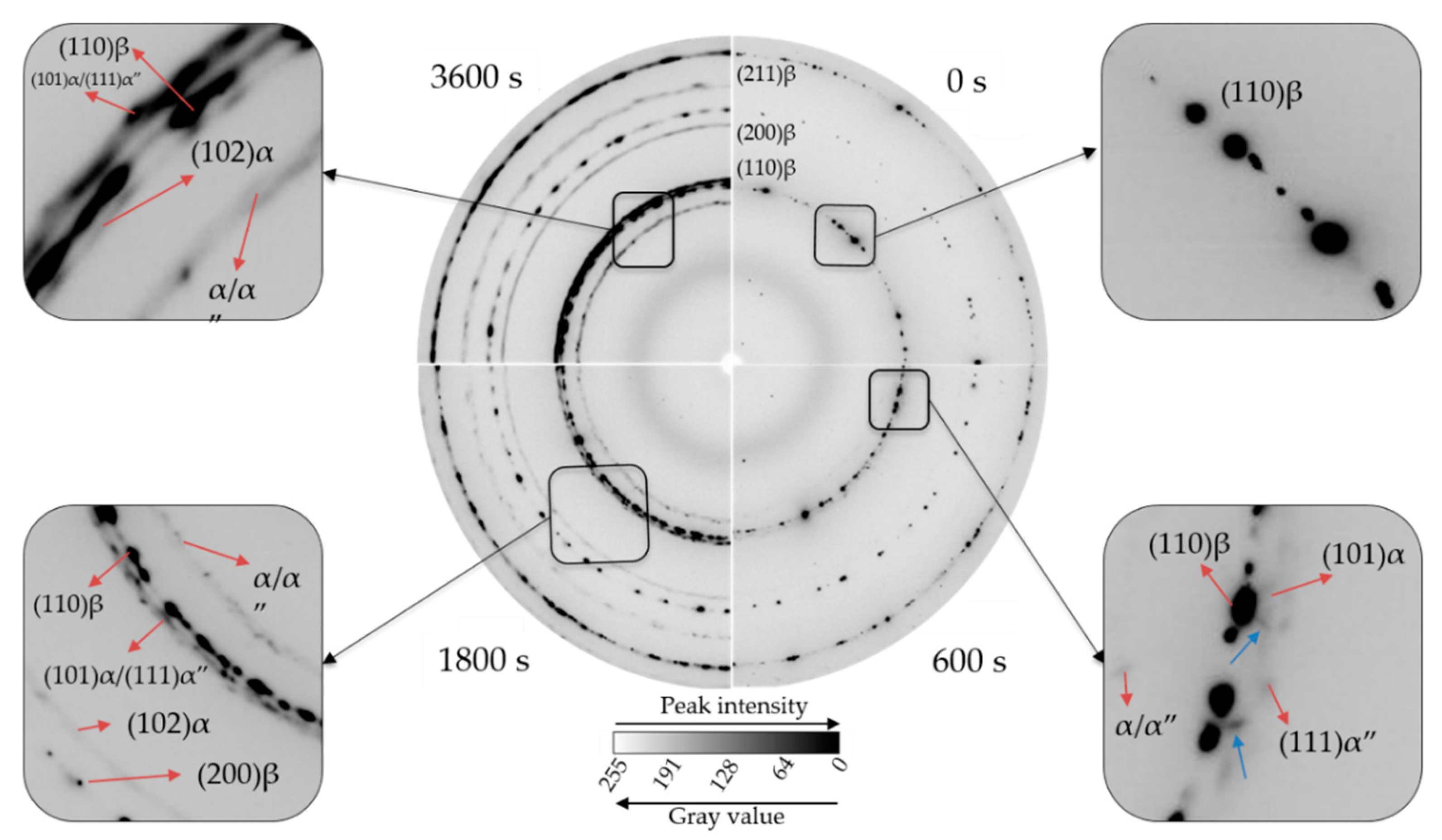

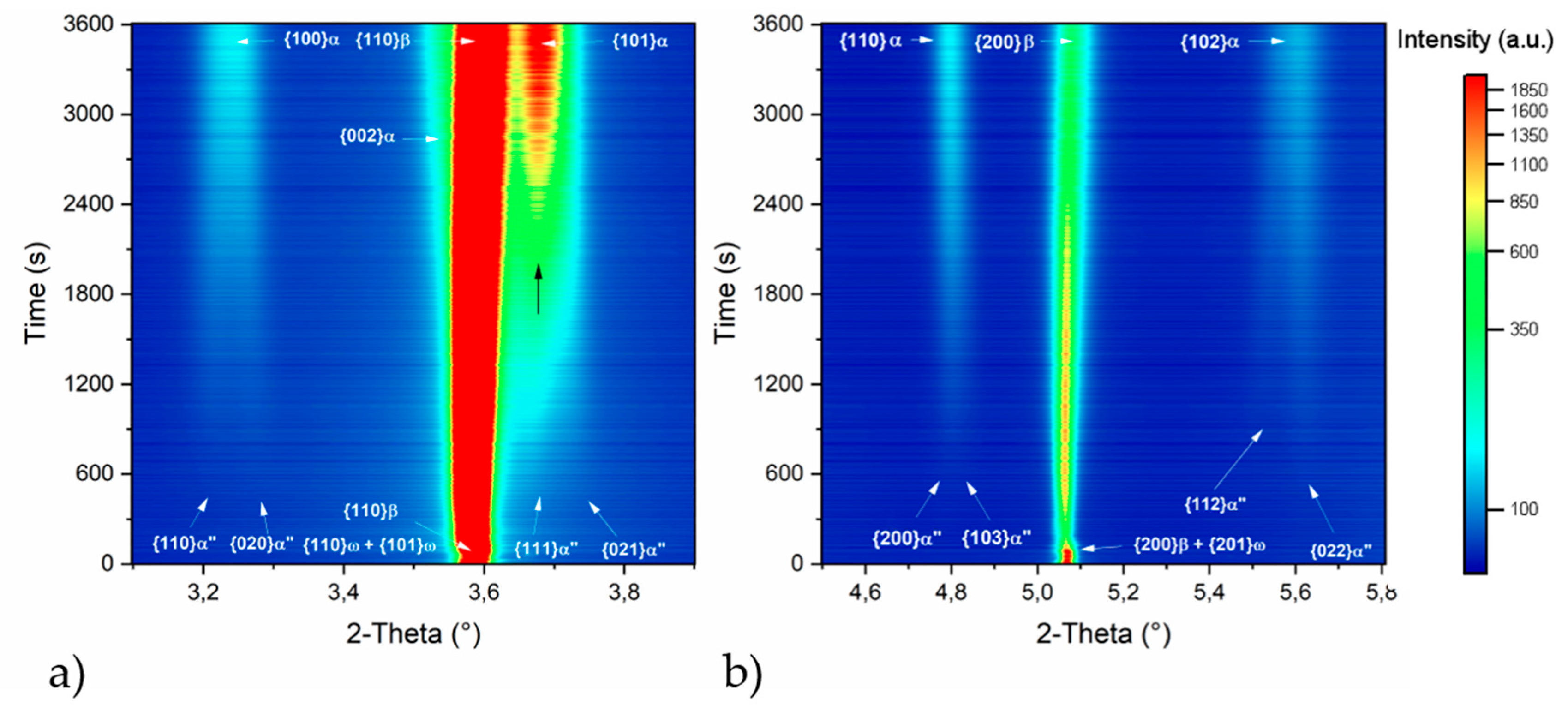


| Phase | A (Å) | B (Å) | C (Å) | Structure | Space Group | Atomic Positions (x, y, z) | Reference |
|---|---|---|---|---|---|---|---|
| α | 2.93 | - | 4.60 | Hexagonal | P63/mmc | (1/3, 2/3, 1/4) (2/3, 1/3, 3/4) | [21] |
| β | 3.23 | - | - | Cubic | Imm | (0,0,0) (1/2, 1/2, 1/2) | [21] |
| ω | 4.55 | - | 2.78 | Hexagonal | P6/mmm | (0,0,0) (1/3, 2/3, 1/3 + zω) (2/3, 1/3, 2/3-zω); zω = 1/6 | [22] |
| α” | 3.00 | 4.97 | 4.56 | Orthorhombic | Cmcm | (0, y, 1/4) (0, 1-y, 3/4) (1/2, ½ + y, 1/4) (1/2, 1/2-y, 3/4); y0 = 0.18 | [23] |
| Area | Composition (wt %) | |||||
|---|---|---|---|---|---|---|
| Ti | Al | Mo | V | Cr | Zr | |
| Dark | 82.6 | 5.2 | 4.3 | 4.5 | 2.4 | 1.0 |
| Bright | 79.9 | 5.5 | 5.6 | 5.0 | 2.9 | 1.1 |
| Area | Composition (wt %) | |||||
|---|---|---|---|---|---|---|
| Ti | Al | Mo | V | Cr | Zr | |
| Dark | 81.4 | 5.5 | 4.8 | 4.8 | 2.6 | 0.9 |
| Bright | 80.2 | 5.7 | 4.8 | 5.1 | 3.1 | 1.2 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paiotti Marcondes Guimarães, R.; Callegari, B.; Warchomicka, F.; Aristizabal, K.; Soldera, F.; Mücklich, F.; Cavalcanti Pinto, H. In Situ Analysis of the Phase Transformation Kinetics in the β-Water-Quenched Ti-5Al-5Mo-5V-3Cr-1Zr Alloy during Ageing after Fast Heating. Quantum Beam Sci. 2020, 4, 12. https://doi.org/10.3390/qubs4010012
Paiotti Marcondes Guimarães R, Callegari B, Warchomicka F, Aristizabal K, Soldera F, Mücklich F, Cavalcanti Pinto H. In Situ Analysis of the Phase Transformation Kinetics in the β-Water-Quenched Ti-5Al-5Mo-5V-3Cr-1Zr Alloy during Ageing after Fast Heating. Quantum Beam Science. 2020; 4(1):12. https://doi.org/10.3390/qubs4010012
Chicago/Turabian StylePaiotti Marcondes Guimarães, Rafael, Bruna Callegari, Fernando Warchomicka, Katherine Aristizabal, Flavio Soldera, Frank Mücklich, and Haroldo Cavalcanti Pinto. 2020. "In Situ Analysis of the Phase Transformation Kinetics in the β-Water-Quenched Ti-5Al-5Mo-5V-3Cr-1Zr Alloy during Ageing after Fast Heating" Quantum Beam Science 4, no. 1: 12. https://doi.org/10.3390/qubs4010012
APA StylePaiotti Marcondes Guimarães, R., Callegari, B., Warchomicka, F., Aristizabal, K., Soldera, F., Mücklich, F., & Cavalcanti Pinto, H. (2020). In Situ Analysis of the Phase Transformation Kinetics in the β-Water-Quenched Ti-5Al-5Mo-5V-3Cr-1Zr Alloy during Ageing after Fast Heating. Quantum Beam Science, 4(1), 12. https://doi.org/10.3390/qubs4010012








