In Situ Coherent X-ray Diffraction during Three-Point Bending of a Au Nanowire: Visualization and Quantification
Abstract
1. Introduction
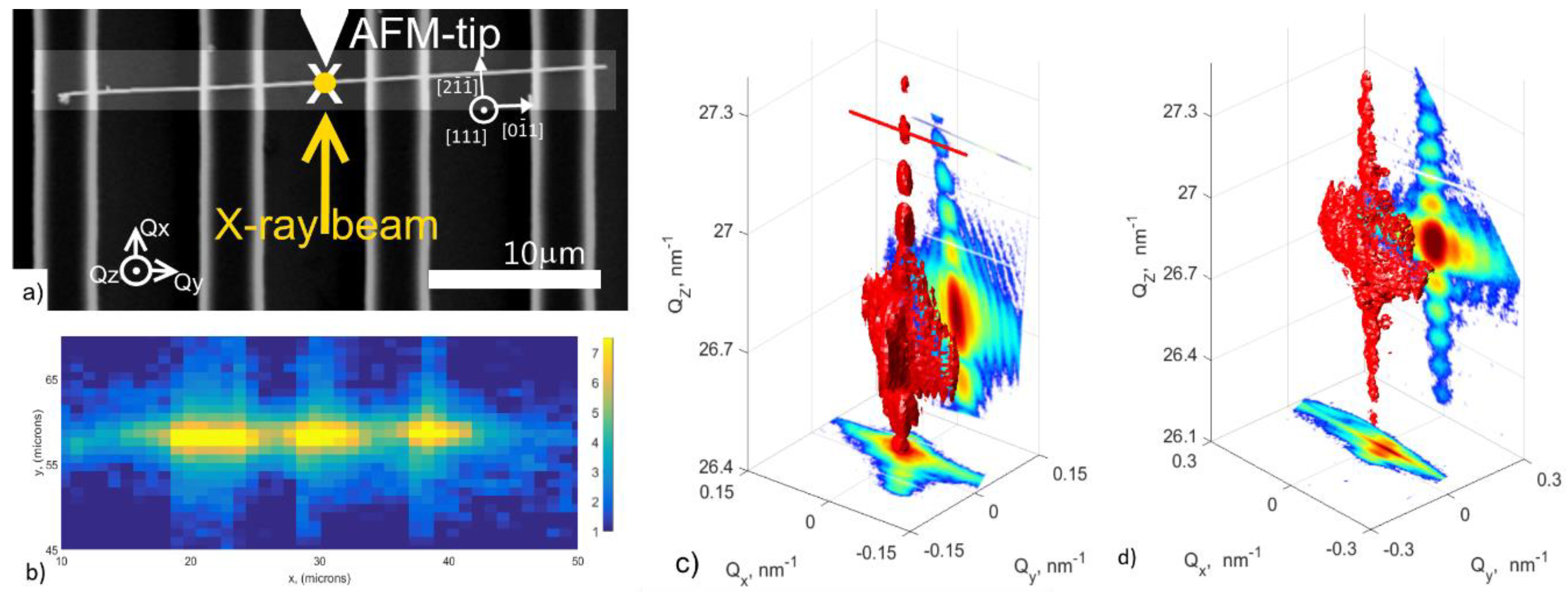
2. Materials and Methods
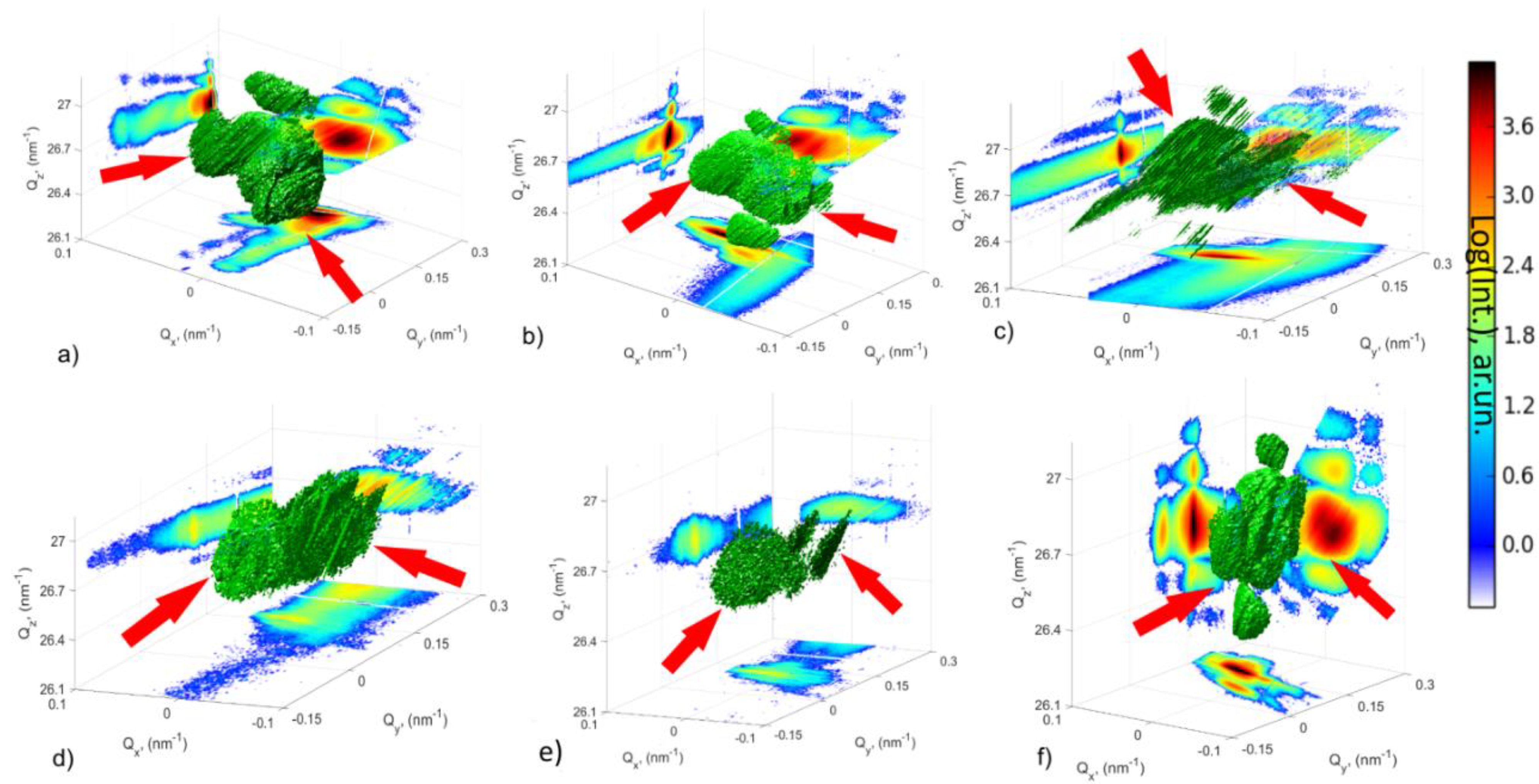
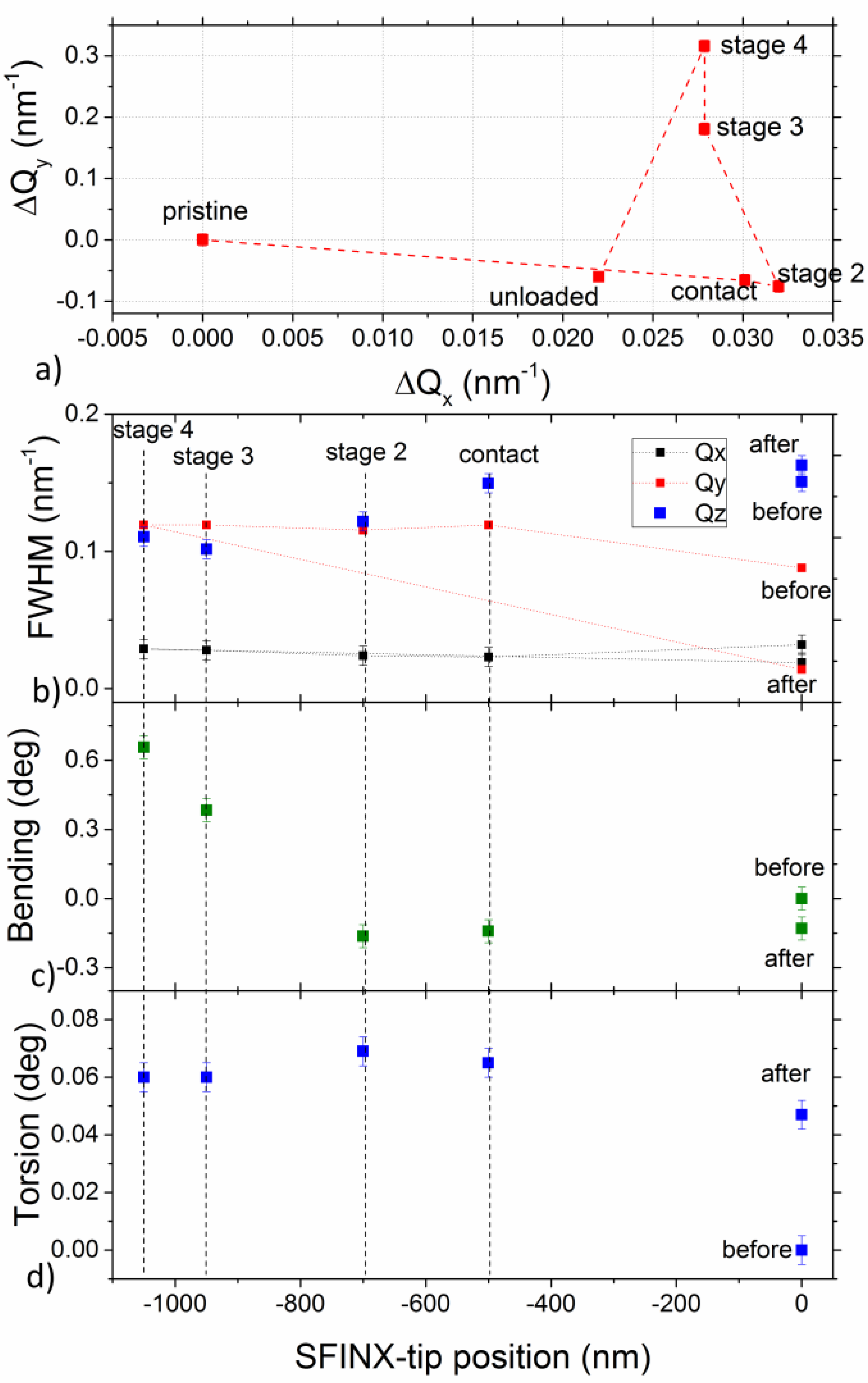
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gall, Z.; Zhong, X.; Schulman, D.; Kang, M.; Razavieh, A.; Mayer, T. Titanium dioxide nanowire sensor array integration on CMOS platform using deterministic assembly. Nanotechnology 2017, 28, 265501. [Google Scholar] [CrossRef] [PubMed]
- Yao, S.; Myers, A.; Malhotra, A.; Lin, F.; Bozkurt, A.; Muth, J.; Zhu, Y. A Wearable Hydration Sensor with Conformal Nanowire Electrodes. Adv. Healthc. Mater. 2017, 6, 1601159. [Google Scholar] [CrossRef] [PubMed]
- Yan, W.; Qu, Y.; Das Gupta, T.; Darga, A.; Nguyên, D.; Page, A.; Rossi, M.; Ceriotti, M.; Sorin, F. Semiconducting Nanowire-Based Optoelectronic Fibers. Adv. Mater. 2017, 2017 29, 1700681. [Google Scholar] [CrossRef]
- Shainline, J.; Buckley, S.; Mirin, R.; Nam, S. Superconducting Optoelectronic Circuits for Neuromorphic Computing. Phys. Rev. Appl. 2017, 7, 034013. [Google Scholar] [CrossRef]
- Mercier de Lépinay, L.; Pigeau, B.; Besga, B.; Vincent, P.; Poncharal, P.; Arcizet, O. A universal and ultrasensitive vectorial nanomechanical sensor for imaging 2D force field. Nat. Nanotechnol. 2017, 12, 156. [Google Scholar] [CrossRef] [PubMed]
- Uchic, M.D.; Dimiduk, D.M.; Florando, J.N.; Nix, W.D. Sample dimensions influence strength and crystal plasticity. Science 2004, 986–989. [Google Scholar] [CrossRef] [PubMed]
- Clark, J.; Ihli, J.; Schenk, A.; Kim, Y.; Kulak, A.; Campbell, J.; Nisbet, G.; Meldrum, F.; Robinson, I. Three-dimensional imaging of dislocation propagation during crystal growth and dissolution. Nat. Mater. 2015, 14, 780–784. [Google Scholar] [CrossRef] [PubMed]
- Hintsala, E.; Kiener, D.; Jackson, J.; Gerberich, W. In-situ measurements of free-standing, ultra-thin film crackingin bending. Exp. Mech. 2015, 55, 1681–1690. [Google Scholar] [CrossRef]
- Zou, Y.; Wheeler, J.; Ma, H.; Okle, P.; Spolenak, R. Nanocrystaline high-entropy alloys: A new paradigm in high-temperature strength and stability. Nano Lett. 2017, 17, 1569–1574. [Google Scholar] [CrossRef] [PubMed]
- Chahine, G.A.; Zoellner, M.H.; Richard, M.I.; Guha, S.; Reich, C.; Zaumseil, P.; Capellini, G.; Schroeder, T.; Schülli, T.U. Strain and lattice orientation distribution in SiN/Ge complementary metal-oxide-semiconductor compatible light emitting microstructures by quick X-ray nano-diffraction microscopy. Appl. Phys. Lett. 2015, 106, 071902. [Google Scholar] [CrossRef]
- Cornelius, T.W.; Thomas, O. Progress of in-situ synchrotron X-ray diffraction studies on the mechanical behavior of materials at small scale. Prog. Mater. Sci. 2018, 94, 384–434. [Google Scholar] [CrossRef]
- Lee, S.; Im, J.; Yoo, Y.; Bitzek, E.; Kiener, D.; Richter, G.; Kim, B.; Oh, S.H. Reversible cyclic deformation mechanism of gold nanowires by twinning-detwinning transition evidenced from in-situ TEM. Nat. Commun. 2014, 5, 3033. [Google Scholar] [CrossRef] [PubMed]
- Maaß, R.; Van Petegem, S.; Ma, D.; Zimmermann, J.; Grolimund, D.; Roters, F.; Van Swygenhoven, H.; Raabe, D. Smaller is stronger: The effect of strain hardening. Acta Mater. 2009, 57, 5996–6005. [Google Scholar] [CrossRef]
- Ren, Z.; Cornelius, T.W.; Leclere, C.; Davydok, A.; Micha, J.-S.; Robach, O.; Ullrich, O.; Richter, G.; Thomas, O. Three-point bending behavior of Au nanowires studied by in situ Laue microdiffraction. J. Appl. Phys. 2018, in press. [Google Scholar] [CrossRef]
- Bouscaud, D.; Morawiec, A.; Pesci, R.; Berveiller, S.; Patoor, E. Strain resolution of scanning electron microscopy based Kossel microdiffraction. J. Appl. Cryst. 2014, 47, 1699–1707. [Google Scholar] [CrossRef]
- Oku, T. Direct analysis of advanced nanomaterials by high-resolution electron microscopy. Nanotechnol. Rev. 2012, 1, 389–425. [Google Scholar] [CrossRef]
- Biermanns, A.; Breuer, S.; Trampert, A.; Davydok, A.; Geelhaar, L.; Pietsch, U. Strain accomodation in Ga-assisted GaAs nanowires grown on silicon (111). Nanotechnology 2012, 23, 305703. [Google Scholar] [CrossRef] [PubMed]
- Labat, S.; Richard, M.-I.; Dupraz, M.; Gailhanou, M.; Beutier, G.; Verdier, M.; Mastropietro, F.; Cornelius, T.W.; Schülli, T.U.; Eymery, J.; et al. Inversion domain boundaries in GaN wires revealed by coherent bragg imaging. ACS Nano 2015, 9, 9210–9216. [Google Scholar] [CrossRef] [PubMed]
- Dupraz, M.; Beutier, G.; Cornelius, T.W.; Parry, G.; Ren, Z.; Labat, S.; Richard, M.-I.; Chahine, G.A.; Kovalenko, O.; Boissieu, M.D.; et al. 3D imaging of a dislocation loop at the onset of plasticity in an indented nanocrystal. Nano Lett. 2017, 17, 6696–6701. [Google Scholar] [CrossRef] [PubMed]
- Shin, J.; Cornelius, T.W.; Labat, S.; Lauraux, F.; Richard, M.-I.; Richter, G.; Blanchard, N.; Gianola, D.C.; Thomas, O. In-situ bragg coherent X-ray diffraction during tensile testing of an individual Au nanowire. J. Appl. Cryst. 2018, 51, 781–788. [Google Scholar] [CrossRef]
- Cornelius, T.W.; Davydok, A.; Jacques, V.L.R.; Grifone, R.; Schülli, T.; Richard, M.-I.; Beutier, G.; Verdier, M.; Metzger, T.H.; Pietsch, U.; et al. In-situ three-dimensional reciprocal-space mapping during mechanical deformation. J. Synchrotron Radiat. 2012, 19, 688–694. [Google Scholar] [CrossRef] [PubMed]
- Cha, W.; Ulvestad, A.; Allain, M.; Chamard, V.; Harder, R.; Leake, S.J.; Maser, J.; Fuoss, P.H.; Hruszekewycz, S.O. Three dimensional variable-wavelength X-ray bragg coherent diffraction imaging Phys. Rev. Lett. 2016, 117, 225501. [Google Scholar] [CrossRef] [PubMed]
- Ren, Z.; Mastropietro, F.; Langlais, S.; Davydok, A.; Richard, M.-I.; Thomas, O.; Dupraz, M.; Verdier, M.; Beutier, G.; Boesecke, P.; et al. Scanning force microscope for in situ nanofocused X-ray diffraction studies. J. Synchrotron Radiat. 2014, 21, 1128–1133. [Google Scholar] [CrossRef] [PubMed]
- Leclere, C.; Cornelius, T.W.; Ren, Z.; Davydok, A.; Micha, J.-S.; Robach, O.; Richter, G.; Belliard, L. Thomas O. In-situ bending of an Au nanowire monitored by micro Laue diffraction. J. Appl. Cryst. 2015, 48, 291–296. [Google Scholar] [CrossRef] [PubMed]
- Richter, G.; Hillerich, K.; Gianola, D.S.; Mönig, R.; Kraft, O.; Volkert, C.A. Ultrahigh strength single crystalline nanowhiskers grown by physical vapor deposition. Nano Lett. 2009, 9, 3048–3052. [Google Scholar] [CrossRef] [PubMed]
- Leake, S.J.; Favre-Nicolin, V.; Zatterin, E.; Richard, M.-I.; Fernandez, S.; Chahine, G.; Zhou, T.; Boesecke, P.; Djazouli, H.; Schülli, T.U. Coherent nanoscale X-ray probe for crystal interrogation at ID01, ESRF—The European Synchrotron. Mater. Des. 2017, 119, 470–471. [Google Scholar] [CrossRef]
- Schülli, T.U.; Leake, S.J. X-ray nanobeam diffraction imaging of materials. Curr. Opin. Solid State Mater. Sci. 2018. [Google Scholar] [CrossRef]
- Mastropietro, F.; Carbone, D.; Diaz, A.; Eymery, J.; Sentenac, A.; Metzger, T.H.; Chamard, V.; Favre-Nicolin, V. Coheren X-ray wavefront reconstruction of a partially illuminated Fresnel zone plate. Opt. Express 2011, 19, 19223. [Google Scholar] [CrossRef] [PubMed]
- Leclere, C.; Cornelius, T.W.; Ren, Z.; Robach, O.; Micha, J.-S.; Davydok, A.; Ullrich, O.; Richter, G.; Thomas, O. KB-scanning of X-ray beam for Laue microdiffraction on accelero-phobic samples: Application to in situ mechanically loaded nanowires. J. Synchrotron Radiat. 2016, 23, 1395–1400. [Google Scholar] [CrossRef] [PubMed]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Davydok, A.; Cornelius, T.W.; Ren, Z.; Leclere, C.; Chahine, G.; Schülli, T.; Lauraux, F.; Richter, G.; Thomas, O. In Situ Coherent X-ray Diffraction during Three-Point Bending of a Au Nanowire: Visualization and Quantification. Quantum Beam Sci. 2018, 2, 24. https://doi.org/10.3390/qubs2040024
Davydok A, Cornelius TW, Ren Z, Leclere C, Chahine G, Schülli T, Lauraux F, Richter G, Thomas O. In Situ Coherent X-ray Diffraction during Three-Point Bending of a Au Nanowire: Visualization and Quantification. Quantum Beam Science. 2018; 2(4):24. https://doi.org/10.3390/qubs2040024
Chicago/Turabian StyleDavydok, Anton, Thomas W. Cornelius, Zhe Ren, Cedric Leclere, Gilbert Chahine, Tobias Schülli, Florian Lauraux, Gunther Richter, and Olivier Thomas. 2018. "In Situ Coherent X-ray Diffraction during Three-Point Bending of a Au Nanowire: Visualization and Quantification" Quantum Beam Science 2, no. 4: 24. https://doi.org/10.3390/qubs2040024
APA StyleDavydok, A., Cornelius, T. W., Ren, Z., Leclere, C., Chahine, G., Schülli, T., Lauraux, F., Richter, G., & Thomas, O. (2018). In Situ Coherent X-ray Diffraction during Three-Point Bending of a Au Nanowire: Visualization and Quantification. Quantum Beam Science, 2(4), 24. https://doi.org/10.3390/qubs2040024






