Bond Stress Behavior of a Steel Reinforcing Bar Embedded in Geopolymer Concrete Incorporating Natural and Recycled Aggregates
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Program
2.2. Materials
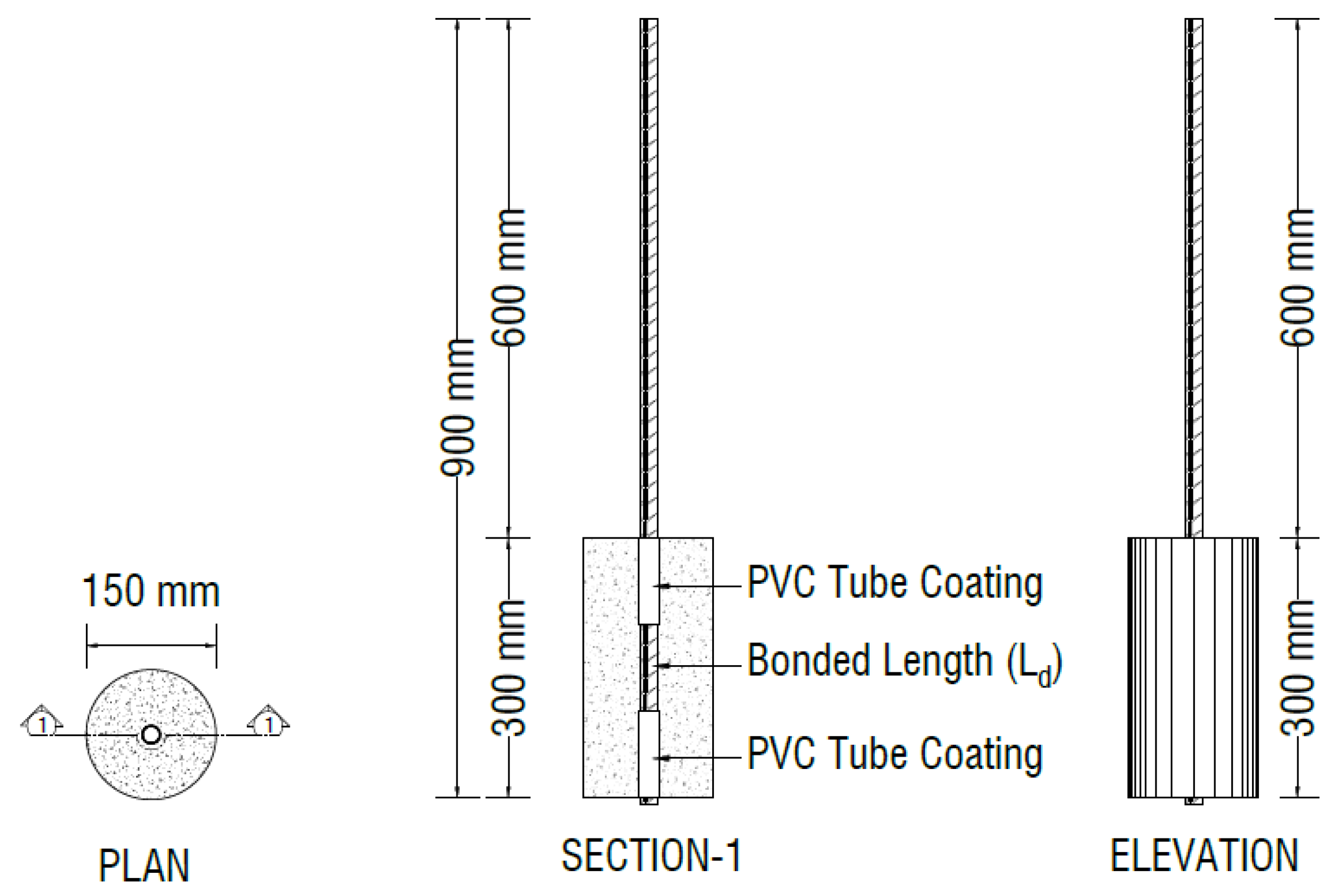
2.3. Preparation of Test Samples
2.4. Testing Procedure
3. Results and Discussion
- = Maximum failure load (N);
- = Diameter of steel bar (mm);
- = Embedment length (mm).
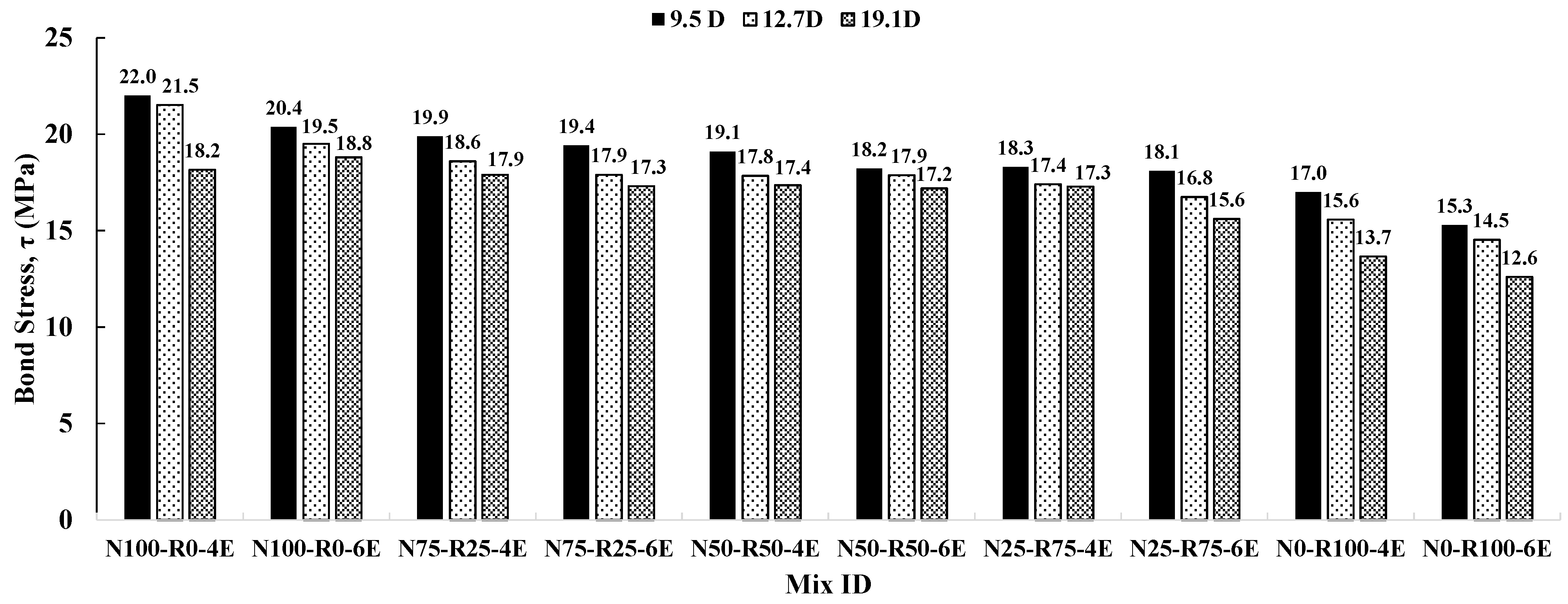
3.1. Influence of Embedment Length
3.2. Influence of Bar Diameter
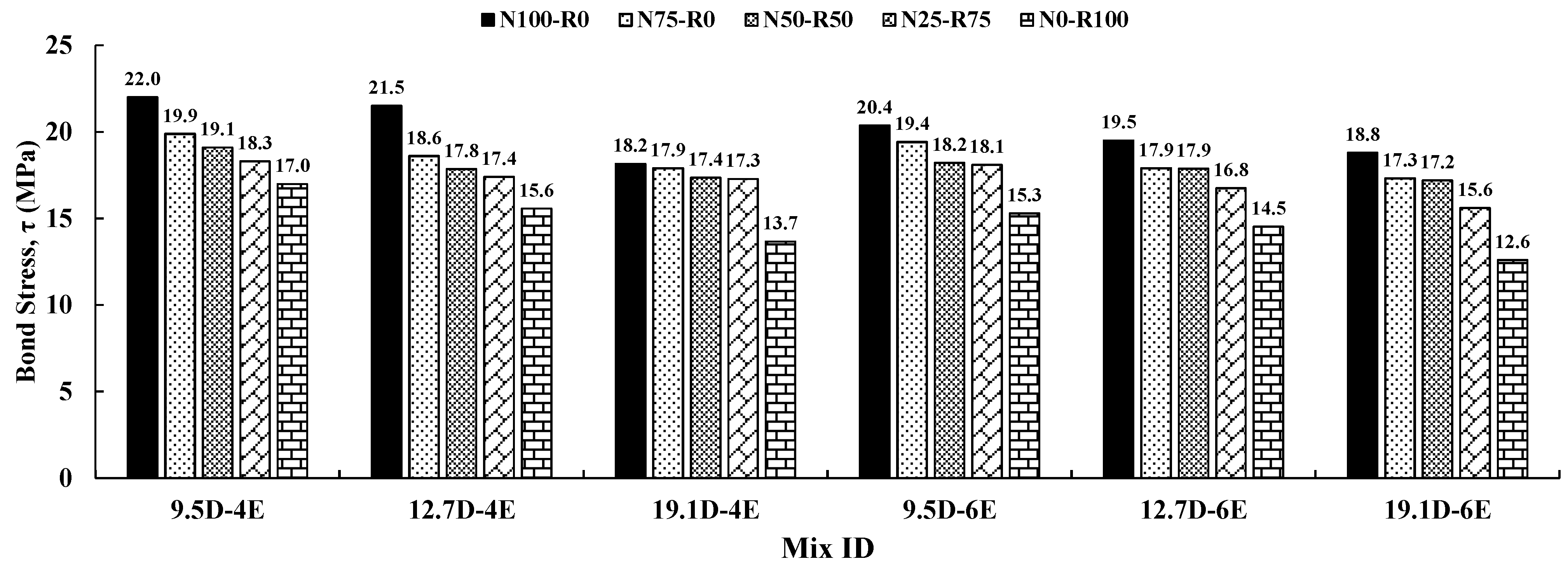
3.3. Influence of Percentage Replacements of NCA with RCA
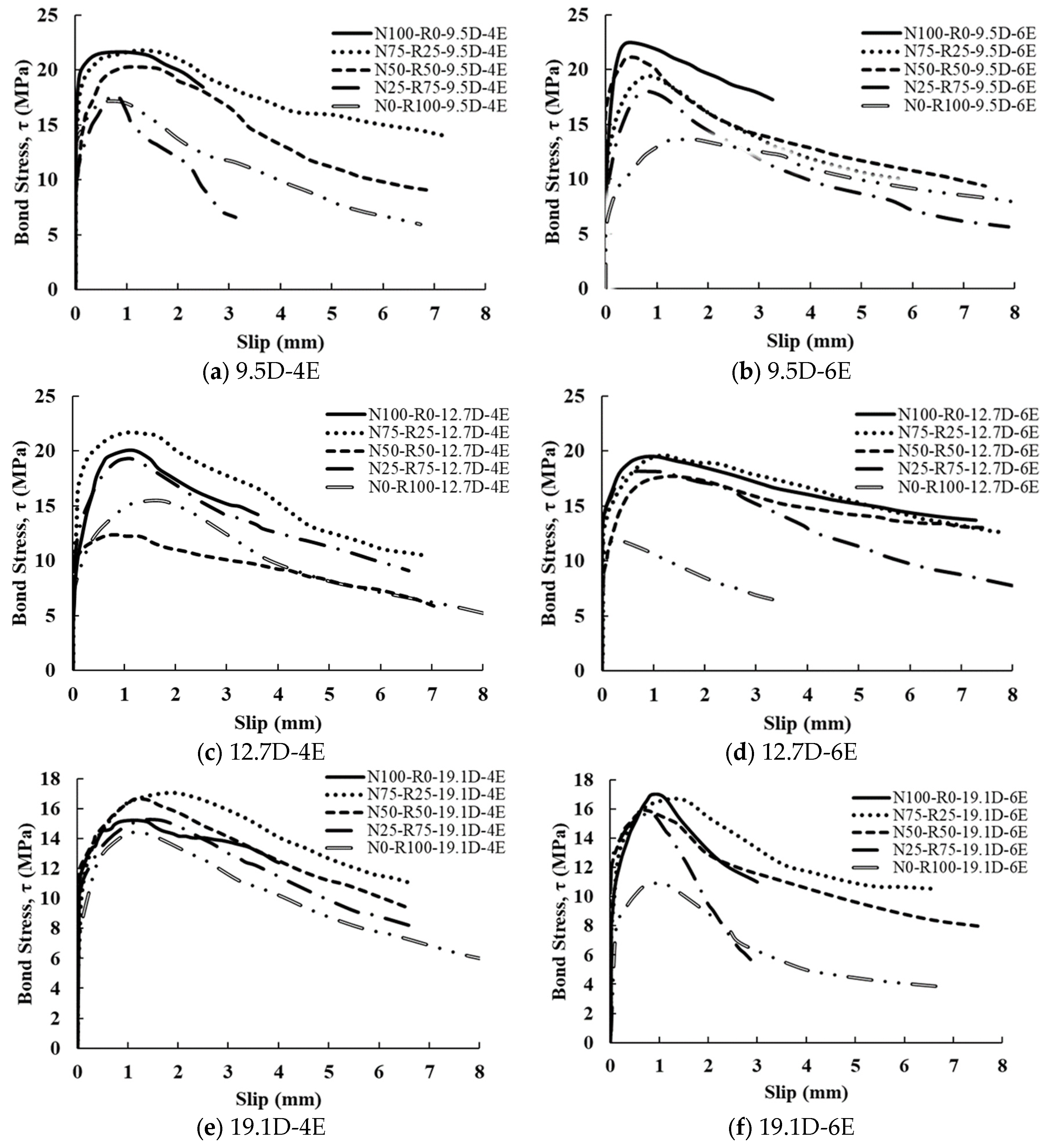
3.4. Stress–Slip Curves
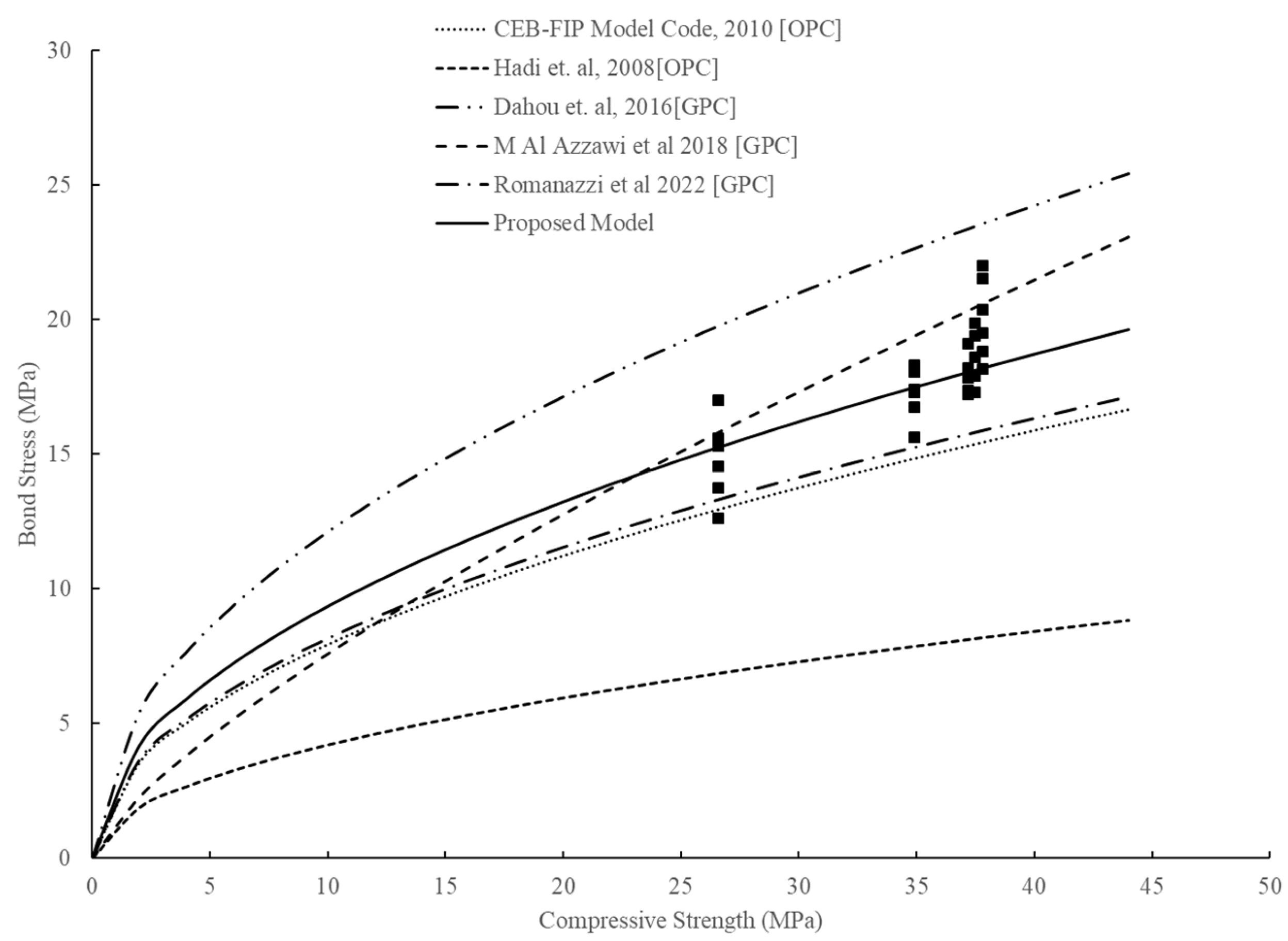
3.5. Theoretical Bond Stress Models
4. Economic Comparison
5. Environmental Assessment
6. Conclusions
- The bond stress of the CRAC mix was decreased with increasing embedment length from 4 to 6. An average decrease in bond stress of 5.1%, 4.7%, and 6.7%, respectively, was noted for 9.5 mm, 12.7 mm, and 19.1 mm diameter steel bars, with increasing percentage replacements of NCA with RCA from 0 to 100%. The decrease in bond stress with increasing embedment length was attributed to the fact that longer embedment lengths distributed the load over a larger contact area between the reinforcing bar and the surrounding concrete.
- The bond stress of CRAC specimens was also observed to decrease with the increasing diameter of the steel bar from 9.5 mm to 12.7 mm and 12.7 mm to 19.1 mm. An average decrease in bond stress of 5.75% and 6.84%, respectively, was noted when increasing the diameter from 9.5 mm to 12.7 mm and 12.7 mm to 19.1 mm for an embedment length of 4, with increasing percentage replacements of NCA with RCA from 0 to 100%. Similarly, an average decrease in bond stress of 5.24% and 6.20%, respectively, was noted with increasing diameter from 9.5 mm to 12.7 mm and 12.7 mm to 19.1 mm for an embedment length of 6, with increasing percentage replacements of NCA with RCA from 0 to 100%.
- The study found significant reductions in bond stress as the percentage replacement of NCA with RCA was increased from 0 to 100%. The reduction in the average bond stress was 7.63%, 3.58%, 3.84%, and 14.45%, respectively, as the percentage replacements of NCA with RCA increased from 0 to 25%, 25% to 50%, 50% to 75%, and 75% to 100%. This reduction in bond stress was attributed to the reduced mechanical properties of RCA compared to NCA, i.e., the irregularity in shape of RCA particles and the presence of adhered mortar on the RCA surface.
- The proposed model to predict the bond stress of CRAC mixes (CRAC) matched well with the experimental data and test results of existing studies.
- The CRAC mixes with varying percentage replacements exhibited 31.3% to 43.8% higher costs per cubic meter than the OPC concrete mix. However, CRAC mixes with varying percentage replacements exhibited 57.1% to 65.1% reduced e-CO2 emissions compared to the OPC concrete mix.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lehne, J.; Preston, F. Making concrete change. In Innovation in Low-Carbon Cement and Concrete; Chatham House: London, UK, 2018. [Google Scholar]
- Robayo-Salazar, R. Life cycle assessment (LCA) of an alkali-activated binary concrete based on natural volcanic pozzolan: A comparative analysis to OPC concrete. Constr. Build. Mater. 2018, 176, 103–111. [Google Scholar] [CrossRef]
- Davidovits, J. Chemistry of Geopolymeric systems, terminology. In Proceedings of the 99 Geopolymer International Conference, France, 30 June–2 July 1999; Davidovits, J., Davidovits, R., James, C., Eds.; Geopolymer Institute: Saint-Quentin, France, 1999. [Google Scholar]
- Davidovits, J. Geopolymer cement. A review. Geopolymer Inst. Tech. Pap. 2013, 21, 1–11. [Google Scholar]
- Singh, B. Geopolymer concrete: A review of some recent developments. Constr. Build. Mater. 2015, 85, 78–90. [Google Scholar] [CrossRef]
- Mo, K.H.; Alengaram, U.J.; Jumaat, M.Z. Structural performance of reinforced geopolymer concrete members: A review. Constr. Build. Mater. 2016, 120, 251–264. [Google Scholar] [CrossRef]
- Ding, Y.; Dai, J.-G.; Shi, C.-J. Mechanical properties of alkali-activated concrete: A state-of-the-art review. Constr. Build. Mater. 2016, 127, 68–79. [Google Scholar] [CrossRef]
- Ma, C.-K.; Awang, A.Z.; Omar, W. Structural and material performance of geopolymer concrete: A review. Constr. Build. Mater. 2018, 186, 90–102. [Google Scholar] [CrossRef]
- Sumajouw, M.; Rangan, B.V. Low-Calcium Fly Ash-Based Geopolymer Concrete: Reinforced Beams and Columns. 2006. Available online: http://hdl.handle.net/20.500.11937/23928 (accessed on 15 August 2022).
- Sujatha, T.; Kannapiran, K.; Nagan, S. Strength assessment of heat cured geopolymer concrete slender column. Asian J. Civ. Eng. (Build. Hous.) 2012, 13, 635–646. [Google Scholar]
- Xiao, J.; Wang, C.; Ding, T.; Akbarnezhad, A. A recycled aggregate concrete high-rise building: Structural performance and embodied carbon footprint. J. Clean. Prod. 2018, 199, 868–881. [Google Scholar] [CrossRef]
- Ahmed, H. Recycled aggregate concrete from large-scale production to sustainable field application. Constr. Build. Mater. 2020, 262, 119979. [Google Scholar] [CrossRef]
- Price, W.H. Factors influencing concrete strength. J. Proc. 1951, 47, 417–432. [Google Scholar] [CrossRef]
- Sarker, P.K. Bond strength of reinforcing steel embedded in fly ash-based geopolymer concrete. Mater. Struct. 2011, 44, 1021–1030. [Google Scholar] [CrossRef]
- Castel, A.; Foster, S.J. Bond strength between blended slag and Class F fly ash geopolymer concrete with steel reinforcement. Cem. Concr. Res. 2015, 72, 48–53. [Google Scholar] [CrossRef]
- Shen, D.; Shi, X.; Zhang, H.; Duan, X.; Jiang, G. Experimental study of early-age bond behavior between high strength concrete and steel bars using a pull-out test. Constr. Build. Mater. 2016, 113, 653–663. [Google Scholar] [CrossRef]
- Boopalan, C.; Rajamane, N.P.; Jeyalakshmi, R. Studies on adhesive bond strength of steel reinforcing bars with fly ash based-ambient cured geopolymer concrete. In AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2018; Volume 2030, No. 1. [Google Scholar]
- Hosseini, S.A.; Bagheri, M. The effect of fly ash on the bond strength of steel reinforcement and concrete. Iran. J. Sci. Technol. Trans. Civ. Eng. 2022, 46, 285–292. [Google Scholar] [CrossRef]
- Waqas, R.M.; Butt, F.; Zhu, X.; Jiang, T.; Tufail, R.F. A comprehensive study on the factors affecting the workability and mechanical properties of ambient cured fly ash and slag based geopolymer concrete. Appl. Sci. 2021, 11, 8722. [Google Scholar] [CrossRef]
- Vinothini, M. Bond strength behaviour of geopolymer concrete. Malays. J. Civ. Eng. 2015, 27, 371–381. [Google Scholar]
- Le, T.A.; Nguyen, T.N.; Nguyen, K.T. Experimental, numerical, and theoretical studies of bond behavior of reinforced fly ash-based geopolymer concrete. Appl. Sci. 2022, 12, 7812. [Google Scholar] [CrossRef]
- Ganesan, N.; Indira, P.V.; Santhakumar, A. Bond behaviour of reinforcing bars embedded in steel fibre reinforced geopolymer concrete. Mag. Concr. Res. 2015, 67, 9–16. [Google Scholar] [CrossRef]
- Cui, Y.; Zhang, P.; Bao, J. Bond Stress between Steel-Reinforced Bars and Fly Ash-Based Geopolymer Concrete. Adv. Mater. Sci. Eng. 2020, 2020, 9812526. [Google Scholar] [CrossRef]
- Majain, N.; Rahman, A.B.A.; Adnan, A.; Mohamed, R.N. Bond behaviour of deformed steel bars in steel fibre high-strength self-compacting concrete. Constr. Build. Mater. 2022, 318, 125906. [Google Scholar] [CrossRef]
- Romanazzi, V.; Leone, M.; Aiello, M.A.; Pecce, M.R. Bond behavior of geopolymer concrete with steel and GFRP bars. Compos. Struct. 2022, 300, 116150. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, Y.; Zhao, D.; Tao, X.; Zhang, M. Bond stress-slip behavior of ultrahigh-strength steel bars with spiral grooves embedded in plain and steel fiber high-strength recycled aggregate concrete. J. Build. Eng. 2023, 80, 108056. [Google Scholar] [CrossRef]
- Hameed, R.; Akmal, U.; Khan, Q.S.; Cheema, M.A.; Riaz, M.R. Effect of fibers on the bond behavior of deformed steel bar embedded in recycled aggregate concrete. Mehran Univ. Res. J. Eng. Technol. 2020, 39, 846–858. [Google Scholar] [CrossRef]
- Gao, D.; Huang, Y.; Chen, G.; Yang, L. Bond stress distribution analysis between steel bar and steel fiber reinforced concrete using midpoint stress interpolation method. Constr. Build. Mater. 2020, 260, 119866. [Google Scholar] [CrossRef]
- Xiao, J.; Falkner, H. Bond behaviour between recycled aggregate concrete and steel rebars. Constr. Build. Mater. 2007, 21, 395–401. [Google Scholar] [CrossRef]
- Prince, M.J.R.; Singh, B. Bond behaviour of deformed steel bars embedded in recycled aggregate concrete. Constr. Build. Mater. 2013, 49, 852–862. [Google Scholar] [CrossRef]
- Ghafoor, M.T.; Khan, Q.S.; Qazi, A.U.; Sheikh, M.N.; Hadi, M.N.S. Influence of alkaline activators on the mechanical properties of fly ash based geopolymer concrete cured at ambient temperature. Constr. Build. Mater. 2021, 273, 121752. [Google Scholar] [CrossRef]
- ASTM-C188; Standard Test Method for Density of Hydraulic Cement 2017. American Society of Testing and Materials: West Conshohocken, PA, USA, 2017.
- ASTM-C114; Standard Test Methods for Chemical Analysis 2023. American Society of Testing and Materials: West Conshohocken, PA, USA, 2023.
- ASTM-C618; Standard Specification for Coal Fly Ash and Raw or Calcined Natural Pozzolan for Use in Concrete 2022. American Society of Testing and Materials: West Conshohocken, PA, USA, 2022.
- BS 812-110; Methods for Determination of Aggregate Crushing Value (ACV). British Standards Institution: London, UK, 1990.
- BS 812-112; Methods for Determination of Aggregate Impact Value (AIV). British Standards Institution: London, UK, 1990.
- ASTM-C127; Standard Test Method for Relative Density (Specific Gravity) and Absorption of Coarse Aggregate 2015. American Society of Testing and Materials: West Conshohocken, PA, USA, 2015.
- ASTM-C29; Standard Test Method for Bulk Density (“Unit Weight”) and Voids in Aggregate 1997. American Society of Testing and Materials: West Conshohocken, PA, USA, 1997.
- ASTM-C136; Standard Test Method for Sieve Analysis of Fine and Coarse Aggregates 2019. American Society of Testing and Materials: West Conshohocken, PA, USA, 2019.
- ASTM-A615; Standard Specification for Deformed and Plain Carbon-Steel Bars for Concrete Reinforcement 2022. American Society of Testing and Materials: West Conshohocken, PA, USA, 2022.
- ASTM-C143; Standard Test Method for Slump of Hydraulic-Cement Concrete 2020. American Society of Testing and Materials: West Conshohocken, PA, USA, 2020.
- ASTM-C900; Standard Test Method for Pullout Strength of Hardened Concrete 2019. American Society of Testing and Materials: West Conshohocken, PA, USA, 2019.
- ASTM-C39; Compressive Strength of Concrete Cylinders 2020. American Society of Testing and Materials: West Conshohocken, PA, USA, 2020.
- Kathirvel, P.; Thangavelu, M.; Gopalan, R.; Kaliyaperumal, S.R.M. Bond characteristics of reinforcing steel embedded in geopolymer concrete. IOP Conf. Ser. Earth Environ. Sci. 2017, 80, 012001. [Google Scholar] [CrossRef]
- Kim, J.S.; Park, J. An experimental evaluation of development length of reinforcements embedded in geopolymer concrete. Appl. Mech. Mater. 2014, 578, 441–444. [Google Scholar] [CrossRef]
- Zhang, J.; Tao, X.; Li, X.; Zhang, Y.; Liu, Y. Analytical and experimental investigation of the bond behavior of confined high-strength recycled aggregate concrete. Constr. Build. Mater. 2022, 315, 125636. [Google Scholar] [CrossRef]
- Jiang, J.; Yang, H.; Deng, Z.; Li, Z. Bond performance of deformed rebar embedded in recycled aggregate concrete subjected to repeated loading after freeze–thaw cycles. Constr. Build. Mater. 2022, 318, 125954. [Google Scholar] [CrossRef]
- Walraven, J.C.; Bigaj-van Vliet, A. The 2010 fib Model Code for Structural Concrete: A new approach to structural engineering. Struct. Concr. 2011, 12, 139–147. [Google Scholar] [CrossRef]
- Dahou, Z.; Castel, A.; Noushini, A. Prediction of the steel-concrete bond strength from the compressive strength of Portland cement and geopolymer concretes. Constr. Build. Mater. 2016, 119, 329–342. [Google Scholar] [CrossRef]
- Fernandez-Jimenez, A.M.; Palomo, A.; Lopez-Hombrados, C. Engineering properties of alkali-activated fly ash concrete. ACI Mater. J. 2006, 103, 106. [Google Scholar]
- Hadi, M.N. Bond of high strength concrete with high strength reinforcing steel. Open Civ. Eng. J. 2008, 2, 143–147. [Google Scholar] [CrossRef]
- Al-Azzawi, M.; Yu, T.; Hadi, M.N. Factors affecting the bond strength between the fly ash-based geopolymer concrete and steel reinforcement. Structures 2018, 14, 262–272. [Google Scholar] [CrossRef]
- Tauqir, M.; Qazi, A.U.; Khan, Q.S.; Munir, M.J.; Kazmi, S.M.S. Shear behavior of geopolymer concrete slender beams. Buildings 2023, 13, 1191. [Google Scholar] [CrossRef]










| Element (%) | CaO | MgO | SiO2 | SO3 | Al2O3 | Fe2O3 | L.O.I |
| Fly Ash | 7.65 | 2.12 | 79.15 | 0.32 | 3.01 | 2.19 | 3.1 |
| Diameter of Steel Bar (mm) | Yield Strength (MPa) | Ultimate Strength (MPa) | Percentage Elongation |
|---|---|---|---|
| 9.5 | 477.3 | 640.6 | 12 |
| 12.7 | 455.0 | 672.1 | 13 |
| 19.1 | 438.0 | 708.0 | 10.5 |
| Pull-Out ID | NCA (%) | RCA (%) | Bar Diameter (mm) | Embedment Length |
|---|---|---|---|---|
| N100-R0-9.5D-4E | 100 | 0 | 9.5 | |
| N100-R0-12.7D-4E | 12.7 | |||
| N100-R0-19.1D-4E | 19.1 | |||
| N100-R0-9.5D-6E | 9.5 | |||
| N100-R0-12.7D-6E | 12.7 | |||
| N100-R0-19.1D-6E | 19.1 | |||
| N75-R25-9.5D-4E | 75 | 25 | 9.5 | |
| N75-R25-12.7D-4E | 12.7 | |||
| N75-R25-19.1D-4E | 19.1 | |||
| N75-R25-9.5D-6E | 9.5 | |||
| N75-R25-12.7D-6E | 12.7 | |||
| N75-R25-19.1D-6E | 19.1 | |||
| N50-R50-9.5D-4E | 50 | 50 | 9.5 | |
| N50-R50-12.7D-4E | 12.7 | |||
| N50-R50-19.1D-4E | 19.1 | |||
| N50-R50-9.5D-6E | 9.5 | |||
| N50-R50-12.7D-6E | 12.7 | |||
| N50-R50-19.1D-6E | 19.1 | |||
| N25-R75-9.5D-4E | 25 | 75 | 9.5 | |
| N25-R75-12.7D-4E | 12.7 | |||
| N25-R75-19.1D-4E | 19.1 | |||
| N25-R75-9.5D-6E | 9.5 | |||
| N25-R75-12.7D-6E | 12.7 | |||
| N25-R75-19.1D-6E | 19.1 | |||
| N0-R100-9.5D-4E | 0 | 100 | 9.5 | |
| N0-R100-12.7D-4E | 12.7 | |||
| N0-R100-19.1D-4E | 19.1 | |||
| N0-R100-9.5D-6E | 9.5 | |||
| N0-R100-12.7D-6E | 12.7 | |||
| N0-R100-19.1D-6E | 19.1 |
| Mix ID | Embedment Length (mm) | Pull-Out Load (kN) | Bond Stress (MPa) | Average Bond Stress (MPa) |
|---|---|---|---|---|
| N100-R0-9.5D-4E-1 | 38 | 24.5 | 21.60 | 22.00 |
| N100-R0-9.5D-4E-2 | 25.4 | 22.40 | ||
| N100-R0-12.7D-4E-1 | 50.8 | 40.7 | 20.09 | 21.51 |
| N100-R0-12.7D-4E-2 | 46.5 | 22.94 | ||
| N100-R0-19.1D-4E-1 | 76.4 | 69.8 | 15.23 | 18.15 |
| N100-R0-19.1D-4E-2 | 96.7 | 21.08 | ||
| N100-R0-9.5D-6E-1 | 57 | 31.1 | 18.26 | 20.37 |
| N100-R0-9.5D-6E-2 | 38.3 | 22.48 | ||
| N100-R0-12.7D-6E-1 | 76.2 | 56.3 | 18.53 | 19.50 |
| N100-R0-12.7D-6E-2 | 62.3 | 20.47 | ||
| N100-R0-19.1D-6E-1 | 114.6 | 124.7 | 18.13 | 18.80 |
| N100-R0-19.1D-6E-2 | 133.9 | 19.47 | ||
| N75-R25-9.5D-4E-1 | 38 | 24.5 | 21.64 | 19.88 |
| N75-R25-9.5D-4E-2 | 20.6 | 18.12 | ||
| N75-R25-12.7D-4E-1 | 50.8 | 42.2 | 20.82 | 18.60 |
| N75-R25-12.7D-4E-2 | 33.2 | 16.37 | ||
| N75-R25-19.1D-4E-1 | 76.4 | 78.2 | 17.06 | 17.89 |
| N75-R25-19.1D-4E-2 | 85.8 | 18.72 | ||
| N75-R25-9.5D-6E-1 | 57 | 31.6 | 18.55 | 19.41 |
| N75-R25-9.5D-6E-2 | 34.5 | 20.28 | ||
| N75-R25-12.7D-6E-1 | 76.2 | 56.1 | 18.46 | 17.90 |
| N75-R25-12.7D-6E-2 | 52.7 | 17.34 | ||
| N75-R25-19.1D-6E-1 | 114.6 | 123.0 | 17.88 | 17.30 |
| N75-R25-19.1D-6E-2 | 115.0 | 16.72 | ||
| N50-R50-9.5D-4E-1 | 38 | 21.1 | 18.62 | 19.10 |
| N50-R50-9.5D-4E-2 | 22.2 | 19.57 | ||
| N50-R50-12.7D-4E-1 | 50.8 | 34.5 | 17.02 | 17.84 |
| N50-R50-12.7D-4E-2 | 37.8 | 18.66 | ||
| N50-R50-19.1D-4E-1 | 76.4 | 82.3 | 17.96 | 17.36 |
| N50-R50-19.1D-4E-2 | 76.8 | 16.75 | ||
| N50-R50-9.5D-6E-1 | 57 | 29.1 | 17.08 | 18.21 |
| N50-R50-9.5D-6E-2 | 32.9 | 19.34 | ||
| N50-R50-12.7D-6E-1 | 76.2 | 55.5 | 18.25 | 17.87 |
| N50-R50-12.7D-6E-2 | 53.2 | 17.50 | ||
| N50-R50-19.1D-6E-1 | 114.6 | 126.3 | 18.36 | 17.20 |
| N50-R50-19.1D-6E-2 | 110.3 | 16.04 | ||
| N25-R75-9.5D-4E-1 | 38 | 21.2 | 18.72 | 18.30 |
| N25-R75-9.5D-4E-2 | 20.3 | 17.88 | ||
| N25-R75-12.7D-4E-1 | 50.8 | 31.2 | 15.38 | 17.40 |
| N25-R75-12.7D-4E-2 | 39.4 | 19.42 | ||
| N25-R75-19.1D-4E-1 | 76.4 | 69.2 | 15.09 | 17.29 |
| N25-R75-19.1D-4E-2 | 89.3 | 19.48 | ||
| N25-R75-9.5D-6E-1 | 57 | 32.2 | 18.93 | 18.09 |
| N25-R75-9.5D-6E-2 | 29.4 | 17.25 | ||
| N25-R75-12.7D-6E-1 | 76.2 | 55.0 | 18.10 | 16.75 |
| N25-R75-12.7D-6E-2 | 46.8 | 15.40 | ||
| N25-R75-19.1D-6E-1 | 114.6 | 106.2 | 15.44 | 15.61 |
| N25-R75-19.1D-6E-2 | 108.5 | 15.77 | ||
| N0-R100-9.5D-4E-1 | 38 | 19.6 | 17.24 | 16.99 |
| N0-R100-9.5D-4E-2 | 19.0 | 16.73 | ||
| N0-R100-12.7D-4E-1 | 50.8 | 32.9 | 16.21 | 15.57 |
| N0-R100-12.7D-4E-2 | 30.3 | 14.93 | ||
| N0-R100-19.1D-4E-1 | 76.4 | 65.0 | 14.18 | 13.66 |
| N0-R100-19.1D-4E-2 | 60.3 | 13.14 | ||
| N0-R100-9.5D-6E-1 | 57 | 26.5 | 15.58 | 15.28 |
| N0-R100-9.5D-6E-2 | 25.5 | 14.99 | ||
| N0-R100-12.7D-6E-1 | 76.2 | 45.1 | 14.83 | 14.52 |
| N0-R100-12.7D-6E-2 | 43.2 | 14.21 | ||
| N0-R100-19.1D-6E-1 | 114.6 | 75.3 | 10.95 | 12.60 |
| N0-R100-19.1D-6E-1 | 98.0 | 14.25 |
| Research Study | Concrete Type | Bond Stress Model |
|---|---|---|
| Romanazzi et al. [25] | GPC | |
| CEB-FIP, fib model code [48] | OPC | |
| Azzawi et al. [52] | GPC | |
| Dahou et al. [49] | GPC | |
| Hadi et al. [51] | OPC |
| Compressive Strength (MPa) | Predicted Bond Stress (MPa) | |||||
|---|---|---|---|---|---|---|
| Hadi et al. [51] [OPC] | CEB-FIP Model Code [48] [OPC] | Dahou et al. [49] [GPC] | Azzawi et al. [52] [GPC] | Romanazzi et al. [25] [GPC] | Proposed Model [GPC] | |
| 0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 5 | 3.0 | 5.6 | 8.6 | 4.5 | 5.8 | 6.6 |
| 10 | 4.2 | 7.9 | 12.1 | 7.6 | 8.2 | 9.4 |
| 15 | 5.2 | 9.7 | 14.8 | 10.3 | 10.0 | 11.5 |
| 20 | 5.9 | 11.2 | 17.1 | 12.8 | 11.5 | 13.2 |
| 25 | 6.7 | 12.6 | 19.2 | 15.1 | 12.9 | 14.8 |
| 30 | 7.3 | 13.7 | 21.0 | 17.3 | 14.1 | 16.2 |
| 35 | 7.9 | 14.8 | 22.7 | 19.4 | 15.3 | 17.5 |
| 40 | 8.4 | 15.9 | 24.2 | 21.5 | 16.3 | 18.7 |
| 45 | 8.9 | 16.8 | 25.7 | 23.5 | 17.3 | 19.9 |
| 50 | 9.4 | 17.7 | 27.1 | 25.4 | 18.2 | 20.9 |
| Mix ID | Fly Ash | Coarse Aggregates | Fine Aggregates | NaOH Solution | Na2SiO3 Solution | Total Cost USD/m3 | |
|---|---|---|---|---|---|---|---|
| Natural | Recycled | ||||||
| (kg/m3) | (kg/m3) | (kg/m3) | (kg/m3) | (kg/m3) | (kg/m3) | ||
| N100-R0 | 368 | 1294 | 0 | 554 | 29 | 110 | 49.5 |
| N75-R25 | 368 | 971 | 324 | 554 | 29 | 110 | 48.6 |
| N50-R50 | 368 | 647 | 647 | 554 | 29 | 110 | 47.7 |
| N25-R75 | 368 | 324 | 971 | 554 | 29 | 110 | 46.1 |
| N0-R100 | 368 | 0 | 1294 | 554 | 29 | 110 | 45.2 |
| Concrete Type | Cement | Coarse Aggregates | Fine Aggregates | Water | Cost |
|---|---|---|---|---|---|
| (kg/m3) | (kg/m3) | (kg/m3) | (kg/m3) | (USD) | |
| OPC (35 MPa) | 400 | 1160 | 635 | 200 | 34.4 |
| Mix ID | Volume of Concrete (m3) | Fly Ash | Coarse Aggregates | Fine Aggregates | NaOH Solids | Na2SiO3 Solution | e-CO2 Emissions kg/m3 | |
|---|---|---|---|---|---|---|---|---|
| Natural | Recycled | |||||||
| Qty (kg/m3) | Qty (kg/m3) | Qty (kg/m3) | Qty (kg/m3) | Qty (kg/m3) | Qty (kg/m3) | |||
| N100-R0 | 1.0 | 368 | 1294 | 0 | 554 | 29 | 110 | 173.1 |
| N75-R25 | 1.0 | 368 | 971 | 324 | 554 | 29 | 110 | 165.1 |
| N50-R50 | 1.0 | 368 | 647 | 647 | 554 | 29 | 110 | 157.1 |
| N25-R75 | 1.0 | 368 | 324 | 971 | 554 | 29 | 110 | 149.1 |
| N0-R100 | 1.0 | 368 | 0 | 1294 | 554 | 29 | 110 | 141.1 |
| Concrete Type | Cement | Coarse Aggregates | Fine Aggregates | Water | e-CO2 Emissions |
|---|---|---|---|---|---|
| (kg/m3) | (kg/m3) | (kg/m3) | (kg/m3) | kg/m3 | |
| OPC (35 MPa) | 400 | 1160 | 635 | 200 | 404.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, Q.S.; Akbar, H.; Qazi, A.U.; Kazmi, S.M.S.; Munir, M.J. Bond Stress Behavior of a Steel Reinforcing Bar Embedded in Geopolymer Concrete Incorporating Natural and Recycled Aggregates. Infrastructures 2024, 9, 93. https://doi.org/10.3390/infrastructures9060093
Khan QS, Akbar H, Qazi AU, Kazmi SMS, Munir MJ. Bond Stress Behavior of a Steel Reinforcing Bar Embedded in Geopolymer Concrete Incorporating Natural and Recycled Aggregates. Infrastructures. 2024; 9(6):93. https://doi.org/10.3390/infrastructures9060093
Chicago/Turabian StyleKhan, Qasim Shaukat, Haroon Akbar, Asad Ullah Qazi, Syed Minhaj Saleem Kazmi, and Muhammad Junaid Munir. 2024. "Bond Stress Behavior of a Steel Reinforcing Bar Embedded in Geopolymer Concrete Incorporating Natural and Recycled Aggregates" Infrastructures 9, no. 6: 93. https://doi.org/10.3390/infrastructures9060093
APA StyleKhan, Q. S., Akbar, H., Qazi, A. U., Kazmi, S. M. S., & Munir, M. J. (2024). Bond Stress Behavior of a Steel Reinforcing Bar Embedded in Geopolymer Concrete Incorporating Natural and Recycled Aggregates. Infrastructures, 9(6), 93. https://doi.org/10.3390/infrastructures9060093









