Intrinsic Properties of Composite Double Layer Grid Superstructures †
Abstract
:1. Introduction
2. Bridge Decks Composed of Double Layer Grids and Reinforced Concrete Decking
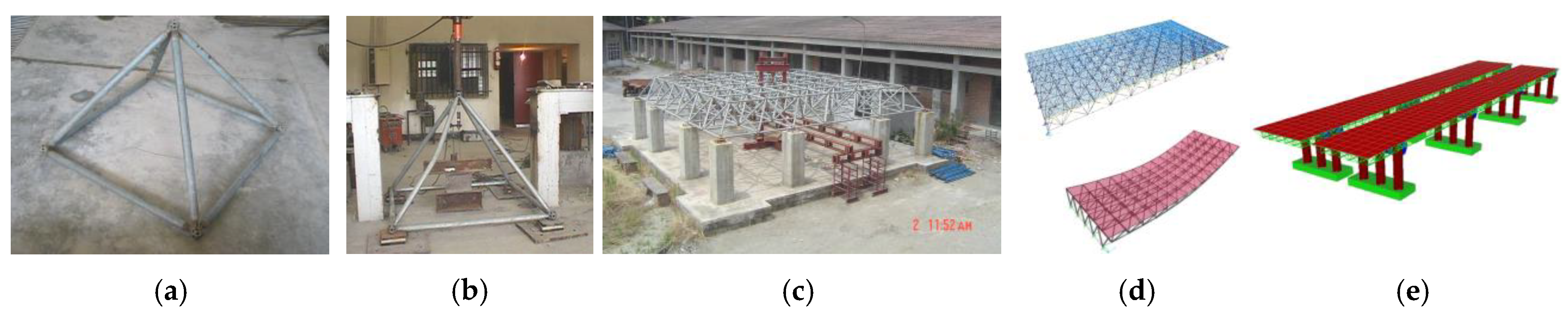
2.1. Characteristics of the Proposed CDLGS
2.2. Experimental Description
2.3. Single Span Bridges with CDGLS Deck System
2.3.1. Experiment Setup
2.3.2. Result of Comparison with Benchmark Deck
- Stage (1): where the double layer grid should bear its own weight plus the weight of fresh concrete and probable additional construction loads, followed by;
- Stage (2): in which the composite system after the hardening of concrete should resist any additional dead weight of paving, piping, guard rails, etc., together with live loads on the bridge.
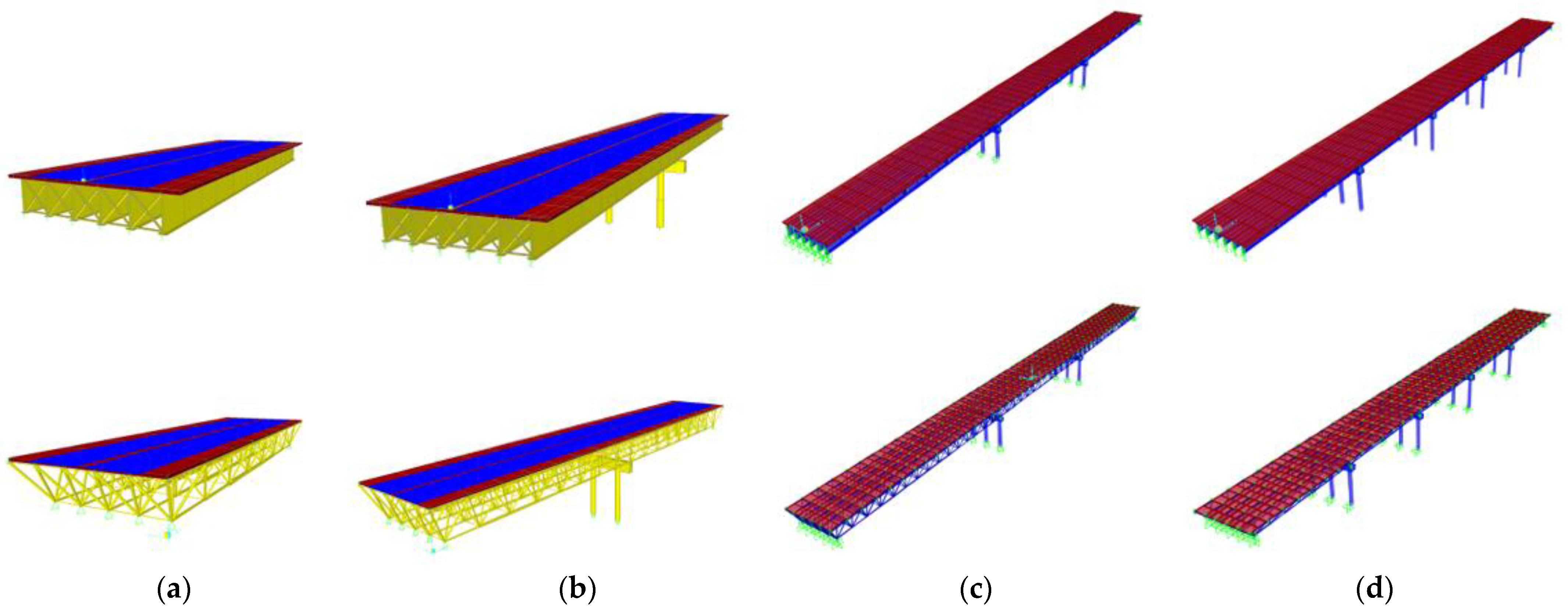
2.4. Extension to Continuous Superstructures Supported by Reinforced Concrete Bents
2.4.1. Weight Reduction Compared to Benchmark
2.4.2. Seismic Response Compared to Benchmark
2.5. Impact of Number and Lengths of Spans
2.5.1. Experiment Configuration
- CPGS bridge configurations: The lateral distance between the stringer beams was taken at 2 m and, the heights of plate girders were taken as 1/20 of the span lengths. The longitudinal distance between the transverse vertical diaphragms for the CPGS was between 5 to 6 m.
- CDLGS bridge configurations: The transversal subdivisions were considered at 2 m and the longitudinal subdivisions were equal to 2, 2.6, 3.2, and 4 m for the 20, 26, 32, and 40m span bridges, respectively. Three different ratios of the heights of superstructures to span lengths of 1/12.5, 1/15, and 1/17.5 were investigated for each span length (a total of 12 cases).
2.5.2. Weight Reduction Compared to Benchmark
- Single-span bridges: The reduction in steel material consumption was calculated as 33%, 36%, 40%, and 43% for the 20, 26, 32, and 40 m bridge spans, respectively.
- Two-span bridges: The weight of steel reduction was higher, ranging from 45%, 47%, 50%, and 52% for 2 × 20, 2 × 26, 2 × 32, and 2 × 40 m span bridges, respectively.
- Three-span bridges: The reduction was even higher ranging from 47%, 50%, 52%, and 56% for 3 × 20, 3 × 26, 3 × 32, and 3 × 40 m span bridges, respectively.
2.6. Load Transfer in the Traverse Direction
2.6.1. Experiment Configuration
2.6.2. Load Transfer Efficiency Compared to Benchmark
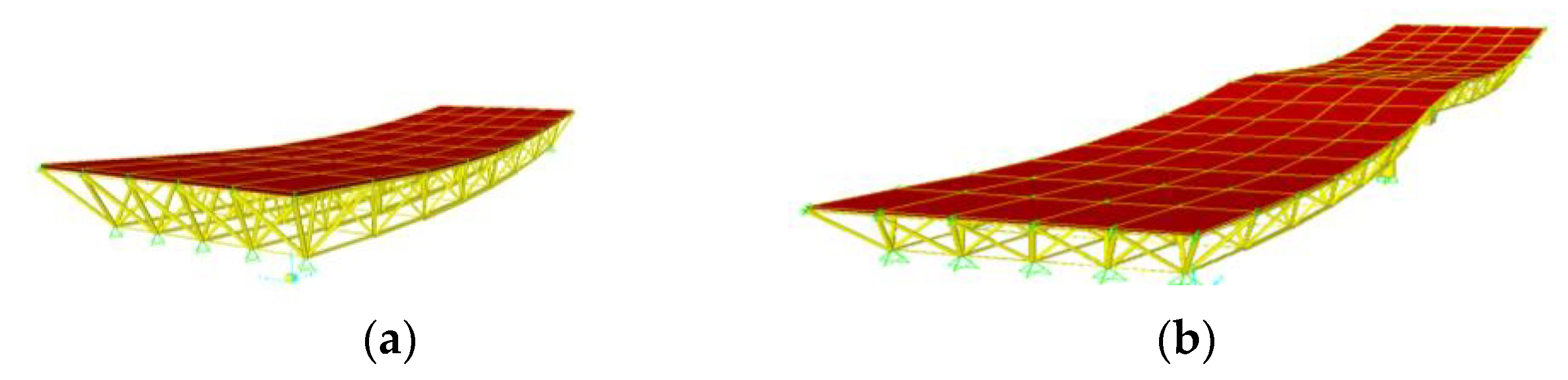
2.7. Effect of the Vertical Component of Earthquake Ground Motion
2.7.1. Experimental Setup
- CPGS bridge configurations: The span-to-length ratio was considered as 20. The side-by-side distance of the stringer beams was 2 m. The slab thickness was calculated as 180 mm and taken as 200 mm for the analysis to account for the one-way behavior and arching action in CPGS bridges. The distance between the transverse vertical diaphragms was set as 5 m in all cases for the CPGS.
- CDLGS bridge configurations: The span length to depth ratios were taken as 16. Regular 2 × 2 m modules (Figure 1a) were employed. The slab thickness was calculated as 120 mm controlled by punching shear, however, increased to 150 mm to preserve practicality and durability.
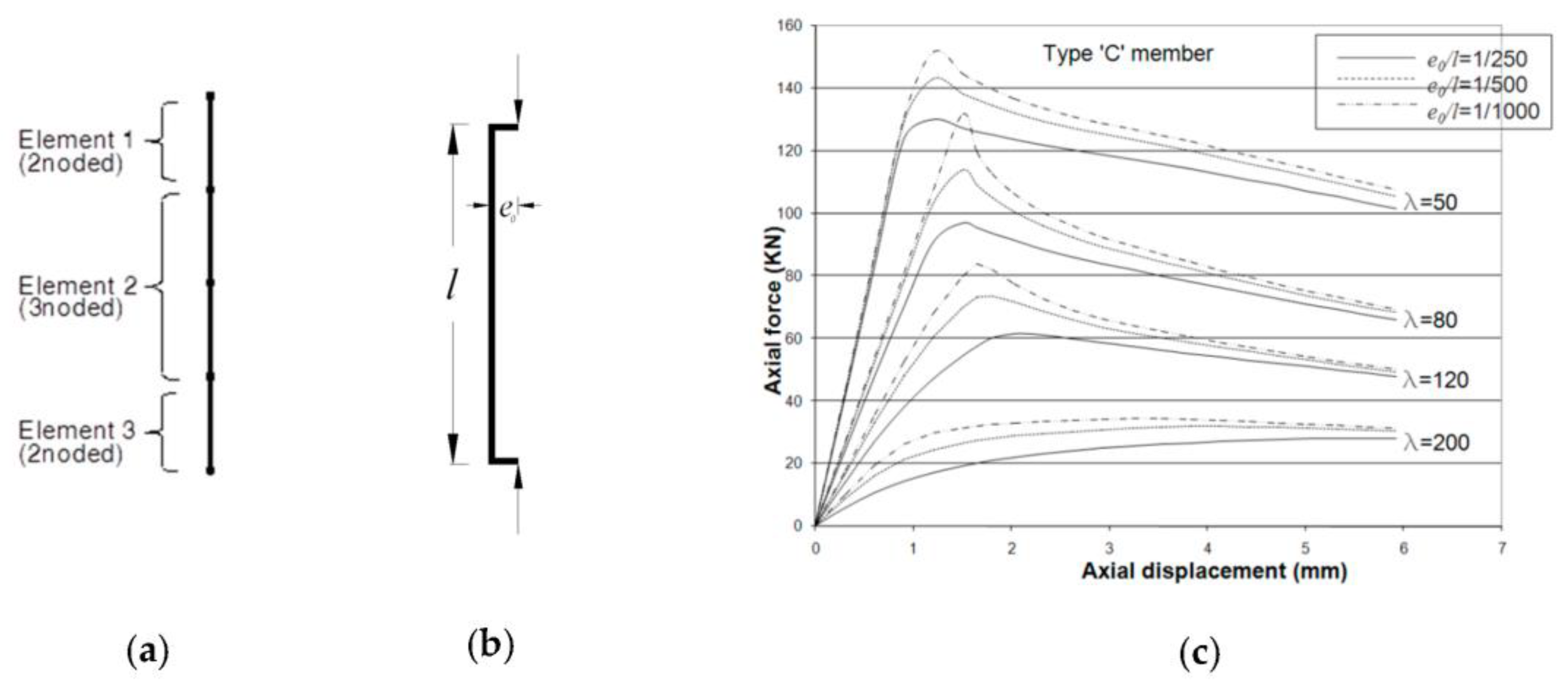
2.7.2. Space Grid CDLGS Member Modelling
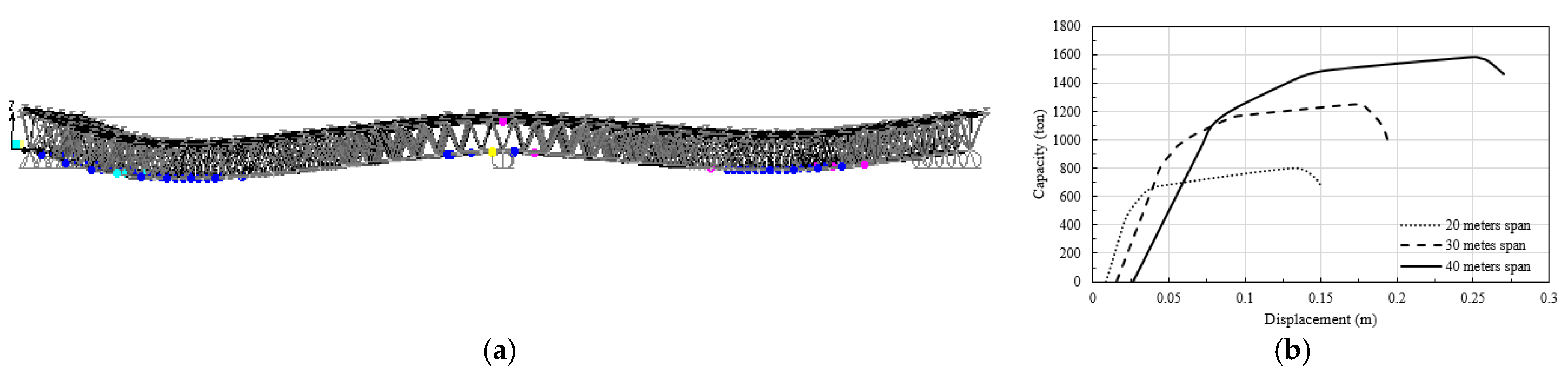
2.7.3. Nonlinear Static Analyses Results
- push from the top towards the bottom, in which the incremental loading was applied in the same direction as the gravity loading (Figure 7a); and
- push from the bottom towards the top, in which the incremental loading was applied in the opposite direction of the gravity loading.
2.7.4. Nonlinear Dynamic Analyses Results
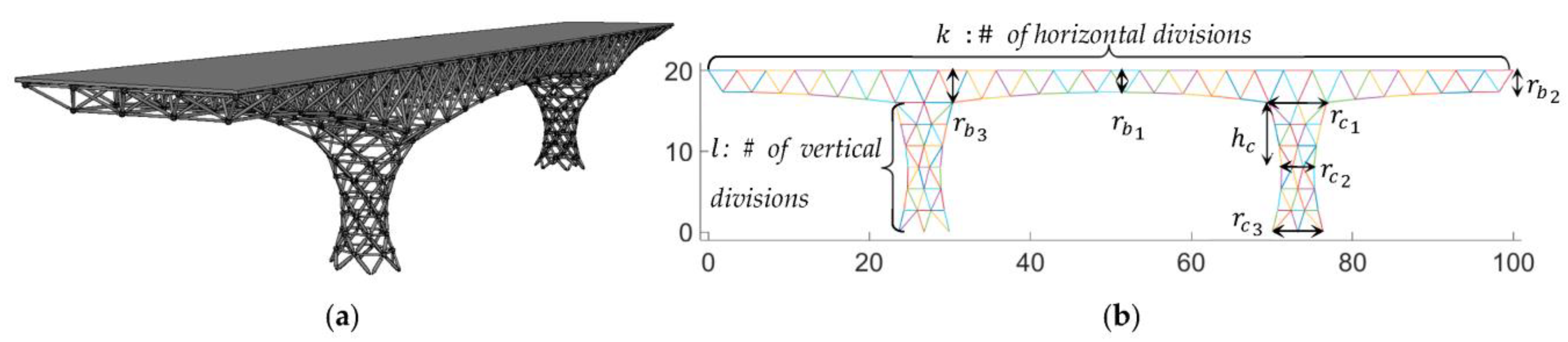
2.8. Multi-Objective Design Optimization
2.8.1. Design Optimization Setup
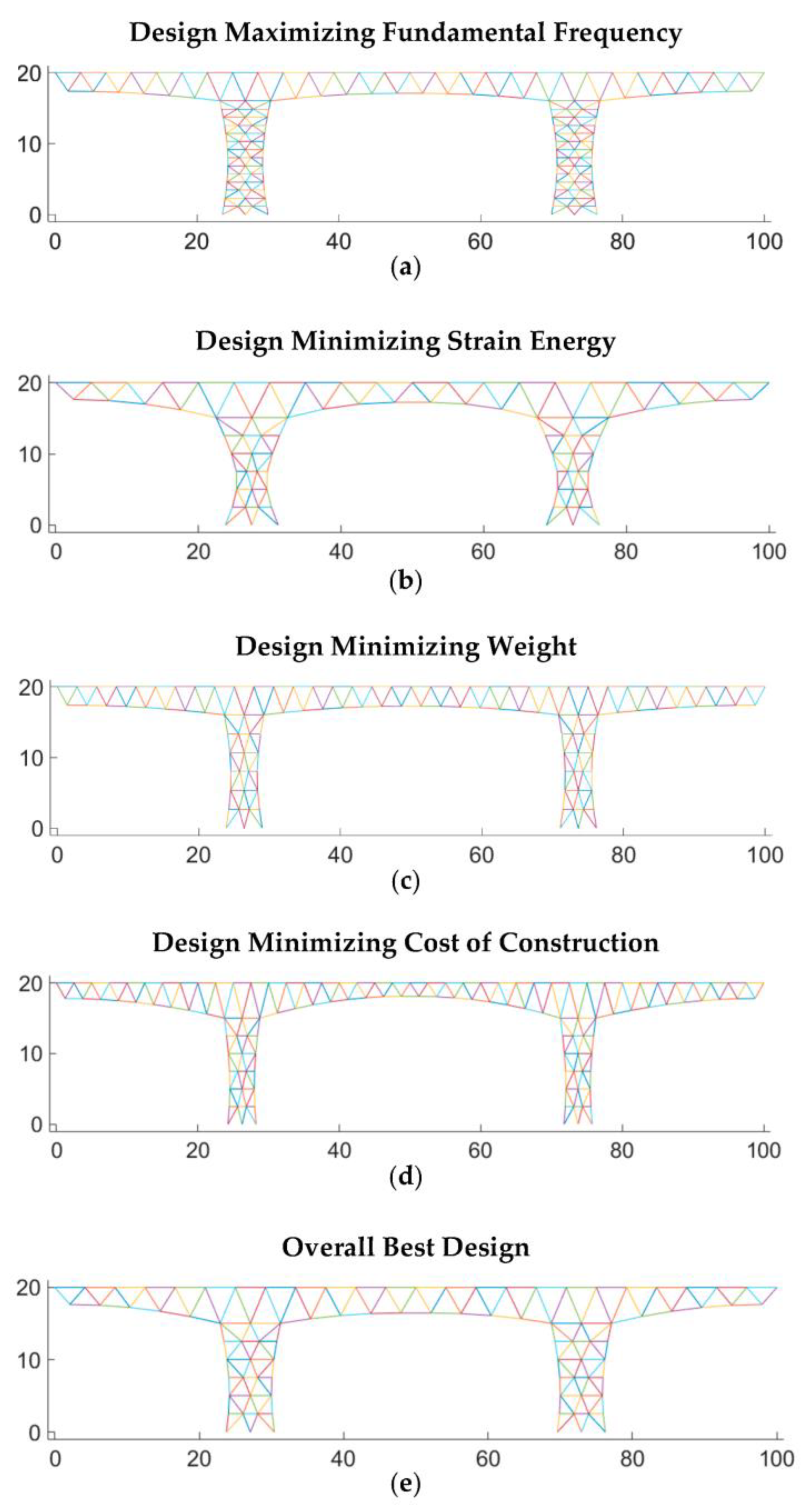
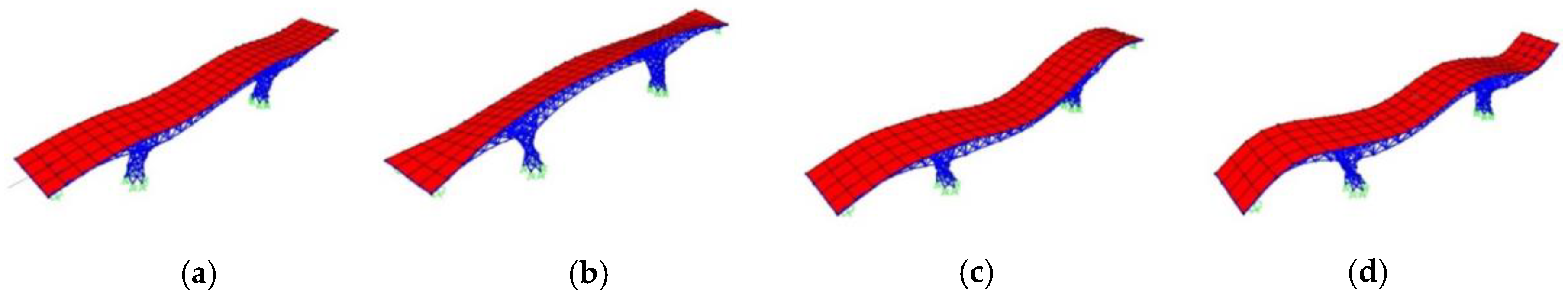
2.8.2. Design Optimization Results
3. Discussions: General Construction and Functionality Considerations
3.1. Further Design Considerations
3.2. Corrosion
3.3. Maintenance, Inspection, and Health Monitoring
3.4. Fatigue
3.5. Mass Production and Industrialization
3.6. Quality Control
3.7. Economic and Affordability Considerations
3.8. Sustainability
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Behnejad, S.A.; Parke, G.A.R. Half a Century with the Space Structures Research Centre of the University of Surrey. Int. J. Sp. Struct. 2014, 29, 205–214. [Google Scholar] [CrossRef]
- Makowski, Z.S. New trends in spatial structures. J. Int. Assoc. Shell Spat. Struct. 1986, 90, 21–43. [Google Scholar]
- Parke, G.A.R.; Behnejad, S.A. Z S Makowski: A Pioneer of Space Structures. Int. J. Sp. Struct. 2015, 30, 191–201. [Google Scholar] [CrossRef]
- Krishnan, S.; Liao, Y. Geometric Design of Deployable Spatial Structures Made of Three-Dimensional Angulated Members. J. Archit. Eng. 2020, 26, 04020029. [Google Scholar] [CrossRef]
- Dev, K.N.; Das, A.K. Design of Bamboo Shelter Kit for Post-Disaster Temporary Shelter Response. In Smart Innovation, Systems and Technologies; Springer: Singapore, 2021; Volume 221. [Google Scholar]
- Luo, Y.; Bao, J. A Material-Field Series-Expansion Method for Topology Optimization of Continuum Structures. Comput. Struct. 2019, 225, 106122. [Google Scholar] [CrossRef]
- Brütting, J.; Senatore, G.; Fivet, C. Design and Fabrication of a Reusable Kit of Parts for Diverse Structures. Autom. Constr. 2021, 125, 103614. [Google Scholar] [CrossRef]
- Rao, S.S. Engineering Optimization: Theory and Practice; John Wiley & Sons Inc.: Hoboken, NJ, USA, 2019. [Google Scholar]
- Deb, K. Multi-Objective Optimization Using Evolutionary Algorithms Kalyanmoy; Wiley: Hoboken, NJ, USA, 2001; Volume 16. [Google Scholar]
- Chilton, J. Space Grid Structures; Routledge: London, UK, 2007. [Google Scholar]
- Schober, H. Transparent Shells: Form, Topology, Structure; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Tzortzopoulos, P.; Kagioglou, M.; Koskela, L. Lean Construction-Core Concepts and New Frontiers; Routledge: London, UK, 2020; Volume 53, ISBN 9780429203732. [Google Scholar]
- Baldwin, A.; Bordoli, D. Handbook for Construction Planning and Scheduling; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2014; ISBN 9781118838167. [Google Scholar]
- Da Silveira, G.; Borenstein, D.; Fogliatto, F.S. Mass Customization: Literature Review and Research Directions. Int. J. Prod. Econ. 2001, 72, 1–13. [Google Scholar] [CrossRef]
- Li, X.; Wu, C.; Yang, Z.; Guo, Y.; Jiang, R. Knowledge Graph-Enabled Adaptive Work Packaging Approach in Modular Construction. Knowl.-Based Syst. 2023, 260, 110115. [Google Scholar] [CrossRef]
- Bertolesi, E.; Buitrago, M.; Adam, J.M.; Calderón, P.A. Fatigue Assessment of Steel Riveted Railway Bridges: Full-Scale Tests and Analytical Approach. J. Constr. Steel Res. 2021, 182, 106664. [Google Scholar] [CrossRef]
- Nettis, A.; Iacovazzo, P.; Raffaele, D.; Uva, G.; Adam, J.M. Displacement-Based Seismic Performance Assessment of Multi-Span Steel Truss Bridges. Eng. Struct. 2022, 254, 113832. [Google Scholar] [CrossRef]
- Pipinato, A. Extending the Lifetime of Steel Truss Bridges by Cost-Efficient Strengthening Interventions. Struct. Infrastruct. Eng. 2018, 14, 1611–1627. [Google Scholar] [CrossRef]
- Shahbazi-Reveshti, P.; Maalek, S.; Akbari, R. Buckling Behaviour of Composite Double-Layer Braced Barrel Vaults. Proc. Inst. Civ. Eng. Struct. Build. 2022, 175, 387–400. [Google Scholar] [CrossRef]
- Maalek, S. Shear Testing of Butt Joints. J. Test. Eval. 2014, 42, 1025–1045. [Google Scholar] [CrossRef]
- Ahmadizadeh, M.; Maalek, S. An Investigation of the Effects of Socket Joint Flexibility in Space Structures. J. Constr. Steel Res. 2014, 102, 72–81. [Google Scholar] [CrossRef]
- Gholampour, S.; Maalek, S. Experimental Study of the Influence of the Degree of Bolt Tightness on the Effective Length of Compression Members of Double Layer Space Structures Composed of Ball Joints. Modares Civ. Eng. J. 2012, 12, 37–50. [Google Scholar]
- Maalek, S. Structural Assessment and Quality Control Procedures for the Homa Aircraft Hangar No. 3. Int. J. Sp. Struct. 1999, 14, 167–184. [Google Scholar] [CrossRef]
- Peng, J.; Feng, Y.; Zhang, Q.; Liu, X. Multi-Objective Integrated Optimization Study of Prefabricated Building Projects Introducing Sustainable Levels. Sci. Rep. 2023, 13, 2821. [Google Scholar] [CrossRef] [PubMed]
- Deb, K.; Pratap, A.; Agarwal, S.; Meyarivan, T. A Fast and Elitist Multiobjective Genetic Algorithm: NSGA-II. IEEE Trans. Evol. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Nooshin, H.; Disney, P. Formex Configuration Processing II. Int. J. Sp. Struct. 2001, 16, 1–56. [Google Scholar] [CrossRef]
- Computers and Structures Inc. (CSi). SAP2000–Version 14. In Structural and Earthquake Engineering Software; Computers and Structures Inc.: Walnut Creek, CA, USA, 2009. [Google Scholar]
- American Association of State Highway and Transportation Officials (AASTHO). LRFD Bride Design Specifications, 9th ed.; AASHTO: Washington, DC, USA, 2020. [Google Scholar]
- Maalek, S.; Akbari, R.; Maheri, M.R. The Effect of Higher Modes on the Regularity of Single-Column-Bent Highway Viaducts. Bridg. Struct. 2009, 5, 29–43. [Google Scholar] [CrossRef]
- Maalek, S.; Nooshin, H.; Dianat, N.; Abedi, K.; Heristchian, M.; Chenaghlou, M.R. Code of Practice for Skeletal Steel Space Structures; Management and Planning Organization of Iran: Tehran, Iran, 2011. [Google Scholar]
- Ghadirli, B.; Maalek, S. A Feasibility Study on the Application of Composite Space Grid to Straight Bridges—A Technical and Economical Investigation. In Proceedings of the 4th National Conference on Spatial Structures, Terhan, Iran, 25–26 May 2014. [Google Scholar]
- Heydari-Digehsara, P.; Maalek, S. An Investigation of the Dynamic Vibration Behavior 0f Composite Space Grid Superstructures. In Proceedings of the 6th International Conference on Structures and Earthquakes, Christchurch, New Zealand, 1–4 November 2015; Kerman Bahonar University: Kerman, Iran, 2015. [Google Scholar]
- Maalek, S. A Formex Formulation for Substructure Analysis of Open Web Grids. Int. J. Sp. Struct. 1989, 4, 43–64. [Google Scholar] [CrossRef]
- Pirhadi, P.; Maalek, S. Seismic Behavior of Bridges with Composite Double Layer Grid Superstructures. In Proceedings of the 11th International Congress on Civil Engineering, Tehran, Iran, 8–10 May 2018. [Google Scholar]
- ASTM International ASTM A615; Standard Specification for Deformed and Plain Carbon-Steel Bars for Concrete Reinforcement. ASTM International: West Conshohocken, PA, USA, 2016.
- Vu, C.C.; Plé, O.; Weiss, J.; Amitrano, D. Revisiting the Concept of Characteristic Compressive Strength of Concrete. Constr. Build. Mater. 2020, 263, 120126. [Google Scholar] [CrossRef]
- Nooshin, H. The Formex Approach. J. Int. Assoc. Shell Spat. Struct. 1988, 96, 25–41. [Google Scholar]
- Baqershahi, M.H.; Maalek, S. Dynamic Transverse Load Distribution in Integral Bridges Composed of Tapered Composite Double Layer Superstructure and Tree-Shaped Piers. In Proceedings of the 5th International Conference on Bridges (5IBC 2019), Tehran, Iran, 1–3 May 2019. [Google Scholar]
- Jones, C.; Hammond, G. Inventory of Carbon and Energy (ICE); Version 3.0; Sustainable Energy Research Team, Department of Mechanical Engineering, University of Bath: Bath, UK, 2019. [Google Scholar]
- Hammond, G.; Jones, C. Embodied Carbon: The Inventory of Carbon and Energy (ICE); Version 2.0; A Building Services Research & Information Association (BSRIA) Guide; Institution of Civil Engineers (ICE): Berkshire, UK, 2011. [Google Scholar]
- Hammond, G.P.; Jone, C.I. Inventory of Carbon and Energy (ICE); Version 1.6a; University of Bath: Bath, UK, 2008. [Google Scholar]
- Kaartinen, E.; Dunphy, K.; Sadhu, A. LiDAR-Based Structural Health Monitoring: Applications in Civil Infrastructure Systems. Sensors 2022, 22, 4610. [Google Scholar] [CrossRef]
- Sánchez-Rodríguez, A.; Riveiro, B.; Soilán, M.; González-deSantos, L.M. Automated Detection and Decomposition of Railway Tunnels from Mobile Laser Scanning Datasets. Autom. Constr. 2018, 96, 171–179. [Google Scholar] [CrossRef]
- Boje, C.; Guerriero, A.; Kubicki, S.; Rezgui, Y. Towards a Semantic Construction Digital Twin: Directions for Future Research. Autom. Constr. 2020, 114, 103179. [Google Scholar] [CrossRef]
- Maalek, R.; Maalek, S. Automatic Recognition and Digital Documentation of Cultural Heritage Hemispherical Domes Using Images. J. Comput. Cult. Herit. 2023, 16, 6. [Google Scholar] [CrossRef]
- Sankaran, B.; Nevett, G.; O’Brien, W.J.; Goodrum, P.M.; Johnson, J. Civil Integrated Management: Empirical Study of Digital Practices in Highway Project Delivery and Asset Management. Autom. Constr. 2018, 87, 84–95. [Google Scholar] [CrossRef]
- Sankaran, B.; O’Brien, W.J. Impact of CIM Technologies and Agency Policies on Performance for Highway Infrastructure Projects. J. Constr. Eng. Manag. 2018, 144, 04018052. [Google Scholar] [CrossRef]
- Martínez, J.A.S.; Román, D.; Ozuna, L. Mixed Integer Programming Model for Facility Location Problems: Case Study for Consolidation Centers. Mob. Networks Appl. 2020, 25, 2118–2125. [Google Scholar] [CrossRef]
- Dutta, P.; Das, D.; Schultmann, F.; Fröhling, M. Design and Planning of a Closed-Loop Supply Chain with Three Way Recovery and Buy-Back Offer. J. Clean. Prod. 2016, 135, 604–619. [Google Scholar] [CrossRef]
- Hsu, P.Y.; Angeloudis, P.; Aurisicchio, M. Optimal Logistics Planning for Modular Construction Using Two-Stage Stochastic Programming. Autom. Constr. 2018, 94, 47–61. [Google Scholar] [CrossRef]
- Petersen, P.B. Total Quality Management and the Deming Approach to Quality Management. J. Manag. Hist. 1999, 5, 468–488. [Google Scholar] [CrossRef]
- Lehtola, V.V.; Kaartinen, H.; Nüchter, A.; Kaijaluoto, R.; Kukko, A.; Litkey, P.; Honkavaara, E.; Rosnell, T.; Vaaja, M.T.; Virtanen, J.P.; et al. Comparison of the Selected State-of-the-Art 3D Indoor Scanning and Point Cloud Generation Methods. Remote Sens. 2017, 9, 796. [Google Scholar] [CrossRef]
- Wang, Q.; Tan, Y.; Mei, Z. Computational Methods of Acquisition and Processing of 3D Point Cloud Data for Construction Applications. Arch. Comput. Methods Eng. 2020, 27, 479–499. [Google Scholar] [CrossRef]
- Ma, Z.; Liu, S. A Review of 3D Reconstruction Techniques in Civil Engineering and Their Applications. Adv. Eng. Inform. 2018, 37, 163–174. [Google Scholar] [CrossRef]
- Son, H.; Bosché, F.; Kim, C. As-Built Data Acquisition and Its Use in Production Monitoring and Automated Layout of Civil Infrastructure: A Survey. Adv. Eng. Inform. 2015, 29, 172–183. [Google Scholar] [CrossRef]
- Maalek, R.; Lichti, D.D.; Walker, R.; Bhavnani, A.; Ruwanpura, J.Y. Extraction of Pipes and Flanges from Point Clouds for Automated Verification of Pre-Fabricated Modules in Oil and Gas Refinery Projects. Autom. Constr. 2019, 103, 150–167. [Google Scholar] [CrossRef]
- Xu, X.; You, J.; Wang, Y.; Luo, Y. Analysis and Assessment of Life-Cycle Carbon Emissions of Space Frame Structures. J. Clean. Prod. 2023, 385, 135521. [Google Scholar] [CrossRef]
- United Nations Environment Programme. Global Status Report for Buildings and Construction: Towards a Zero-Emission, Efficient and Resilient Buildings and Construction Sector; United Nations Environment Programme: Nairobi, Kenya, 2022. [Google Scholar]
- Laali, A.; Nourzad, S.H.H.; Faghihi, V. Optimizing Sustainability of Infrastructure Projects through the Integration of Building Information Modeling and Envision Rating System at the Design Stage. Sustain. Cities Soc. 2022, 84, 104013. [Google Scholar] [CrossRef]



| Considered Application Areas | Metrics of Validation/Comparison | |
|---|---|---|
| Composite Steel Double Layer Grid Superstructure (CDLGS) vs. Composite Plate-Girder Superstructure (CPGS-Benchmark) | Single Span Bridges | Material Consumption (Weight). |
| Continuous Superstructures on Reinforced Concrete Bents | Material Consumption (Wight); Seismic Response. | |
| Impact of Number and Length of Spans | Material Consumption (Weight). | |
| Load Transfer Efficiency in Traverse Direction | Time−series Deflection. | |
| Effect of Vertical Component of Earthquake Ground Motion | Non-linear Static; Non-linear Dynamic. | |
| Integral Variable Depth Spatial Grid Bridge Structures (IVD-CDLGS) | Multi-objective Design Optimization | Weight (representing Embodied Carbo and Energy); Fundamental Frequency; Strain Energy; Cost of Construction. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maalek, S.; Maalek, R.; Maalek, B. Intrinsic Properties of Composite Double Layer Grid Superstructures. Infrastructures 2023, 8, 129. https://doi.org/10.3390/infrastructures8090129
Maalek S, Maalek R, Maalek B. Intrinsic Properties of Composite Double Layer Grid Superstructures. Infrastructures. 2023; 8(9):129. https://doi.org/10.3390/infrastructures8090129
Chicago/Turabian StyleMaalek, Shahrokh, Reza Maalek, and Bahareh Maalek. 2023. "Intrinsic Properties of Composite Double Layer Grid Superstructures" Infrastructures 8, no. 9: 129. https://doi.org/10.3390/infrastructures8090129
APA StyleMaalek, S., Maalek, R., & Maalek, B. (2023). Intrinsic Properties of Composite Double Layer Grid Superstructures. Infrastructures, 8(9), 129. https://doi.org/10.3390/infrastructures8090129








