Seismic Capacity Estimation of a Masonry Bell-Tower with Verticality Imperfection Detected by a Drone-Assisted Survey †
Abstract
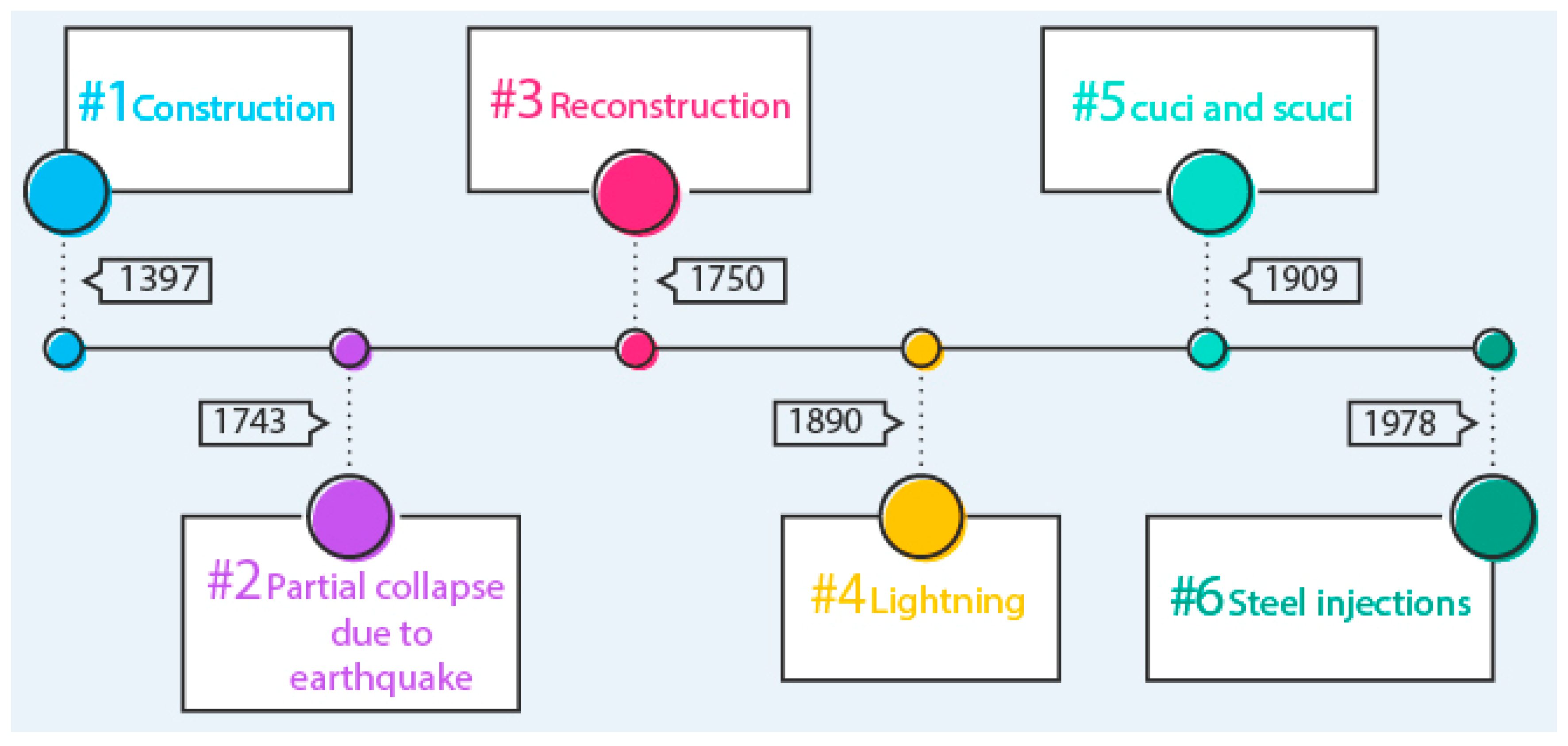
1. Introduction
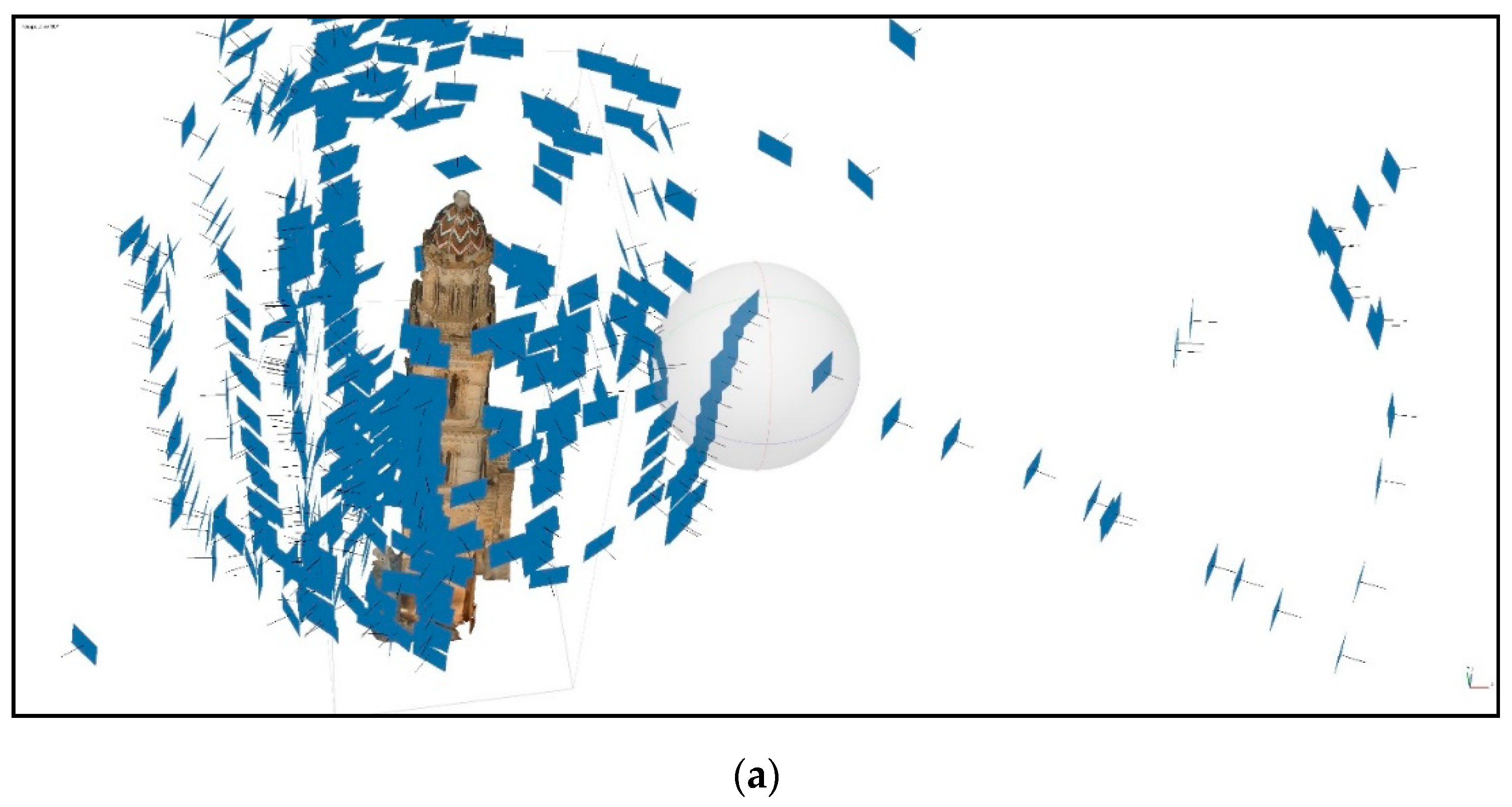
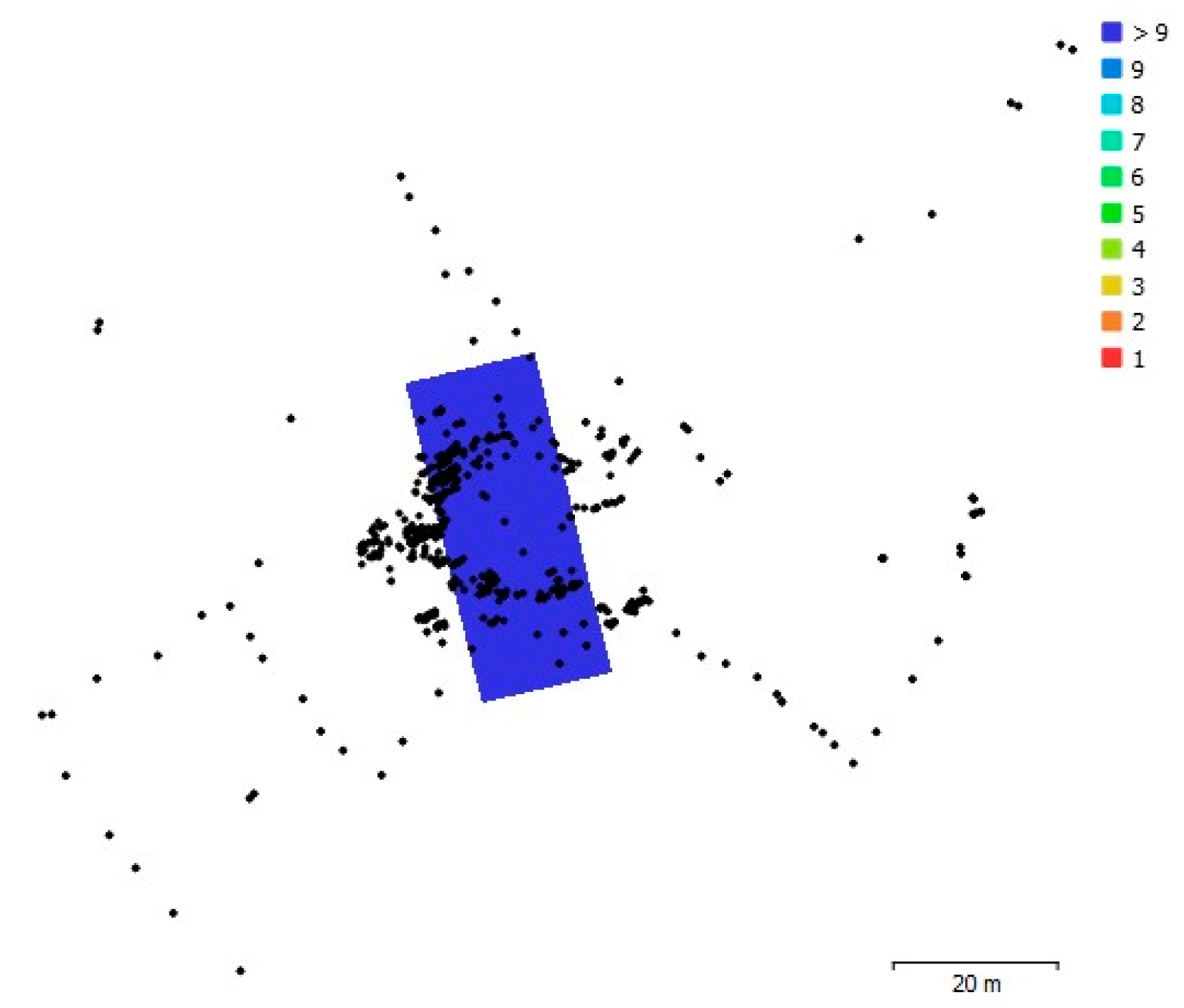
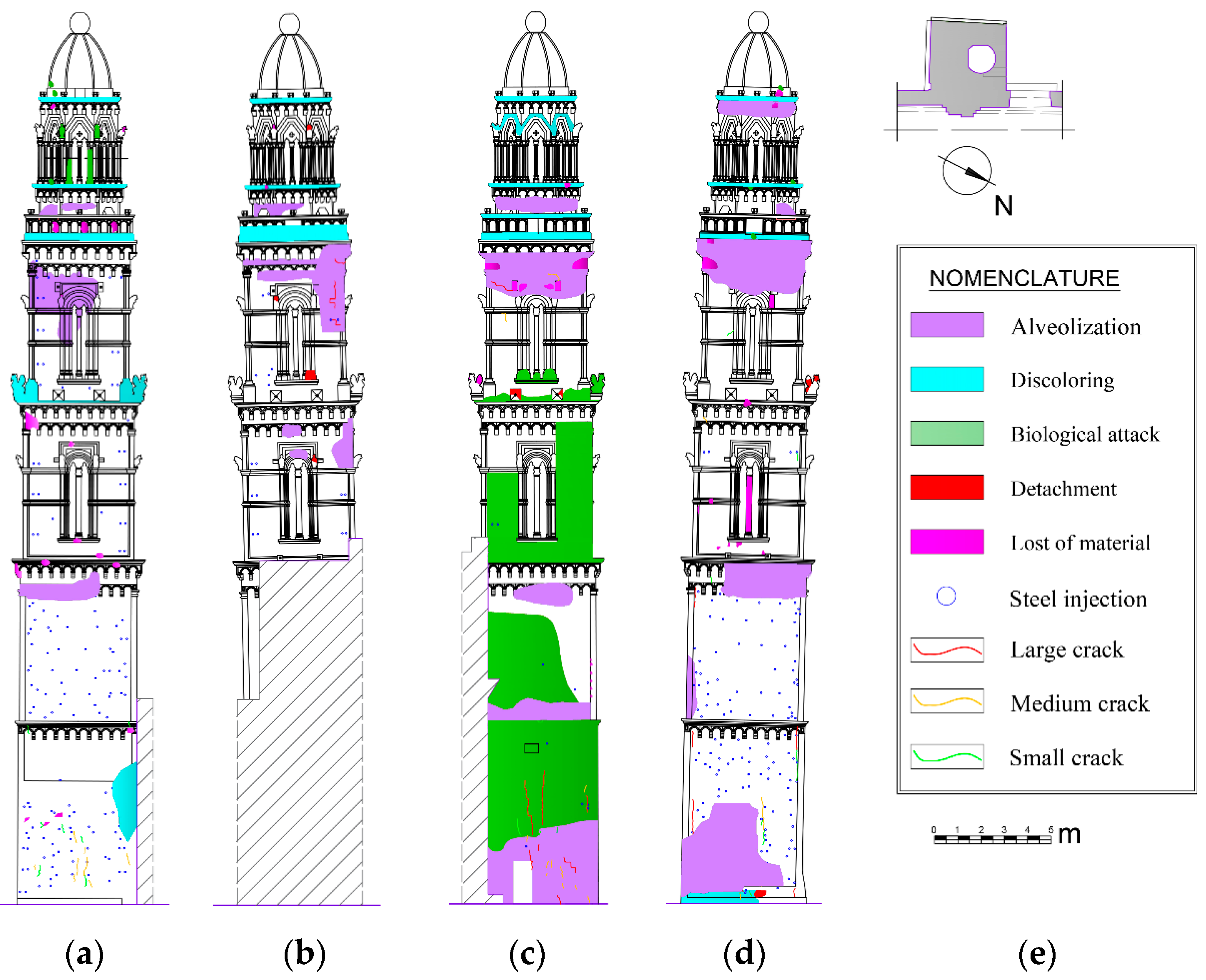
2. Drone-Assisted Survey
3. Structural Analysis
3.1. Linear Dynamic Analysis
3.2. Nonlinear Static Analysis (Pushover)
3.3. Kinematic Linear Analysis
- the compressive strength of masonry is infinite;
- the tensile strength of masonry is null;
- the sliding between the block is forbidden by the method;
- masonry acts as an assemblage of rigid blocks which means that the collapse is due to the formation of a sufficient number of hinges between the blocks themselves.
- M1—Total tower overturning with respect to the X-direction. The fracture plane is horizontal, and the rotation performs around a cylindrical hinge;
- M2—Half tower overturning with respect to the X-direction. The fracture plane is horizontal, and the rotation performs around a cylindrical hinge;
- M3—Half tower overturning with respect to the X-direction. The fracture plane is inclined, and the rotation performs around a cylindrical hinge;
- M4—Half tower overturning with respect to the Y-direction. The fracture plane is horizontal, and the rotation performs around a cylindrical hinge;
- M5—Half tower overturning with respect to the Y-direction. The fracture plane is inclined, and the rotation performs around a cylindrical hinge;
- M6—Half tower overturning with respect to the diagonal of the cross-section. The fracture plane is inclined, and the rotation performs around a spherical hinge.
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ferraioli, M.; Miccoli, L.; Abruzzese, D.; Mandara, A. Dynamic characterisation and seismic assessment of medieval masonry towers. Nat. Hazards 2017, 86, 489–515. [Google Scholar] [CrossRef]
- Saisi, A.; Gentile, C.; Guidobaldi, M.; Xu, M. Dynamic monitoring and seismic response of a historic masonry tower. Key Eng. Mater. 2014, 628, 55–60. [Google Scholar] [CrossRef]
- Facchini, L.; Betti, M. Simplified seismic analysis of disordered masonry towers. ASCE-ASME J. Risk Uncertain. Eng. Syst. Part A Civ. Eng. 2016, 2, C4015010. [Google Scholar] [CrossRef]
- Peña, F.; Lourenço, P.B.; Mendes, N.; Oliveira, D.V. Numerical models for the seismic assessment of an old masonry tower. Eng. Struct. 2010, 32, 1466–1478. [Google Scholar] [CrossRef]
- Fragonara, L.Z.; Boscato, G.; Ceravolo, R.; Russo, S.; Ientile, S.; Pecorelli, M.L.; Quattrone, A. Dynamic investigation on the Mirandola bell-tower in post-earthquake scenarios. Bull. Earthq. Eng. 2016, 15, 313–337. [Google Scholar] [CrossRef]
- Diaferio, M.; Foti, D. Seismic risk assessment of Trani’s Cathedral bell-tower in Apulia, Italy. Int. J. Adv. Struct. Eng. 2017, 9, 259–267. [Google Scholar] [CrossRef]
- Bartoli, G.; Betti, M.; Vignoli, A. A numerical study on seismic risk assessment of historic masonry towers: A case study in San Gimignano. Bull. Earthq. Eng. 2016, 14, 1475–1518. [Google Scholar] [CrossRef]
- Bernardeschi, K.; Padovani, C.; Pasquinelli, G. Numerical modelling of the structural behaviour of Buti’s bell-tower. J. Cult. Herit. 2004, 5, 371–378. [Google Scholar] [CrossRef]
- Acito, M.; Bocciarelli, M.; Chesi, C.; Milani, G. Collapse of the clock tower in Finale Emilia after the May 2012 Emilia Romagna earthquake sequence: Numerical insight. Eng. Struct. 2014, 72, 70–91. [Google Scholar] [CrossRef]
- Valente, M.; Milani, G. Non-linear dynamic and static analyses on eight historical masonry towers in the North-East of Italy. Eng. Struct. 2016, 114, 241–270. [Google Scholar] [CrossRef]
- D’Ambrisi, A.; Mariani, V.; Mezzi, M. Seismic assessment of a historical masonry tower with nonlinear static and dynamic analyses tuned on ambient vibration tests. Eng. Struct. 2012, 36, 210–219. [Google Scholar] [CrossRef]
- Rossetti, P. Maria SS.ma Assunta–Soleto; Editrice Salentina: Lecce, Italy, 2011. (In Italian) [Google Scholar]
- Rizzo, T. Restauri Satanici, Tipografia; G. Capponi srl–Firenze: Florence, Italy, 1994. (In Italian) [Google Scholar]
- Duque, L.; Seo, J.; Wacker, J. Bridge Deterioration Quantification Protocol Using UAV. ASCE J. Bridge Eng. 2018, 23, 04018080. [Google Scholar] [CrossRef]
- Burger, W.; Mark, J. Burge, Digital Image Processing: An Algorithmic Approach Using Java; Springer: Berlin/Heidelberg, Germany, 2007; ISBN 978-1-84628-379-6. [Google Scholar]
- Fortunato, G.; Funari, M.F.; Lonetti, P. Survey and seismic vulnerability assessment of the Baptistery of San Giovanni in Tumba (Italy). J. Cult. Herit. 2017, 26, 64–78. [Google Scholar] [CrossRef]
- Ridolfi, E.; Buffi, G.; Venturi, S.; Manciola, P. Accuracy analysis of a dam model from drone surveys. Sensors 2017, 17, 1777. [Google Scholar] [CrossRef] [PubMed]
- Barrile, V.; Bilotta, G.; D’Amore, E.; Meduri, G.M.; Trovato, S. Structural modeling of a historic castle using close range photogrammetry. Int. J. Math Comput. Simul. 2016, 10, 370–380. [Google Scholar]
- Marmo, F.; Demartino, C.; Candela, G.; Sulpizio, C.; Briseghella, B.; Spagnuolo, R.; Rosati, L. On the form of the Musmeci’s bridge over the Basento river. Eng. Struct. 2019, 191, 658–673. [Google Scholar] [CrossRef]
- Micelli, F.; Cascardi, A. Structural assessment and seismic analysis of a 14th century masonry tower. Eng. Fail. Anal. 2020, 107, 104198. [Google Scholar] [CrossRef]
- Midas FEA. Advanced Nonlinear and Detail Program. Analysis and Algorithm. 1989. Available online: https://en.midasuser.com/product/fea_overview.asp (accessed on 8 July 2020).
- Thorenfeldt, E.; Tomaszewicz, A.; Jensen, J.J. Mechanical properties of high-strength concrete and applications in design. In Proceedings of the Symposium Utilization of High-Strength Concrete, Stavanger, Norway, 15–18 June 1987; Tapir: Trondheim, Norway, 1987. [Google Scholar]
- Cornelissen, H.A.W.; Hordijk, D.A.; Reinhardt, H.W. Experimental determination of crack softening characteristics of normalweight and lightweight concrete. Heron 1986, 31, 2. [Google Scholar]
- Sarhosis, V.; Milani, G.; Formisano, A.; Fabbrocino, F. Evaluation of different approaches for the estimation of the seismic vulnerability of masonry towers. Bull. Earthq. Eng. 2017, 16, 1511–1545. [Google Scholar] [CrossRef]
- Micelli, F.; Cascardi, A.; Aiello, M.A. Seismic behavior of a masonry bell-tower with verticality defect. In Proceedings of the Euro-American Congress REHABEND 2020 on Construction Pathology, Rehabilitation Technology and Heritage Management, Granada, Spain, 24–27 March 2020. [Google Scholar]




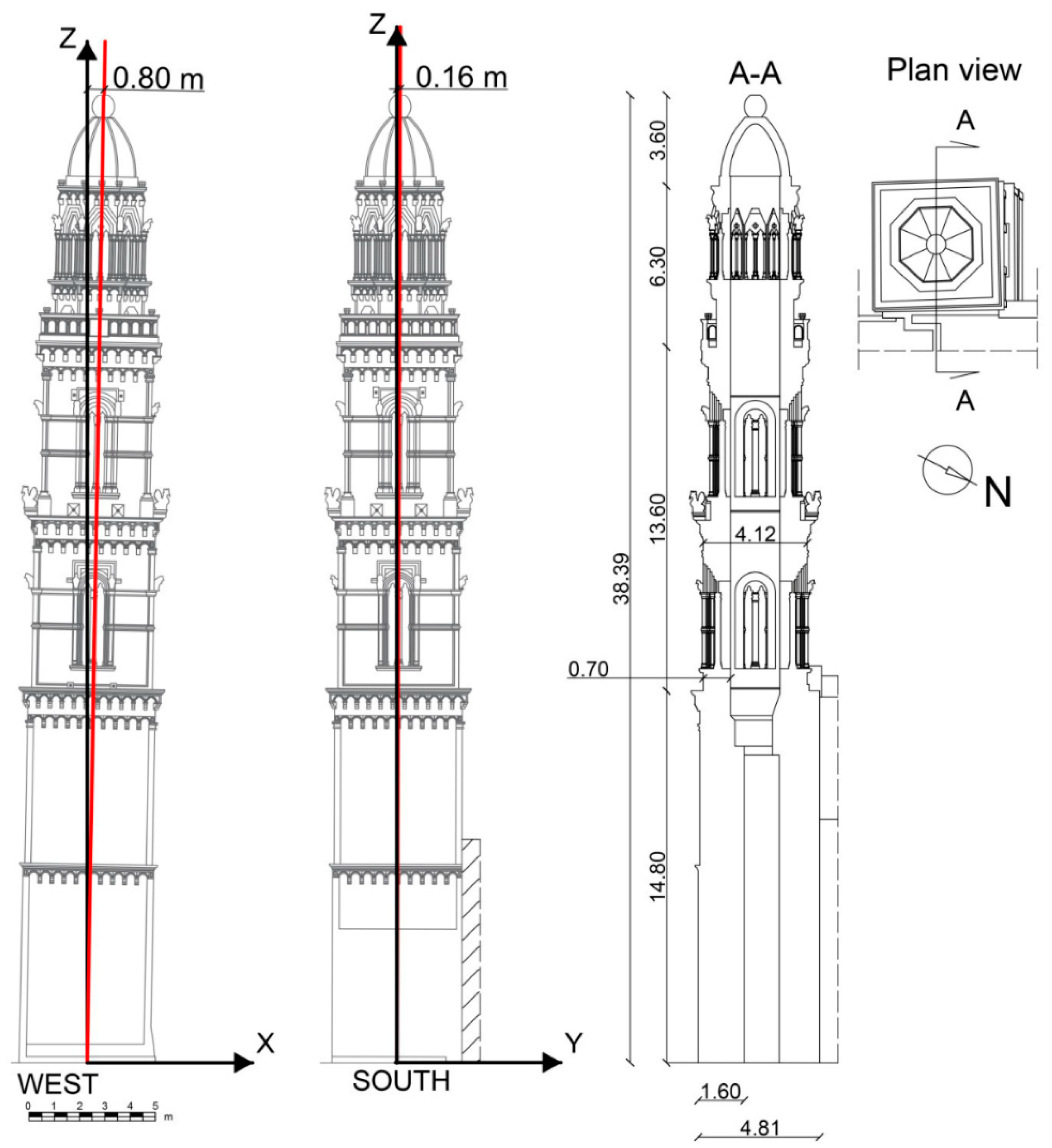
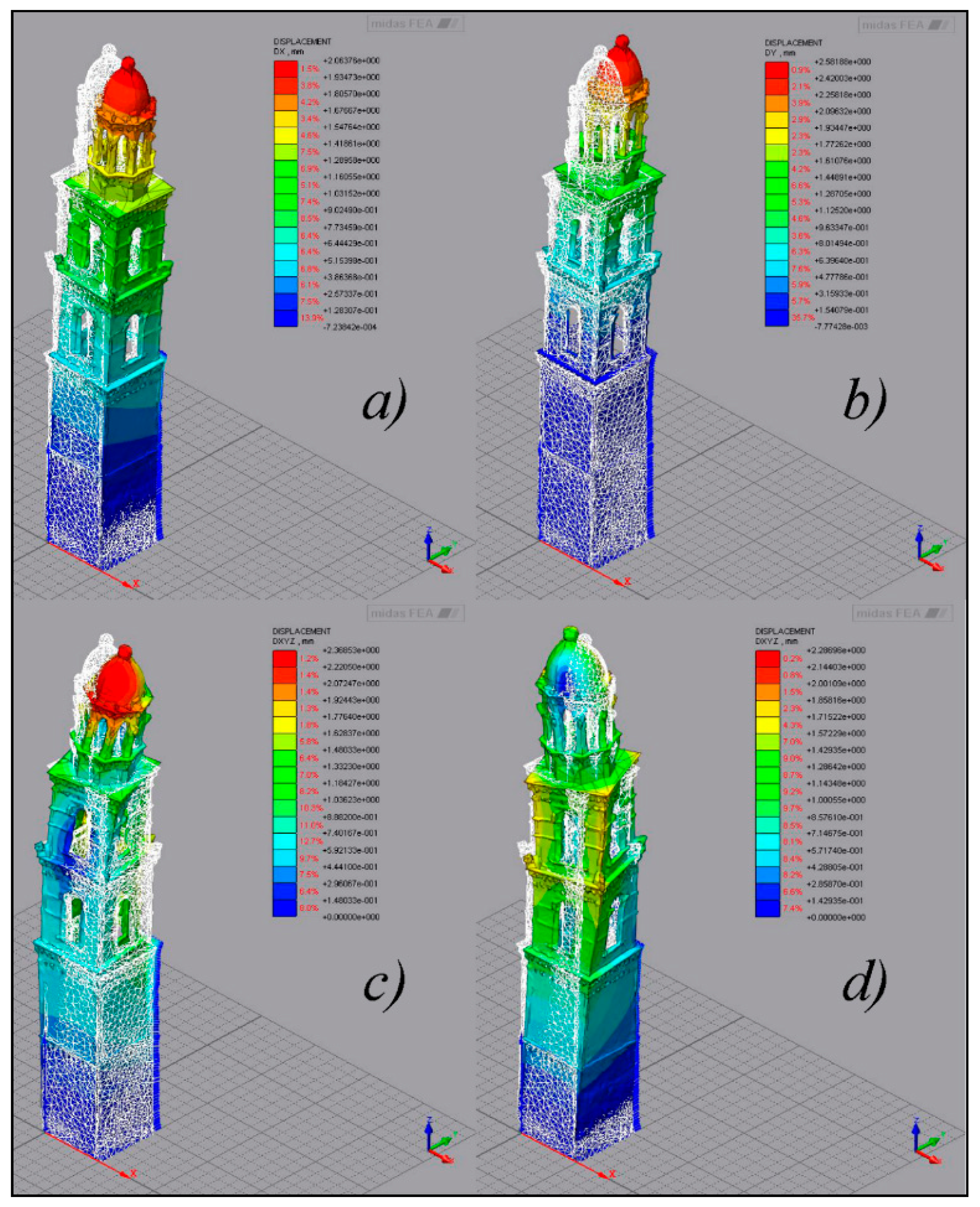

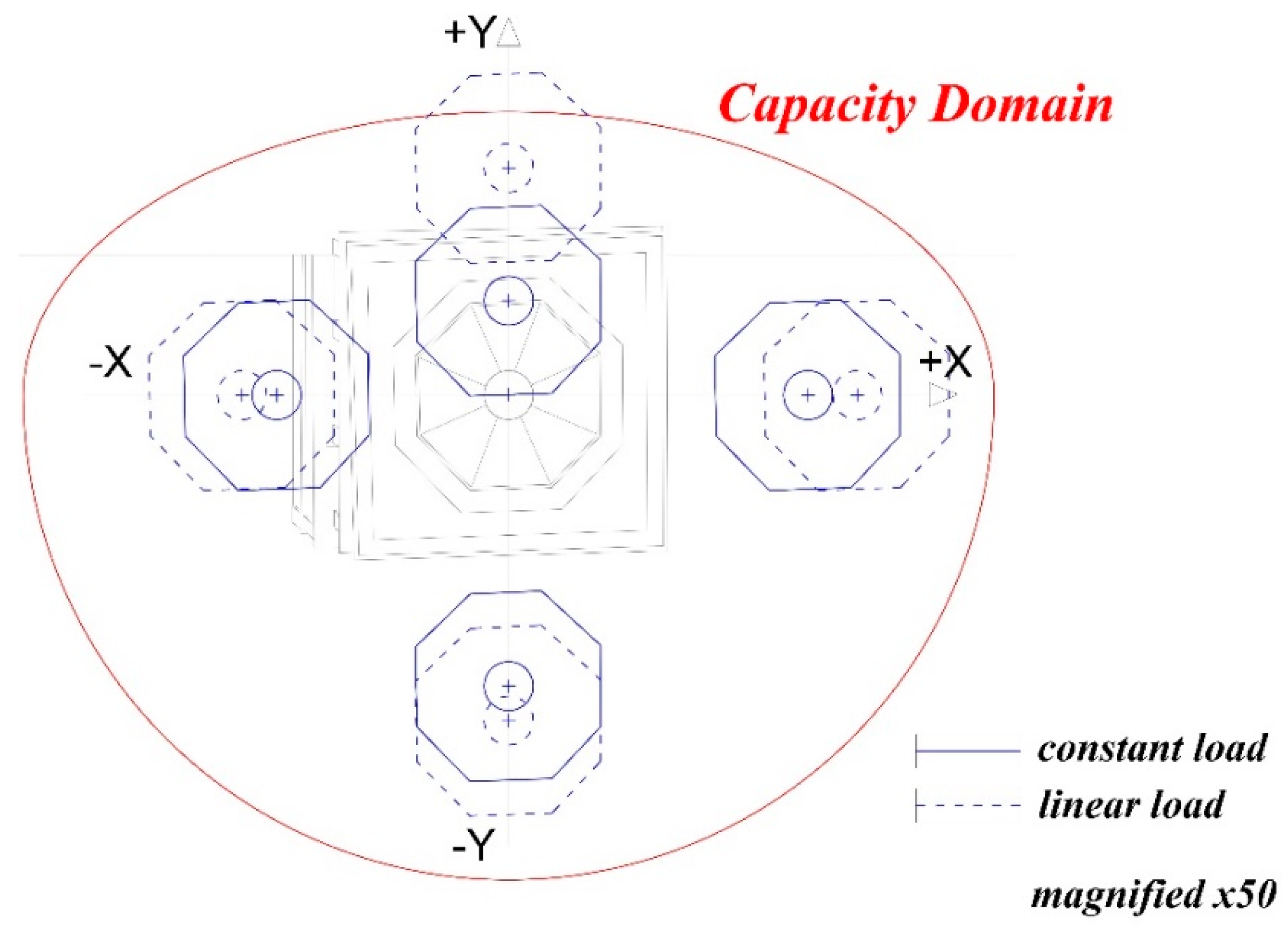
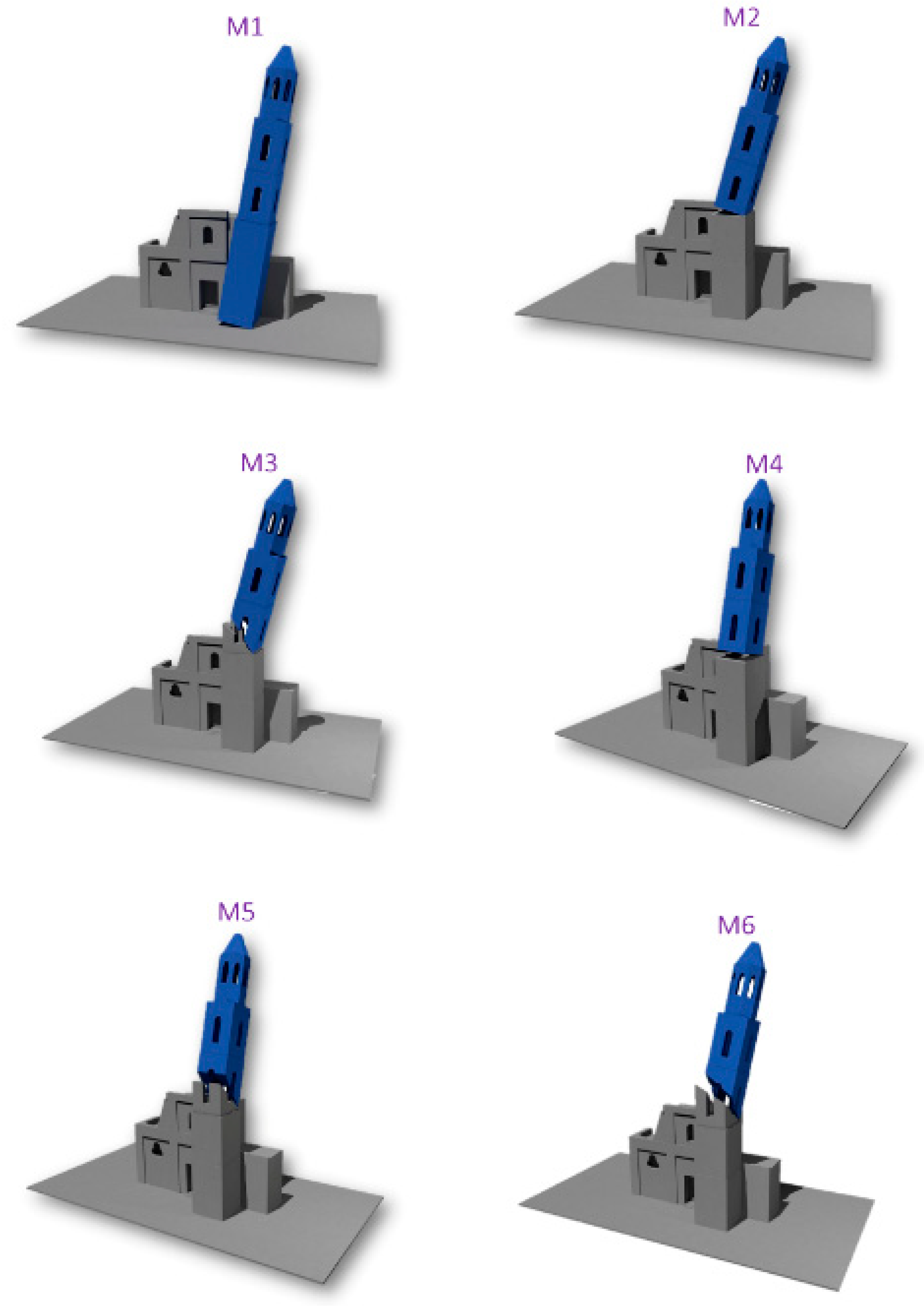

| Variable | Tuff | Lecce-Stone | Units |
|---|---|---|---|
| Masonry compressive strength | 5.77 | 7.2 | MPa |
| Masonry tensile strength | 0.50 | 0.70 | MPa |
| Masonry shear strength | 0.8 | 0.9 | MPa |
| Young’s modulus | 2197 | 3360 | MPa |
| Shear modulus | 729 | 1120 | MPa |
| Weight density | 16 | 22 | kN/m3 |
| Mode | Frequency [rad/sec] | Period [sec] | Participating Mass [%] | Description |
|---|---|---|---|---|
| 1 | 5.644 | 1.113 | 59.26 | X-translation |
| 2 | 7.851 | 0.800 | 52.23 | Y-translation |
| 3 | 16.373 | 0.384 | 68.41 | Global X-rotation |
| 4 | 18.234 | 0.345 | 77.17 | Global Z-rotation |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Micelli, F.; Cascardi, A.; Aiello, M.A. Seismic Capacity Estimation of a Masonry Bell-Tower with Verticality Imperfection Detected by a Drone-Assisted Survey. Infrastructures 2020, 5, 72. https://doi.org/10.3390/infrastructures5090072
Micelli F, Cascardi A, Aiello MA. Seismic Capacity Estimation of a Masonry Bell-Tower with Verticality Imperfection Detected by a Drone-Assisted Survey. Infrastructures. 2020; 5(9):72. https://doi.org/10.3390/infrastructures5090072
Chicago/Turabian StyleMicelli, Francesco, Alessio Cascardi, and Maria Antonietta Aiello. 2020. "Seismic Capacity Estimation of a Masonry Bell-Tower with Verticality Imperfection Detected by a Drone-Assisted Survey" Infrastructures 5, no. 9: 72. https://doi.org/10.3390/infrastructures5090072
APA StyleMicelli, F., Cascardi, A., & Aiello, M. A. (2020). Seismic Capacity Estimation of a Masonry Bell-Tower with Verticality Imperfection Detected by a Drone-Assisted Survey. Infrastructures, 5(9), 72. https://doi.org/10.3390/infrastructures5090072







