Fundamental Approaches to Predict Moisture Damage in Asphalt Mixtures: State-of-the-Art Review
Abstract
1. Introduction
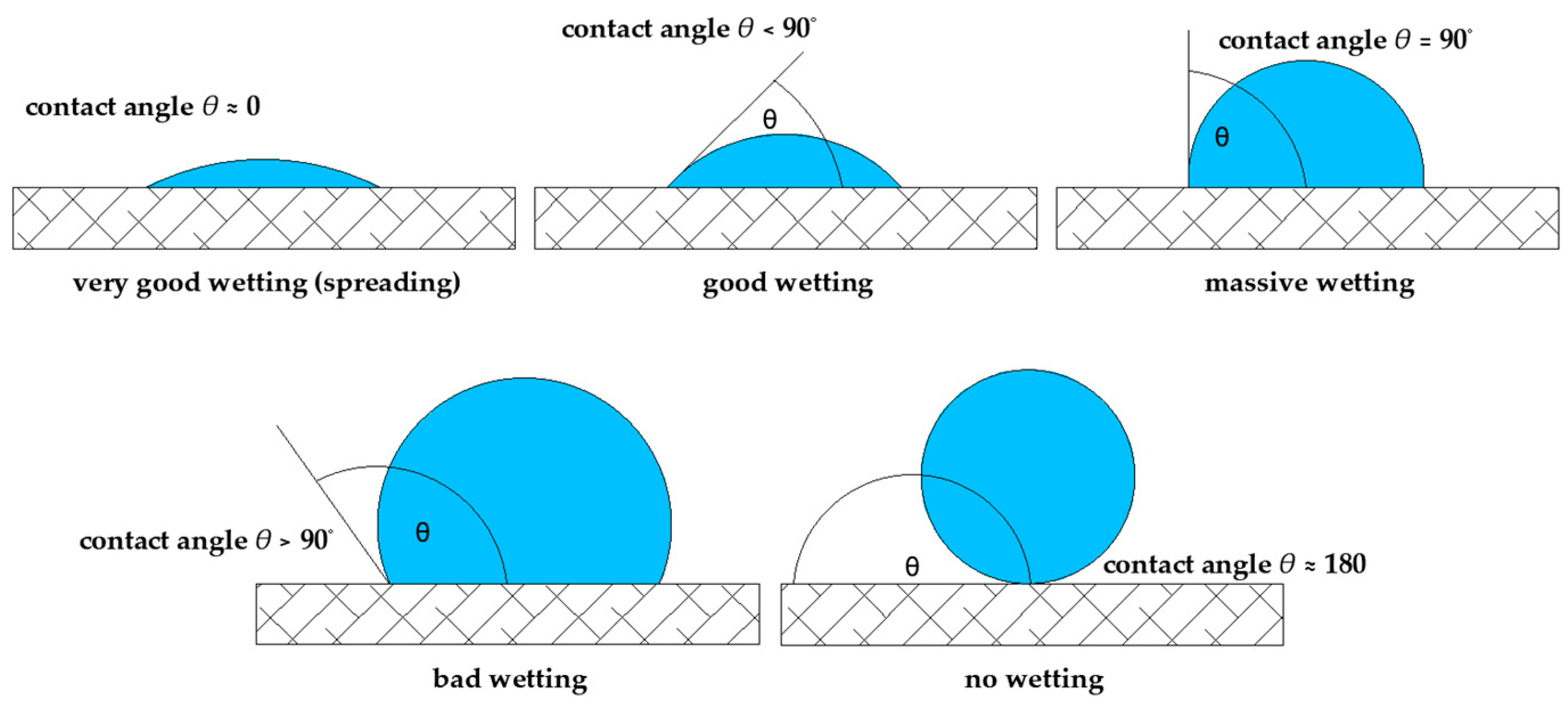
2. Concepts to Calculate the Bitumen–Aggregate Adhesion
2.1. Calculation of Adhesive Bond Strength and Debonding by Water from Surface Energy Components
- γ—total surface energy;
- γLW—dispersive part of the surface energy;
- γAB—acid base part of the surface energy;
- γ+—Lewis acid component or electron acceptor of surface energy;
- γ−—Lewis base or electron donor component of surface energy.
2.2. Calculation of Adhesive Bond Strengths in Various Media Based on the Hamaker Equation
- εi—the static dielectric constant for material/medium I (in vacuum ε3 = 1);
- ni—the refractive index of the material/medium I, in the visible region (in vacuum n3 = 1);
- h—Planck’s constant (= 6.6261 × 10−34 Js);
- k—Boltzmann constant (= 1.3807 × 10−23 J/K);
- T—the absolute temperature;
- υ—the main electronic absorption frequency (typically ± 3 × 1015 s−1).
- rii—the separation distance between interacting atoms or molecules;
- γLW—the dispersive part of the surface energy.
3. Summary of Experimental Studies Based on SFE Approach
3.1. Overview of Test Procedures to Determine Surface Energies
3.1.1. SFE Measurement Results on Bituminous Binders
3.1.2. SFE Measurement Results on Aggregates
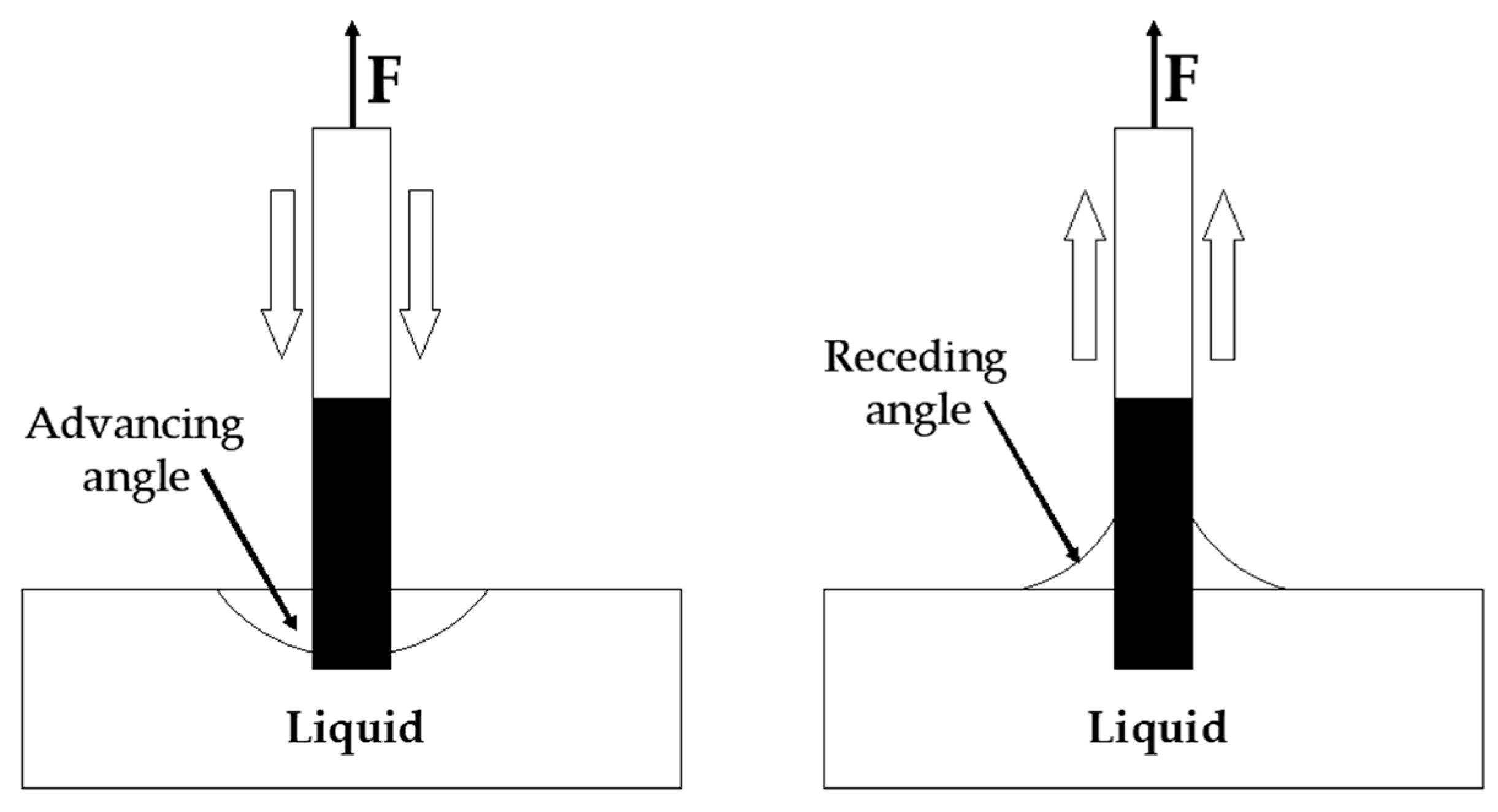
- The use of contact angle methods such as the sessile drop method are limited as they require a flat stone surface; and a large number of repeats are needed [53];
3.1.3. Dry and Wet Adhesion
3.2. Sessile Drop Measurements in Dry and Wet Conditions
3.3. Correlations between SFE Measurements and Water Sensitivity Observations
3.3.1. Correlations between Calculated Bond Strengths and Laboratory Tests Indicative of Moisture Damage
3.3.2. Correlations between Calculated Bond Strengths and Field Experience
4. Summary of Experimental Studies Based on the Hamaker Approach
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lu, Q.; Harvey, J.T.; Monismith, C.L. Investigation of Conditions for Moisture Damage in Asphalt Concrete and Appropriate Laboratory Test Methods: Summary Report, University of California, Pavement Research Center UC Davis and Berkeley. Available online: http://www.ucprc.ucdavis.edu/PDF/UCPRC-SR-2005-01.pdf (accessed on 26 January 2020).
- Skaf, M.; Pasquini, E.; Revilla-Cuesta, V.; Ortega-Lopez, V. Performance and durability of porous asphalt mixtures manufactured exclusively with electric steel slag. Materials 2019, 12, 3306. [Google Scholar] [CrossRef] [PubMed]
- Ingrassia, L.P.; Lu, X.; Ferrotti, G.; Canestrari, F. Renewable materials in bituminous binders and mixtures: Speculative pretext or reliable opportunity? Resour. Conserv. Rec. 2019, 144, 209–222. [Google Scholar] [CrossRef]
- Ma, J.; Sun, D.; Pang, Q.; Sun, G.; Hu, M.; Lu, T. Potential of recycled concrete aggregate pretreated with waste cooking oil residue for hot mix asphalt. J. Clean. Prod. 2019, 221, 469–479. [Google Scholar] [CrossRef]
- Terrel, R.; Al-Swailmi, S. Water Sensitivity of Asphalt–Aggregate Mixes: Test Selection. In SHRP Report A-403; National Research Council: Washington, DC, USA, 1994; p. 183. Available online: http://onlinepubs.trb.org/onlinepubs/shrp/SHRP-A-403.pdf (accessed on 10 October 2019).
- Caro, S.; Masad, E.; Bhasin, A.; Little, D.N. Moisture susceptibility of asphalt mixtures, Part 1: mechanisms. Int. J. Pavement Eng. 2008, 9, 81–98. [Google Scholar] [CrossRef]
- Abuawad, I.M.A. Mechanical and Surface Free Energy Characterization of Asphalt Concrete for Moisture Damage Detection. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign County, IL, USA, 2016; p. 179. Available online: https://pdfs.semanticscholar.org/7260/8d5a5564df3fbeec1c50857d5b759fa86c72.pdf (accessed on 13 February 2020).
- Cheng, D.; Little, D.N.; Lytton, R.; Holste, J.C. Surface energy measurement of asphalt and its application to predicting fatigue and healing in asphalt mixtures. Transp. Res. Rec. 2002, 1810, 44–53. [Google Scholar] [CrossRef]
- Little, D.L.; Allen, D.H.; Bhasin, A. Modeling and Design of Flexible Pavements and Materials; Springer International Publishing AG: Cham, Switzerland, 2018; p. 693. [Google Scholar] [CrossRef]
- Caro, S.; Masad, E.; Bhasin, A.; Little, D.N. Moisture susceptibility of asphalt mixtures, Part 2: Characterisation and modelling. Int. J. Pavement Eng. 2008, 9, 99–114. [Google Scholar] [CrossRef]
- Bagampadde, U.; Isacsson, U.; Kiggundu, B.M. Classical and Contemporary Aspects of Stripping in Bituminous Mixes. RMPD 2004, 5, 7–43. [Google Scholar] [CrossRef]
- Ensley, E.K. Multilayer adsorption with molecular orientation of asphalt on mineral aggregate and other substrates. J. Appl. Chem. Biotechnol. 1975, 25, 671–682. [Google Scholar] [CrossRef]
- Yao, H.; Dai, Q.; You, Z. Chemo-physical analysis and molecular dynamics (MD) simulation of moisture susceptibility of nano hydrated lime modified asphalt mixtures. Constr. Build. Mater. 2015, 101, 536–547. [Google Scholar] [CrossRef]
- Terrel, R.L.; Shute, J.W. Summary Report on Water Sensitivity. Report SHRP-A/IR-89-003; Strategic Highway Research Program, National Research Council: Washington, DC, USA, 1989; p. 102. Available online: http://onlinepubs.trb.org/Onlinepubs/shrp/shrp-A-IR-89-003/SHRP-AIR-003.pdf (accessed on 10 October 2019).
- Apeagyei, A.K.; Grenfell, J.R.A.; Airey, G.D. Moisture-induced strength degradation of aggregate–asphalt mastic bonds. RMPD 2014, 15, 239–262. [Google Scholar] [CrossRef]
- Van Oss, C.J.; Chaudhury, M.K.; Good, R.J. Interfacial Lifshitz-van der Waals and polar interactions in macroscopic systems. Chem. Rev. 1988, 88, 927–941. [Google Scholar] [CrossRef]
- Vansteenkiste, S.; Soenen, H. Correlation between Bitumen and Asphalt Properties. In Project report FunDBitS. CEDR Call 2013: Energy Efficient–Materials and Technology; 2016; p. 51. Available online: https://www.cedr.eu/download/other_public_files/research_programme/call_2013/energy_efficiency/fundbits/D.2e_bitumen-aggregate-interaction.pdf (accessed on 6 September 2019).
- Hefer, A.W. Adhesion in Bitumen-Aggregate Systems and Quantification of the Effects of Water on the Adhesive Bond. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2004; p. 222. Available online: http://hdl.handle.net/1969.1/1457 (accessed on 9 September 2019).
- Bhasin, A.; Masad, E.; Little, D.; Lytton, R. Limits on Adhesive Bond Energy for Improved Resistance of Hot-Mix Asphalt to Moisture Damage. Transp. Res. Rec. 2006, 1970, 3–13. [Google Scholar] [CrossRef]
- Little, D.N.; Bhasin, A. Using surface energy measurements to select materials for asphalt pavement. In Final Report for National Cooperative Highway Research Program RRD 316; National Cooperative Highway Research Program: Washington, DC, USA, 2007. [Google Scholar] [CrossRef]
- Arabani, M.; Hamedi, G.H. Using the surface free energy method to evaluate the effects of polymeric aggregate treatment on moisture damage in hot-mix asphalt. J. Mater. Civ. Eng. 2011, 23, 802–811. [Google Scholar] [CrossRef]
- Zhang, J.; Airey, G.D.; Grenfell, J.; Apeagyei, A.K. Moisture sensitivity examination of asphalt mixtures using thermodynamic, direct adhesion peel and compacted mixture mechanical tests. Road Mater. Pavement Des. 2016, 19, 120–138. [Google Scholar] [CrossRef]
- Alvarez, A.E.; Ovalles, E.; Caro, S. Assessment of the effect of mineral filler on asphalt–aggregate interfaces based on thermodynamic properties. Constr. Build. Mater. 2012, 28, 599–606. [Google Scholar] [CrossRef]
- Howson, J.; Masad, E.; Little, D.; Kassem, E. Relationship between bond energy and total work of fracture for asphalt binder-aggregate systems. Road Mater. Pavement Des. 2012, 13, 281–303. [Google Scholar] [CrossRef]
- Kim, S.-H.; Jeong, J.-H.; Kim, N. Use of Surface Free Energy Properties to Predict Moisture Damage Potential of Asphalt Concrete Mixture in Cyclic Loading Condition. KSCE J. Civ. Eng. 2003, 7, 381–387. [Google Scholar] [CrossRef]
- Grenfell, J.; Ahmad, N.; Liu, Y.; Apeagyei, A.; Large, D.; Airey, G. Assessing asphalt mixture moisture susceptibility through intrinsic adhesion bitumen stripping and mechanical damage. RMPD 2014, 15, 131–152. [Google Scholar] [CrossRef]
- Kloubek, J. Development of methods for surface free energy determination using contact angles of liquids on solids. Adv. Colloid. Interfac. 1992, 38, 99–142. [Google Scholar] [CrossRef]
- Bhasin, A. Development of Methods to Quantify Bitumen-Aggregate Adhesion and Loss of Adhesion Due to Water. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2006; p. 158. Available online: http://hdl.handle.net/1969.1/5934 (accessed on 9 September 2019).
- Bhasin, A.; Howson, J.; Masad, E.; Little, D.N.; Lytton, R.L. Effect on modification processes on bond energy of asphalt binders. Transp. Res. Rec. 2007, 1998, 29–37. [Google Scholar] [CrossRef]
- Bhasin, A.; Little, D.N.; Vasconcelos, K.L.; Masad, E. Surface free energy to identify moisture sensitivity of materials for asphalt mixes. Transp. Res. Rec. 2007, 2001, 37–45. [Google Scholar] [CrossRef]
- Hamedi, G.H.; Moghadas Nejad, F. Using energy parameters based on the surface free energy concept to evaluate the moisture susceptibility of hot mix asphalt. RMPD 2015, 16, 239–255. [Google Scholar] [CrossRef]
- Lyne, A.L.; Birgisson, B.; Redelius, P. Interaction forces between mineral aggregates and bitumen calculated using the hamaker constant. RMPD Spec. Issue Asph. Technol. 2010, 11, 305–323. [Google Scholar] [CrossRef]
- Israelachvili, J.N. Van der Waals forces in biological systems. Q. Rev. Biophys. 1973, 6, 341–387. [Google Scholar] [CrossRef]
- Hefer, A.; Little, D. Towards Quantification of Adhesion and Water Stripping in Bituminous Materials Using Modern Surface Energy Theory. In Proceedings of the 24th South African Transport Conference (SATC2005), Pretoria, South Africa, 12 July 2005; pp. 115–124. Available online: https://repository.up.ac.za/bitstream/handle/2263/6314/015.pdf;sequence=1 (accessed on 9 September 2019).
- Hefer, A.; Little, D.N. Adhesion in Bitumen-Aggregate Systems and Quantification of the Effects of Water on the Adhesive Bond. In Technical Report ICAR-505-1; Texas A&M University: College Station, TX, USA, 2005; p. 218. Available online: http://hdl.handle.net/2152/35371 (accessed on 9 September 2019).
- Masad, E.; Zollinger, C.; Bulut, R.; Little, D.N.; Lytton, R.L. Characterization of HMA moisture damage using surface energy and fracture properties. Assoc. Asph. Paving Technol. Proc. Tech. Sess. 2006, 75, 713–754. [Google Scholar]
- Wasiuddin, N.M.; Zaman, M.M.; O’Rear, E.A. Effect of Sasobit and Aspha-min on wettability and adhesion between asphalt binders and aggregates. Transp. Res. Rec. 2008, 2051, 80–89. [Google Scholar] [CrossRef]
- Howson, J.E.; Bhasin, A.; Masad, E.; Lytton, R.; Little, D. Development of a Database for Surface Energy of Aggregates and Asphalt Binder. In Report FHWA/TX-09/5-4524-01-1; Federal Highway Administration/Texas Deptartment of Transportation: Austin, TX, USA, 2009; p. 76. Available online: http://tti.tamu.edu/documents/5-4524-01-1.pdf (accessed on 9 September 2019).
- Bahramian, A. Evaluating surface energy components of asphalt binders using wilhelmy plate and sessile drop techniques. In Degree Project in Highway and Railway Engineering; KTH: Stockholm, Sweden, 2012; p. 40. Available online: https://www.diva-portal.org/smash/get/diva2:601925/FULLTEXT01.pdf (accessed on 9 September 2019).
- Moghadas Nejad, F.; Hamedi, G.H.; Azarhoosh, A.R. Use of surface free energy method to evaluate effect of hydrate lime on moisture damage in hot-mix asphalt. J. Mater. Civ. Eng. 2013, 25, 1119–1126. [Google Scholar] [CrossRef]
- Liu, Y.; Apeagyei, A.; Ahmad, N.; Grenfell, J.; Airey, G. Examination of moisture sensitivity of aggregate–bitumen bonding strength using loose asphalt mixture and physico-chemical surface energy property tests. Int. J. Pavement. Eng. 2014, 15, 657–670. [Google Scholar] [CrossRef]
- Luo, R.; Zhang, D.; Zeng, Z.; Lytton, R. Effect of surface tension on the measurement of surface energy components of asphalt binders using the Wilhelmy Plate Method. Constr. Build. Mater. 2015, 98, 900–909. [Google Scholar] [CrossRef]
- Shafabakhsh, G.H.; Faramarzi, M.; Sadeghnejad, M. Use of surface free energy method to evaluate the moisture susceptibility of sulfur extended asphalts modified with antistripping agents. Constr. Build. Mater. 2015, 98, 456–464. [Google Scholar] [CrossRef]
- Hamedi, G.H. Evaluating the effect of asphalt binder modification using nanomaterials on the moisture damage of hot mix asphalt. RMPD 2016, 18, 1375–1394. [Google Scholar] [CrossRef]
- Hamedi, G.H.; Moghadas Nejad, F. Evaluating the effect of mix design and thermodynamic parameters on moisture sensitivity of hot mix asphalt. J. Mater. Civ. Eng. 2017, 29, 04016207. [Google Scholar] [CrossRef]
- Porot, L.; Soenen, H.; Besamusca, J.; Apeagyei, A.; Grenfell, J.; Vansteenkiste, S.; Chailleux, E.; Gaudefroy, V.; Chaturabong, P.; Tozzo, C.; et al. Bituminous Binder. In Testing and Characterization of Sustainable Innovative Bituminous Materials and Systems; RILEM State-of-the-Art Reports 24, Partl; Porot, L., Di Benedetto, H., Canestrari, F., Marsac, P., Tebaldi, G., Eds.; Springer International Publishing: Cham, Switzerland, 2018; pp. 15–74. [Google Scholar] [CrossRef]
- Kakar, M.R.; Hamzah, M.O.; Akhtar, M.N.; Saleh, J.M. Evaluating the Surface Free Energy and Moisture Sensitivity of Warm Mix Asphalt Binders Using Dynamic Contact Angle. Adv. Civ. Eng. 2019, 19, 15. [Google Scholar] [CrossRef]
- Koc, M.; Bulut, R. Characterization of warm mix asphalt additives using direct contact angle measurements and surface free energies. In Proceedings of the Transportation Research Board 93rd Annual Meeting, Washington, DC, USA, 12–16 January 2014. [Google Scholar]
- Aguiar-Moya, J.P.; Salazar-Delgado, J.; Baldi-Sevilla, A.; Leiva-Villacorta, F.; Loria-Salazar, L. Effect of aging on adhesion properties of asphalt mixtures with the use of bitumen bond strength and surface energy measurement tests. Transp. Res. Rec. 2015, 2505, 57–65. [Google Scholar] [CrossRef]
- Ji, J.; Liu, L.; Suo, Z.; Zhai, P.; Yang, X.; You, Z. Adhesion Evaluation of Asphalt-Aggregate Interface Using Surface Free Energy Method. Appl. Sci. Basel 2017, 7, 156. [Google Scholar] [CrossRef]
- Moraes, R.; Velasquez, R.; Bahia, H. Using bond strength and surface energy to estimate moisture resistance of asphalt-aggregate systems. Constr. Build. Mater. 2017, 130, 156–170. [Google Scholar] [CrossRef]
- Korn, S. Beurteilung der Benetzbarkeit und des Adhäsionsvermögens von Bitumen mittels Kontaktwinkelmessungen. Ph.D. Thesis, Hamburg University of Applied Sciences, Hamburg, Germany, 2004. [Google Scholar]
- Hirsch, V.; Friemel-Göttlich, B. Bestimmung des adhäsiven Potentials von Bitumen und Gesteinsoberflächen mit Hilfe der Kontaktwinkel-messmethode. In Berichte der Bundesanstalt für Straßenwesen Straßenbau (BAsT); Heft S 59; Bergisch Gladbach, Germany, 2009; Available online: https://bast.opus.hbz-nrw.de/opus45-bast/frontdoor/deliver/index/docId/71/file/S59.pdf (accessed on 9 September 2019).
- Khan, A.; Redelius, P.; Kringos, N. Evaluation of adhesive properties of mineral-bitumen interfaces in cold asphalt mixtures. Constr. Build. Mater. 2016, 125, 1005–1021. [Google Scholar] [CrossRef]
- Wasiuddin, N.M.; Saltibus, N.E.; Mohammad, L.N. Novel moisture-conditioning method for adhesive failure of hot- and warm-mix asphalt binders. Transp. Res. Rec. 2011, 2208, 108–117. [Google Scholar] [CrossRef]
- Wistuba, M.P.; Grothe, H.; Grönniger, J.; Handle, F. Adhesion of bitumen: Screening and evaluating laboratory testing techniques. Proceedings of 5th Eurasphalt & Eurobitume Congress, Istanbul, Turkey, 13–15 June 2012; p. 12. [Google Scholar]
- Radenberg, M. Einfluss der chemischen, rheologischen und physikalischen Grundeigenschaften von Straßenbaubitumen auf das Adhäsionsverhalten unterschiedlicher Gesteinskörnungen. In Schlussbericht; 16639 N/1, Bochum, Germany, 2014; p. 104. Available online: http://www.lvw.ruhr-uni-bochum.de/mam/content/forschung/projekte/bericht_aif_igf_16639_n-1.pdf (accessed on 9 September 2019).
- Miller, C.M. Adhesion and the Surface Energy Components of Natural Minerals and Aggregates. Master’s Thesis, Texas A&M University, College Station, TX, USA, 2010; p. 218. Available online: http://hdl.handle.net/1969.1/ETD-TAMU-2010-08-8237 (accessed on 9 September 2019).
- Miller, C.; Little, D.N.; Bhasin, A. Surface energy characteristics of natural minerals and their impact of aggregate-bitumen bond strengths and asphalt mixture durability. Transp. Res. Rec. 2012, 2267, 45–55. [Google Scholar] [CrossRef]
- Azarion, Y.; Shirmohammadi, H.; Hamedi, G.H.; Saedi, D. Model for Predicting Moisture Susceptibility of Asphalt Mixtures Based on Material Properties. J. Mater. Civ. Eng. 2019, 31. [Google Scholar] [CrossRef]
- Porot, L.; Besamusca, J.; Soenen, H.; Apeagyei, A.; Grenfell, J.; Sybilski, D. Bitumen/aggregate affinity – RILEM Round Robin test. In 8th RILEM International Symposium on Testing and Characterization of Sustainable and Innovative Bituminous Materials; Canestrari, F., Partl, M.N., Eds.; Springer: Dordrecht, The Netherlands, 2016; RILEM 11; pp. 153–164. [Google Scholar] [CrossRef]
- Rudawska, A.; Jacniacka, E. Analysis for determining surface free energy uncertainty by the Owen-Wendt method. Int. J. Adhes. Adhes. 2009, 29, 451–457. [Google Scholar] [CrossRef]
- Cui, S.; Blackman, B.R.K.; Kinlock, A.J.; Taylor, A.C. Durability of asphalt mixtures: Effect of aggregate type and adhesion promoters. Int. J. Adhes. Adhes. 2014, 54, 100–111. [Google Scholar] [CrossRef]
- Horgnies, M.; Darque-Ceretti, E.; Fezai, H.; Felder, E. Influence of the interfacial composition on the adhesion between aggregates and bitumen: Investigations by EDX, XPS and peel tests. Int. J. Adhes. Adhes. 2011, 31, 238–247. [Google Scholar] [CrossRef]
- Lyne, A.L.; Redelius, P.; Collin, M.; Birgisson, B. Characterization of stripping properties of stone material in asphalt. Mater. Struct. 2013, 46, 47–61. [Google Scholar] [CrossRef]
- Lyne, A.L.; Krivosheeva, O.; Birgisson, B. Adhesion between bitumen and aggregate: Implementation of spectroscopic ellipsometry characterisation and estimation of Hamaker’s constant. Mater. Struct. 2013, 46, 1737–1745. [Google Scholar] [CrossRef]
- Schellenberger, W. Haftung zwischen Bitumen und Mineralstoff [Adhesion between bitumen and mineral aggregates]. In Asphalt; Germany, 2004; p. 8. [Google Scholar]
- Nehrings, A. Affinitätsproblem zwischen Gestein und Bitumen Lösungsansatz: Einsatz von Haftvermittlern. In Zeitschrift für Asphalt; Germany, 2009; p. 8. Available online: https://docplayer.org/33746588-Affinitaetsproblem-zwischen-gestein-und-bitumen-loesungsansatz-einsatz-von-haftvermittlern.html (accessed on 9 September 2019).
- Aguiar-Moya, J.P.; Loria-Salazar, L.; Salazar, J.; Villegas, E.; Corrales-Azofeifa, J.P.; Hajj, E.Y. Evaluation of Adhesion Properties of Costa Rican Asphalt Mixtures Using Bitumen Bond Strength and Contact-Angle Measurement Tests. Available online: https://www.lanamme.ucr.ac.cr/repositorio/bitstream/handle/50625112500/545/TRB%202013%20Evaluation%20of%20Adhesion%20Properties%20of%20Costa%20Rican%20Asphalt%20Mixtures.pdf?sequence=1&isAllowed=y (accessed on 9 September 2019).
- Aranowski, R.; Wojewodka, P.; Blazejowski, K. Determination of binder-aggregate adhesion by contact angle measurement. In Asphalt Pavements; Kim, Y.R., Ed.; Taylor&Francis Group: London, UK, 2014; pp. 617–624. [Google Scholar] [CrossRef]
- Apeagyei, A.K.; Grenfell, J.R.A.; Airey, G.D. Durability of asphalt mixtures exposed to long-term moisture conditioning. In Proceedings of the TRB 93rd Annual Meeting, Washington, DC, USA, 12–16 January 2014; pp. 1–16. Available online: https://www.researchgate.net/publication/260002328_Durability_of_asphalt_mixtures_exposed_to_long-term_moisture_conditioning (accessed on 27 January 2020).
- EN 12697-11. Bituminous Mixtures–Test Methods for Hot Asphalt–Part 11: Determination of the Affinity between Aggregates and Bitumen. European Committee for Standardization: Brussels, Belgium, 2012. [Google Scholar]
- AASHTO T283. Standard Method of Test for Resistance of Compacted Asphalt Mixtures to Moisture-Induced Damage; AASHTO: Washington, DC, USA, 2014. [Google Scholar]
- ASTM D 1664-80. Test Method for Coating and Stripping of Bitumen Aggregates Mixtures; ASTM International: West Conshohocken, PA, USA, 1985. [Google Scholar]
- ASTM D 3625-96. Standard Practice for Effect of Water on Bituminous-Coated Aggregate Using Boiling Water; ASTM International: West Conshohocken, PA, USA, 2005. [Google Scholar]
- EN 1097-7:2008. Tests for mechanical and physical properties of aggregates. In Determination of the Particle Density of Filler. Pyknometer Method; European Committee for Standardization: Brussels, Belgium, 2009. [Google Scholar]
- ASTM D 4541-17. Standard Test Method for Pull-Off Strength of Coatings Using Portable Adhesion Testers; ASTM International: West Conshohocken, PA, USA, 2017. [Google Scholar]
- Moghadas Nejad, F.; Asadi, M.; Hamedi, G.H.; Esmaeeli, M.R. Using hydrophobic coating on aggregate surfaces to reduce moisture damage in asphalt mixture. J. Mater. Civ. Eng. 2018, 30, 04018238. [Google Scholar] [CrossRef]
- Zhang, J.; Airey, G.D.; Grenfell, J.; Apeagyei, A.K.; Barrett, M. Development of a composite substrate peel test to assess moisture sensitivity of aggregate–bitumen bonds. Int. J. Adhes. Adhes. 2016, 68, 133–141. [Google Scholar] [CrossRef]
- Cordon, W.A. Properties, Evaluation and Control of Engineering Materials; McGraw-Hill College: New York, NY, USA, 1979; p. 550. ISBN 9780070131231. [Google Scholar]
- Lu, X.; Sjövall, P.; Soenen, H. Structural and chemical analysis of bitumen using time-of-flight secondary ion mass spectrometry (TOF-SIMS). Fuel 2017, 199, 206–218. [Google Scholar] [CrossRef]
- Blom, J.; Soenen, H.; Katsiki, A.; Van den Brande, N.; Rahier, H.; Van den bergh, W. Investigation of the bulk and surface microstructure of bitumen by atomic force microscopy. Constr. Build. Mater. 2018, 177, 158–169. [Google Scholar] [CrossRef]
- Ramm, A.; Downer, M.C.; Sakib, N.; Bhasin, A. Morphology and kinetics of as-phalt binder microstructure at gas, liquid, and solid interfaces. J. Microsc. 2019, 109–117. [Google Scholar] [CrossRef]
- Awaja, F.; Gilbert, M.; Kellya, G.; Foxa, B.; Pigram, P.J. Adhesion of polymers. Prog. Polym. Sci. 2009, 34, 948–968. [Google Scholar] [CrossRef]
| Reference(s) | Method to determine surface energies of bituminous binders |
| [18,20,21,23,24,26,28,29,30,34,35,36,37,38,39,40,41,42,43,44,45,46,47] | Wilhelmy plate tests in probe liquids (VCG), ambient |
| [20,39,48,49,50,51] | Sessile drops of probe liquids on bitumen surface (VCG) ambient |
| [18,20,35] | Inverse gas chromatography (CVS) |
| [46,52] | Pending drop (100–140 °C) combined with sessile drop on PTFE (OW) |
| [53] | Sessile drops on a microtome-cut bitumen surface 20 °C (OW) |
| [39,54] | Sessile drops of probe liquids on bitumen surface (OW) ambient |
| [55] | Dynamic sessile drop measurements of probe liquids on a bitumen surface (VCG) |
| [56] | Pending drops of bitumen (100–130 °C) (γ total) |
| [53] | Pending drops at equiviscous temperatures (γ total) |
| [57] | Pending drops of bitumen at a fixed G* 209 Pa (γ total) |
| [39] | Wilhelmy plate tests in probe liquids (OW) ambient |
| [20] | Atomic force microscopy (dispersive component) |
| Reference(s) | Method to determine surface energies of aggregates used in asphalt applications |
| [18,19,21,23,24,26,28,29,30,35,36,38,40,41,45,46,58,59,60] | Universal sorption device (VCG) |
| [49,50,51,55] | Sessile drops of probe liquids on flat aggregate (VCG) |
| [53,57,61] | Sessile drops of probe liquids on flat aggregate (OW) |
| [20,28] | Micro calorimeter (VCG) |
| [20] | Inverse gas chromatography (VCG) |
| γTotal | γLW | γAB | γ+ | γ− | Reference(s) | Notes |
|---|---|---|---|---|---|---|
| mJ/m2 | mJ/m2 | mJ/m2 | mJ/m2 | mJ/m2 | ||
| 14–32 | 13–32 | 0–3 | 0–1.5 | 0–3 | [18,20,24,28,29,30,35,36] | |
| 12.1–12.5 | 10.6–11.2 | 1.3–1.5 | 0.7–0.8 | 0.6–0.7 | [55] | |
| 15.6 | 13.7 | 1.9 | 1.5 | 0.7 | [21] | |
| 19.1–30.6 | 18.8–30.6 | 0–0.4 | 0–0.34 | 0–5.08 | [15,26,41,46] | |
| 33.6–38.1 | 32.9–37.5 | – | 0.0–0.01 | 2.1–3.5 | [22] | Two binders |
| 14.0–15.6 | 12.5–13.5 | 1.5–1.9 | 1.4–1.5 | 0.4–0.7 | [31] | Two binders |
| 14.2–15.9 | 11.8–13.7 | 2.2–2.4 | 2.6–3.0 | 0.5–0.5 | [45] | Two binders |
| 10.45 | 6.85 | 3.60 | 3.47 | 0.93 | [42] | Revised calculation method; one unmodified binder |
| 20.2 | 19.3 | 0.9 | 1.2 | 0.2 | [43] | One unmodified binder |
| 26–39 | 18–33 | 6–8 | 4.9–6.7 | 1.7–2.4 | [39] | Six pen grade 70/100 binders |
| 20.5 | 17.8 | 2.5 | 1.5 | 1.2 | [47] | One unmodified binder |
| Effect Studied | Finding(s)—Conclusion(s) | Reference(s) * |
|---|---|---|
| Type of bitumen | • γtotal parafinic -> γtotal naphthenic; γAB napthenic -> γAB paraffinic; | [52] |
| • γAB napthenic -> γAB paraffinic; | ||
| • SHRP library binders, differences between the binders were observed but this did not relate to another bitumen parameter; | [18,20,28,34,35] | |
| • Very little difference between binders in γtotal. | [53,57] | |
| Antistrip agent (0.4%–1% addition) | ✓ Slight increase in γtotal but decrease in γAB; | [52] |
| ✓ No clear trend on surface energy and SFE components; | [18,20,28,35] | |
| ✓ γtotal and γ− increase slightly, only for the harder binder; | [41] | |
| ✓ γtotal, γLW, and γ− increase slightly; | [48] | |
| ✓ γtotal, γLW, and γ− increase; | [31,45,60] | |
| ✓ Method A (see text) γLW ≈ 40, γAB ≈ 0; | [54] | |
| ✓ Method B (see text) γLW ≈ 20–25, γAB ≈ 10–40. | ||
| Hydrated lime | • No effect. | [20,49] |
| Wax addition 3% wax addition | • Slight increase in γtotal and γAB due to wax; | [52] |
| sasobit (2%–8%), paraffin wax (8%) | • Sasobit decreases γtotal, γ+ is increased no effect on γ− similar effect of paraffin wax, no effect of aspha-min; | [37,55] |
| sasobit (0.5%–8.0%), evotherm (0.5%–1.5%) | • γtotal, γLW, and γ− increase slightly; | [48] |
| sasobit (3%), and RH (3%) (RH is a type of organic wax) | • For sasobit: for one binder γtotal decreases, for the second binder γtotal increases, basic part increases, acidic part decreases. For RH: For both binders γtotal decreases, basic part increases acidic part decreases. | [50] |
| Polymer modification [46,52,53]: SBS | ✓ No effect on γtotal; | [52] |
| ✓ γtotal increases; | [53] | |
| [49]: SBS (3%), SBR (3%) | ✓ SBS results in an increase in γLW, decrease in γAB, SBR almost no effect; | [49] |
| ✓ γtotal increases slightly for Wilhelmy plate tests at 23 °C, no effect for pendant drop tests at 120 °C. | [46] | |
| Ageing (RTFOT + PAV) | • γ− increases slightly; | [20] |
| • No clear effect on γtotal; | [18] | |
| • γtotal increases, γLW increases, γ+ decreases, γ− varies, γAB decreases; | [49] | |
| • Increase after RTFOR, reduction after PAV in γtotal, similar trend for LW and basic component, the acidic component increases after each aging step. | [47] | |
| Nanomaterials carbon nanotubes 3%, nano-SiO2 (6%), diatomite (6%) | ✓ These additives have almost no influence. | [49] |
| WMA additive Aspha-min (1%–6%) | • No effect; | [37] |
| Cecabase RT® (0.2%–0.4%) | • γtotal increases; | [47] |
| Zycotherm (NZ) | • γtotal and all components increase. | [43] |
| Materials | Lab Tests | Ref. nr. |
|---|---|---|
| Four binder–aggregate combinations; two binders (AAD-1 and AAM-1); two aggregates (a Texas limestone and a river gravel), the gravel aggregate was tested with and without hydrated lime. Mixtures with 4% air voids. | - Repeated compressive loading, in controlled stress mode, at 40 °C, 1 Hz haversine loading; - Water conditioning prior to the testing. | [71] |
| Twelve binder–aggregate combinations: Four binders (AAB, AAD, ABD, AAE), and three aggregates (granite, basalt, gravel). Mixtures with 4% air voids. | - Dynamic modulus in compression and in tension; dynamic creep; fatigue: Number of cycles to reach 1% permanent μ strain; - Water conditioning prior to testing; submerging in deionized water for 24 h at 50 °C, followed by air drying for 24 h. | [20,28,30] |
| Six binder aggregate combinations: Three binders (AAB, AAD, and ABD).Two aggregates: Limestone (good field performance in terms of resistance to moisture damage) + andesite (poor observed field performance). | - Uniaxial binder pull-off; samples were all prepared with a film thickness of 30 μm and tested at 23 °C, at a loading rate of 0.01 mm/s (aggregates surface slightly polished); - Binder–aggregate samples were submerged in distilled water for time periods of 0, 12, 24, and 48 h. | [24] |
| Twenty-five binder–aggregate combinations: Five stones, five binders. | Rolling bottle test (EN 12697-11 clause 5 [72]). | [53] |
| Three binder–aggregate combinations: One binder (60/70 pen grade) + three aggregates (limestone, granite, and quartzite), each aggregate was tested in three forms; unmodified and coated with two types of polyethylene (HDPE, LDPE), 0.43% by dry weight of aggregate. Mixtures with 4% air voids. | - Repeated unconfined, compressive loading, in controlled stress mode at 25 °C and at 1 Hz under haversine loading; - Preconditioning: AASHTO T283 [73]. | [21] |
| Four binder–aggregate combinations, an unmodified PG 64-22, and a PmB PG 76-22. Binders were also tested after adding Sasobit and an amine-based liquid antistrip additive at 1% and 2%. Two aggregates: Limestone + novaculite. | - A modified PATTI test, pull-off tensile strength; - Plain glass plates, coated with bitumen, placed overnight in an oven at 64 °C in submerged condition. | [55] |
| Two unmodified binders (40/60 +160/220); 40/60 binder was modified with liquid antistripping agents (0.5 wt%)and four amine-based and one non-amine antistripping agent;three limestone + three granite aggregates. | - Static immersion test (ASTM D1664 [74]); - Rolling bottle test (EN 12697-11 [72]); - Boiling water test (ASTM D3625-96 [75]); - A total water immersion test; - An ultrasonic method. | [41] |
| Four aggregates: Two limestone + two granite; three binders: 15, 50 + 100 pen grades; aggregates similar to [41]. | - Rolling bottle test (EN 12697-11 [72]); - Saturated ageing tensile stiffness (SATS) (combined ageing and water damage). | [26] |
| One limestone aggregate, two mineral fillers (granite and limestone), a 40/60 pen binder compaction to three nominal air void levels (4%, 6%, and 8%). | - Indirect tensile stiffness (20 °C); - Indirect tensile strength (ITS) tests (20 °C); - Water immersion at 60 °C, up to 70 days. | [71] |
| - Limestone and granite fine aggregate: Passing the 1 mm sieve and retained on 0.125 mm sieve. - Limestone and granite mineral fillers satisfying EN 1097-7-2008 [76]. - A 40/60 pen grade. | - Mineralogy; - Aggregate and mastic moisture absorption; - Mastic adhesion strength and mastic cohesion; - Water conditioning, depended on the test method. | [15] |
| Two binders (40/60, 70/100); five aggregates (two limestones + three granite). | - Tensile force during a binder peel-off test; - SATS tests [26]; - Whole specimens were submersed in water at 20 °C for 7 and 14 days. | [22] |
| Four binders (AC 60-70); eight aggregates; (three limestones, two granite, two sandstones and quartzite) one liquid antistrip additive. | Modified Lottman indirect tension test; procedure (AASHTO T283 [73]). | [31] |
| Twenty-four combinations: Three aggregates (limestone, granite and quartzite, each with and without nano CaCO3) and two binders (AC 60-70 and 85-100; with and without nano ZnO and Wetfix BE).Mixtures with air void 7%. | - Modified Lottman indirect tension test procedure (AASHTO T283 [73]) [45]; - Number of cycles to failure wet/dry [60]. | [45,60] |
| Four aggregates, various empirical degrees of stripping (limestone, basal, greywacke, and granite) and three binders, two unmodified, and one polymer modified. | - Rolling bottle test (EN 12697-11 clause 5 [72]); - Boiling water test (EN 12697-11 clause 7 [72]); - Bitumen bond strength (ASTM D 4541 [77]). | [46] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Soenen, H.; Vansteenkiste, S.; Kara De Maeijer, P. Fundamental Approaches to Predict Moisture Damage in Asphalt Mixtures: State-of-the-Art Review. Infrastructures 2020, 5, 20. https://doi.org/10.3390/infrastructures5020020
Soenen H, Vansteenkiste S, Kara De Maeijer P. Fundamental Approaches to Predict Moisture Damage in Asphalt Mixtures: State-of-the-Art Review. Infrastructures. 2020; 5(2):20. https://doi.org/10.3390/infrastructures5020020
Chicago/Turabian StyleSoenen, Hilde, Stefan Vansteenkiste, and Patricia Kara De Maeijer. 2020. "Fundamental Approaches to Predict Moisture Damage in Asphalt Mixtures: State-of-the-Art Review" Infrastructures 5, no. 2: 20. https://doi.org/10.3390/infrastructures5020020
APA StyleSoenen, H., Vansteenkiste, S., & Kara De Maeijer, P. (2020). Fundamental Approaches to Predict Moisture Damage in Asphalt Mixtures: State-of-the-Art Review. Infrastructures, 5(2), 20. https://doi.org/10.3390/infrastructures5020020






