Design Optimization of Polymer Heat Exchanger for Automated Household-Scale Solar Water Pasteurizer
Abstract
:1. Introduction
2. Background
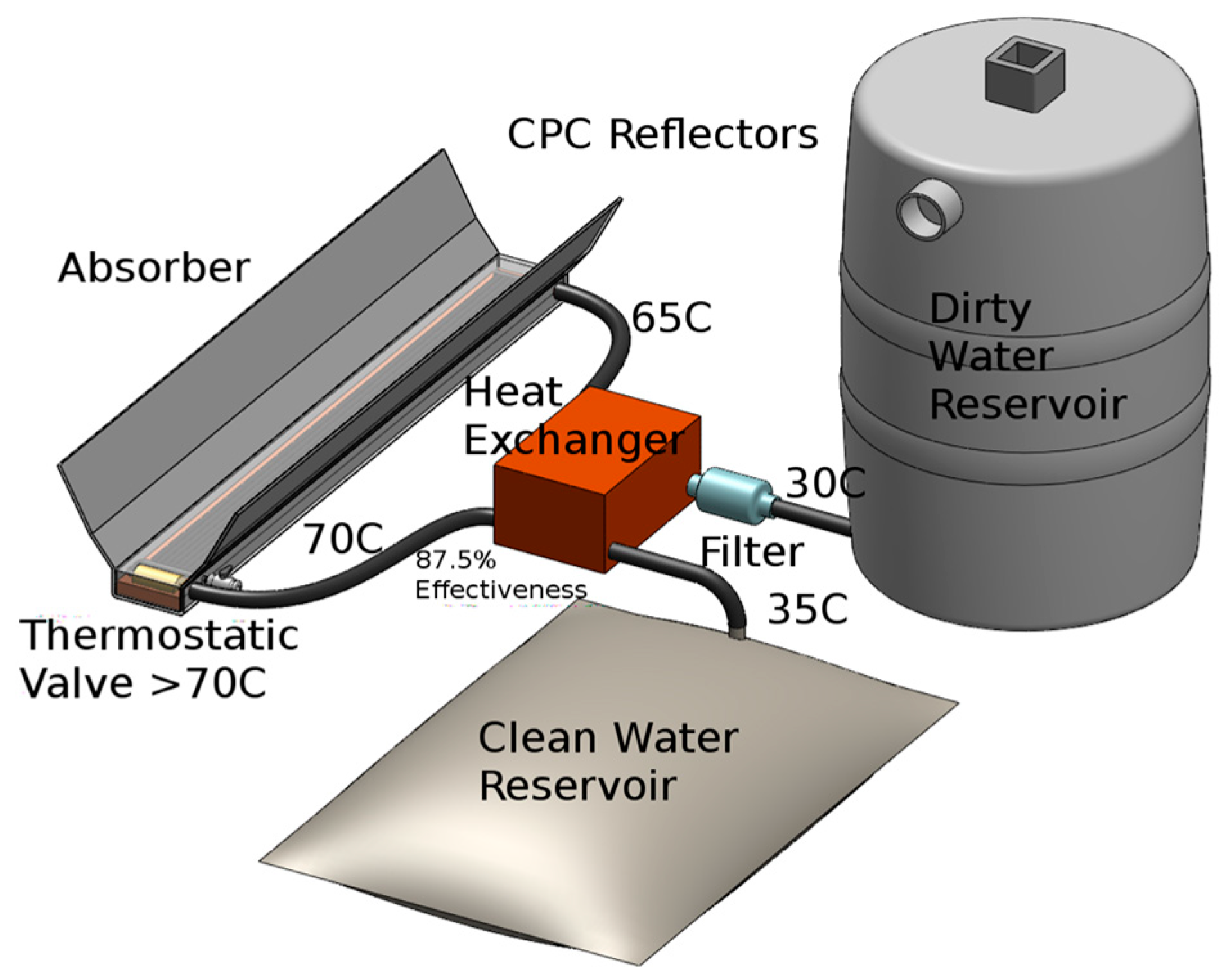
2.1. Flow-Through Solar Water Pasteurizer
2.2. Microchannel Heat Exchangers
2.3. Approaches to Achieving High Effectiveness with Polymer Microchannel HXs
- 1 mm polymer wall thickness has little effect when h ~ 100 W/(m2K), which turbulent gas flow or with laminar gas flow in 1 mm diameter channels can achieve.
- 0.1 mm polymer wall thickness has little effect when h ~ 1000 W/(m2K), which laminar gas flow in 0.1 mm diameter tubes or with turbulent liquid flow or laminar liquid flow in 1 mm channels can achieve.
- 0.01 mm polymer wall thickness has little effect when h ~ 10,000 W/(m2K), which laminar liquid flow in 0.1 mm diameter channels can achieve.
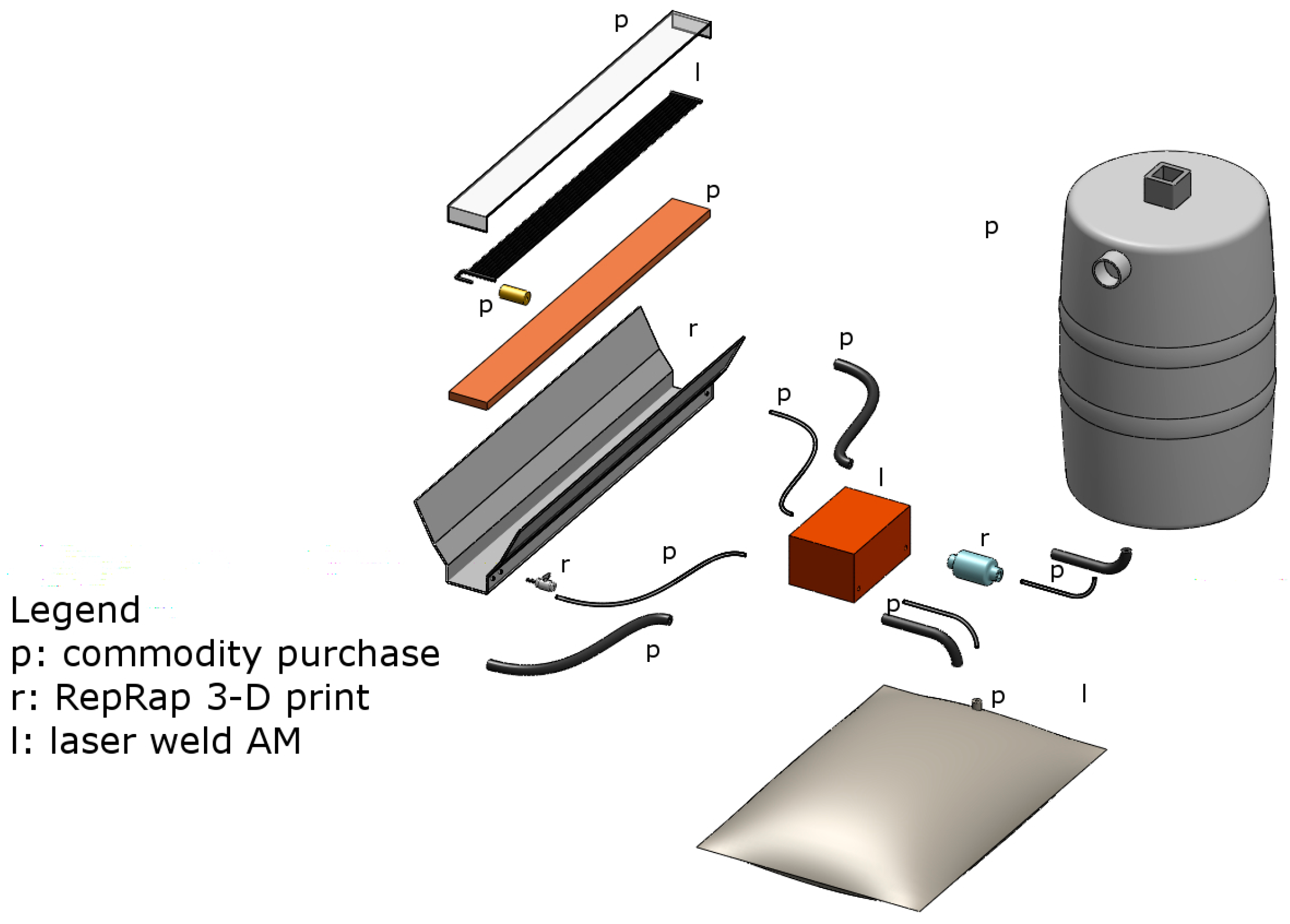
3. Materials and Methods
4. Results
4.1. Simulation Results
4.2. Case Study 1: Substitution of Metal HX with Polymer HX in Solar Water Pasteurizer
4.3. Case Study 2: Design for 3-D Printed Collector of Arbitrary Size
5. Discussion
5.1. Challenges of Polymer HXs
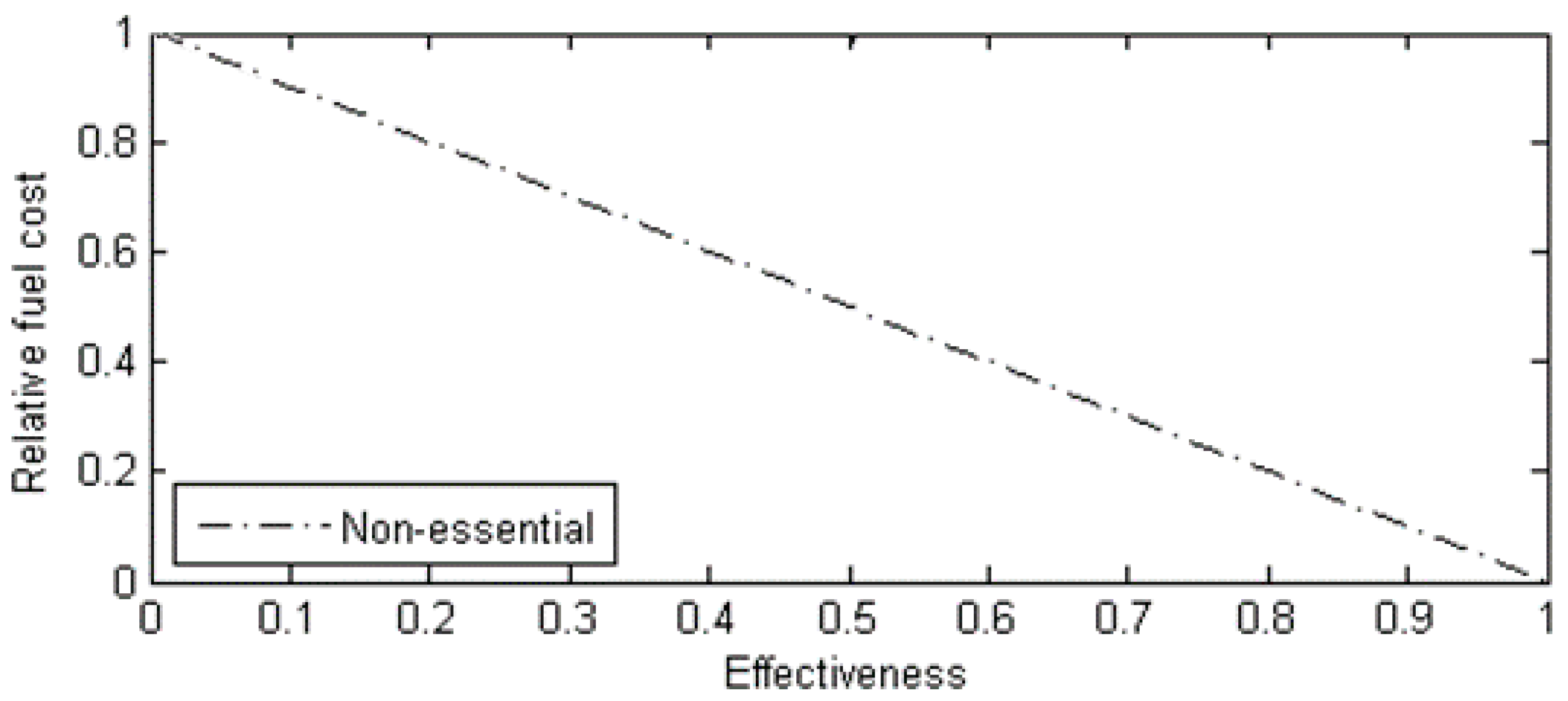
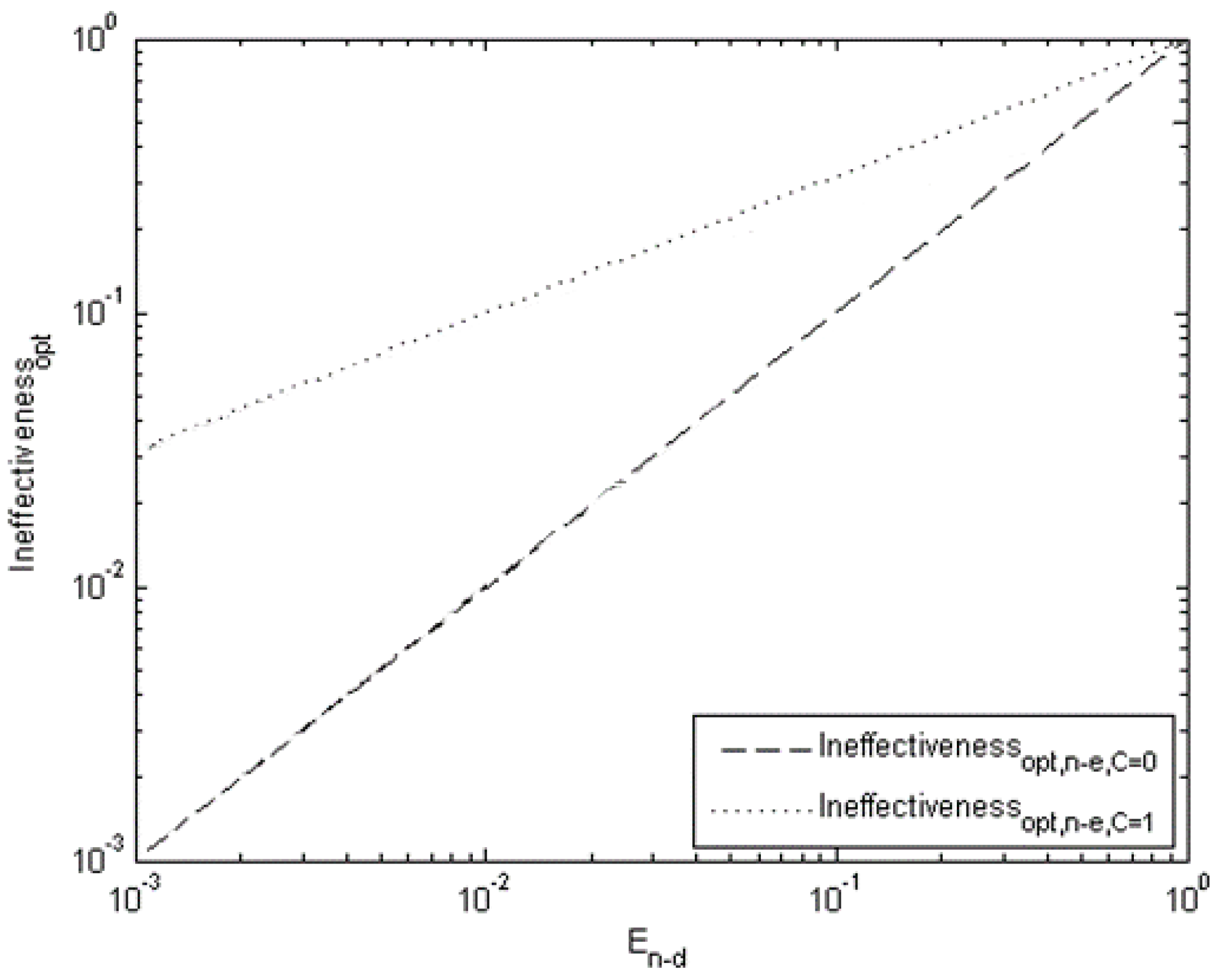
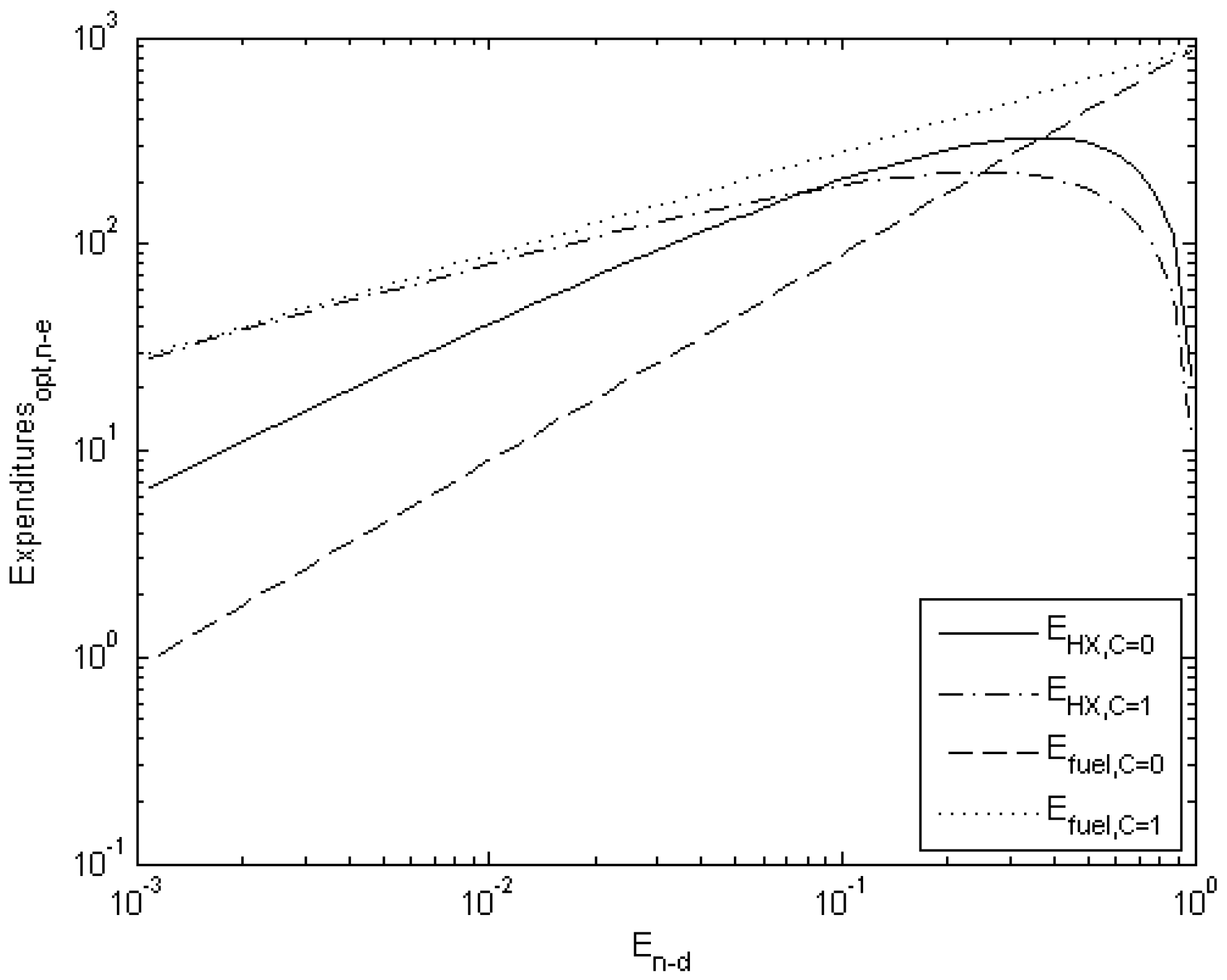
5.2. Economics
5.3. Future Work
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| Symbol | Units | Explanation |
|---|---|---|
| A | m2 | Heat transfer area |
| C | - | Heat capacity rate ratio |
| Cc | W/K | Cold heat capacity rate |
| Ch | W/K | Hot heat capacity rate |
| Cp | J/(kgK) | Specific heat at constant pressure |
| Efuel | $ | Expenditure on fuel |
| EHX | $ | Expenditure on heat exchanger |
| En-d | - | Non-dimensional expenditure |
| h | W/(m2K) | Heat transfer coefficient |
| H | hours/year | Utilization of the heat exchanger |
| HDPE | - | High density polyethylene |
| HX | - | Heat exchanger |
| k | W/(mK) | Thermal conductivity |
| k1 | $/m2 | Price per heat transfer area, constant for optimization |
| k2 | 1/m2 | Inverse of area required for one NTU, constant for optimization |
| k3 | $ | Fuel expenditure for zero effectiveness for non-essential heat exchanger case, or unit effectiveness for essential, constant for optimization |
| LDPE | - | Low density polyethylene |
| LLDPE | - | Linear low density polyethylene |
| kg/s | Mass flow rate | |
| NTU | - | Number of transfer units |
| Nu | - | Nusselt number |
| Pfuel | $/GJ | Price of fuel |
| PHT | $/(W/K) | Price per heat transfer ability |
| PNTU | $ | Price per NTU |
| PV | $/m3 | Price of heat exchanger material per volume |
| PP | - | Polypropylene |
| PS | - | Polystyrene |
| W | Heat transfer rate | |
| r | - | Interest rate |
| t | m | Wall thickness |
| T | °C | Temperature |
| U | W/(m2K) | Overall heat transfer coefficient |
| Greek | ||
| ΔTf | K | Temperature change of one of the fluids |
| ΔTi | K | Logarithmic mean temperature difference between the hot and cold fluid inside the heat exchanger |
| ΔTt | K | Total temperature difference for the heat exchanger |
| η | - | Effectiveness |
| Subscripts | ||
| c | - | Cold |
| e | - | Essential heat exchanger case |
| h | - | Hot |
| HT | - | Heat transfer |
| i | - | In |
| max | - | Maximum |
| n-e | - | Non-essential heat exchanger case |
| o | - | Out |
| opt | - | Optimum |
| t | - | Total |
| w | - | Wall |
References
- World Health Organization. Monitoring Health for the SDGs: Sustainable Development Goals; World Health Statistics; World Health Organization: Geneva, Switzerland, 2017; ISBN 978-92-4-156548-6. [Google Scholar]
- World Health Organization. Preventing Diarrhoea through Better Water, Sanitaiton and Hygiene: Exposures and Impacts in Low- and Middle-Income Countries; World Health Organization: Geneva, Switzerland, 2014; ISBN 978-92-4-156482-3. [Google Scholar]
- Prüss-Üstün, A.; Wolf, J.; Corvalán, C.; Bos, R.; Neira, M. Preventing Disease through Healthy Environments: A Global Assessment of the Burden of Disease from Environmental Risks, 2nd ed.; World Health Organization: Geneva, Switzerland, 2016; ISBN 978-92-4-156519-6. [Google Scholar]
- WHO/UNICEF Joint Water Supply and Sanitation Monitoring Programme. Progress on Sanitation and Drinking Water; World Health Organization; UNICEF: Geneve, Switzerland, 2015; ISBN 978-92-4-150914-5. [Google Scholar]
- Hutton, G.; Varughese, M. The Costs of Meeting the 2030 Sustainable Development Goal Targets on Drinking Water, Sanitation, and Hygiene; The World Bank: Washington, DC, USA, 2016. [Google Scholar]
- Hardoon, D. Wealth: Having it All and Wanting More; Policy & Practice; Oxfam International: Oxford, UK, 2015. [Google Scholar]
- Blanco, J.; Malato, S.; Fernández-Ibañez, P.; Alarcón, D.; Gernjak, W.; Maldonado, M.I. Review of feasible solar energy applications to water processes. Renew. Sustain. Energy Rev. 2009, 13, 1437–1445. [Google Scholar] [CrossRef]
- Khattab, N.M.; Soliman, H.; Metias, M.; El-Seesy, I.; Mettawee, E.; El-Shenawy, E.; Hassan, M. Implementation of Solar Technologies in the Development of Rural, Remote and Sub urban Communities. Int. J. Therm. Environ. Eng. 2010, 3, 59–66. [Google Scholar] [CrossRef]
- Kaushal, A. Varun Solar stills: A review. Renew. Sustain. Energy Rev. 2010, 14, 446–453. [Google Scholar] [CrossRef]
- Velmurugan, V.; Srithar, K. Performance analysis of solar stills based on various factors affecting the productivity—A review. Renew. Sustain. Energy Rev. 2011, 15, 1294–1304. [Google Scholar] [CrossRef]
- Arunkumar, T.; Velraj, R.; Denkenberger, D.C.; Sathyamurthy, R.; Kumar, K.V.; Ahsan, A. Productivity enhancements of compound parabolic concentrator tubular solar stills. Renew. Energy 2016, 88, 391–400. [Google Scholar] [CrossRef]
- Arunkumar, T.; Denkenberger, D.; Ahsan, A.; Jayaprakash, R. The augmentation of distillate yield by using concentrator coupled solar still with phase change material. Desalination 2013, 314, 189–192. [Google Scholar] [CrossRef]
- Arunkumar, T.; Jayaprakash, R.; Denkenberger, D.; Ahsan, A.; Okundamiya, M.; Tanaka, H.; Aybar, H. An experimental study on a hemispherical solar still. Desalination 2012, 286, 342–348. [Google Scholar] [CrossRef]
- Kabeel, A.; Arunkumar, T.; Denkenberger, D.; Sathyamurthy, R. Performance enhancement of solar still through efficient heat exchange mechanism—A review. Appl. Therm. Eng. 2017, 114, 815–836. [Google Scholar] [CrossRef]
- Suneesh, P.; Paul, J.; Jayaprakash, R.; Kumar, S.; Denkenberger, D. Augmentation of distillate yield in “V”-type inclined wick solar still with cotton gauze cooling under regenerative effect. Cogent Eng. 2016, 3, 1202476. [Google Scholar] [CrossRef]
- McGuigan, K.G.; Conroy, R.M.; Mosler, H.-J.; du Preez, M.; Ubomba-Jaswa, E.; Fernandez-Ibañez, P. Solar water disinfection (SODIS): A review from bench-top to roof-top. J. Hazard. Mater. 2012, 235–236, 29–46. [Google Scholar] [CrossRef] [PubMed]
- Dawney, B.; Pearce, J.M. Optimizing the solar water disinfection (SODIS) method by decreasing turbidity with NaCl. J. Water Sanitat. Hyg. Dev. 2012, 2, 87–94. [Google Scholar] [CrossRef]
- Byrne, J.A.; Fernandez-Ibañez, P.A.; Dunlop, P.S.M.; Alrousan, D.M.A.; Hamilton, J.W.J. Photocatalytic Enhancement for Solar Disinfection of Water: A Review. Int. J. Photoenergy 2011, 798051. [Google Scholar] [CrossRef]
- Oates, P.M.; Shanahan, P.; Polz, M.F. Solar disinfection (SODIS): Simulation of solar radiation for global assessment and application for point-of-use water treatment in Haiti. Water Res. 2003, 37, 47–54. [Google Scholar] [CrossRef]
- Dawney, B.; Cheng, C.; Winkler, R.; Pearce, J.M. Evaluating the geographic viability of the solar water disinfection (SODIS) method by decreasing turbidity with NaCl: A case study of South Sudan. Appl. Clay Sci. 2014, 99, 194–200. [Google Scholar] [CrossRef]
- Pearce, J.M.; & Denkenberger, D.C. Numerical simulation of the direct application of compound parabolic concentrators to a single effect basin solar still. In Proceedings of the 2006 International Conference of Solar Cooking and Food Processing, Granada, Spain, 12–16 July 2006; Volume 18. [Google Scholar]
- Denkenberger, D.C.; Pearce, J.M. Compound parabolic concentrators for solar water heat pasteurization: Numerical simulation. In Proceedings of the 2006 International Conference of Solar Cooking and Food Processing, Granada, Spain, 12–16 July 2006; Volume 18. [Google Scholar]
- Burch, J.D.; Thomas, K.E. Water disinfection for developing countries and potential for solar thermal pasteurization. Solar Energy 1998, 64, 87–97. [Google Scholar] [CrossRef]
- Burch, J.; Thomas, K.E. An Overview of Water Disinfection in Developing Countries and the Potential for Solar Thermal Water Pasteurization; National Renewable Energy Lab.: Golden, CO, USA, 1998.
- Safapour, N.; Metcalf, R.H. Enhancement of Solar Water Pasteurization with Reflectors. Appl. Environ. Microbiol. 1999, 65, 859–861. [Google Scholar] [PubMed]
- Onyango, E.A.; Thoruwa, T.F.N.; Maingi, S.M.; Njagi, E.M. Performance of a 2-element plane reflector augmented galvanised pipe flat plate collector for solar water pasteurisation. J. Food Technol. 2009, 7, 12–19. [Google Scholar]
- Duff, W.S.; Hodgson, D.A. A simple high efficiency solar water purification system. Solar Energy 2005, 79, 25–32. [Google Scholar] [CrossRef]
- Bigoni, R.; Kötzsch, S.; Sorlini, S.; Egli, T. Solar water disinfection by a Parabolic Trough Concentrator (PTC): Flow-cytometric analysis of bacterial inactivation. J. Clean. Prod. 2014, 67, 62–71. [Google Scholar] [CrossRef]
- Dayem, A.M.A.; El-Ghetany, H.H.; El-Taweel, G.E.; Kamel, M.M. Thermal performance and biological evaluation of solar water disinfection systems using parabolic trough collectors. Desalin. Water Treat. 2011, 36, 119–128. [Google Scholar] [CrossRef]
- Abraham, J.P.; Plourde, B.D.; Minkowycz, W.J. Continuous flow solar thermal pasteurization of drinking water: Methods, devices, microbiology, and analysis. Renew. Energy 2015, 81, 795–803. [Google Scholar] [CrossRef]
- Amsberry, A.; Tyler, C.; Steinhauff, W.; Pommerenck, J.; Yokochi, A.T.F. Simple Continuous-Flow Device for Combined Solar Thermal Pasteurization and Solar Disinfection for Water Sterilization. J. Humanit. Eng. 2015, 3, 1–7. [Google Scholar]
- Carielo da Silva, G.; Tiba, C.; Calazans, G.M.T. Solar pasteurizer for the microbiological decontamination of water. Renew. Energy 2016, 87, 711–719. [Google Scholar] [CrossRef]
- Carielo, G.; Calazans, G.; Lima, G.; Tiba, C. Solar water pasteurizer: Productivity and treatment efficiency in microbial decontamination. Renew. Energy 2017, 105, 257–269. [Google Scholar] [CrossRef]
- Saitoh, T.S.; El-Ghetany, H.H. Solar water-sterilization system with thermally-controlled flow. Appl. Energy 1999, 64, 387–399. [Google Scholar] [CrossRef]
- Konersmann, L.; Frank, E. Solar Water Disinfection: Field Test Results and Implementation Concepts; International Solar Energy Society: Freiburg im Breisgau, Germany, 2011; pp. 1–11. [Google Scholar]
- Pearce, J.M. The case for open source appropriate technology. Environ. Dev. Sustain. 2012, 14, 425–431. [Google Scholar] [CrossRef]
- Buitenhuis, J.; Pearce, J.M. Open design-based strategies to enhance appropriate technology development. In Proceedings of the 14th Annual National Collegiate Inventors and Innovators Alliance Conference, San Francisco, CA, USA, 25–27 March 2010; pp. 1–12. [Google Scholar]
- Oberloier, S.; Pearce, J.M. General Design Procedure for Free and Open-Source Hardware for Scientific Equipment. Designs 2017, 2, 2. [Google Scholar] [CrossRef]
- Pearce, J.M.; Blair, C.M.; Laciak, K.J.; Andrews, R.; Nosrat, A.; Zelenika-Zovko, I. 3-D Printing of Open Source Appropriate Technologies for Self-Directed Sustainable Development. J. Sustain. Dev. 2010, 3, 17. [Google Scholar] [CrossRef]
- King, D.L.; Babasola, A.; Rozario, J.; Pearce, J.M. Mobile Open-Source Solar-Powered 3-D Printers for Distributed Manufacturing in Off-Grid Communities. Chall. Sustain. 2014, 2, 18–27. [Google Scholar] [CrossRef]
- Gwamuri, J.; Franco, D.; Khan, K.Y.; Gauchia, L.; Pearce, J.M. High-Efficiency Solar-Powered 3-D Printers for Sustainable Development. Machines 2016, 4, 3. [Google Scholar] [CrossRef]
- Andreatta, D.; Yegian, D.; Connelly, L.; Metcalf, R. Recent advances in devices for the heat pasteurization of drinking water in the developing world. In Proceedings of the Intersociety Energy Conversion Engineering Conference, 1994; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1994; p. 1741. [Google Scholar] [CrossRef]
- Zaheed, L.; Jachuck, R.J.J. Review of polymer compact heat exchangers, with special emphasis on a polymer film unit. Appl. Ther. Eng. 2004, 24, 2323–2358. [Google Scholar] [CrossRef]
- Ashman, S.; Kandlikar, S.G. A Review of Manufacturing Processes for Microchannel Heat Exchanger Fabrication. In Proceedings of the ASME 4th International Conference on Nanochannels, Microchannels, and Minichannels, Limerick, Ireland, 19–21 June 2006; pp. 855–860. [Google Scholar] [CrossRef]
- Denkenberger, D.C.; Brandemuehl, M.J.; Pearce, J.M.; Zhai, J. Expanded microchannel heat exchanger: Design, fabrication, and preliminary experimental test. Proc. Inst. Mech. Eng. A 2012, 226, 532–544. [Google Scholar] [CrossRef]
- Garst, S.; Schuenemann, M.; Solomon, M.; Atkin, M.; Harvey, E. Fabrication of multilayered microfluidic 3D polymer packages. In Proceedings of the ECTC ’05 Electronic Components and Technology, Lake Buena Vista, FL, USA, 31 May–4 June 2005; Volume 1, pp. 603–610. [Google Scholar]
- Bachmann, F.G.; Russek, U.A. Laser welding of polymers using high-power diode lasers. In Proceedings of the Photon Processing in Microelectronics and Photonics; International Society for Optics and Photonics: San Jose, CA, USA, 18 June 2002; Volume 4637, pp. 505–519. [Google Scholar]
- Pearce, J.M. Open-Source Lab: How to Build Your Own Hardware and Reduce Research Costs; Elsevier: New York, NY, USA, 2013; ISBN 978-0-12-410486-0. [Google Scholar]
- Laureto, J.J.; Dessiatoun, S.V.; Ohadi, M.M.; Pearce, J.M. Open Source Laser Polymer Welding System: Design and Characterization of Linear Low-Density Polyethylene Multilayer Welds. Machines 2016, 4, 14. [Google Scholar] [CrossRef]
- Arie, M.A.; Shooshtari, A.H.; Tiwari, R.; Dessiatoun, S.V.; Ohadi, M.M.; Pearce, J.M. Experimental characterization of heat transfer in an additively manufactured polymer heat exchanger. Appl. Ther. Eng. 2017, 113, 575–584. [Google Scholar] [CrossRef]
- Denkenberger, D.; Parisi, M.; Pearce, J.M. Towards Low-Cost Microchannel Heat Exchangers: Vehicle Heat Recovery Ventilator Prototype. In Proceedings of the 10th International Conference on Heat Transfer, Fluid Mechanics and Thermodynamics (HEFAT), Orlando, FL, USA, 14–16 July 2014. [Google Scholar]
- Borbely, A.-M.; Kreider, J.F. Distributed Generation; CRC Press: New York, NY, USA, 2001. [Google Scholar]
- Smith, E.M. Advances in Thermal Design of Heat Exchangers: A numerical approach: Direct-Sizing, Step-Wise Rating, and Transients; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Grandinetti, J. Safe Water Systems. Personal communication, 2010. [Google Scholar]
- Grafman, L. To Catch the Rain. 2018. Available online: http://www.tocatchtherain.org/ (accessed on 23 March 2018).
- Meera, V.; Ahammed, M.M. Water quality of rooftop rainwater harvesting systems: A review. J. Water Supply Res. Technol. AQUA 2006, 55, 257–268. [Google Scholar]
- Reyneke, B.; Cloete, T.E.; Khan, S.; Khan, W. Rainwater harvesting solar pasteurization treatment systems for the provision of an alternative water source in peri-urban informal settlements. Environ. Sci. Water Res. Technol. 2018, 4, 291–302. [Google Scholar] [CrossRef]
- Canessa, E.; Fonda, C.; Zennaro, M. Low–Cost 3D Printing for Science, Education and Sustainable Development; ICTP—The Abdus Salam International Centre for Theoretical Physics: Trieste, Italy, 2013; ISBN 92-95003-48-9. [Google Scholar]
- Birtchnell, T.; Hoyle, W. 3D Printing for Development in the Global South: The 3D4D Challenge; Springer: Berlin, Germany, 2014; ISBN 978-1-137-36566-8. [Google Scholar]
- James, E.; Gilman, D. Shrinking the Supply Chain: Hyperlocal Manufacturing and 3D Printing in Humanitarian Response. In OCHA Policy and Studies Series; OCHA: New York, NY, USA, 2015; Volume 14. [Google Scholar]
- De la Torre, N.; Espinosa, M.M.; Domínguez, M. Rapid Prototyping in Humanitarian Aid to Manufacture Last Mile Vehicles Spare Parts: An Implementation Plan. Hum. Factors Ergon. Manuf. Serv. Ind. 2016, 26, 533–540. [Google Scholar] [CrossRef]
- James, E.; James, L. 3D Printing Humanitarian Supplies in the Field. Humanit. Exch. 2016, 66, 43–45. [Google Scholar]
- Loy, J.; Tatham, P.; Healey, R.; Tapper, C.L. 3D Printing Meets Humanitarian Design Research: Creative Technologies in Remote Regions. Creat. Technol. Multidiscip. Appl. 2016, 54–75. [Google Scholar] [CrossRef]
- McBride, A. 3D Printing Takes Emergency Response to another Level. Policy and Practice Blog, 2014. Available online: https://policy-practice.oxfam.org.uk/blog/2014/05/3d-printing-takes-emergency-response-to-another-level (accessed on 4 February 2018).
- Ott, D. Can Digital Fabrication Revolutionise Humanitarian Action? ICRC Blog, 2016. Available online: http://blogs.icrc.org/gphi2/2016/02/22/can-digital-fabrication-revolutionize-humanitarian-action/ (accessed on 4 February 2018).
- Scott, C. ICRC Enable Makeathon Endeavors to Help People with Disabilities in Remote Areas through 3D Printing and Other Technologies. 3D Print, 2015. Available online: https://3dprint.com/tag/icrc/ (accessed on 4 February 2018).
- Saripalle, S.; Maker, H.; Bush, A.; Lundman, N. 3D Printing for Disaster Preparedness: Making Life-Saving Supplies on-Site, on-Demand, on-Time. In Proceedings of the IEEE Global Humanitarian Technology Conference, Seattle, WA, USA, 13–16 October 2016; pp. 205–208. [Google Scholar]
- Savonen, B.L.; Mahan, T.J.; Curtis, M.W.; Schreier, J.W.; Gershenson, J.K.; Pearce, J.M. Development of a Resilient 3-D Printer for Humanitarian Crisis Response. Technologies 2018, 6, 30. [Google Scholar] [CrossRef]
- Thryft, A.R. This Startup Wants Industrial 3D Printing to be Affordable in the Developing World. Available online: https://www.designnews.com/content/startup-wants-industrial-3D-printing-be-affordable-developing-world/119436841439281 (accessed on 13 March 2018).
- ESD Ultem 3D Printing Filament, 3DXTech. 2018. Available online: https://www.3dxtech.com/esd-ultem-3d-printing-filament/ (accessed on 23 March 2018).
- Tyson, E. Want to Use ABS in Hot Sun? We Compare ABS vs. ASA Filaments, Rigid Ink. 2016. Available online: https://rigid.ink/blogs/news/175845063-the-difference-between-abs-and-asa (accessed on 23 March 2018).
- Doalman, J.D.B. The mystery of electronic water treatment unveiled. Ind. Technol. News 2005, 25. [Google Scholar]
- Shah, R.K.; Subbarao, E.C.; Mashelkar, R.A. Heat Transfer Equipment Design; CRC Press: Boca Raton, FL, USA, 1988; ISBN 978-0-89116-729-7. [Google Scholar]
- Goddard, J.B. Abrasion Resistance of Piping Systems; ADS Technical Note 2.116; Advanced Drainage Syst.: Hilliard, OH, USA, 1994. [Google Scholar]
- Bongaarts, J. Can family planning programs reduce high desired family size in sub-Saharan Africa? Int. Perspect. Sex. Reprod. Health 2011, 37, 209–216. [Google Scholar] [CrossRef] [PubMed]
- National Research Council. Water and Sustainable Development: Opportunities for the Chemical Sciences: A Workshop Report to the Chemical Sciences Roundtable; National Academies Press: Washington, DC, USA, 2004. [Google Scholar]
- Pearce, J.M. Applications of open source 3-D printing on small farms. Org. Farming 2015, 1, 19–35. [Google Scholar] [CrossRef]
- Baechler, C.; DeVuono, M.; Pearce, J.M. Distributed recycling of waste polymer into RepRap feedstock. Rapid Prototyp. J. 2013, 19, 118–125. [Google Scholar] [CrossRef]
- Cruz Sanchez, F.A.; Lanza, S.; Boudaoud, H.; Hoppe, S.; Camargo, M. Polymer Recycling and Additive Manufacturing in an Open Source context: Optimization of processes and methods. In Proceedings of the Annual International Solid Freeform Fabrication Symposium (ISSF), Austin, TX, USA, 10–12 August 2015; pp. 1591–1600. [Google Scholar]
- Cruz Sanchez, F.A.; Boudaoud, H.; Hoppe, S.; Camargo, M. Polymer recycling in an open-source additive manufacturing context: Mechanical issues. Addit. Manuf. 2017, 17, 87–105. [Google Scholar] [CrossRef]
- Anderson, I. Mechanical Properties of Specimens 3D Printed with Virgin and Recycled Polylactic Acid. 3D Print. Addit. Manuf. 2017, 4, 110–115. [Google Scholar] [CrossRef]
- Pakkanen, J.; Manfredi, D.; Minetola, P.; Iuliano, L. About the Use of Recycled or Biodegradable Filaments for Sustainability of 3D Printing. In Sustainable Design and Manufacturing; Smart Innovation, Systems and Technologies; Springer: Cham, Switzerland, 2017; pp. 776–785. [Google Scholar]
- Chong, S.; Pan, G.-T.; Khalid, M.; Yang, T.C.-K.; Hung, S.-T.; Huang, C.-M. Physical Characterization and Pre-assessment of Recycled High-Density Polyethylene as 3D Printing Material. J. Polym. Environ. 2017, 25, 136–145. [Google Scholar] [CrossRef]
- Mohammed, M.I.; Mohan, M.; Das, A.; Johnson, M.D.; Badwal, P.S.; McLean, D.; Gibson, I. A low carbon footprint approach to the reconstitution of plastics into 3D-printer filament for enhanced waste reduction. KnE Eng. 2017, 2, 234–241. [Google Scholar] [CrossRef]
- Mohammed, M.I.; Das, A.; Gomez-Kervin, E.; Wilson, D.; Gibson, I. EcoPrinting: Investigating the use of 100% recycled Acrylonitrile Butadiene Styrene (ABS) for Additive Manufacturing. Solid Freeform Fabrication 2017. In Proceedings of the 28th Annual International Solid Freeform Fabrication Symposium, Austin, TX, USA, 13–15 August 2018; Available online: http://sffsymposium.engr.utexas.edu/sites/default/files/2017/Manuscripts/EcoprintingInvestigatingtheUseof100Recycle.pdf (accessed on 3 March 2018).
- Zhong, S.; Pearce, J.M. Tightening the loop on the circular economy: Coupled distributed recycling and manufacturing with recyclebot and RepRap 3-D printing. Resour. Conserv. Recycl. 2018, 128, 48–58. [Google Scholar] [CrossRef]
- Pringle, A.M.; Rudnicki, M.; Pearce, J. Wood Furniture Waste-Based Recycled 3-D Printing Filament. For. Prod. J. 2018. [Google Scholar] [CrossRef]
- Tian, X.; Liu, T.; Wang, Q.; Dilmurat, A.; Li, D.; Ziegmann, G. Recycling and remanufacturing of 3D printed continuous carbon fiber reinforced PLA composites. J. Clean. Prod. 2017, 142, 1609–1618. [Google Scholar] [CrossRef]
- Appropedia. 2018. Available online: http://www.appropedia.org/ (accessed on 23 March 2018).
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Denkenberger, D.C.; Pearce, J.M. Design Optimization of Polymer Heat Exchanger for Automated Household-Scale Solar Water Pasteurizer. Designs 2018, 2, 11. https://doi.org/10.3390/designs2020011
Denkenberger DC, Pearce JM. Design Optimization of Polymer Heat Exchanger for Automated Household-Scale Solar Water Pasteurizer. Designs. 2018; 2(2):11. https://doi.org/10.3390/designs2020011
Chicago/Turabian StyleDenkenberger, David C., and Joshua M. Pearce. 2018. "Design Optimization of Polymer Heat Exchanger for Automated Household-Scale Solar Water Pasteurizer" Designs 2, no. 2: 11. https://doi.org/10.3390/designs2020011
APA StyleDenkenberger, D. C., & Pearce, J. M. (2018). Design Optimization of Polymer Heat Exchanger for Automated Household-Scale Solar Water Pasteurizer. Designs, 2(2), 11. https://doi.org/10.3390/designs2020011







