A Tutorial on Robust Control, Adaptive Control and Robust Adaptive Control—Application to Robotic Manipulators
Abstract
:1. Introduction
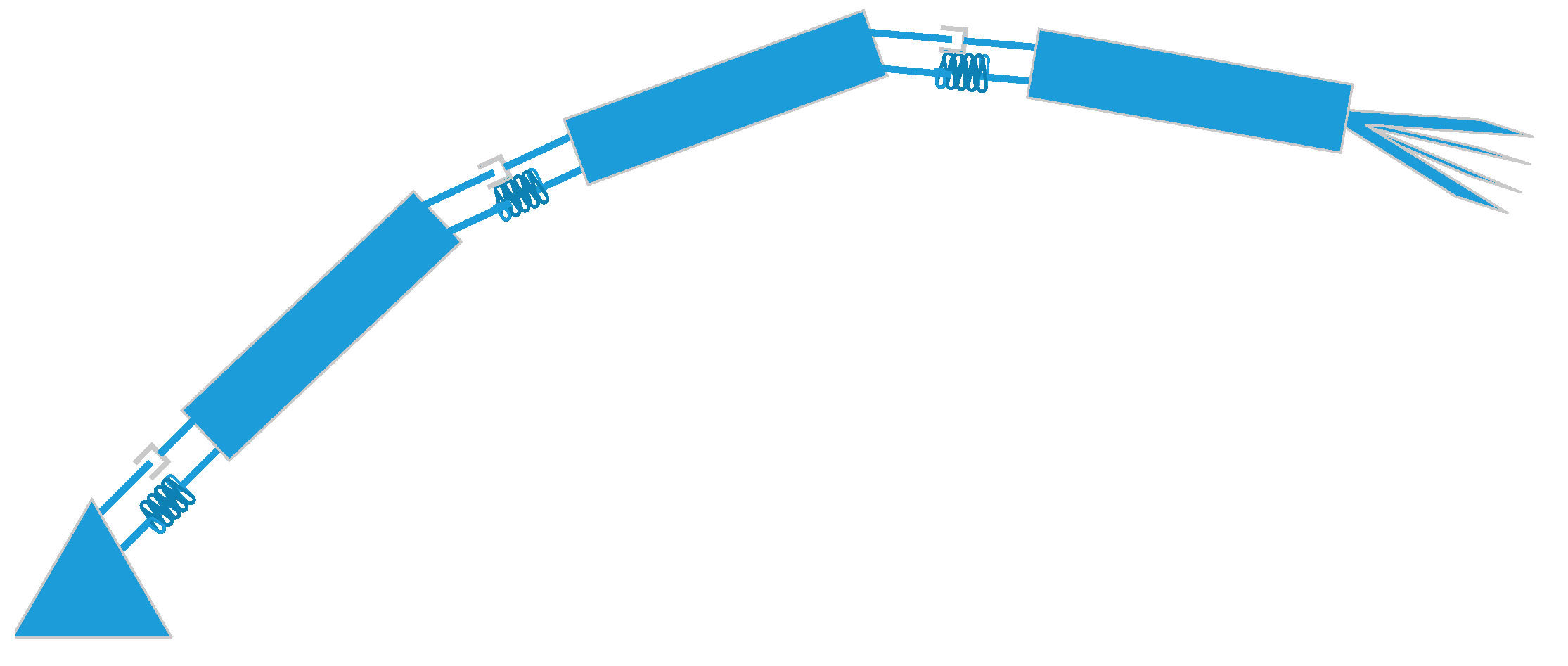
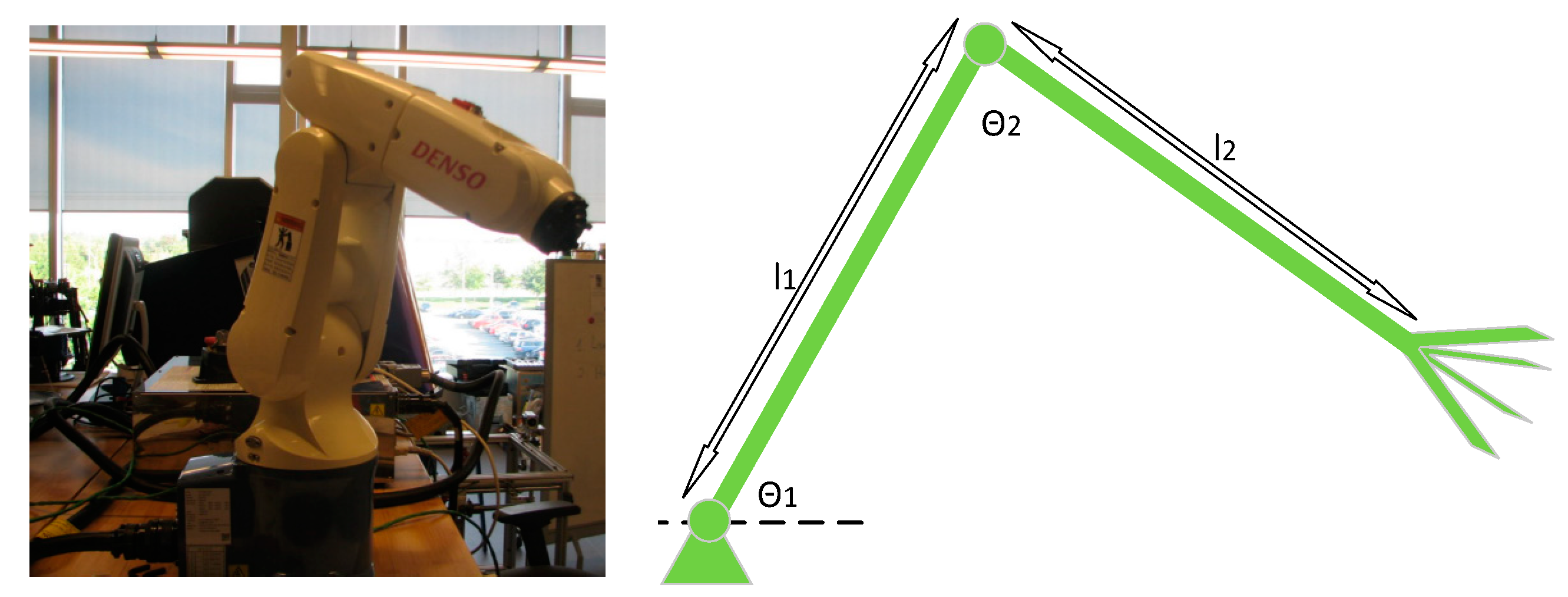
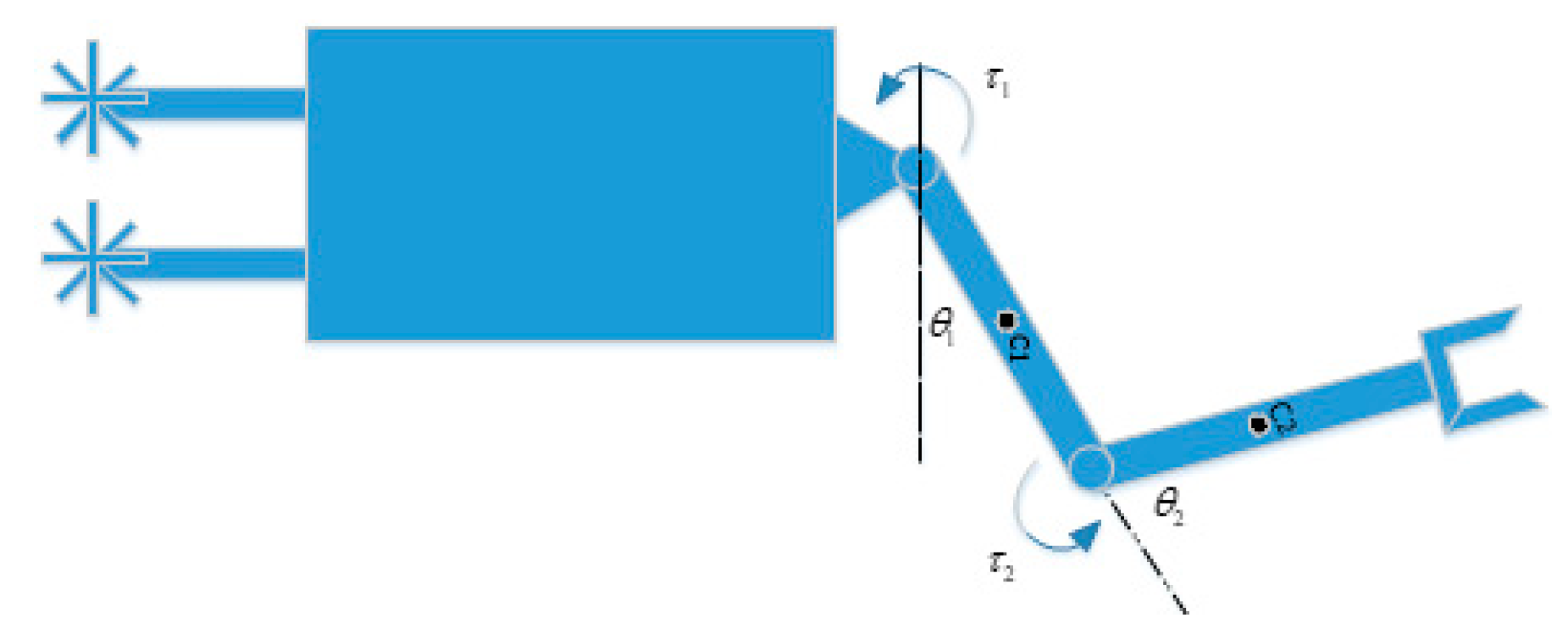
2. Joint Space Control and Operational Space Control
3. Robust Control
4. Adaptive Control
5. Robust Adaptive Control
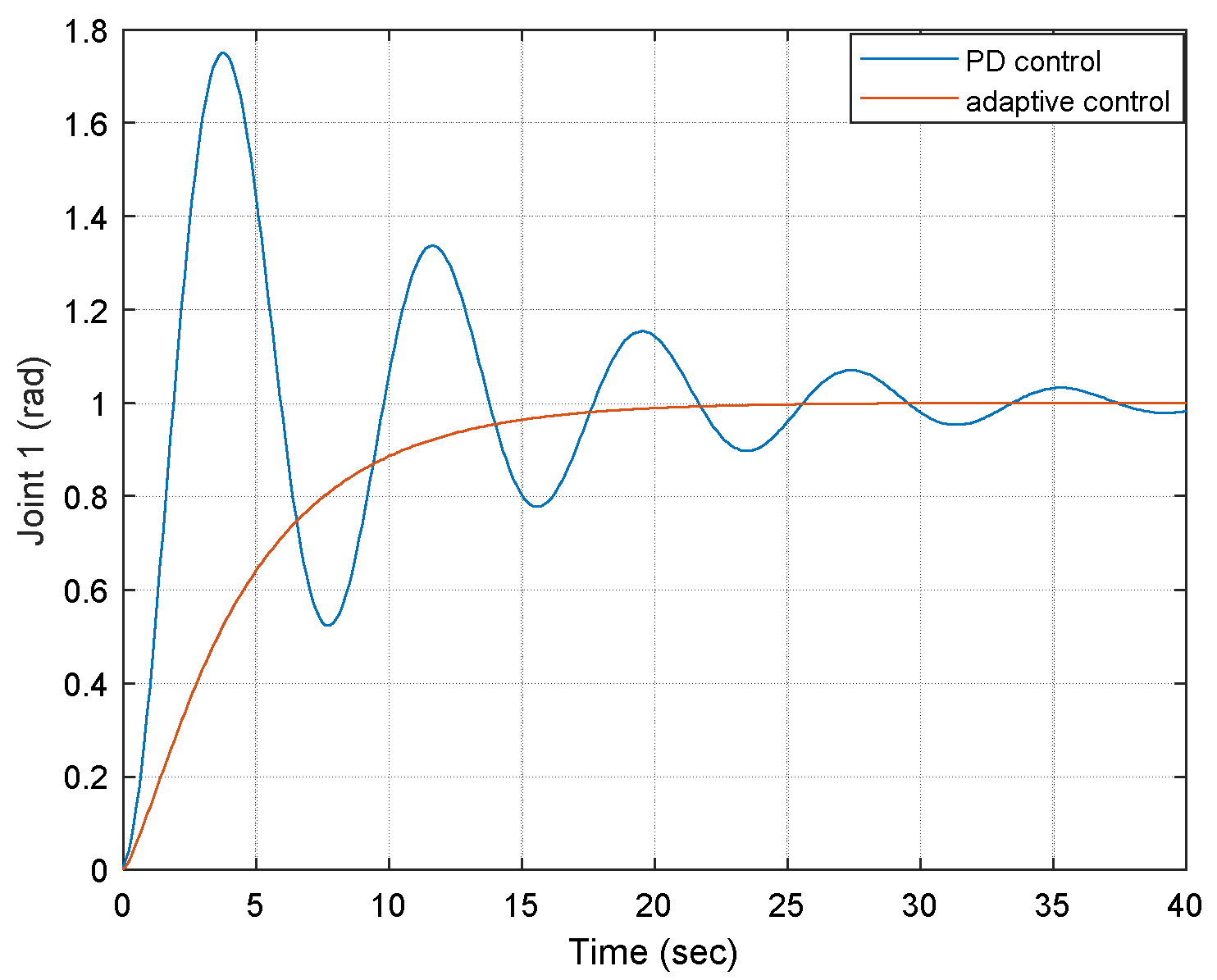
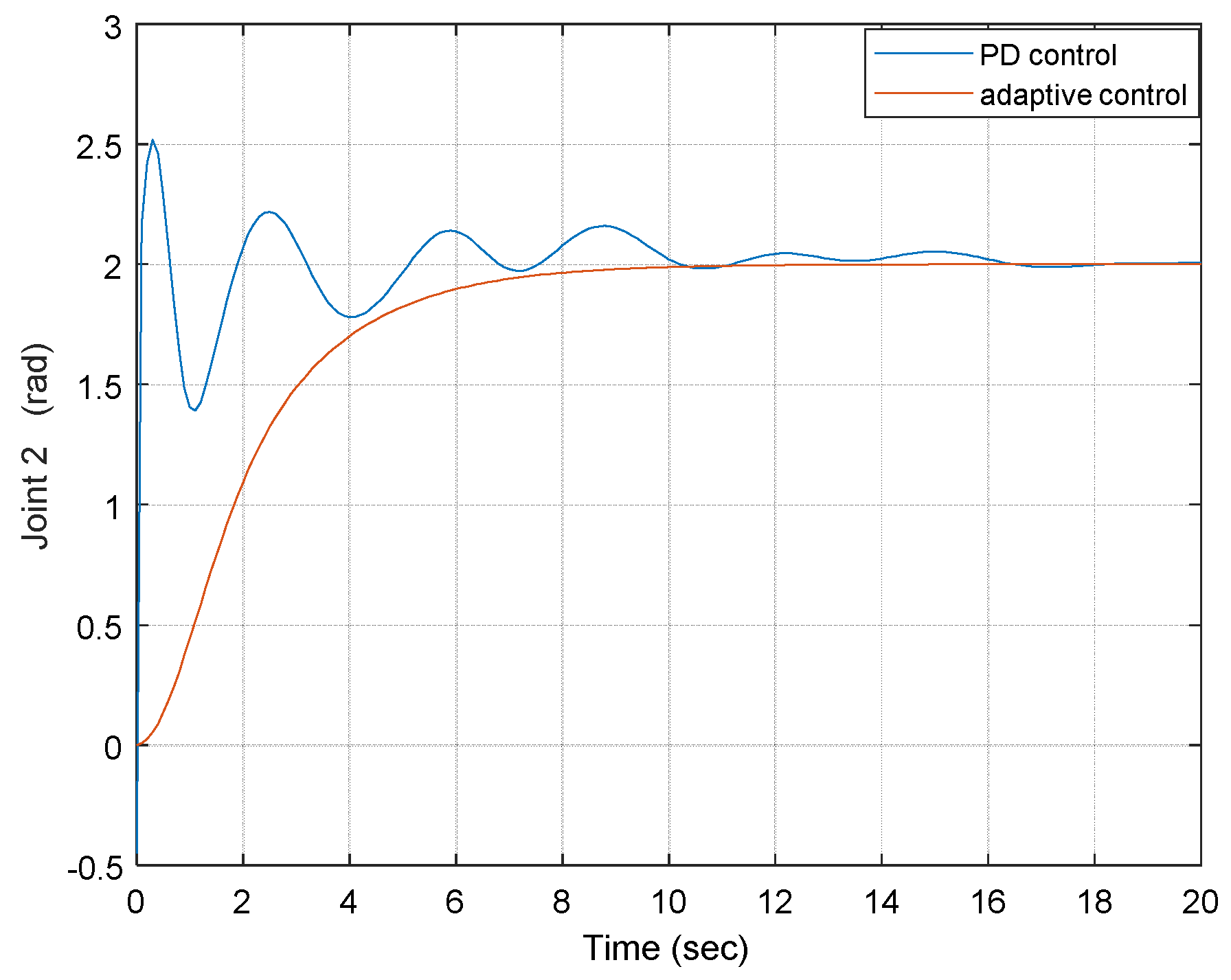
6. Adaptive Control
7. Conclusions
Funding
Conflicts of Interest
Abbreviations
| Term | Meaning |
| unknow constant (see Equation (15)) | |
| estimate of | |
| parameter estimation error | |
| unknown friction term | |
| estimate of |
References
- Schreiber, L.; Gosselin, C.; Laliberte, T. Kinematics and dynamic analysis of a skating robot. Trans. Can. Soc. Mech. Eng. 2014, 38, 185–197. [Google Scholar] [CrossRef]
- St-Onge, D.; Gosselin, C. Synthesis and Design of a One Degree-of-Freedom Planar Deployable Mechanism with a Large Expansion Ratio. J. Mech. Robot. 2016, 8, 021025. [Google Scholar] [CrossRef]
- Zhang, D.; Wei, B. Design and optimization of a Tripod-based hybrid manipulator. Romansy- 2014, CISM-IFToMM Symposium on Theory and Practice of Robots and Manipulators. Mech. Mach. Sci. 2014, 22, 83–91. [Google Scholar]
- Cavusoglu, M.; Villanueva, I.; Tendick, F. Workspace analysis of robotic manipulators for a teleoperated suturing task. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Maui, HI, USA, 29 October–3 November 2001; pp. 2234–2239. [Google Scholar]
- Bouzgou, K.; Ahmed-Foitih, Z. Workspace Analysis and Geometric Modeling of 6 DOF Fanuc 200IC Robot. Procedia Soc. Behav. Sci. 2015, 182, 703–709. [Google Scholar] [CrossRef] [Green Version]
- Isaksson, M.; Gosselin, C.; Marlow, K. Singularity analysis of a class of kinematically redundant parallel Schönflies motion generators. Mech. Mach. Theory 2017, 112, 172–191. [Google Scholar] [CrossRef]
- Jiang, Q.; Gosselin, C.M.; Wang, Y.; Fang, C. Maximal singularity-free orientation workspace over a position region of Gough–Stewart platform. Adv. Robot. 2015, 29, 1–10. [Google Scholar] [CrossRef]
- Klimchik, A.; Caro, S.; Wu, Y.; Chablat, D.; Furet, B.; Pashkevich, A. Stiffness modeling of robotic manipulator with gravity compensator. In Proceedings of the 6th International Workshop on Computational Kinematics (CK2013), Barcelona, Spain, 12–15 May 2013; pp. 161–168. [Google Scholar]
- Zhang, D.; Wei, B. Design, kinematic and dynamic modeling of a novel tripod-based manipulator. Robotica 2016, 34, 2186–2204. [Google Scholar] [CrossRef]
- Flacco, F.; De Luca, A.; Khatib, O. Control of Redundant Robots under Hard Joint Constraints: Saturation in the Null Space. IEEE Trans. Robot. 2015, 31, 637–654. [Google Scholar] [CrossRef]
- Menon, S.; Fok, S.; Neckar, A.; Khatib, O.; Boahen, K. Controlling articulated robots in task-space with spiking silicon neurons. In Proceedings of the 5th IEEE RAS/EMBS International Conference on Biomedical Robotics and Biomechatronics, Sao Paulo, Brazil, 12–15 August 2014; pp. 181–186. [Google Scholar]
- Conti, F.; Park, J.; Khatib, O. Interface Design and Control Strategies for a Robot Assisted Ultrasonic Examination System. In Experimental Robotics; Springer: Berlin/Heidelberg, Germany, 2014; pp. 97–113. [Google Scholar]
- Khatib, O. A unified approach for motion and force control of robot manipulators: The operational space formulation. IEEE J. Robot. Autom. 1987, 3, 43–53. [Google Scholar] [CrossRef]
- Sentis, L.; Park, J.; Khatib, O. Compliant control of multi-contact and center of mass behaviors in humanoid robots. IEEE Trans. Robot. 2010, 26, 483–501. [Google Scholar] [CrossRef]
- Slotine, J.E.; Li, W. Applied Nonlinear Control; Prentice-Hall: Upper Saddle River, NJ, USA, 1991. [Google Scholar]
- Santibáñez, V.; Kelly, R. PD control with feedforward compensation for robot manipulators: Analysis and experimentation. Robotica 2001, 19, 11–19. [Google Scholar] [CrossRef]
- Zhang, D.; Wei, B. Adaptive Control for Robotic Manipulators; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Li, W.; Slotine, J.-J.E. On the Adaptive Control of Robot Manipulators. Int. J. Robot. Res. 1987, 6, 49–59. [Google Scholar]
- Tomizuka, M.; Horowitz, R. An Adaptive Control Scheme for Mechanical Manipulators—Compensation of Nonlinearity and Decoupling Control. J. Dyn. Syst. Meas. Control 1986, 108, 127–135. [Google Scholar]
- Tong, S.; Li, Y.; Sui, S. Adaptive Fuzzy Tracking Control Design for SISO Uncertain Nonstrict Feedback Nonlinear Systems. IEEE Trans. Fuzzy Syst. 2016, 24, 1441–1454. [Google Scholar] [CrossRef]
- Li, Y.; Tong, S.; Liu, L.; Feng, G. Adaptive output-feedback control design with prescribed performance for switched nonlinear systems. Automatica 2017, 80, 225–231. [Google Scholar] [CrossRef]
- Craig, J.J. Adaptive Control of Mechanical Manipulators; Addison-Wesley: Boston, MA, USA, 1988. [Google Scholar]
- Wang, C.; Zhao, Y.; Chen, Y.; Tomizuka, M. Nonparametric statistical learning control of robot manipulators for trajectory or contour tracking. Robot. Comput. Manuf. 2015, 35, 96–103. [Google Scholar] [CrossRef]
- Armin, S.; Goele, P.; Jan, S. Optimization-based iterative learning control for robotic manipulators. In Proceedings of the 2016 Benelux Meeting on Systems and Control, Soesterberg, The Netherlands, 22–24 March 2016. [Google Scholar]
- Verrelli, C.M.; Pirozzi, S.; Tomei, P.; Natale, C. Linear Repetitive Learning Controls for Robotic Manipulators by Padé Approximants. IEEE Trans. Control Syst. Technol. 2015, 23, 2063–2070. [Google Scholar] [CrossRef]
- Ernesto, H.; Pedro, J.O. Iterative Learning Control with Desired Gravity Compensation under Saturation for a Robotic Machining Manipulator. Math. Probl. Eng. 2015, 2015, 187948. [Google Scholar] [CrossRef]
- Delchev, K.; Boiadjiev, G.; Kawasaki, H.; Mouri, T. Iterative learning control with sampled-data feedback for robot manipulators. Arch. Control Sci. 2014, 24, 299–319. [Google Scholar] [CrossRef] [Green Version]
- Delchev, K. Iterative learning control for robotic manipulators: A bounded-error algorithm. Int. J. Adapt. Control Signal Process. 2014, 28, 1454–1473. [Google Scholar] [CrossRef]
- Munadi, M.; Naniwa, T. Experimental verification of adaptive dominant type hybrid adaptive and learning controller for trajectory tracking of robot manipulators. J. Robot. Mechatron. 2013, 25, 737–747. [Google Scholar] [CrossRef]
- Queen, M.P.; Kuma, M.; Aurtherson, P. Repetitive Learning Controller for Six Degree of Freedom Robot Manipulator. Int. Rev. Autom. Control 2013, 6, 286–293. [Google Scholar]
- Marino, R.; Tomei, P.; Verrelli, C.M. Robust adaptive learning control for nonlinear systems with extended matching unstructured uncertainties. Int. J. Robust Nonlinear Control 2012, 22, 645–675. [Google Scholar] [CrossRef]
- Bouakrif, F.; Boukhetala, D.; Boudjema, F. Velocity observer-based iterative learning control for robot manipulators. Int. J. Syst. Sci. 2013, 44, 214–222. [Google Scholar] [CrossRef]
- Zhang, D.; Wei, B. On the development of learning control for robotic manipulators. Robotics 2017, 6, 23. [Google Scholar] [CrossRef]
- Liu, J.; Wang, X. Sliding mode control for robot. In Advanced Sliding Mode Control for Mechanical Systems; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Bennassar, A.; Banerjee, S.; Jamma, M.; Essalmi, A.; Akherraz, M. Real Time High Performance of Sliding Mode Controlled Induction Motor Drives. Procedia Comput. Sci. 2018, 132, 971–982. [Google Scholar] [CrossRef]
- Faris, F.; Moussaoui, A. Design and real-time implementation of a decentralized sliding mode controller for twin rotor multi-input multi-output system. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2016, 231, 3–13. [Google Scholar] [CrossRef]
- Castanos, F.; Fridman, L. Analysis and Design of Integral Sliding Manifolds for Systems with Unmatched Perturbations. IEEE Trans. Autom. Control 2006, 51, 853–858. [Google Scholar] [CrossRef]
- Shi, J.; Liu, H.; Bajcinca, N. Robust control of robotic manipulators based on integral sliding mode. Int. J. Control 2008, 81, 1537–1548. [Google Scholar] [CrossRef]
- Piltan, F.; Sulaiman, N. Review of sliding mode control of robotic manipulator. World Appl. Sci. J. 2012, 18, 1855–1869. [Google Scholar]
- Jin, S.; Bak, J.; Kim, J.; Seo, T.; Kim, H.S. Switching PD-based sliding mode control for hovering of a tilting-thruster underwater robot. PLoS ONE 2018, 13, e0194427. [Google Scholar] [CrossRef] [PubMed]
- Bendaas, I.; Naceri, F. A new method to minimize the chattering phenomenon in sliding mode control based on intelligent control for induction motor drives. Serb. J. Electr. Eng. 2013, 10, 231–246. [Google Scholar] [CrossRef]
- Barambones, O.; Garrido, A. Adaptive sensorless robust control of AC drives based on sliding mode control theory. Int. J. Robust Nonlinear Control 2006, 72, 862–879. [Google Scholar] [CrossRef]


© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, B. A Tutorial on Robust Control, Adaptive Control and Robust Adaptive Control—Application to Robotic Manipulators. Inventions 2019, 4, 49. https://doi.org/10.3390/inventions4030049
Wei B. A Tutorial on Robust Control, Adaptive Control and Robust Adaptive Control—Application to Robotic Manipulators. Inventions. 2019; 4(3):49. https://doi.org/10.3390/inventions4030049
Chicago/Turabian StyleWei, Bin. 2019. "A Tutorial on Robust Control, Adaptive Control and Robust Adaptive Control—Application to Robotic Manipulators" Inventions 4, no. 3: 49. https://doi.org/10.3390/inventions4030049
APA StyleWei, B. (2019). A Tutorial on Robust Control, Adaptive Control and Robust Adaptive Control—Application to Robotic Manipulators. Inventions, 4(3), 49. https://doi.org/10.3390/inventions4030049



