Dynamic Correlations in Disordered Systems: Implications for High-Temperature Superconductivity
Abstract
1. Introduction
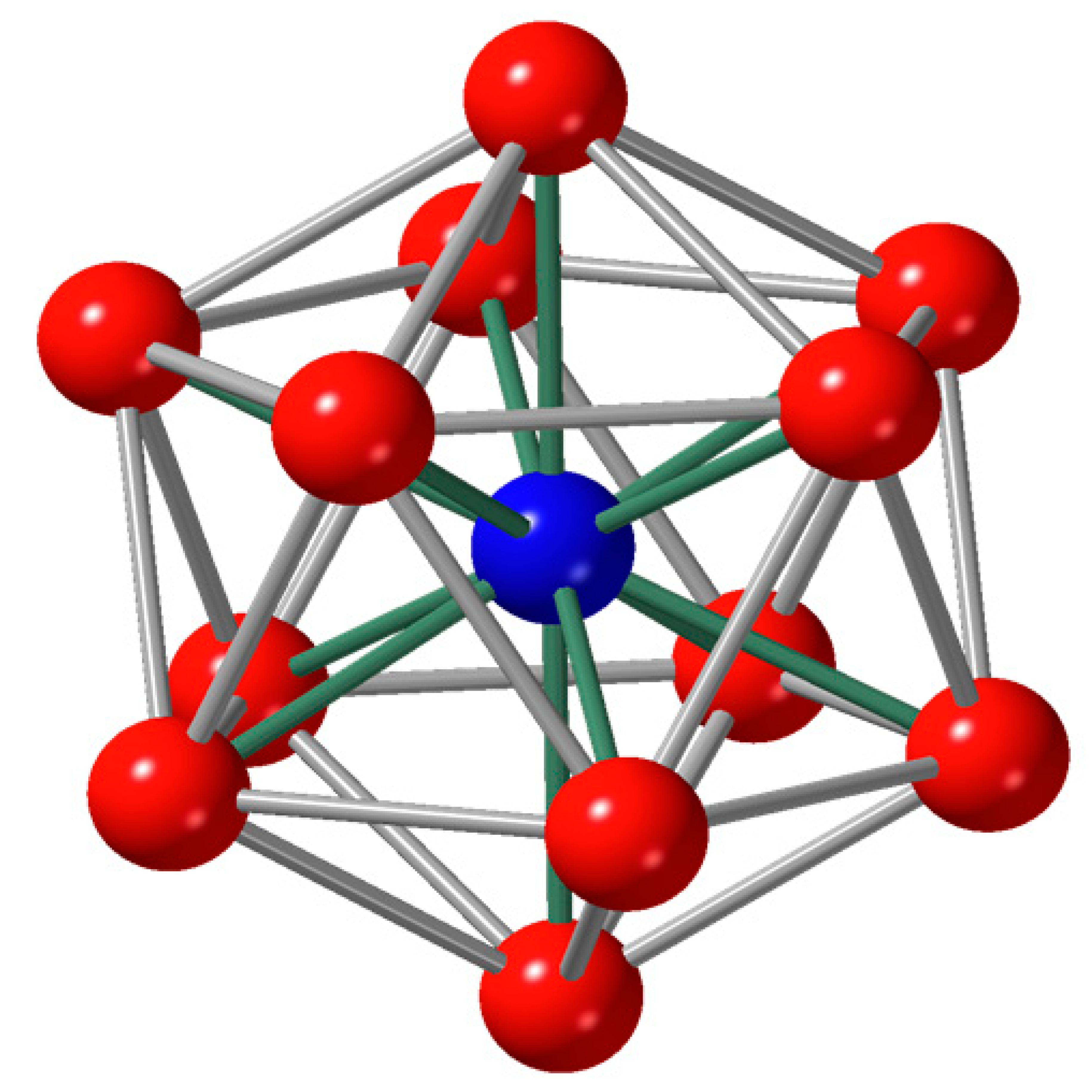
2. Local Structure of Liquid and Glass
3. Density Wave Theory of Medium-Range Order
4. Dynamic Correlation in Superfluid 4He
5. Dynamic Correlation among Electrons
6. Implication on High-Temperature Superconductivity
7. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Landau, L.D.; Lifshitz, E.M. Statistical Physics; Sykes, J.B.; Kearsley, M.J., Translators; Addison-Wesley: Boston, MA, USA, 1958. [Google Scholar]
- Egelstaff, P.A. An Introduction to the Liquid State, 2nd ed.; Oxford University Press: Oxford, UK, 1991. [Google Scholar]
- Croxton, C.A. Liquid State Physics—A Statistical Mechanical Introduction; Cambridge University Press: Cambridge, MA, USA, 1974. [Google Scholar]
- Hansen, J.-P.; McDonald, I.R. Theory of Simple Liquids, 2nd ed.; Academic Press: Cambridge, MA, USA, 2006. [Google Scholar]
- Wallace, D.C. Statistical mechanics of monoatomic liquids. Phys. Rev. E 1997, 56, 4179–4186. [Google Scholar] [CrossRef]
- Wallace, D.C. Liquid dynamics theory of high-temperature specific heat. Phys. Rev. E 1998, 57, 1717–1722. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics; John Wiley & Sons: Hoboken, NJ, USA, 1953. [Google Scholar]
- Egami, T. Understanding the Properties and Structure of Metallic Glasses at the Atomic Level. J. Metals 2010, 62, 70–75. [Google Scholar] [CrossRef]
- Moon, J.; Lindsay, L.; Egami, T. Atomic dynamics in fluids: Normal mode analysis revisited. Phys. Rev. E 2023, 108, 014601. [Google Scholar] [CrossRef] [PubMed]
- Ornstein, L.S.; Zernike, F. Accidental deviations of density and opalescence at the critical point of a single substance. Roy. Neth. Acad. Arts Sci. (KNAW) 1914, 17, 793–806. [Google Scholar]
- Perkus, J.K.; Yevick, G.J. Analysis of classical statistical mechanics by means of collective coordinates. Phys. Rev. 1958, 110, 1–13. [Google Scholar] [CrossRef]
- Bernal, J.D. A geometrical approach to the structure of liquids. Nature 1959, 183, 141–147. [Google Scholar] [CrossRef]
- Bernal, J.D. Geometry of the structure of monatomic liquids. Nature 1960, 185, 68–70. [Google Scholar] [CrossRef]
- Finny, J. Random packing and the structure of simple liquids. I. The geometry of random close packing. Proc. Roy. Soc. Lond. A 1970, 319, 479–493. [Google Scholar]
- Cohen, M.H.; Turnbull, D. Molecular transport in liquids and glasses. J. Chem. Phys. 1959, 31, 1164–1169. [Google Scholar] [CrossRef]
- Turnbull, D.; Cohen, M.H. Free-volume model of the amorphous phase: Glass transition. J. Chem. Phys. 1961, 34, 120–125. [Google Scholar] [CrossRef]
- Turnbull, D.; Cohen, M.H. On the free-volume model of the liquid-glass transition. J. Chem. Phys. 1970, 52, 3038–3041. [Google Scholar] [CrossRef]
- Mori, H. Transport, collective motion and Brownian motion. Prog. Theor. Phys. 1965, 33, 423. [Google Scholar] [CrossRef]
- Kadanoff, L.; Swift, J. Transport coefficients near liquid-gas critical point. Phys. Rev. 1968, 166, 89–101. [Google Scholar] [CrossRef]
- Kawasaki, K. Kinetic equations and time correlation functions of critical fluctuations. Ann. Phys. N. Y. 1970, 61, 1–56. [Google Scholar] [CrossRef]
- Goldstein, M. Viscous liquids and the glass transition: A potential energy barrier picture. J. Chem. Phys. 1969, 51, 3728–3739. [Google Scholar] [CrossRef]
- Stillinger, F.H. A topological view of supercooled liquids and glass formation. Science 1995, 267, 1935–1939. [Google Scholar] [CrossRef]
- Wales, D.J. Energy Landscapes; Cambridge University Press: Cambridge, MA, USA, 2003. [Google Scholar]
- Dyre, J.C.; Olsen, N.B.; Christensen, T. Local elastic expansion model for viscous-flow activation energies of glass-forming molecular liquids. Phys. Rev. B 1996, 53, 2171–2174. [Google Scholar] [CrossRef]
- Leutheusser, E. Dynamical model of the liquid-glass transition. Phys. Rev. A 1984, 29, 2765–2773. [Google Scholar] [CrossRef]
- Götze, W.; Sjogren, L. Relaxation processes in supercooled liquids. Rep. Prog. Phys. 1992, 55, 241–376. [Google Scholar]
- Das, S.P. Mode-coupling theory and the glass transition in supercooled liquids. Rev. Mod. Phys. 2004, 76, 785–851. [Google Scholar] [CrossRef]
- Angell, C.A. Formation of glasses from liquids and biopolymers. Science 1995, 267, 1924–1935. [Google Scholar] [CrossRef] [PubMed]
- Debenedetti, P.G.; Stillinger, F.H. Supercooled liquids and the glass transition. Nature 2001, 410, 259–267. [Google Scholar] [CrossRef]
- March, N.H.; Tosi, M.P. Introduction to Liquid State Physics; World Scientific: Singapore, 2002. [Google Scholar]
- Faupel, F.; Macht, M.P.; Mehrer, H.; Naundorf, V.; Rätzke, K.; Schober, H.R.; Sharma, S.K.; Teichler, H. Diffusion in metallic glasses and supercooled melts. Rev. Mod. Phys. 2003, 75, 237–280. [Google Scholar] [CrossRef]
- Scopigno, T.; Ruocco, G.; Sette, F. Microscopic dynamics in liquid metals: The experimental point of view. Rev. Mod. Phys. 2005, 77, 881–933. [Google Scholar] [CrossRef]
- Dyre, J.C. The glass transition and elastic models of glass-forming liquids. Rev. Mod. Phys. 2006, 78, 953–972. [Google Scholar] [CrossRef]
- Lubchenko, V.; Wolynes, P.G. Theory of structural glasses and supercooled liquids. Ann. Rev. Phys. Chem. 2007, 58, 235–266. [Google Scholar] [CrossRef]
- Götze, W. Complex Dynamics of Glass-Forming Liquids; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Donth, E. The Glass Transition: Relaxation Dynamics of Liquids and Disordered Materials; Springer: Berlin, Germany, 2010. [Google Scholar]
- Liu, A.J.; Nagel, S.R. The jamming transition and the marginally jammed solid. Ann. Rev. Cond. Mat. 2010, 1, 347–369. [Google Scholar] [CrossRef]
- Parisi, G.; Zamponi, F. Mean-field theory of hard sphere glasses and jamming. Rev. Mod. Phys. 2010, 82, 789–845. [Google Scholar] [CrossRef]
- Berthier, L.; Biroli, G. Theoretical perspective on the glass transition and amorphous materials. Rev. Mod. Phys. 2011, 83, 587–645. [Google Scholar] [CrossRef]
- Edigar, M.D.; Harrowell, P. Perspective: Supercooled liquids and glasses. J. Chem. Phys. 2012, 137, 080901. [Google Scholar] [CrossRef]
- Dyre, J.C. Perspective: Excess-entropy scaling. J. Chem. Phys. 2018, 149, 210901. [Google Scholar] [CrossRef] [PubMed]
- Parisi, G.; Urbani, P.; Zamponi, F. Theory of Simple Glasses: Exact Solutions in Infinite Dimensions; Cambridge University Press: Cambridge, MA, USA, 2020. [Google Scholar]
- Weeks, J.D.; Chandler, D.; Andersen, H.C. Role of repulsive forces in determining the equilibrium structure of simple liquids. J. Chem. Phys. 1971, 54, 5237–5247. [Google Scholar] [CrossRef]
- Egami, T. Atomic level stresses. Progr. Mater. Sci. 2011, 56, 637–653. [Google Scholar] [CrossRef]
- Egami, T.; Ryu, C.W. Structural principles in metallic liquids and glasses: Bottom-up or top-down. Front. Mater. 2022, 9, 874191. [Google Scholar] [CrossRef]
- Egami, T.; Ryu, C.W. Medium-range atomic correlation in simple liquid. II. Theory of temperature dependence. Phys. Rev. E 2021, 104, 064110. [Google Scholar] [CrossRef] [PubMed]
- Egami, T.; Poon, S.J.; Zhang, Z.; Keppens, V. Glass transition in metallic glasses: A microscopic model of topological fluctuations in the bonding network. Phys. Rev. B 2007, 76, 024203. [Google Scholar] [CrossRef]
- Dmowski, W.; Diallo, S.O.; Lokshin, K.; Ehlers, G.; Ferré, G.; Boronat, J.; Egami, T. Observation of dynamic atom-atom correlation in liquid helium in real space. Nat. Commun. 2017, 8, 15294. [Google Scholar] [CrossRef]
- Egami, T.K. Alex Müller and superconductivity. Physica C 2023, 613, 1354345. [Google Scholar] [CrossRef]
- Frank, F.C. Supercooling of liquids. Proc. Roy. Soc. Lond. A 1952, 215, 43–46. [Google Scholar]
- Turnbull, D. Kinetics of solidification of supercooled liquid mercury droplets. J. Chem. Phys. 1952, 20, 411–424. [Google Scholar] [CrossRef]
- Sadoc, J.F. Use of regular polytopes for the mathematical description of the order in amorphous structures. J. Non-Cryst. Solids 1981, 44, 17–30. [Google Scholar] [CrossRef]
- Sethna, J.P. Frustration and curvature: Glasses and the cholesteric blue phase. Phys. Rev. Lett. 1983, 51, 2198–2201. [Google Scholar] [CrossRef]
- Nelson, D.R. Order, frustration, and defects in liquids and glasses. Phys. Rev. B 1983, 28, 5515–5535. [Google Scholar] [CrossRef]
- Steinhardt, P.J.; Nelson, D.R.; Ronchetti, M. Icosahedral bond order in supercooled liquids. Phys. Rev. Lett. 1981, 47, 1297–1300. [Google Scholar] [CrossRef]
- Tomida, T.; Egami, T. Molecular-dynamics study of orientational order in liquids and glasses and its relation to the glass transition. Phys. Rev. B 1995, 52, 3290–3308. [Google Scholar] [CrossRef] [PubMed]
- Miracle, D.B. A structural model for metallic glasses. Nat. Mater. 2004, 3, 697–702. [Google Scholar] [CrossRef]
- Sheng, H.W.; Luo, W.K.; Alamgir, F.M.; Bai, J.M.; Ma, E. Atomic packing and short-to-medium-range order in metallic glasses. Nature 2006, 439, 419–425. [Google Scholar] [CrossRef]
- Warren, B.E. X-ray Diffraction; Addison-Wesley, Reading: Boston, MA, USA, 1969. [Google Scholar]
- Egami, T. Local Density Correlations in Liquids. Front. Phys. 2020, 8, 50. [Google Scholar] [CrossRef]
- Zhou, Y.; Mei, B.; Schweizer, K.S. Integral equation theory of thermodynamics, pair structure, and growing static length scale in metastable hard sphere and Weeks-Chandler-Andersen fluids. Phys. Rev. E 2020, 101, 042121. [Google Scholar] [CrossRef]
- Kleban, P. Toward a microscopic basis for the de Gennes narrowing. J. Stat. Phys. 1974, 11, 317–322. [Google Scholar] [CrossRef]
- Cargill, G.S., III. Structure of metallic alloy glasses. Solid State Phys. 1975, 30, 227–320. [Google Scholar]
- Ryu, C.W.; Dmowski, W.; Egami, T. Ideality of liquid structure: A case study for metallic alloy liquids. Phys. Rev. E 2020, 101, 030601(R). [Google Scholar] [CrossRef] [PubMed]
- Ryu, C.W.; Dmowski, W.; Kelton, K.F.; Lee, G.W.; Park, E.S.; Morris, J.R.; Egami, T. Curie-Weiss behavior of liquid structure and ideal glass state. Sci. Rep. 2019, 9, 18579. [Google Scholar] [CrossRef]
- Egami, T.; Ryu, C.W. Origin of medium-range atomic correlation in simple liquid: Density wave theory. AIP Adv. 2023, 13, 085308. [Google Scholar] [CrossRef]
- Ryu, C.W.; Egami, T. Origin of liquid fragility. Phys. Rev. E 2020, 102, 042615. [Google Scholar] [CrossRef] [PubMed]
- Levashov, V.A.; Aga, R.S.; Morris, J.R.; Egami, T. Equipartition theorem and the dynamics of liquids. Phys. Rev. B 2008, 78, 064205. [Google Scholar] [CrossRef]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. Roy. Soc. Lond. A 1957, 241, 376–396. [Google Scholar]
- Glyde, H.R. Excitations in Liquid and Solid Helium; Clarendon Press: Oxford, UK, 1994. [Google Scholar]
- Svensson, E.C.; Sears, V.F.; Woods, A.D.B.; Martel, P. Neutron-diffraction study of the static structure factor and pair correlations in liquid 4He. Phys. Rev. B 1980, 21, 3538–3651. [Google Scholar] [CrossRef]
- Anderson, P.W. The resonating valence bond state in La2CuO4 and superconductivity. Science 1987, 235, 1196–1198. [Google Scholar] [CrossRef]
- Keffer, F. Spin waves, in Handbuch Der Physik, 18, pt. 2; Springer: Berlin, Germany, 1966. [Google Scholar]
- Fulde, P. Electron Correlations in Molecules and Solids; Springer: Berlin, Germany, 2012. [Google Scholar]
- Van Hove, L. Correlation in space and time and Born approximation scattering in systems of interacting particles. Phys. Rev. 1954, 95, 249–262. [Google Scholar] [CrossRef]
- Egami, T.; Shinohara, Y. Perspective: Correlated atomic dynamics in liquid seen in real space and time. J. Chem. Phys. 2020, 153, 180902. [Google Scholar] [CrossRef] [PubMed]
- Platzman, P.M.; Tzor, N. X-ray scattering from electron gas. Phys. Rev. 1965, 139, A410–A413. [Google Scholar] [CrossRef]
- Abbamonte, P.; Finkelstein, K.D.; Collins, M.D.; Gruner, S.M. Imaging density disturbances in water with a 41.3-attosecond time resolution. Phys. Rev. Lett. 2004, 92, 237401. [Google Scholar] [CrossRef] [PubMed]
- Lovesey, S.W. Theory of Neutron Scattering from Condensed Matter; Oxford University Press: Oxford, UK, 1984. [Google Scholar]
- Bista, R.; Upton, M.; Shinohara, Y.; Egami, T. Direct observation of electron correlation in space by inelastic x-ray scattering. 2024; unpublished. [Google Scholar]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Li, Q.; Huang, H.-Y.; Ren, T.; Weschke, E.; Ju, L.; Zou, C.; Zhang, S.; Qiu, Q.; Liu, J.; Ding, S.; et al. Prevailing charge order in overdoped La2-xSrxCuO4 beyond the superconducting dome. Phys. Rev. Lett. 2023, 131, 116002. [Google Scholar] [CrossRef]
- Emery, V.J.; Kivelson, S.A. Importance of phase fluctuations in superconductors with small superfluid density. Nature 1995, 374, 434–437. [Google Scholar] [CrossRef]
- Uemura, Y.J. Bose-Einstein to BCS crossover picture for high-Tc cuprates. Phys. C 1997, 282–287, 194–197. [Google Scholar] [CrossRef]
- Xu, Z.A.; Ong, N.P.; Wnag, Y.; Kakeshita, T.; Uchida, S. Vortex-like excitations and the onset of superconducting phase fluctuation in underdoped La2-xSrxCuO4. Nature 2000, 406, 486–488. [Google Scholar] [CrossRef]
- Tallon, J.L.; Lorum, J.W. The doping dependence of T*—What is the real Tc phase diagram? Phys. C 2001, 349, 53–68. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Egami, T. Dynamic Correlations in Disordered Systems: Implications for High-Temperature Superconductivity. Condens. Matter 2024, 9, 12. https://doi.org/10.3390/condmat9010012
Egami T. Dynamic Correlations in Disordered Systems: Implications for High-Temperature Superconductivity. Condensed Matter. 2024; 9(1):12. https://doi.org/10.3390/condmat9010012
Chicago/Turabian StyleEgami, Takeshi. 2024. "Dynamic Correlations in Disordered Systems: Implications for High-Temperature Superconductivity" Condensed Matter 9, no. 1: 12. https://doi.org/10.3390/condmat9010012
APA StyleEgami, T. (2024). Dynamic Correlations in Disordered Systems: Implications for High-Temperature Superconductivity. Condensed Matter, 9(1), 12. https://doi.org/10.3390/condmat9010012





