Superconducting and Mechanical Properties of the Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Superconductor Phase Substituted by Lanthanum and Samarium Fluorides
Abstract
1. Introduction
2. Experimental Techniques
2.1. Sample Preparation
2.2. Samples Characterization and Measurements
3. Results and Discussion
3.1. Sample Investigation
3.2. Electrical Transport Measurements
3.2.1. Electrical Resistivity Measurements
3.2.2. Critical Current Density (Jc) Measurements
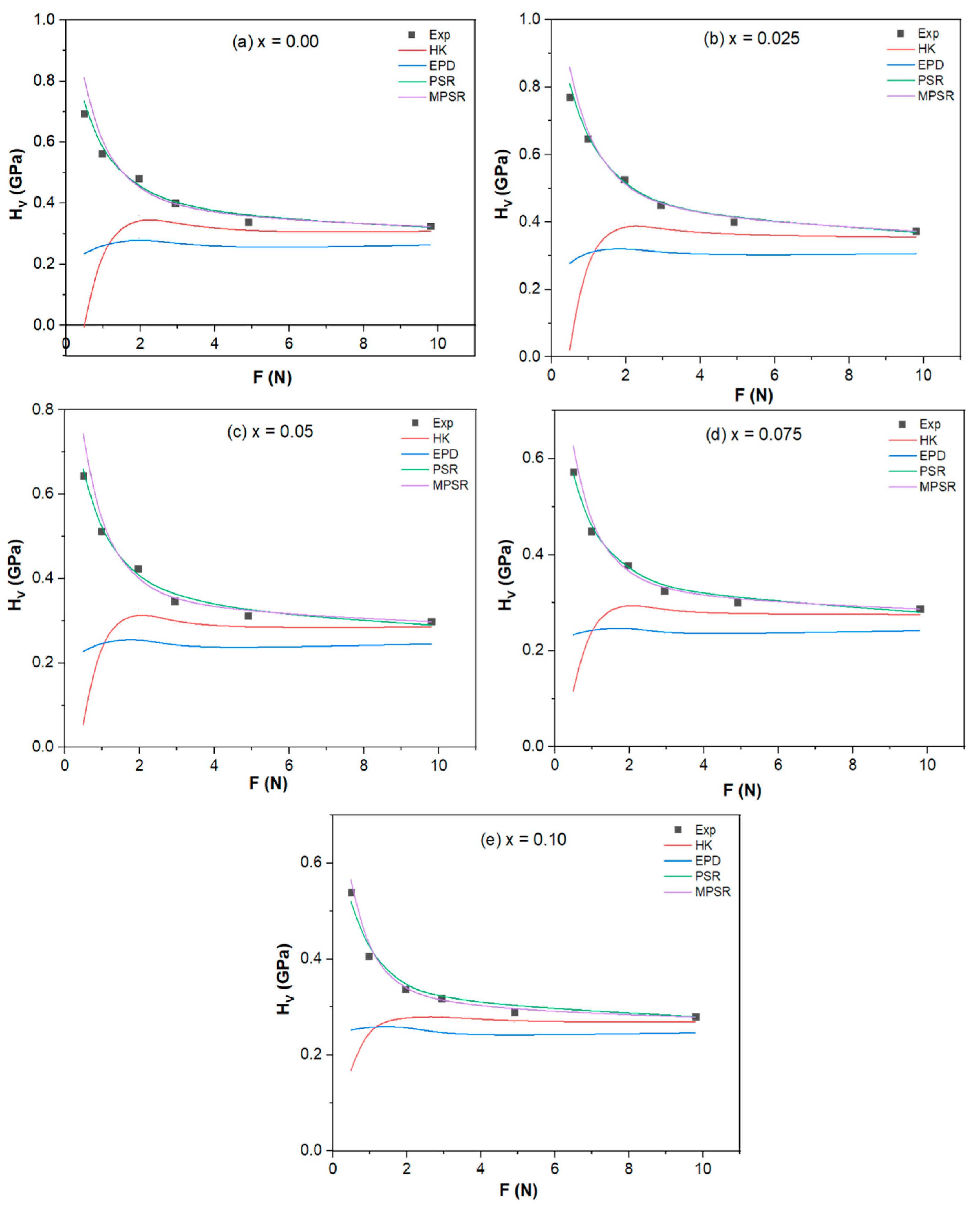
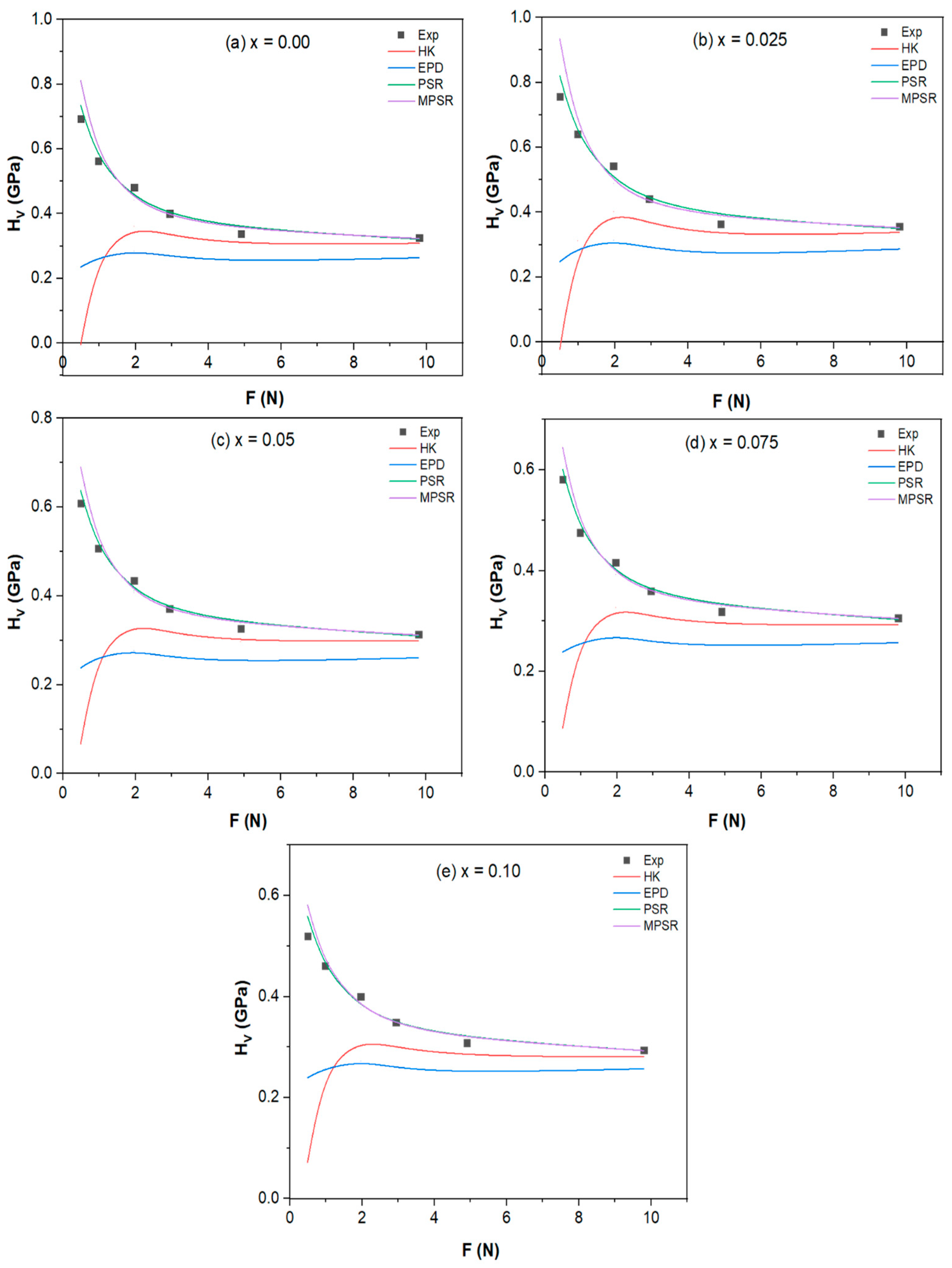
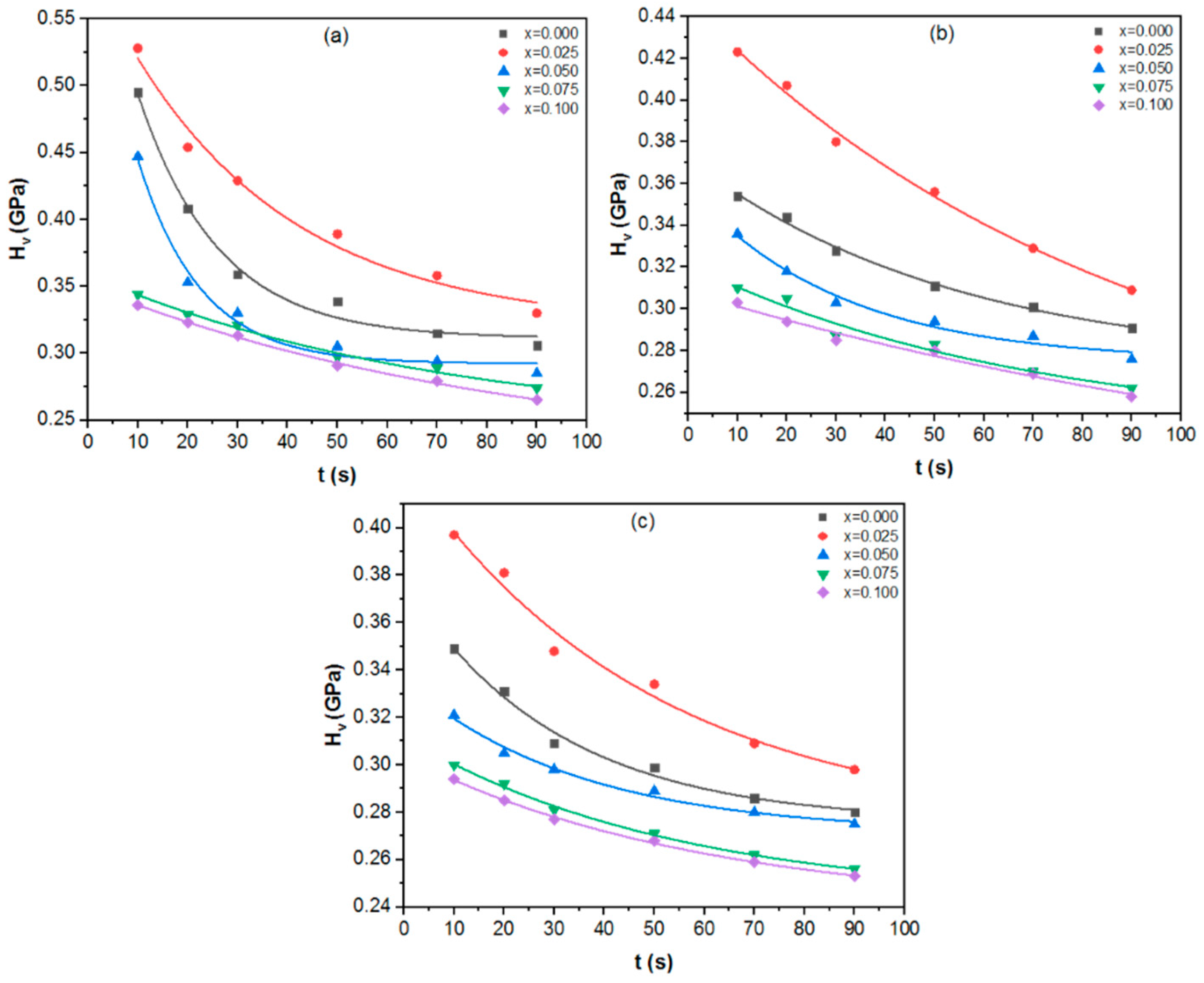
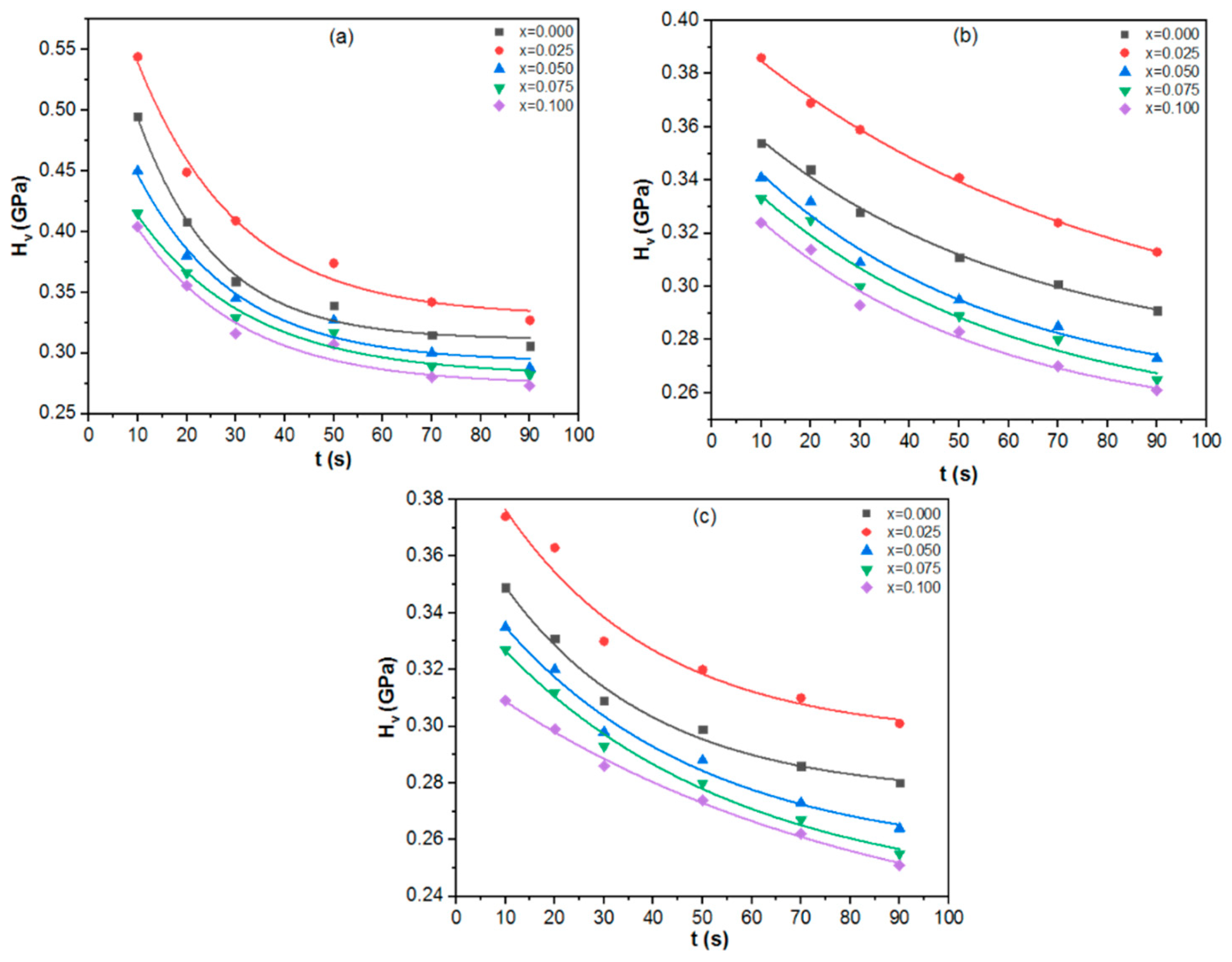
3.3. Vickers Microhardness Measurement Examination
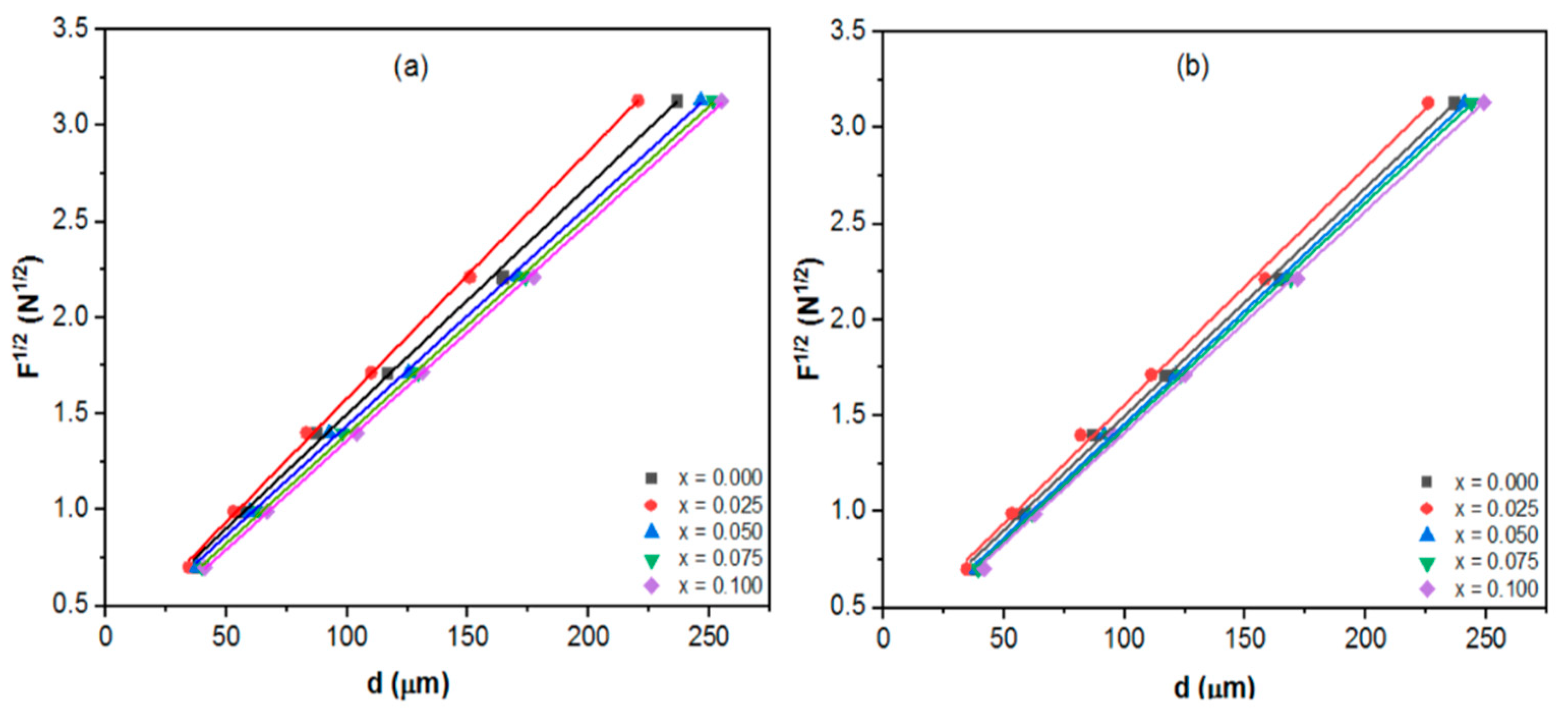
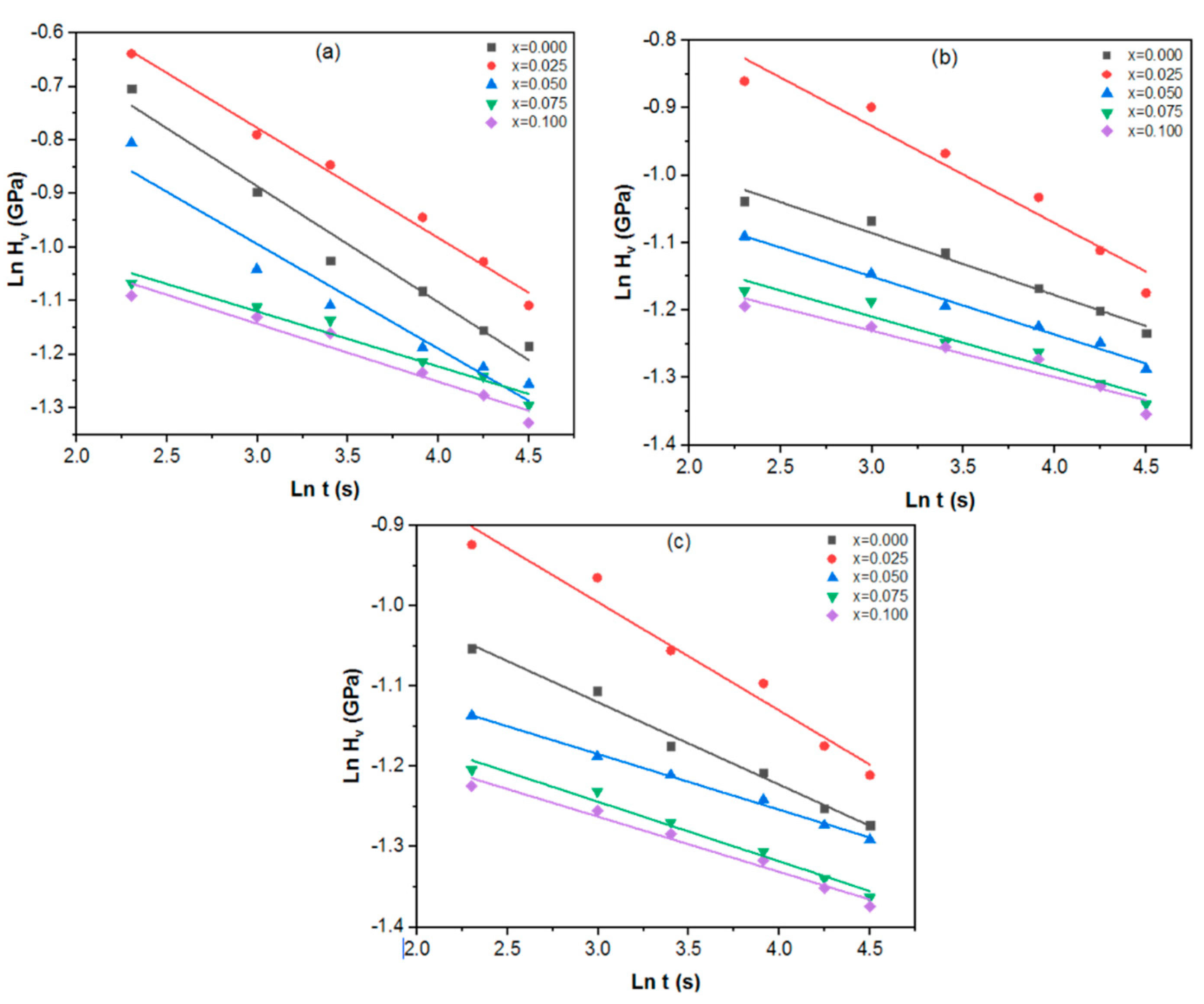
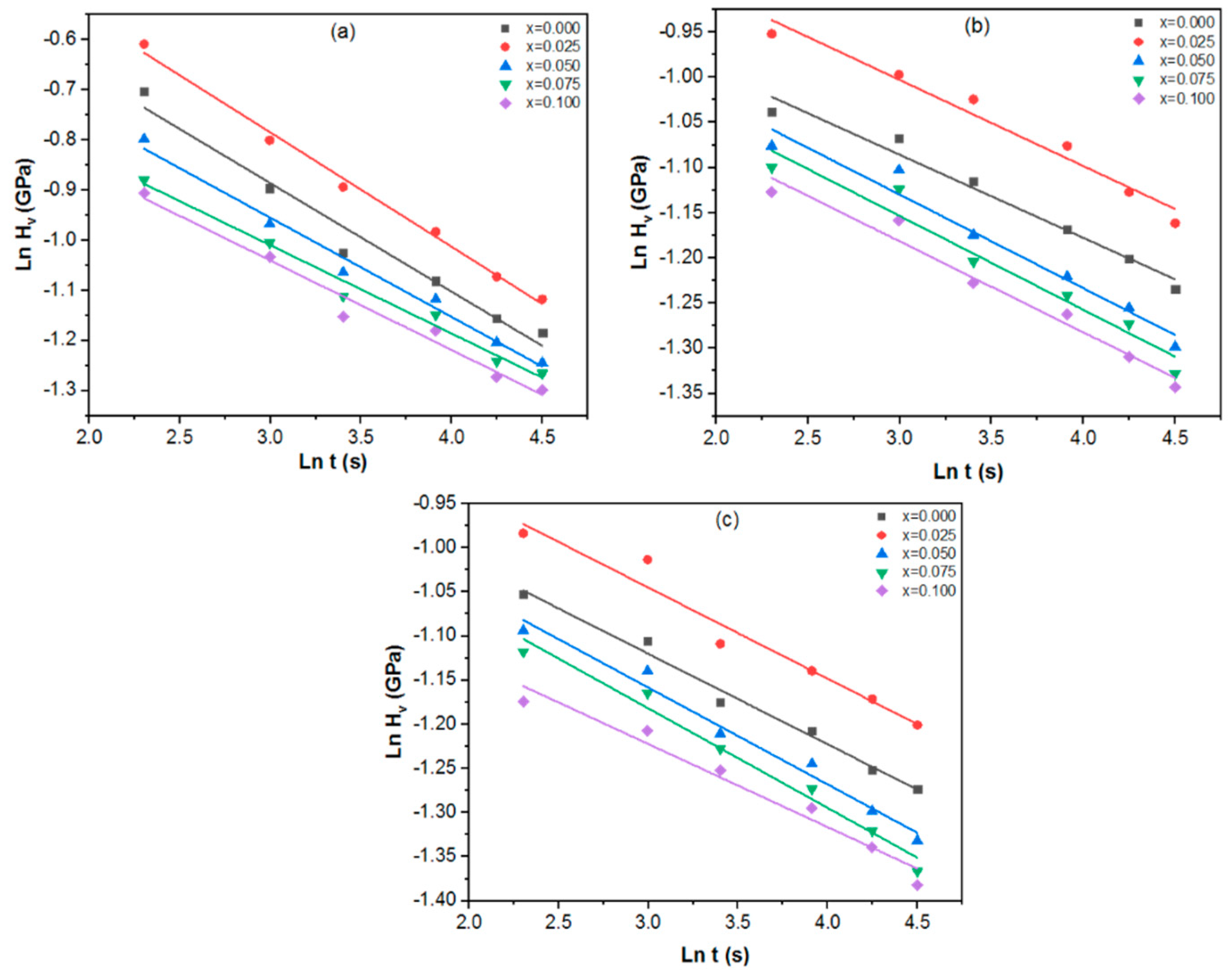
3.3.1. Meyer’s Law
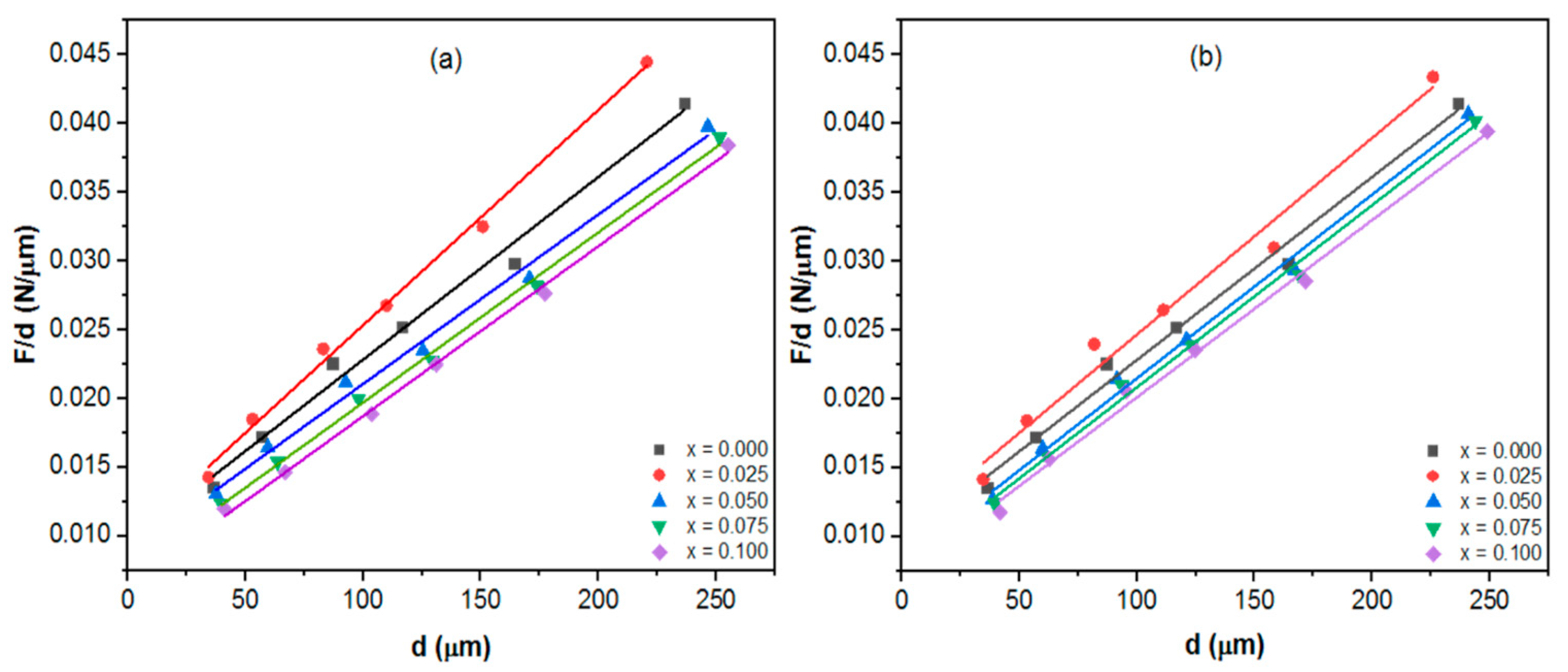
3.3.2. Hays–Kendall Approach
3.3.3. Elastic/Plastic Deformation Model
3.3.4. Proportional Specimen Resistance (PSR) Model
3.3.5. Modified Proportional Specimen Resistance Model (MPSR)
3.3.6. Indentation Creep
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bednorz, J.G.; Müller, K.A. Possible high Tc Superconductivity in the Ba-La-Cu-O System. Z. Phys. B Condens. Matter 1986, 64, 189–193. [Google Scholar] [CrossRef]
- Cigáň, A.; Maňka, J.; Mair, M.; Gritzner, G.; Plesch, G.; Zrubec, V. Influence of the Tl- and Hg-Content on Magnetic and Transport Properties of the Pb, Sr-Doped Tl-1223 and Hg-1223 Superconductors. Phys. C Supercond. 1999, 320, 267–276. [Google Scholar] [CrossRef]
- Piehler, A.; Ströbel, J.P.; Reschauer, N.; Löw, R.; Schönberger, R.; Renk, K.F.; Kraus, M.; Daniel, J.; Saemann-Ischenko, G. Critical Current Density of TlBa2Ca2Cu3O9 Thin Films on MgO (100) in Magnetic Fields. Phys. C Supercond. 1994, 223, 391–395. [Google Scholar] [CrossRef]
- Selvamanickam, V. High Temperature Superconductor (HTS) Wires and Tapes. In High Temperature Superconductors (HTS) for Energy Applications; Elsevier: Amsterdam, The Netherlands, 2012; pp. 34–68. ISBN 978-0-85709-012-6. [Google Scholar]
- Metskhvarishvili, I.; Lobzhanidze, T.E.; Dgebuadze, G.N.; Bendeliani, B.G.; Metskhvarishvili, M.R.; Rusia, M.S.; Gabunia, V.M.; Komakhidze, K. SG and SSR Approach in the Preparation of Precursors and Influence on Superconducting Properties of Tl-1223 Superconductors. Res. Sq. 2020. in review. [Google Scholar] [CrossRef]
- Abed, N.S.; Fathi, S.J.; Jassim, K.A.; Mahdi, S.H. Partial Substitution of Zn Effects on the Structural and Electrical Properties of High Temperature Hg0.95Ag0.05Ba2Ca2Cu3O8+δ Superconductors. J. Phys. Conf. Ser. 2018, 1003, 012098. [Google Scholar] [CrossRef]
- Flores-Livas, J.A.; Boeri, L.; Sanna, A.; Profeta, G.; Arita, R.; Eremets, M. A Perspective on Conventional High-Temperature Superconductors at High Pressure: Methods and Materials. Phys. Rep. 2020, 856, 1–78. [Google Scholar] [CrossRef]
- Drozdov, A.P.; Eremets, M.I.; Troyan, I.A.; Ksenofontov, V.; Shylin, S.I. Conventional Superconductivity at 203 Kelvin at High Pressures in the Sulfur Hydride System. Nature 2015, 525, 73–76. [Google Scholar] [CrossRef]
- Kvashnin, A.G.; Semenok, D.V.; Kruglov, I.A.; Wrona, I.A.; Oganov, A.R. High-Temperature Superconductivity in a Th–H System under Pressure Conditions. ACS Appl. Mater. Interfaces 2018, 10, 43809–43816. [Google Scholar] [CrossRef]
- Chang, I.C.; Liu, J.Z.; Lan, M.D.; Klavins, P.; Shelton, R.N. Superconducting Properties of the System Tl1−xHgxBa2Ca2Cu3O8+δ. Chin. J. Phys. 1996, 34, 497–504. [Google Scholar]
- Pandey, A.K.; Verma, G.D.; Srivastava, O.N. Investigations on the Tl-Doped Hg–Ba–Ca–Cu–O High Temperature Superconductors in Regard to Hole Doping and Microstructural Characteristics. Phys. C Supercond. 1998, 306, 47–57. [Google Scholar] [CrossRef]
- Abou-Aly, A.I.; Awad, R.; Ibrahim, I.H.; Abdeen, W. Effect of Sm-Substitution on the Electrical and Magnetic Properties of (Tl0.8Hg0.2)-1223. J. Alloys Compd. 2009, 481, 462–469. [Google Scholar] [CrossRef]
- Prabitha, V.G.; Biju, A.; Abhilash Kumar, R.G.; Sarun, P.M.; Aloysius, R.P.; Syamaprasad, U. Effect of Sm Addition on (Bi,Pb)-2212 Superconductor. Phys. C Supercond. 2005, 433, 28–36. [Google Scholar] [CrossRef]
- Abou-Aly, A.I.; Awad, R.; Kamal, M.; Anas, M. Excess Conductivity Analysis of (Cu0.5Tl0.5)-1223 Substituted by Pr and La. J. Low Temp. Phys. 2011, 163, 184–202. [Google Scholar] [CrossRef]
- Anas, M. The Effect of PbF2 Doping on the Structural, Electrical and Mechanical Properties of (Bi,Pb)–2223 Superconductor. Chem. Phys. Lett. 2020, 742, 137033. [Google Scholar] [CrossRef]
- Hamdan, N.M.; Ziq, K.A.; Al-Harthi, A.S. Effect of Fluorine on the Phase Formation and Superconducting Properties of Tl-1223 Superconductors. Phys. C Supercond. 1999, 314, 125–132. [Google Scholar] [CrossRef]
- Mohammed, N.H.; Abou-Aly, A.I.; Ibrahim, I.H.; Awad, R.; Rekaby, M. Effect of Nano-Oxides Addition on the Mechanical Properties of (Cu0.5Tl0.5)-1223 Phase. J. Supercond. Nov. Magn. 2011, 24, 1463–1472. [Google Scholar] [CrossRef]
- Awad, R.; Abou Aly, A.I.; Kamal, M.; Anas, M. Mechanical Properties of (Cu0.5Tl0.5)-1223 Substituted by Pr. J. Supercond. Nov. Magn. 2011, 24, 1947–1956. [Google Scholar] [CrossRef]
- Mohammed, L.A.; Jasim, K.A. Synthesis and Study the Structural and Electrical and Mechanical Properties of High Temperature Superconductor Tl0.5Pb0.5Ba2Can−1Cun−xNixO2n+3−δ Substituted with Nickel Oxide for N = 3. IHJPAS 2018, 31, 26–32. [Google Scholar] [CrossRef]
- Khattar, R.F.; Habanjar, K.; Awad, R.; Anas, M. Comparative Study of Structural, Electrical, and Mechanical Properties of (Tl, Hg)-1223 High Temperature Superconducting Phase Substituted by Lead Oxide and Lead Dioxide. J. Low Temp. Phys. 2023, 211, 166–192. [Google Scholar] [CrossRef]
- Abou-Aly, A.I.; Mohammed, N.H.; Roumié, M.; El Khatib, A.; Awad, R.; Nour El Dein, S.A. Ion Beam Analysis and Physical Properties Measurements of (Tl0.8Hg0.2−xSbx)Ba2Ca2Cu3O9−δ. J. Supercond. Nov. Magn. 2009, 22, 495–504. [Google Scholar] [CrossRef]
- Awad, R.; Abou-Aly, A.I.; Ibrahim, I.H.; Abdeen, W. Normal-State Hall Effect Measurements for Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Substituted by Sm and Yb. Solid State Commun. 2008, 146, 92–96. [Google Scholar] [CrossRef]
- El Makdah, M.H.; El Ghouch, N.; El-Dakdouki, M.H.; Awad, R.; Matar, M. Structural, Electrical and Mechanical Properties of the (NdFeO3)x/(CuTl)-1223 Superconductor Phase. Appl. Phys. A 2023, 129, 265. [Google Scholar] [CrossRef]
- Cao, R.; Wang, W.; Ren, Y.; Hu, Z.; Zhou, X.; Xu, Y.; Luo, Z.; Liang, A. Synthesise, Energy Transfer and Tunable Emission Properties of Ba2La2ZnW2O12:Sm3+ Phosphors. J. Lumin. 2021, 235, 118054. [Google Scholar] [CrossRef]
- Bae, E.G.; Jeong, J.; Han, S.C.; Kwak, J.S.; Pyo, M. Calcium-Doping for Structure Stabilization of Sodium Transition Metal Oxide Cathodes in Sodium Ion Batteries. Meet. Abstr. 2014, MA2014-04, 390. [Google Scholar] [CrossRef]
- Nasser, A.; Srour, A.; El Ghouch, N.; Malaeb, W.; Al-Oweini, R.; Awad, R. Investigation of the Physical Properties of (Cu0.5Tl0.5)Ba2Ca2Cu3O10−δ Impregnated with Mono Cobalt(II)-Substituted Undecatungstosilicate Nanoparticles. Appl. Phys. A 2020, 126, 951. [Google Scholar] [CrossRef]
- Anas, M.; El-Shorbagy, G.A. Impact of Nano-Sized Diluted Magnetic Semiconductors Addition on (Cu, Tl)1234 Superconducting Phase. J. Low Temp. Phys. 2019, 194, 183–196. [Google Scholar] [CrossRef]
- Abou Aly, A.; Ibrahim, I.; Awad, R.; El-Harizy, A.; Khalaf, A. Stabilization of Tl-1223 Phase by Arsenic Substitution. J. Supercond. Nov. Magn. 2010, 23, 1325–1332. [Google Scholar] [CrossRef]
- Ulgen, A.T.; Turgay, T.; Terzioglu, C.; Yildirim, G.; Oz, M. Role of Bi/Tm Substitution in Bi-2212 System on Crystal Structure Quality, Pair Wave Function and Polaronic States. J. Alloys Compd. 2018, 764, 755–766. [Google Scholar] [CrossRef]
- Aftabi, A.; Mozaffari, M. Fluctuation Induced Conductivity and Pseudogap State Studies of Bi1.6Pb0.4Sr2Ca2Cu3O10+δ Superconductor Added with ZnO Nanoparticles. Sci. Rep. 2021, 11, 4341. [Google Scholar] [CrossRef]
- Mortada-Hamid, H.; Ilhamsyah, A.B.P.; Abd-Shukor, R. Formation of Tl-1212 Phase in Ga-Substituted Tl(BaSr)Ca1−xGaxCu2O7 Superconductor. J. Mater. Sci. Mater. Electron. 2020, 31, 5316–5323. [Google Scholar] [CrossRef]
- Talantsev, E.F. The Electron–Phonon Coupling Constant and the Debye Temperature in Polyhydrides of Thorium, Hexadeuteride of Yttrium, and Metallic Hydrogen Phase III. J. Appl. Phys. 2021, 130, 195901. [Google Scholar] [CrossRef]
- Awad, R.; Abou-Aly, A.I.; Mahmoud, S.A.; Barakat, M.M. Thermal Expansion Measurements Using X-Ray Powder Diffraction of Tl-1223 Substituted by Molybdenum. Supercond. Sci. Technol. 2007, 20, 401–405. [Google Scholar] [CrossRef]
- Abou Aly, A.I.; Awad, R.; Ibrahim, I.H.; Kamal, M.; Anas, M. Thermomechanical Analysis of (Cu0.5Tl0.5)-1223 Substituted by Pr and La. J. Mater. Sci. Technol. 2012, 28, 169–176. [Google Scholar] [CrossRef]
- Seiler, E.; Gömöry, F.; Mišík, J.; Richter, D. Critical Current Density of Coated Conductors Determined from Rescaled Magnetic Moment at Temperatures Close to 77 K. Phys. C Supercond. Its Appl. 2018, 551, 66–71. [Google Scholar] [CrossRef]
- Vallès, F.; Palau, A.; Rouco, V.; Mundet, B.; Obradors, X.; Puig, T. Angular Flux Creep Contributions in YBa2Cu3O7−δ Nanocomposites from Electrical Transport Measurements. Sci. Rep. 2018, 8, 5924. [Google Scholar] [CrossRef]
- Katona, T.M.; Pierson, S.W. Zero-Field Current-Voltage Characteristics in High-Temperature Superconductors. Phys. C Supercond. 1996, 270, 242–248. [Google Scholar] [CrossRef]
- Jukna, A. Study of Energy Dissipation in the Mixed-State YBa2Cu3O7−δ Superconductor with Partially Deoxygenated Structures. Materials 2022, 15, 4260. [Google Scholar] [CrossRef]
- Oboudi, S.F. Synthesis and Magnetic Properties of Bi1.7Pb0.3Sr2Ca2Cu3O10+δ Added with Nano Y. J. Supercond. Nov. Magn. 2017, 30, 1473–1482. [Google Scholar] [CrossRef]
- Ghazanfari, N.; Kılıç, A.; Gencer, A.; Özkan, H. Effects of Nb2O5 Addition on Superconducting Properties of BSCCO. Solid State Commun. 2007, 144, 210–214. [Google Scholar] [CrossRef]
- Nurbaisyatul, E.S.; Azhan, H.; Ibrahim, N.; Saipuddin, S.F. Structural and Superconducting Properties of Low-Density Bi(Pb)-2223 Superconductor: Effect of Eu2O3 Nanoparticles Addition. Cryogenics 2021, 119, 103353. [Google Scholar] [CrossRef]
- Zalaoglu, Y.; Bekiroglu, E.; Dogruer, M.; Yildirim, G.; Ozturk, O.; Terzioglu, C. Comparative Study on Mechanical Properties of Undoped and Ce-Doped Bi-2212 Superconductors. J. Mater. Sci. Mater. Electron. 2013, 24, 2339–2345. [Google Scholar] [CrossRef]
- Leenders, A.; Mich, M.; Freyhard, H.C. Influence of Thermal Cycling on the Mechanical Properties of VGF Melt-Textured YBCO. Phys. C Supercond. 1997, 279, 173–180. [Google Scholar] [CrossRef]
- Tickoo, R.; Tandon, R.P.; Bamzai, K.K.; Kotru, P.N. Microindentation Studies on Samarium-Modified Lead Titanate Ceramics. Mater. Chem. Phys. 2003, 80, 446–451. [Google Scholar] [CrossRef]
- Sahoo, B.; Behera, D. Investigation of Superconducting and Elastic Parameters of YBCO/LSMO Thick Films. J. Mater. Sci. Mater. Electron. 2019, 30, 12992–13004. [Google Scholar] [CrossRef]
- Foerster, C.E.; Lima, E.; Rodrigues Jr, P.; Serbena, F.C.; Lepienski, C.M.; Cantão, M.P.; Jurelo, A.R.; Obradors, X. Mechanical Properties of Ag-Doped Top-Seeded Melt-Grown YBCO Pellets. Braz. J. Phys. 2008, 38, 341–345. [Google Scholar] [CrossRef]
- Saritekin, N.K.; Üzümcü, A.T. Improving Superconductivity, Microstructure, and Mechanical Properties by Substituting Different Ionic Pb Elements to Bi and Ca Elements in Bi-2223 Superconductors. J. Supercond. Nov. Magn. 2022, 35, 2259–2273. [Google Scholar] [CrossRef]
- Khalil, S.M. Effect of Y3+ Substitution for Ca on the Transport and Mechanical Properties of Bi2Sr2Ca1−xYxCu2O8+δ System. J. Phys. Chem. Solids 2003, 64, 855–861. [Google Scholar] [CrossRef]
- Koralay, H.; Arslan, A.; Cavdar, S.; Ozturk, O.; Asikuzun, E.; Gunen, A.; Tasci, A.T. Structural and Mechanical Characterization of Bi1.75Pb0.25Sr2Ca2Cu3−xSnxO10+y Superconductor Ceramics Using Vickers Microhardness Test. J. Mater. Sci. Mater. Electron. 2013, 24, 4270–4278. [Google Scholar] [CrossRef]
- Terzioglu, R.; Altintas, S.P.; Varilci, A.; Terzioğlu, C. Modeling of Micro-Hardness in the Au-Doped YBCO Bulk Superconductors. J. Supercond. Nov. Magn. 2019, 32, 3377–3383. [Google Scholar] [CrossRef]
- Hays, C.; Kendall, E.G. An Analysis of Knoop Microhardness. Metallography 1973, 6, 275–282. [Google Scholar] [CrossRef]
- Lawn, B.R.; Howes, V.R. Elastic Recovery at Hardness Indentations. J. Mater. Sci. 1981, 16, 2745–2752. [Google Scholar] [CrossRef]
- Dogruer, M.; Yildirim, G.; Ozturk, O.; Terzioglu, C. Analysis of Indentation Size Effect on Mechanical Properties of Cu-Diffused Bulk MgB2 Superconductor Using Experimental and Different Theoretical Models. J. Supercond. Nov. Magn. 2013, 26, 101–109. [Google Scholar] [CrossRef]
- Li, H.; Bradt, R.C. The Microhardness Indentation Load/Size Effect in Rutile and Cassiterite Single Crystals. J. Mater. Sci. 1993, 28, 917–926. [Google Scholar] [CrossRef]
- Gong, J.; Miao, H.; Zhao, Z.; Guan, Z. Load-Dependence of the Measured Hardness of Ti (C, N)-Based Cermets. Mater. Sci. Eng. A 2001, 303, 179–186. [Google Scholar] [CrossRef]
- Quinn, J.B.; Quinn, G.D. Indentation Brittleness of Ceramics: A Fresh Approach. J. Mater. Sci. 1997, 32, 4331–4346. [Google Scholar] [CrossRef]
- Rekaby, M.; Mohammed, N.H.; Ahmed, M.; Abou-Aly, A.I. Synthesis, Microstructure and Indentation Vickers Hardness for (Y3Fe5O12)x/Cu0.5Tl0.5Ba2Ca2Cu3O10−δ Composites. Appl. Phys. A 2022, 128, 261. [Google Scholar] [CrossRef]
- Turkoz, M.B.; Zalaoglu, Y.; Turgay, T.; Ozturk, O.; Akkurt, B.; Yildirim, G. Evaluation of Key Mechanical Design Properties and Mechanical Characteristic Features of Advanced Bi-2212 Ceramic Materials with Homovalent Bi/Ga Partial Replacement: Combination of Experimental and Theoretical Approaches. Ceram. Int. 2019, 45, 21183–21192. [Google Scholar] [CrossRef]
- Zewen, W.; Wanqi, J. Microhardness of Hg1−xMnxTe. Mater. Sci. Eng. A 2007, 452–453, 508–511. [Google Scholar] [CrossRef]
- Zaki, H.M.; Abdel-Daiem, A.M.; Swilem, Y.I.; El-Tantawy, F.; Al-Marzouki, F.M.; Al-Ghamdi, A.A.; Al-Heniti, S.; Al-Hazmi, F.S.; Al-Harbi, T.S. Indentation Creep Behavior and Microstructure of Cu-Ge Ferrites. Mater. Sci. Appl. 2011, 2, 1076. [Google Scholar] [CrossRef][Green Version]
- PM, S.; Ashby, M.F. Indentation Creep. Mater. Sci. Technol. 1992, 8, 594–601. [Google Scholar] [CrossRef]
- Langdon, T.G. Identifiying Creep Mechanisms at Low Stresses. Mater. Sci. Eng. A 2000, 283, 266–273. [Google Scholar] [CrossRef]
- Walser, B.; Sherby, O.D. The Structure Dependence of Power Law Creep. Scr. Metall. 1982, 16, 213–219. [Google Scholar] [CrossRef]
- Sharma, G.; Ramanujan, R.V.; Kutty, T.R.G.; Tiwari, G.P. Hot Hardness and Indentation Creep Studies of a Fe–28Al–3Cr–0.2 C Alloy. Mater. Sci. Eng. A 2000, 278, 106–112. [Google Scholar] [CrossRef]
- Goetze, C.; Brace, W.F. Laboratory Observations of High-Temperature Rheology of Rocks. In Developments in Geotectonics; Elsevier: Amsterdam, The Netherlands, 1972; Volume 4, pp. 583–600. [Google Scholar] [CrossRef]
- Abd El-Salam, F.; Wahab, L.A.; Nada, R.H.; Zahran, H.Y. Temperature and Dwell Time Effect on Hardness of Al-Base Alloys. J. Mater. Sci. 2007, 42, 3661–3669. [Google Scholar] [CrossRef]

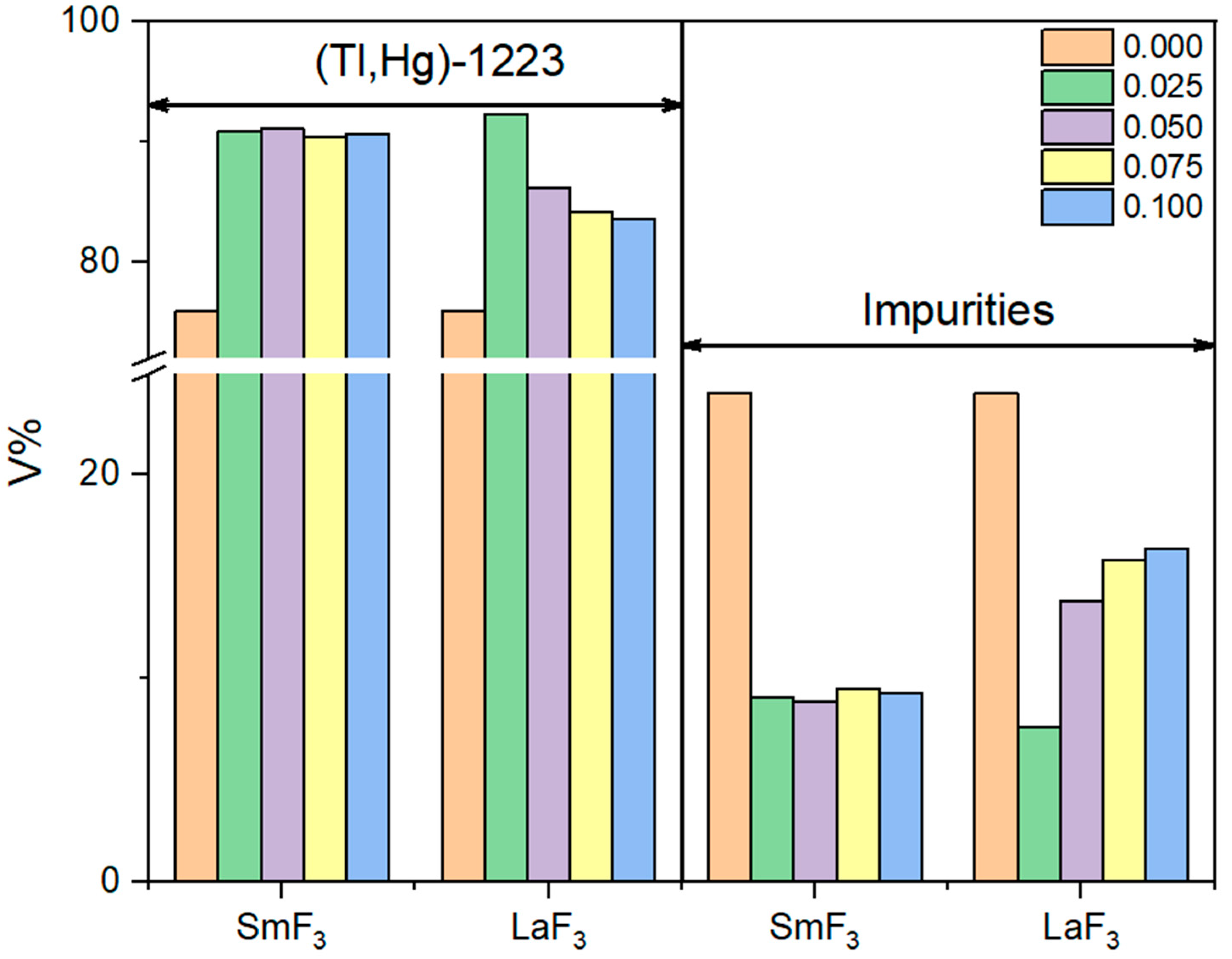



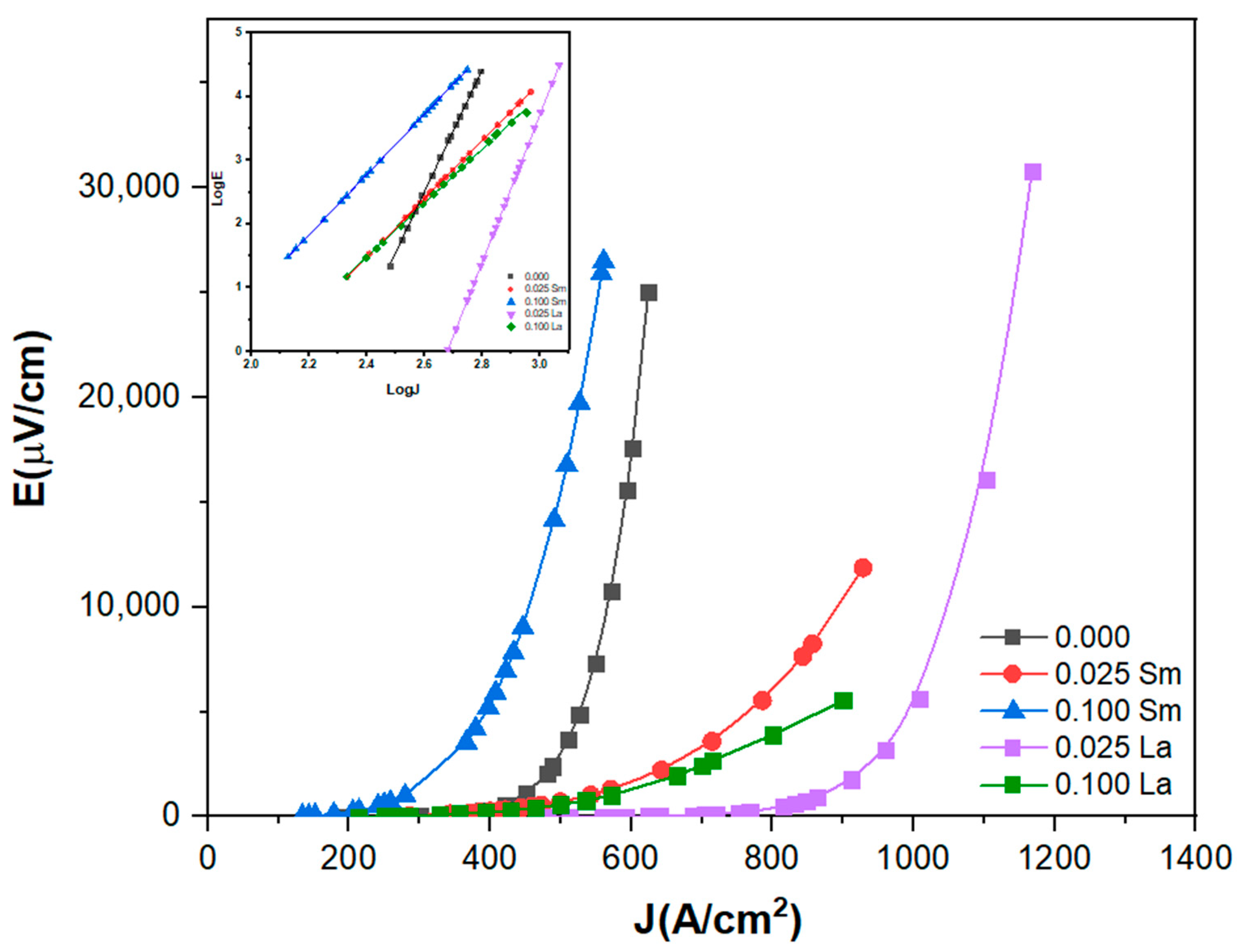

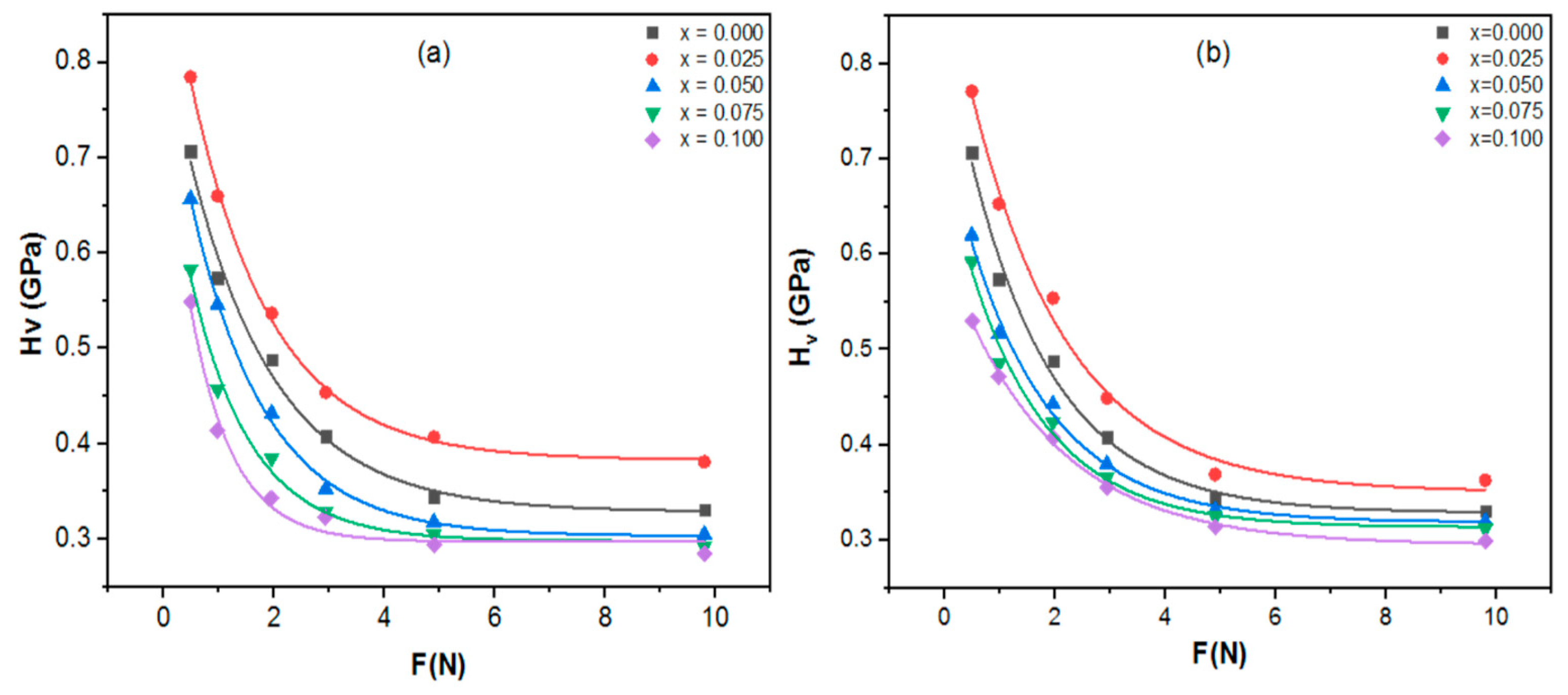
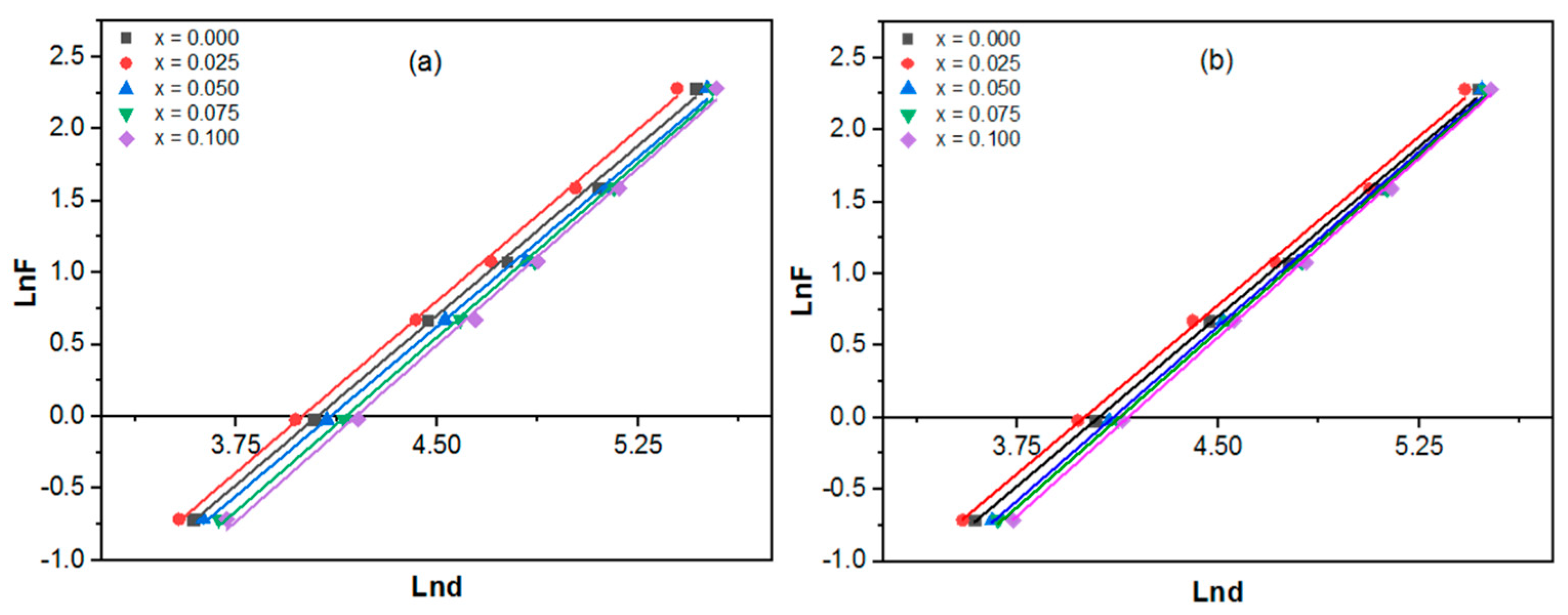



| x | α (mΩ.cm/K) | T0 (K) | Tc (K) | ΔT (K) | ρo (Ω.cm) | ρn (Ω.cm) | (K) | λ |
|---|---|---|---|---|---|---|---|---|
| 0.000 | 2.73 | 102.6 | 105.4 | 2.85 | −0.071 | 0.736 | 264.81 | 0.950 |
| Sm Substitution | ||||||||
| 0.025 | 2.16 | 103.4 | 112.0 | 8.60 | 0.029 | 0.679 | 345.42 | 0.795 |
| 0.050 | 1.78 | 100.3 | 110.5 | 10.15 | 0.033 | 0.563 | 342.52 | 0.792 |
| 0.075 | 1.23 | 99.3 | 105.3 | 6.00 | 0.148 | 0.514 | 339.37 | 0.768 |
| 0.100 | 1.74 | 91.3 | 99.9 | 8.70 | 0.264 | 0.781 | 313.48 | 0.784 |
| La Substitution | ||||||||
| 0.025 | 2.25 | 105.8 | 116.7 | 10.90 | 0.096 | 0.706 | 334.88 | 0.843 |
| 0.050 | 1.04 | 102.1 | 109.5 | 7.40 | 0.050 | 0.360 | 315.94 | 0.840 |
| 0.075 | 3.16 | 99.3 | 106.5 | 7.20 | 0.086 | 1.016 | 308.01 | 0.838 |
| 0.100 | 2.45 | 97.0 | 105.2 | 7.80 | 0.170 | 0.893 | 304.77 | 0.837 |
| x | Meyer | HK | EPD | PSR | MPSR | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| n | A′ × 10−3 | W | A1 ×10−4 | A2 × 10−3 | d0 | α × 10−3 | β × 10−4 | α2 | α3 × 10−2 | α4 × 10−4 | |
| (N/µm2) | (N) | (N/µm2) | (N/µm2) | (µm) | (N/µm) | (N/µm2) | (N) | (N/µm) | (N/µm2) | ||
| 0.000 | 1.603 | 1.405 | 0.493 | 1.670 | 0.142 | 25.982 | 9.240 | 1.350 | 0.148 | 0.654 | 1.440 |
| Sm Substituted Samples | |||||||||||
| 0.025 | 1.590 | 1.750 | 0.476 | 1.930 | 0.166 | 22.828 | 9.660 | 1.570 | 0.079 | 0.800 | 1.630 |
| 0.050 | 1.569 | 1.607 | 0.449 | 1.540 | 0.131 | 25.696 | 8.710 | 1.230 | 0.201 | 0.448 | 1.390 |
| 0.075 | 1.613 | 1.227 | 0.391 | 1.490 | 0.129 | 22.591 | 7.320 | 1.240 | 0.163 | 0.395 | 1.360 |
| 0.100 | 1.637 | 1.104 | 0.337 | 1.460 | 0.128 | 20.160 | 6.350 | 1.240 | 0.164 | 0.293 | 1.360 |
| La Substituted Samples | |||||||||||
| 0.025 | 1.616 | 1.940 | 0.550 | 1.607 | 0.137 | 24.803 | 10.380 | 1.430 | 0.207 | 0.594 | 1.600 |
| 0.050 | 1.631 | 1.311 | 0.505 | 1.290 | 0.101 | 36.993 | 8.120 | 1.340 | 0.122 | 0.572 | 1.420 |
| 0.075 | 1.657 | 1.184 | 0.549 | 1.940 | 0.164 | 25.612 | 7.590 | 1.320 | 0.105 | 0.557 | 1.400 |
| 0.100 | 1.580 | 1.010 | 0.534 | 1.860 | 0.158 | 25.383 | 7.220 | 1.290 | 0.045 | 0.657 | 1.310 |
| F | x = 0.00 | x = 0.025 | x = 0.050 | x = 0.075 | x = 0.10 |
|---|---|---|---|---|---|
| Sm Substitution | |||||
| 2.94 | 4.625 | 4.875 | 5.125 | 9.749 | 9.251 |
| 4.9 | 10.875 | 6.949 | 11.627 | 12.907 | 14.550 |
| 9.8 | 9.749 | 7.423 | 14.447 | 13.490 | 14.554 |
| La Substitution | |||||
| 2.94 | 4.625 | 4.388 | 5.058 | 5.683 | 5.610 |
| 4.9 | 10.875 | 10.926 | 9.653 | 9.631 | 9.937 |
| 9.8 | 9.749 | 9.690 | 9.117 | 8.869 | 10.628 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khattar, R.F.; Anas, M.; Awad, R.; Habanjar, K. Superconducting and Mechanical Properties of the Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Superconductor Phase Substituted by Lanthanum and Samarium Fluorides. Condens. Matter 2023, 8, 87. https://doi.org/10.3390/condmat8040087
Khattar RF, Anas M, Awad R, Habanjar K. Superconducting and Mechanical Properties of the Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Superconductor Phase Substituted by Lanthanum and Samarium Fluorides. Condensed Matter. 2023; 8(4):87. https://doi.org/10.3390/condmat8040087
Chicago/Turabian StyleKhattar, Rola F., Mohammed Anas, Ramadan Awad, and Khulud Habanjar. 2023. "Superconducting and Mechanical Properties of the Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Superconductor Phase Substituted by Lanthanum and Samarium Fluorides" Condensed Matter 8, no. 4: 87. https://doi.org/10.3390/condmat8040087
APA StyleKhattar, R. F., Anas, M., Awad, R., & Habanjar, K. (2023). Superconducting and Mechanical Properties of the Tl0.8Hg0.2Ba2Ca2Cu3O9−δ Superconductor Phase Substituted by Lanthanum and Samarium Fluorides. Condensed Matter, 8(4), 87. https://doi.org/10.3390/condmat8040087









