Is Nematicity in Cuprates Real?
Abstract
1. Introduction
1.1. Electronic Nematicity
1.2. Nematicity in LSCO
1.3. Transverse Voltage Due to Anisotropic Resistivity
1.4. The Angle-Resolved Transverse Resistivity Measurements
1.5. Key Experimental Observations: Unexpected Transverse Voltage
2. Reproducibility and Statistics
3. Artifacts
3.1. Contact Misalignment
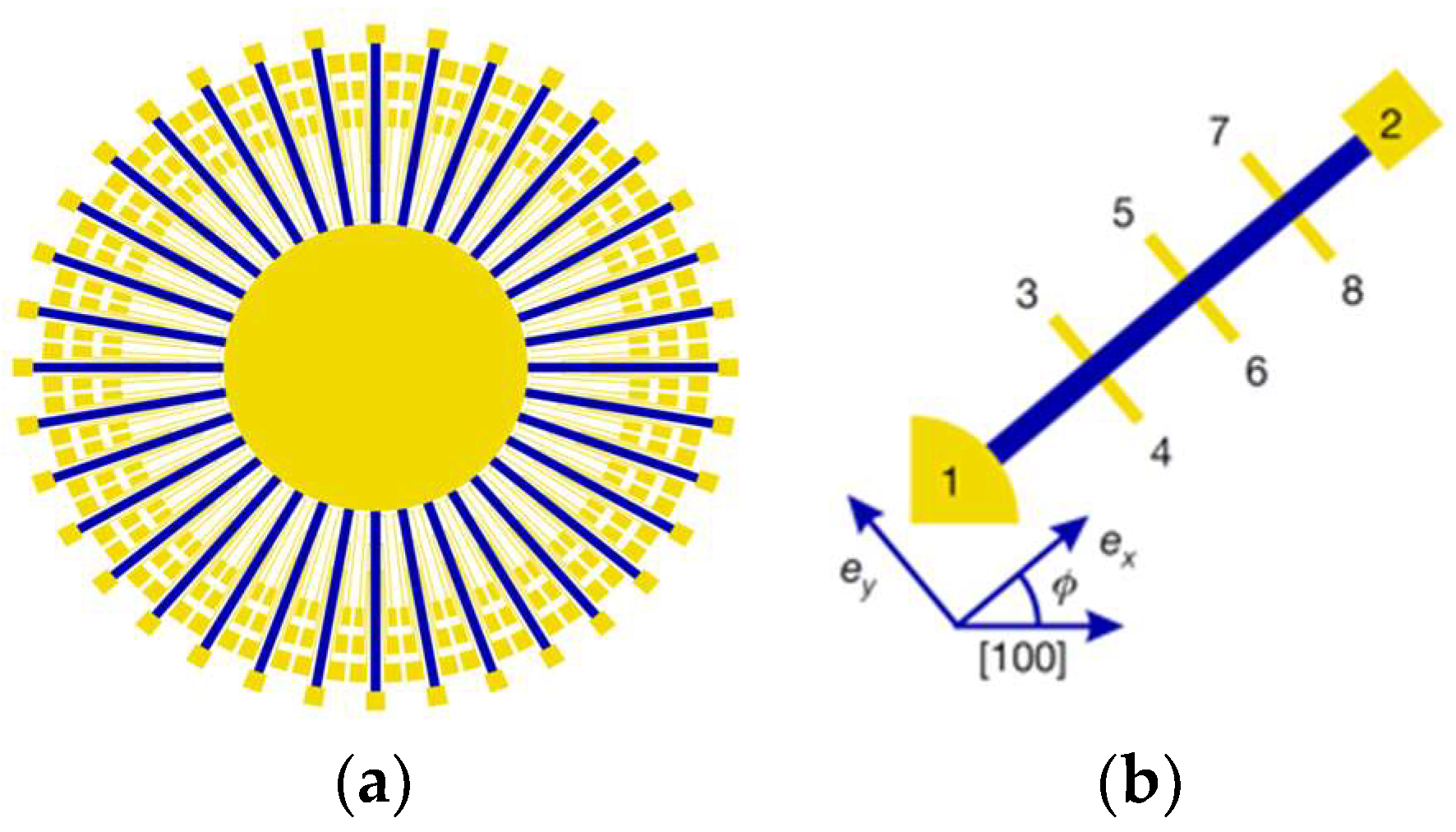
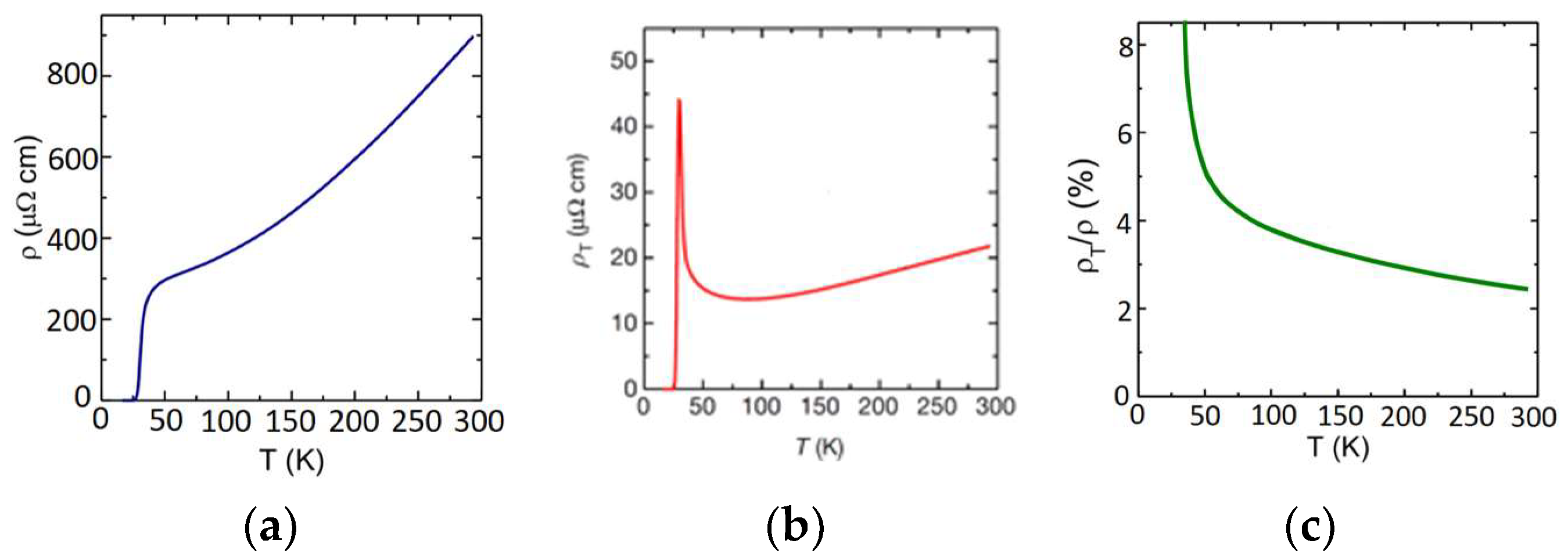
- The observed effect is way too large. In the lithography pattern we used (Figure 1), the width of the Hall bar is W = 100 µm, and the distance between the two neighboring voltage contacts is L = 300 µm. Assuming, conservatively, the upper limit for the misalignment, l = 0.5 µm, this would give and . However, this is much smaller than the experimental value in Figure 2c, where at room temperature, while near Tc it surges to 30%. To produce such a big effect, the contact misalignment would have to be 30 µm, and that would be visible under an optical microscope or even to the naked eye.
- does not scale with. From the above formula, the ratio is determined solely by the value of , so it should be independent of the temperature. However, this contradicts our experimental observations; as illustrated in Figure 2, the ratio varies significantly with T, increasing very steeply near Tc.
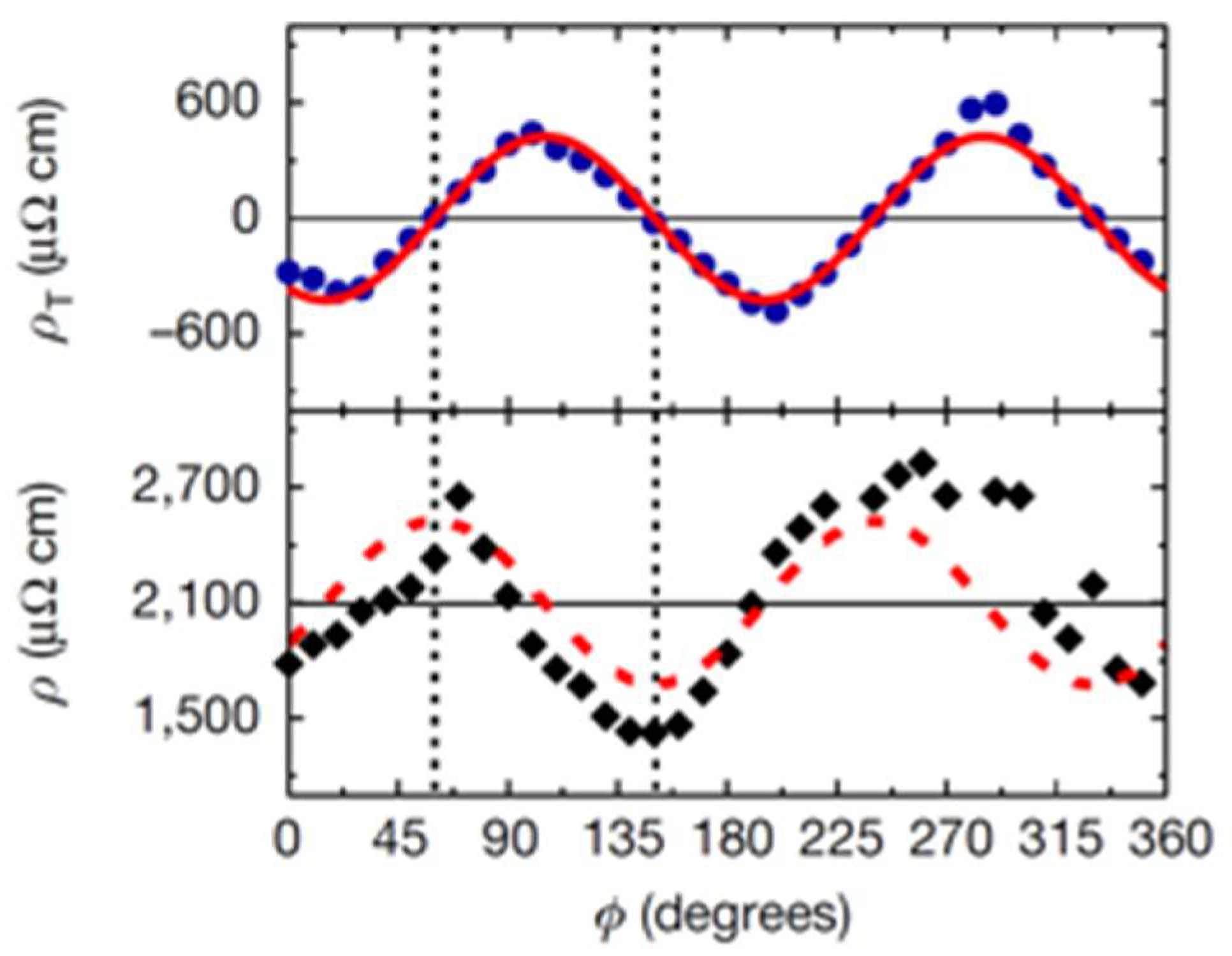
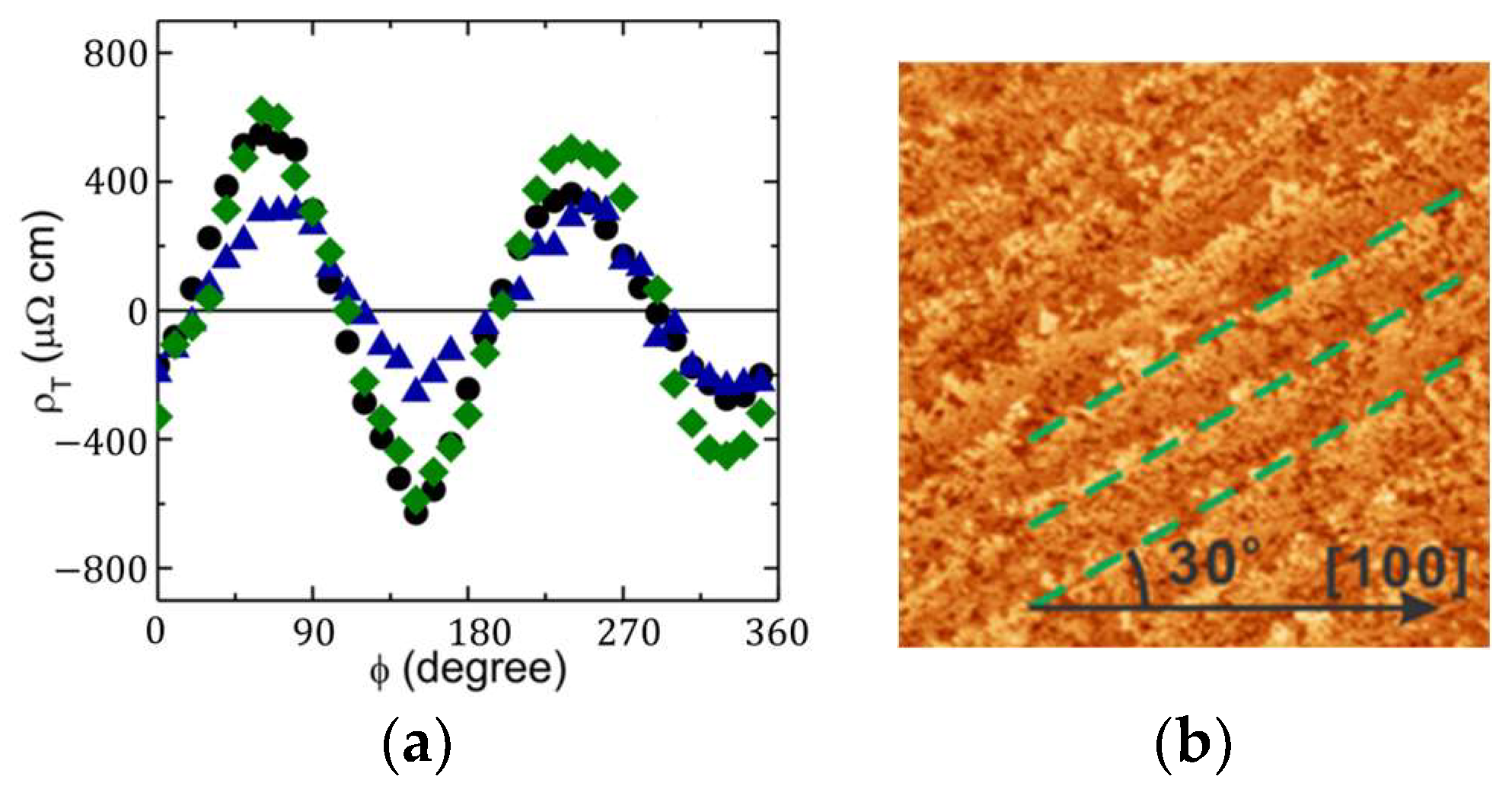
- does not scale with. We demonstrated that both and vary strongly and systematically with the azimuth angle —they oscillate with the same amplitude and period () while they are phase-shifted by from one another, as shown in Figure 3. The misalignment of transverse voltage contacts certainly cannot explain the observed angular dependence of the longitudinal resistivity, .
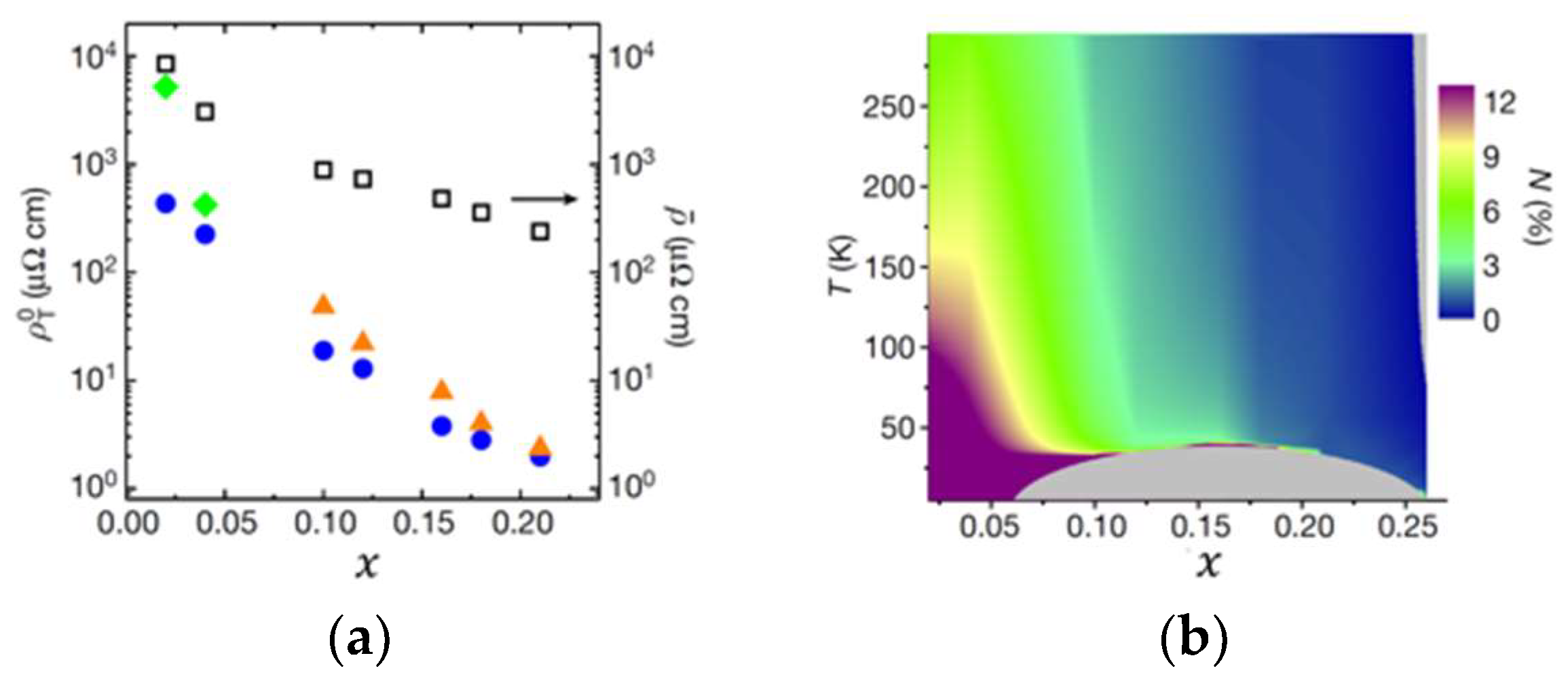
- does not scale with. We studied the dependence of and in LSCO as a function of the level of chemical doping x and found that the ratio varies strongly and systematically with x, from more than 50% for x = 0.02 to less than 0.5% for x = 0.22 (see Figure 5). Since we used the same lithography mask to fabricate all these devices, the contact misalignment should not change with x.
3.2. Orthorhombic Distortion
- The observed effect is way too large. The orthorhombic distortions in our films are suppressed because the films are very thin (20-unit-cells thick) and epitaxially anchored to the tetragonal LaSrAlO4 (LSAO) substrates. In consequence, the crystal structure of our LSCO films is almost exactly tetragonal at all doping levels. To be quantitative, X-ray diffraction data in twice-thicker LSCO films grown on LSAO substrates by ALL-MBE show the in-plane orthorhombic distortion of just 0.08% in insulating, 0.04% in optimally doped, and 0.01% in overdoped metallic LSCO films, respectively [31]. Hence, even at the lowest doping levels, the orthorhombic distortion in films is quite small—at least a factor of 20 smaller than in the corresponding bulk samples—and the distortion energy must be reduced even more. Consider a charge-density wave (CDW) in the simple Peierls’ model for a rough estimate. The total CDW condensation energy E(Q) should scale as , where Q is the distortion amplitude. Using this rule of thumb, the estimated E(Q) in our thin films should be about three orders of magnitude smaller than in the bulk crystals, i.e., basically negligible.
- does not depend on substrate. As a control experiment, we deposited LSCO on orthorhombic NdGaO3 (NGO) substrates. In these substrates and the thin LSCO films grown on top, the orthorhombic distortion is substantial, with , where a and b are the in-plane lattice constants. Nevertheless, we found that the amplitude of nematicity, was nearly the same in these orthorhombic LSCO films as in the tetragonal LSCO films on LSAO substrates. The only difference was that in the films on NGO, the nematic director was pinned to the crystallographic [100] direction. In contrast, in the LSCO films on LSAO, the angle was generally different and dependent on doping and temperature.
- does not depend on uniaxial pressure. In the second control experiment, we used a custom-built mechanical clamping device to apply uniaxial pressure on LSCO films grown on LSAO substrates. We could achieve up to 0.9% uniaxial strain and orthorhombic distortion. However, we found no effect on the amplitude of nematicity [32], consistent with paragraph 2 above. Note that this distortion is larger than the one spontaneously occurring in free-standing LSCO crystals (0.8% in undoped La2CuO4 and decreasing with Sr doping).
- The temperature dependence ofdoes not match. In free-standing bulk LSCO crystals, orthorhombic distortion develops in a mean-field manner and becomes nearly constant below about 0.5 . In stark contrast, shows a strong peak near Tc, rising by a factor of 5–10 in a narrow temperature interval above Tc.
3.3. Strain
3.4. Substrate Miscut
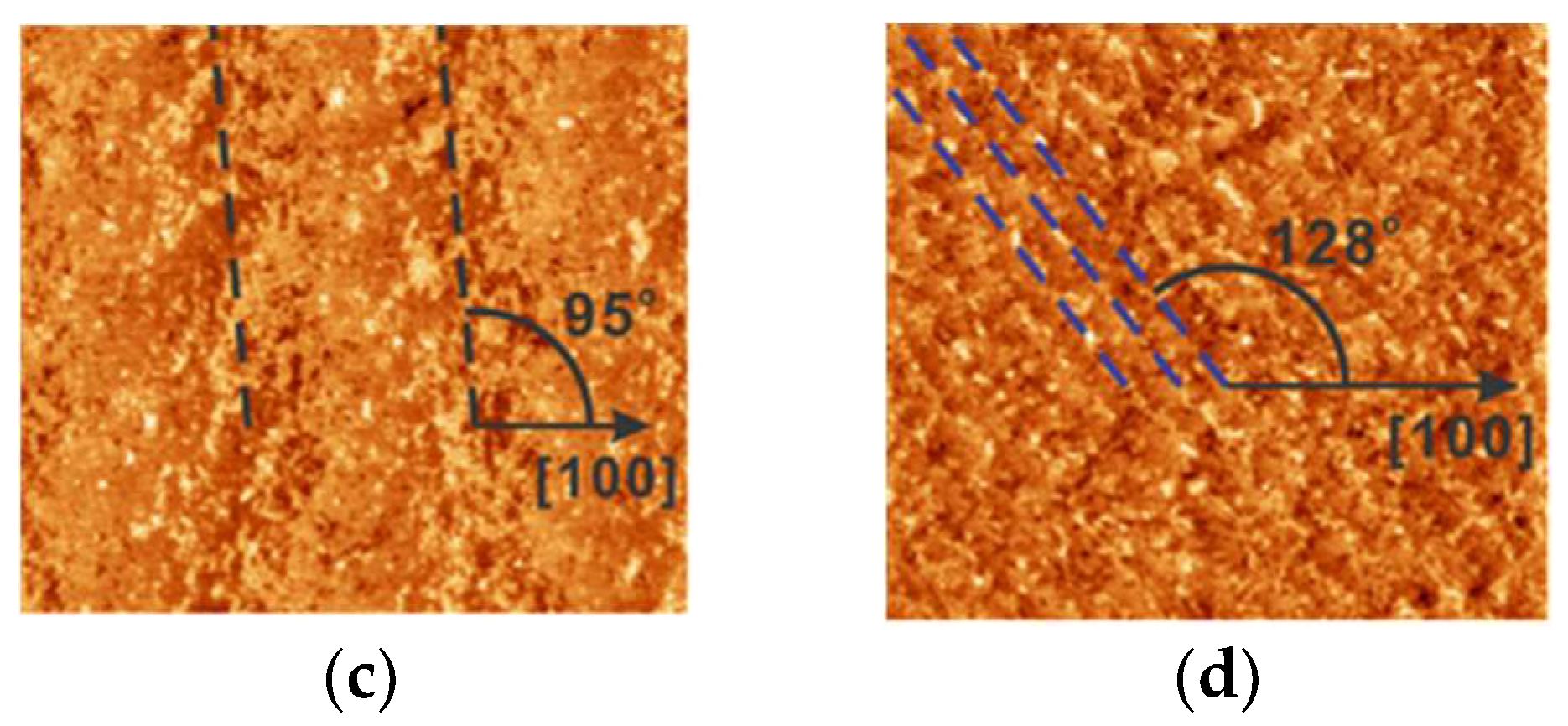
- does not depend on the miscut angle and orientation. We have investigated in detail whether there is a correlation between the step orientation and the uniaxial anisotropy in the electronic transport, i.e., the direction in which the maximum of occurs. Since the miscut angle varies randomly from one substrate to another, the same is true of the density and orientation of the surface steps. However, the nematicity amplitude and director orientation are reproducible in the films with the same doping level, while they vary systematically with doping and temperature. This is illustrated in Figure 8, where we present AFM images of three LSAO substrates, each showing clear atomic steps. The postgrowth AFM scans of the LSCO films deposited on these LSAO substrates confirmed that the steps were carried over into the films to the surface. The densities and orientations of atomic steps vary significantly from one to another of these three films (Figure 8b–d). Yet, both the amplitude and the phase of the angular oscillations of remain nearly the same (Figure 8a).
- is the same in films and bulk. We also observed nematicity in bulk single crystals of LSCO, with the amplitude essentially the same as in LSCO films doped to the same level. Our data agree with those obtained independently by other groups [6,7]. These millimeter-thick bulk LSCO crystals have neither misfit nor antiphase dislocations, and the atomic steps at the surface have an unmeasurably small effect on the transport properties. Altogether, the observed in both LSCO films and bulk crystals cannot originate from surface steps.
3.5. Thermal Gradient
3.6. Thickness Gradient
- Our ALL-MBE system is equipped with a scanning quartz-crystal oscillator monitor, which enables the measurement of the local deposition rates across the substrate [9]. If we use a single thermal effusion cell, the deposition rate varies linearly across the substrate, and the gradient is 4% per 1 cm. However, we compensate for this gradient by using a pair of identical sources placed symmetrically (at azimuth angles that differ by 180°). The resulting gradient is thus much less than 1% over 1 cm, so the variations across a single 100 µm wide Hall bar are negligible.
- We digitally control the film thickness by monitoring the intensity of the Reflection High-Energy Electron Diffraction (RHEED). By scanning the RHEED beam across the film surface, we can verify that the thickness is uniform. Moreover, we can also see that the RHEED pattern and the atomic termination of the film surface are the same across the entire wafer.
- The geometry of the synthesis system determines the variations in film thickness. These should reproduce from one film to another, while we see that has a strong and systematic doping dependence.
- Moreover, strongly depends on temperature, and so does the orientation of the nematic director. That rules out any purely geometric origin.
- Within this scenario, the measured would always be proportional to the measured , at every temperature, the azimuth angle , doping, etc., which is at variance with the experimental facts.
3.7. Composition Gradient
- Since the geometry of our MBE system fixes our source positions and orientations with respect to the substrate, such gradients would not change from one film to another if the targeted film composition is kept the same.
- This would change with the doping level, but the doping dependence would be the opposite of what we see. The effect (if any) of such a gradient would increase with doping (it would scale with x). We see that decreases fast with doping, by a few orders of magnitude, until, on the extremely overdoped side, we hit the noise floor (see Figure 5). To throw in some numbers, if, for example, the residual gradient was 1% over 1 cm, for x = 0.20, this would amount to , while for , it would be 10 times smaller, i.e., . In contrast, the observed nematicity amplitude is, in fact, more than an order of magnitude larger in LSCO, with rather than .
- The nematicity amplitude varies strongly and systematically with the temperature, while the gradient in doping level would not depend on T.
- Moreover, had this been the culprit, the observed nematic director would always point in the same direction, while its orientation, in fact, changes substantially with the doping level and temperature.
3.8. Schottky Barrier Contacts
4. Sample Inhomogeneity
4.1. Phase Separation
- Our LSCO films are not granular. The X-ray diffraction and atomic force microscopy (AFM) studies indicate that the crystalline structure and morphology of our LSCO films are very uniform both microscopically and macroscopically. According to RHEED, high-resolution XRD, AFM, scanning cross-section transmission electron microscopy (STEM), etc., our LSCO films synthesized by ALL-MBE are single crystals, atomically smooth, and nearly perfect. This results from 30 years of sustained and focused development of the ALL-MBE technique and technology [36,37,38,39,40,41]. No static ordered phase (CDW, SDW, ferro- and antiferromagnetism) is observed in the entire doping range, while some nematicity is always present. While CDW and AF fluctuations may be present, these would average to zero with time and space and would not be macroscopically aligned.
- Our LSCO films are ‘clean’. One figure of merit, frequently used in the literature to benchmark the film quality, is the residual resistivity or, more tellingly, the residual resistivity ratio (RRR), defined as . In Figure 11, we show the dependence in a representative LSCO film that we synthesized using ALL-MBE, while the measurements were carried by an independent group (at NHMFL) [41]. It shows that , and RRR > 200. By this standard, this (single-crystal) LSCO film is more perfect and less disordered, by far, than any bulk LSCO single-crystal reported in the literature. Suppose one uses the standard Drude formula and inserts the measured and broadly accepted values for the Fermi velocity, effective mass, and carrier density; the inferred mean free path would be longer than 1.7 µm, i.e., more than 4000 lattice constants. This should be considered exceptionally clean within the accepted standards and terminology [42]. An alternative is to postulate a breakdown of the canonical description of metals and superconductors. Nevertheless, this film shows a substantial nonzero transverse resistivity already at room temperature and down to Tc. It also features a pronounced peak near , while no such peak exists in the longitudinal resistivity. Moreover, changes the sign four times as the in-plane angle varies [1]. Therefore, is proportional neither to nor to the derivative
- is linear in T. In a clean unconventional superconductor with a V-shaped d-wave gap, the dependence of the superfluid density on temperature is expected to be linear. Scattering on any disorder, impurities, and other defects would cause the breaking of near-nodal pairs, filling in the gap and rounding it near the node, and leading to the dependence of below some characteristic temperature T*. We have shown that in LSCO, is linear for all doping levels, in some films down to 1 K. This is only expected in a very clean d-wave superconductor [29].
- anddependences are systematic. The strong, smooth, and reproducible dependences of the nematicity amplitude and orientation on and , impose very tight constraints on any model. It is difficult to account for all of these within any inhomogeneity scenario. Handwaving is not sufficient—one ought to present a concrete model of inhomogeneity that can at least qualitatively reproduce the dependence of coincident with the dependence of at fixed T and doping; the decrease in with x; the rotation of the nematic director with T, B, and x; the disappearance of the peak in with B [43], etc.
4.2. Guided Vortex Motion
- In one device, and for one pair of voltage contacts, a scratch, a microcrack, or local inhomogeneity in oxygen distribution could redirect the current locally, thus generating a transverse voltage. It is almost impossible to believe, though, that this could, just by chance, happen at all 108 positions measured and in such a way to create a curve in every film and for every doping.
- Even if the guided vortex motion was relevant for explaining the pronounced peak in near Tc, such as in Figure 2b, it could not be at the origin of the electronic nematicity we observe at room temperature, where no superconducting vortices are present.
- The hypothesis of guided vortices is also incompatible with our angle-resolved transverse magnetoresistance (ARTMR) data [43]. If the moving vortices are preferentially driven in a particular direction—by a gradient in the distribution of dopant ions or some other defects, step edges and antiphase dislocations due to substrate miscut, etc.—one could expect some anisotropic transverse voltage because of broken C4 symmetry, and this could peak near Tc. However, as more vortices are generated, this effect should grow stronger with the magnetic field. Moreover, one would also expect to see the same effect in the longitudinal resistivity. This is the opposite of what we observe. First, the peak near Tc in decreases by an order of magnitude in the field B = 6 T. Second, there is no peak (and almost no MR whatsoever) in at the same temperature and field. Third, if the sample were effectively orthorhombic due to some compositional or structural reasons, one would expect this to change neither with the magnetic field nor with the temperature, while the nematicity magnitude and director orientation show a strong and systematic T- and B-dependence [43].
4.3. Randomness of Sr Doping
- This randomness averages out on the relevant (µm, mm) length scales. The average Sr–Sr distance is smaller than 1 nm, and since the distribution of Sr atoms is random, local variations are expected on a very small (nm) scale. However, this randomness should be averaged out already on the scale of, say, 100 nm. It should be thoroughly washed out on the scale of the width of a single Hall bar, 100 µm, and even more so on the scale of the sunbeam device illustrated in Figure 1a, which is larger than 0.5 cm.
- Sr clustering is not seen by structural probes. In one proposed model, the density of Sr atoms is assumed to vary locally, forming domains or clusters. However, this hypothesis can be refuted based on the arguments listed in Section 4.1, which rule out phase separation and granularity of whatever origin. Sr clusters have been observed neither by nanoscale synchrotron-based techniques such as X-ray fluorescence nor by TEM-based high-resolution electron-energy-loss spectroscopy (HR-EELS).
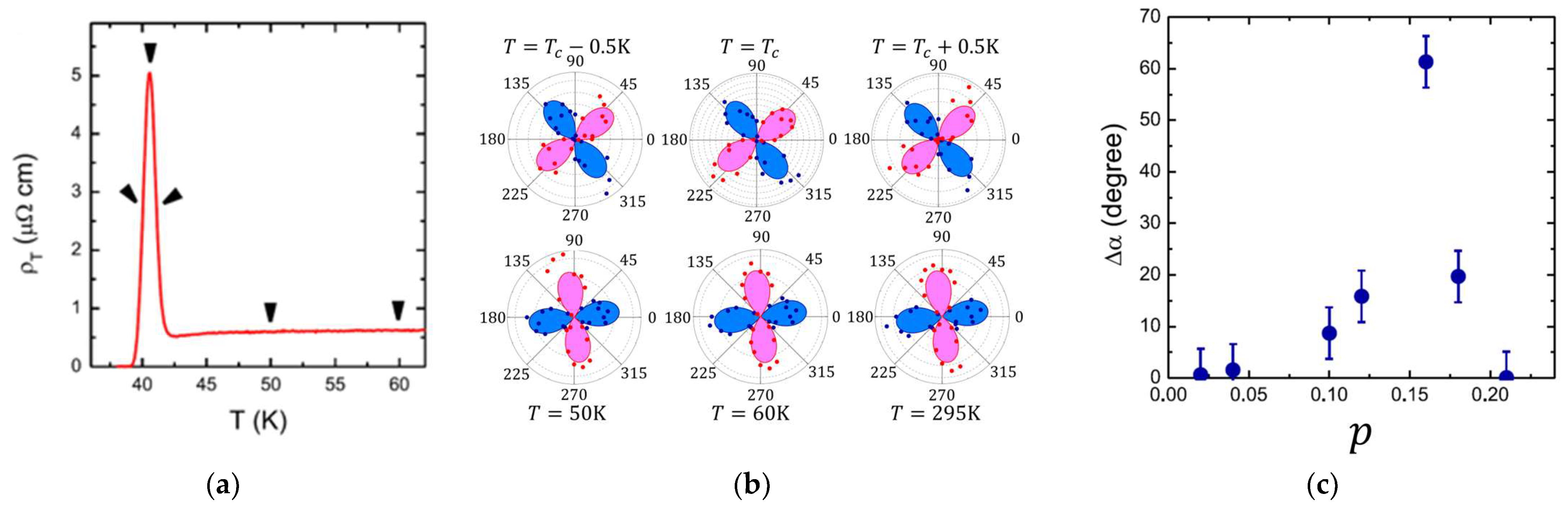
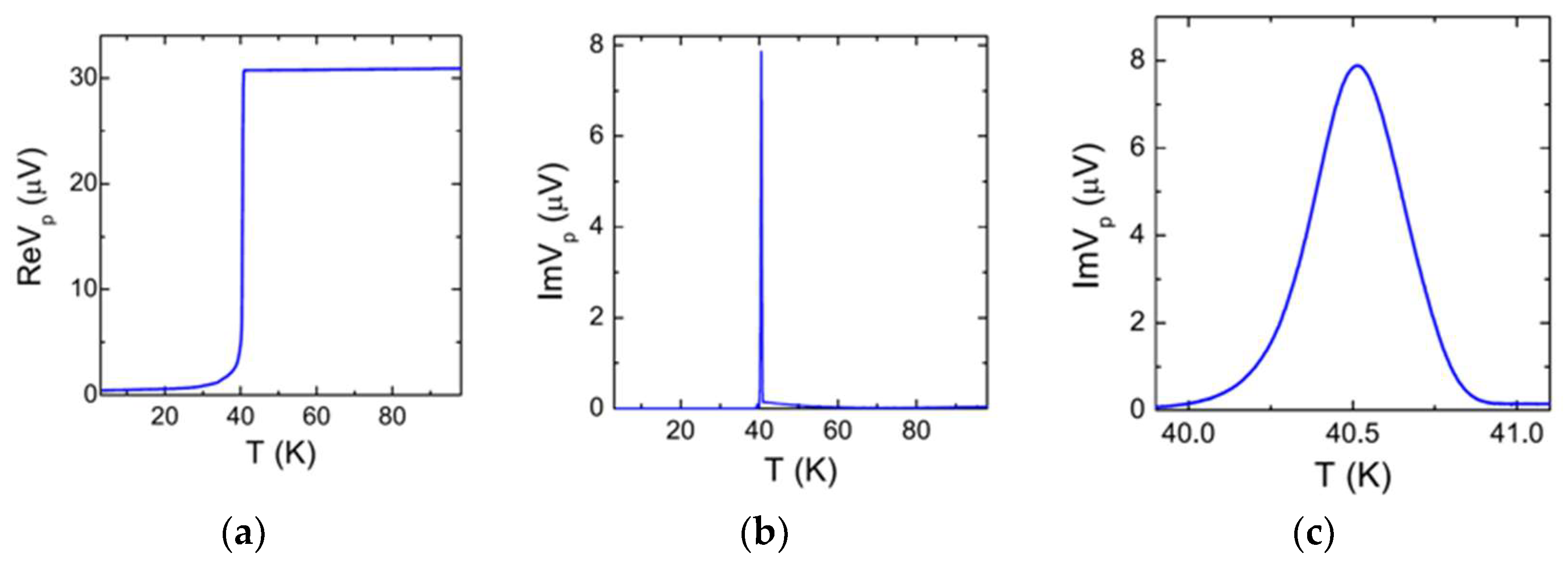
- Sr clustering is inconsistent with the transport and susceptibility data. In Figure 12, we show the two-coil mutual inductance data for an optimally doped (x = 0.16) LSCO film. The in-phase signal measures the reactive response and shows the Meissner effect with a sharp onset at Tc = 40.8 K. The half-width-at-half-maximum of the peak in the out-of-phase (dissipative) response puts an upper limit on the variations of Tc in this film, , over a large area of 10 × 10 mm2. Using the empirically known relations that link , and x, one can estimate the corresponding upper limit on the gross local variations in . For , and assuming the worst-case scenario when the variation is in the form of a gradient along one line, this would result in measured —at least two orders of magnitude smaller than the anisotropy we measure near Tc. This rules out attribution to a substantial inhomogeneity in the film of the observed phenomena, including, in particular, the peak in VT near Tc, which is typically 1–2 orders of magnitude broader.
- Sr clustering is inconsistent with thedependence. The probability that sufficiently large domains, with a large enough difference in stoichiometry, could be present in our films is quite small. However, let us grossly overestimate it as P = 10% for one device. What, then, is the probability that this happens in each of the 108 devices we fabricate out of one film, and that this happens in such a way as to generate the dependence on the azimuth angle, with the shift between and , as shown in Figure 3. Essentially, it is null. Moreover, inhomogeneity would have to be organized on a macroscopic scale in such a way that (a) the film shows a preferred transport direction, (b) when the film is ‘sliced’ into 108 small devices, this remains the same in each device, in both magnitude and orientation, (c) this reproduces in other films of the same doping, and (d) as we change the doping level, this “organized inhomogeneity” would need to have a smooth and monotonic doping dependence. More is different here; our extensive statistics allow for definitive statements and rule out the dominant role of such random agents.
- Sr clustering is inconsistent with thedependence. The systematic temperature dependence, particularly the director rotation, is the ultimate challenge to the Sr-clustering model and any other scenario based on random or organized chemical or structural disorder. The Sr distribution does not change with the temperature.
4.4. Tc Distribution
- Not at room temperature. This mechanism only works near Tc, where R(T) drops sharply. It does not work at room T, where R(T) evolves gradually. Even if the observed broadening of the resistive transition originated entirely from the disorder and inhomogeneity—which, as we argue in detail in (vi) below, is decidedly not the case—it is unclear how this could account for the observed nematicity at room temperature.
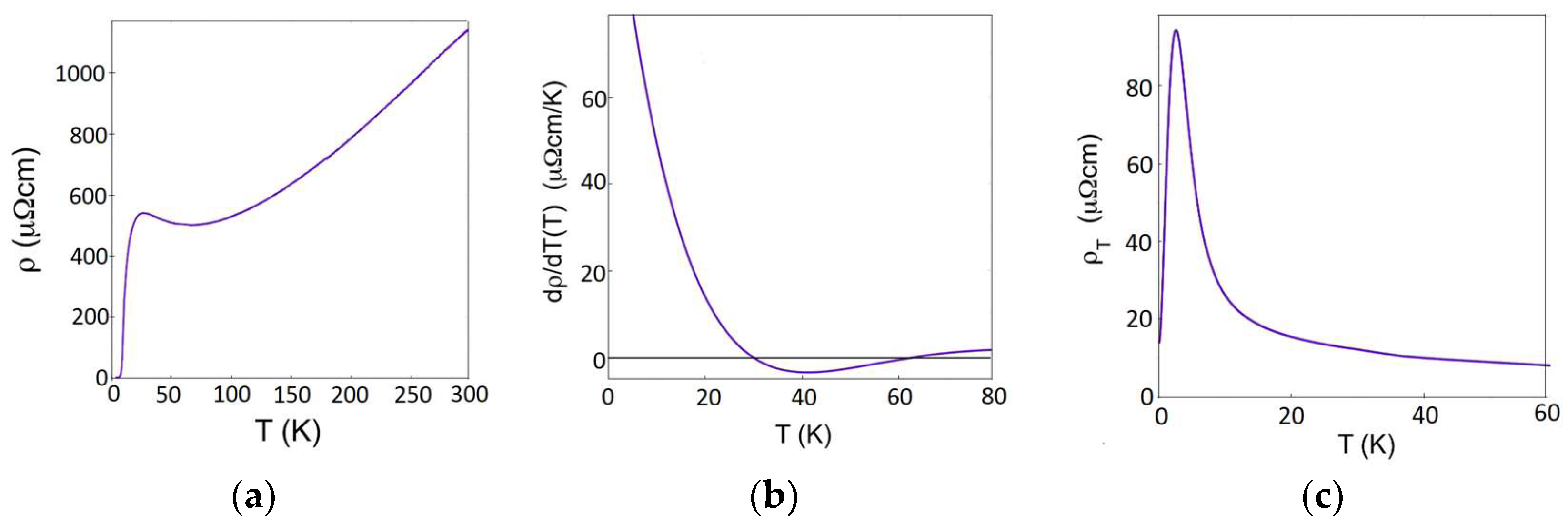
- Not for x ≲ 0.07. As shown in Figure 13, in LSCO with x = 0.063, the dependence is not monotonous. As T decreases, decreases (like in metal) but then flattens and starts to increase (like in a semiconductor) until it starts dropping fast again in the region near Tc where superconducting fluctuations take over. Hence, the derivative changes sign twice with temperature. In contrast, always stays positive. For , LSCO is neither superconducting nor metallic; is semiconductor-like, and is negative in the entire range . In contrast, is always positive in this temperature interval.
- Not for. As we mentioned in Section 1.5 (ii) above, , and, hence, for . On the other hand, , so for , , while for , . Since neither nor is zero anywhere above Tc, clearly, .
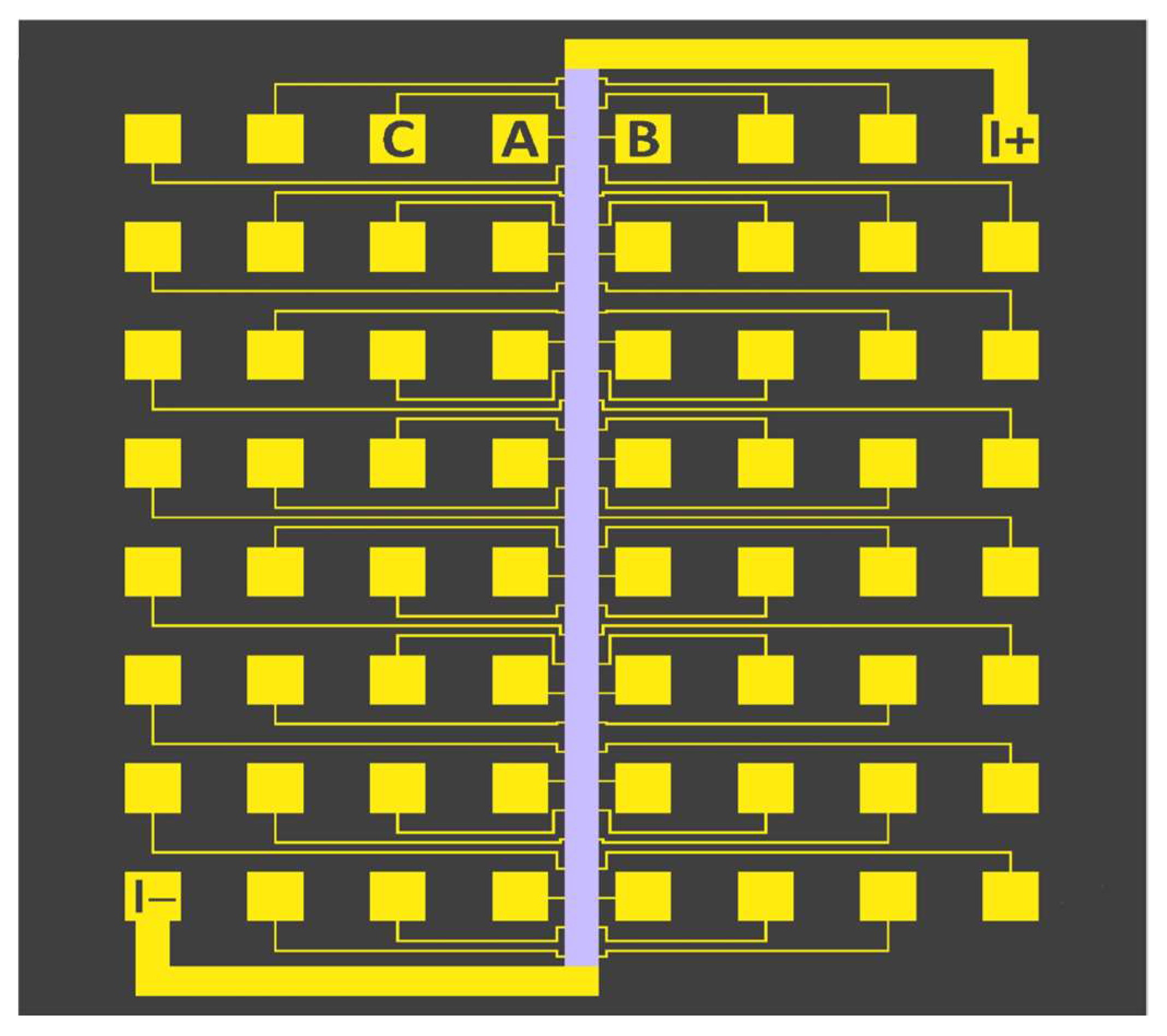
- Not random; systematic x-dependence. Here, inhomogeneity means the randomness of some structural and electronic features at different locations in the film. If this was the dominant source of VT and if we systematically measure at various locations along a strip that is patterned in the film, the recorded should either vary randomly with the position, if the domains are relatively large, or be constant (i.e., independent on the position) if the domains are much smaller than the strip width, and thus, averaged out. To test this possibility, we synthesized an LSCO film with a built-in continuous gradient in doping level x by means of the combinatorial molecular beam epitaxy (COMBE) [30,36]. Then, we used lithography to pattern this film, as shown in Figure 10a. This pattern, with 2 current contacts and 31 pairs of voltage contacts, allows us to measure and at 30 positions in the LSCO film with a continuous doping gradient. We built the corresponding electronics that allow us to measure all 30 channels simultaneously, thus reducing the scatter due to possible variations in temperature, etc. As seen in Figure 10b, the measured ratio shows a linear dependence on p; clearly, it is not random at different locations in the film.
- Broadening of the resistive transition in cuprates does not arise from disorder. In Section 4.3 (iv) above, we showed that in our LSCO films, the spread in Tc, as measured by the mutual inductance technique, can be as small as ±0.1 K over the 10 × 10 mm2 area. On the other hand, in LSCO (and all other superconducting cuprates), the resistive transitions are quite broad [42], typically, DTc ≈ 5–10 K. The actual value is somewhat vague since various definitions of Tc are possible and indeed have been used in the literature, e.g., the transition onset TO; the temperature at which the resistivity drops to 90%, 50%, or 10% of the normal-state value just above the onset, etc. However, all of these suffer from ambiguity in the choice of the normal-state resistivity. It is less subjective to use the temperature TM at which the slope of is the largest; this can be determined unambiguously as the temperature at which the derivative curve, , reaches the maximum. The most conservative choice, however, and the one we always adhere to, is to use the temperature at which the sample resistivity vanishes, i.e., Tc ≡ T(R = 0). We found (with extensive statistics) that this is also the temperature at which the Meissner effect onsets. Using this definition, we find that Tc (as determined by high-precision mutual inductance measurements) is quite sharp. In Figure 14, we show a set of characteristics measured in one LSCO film patterned into a linear combinatorial library of devices [30]. For each device, we indicated the transition onset TO, the temperature T1 at which the resistivity drops to 90%, T2 where it drops to 50%, T3 at which it drops to 10%, and TM at which the derivative has the maximum. It is apparent from Figure 14b that, as long as we consistently use any of these characteristic temperatures, it stays essentially constant, on the scale of 0.1 K, in all devices across the entire film.
- Broadening of the transition in cuprates arises from superconducting phase fluctuations. The resolution of this apparent ‘paradox’—the simultaneous occurrence of a very sharp Meissner signal and a broad resistive transition—is well-known [42,48,49,50,51,52].
- Cuprates are extreme type-II superconductors with the ratio , where is the London penetration depth and the coherence length. Therefore, one indeed expects, from the Ginzburg–Levanyuk criterion, that there should be a few Kelvin-wide temperature regions in which superconducting fluctuations dominate the transport. As the temperature is increased, vortex–antivortex pairs are generated and thermally dissociated. The probe current causes free vortices to move, and vortex flow causes dissipation and finite resistance [42].
- In LSCO, at every doping level, the superfluid density is very low, i.e., a few orders of magnitude lower than in conventional superconductors such as Nb or Pb. Expressed in the units of Kelvin, the phase stiffness is nearly equal to Tc [29]. This fact implies that thermal phase fluctuations essentially determine the superconducting transition by destroying the global phase coherence [52].
- Cuprates are quasi-two-dimensional—they behave like vertical stacks of intrinsic Josephson junctions. A single LSCO layer can host HTS with Tc, with the superfluid density equal to that in the bulk samples [29]. Hence, in cuprates, one should expect to see Berezinskii–Kosterlitz–Thouless (BKT)-like physics. Indeed, dynamic BKT transition has been observed by MHz susceptibility measurements [48] and microwave [49] and THz spectroscopies [50,51].
- Almost every type of experiment that could detect thermal phase fluctuations in principle has shown that in cuprates, they persist in a vast temperature region. Both ARPES and scanning tunneling microscopy (STM) data show that the superconducting gap does not close at Tc but persists well above, up to as much as 1.5 Tc. Microwave and THz spectroscopies show superconducting phase fluctuations up to 20–30 K above Tc [49,50,51]. Magnetoresistance data show superconducting fluctuations well above the critical field that causes nonzero resistance. In underdoped LSCO, evidence for superconducting vortices at temperatures as high as 250 K has been found in the Nernst effect, diamagnetism, torque magnetometry, etc.
5. Control Experiments Using Ti Films
6. Nematicity beyond ARTR in LSCO
6.1. Other Cuprates
6.2. Bulk Crystals
6.3. Other Techniques
- Some techniques, such as scanning tunneling microscopy (STM) and angle-resolved photoemission spectroscopy (ARPES), are very surface-sensitive. Then, one needs to worry whether all the structural and electronic properties of the surface layer (that is probed) are the same as the bulk (that is not probed by ARPES or STM but is probed by transport or magnetic measurements to determine Tc). In fact, in most ionic crystals, it is guaranteed that the topmost surface layer will not have the bulk structure because of the long-range (Madelung) origin of the cohesion [31,54]. Here, BSCCO may be an exception because of the weak Van der Waals coupling between the layers (which also makes it cleavable).
- Most of these other techniques have background-related problems since one is looking for small angle-dependent deviations from some relatively large average value. Then, it is the question of how big these deviations are compared to the error bars and sensitivity of the measurement for one orientation.
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wu, J.; Bollinger, A.T.; He, X.; Božović, I. Spontaneous breaking of rotational symmetry in copper oxide superconductors. Nature 2017, 547, 432–435. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Bollinger, A.T.; He, X.; Božović, I. Detecting Electronic Nematicity by the Angle-Resolved Transverse Resistivity Measurements. J. Supercond. Nov. Magn. 2019, 32, 1623–1628. [Google Scholar] [CrossRef]
- Zaanen, J.; Gunnarsson, O. Charged magnetic domain lines and the magnetism of high-Tc oxides. Phys. Rev. B 1989, 40, 7391–7394. [Google Scholar] [CrossRef] [PubMed]
- Chandra, P.; Coleman, P.; Larkin, A.I. Ising transition in frustrated Heisenberg models. Phys. Rev. Lett. 1990, 64, 88–91. [Google Scholar] [CrossRef]
- Zaanen, J.; Oleś, A.M. Striped phase in the cuprates as a semiclassical phenomenon. Ann. Phys. 1996, 508, 224–246. [Google Scholar] [CrossRef]
- Kivelson, S.A.; Fradkin, E.; Emery, V.J. Electronic liquid-crystal phases of a doped Mott insulator. Nature 1998, 393, 550–553. [Google Scholar] [CrossRef]
- Oganesyan, V.; Kivelson, S.A.; Fradkin, E. Quantum theory of a nematic Fermi fluid. Phys. Rev. B 2001, 64, 195109. [Google Scholar] [CrossRef]
- Fradkin, E.; Kivelson, S.A.; Lawler, M.J.; Eisenstein, J.P.; Mackenzie, A.P. Nematic Fermi Fluids in Condensed Matter Physics. Ann. Rev. Cond. Mat. Phys. 2010, 1, 153–178. [Google Scholar] [CrossRef]
- Carlson, E.W.; Dahmen, K.A. Using disorder to detect locally ordered electron nematics via hysteresis. Nat. Commun. 2001, 2, 379. [Google Scholar] [CrossRef]
- Phillabaum, B.; Carlson, E.W.; Dahmen, K.A. Spatial complexity due to bulk electronic nematicity in a superconducting underdoped cuprate. Nat. Commun. 2011, 3, 915. [Google Scholar] [CrossRef]
- Fernandes, R.M.; Chubukov, A.V.; Schmalian, J. What drives nematic order in iron-based superconductors? Nat. Phys. 2014, 10, 97–104. [Google Scholar] [CrossRef]
- Beekman, A.J.; Nissinen, J.; Wu, K.; Liu, K.; Slager, R.-J.; Nussinov, Z.; Cvetkovic, V.; Zaanen, J. Dual gauge field theory of quantum liquid crystals in two dimensions. Phys. Rep. 2017, 683, 1–110. [Google Scholar] [CrossRef]
- Hinkov, V.; Haug, D.; Fauqué, B.; Bourges, P.; Sidis, Y.; Ivanov, A.; Bernhard, C.; Lin, C.T.; Keimer, B. Electronic Liquid Crystal State in the High-Temperature Superconductor YBa2Cu3O6.45. Science 2008, 319, 597–600. [Google Scholar] [CrossRef]
- Lawler, M.J.; Fujita, K.; Lee, J.; Schmidt, A.R.; Kohsaka, Y.; Kim, C.K.; Eisaki, H.; Uchida, S.; Davis, J.C.; Sethna, J.P.; et al. Intra-unit-cell electronic nematicity of the high-Tc copper-oxide pseudogap states. Nature 2010, 466, 347–351. [Google Scholar] [CrossRef] [PubMed]
- Daou, R.; Chang, J.; LeBoeuf, D.; Cyr-Choinière, O.; Laliberté, F.; Doiron-Leyraud, N.; Ramshaw, B.J.; Liang, R.; Bonn, D.A.; Hardy, W.N.; et al. Broken rotational symmetry in the pseudogap phase of a high-Tc superconductor. Nature 2010, 463, 519–522. [Google Scholar] [CrossRef]
- Mesaros, A.; Fujita, K.; Eisaki, H.; Uchida, S.; Davis, J.C.; Sachdev, S.; Zaanen, J.; Lawler, M.J.; Kim, E.-A. Topological Defects Coupling Smectic Modulations to Intra–Unit-Cell Nematicity in Cuprates. Science 2011, 333, 426–430. [Google Scholar] [CrossRef]
- Li, L.; Alidoust, N.; Tranquada, J.M.; Gu, G.D.; Ong, N.P. Unusual Nernst Effect Suggesting Time-Reversal Violation in the Striped Cuprate Superconductor La2-xBaxCuO4. Phys. Rev. Lett. 2011, 107, 277001. [Google Scholar] [CrossRef]
- Fujita, K.; Kim, C.K.; Lee, I.; Lee, J.; Hamidian, M.H.; Firmo, I.A.; Mukhopadhyay, S.; Eisaki, H.; Uchida, S.; Lawler, M.J.; et al. Simultaneous Transitions in Cuprate Momentum-Space Topology and Electronic Symmetry Breaking. Science 2014, 344, 612–616. [Google Scholar] [CrossRef]
- Lubashevsky, Y.; Pan, L.; Kirzhner, T.; Koren, G.; Armitage, N.P. Optical Birefringence and Dichroism of Cuprate Superconductors in the THz Regime. Phys. Rev. Lett. 2014, 112, 147001. [Google Scholar] [CrossRef]
- Cyr-Choinière, O.; Grissonnanche, G.; Badoux, S.; Day, J.; Bonn, D.A.; Hardy, W.N.; Liang, R.; Doiron-Leyraud, N.; Taillefer, L. Two types of nematicity in the phase diagram of the cuprate superconductor YBa2Cu3Oy. Phys. Rev. B 2015, 92, 224502. [Google Scholar] [CrossRef]
- Zhang, J.; Levenson-Falk, E.M.; Ramshaw, B.J.; Bonn, D.A.; Liang, R.; Hardy, W.N.; Hartnoll, S.A.; Kapitulnik, A. Anomalous thermal diffusivity in underdoped YBa2Cu3O6+x. Proc. Natl. Acad. Sci. USA 2017, 114, 5378–5383. [Google Scholar] [CrossRef] [PubMed]
- Chu, J.-H.; Analytis, J.G.; De Greve, K.; McMahon, P.L.; Islam, Z.; Yamamoto, Y.; Fisher, I.R. In-Plane Resistivity Anisotropy in an Underdoped Iron Arsenide Superconductor. Science 2010, 329, 824–826. [Google Scholar] [CrossRef] [PubMed]
- Chu, J.-H.; Kuo, H.-H.; Analytis, J.G.; Fisher, I.R. Divergent Nematic Susceptibility in an Iron Arsenide Superconductor. Science 2012, 337, 710–712. [Google Scholar] [CrossRef] [PubMed]
- Worasaran, T.; Ikeda, M.S.; Palmstrom, J.C.; Straquadine, J.A.W.; Kivelson, S.A.; Fisher, I.R. Nematic quantum criticality in an Fe-based superconductor revealed by strain-tuning. Science 2021, 372, 973–977. [Google Scholar] [CrossRef]
- Kuo, H.-H.; Chu, J.-H.; Palmstrom, J.C.; Kivelson, S.A.; Fisher, I.R. Ubiquitous signatures of nematic quantum criticality in optimally doped Fe-based superconductors. Science 2016, 352, 958–962. [Google Scholar] [CrossRef]
- Wu, J.; Nair, H.P.; Bollinger, A.T.; He, X.; Robinson, I.; Schreiber, N.J.; Shen, K.M.; Schlom, D.G.; Božović, I. Electronic nematicity in Sr2RuO4. Proc. Natl. Acad. Sci. USA 2020, 117, 10654–10659. [Google Scholar] [CrossRef]
- Cao, Y.; Rodan-Legrain, D.; Park, J.M.; Yuan, N.F.Q.; Watanabe, K.; Taniguchi, T.; Fernandes, R.M.; Fu, L.; Jarillo-Herrero, P. Nematicity and competing orders in superconducting magic-angle graphene. Science 2021, 371, 264–271. [Google Scholar] [CrossRef]
- Rubio-Verdú, C.; Turkel, S.; Song, Y.; Klebl, L.; Samajdar, R.; Scheurer, M.S.; Venderbos, J.W.F.; Watanabe, K.; Taniguchi, T.; Ochoa, H.; et al. Moiré nematic phase in twisted double bilayer graphene. Nat. Phys. 2022, 18, 196–202. [Google Scholar] [CrossRef]
- Božović, I.; He, X.; Wu, J.; Bollinger, A.T. Dependence of the critical temperature in overdoped copper oxides on superfluid density. Nature 2016, 536, 309–311. [Google Scholar] [CrossRef]
- Wu, J.; Pelleg, O.; Logvenov, G.; Bollinger, A.T.; Sun, Y.-J.; Boebinger, G.S.; Vanević, M.; Radović, Z.; Božović, I. Anomalous independence of interface superconductivity from carrier density. Nat. Mater. 2013, 12, 877–881. [Google Scholar] [CrossRef]
- Butko, V.Y.; Logvenov, G.; Božović, N.; Radović, Z.; Božović, I. Madelung Strain in Cuprate Superconductors—A Route to Enhancement of the Critical Temperature. Adv. Mater. 2009, 21, 3644–3648. [Google Scholar] [CrossRef]
- Bollinger, A.T.; Wu, Z.-B.; Wu, L.; He, X.; Drozdov, I.; Wu, J.; Robinson, I.; Božović, I. Strain and Electronic Nematicity in La2-xSrxCuO4. J. Supercond. Nov. Magn. 2020, 33, 93–98. [Google Scholar] [CrossRef]
- Ando, Y.; Segawa, K.; Komiya, S.; Lavrov, A.N. Electrical Resistivity Anisotropy from Self-Organized One-Dimensionality in High-Temperature Superconductors. Phys. Rev. Lett. 2002, 88, 137005. [Google Scholar] [CrossRef] [PubMed]
- Ando, Y.; Lavrov, A.N.; Komiya, S. Anisotropic Magnetoresistance in Lightly Doped La2-xSrxCuO4: Impact of Antiphase Domain Boundaries on the Electron Transport. Phys. Rev. Lett. 2003, 90, 247003. [Google Scholar] [CrossRef] [PubMed]
- Dubuis, G.; He, X.; Božović, I. Sub-millikelvin stabilization of a closed cycle cryocooler. Rev. Sci. Instr. 2014, 85, 103902. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Bollinger, A.T.; Sun, Y.-J.; Božović, I. Hall effect in quantum critical charge-cluster glass. Proc. Natl. Acad. Sci. USA 2016, 113, 4284–4289. [Google Scholar] [CrossRef]
- Božović, I. Atomic-Layer Engineering of Superconducting Oxides: Yesterday, Today, Tomorrow. IEEE Trans. Appl. Supercond. 2001, 11, 2686–2695. [Google Scholar] [CrossRef]
- Gozar, A.; Logvenov, G.; Fitting Kourkoutis, L.; Bollinger, A.T.; Giannuzzi, L.A.; Muller, D.A.; Bozovic, I. High-temperature interface superconductivity between a metal and a Mott insulator. Nature 2008, 455, 782–785. [Google Scholar] [CrossRef]
- Logvenov, G.; Gozar, A.; Božović, I. High-Temperature Superconductivity in a Single Copper-Oxygen Plane. Science 2009, 326, 699–702. [Google Scholar] [CrossRef]
- Gasparov, V.; Božović, I. Magnetic field and temperature dependence of complex conductance of ultrathin La1.65Sr0.45CuO4/La2CuO4 films. Phys. Rev. B 2012, 86, 094523. [Google Scholar] [CrossRef]
- Giraldo-Gallo, P.; Galvis, J.A.; Stegen, Z.; Modic, K.A.; Balakirev, F.F.; Betts, J.B.; Lian, X.; Moir, C.; Riggs, S.C.; Wu, J.; et al. Scale-invariant magnetoresistance in a cuprate superconductor. Science 2018, 361, 479–481. [Google Scholar] [CrossRef] [PubMed]
- Tinkham, M. Introduction to Superconductivity, 2nd ed.; McGraw-Hill Book Co.: New York, NY, USA, 1996. [Google Scholar]
- Wårdh, J.; Granath, M.; Wu, J.; Bollinger, A.T.; He, X.; Božović, I. Colossal transverse magnetoresistance due to nematic superconducting phase fluctuations in a copper oxide. arXiv 2022, arXiv:2203.06769. [Google Scholar] [CrossRef]
- Vašek, P.; Janeček, I.; Plechaček, V. Intrinsic pinning and guided motion of vortices in high-Tc superconductors. Phys. C 1995, 247, 381–384. [Google Scholar] [CrossRef]
- Da Luz, M.S.; de Carvalho, F.J.H., Jr.; dos Santos, C.A.M.; Shigue, C.Y.; Machado, A.J.S.; Ricardo da Silva, R. Observation of asymmetric transverse voltage in granular high-Tc superconductors. Phys. C 2005, 419, 71–78. [Google Scholar] [CrossRef]
- Francavilla, T.L.; Cukauskas, E.J.; Allen, L.H.; Broussard, P.R. Observation of a transverse voltage in the mixed state of YBCO thin films. IEEE Trans. Appl. Supercond. 1995, 5, 1717–1720. [Google Scholar] [CrossRef]
- Segal, A.; Karpovski, M.; Gerber, A. Inhomogeneity and transverse voltage in superconductors. Phys. Rev. B 2011, 83, 094531. [Google Scholar] [CrossRef]
- Larkin, A.; Varlamov, A. Theory of Fluctuations in Superconductors; Oxford University Press: Oxford, UK, 2005. [Google Scholar] [CrossRef]
- Grbić, M.S.; Požek, M.; Paar, D.; Hinkov, V.; Raichle, M.; Haug, D.; Keimer, B.; Barišić, N.; Dulčić, A. Temperature range of superconducting fluctuations above Tc in YBa2CuO7−δ single crystals. Phys. Rev. B 2011, 83, 144508. [Google Scholar] [CrossRef]
- Corson, J.; Mallozzi, R.; Orenstein, J.; Eckstein, J.N.; Božović, I. Vanishing of phase coherence in underdoped Bi2Sr2CaCu2O8+δ. Nature 1999, 398, 221–223. [Google Scholar] [CrossRef]
- Bilbro, L.S.; Valdés Aguilar, R.; Logvenov, G.; Pelleg, O.; Božović, I.; Armitage, N.P. Temporal correlations of superconductivity above the transition temperature in La2-xSrxCuO4 probed by terahertz spectroscopy. Nat. Phys. 2011, 7, 298–302. [Google Scholar] [CrossRef]
- Emery, V.; Kivelson, S.A. Importance of phase fluctuations in superconductors with small superfluid density. Nature 1995, 374, 434–437. [Google Scholar] [CrossRef]
- Hammond, R.H.; Bormann, R. Correlation between the in situ growth conditions of YBCO thin films and the thermodynamic stability criteria. Phys. C 1989, 162–164, 703–704. [Google Scholar] [CrossRef]
- Zhou, H.; Yacoby, Y.; Butko, V.Y.; Logvenov, G.; Božović, I.; Pindak, R. Anomalous expansion of the copper-apical oxygen distance in superconducting cuprate bilayers. Proc. Natl. Acad. Sci. USA 2010, 107, 8103–8107. [Google Scholar] [CrossRef] [PubMed]
- Timusk, T.; Statt, B. The pseudogap in high-temperature superconductors: An experimental survey. Rep. Prog. Phys. 1999, 62, 61–122. [Google Scholar] [CrossRef]
- Norman, M.R.; Pines, D.; Kallin, C. The pseudogap: Friend or foe of high Tc? Adv. Phys. 2005, 54, 715–733. [Google Scholar] [CrossRef]
- Kanigel, A.; Norman, M.R.; Randeria, M.; Chatterjee, U.; Souma, S.; Kaminski, A.; Fretwell, H.M.; Rosenkranz, S.; Shi, M.; Sato, T.; et al. Evolution of the pseudogap from Fermi arcs to the nodal liquid. Nat. Phys. 2006, 2, 447–451. [Google Scholar] [CrossRef]
- Hüfner, S.; Hossain, M.A.; Damascelli, A.; Sawatzky, G.A. Two gaps make a high-temperature superconductor? Rep. Prog. Phys. 2008, 71, 062501. [Google Scholar] [CrossRef]
- Hashimoto, M.; Vishik, I.M.; He, R.-H.; Devereaux, T.P.; Shen, Z.-X. Energy gaps in high-transition-temperature cuprate superconductors. Nat. Phys. 2014, 10, 483–495. [Google Scholar] [CrossRef]
- Badoux, S.; Tabis, W.; Laliberté, F.; Grissonnanche, G.; Vignolle, B.; Vignolles, D.; Béard, J.; Bonn, D.A.; Hardy, W.N.; Liang, R.; et al. Change of carrier density at the pseudogap critical point of a cuprate superconductor. Nature 2016, 531, 210–214. [Google Scholar] [CrossRef]
- Vishik, I.M. Photoemission perspective on pseudogap, superconducting fluctuations, and charge order in cuprates: A review of recent progress. Rep. Prog. Phys. 2018, 81, 062501. [Google Scholar] [CrossRef]
- Mukhin, S. Euclidean Q-Balls of Fluctuating SDW/CDW in the ‘Nested’ Hubbard Model of High-Tc Superconductors as the Origin of Pseudogap and Superconducting Behaviors. Condens. Matter 2022, 7, 31. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Božović, I.; He, X.; Bollinger, A.T.; Caruso, R. Is Nematicity in Cuprates Real? Condens. Matter 2023, 8, 7. https://doi.org/10.3390/condmat8010007
Božović I, He X, Bollinger AT, Caruso R. Is Nematicity in Cuprates Real? Condensed Matter. 2023; 8(1):7. https://doi.org/10.3390/condmat8010007
Chicago/Turabian StyleBožović, Ivan, Xi He, Anthony T. Bollinger, and Roberta Caruso. 2023. "Is Nematicity in Cuprates Real?" Condensed Matter 8, no. 1: 7. https://doi.org/10.3390/condmat8010007
APA StyleBožović, I., He, X., Bollinger, A. T., & Caruso, R. (2023). Is Nematicity in Cuprates Real? Condensed Matter, 8(1), 7. https://doi.org/10.3390/condmat8010007








