Nonlinear Excitations in Ultracold Atoms Trapped in Triple Optical Lattices
Abstract
:1. Introduction
2. Solitary Wave Solution Under the Novel TOL Traps
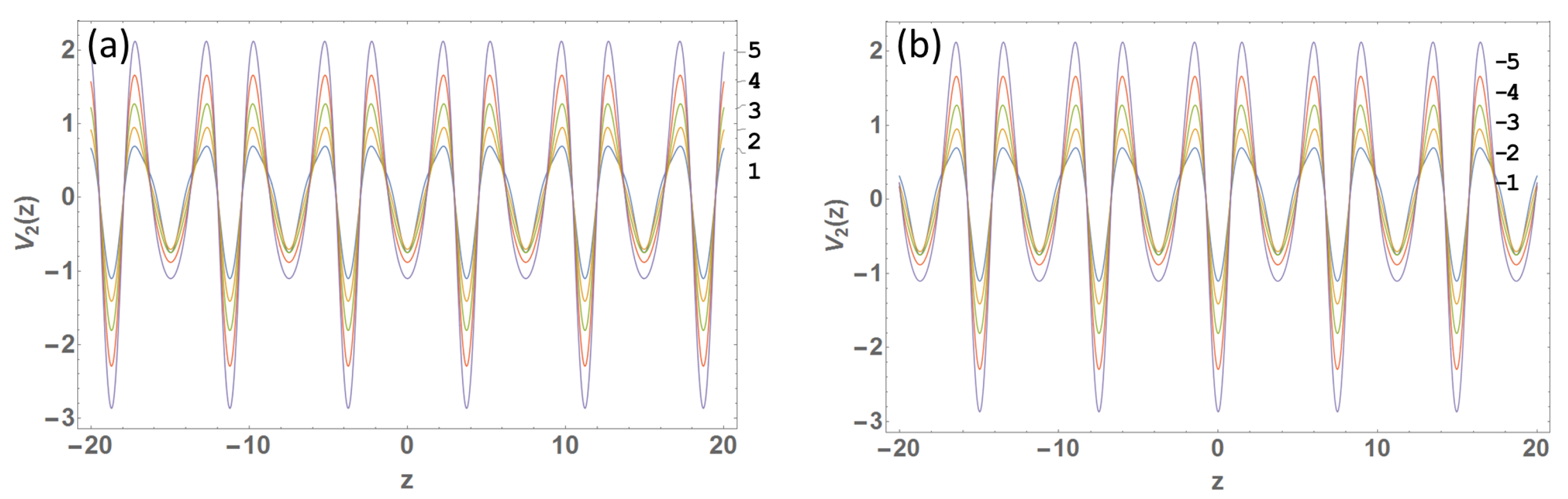
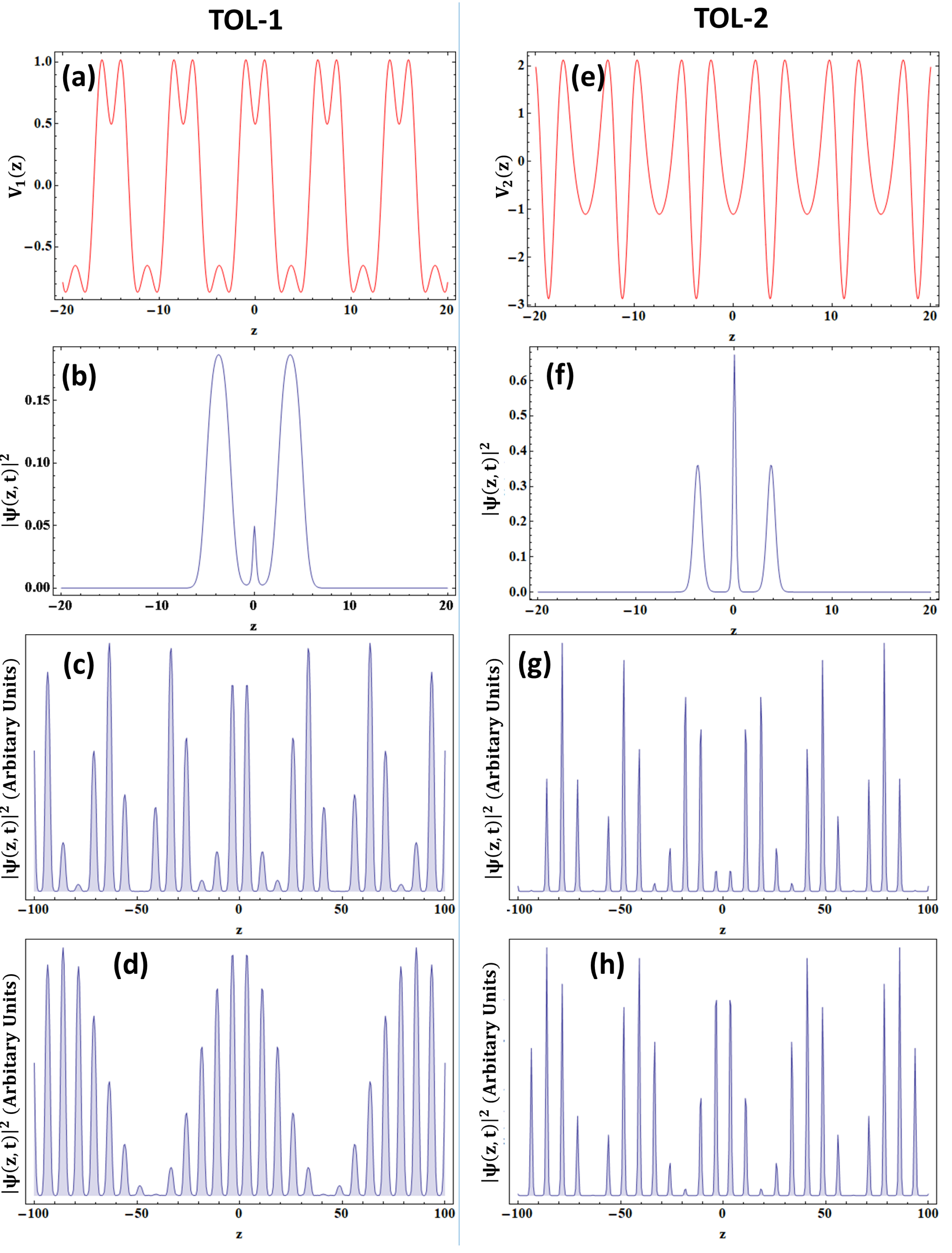
3. Various Trap Configurations and Matter-Wave Density
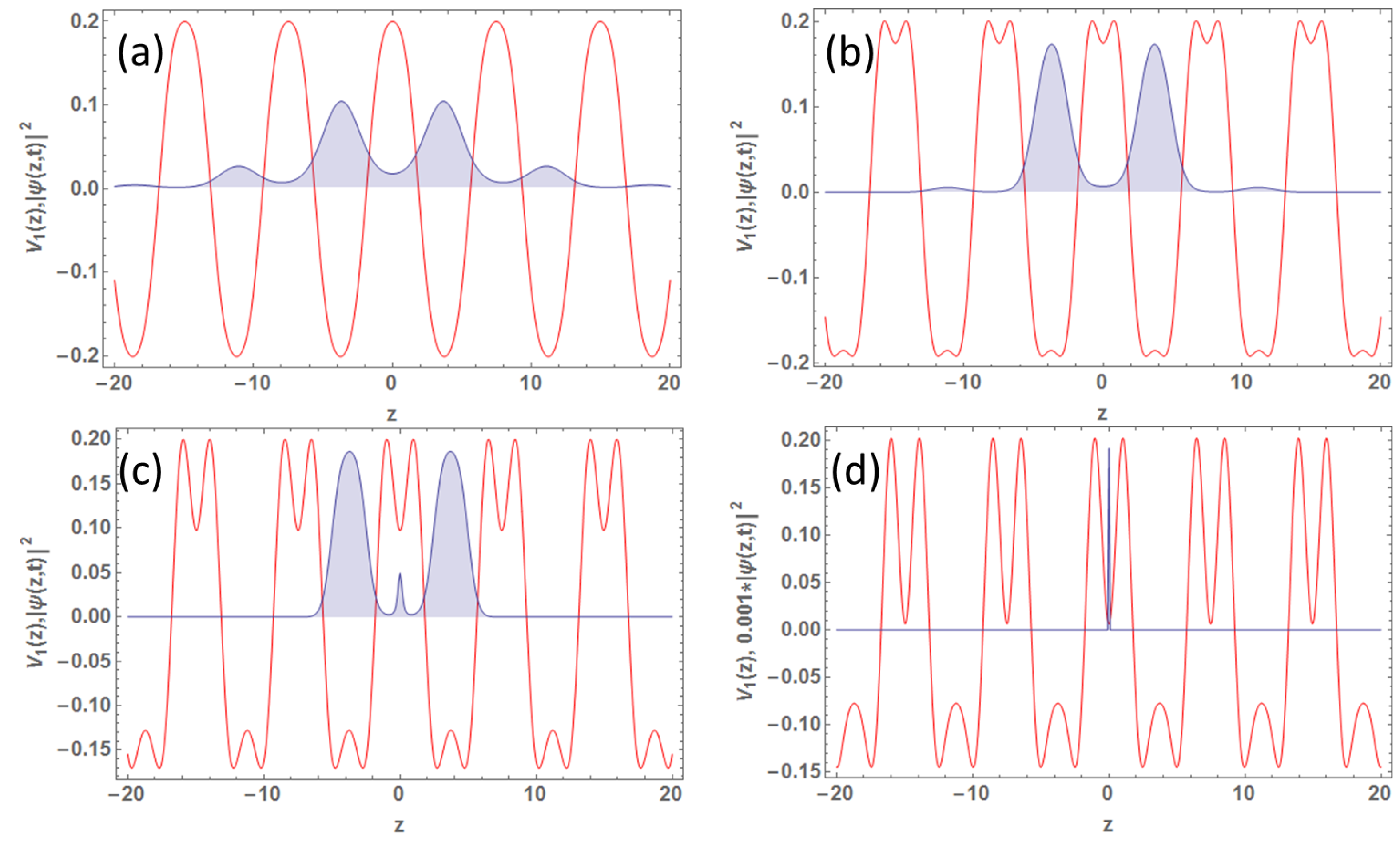
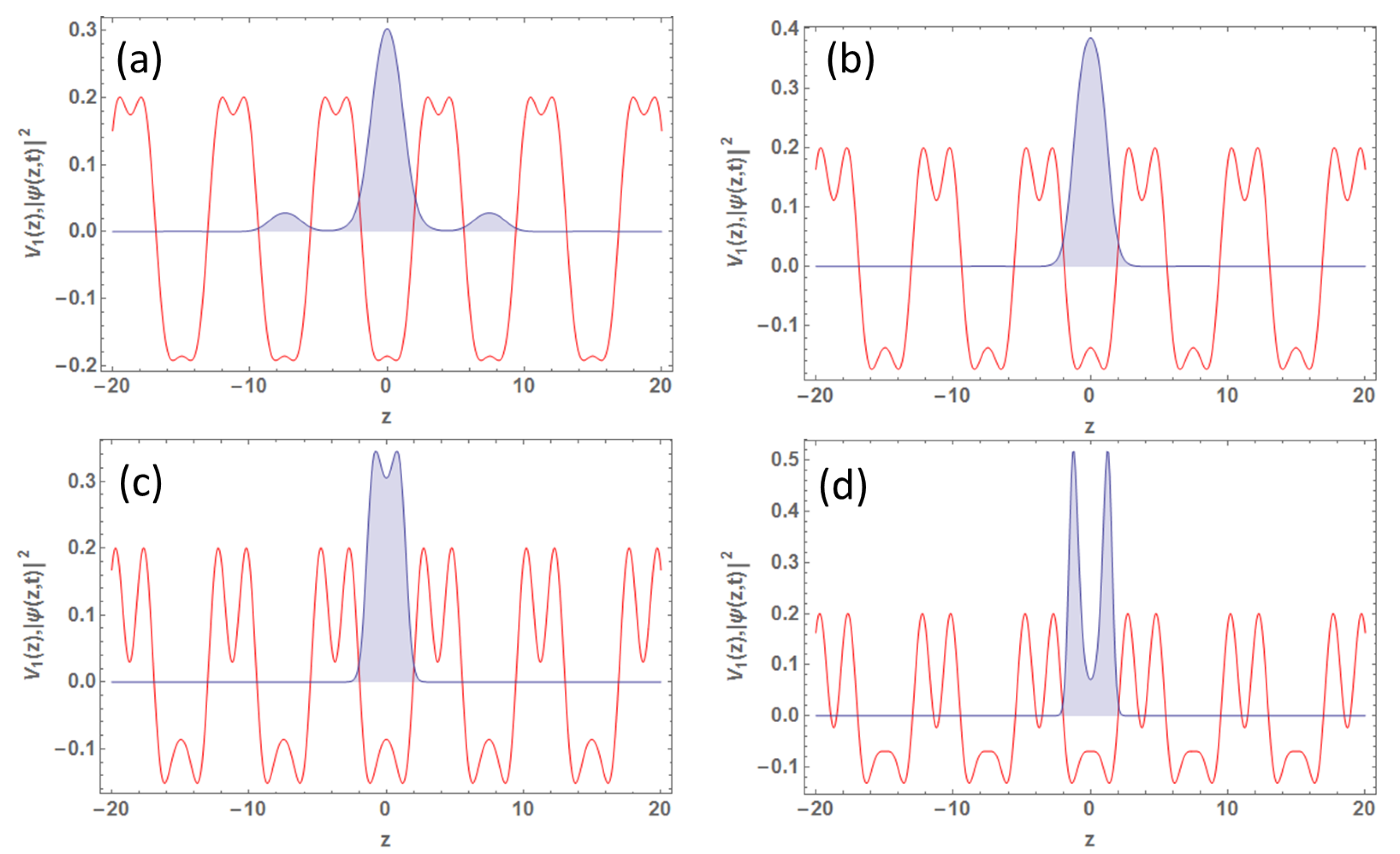
3.1. Localized Condensate Density for TOL-1
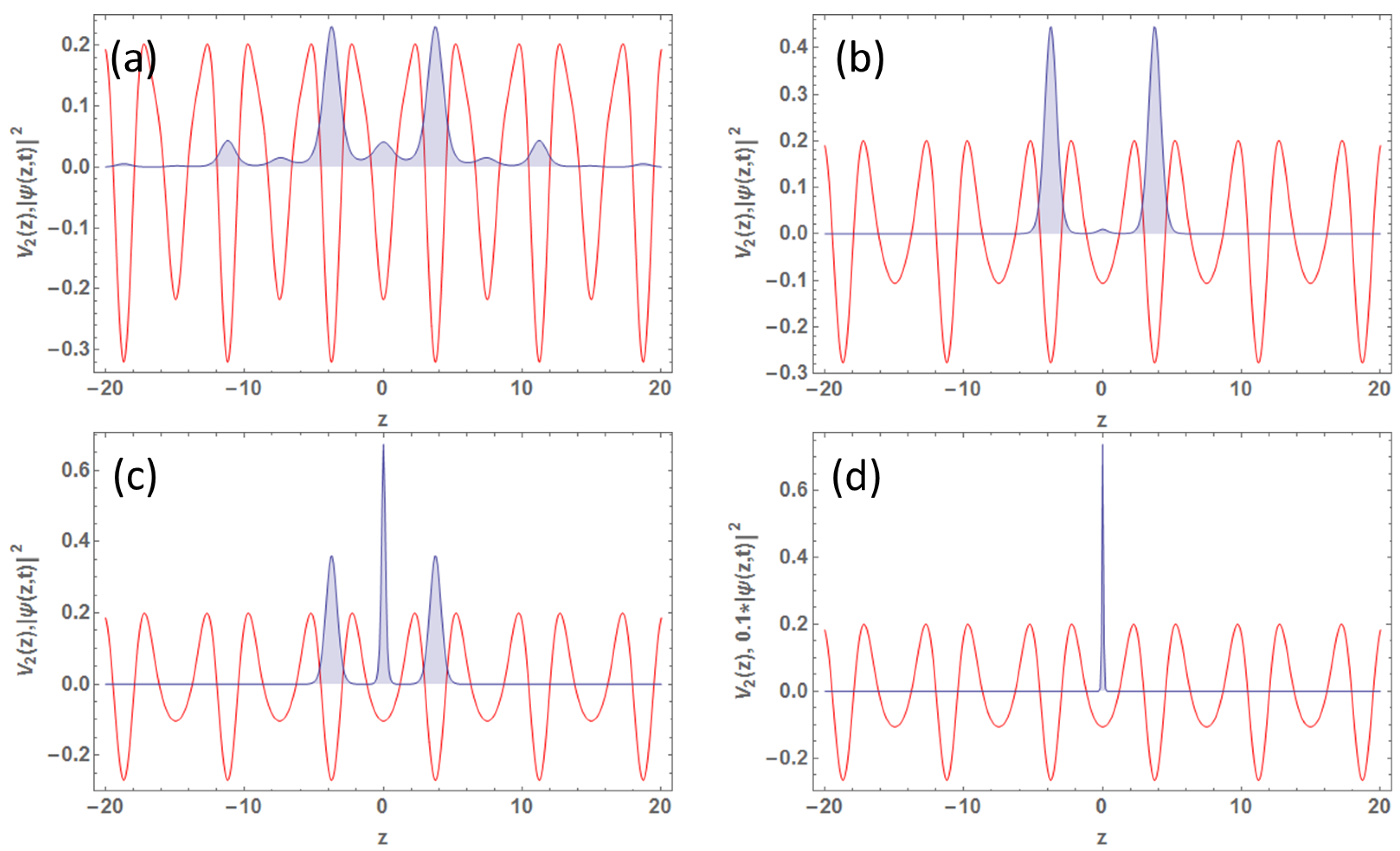
3.2. Localized Condensate Density for TOL-2
3.3. Periodic and Cnoidal Matter-Wave Excitations
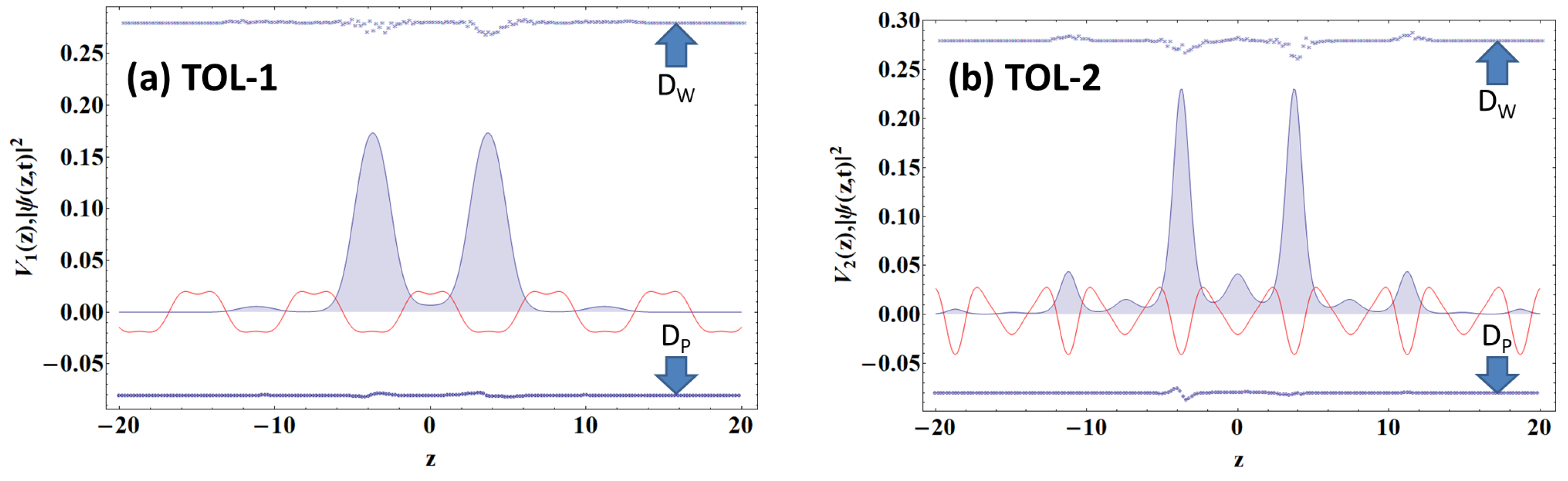
4. Dynamical Stability and Structural Stability
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pethick, C.J.; Smith, H. Bose–Einstein Condensation in Dilute Gases; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Pitaevskii, L.; Stringari, S. Bose-Einstein Condensation; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Nath, A.; Roy, U. A unified model for an external trap in a cigar-shaped Bose–Einstein condensate. J. Phys. A Math. Theor. 2014, 47, 415301. [Google Scholar] [CrossRef]
- Salasnich, L.; Adhikari, S.K. Dimensional reduction and localization of a Bose-Einstein condensate in a quasi-1D bichromatic optical lattice. Acta Phys. Pol. A 2015, 128, 979. [Google Scholar] [CrossRef]
- Khaykovich, L.; Schreck, F.; Ferrari, G.; Bourdel, T.; Cubizolles, J.; Carr, L.D.; Castin, Y.; Salomon, C. Formation of a matter-wave bright soliton. Science 2002, 296, 1290–1293. [Google Scholar] [CrossRef] [PubMed]
- Schulte, T.; Drenkelforth, S.; Kruse, J.; Ertmer, W.; Arlt, J.; Sacha, K.; Zakrzewski, J.; Lewenstein, M. Routes towards Anderson-like localization of Bose-Einstein condensates in disordered optical lattices. Phys. Rev. Lett. 2005, 95, 170411. [Google Scholar] [CrossRef] [PubMed]
- Billy, J.; Josse, V.; Zuo, Z.; Bernard, A.; Hambrecht, B.; Lugan, P.; Clément, D.; Sanchez-Palencia, L.; Bouyer, P.; Aspect, A. Direct observation of Anderson localization of matter waves in a controlled disorder. Nature 2008, 453, 891–894. [Google Scholar] [CrossRef]
- Nath, A.; Bera, J.; Ghosh, S.; Roy, U. exact Analytical Model for Bose-einstein condensate at negative temperature. Sci. Rep. 2020, 10, 9016. [Google Scholar] [CrossRef]
- Kundu, N.; Nath, A.; Bera, J.; Ghosh, S.; Roy, U. Synergy between the Negative Absolute Temperature and the External Trap for a Bose-Einstein Condensate under Optical Lattices. Phys. Lett. A 2022, 427, 127922. [Google Scholar] [CrossRef]
- Yamamoto, D.; Fukuhara, T.; Danshita, I. Frustrated quantum magnetism with Bose gases in triangular optical lattices at negative absolute temperatures. Commun. Phys. 2020, 3, 56. [Google Scholar] [CrossRef]
- D’Errico, C.; Burchianti, A.; Prevedelli, M.; Salasnich, L.; Ancilotto, F.; Modugno, M.; Minardi, F.; Fort, C. Observation of quantum droplets in a heteronuclear bosonic mixture. Phys. Rev. Res. 2019, 1, 033155. [Google Scholar] [CrossRef]
- Perali, A.; Pieri, P.; Pisani, L.; Strinati, G.C. BCS-BEC crossover at finite temperature for superfluid trapped Fermi atoms. Phys. Rev. Lett. 2004, 92, 220404. [Google Scholar] [CrossRef] [Green Version]
- Chen, Q.; Stajic, J.; Tan, S.; Levin, K. BCS–BEC crossover: From high temperature superconductors to ultracold superfluids. Phys. Rep. 2005, 412, 1–88. [Google Scholar] [CrossRef]
- Zwerger, W. The BCS-BEC Crossover and the Unitary Fermi Gas; Springer: Berlin/Heidelberg, Germany, 2011; Volume 836. [Google Scholar]
- Cronin, A.D.; Schmiedmayer, J.; Pritchard, D.E. Optics and interferometry with atoms and molecules. Rev. Mod. Phys. 2009, 81, 1051. [Google Scholar] [CrossRef]
- Billam, T.; Cornish, S.; Gardiner, S. Realizing bright-matter-wave-soliton collisions with controlled relative phase. Phys. Rev. A 2011, 83, 041602. [Google Scholar] [CrossRef]
- Struck, J.; Ölschläger, C.; Le Targat, R.; Soltan-Panahi, P.; Eckardt, A.; Lewenstein, M.; Windpassinger, P.; Sengstock, K. Quantum simulation of frustrated classical magnetism in triangular optical lattices. Science 2011, 333, 996–999. [Google Scholar] [CrossRef]
- Balents, L. Spin liquids in frustrated magnets. Nature 2010, 464, 199–208. [Google Scholar] [CrossRef]
- Yan, S.; Huse, D.A.; White, S.R. Spin-liquid ground state of the S= 1/2 kagome Heisenberg antiferromagnet. Science 2011, 332, 1173–1176. [Google Scholar] [CrossRef]
- Qiu, X.; Zou, J.; Qi, X.; Li, X. Precise programmable quantum simulations with optical lattices. Npj Quantum Inf. 2020, 6, 87. [Google Scholar] [CrossRef]
- Windpassinger, P.; Sengstock, K. Engineering novel optical lattices. Rep. Prog. Phys. 2013, 76, 086401. [Google Scholar] [CrossRef]
- Kundu, N.; Ghosh, S.; Roy, U. Quantum Simulation of Rogue Waves in Bose-Einstein Condensate: An Exact Analytical Method. Phys. Lett. A 2022, 128335. [Google Scholar] [CrossRef]
- Argüello-Luengo, J.; González-Tudela, A.; Shi, T.; Zoller, P.; Cirac, J.I. Quantum simulation of two-dimensional quantum chemistry in optical lattices. Phys. Rev. Res. 2020, 2, 042013. [Google Scholar] [CrossRef]
- Arlinghaus, S.; Holthaus, M. Controlled wave-packet manipulation with driven optical lattices. Phys. Rev. A 2011, 84, 063617. [Google Scholar] [CrossRef]
- Covey, J.P.; Moses, S.A.; Gärttner, M.; Safavi-Naini, A.; Miecnikowski, M.T.; Fu, Z.; Schachenmayer, J.; Julienne, P.S.; Rey, A.M.; Jin, D.S.; et al. Doublon dynamics and polar molecule production in an optical lattice. Nat. Commun. 2016, 7, 11279. [Google Scholar] [CrossRef] [PubMed]
- Casert, C.; Mills, K.; Vieijra, T.; Ryckebusch, J.; Tamblyn, I. Optical lattice experiments at unobserved conditions with generative adversarial deep learning. Phys. Rev. Res. 2021, 3, 033267. [Google Scholar] [CrossRef]
- Homid, A.; Abdel-Aty, M.; Qasymeh, M.; Eleuch, H. Efficient quantum gates and algorithms in an engineered optical lattice. Sci. Rep. 2021, 11, 15402. [Google Scholar] [CrossRef]
- Katori, H. Optical lattice clocks and quantum metrology. Nat. Photon. 2011, 5, 203–210. [Google Scholar] [CrossRef]
- Ghosh, S.; Bera, J.; Panigrahi, P.K.; Roy, U. Sub-fourier quantum metrology through bright solitary trains in Bose–Einstein condensate. Int. J. Quantum Inf. 2019, 17, 1950019. [Google Scholar] [CrossRef]
- Cameron, A.R.; Cheng, S.W.; Schwarz, S.; Kapahi, C.; Sarenac, D.; Grabowecky, M.; Cory, D.G.; Jennewein, T.; Pushin, D.A.; Resch, K.J. Remote state preparation of single-photon orbital-angular-momentum lattices. Phys. Rev. A 2021, 104, L051701. [Google Scholar] [CrossRef]
- Simon, D.S. Quantum sensors: Improved optical measurement via specialized quantum states. J. Sens. 2016, 2016, 6051286. [Google Scholar] [CrossRef]
- Yang, B.; Sun, H.; Huang, C.J.; Wang, H.Y.; Deng, Y.; Dai, H.N.; Yuan, Z.S.; Pan, J.W. Cooling and entangling ultracold atoms in optical lattices. Science 2020, 369, 550–553. [Google Scholar] [CrossRef]
- Howard, L.; Weinhold, T.; Shahandeh, F.; Combes, J.; Vanner, M.; White, A.; Ringbauer, M. Quantum hypercube states. Phys. Rev. Lett. 2019, 123, 020402. [Google Scholar] [CrossRef] [Green Version]
- Shukla, N.; Akhtar, N.; Sanders, B.C. Quantum tetrachotomous states: Superposition of four coherent states on a line in phase space. Phys. Rev. A 2019, 99, 063813. [Google Scholar] [CrossRef]
- Shukla, N.; Nimmrichter, S.; Sanders, B.C. Squeezed comb states. Phys. Rev. A 2021, 103, 012408. [Google Scholar] [CrossRef]
- Roy, U.; Atre, R.; Sudheesh, C.; Kumar, C.N.; Panigrahi, P.K. Complex solitons in Bose–Einstein condensates with two-and three-body interactions. J. Phys. B At. Mol. Opt. 2010, 43, 025003. [Google Scholar] [CrossRef]
- Serkin, V.N.; Hasegawa, A. Novel soliton solutions of the nonlinear Schrödinger equation model. Phys. Rev. Lett. 2000, 85, 4502. [Google Scholar] [CrossRef] [PubMed]
- Liang, Z.; Zhang, Z.; Liu, W. Dynamics of a bright soliton in Bose-Einstein condensates with time-dependent atomic scattering length in an expulsive parabolic potential. Phys. Rev. Lett. 2005, 94, 050402. [Google Scholar] [CrossRef]
- Belmonte-Beitia, J.; Pérez-García, V.M.; Vekslerchik, V.; Torres, P.J. Lie symmetries and solitons in nonlinear systems with spatially inhomogeneous nonlinearities. Phys. Rev. Lett. 2007, 98, 064102. [Google Scholar] [CrossRef]
- Yan, Z.; Jiang, D. Matter-wave solutions in Bose-Einstein condensates with harmonic and Gaussian potentials. Phys. Rev. E 2012, 85, 056608. [Google Scholar] [CrossRef]
- Bludov, Y.V.; Yan, Z.; Konotop, V. Dynamics of inhomogeneous condensates in contact with a surface. Phys. Rev. A 2010, 81, 063610. [Google Scholar] [CrossRef]
- Bronski, J.C.; Carr, L.D.; Deconinck, B.; Kutz, J.N. Bose-Einstein condensates in standing waves: The cubic nonlinear Schrödinger equation with a periodic potential. Phys. Rev. Lett. 2001, 86, 1402. [Google Scholar] [CrossRef] [PubMed]
- Adhikari, S.; Salasnich, L. Localization of a Bose-Einstein condensate in a bichromatic optical lattice. Phys. Rev. A 2009, 80, 023606. [Google Scholar] [CrossRef] [Green Version]
- Nath, A.; Roy, U. Bose–Einstein condensate in a bichromatic optical lattice: An exact analytical model. Laser Phys. Lett. 2014, 11, 115501. [Google Scholar] [CrossRef]
- Halder, B.; Ghosh, S.; Basu, P.; Bera, J.; Malomed, B.; Roy, U. Exact Solutions for Solitary Waves in a Bose-Einstein Condensate under the Action of a Four-Color Optical Lattice. Symmetry 2021, 14, 49. [Google Scholar] [CrossRef]
- Dell’Anna, L.; Mazzarella, G.; Penna, V.; Salasnich, L. Entanglement entropy and macroscopic quantum states with dipolar bosons in a triple-well potential. Phys. Rev. A 2013, 87, 053620. [Google Scholar] [CrossRef]
- Witthaut, D.; Trimborn, F.; Hennig, H.; Kordas, G.; Geisel, T.; Wimberger, S. Beyond mean-field dynamics in open Bose-Hubbard chains. Phys. Rev. A 2011, 83, 063608. [Google Scholar] [CrossRef]
- Dutta, S.; Tsatsos, M.C.; Basu, S.; Lode, A.U. Management of the correlations of UltracoldBosons in triple wells. New J. Phys. 2019, 21, 053044. [Google Scholar] [CrossRef]
- Kengne, E.; Liu, W.M.; Malomed, B.A. Spatiotemporal engineering of matter-wave solitons in Bose–Einstein condensates. Phys. Rep. 2021, 899, 1–62. [Google Scholar] [CrossRef]
- Salasnich, L.; Parola, A.; Reatto, L. Effective wave equations for the dynamics of cigar-shaped and disk-shaped Bose condensates. Phys. Rev. A 2002, 65, 043614. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; US Government Printing Office: Washington, DC, USA, 1964; Volume 55.
- Martone, G.I.; Recati, A.; Pavloff, N. Supersolidity of cnoidal waves in an ultracold Bose gas. Phys. Rev. Res. 2021, 3, 013143. [Google Scholar] [CrossRef]
- Nath, A.; Bera, J.; Ghosh, S.; Panigrahi, P.K.; Roy, U. Soliton dynamics for an ingenious trap combination in a Bose-Einstein condensate. Eur. Phys. J. D 2020, 74, 27. [Google Scholar] [CrossRef]
- Bera, J.; Ghosh, S.; Salasnich, L.; Roy, U. Matter-wave fractional revivals in a ring waveguide. Phys. Rev. A 2020, 102, 063323. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Basu, P.; Halder, B.; Raghav, S.; Roy, U. Nonlinear Excitations in Ultracold Atoms Trapped in Triple Optical Lattices. Condens. Matter 2022, 7, 52. https://doi.org/10.3390/condmat7030052
Basu P, Halder B, Raghav S, Roy U. Nonlinear Excitations in Ultracold Atoms Trapped in Triple Optical Lattices. Condensed Matter. 2022; 7(3):52. https://doi.org/10.3390/condmat7030052
Chicago/Turabian StyleBasu, Pradosh, Barun Halder, Sriganapathy Raghav, and Utpal Roy. 2022. "Nonlinear Excitations in Ultracold Atoms Trapped in Triple Optical Lattices" Condensed Matter 7, no. 3: 52. https://doi.org/10.3390/condmat7030052
APA StyleBasu, P., Halder, B., Raghav, S., & Roy, U. (2022). Nonlinear Excitations in Ultracold Atoms Trapped in Triple Optical Lattices. Condensed Matter, 7(3), 52. https://doi.org/10.3390/condmat7030052






