Phase Separation and Pairing Fluctuations in Oxide Materials
Abstract
1. Introduction
2. Phase Separation in the Mean-Field Approximation
3. Phase Separation and Fluctuations
4. Pair Correlations In Cuprates
4.1. Pairing from Long Range Electron-Phonon Interaction
4.2. Pair Fluctuations in the Symmetry-Broken Ground State
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bednorz, J.G.; Müller, K.A. Possible high Tc superconductivity in the Ba-La-Cu-O system. Z. Physik B 1986, 64, 189. [Google Scholar] [CrossRef]
- Hizhnyakov, V.; Sigmund, E. High-Tc superconductivity induced by ferromagnetic clustering. Phys. C 1988, 156, 655. [Google Scholar] [CrossRef]
- Hizhnyakov, V.; Kristoffel, N.E.; Sigmund, E. On the percolation induced conductivity in high-Tc superconducting materials. Phys. C 1989, 160, 119. [Google Scholar] [CrossRef]
- Hizhnyakov, V.; Sigmund, E.; Schneider, M. Magnetic interactions and dynamics of holes in CuO2 planes of high-Tc superconducting materials. Phys. Rev. B 1991, 44, 795. [Google Scholar] [CrossRef] [PubMed]
- Kremer, R.; Sigmund, E.; Hizhnyakov, V.; Hentsch, F.; Simon, A.; Müller, K.A.; Mehring, M. Percolative phase separation in La2CuO4+δ and La2−xSrxCuO4. Z. Physik B 1992, 86, 319. [Google Scholar] [CrossRef]
- Kremer, R.; Hizhnyakov, V.; Sigmund, E.; Simon, A.; Müller, K.A. Electronic phase separation in La-cuprates. On the role of hole and oxygen diffusion. La2CuO4+y; La2−xSrxCuO4+y. Z. Physik B 1993, 94, 17. [Google Scholar]
- Fratini, M.; Poccia, N.; Ricci, A.; Campi, G.; Burghammer, M.; Aeppli, G.; Bianconi, A. Scale-free structural organization of oxygen interstitials in La2CuO4+y. Nature 2010, 466, 841–844. [Google Scholar] [CrossRef] [PubMed]
- Haase, J.; Slichter, C.P.; Milling, C.T. Static Charge and Spin Inhomogeneity in La2−xSrxCuO4 by NMR. J. Supercond. 2002, 15, 339. [Google Scholar] [CrossRef]
- Krämer, S.; Mehring, M. Low-Temperature Charge Ordering in the Superconducting State of YBa2Cu3O7−δ. Phys. Rev. Lett. 1999, 83, 396. [Google Scholar] [CrossRef]
- Teitel’baum, G.B.; Büchner, B.; de Gronckel, H. Cu NQR Study of the Stripe Phase Local Structure in the Lanthanum Cuprates. Phys. Rev. Lett. 2000, 84, 2949. [Google Scholar] [CrossRef]
- Singer, P.M.; Hunt, A.W.; Imai, T. 63Cu NQR Evidence for Spatial Variation of Hole Concentration in La2−xSrxCuO4. Phys. Rev. Lett. 2002, 88, 047602. [Google Scholar] [CrossRef]
- Müller, K.A.; Benedek, G. (Eds.) Proceedings of the First Workshop on Phase Separation in Cuprate Superconductors; World Scientific: Singapore, 1993. [Google Scholar]
- Sigmund, E.; Müller, K.A. (Eds.) Proceedings of the Second Workshop on Phase Separation in Cuprate Superconductors; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Emery, V.J.; Kivelson, S.A.; Lin, H.Q. Phase separation in the t-J model. Phys. Rev. Lett. 1990, 64, 475. [Google Scholar] [CrossRef] [PubMed]
- Visscher, P.B. Phase separation instability in the Hubbard model. Phys. Rev. B 1974, 10, 943. [Google Scholar] [CrossRef]
- Grilli, M.; Raimondi, R.; Castellani, C.; Castro, C.D.; Kotliar, G. Superconductivity, phase separation, and charge-transfer instability in the U=∞ limit of the three-band model of the CuO2 planes. Phys. Rev. Lett. 1991, 67, 259. [Google Scholar] [CrossRef]
- Moreo, A.; Scalapino, D.; Dagotto, E. Phase separation in the Hubbard model. Phys. Rev. B 1991, 43, 11442. [Google Scholar] [CrossRef]
- Van Dongen, P.G.J. Phase Separation in the Extended Hubbard Model at Weak Coupling. Phys. Rev. Lett. 1995, 74, 182. [Google Scholar] [CrossRef] [PubMed]
- Capone, M.; Sangiovanni, G.; Castellani, C.; Castro, C.D.; Grilli, M. Phase Separation Close to the Density-Driven Mott Transition in the Hubbard-Holstein Model. Phys. Rev. Lett. 2004, 92, 106401. [Google Scholar] [CrossRef] [PubMed]
- Sherman, A.; Schreiber, M. Fluctuating charge-density waves in the Hubbard model. Phys. Rev. B 2008, 77, 155117. [Google Scholar] [CrossRef]
- Löw, U.; Emery, V.J.; Fabricius, K.; Kiverlson, S.A. Study of an Ising model with competing long- and short-range interactions. Phys. Rev. Lett. 1994, 72, 1918. [Google Scholar] [CrossRef]
- Castellani, C.; Castro, C.D.; Grilli, M. Singular Quasiparticle Scattering in the Proximity of Charge Instabilities. Phys. Rev. Lett. 1995, 75, 4650. [Google Scholar] [CrossRef]
- Tranquada, J.M.; Sternlieb, B.J.; Axe, J.D.; Nakamura, Y. Evidence for stripe correlations of spins and holes in copper oxide superconductors. Nature 1995, 375, 56. [Google Scholar] [CrossRef]
- Abbamonte, P.; Rusydi, A.; Smadici, S.; Gu, G.D.; Sawatzky, G.A.; Feng, D.L. Spatially modulated ’Mottness’ in La2−xBaxCuO4. Nat. Phys. 2005, 1, 155. [Google Scholar] [CrossRef]
- Fink, J.; Schierle, E.; Weschke, E.; Geck, J.; Hawthorn, D.; Soltwisch, V.; Wadati, H.; Wu, H.-H. Charge ordering in La1.8-xEu0.2SrxCuO4 studied by resonant soft x-ray diffraction. Phys. Rev. B 2009, 79, 100502. [Google Scholar] [CrossRef]
- Ghiringhelli, G.; Le Tacon, M.; Minola, M.; Blanco-Canosa, S.; Mazzoli, C.; Brookes, N.B.; De Luca, G.M.; Frano, A.; Hawthorn, G.; He, F.; et al. Long-Range Incommensurate Charge Fluctuations in (Y,Nd)Ba2Cu3O6+x. Science 2012, 337, 821. [Google Scholar] [CrossRef] [PubMed]
- Chang, J.; Blackburn, E.; Holmes, A.T.; Christensen, N.B.; Larsen, J.; Mesot, J.; Liang, R.; Bonn, D.A.; Hardy, W.N.; Watenphul, A.; et al. Direct observation of competition between superconductivity and charge density wave order in YBa2Cu3O6.67. Nat. Phys. 2012, 8, 871. [Google Scholar] [CrossRef]
- Neto, E.H.d.; Aynajian, P.; Frano, A.; Comin, R.; Schierle, E.; Weschke, E.; Gyenis, A.; Wen, J.; Schneeloch, J.; Xu, Z.; et al. Ubiquitous Interplay Between Charge Ordering and High-Temperature Superconductivity in Cuprates. Science 2014, 343, 393. [Google Scholar] [CrossRef]
- Comin, R.; Frano, A.; Yess, M.M.; Yoshida, Y.; Eisaki, H.; Schierle, E.; Weschke, E.; Sutarto, R.; He, F.; Soumyanarayanan, A.; et al. Charge Order Driven by Fermi-Arc Instability in Bi2Sr2−xLaxCuO6+δ. Science 2014, 343, 390. [Google Scholar] [CrossRef]
- Hücker, M.; Christensen, N.B.; Holmes, A.T.; Blackburn, E.; Forgan, E.M.; Liang, R.; Bonn, D.A.; Hardy, W.N.; Gutowski, O.; Zimmermann, M.V.; et al. Competing charge, spin, and superconducting orders in underdoped YBa2Cu3Oy. Phys. Rev. B 2014, 90, 054514. [Google Scholar] [CrossRef]
- Doiron-Leyraud, N.; Proust, C.; LeBoeuf, D.; Levallois, J.; Bonnemaison, J.-B.; Liang, R.; Bonn, D.A.; Hardy, W.N.; Taillefer, L. Quantum oscillations and the Fermi surface in an underdoped high-Tc superconductor. Nature 2007, 447, 565. [Google Scholar] [CrossRef]
- Sebastian, S.E.; Harrison, N.; Lonzarich, G.G. Towards resolution of the Fermi surface in underdoped high-Tc superconductors. Rep. Prog. Phys. 2012, 75, 102501. [Google Scholar] [CrossRef]
- Laliberté, F.; Chang, J.; Doiron-Leyraud, N.; Hassinger, E.; Daou, R.; Rondeau, M.; Ramshaw, B.J.; Liang, R.; Bonn, D.A.; Hardy, W.N.; et al. Fermi-surface reconstruction by stripe order in cuprate superconductors. Nat. Commun. 2011, 2, 432. [Google Scholar] [CrossRef] [PubMed]
- Balseiro, C.A.; Falicov, L.M. Superconductivity and charge-density waves. Phys. Rev. B 1979, 20, 4457. [Google Scholar] [CrossRef]
- Valla, T.; Federov, A.V.; Lee, J.; Davis, J.C.; Gu, G.D. The Ground State of the Pseudogap in Cuprate Superconductors. Science 2006, 314, 1914. [Google Scholar] [CrossRef]
- Li, Q.; Hücker, M.; Gu, G.D.; Tsvelik, A.M.; Tranquada, J.M. Two-Dimensional Superconducting Fluctuations in Stripe-Ordered La1.875Ba0.125CuO4. Phys. Rev. Lett. 2007, 99, 067001. [Google Scholar] [CrossRef] [PubMed]
- Müller, K.A. Proceedings of the ICTP Workshop: Intrinsic Multiscale Structure And Dynamics in Complex Electronic Oxides; Bishop, A.R., Shenoy, S.R., Sridhar, S., Eds.; World Scientific Publishing: Singapore, 2003; pp. 1–5. [Google Scholar]
- Müller, K.A. Superconductivity in Complex Systems; Müller, K.A., Bussmann-Holder, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–11. [Google Scholar]
- Müller, K.A. Handbook of High-Temperature Superconductivity; Schrieffer, J.R., Brooks, J.S., Eds.; Springer: New York, NY, USA, 2007; pp. 1–18. [Google Scholar]
- Bussmann-Holder, A.; Keller, H.; Bishop, A.R.; Simon, A.; Müller, K.A. Polaron coherence as origin of the pseudogap phase in high temperature superconducting cuprates. J. Supercond. Nov. Magn. 2008, 21, 353. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Keller, H.; Bishop, A.R.; Simon, A.; Micnas, R.; Müller, K.A. Unconventional isotope effects as evidence for polaron formation in cuprates. EPL 2005, 72, 423. [Google Scholar] [CrossRef]
- Perali, A.; Bianconi, A.; Lanzara, A.; Saini, N.L. The gap amplification at a shape resonance in a superlattice of quantum stripes: A mechanism for high Tc. Solid State Commun. 1996, 100, 181. [Google Scholar] [CrossRef]
- Bianconi, A.; Valletta, A.; Perali, A.; Saini, N.L. High Tc superconductivity in a superlattice of quantum stripes. Solid State Commun. 1997, 102, 369. [Google Scholar] [CrossRef]
- Bianconi, A.; Innocenti, D.; Valletta, A.; Perali, A. Shape Resonances in superconducting gaps in a 2DEG at oxide-oxide interface. J. Phys. Conf. Ser. 2014, 529, 012007. [Google Scholar] [CrossRef]
- Innocenti, D.; Poccia, N.; Ricci, A.; Valletta, A.; Caprara, S.; Perali, A.; Bianconi, A. Resonant and crossover phenomena in a multiband superconductor: Tuning the chemical potential near a band edge. Phys. Rev. B 2010, 82, 184528. [Google Scholar] [CrossRef]
- Innocenti, D.; Valletta, A.; Bianconi, A. Shape resonance at a Lifshitz transition for high temperature superconductivity in multiband superconductors. J. Supercond. Nov. Magn. 2011, 24, 1137. [Google Scholar] [CrossRef]
- Innocenti, D.; Caprara, S.; Poccia, N.; Ricci, A.; Valletta, A.; Bianconi, A. Shape resonance for the anisotropic superconducting gaps near a Lifshitz transition: The effect of electron hopping between layers. Supercond. Sci. Technol. 2010, 24, 015012. [Google Scholar] [CrossRef]
- Perali, A.; Innocenti, D.; Valletta, A.; Bianconi, A. Anomalous isotope effect near a 2.5 Lifshitz transition in a multi-band multi-condensate superconductor made of a superlattice of stripes. Supercond. Sci. Technol. 2012, 25, 124002. [Google Scholar] [CrossRef]
- Seibold, G.; Varlamov, S. Relationship between incommensurability and superconductivity in Peierls- distorted charge-density-wave systems. Phys. Rev. B 1999, 60, 13056. [Google Scholar] [CrossRef]
- Mierzejewski, M.; Zielinski, J.; Entel, P. Phonon-Induced Contributions to Superconductivity in the Presence of Charge-Density Waves. J. Supercond. Nov. Magn. 2001, 14, 449. [Google Scholar] [CrossRef]
- Emery, V.J.; Kivelson, S.A.; Zachar, O. Spin-gap proximity effect mechanism of high-temperature superconductivity. Phys. Rev. B 1997, 56, 6120. [Google Scholar] [CrossRef]
- Perali, A.; Castellani, C.; Castro, C.D.; Grilli, M. d-wave superconductivity near charge instabilities. Phys. Rev. B 1996, 54, 16216. [Google Scholar] [CrossRef]
- Berg, E.; Fradkin, E.; Kim, E.-A.; Kivelson, S.A.; Oganesyan, V.; Tranquada, J.M.; Zhang, S.C. Dynamical Layer Decoupling in a Stripe-Ordered High-Tc Superconductor. Phys. Rev. Lett. 2007, 99, 127003. [Google Scholar] [CrossRef]
- Berg, E.; Fradkin, E.; Kivelson, S.A.; Tranquada, J.M. Striped superconductors: How spin, charge and superconducting orders intertwine in the cuprates. New J. Phys. 2009, 11, 115004. [Google Scholar] [CrossRef]
- Dagotto, E. Nanoscale Phase Separation and Colossal Magnetoresistance; Springer Series in Solid-State Sciences; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Hizhnyakov, V.; Sigmund, E. Anisotropic pairing caused by unscreened long-range interactions. Phys. Rev. B 1996, 53, 5163. [Google Scholar] [CrossRef]
- Hizhnyakov, V.; Sigmund, E. Anisotropic pairing caused by unscreened long-range interactions. J. Supercond. 1996, 9, 335. [Google Scholar] [CrossRef]
- Sigmund, E.; Hizhnyakov, V.; Nevedrov, D.; Bill, A. Anisotropy of the Superconducting Gap in the Presence of Electron-Phonon and Coulomb Interactions. J. Supercond. 1997, 10, 441. [Google Scholar] [CrossRef]
- Shelkan, A.; Hizhnyakov, V.; Sigmund, E. Self-Consistent Calculation of the Self-Trapping Barrier for a Hole in the CuO2 Plane. J. Supercond. 1998, 11, 677. [Google Scholar] [CrossRef]
- Shelkan, A.; Hizhnyakov, V.; Sigmund, E. Free and Spin-Polaron States in High TC Superconductors. In Vibronic Interactions: Jahn-Teller Effect in Crystals and Molecules; NATO Science Series (Series II: Mathematics, Physics and Chemistry); Kaplan, M.D., Zimmerman, G.O., Eds.; Springer: Dordrecht, The Netherlands, 2001. [Google Scholar]
- Hizhnyakov, V.; Sigmund, E.; Zavt, G. Existence of a barrier between free and ferron-type (self-trapped) hole states in high- Tc cuprates. Phys. Rev. B 1991, 44, 12639. [Google Scholar] [CrossRef]
- Shelkan, A.; Zavt, G.; Hizhnyakov, V.; Sigmund, E. Effect of O-O transfer upon a hole in CuO2 planes of HTS. Z. Phys. B 1997, 104, 433. [Google Scholar] [CrossRef]
- Shelkan, A.; Hizhnyakov, V.; Sigmund, E. Self-Trapping Barrier for Phonon Polaron in Anisotropic Crystal. J. Supercond. 2000, 13, 21. [Google Scholar] [CrossRef]
- Ivanov, T.I. Phase separation in the slave-fermion mean-field theory of the t-J model. Phys. Rev. B 1991, 44, 12077. [Google Scholar] [CrossRef]
- Gimm, T.; Salk, S.H.S. Phase separation based on a U(1) slave-boson functional integral approach to the t-J model. Phys. Rev. B 2000, 62, 13930. [Google Scholar] [CrossRef]
- Koch, E.; Zeyher, R. Charge density waves as the origin of dip-hump structures in the differential tunneling conductance of cuprates: The case of d-wave superconductivity. Phys. Rev. B 2004, 70, 094519. [Google Scholar]
- Irkhin, V.Y.; Igoshev, P.A. Electron States and Magnetic Phase Diagrams of Strongly Correlated Systems. Phys. Met. Metallogr. 2018, 119, 1267. [Google Scholar] [CrossRef]
- Kagan, M.Y.; Rice, T.M. Superconductivity in the two-dimensional t-J model at low electron density. J. Phys. Condens. Matter 1994, 6, 3771. [Google Scholar] [CrossRef]
- Kagan, M.Y.; Kugel, K.I. Inhomogeneous charge distributions and phase separation in manganites. Phys. Uspekhi 2001, 44, 553. [Google Scholar] [CrossRef]
- Kugel, K.I.; Khomskii, D.I. The Jahn-Teller effect and magnetism: Transition metal compounds. Sov. Phys. Uspekhi 1982, 25, 231. [Google Scholar] [CrossRef]
- Sboychakov, A.O.; Kugel, K.I.; Rakhmanov, A.L. Phase separation in a two-band model for strongly correlated electrons. Phys. Rev. B 2007, 76, 195113. [Google Scholar] [CrossRef]
- Sherman, A. Negative electron compressibility in the Hubbard model. Phys. Scr. 2020, 95, 015806. [Google Scholar] [CrossRef]
- Vladimir, M.I.; Moskalenko, V.A. Diagram technique for the Hubbard model. Theor. Math. Phys. 1990, 82, 301. [Google Scholar] [CrossRef]
- Metzner, W. Linked-cluster expansion around the atomic limit of the Hubbard model. Phys. Rev. B 1991, 43, 8549. [Google Scholar] [CrossRef]
- Pairault, S.D.; Tremblay, A.-M.S. Strong-coupling perturbation theory of the Hubbard model. Eur. Phys. J. B 2000, 16, 85. [Google Scholar] [CrossRef]
- Sherman, A. Influence of spin and charge fluctuations on spectra of the two-dimensional Hubbard model. J. Phys. Condens. Matter 2018, 30, 195601. [Google Scholar] [CrossRef] [PubMed]
- Sherman, A. Magnetic properties and temperature variation of spectra in the Hubbard model. Eur. Phys. J. B 2019, 92, 55. [Google Scholar] [CrossRef]
- Kubo, R. Generalized Cumulant Expansion Method. J. Phys. Soc. Jpn. 1962, 17, 1100. [Google Scholar] [CrossRef]
- Chang, C.; Zhang, S. Spatially inhomogeneous phase in the two-dimensional repulsive Hubbard model. Phys. Rev. B 2008, 78, 165101. [Google Scholar] [CrossRef]
- Heiselberg, H. Hubbard-model calculations of phase separation in optical lattices. Phys. Rev. A 2009, 79, 063611. [Google Scholar] [CrossRef]
- White, S.R.; Scalapino, D.J. Doping asymmetry and striping in a three-orbital CuO2 Hubbard model. Phys. Rev. B 2015, 92, 205112. [Google Scholar] [CrossRef]
- Kanamori, J. Electron Correlation and Ferromagnetism of Transition Metals. Prog. Theor. Phys. 1963, 30, 275. [Google Scholar] [CrossRef]
- Georges, A.; Medici, L.d.; Mravlje, J. Strong Correlations from Hund’s Coupling. Annu. Rev. Condens. Matter Phys. 2013, 4, 137. [Google Scholar] [CrossRef]
- Sherman, A. Hubbard-Kanamori model: Spectral functions, negative electron compressibility, and susceptibilities. Phys. Scr. 2020, 95, 095804. [Google Scholar] [CrossRef]
- Shenoy, V.B.; Rao, C.N.R. Electronic phase separation and other novel phenomena and properties exhibited by mixed-valent rare-earth manganites and related materials. Phil. Trans. R. Soc. A 2008, 366, 63. [Google Scholar] [CrossRef]
- Dai, P.; Hu, J.; Dagotto, E. Magnetism and its microscopic origin in iron-based high-temperature superconductors. Nat. Phys. 2012, 8, 709. [Google Scholar] [CrossRef]
- Lang, G.; Grafe, H.-J.; Paar, D.; Hammerath, F.; Manthey, K.; Behr, G.; Werner, J.; Büchner, B. Nanoscale Electronic Order in Iron Pnictides. Phys. Rev. Lett. 2010, 104, 097001. [Google Scholar] [CrossRef]
- Müller, K.A. Possible coexistence of s- and d-wave condensates in copper oxide superconductors. Nature 1995, 377, 133. [Google Scholar] [CrossRef]
- Bill, A.; Hizhnyakov, V.; Nevedrov, D.; Seibold, G.; Sigmund, E.Z. Electronic inhomogeneities, electron-lattice and pairing interactions in high-T c superconductors. Phys. B 1997, 104, 753. [Google Scholar] [CrossRef]
- Kresin, V.Z.; Morawitz, H. Layer plasmons and high-Tc superconductivity. Phys. Rev. B 1988, 37, 7854. [Google Scholar] [CrossRef] [PubMed]
- Bill, A.; Morawitz, H.; Kresin, V.Z. Electronic collective modes and superconductivity in layered conductors. Phys. Rev. B 2003, 68, 144519. [Google Scholar] [CrossRef]
- Bill, A.; Morawitz, H.; Kresin, V.Z. Acoustic Plasmons in Layered Systems and the Phonon-Plasmon Mechanism of Superconductivity. J. Low Temp. Phys. 1999, 117, 283. [Google Scholar] [CrossRef]
- Bill, A.; Morawitz, H.; Kresin, V.Z. Dynamical screening and superconducting state in intercalated layered metallochloronitrides. Phys. Rev. B 2002, 66, 100501. [Google Scholar] [CrossRef]
- Hepting, M.; Chaix, L.; Huang, E.W.; Fumagalli, R.; Peng, Y.Y.; Moritz, B.; Kummer, K.; Brookes, N.B.; Lee, W.C.; Hashimoto, M.; et al. Three-dimensional collective charge excitations in electron-doped copper oxide superconductors. Nature 2018, 563, 374. [Google Scholar] [CrossRef] [PubMed]
- Lin, J.; Yuan, J.; Jin, K.; Yin, Z.; Li, G.; Zhou, K.J.; Lu, X.; Dantz, M.; Schmitt, T.; Ding, H.; et al. Doping evolution of the charge excitations and electron correlations in electron-doped superconducting La2−xCexCuO4. NPJ Quantum Mater. 2020, 5, 4. [Google Scholar] [CrossRef]
- Heid, R.; Bohnen, K.-P.; Zeyher, R.; Manske, D. Momentum Dependence of the Electron-Phonon Coupling and Self-Energy Effects in Superconducting YBa2Cu3O7 within the Local Density Approximation. Phys. Rev. Lett. 2008, 100, 137001. [Google Scholar] [CrossRef]
- Heid, R.; Zeyher, R.; Manske, D.; Bohnen, K.-P. Phonon-induced pairing interaction in YBa2Cu3O7 within the local-density approximation. Phys. Rev. 2009, 80, 024507. [Google Scholar] [CrossRef]
- Gunnarsson, O.; Rösch, O. Interplay between electron–phonon and Coulomb interactions in cuprates. J. Phys. Condens. Matter 2008, 20, 043201. [Google Scholar] [CrossRef]
- Capone, M.; Castellani, C.; Grilli, M. Electron-Phonon Interaction in Strongly Correlated Systems. Adv. Condens. Matter Phys. 2010, 2010, 920860. [Google Scholar] [CrossRef]
- von Oelsen, E.; Ciolo, A.D.; Lorenzana, J.; Seibold, G.; Grilli, M. Phonon renormalization from local and transitive electron-lattice couplings in strongly correlated systems. Phys. Rev. B 2010, 81, 155116. [Google Scholar] [CrossRef]
- Seibold, G.; Grilli, M.; Lorenzana, J. Influence of correlations on transitive electron-phonon couplings in cuprate superconductors. Phys. Rev. B 2011, 83, 174522. [Google Scholar] [CrossRef]
- Norman, M.R. Relation of neutron incommensurability to electronic structure in high-temperature superconductors. Phys. Rev. B 2000, 61, 14751. [Google Scholar] [CrossRef]
- Bill, A.; Hizhnyakov, V.; Sigmund, E. Phonon renormalization and symmetry of the superconducting order parameter. Phys. Rev. B 1995, 52, 7637. [Google Scholar] [CrossRef]
- Bill, A.; Hizhnyakov, V.; Sigmund, E. q-Dependent Neutron Scattering: A Signature of the Gap Anisotropy in High-Tc Superconductors. J. Supercond. 1996, 10, 493. [Google Scholar] [CrossRef]
- Maly, J.; Lin, D.Z.; Levin, K. Superconducting order parameter symmetry in multilayer cuprates. Phys. Rev. B 1996, 53, 6786. [Google Scholar] [CrossRef]
- Seibold, G.; Lorenzana, J. Stability of metallic stripes in the one-band extended Hubbard model. Phys. Rev. B 2004, 69, 134513. [Google Scholar] [CrossRef]
- Gebhard, F. Gutzwiller correlated wave functions in finite dimensions d: A systematic expansion in 1/d. Phys. Rev. B 1990, 41, 9452. [Google Scholar] [CrossRef]
- Lorenzana, J.; Seibold, G. Metallic Mean-Field Stripes, Incommensurability, and ChemicalPotential in Cuprates. Phys. Rev. Lett. 2002, 89, 136401. [Google Scholar] [CrossRef] [PubMed]
- Yamada, K.; Lee, C.H.; Kurahashi, K.; Wada, J.; Wakimoto, S.; Ueki, S.; Kimura, H.; Endoh, Y.; Hosoya, S.; Shirane, G.; et al. Doping dependence of the spatially modulated dynamical spin correlations and the superconducting-transition temperature in La2−xSrxCuO4. Phys. Rev. B 1998, 57, 6165. [Google Scholar] [CrossRef]
- Tranquada, J.M.; Woo, H.; Perring, T.G.; Goka, H.; Gu, G.D.; Xu, G.; Fujita, M.; Yamada, K. Quantum magnetic excitations from stripes in copper oxide superconductors. Nature 2004, 429, 534. [Google Scholar] [CrossRef] [PubMed]
- Coldea, R.; Hayden, S.M.; Aeppli, G.; Perring, T.G.; Frost, C.D.; Mason, T.E.; Cheong, S.-W.; Fisk, Z. Spin Waves and Electronic Interactions in LaCuO4. Phys. Rev. Lett. 2001, 86, 5377. [Google Scholar] [CrossRef] [PubMed]
- Seibold, G.; Becca, F.; Lorenzana, J. Theory of Antibound States in Partially Filled Narrow Band Systems. Phys. Rev. Lett. 2008, 100, 016405. [Google Scholar] [CrossRef]
- Seibold, G.; Lorenzana, J. Time-Dependent Gutzwiller Approximation for the Hubbard Model. Phys. Rev. Lett. 2001, 86, 2605. [Google Scholar] [CrossRef]
- Seibold, G.; Lorenzana, J. Magnetic Fluctuations of Stripes in the High Temperature Cuprate Superconductors. Phys. Rev. Lett. 2005, 94, 107006. [Google Scholar] [CrossRef]
- Seibold, G.; Lorenzana, J. Doping dependence of spin excitations in the stripe phase of high-Tc superconductors. Phys. Rev. B 2006, 73, 144515. [Google Scholar] [CrossRef]
- Lorenzana, J.; Seibold, G. Dynamics of Metallic Stripes in Cuprates. Phys. Rev. Lett. 2003, 90, 066404. [Google Scholar] [CrossRef]
- Sawatzky, G.A. Quasiatomic Auger Spectra in Narrow-Band Metals. Phys. Rev. Lett. 1977, 39, 504. [Google Scholar] [CrossRef]
- Cini, M.; Verdozzi, C. Photoemission and Auger CVV spectra of partially filled bands: A cluster approach. Solid State Commun. 1986, 57, 657. [Google Scholar] [CrossRef]
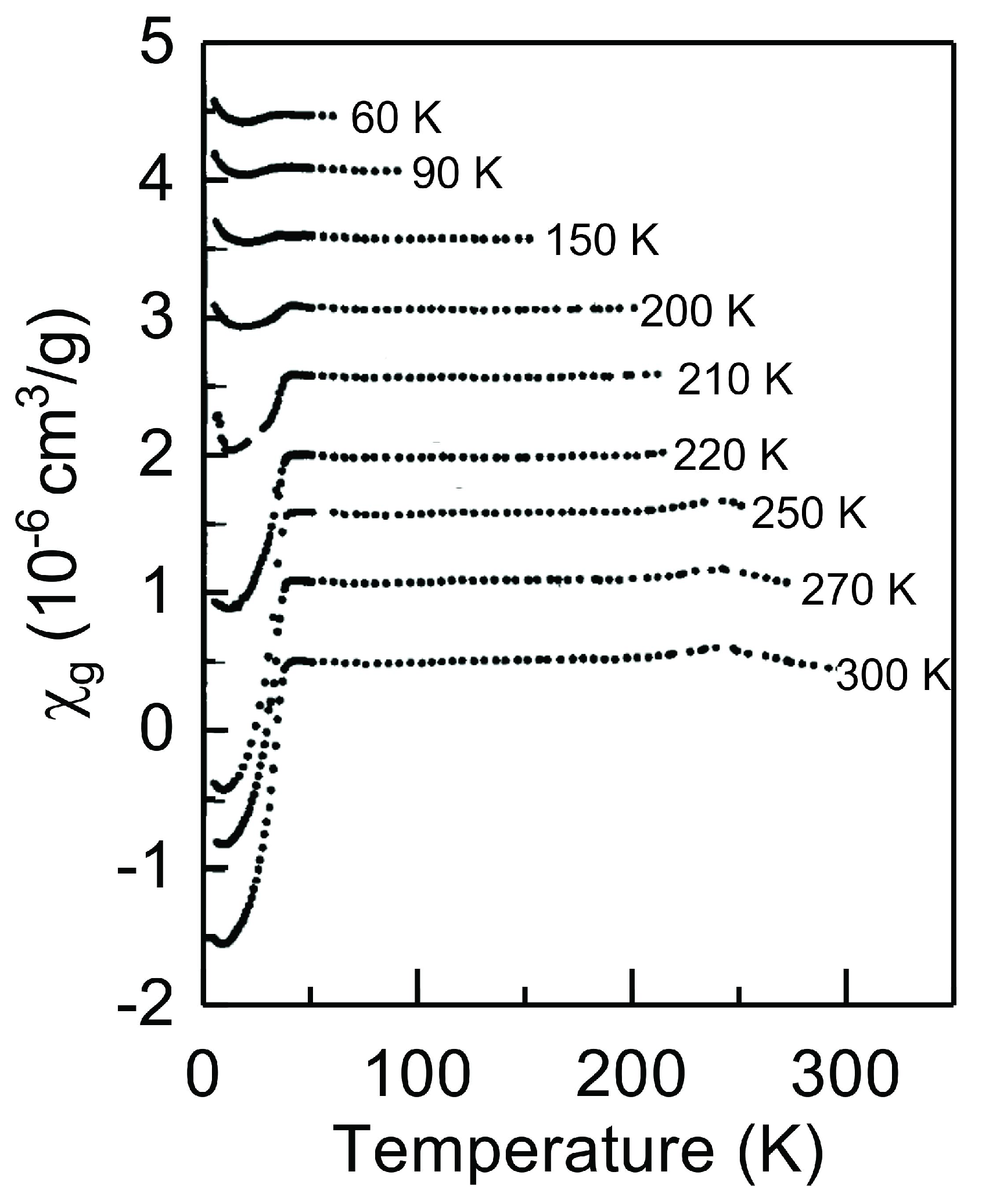
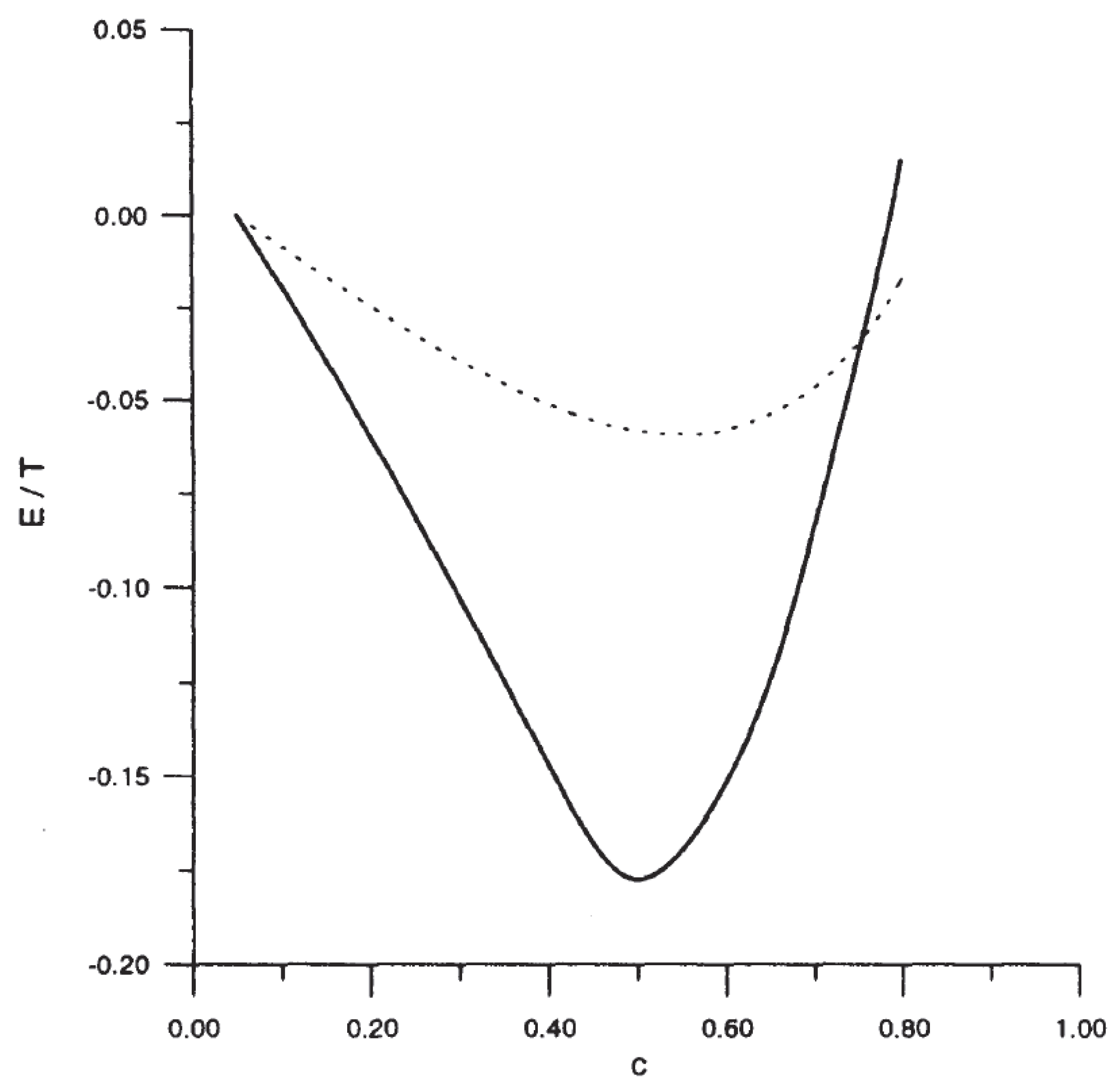
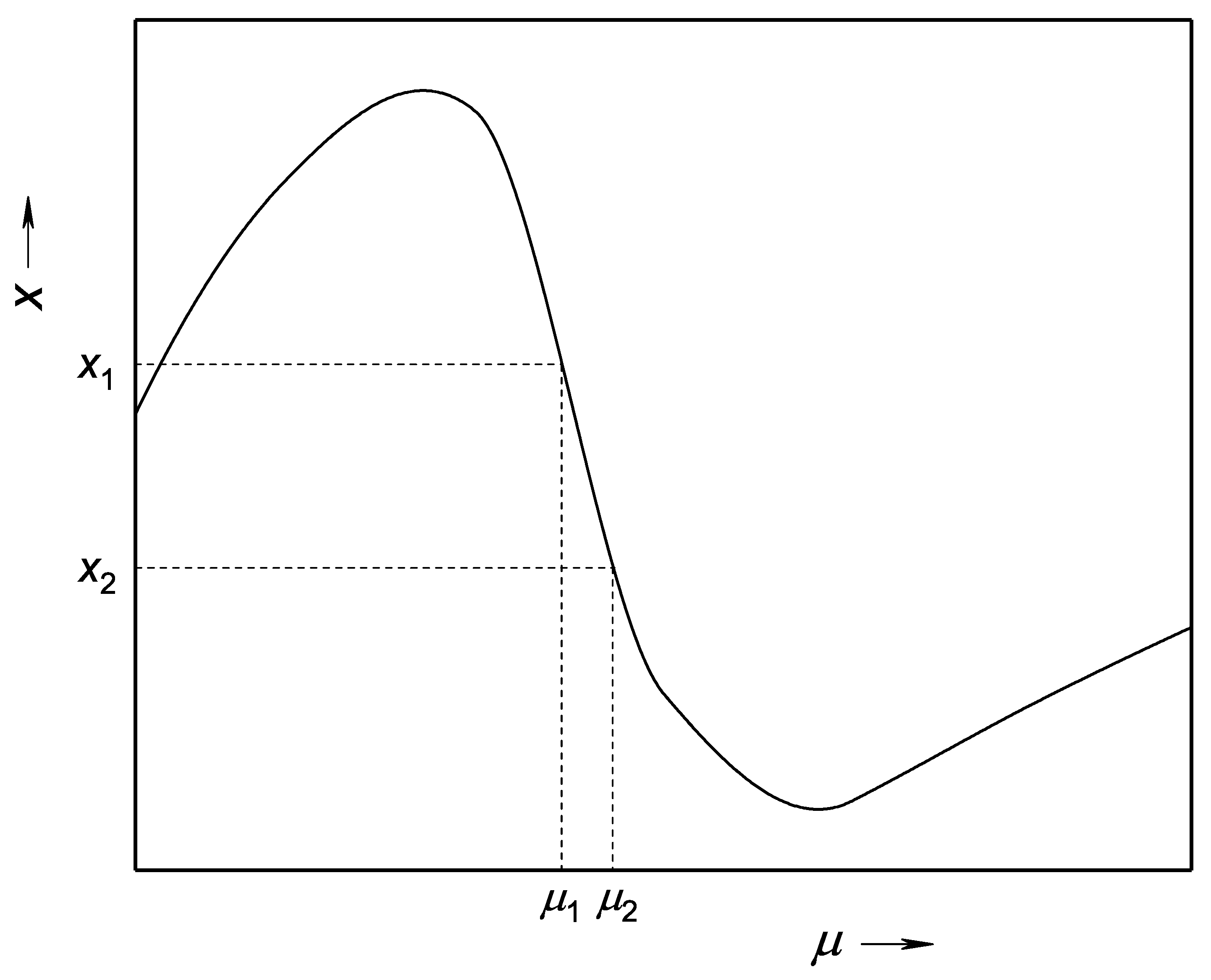
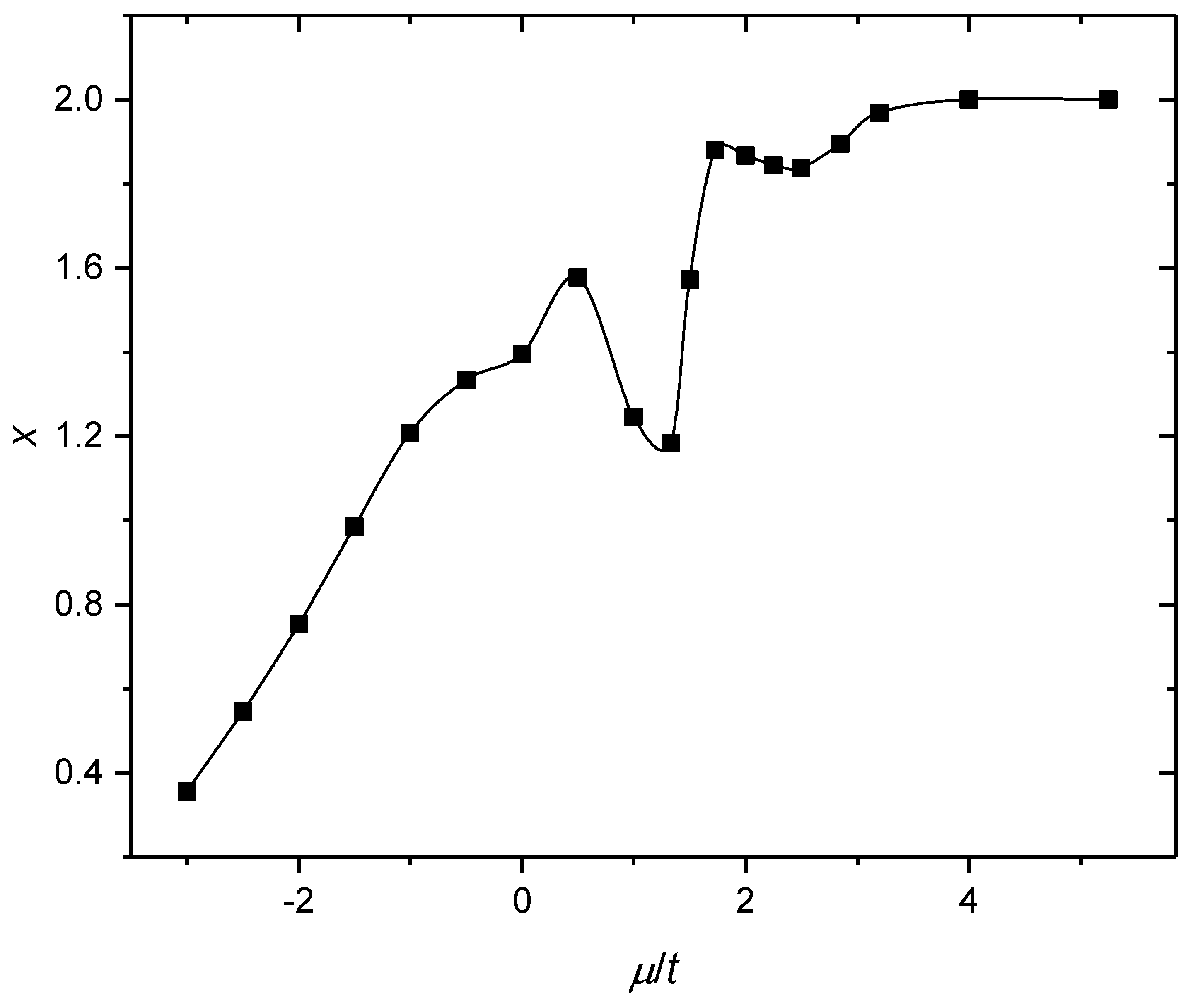
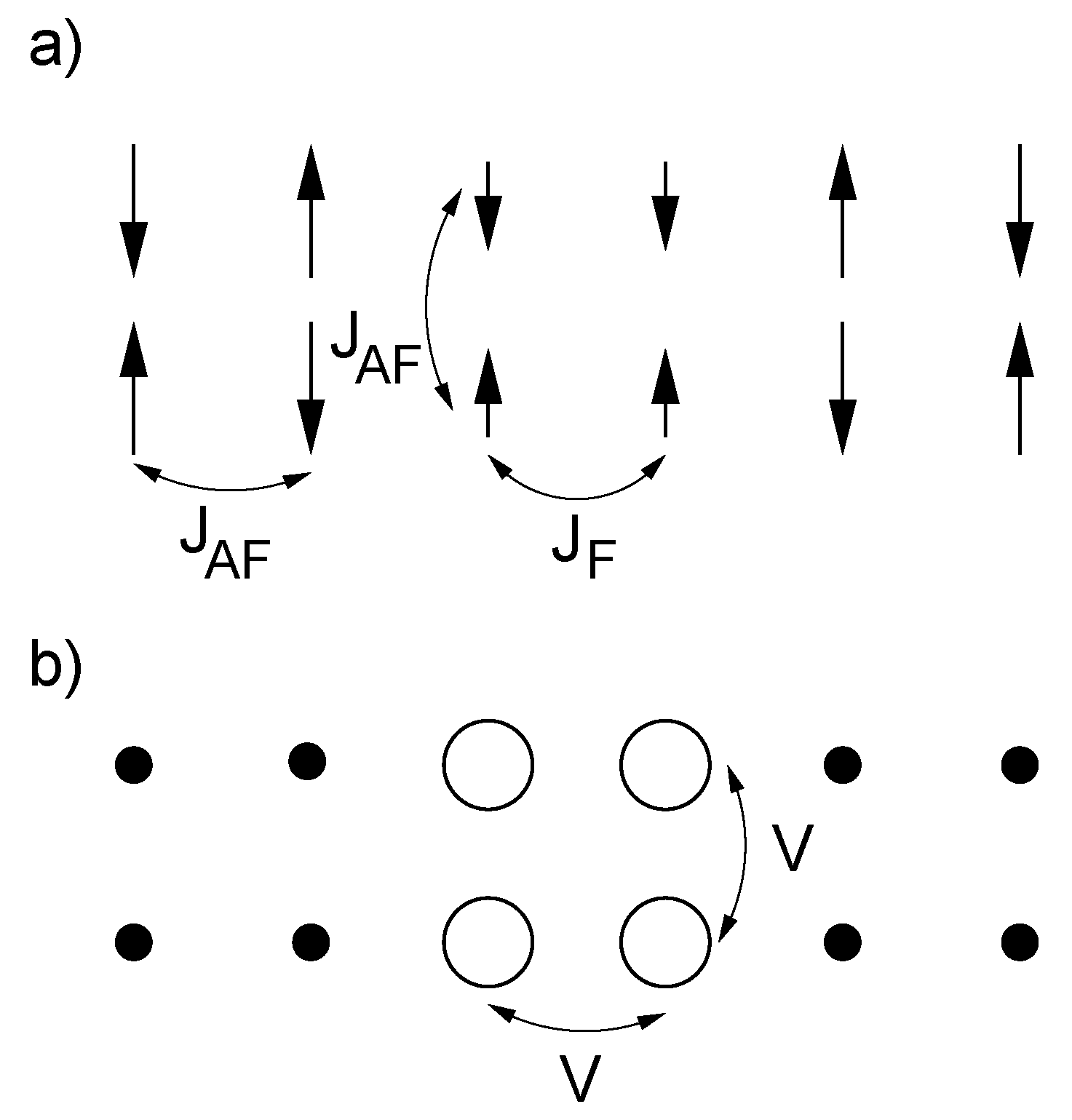
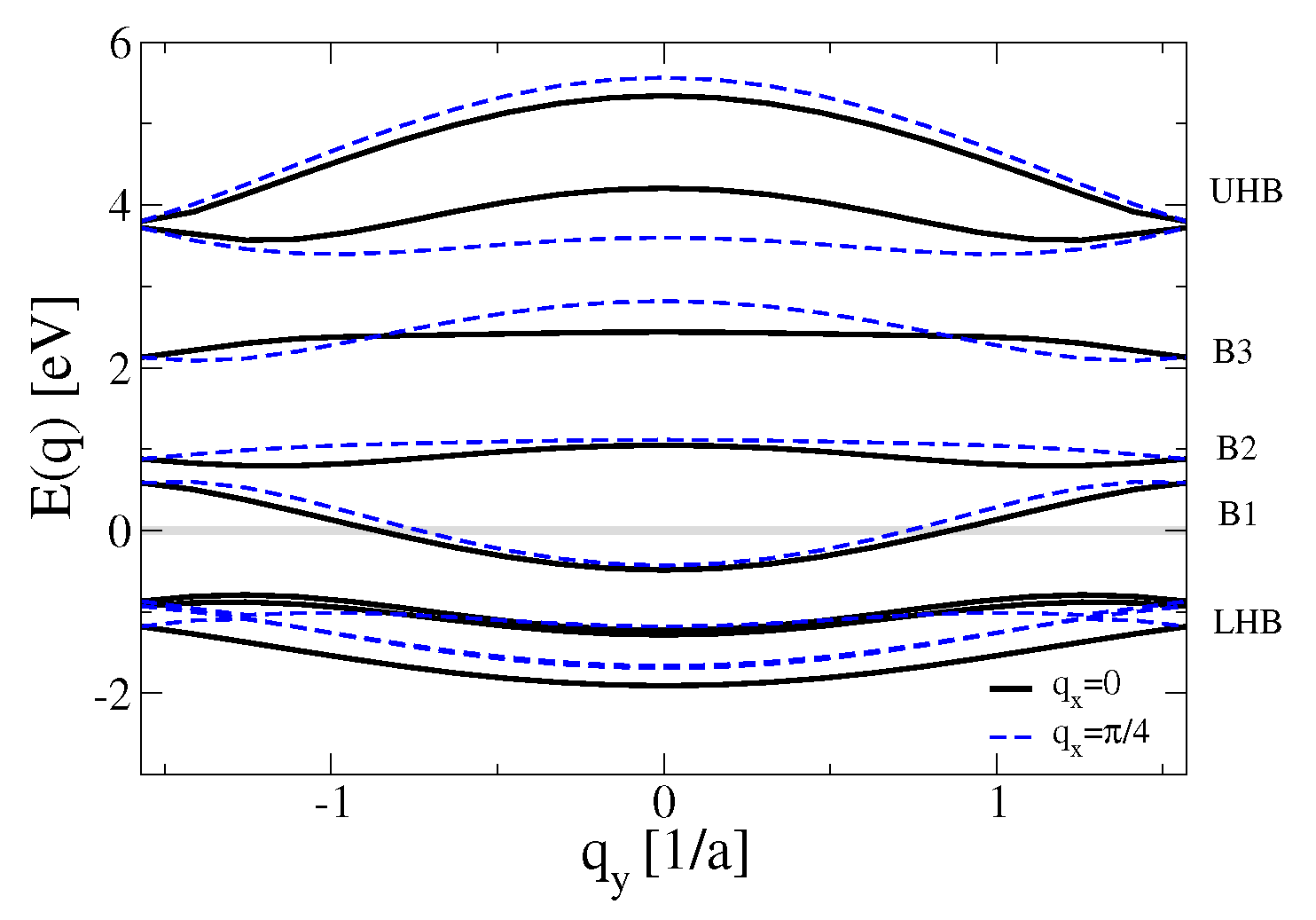
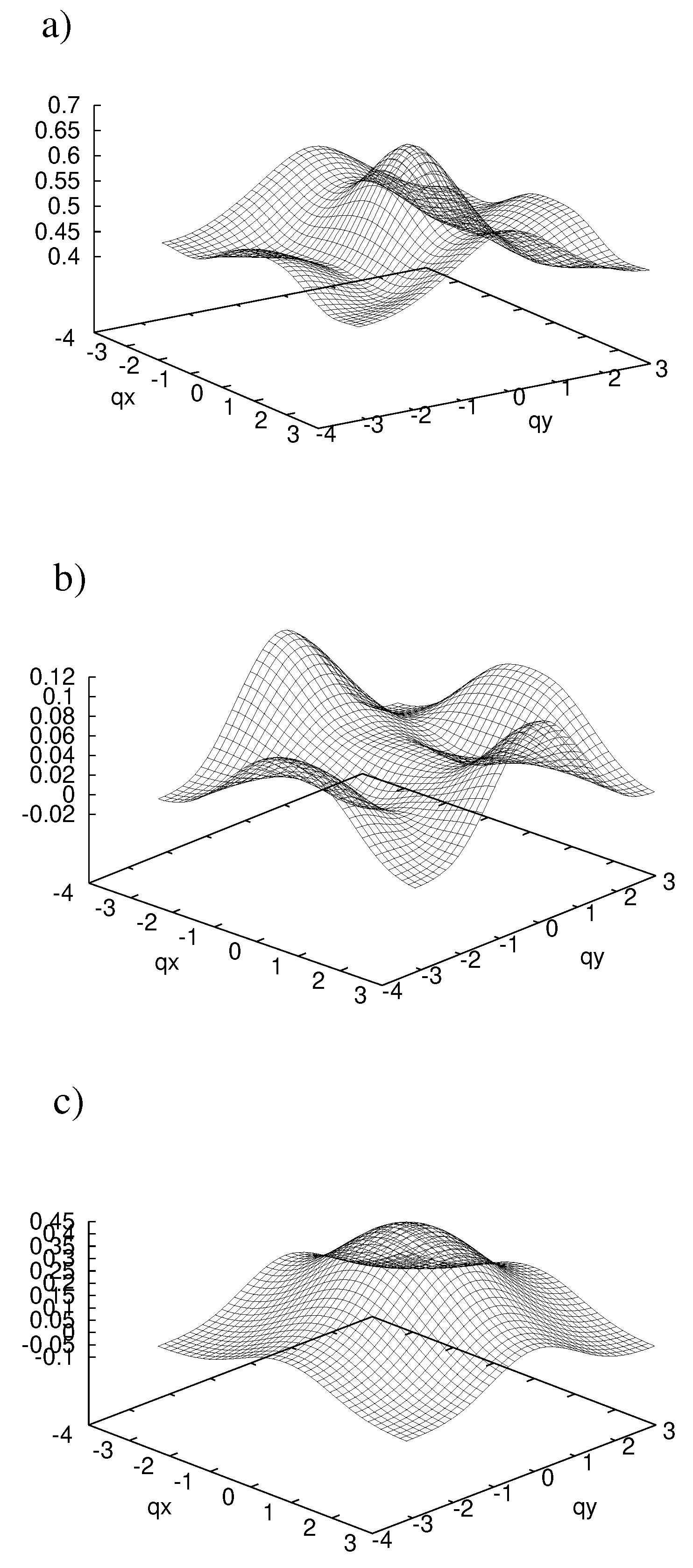
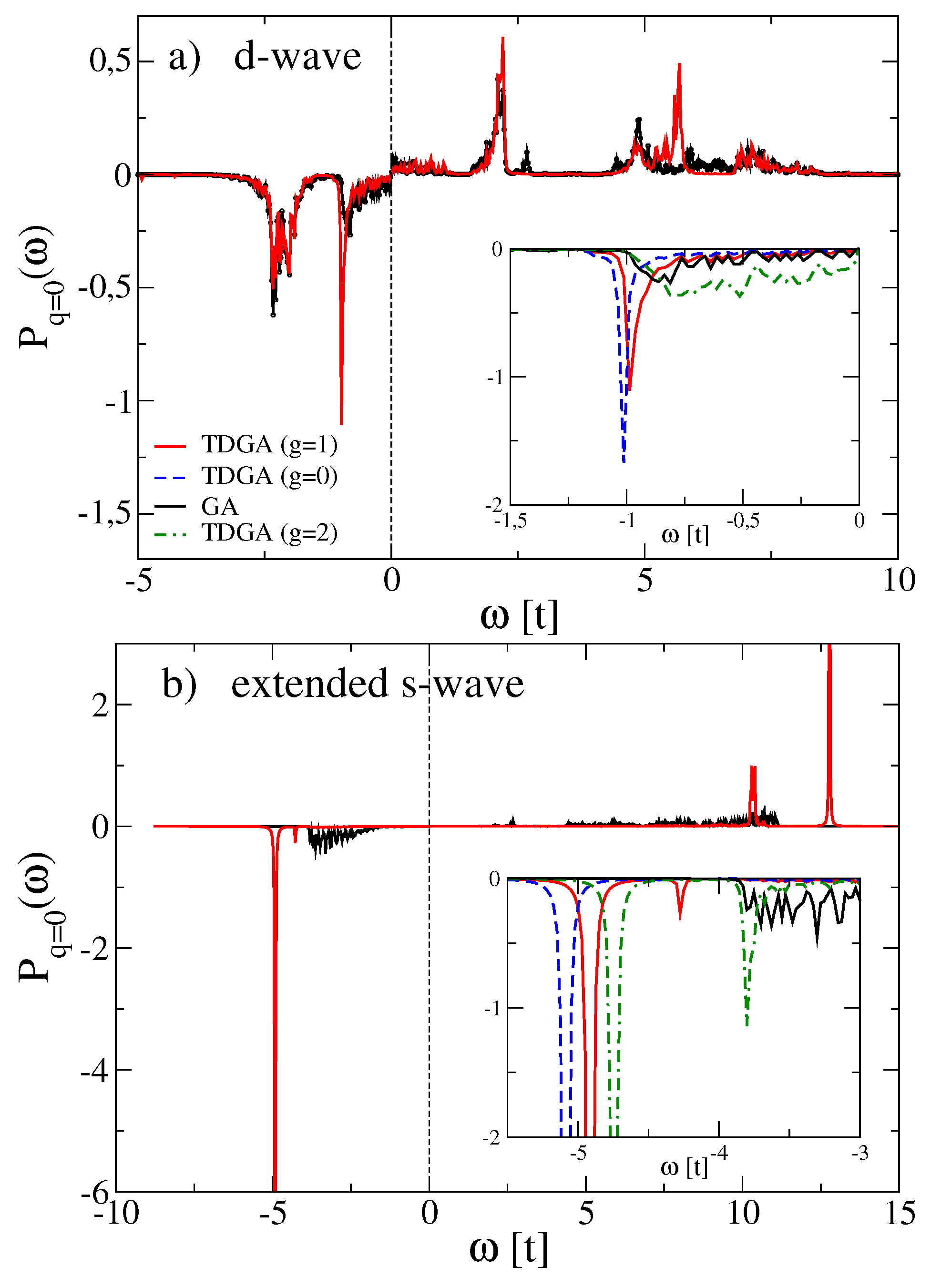
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bill, A.; Hizhnyakov, V.; Kremer, R.K.; Seibold, G.; Shelkan, A.; Sherman, A. Phase Separation and Pairing Fluctuations in Oxide Materials. Condens. Matter 2020, 5, 65. https://doi.org/10.3390/condmat5040065
Bill A, Hizhnyakov V, Kremer RK, Seibold G, Shelkan A, Sherman A. Phase Separation and Pairing Fluctuations in Oxide Materials. Condensed Matter. 2020; 5(4):65. https://doi.org/10.3390/condmat5040065
Chicago/Turabian StyleBill, Andreas, Vladimir Hizhnyakov, Reinhard K. Kremer, Götz Seibold, Aleksander Shelkan, and Alexei Sherman. 2020. "Phase Separation and Pairing Fluctuations in Oxide Materials" Condensed Matter 5, no. 4: 65. https://doi.org/10.3390/condmat5040065
APA StyleBill, A., Hizhnyakov, V., Kremer, R. K., Seibold, G., Shelkan, A., & Sherman, A. (2020). Phase Separation and Pairing Fluctuations in Oxide Materials. Condensed Matter, 5(4), 65. https://doi.org/10.3390/condmat5040065






