1. Introduction
In the last 20 years, the number of stroke cases among people aged 20 to 64 years has increased by 25%. Stroke often leads to neuromotor disabilities that can impair finger movement. Since finger movement is crucial for basic activities of daily life (ADL), there is significant motivation to prioritize finger rehabilitation following injury or stroke [
1]. It is well established that repetitive flexion and extension movements of the fingers, even when performed passively, can promote neuromuscular re-education, help prevent spasticity, and manage pain associated with hand paralysis resulting from acquired brain damage [
2,
3].
In view of the consequences and to help with post-stroke recovery, autonomous solutions focused on the functional rehabilitation of the hand’s prehensile capabilities are being developed, among which are hand exoskeletons. Exoskeletons may offer a more effective option than end-effector robot-assisted devices for treating finger motor impairment in stroke patients [
4]. These exoskeletons are structures that totally or partially cover the affected hand, and according to the functions they can perform, a distinction can be made between augmentative or assistive (improving capabilities for the execution of specific tasks) for teleoperation (haptic devices oriented to virtual models) and hand exoskeletons for rehabilitation (RHEx) [
5]. The RHEx try to recover the motor abilities of patients by forcing repetitive actions on the affected joints: the most common ones are opening and closing the fingers. In this way, it is possible to lengthen the sessions and reduce the physicians’ permanent need for assistance. RHEx are not necessarily portable or lightweight; they can be fixed in a certain position. Even so, they are more versatile if they are portable because the patient can take them home to continue rehabilitation on their own. Moreover, the rapid progress of flexible electronics aligns with the growing demand for portable smart exoskeletons embedded with skin-interfacing flexible electronics (e-skin) [
2,
6,
7] provide fine-grained control and feedback RHEx, allowing for continuous and real-time monitoring of physiological signals. This helps users regain movement and improve strength in affected extremities. Still, the lightness and pragmatism of the exoskeleton topology must be optimized.
The goal population largely conditions the suitability of an RHEx design. The movements allowed by the RHEx should follow patients’ behavior naturally and without generating discomfort. The literature [
5] compiles characteristics and criteria that should guide the design of RHEx. However, the evaluations presented in RHEx proposals only include partial aspects, on a few subjects, and rarely patients [
2]. In this sense, the collaboration with rehabilitators in the context of the present research (DERAPPI project [
8]) has made clear the characteristics of the patient that are suitable for therapy with RHEx:
Plegic hand (MRC 0 [
9,
10] in all muscle groups of the hand).
Flaccid hand (spasticity up to MAS 1 [
11] would be admitted).
Absence of rigidity in the fingers. In case of some rigidity, it cannot interfere with normal intelligent grasping movement.
Skin is intact and there is no edema at the level of the hand.
Cognitive status enough to follow simple instructions.
Behaviorally stable.
For certain patients, it is essential to restrict the range of motion of specific joints to prevent articular degeneration. This goal is difficult to achieve with some RHEx designs inspired by soft robotics. Additionally, there is a lack of devices on the market that simultaneously target the mobilization of the index finger and are easy for either the physician or the patient to place on the hand [
12]. Focusing on the recovery of the index finger is crucial since it plays a key role in many grasps and ADL. Regarding RHEx control, devices operated by a pushbutton activated by the healthy hand or programmed to function in a loop are highly suitable for stroke rehabilitation. Applying the concepts of robustness and simplicity makes it desirable to be able to use a simple control rather than having to command and coordinate several actions. The idea behind underactuation in robotics is to use an ingenious mechanical system that can adapt to the requirements of a grasp or a trajectory with one actuator [
13]. This mechanical intelligence is commonly found in mechanical linkages where the different link lengths and joint types are determined at the design stage to follow a particular trajectory or adopt a particular posture. If this trajectory is entirely predetermined, then only one DOF suffices to follow it. Moreover, using a single actuator contributes to maintaining the lightweight and compact design of the device, which is desirable in a portable RHEx; therefore, the concept of using a single actuator for underactuation also merits consideration.
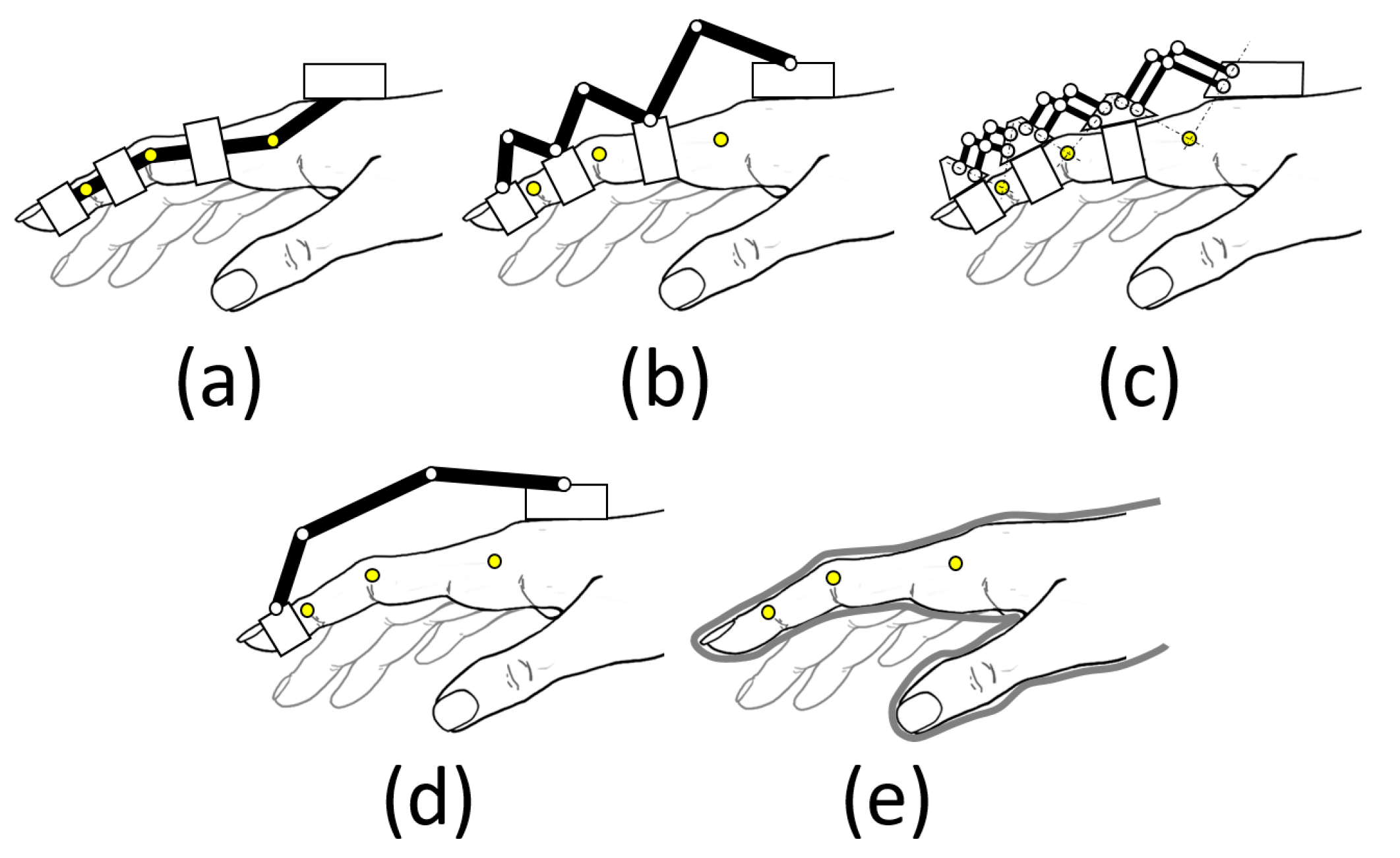
Several RHEx topologies can be discerned [
12,
14]:
- (i)
Those restraining each phalanx (
Figure 1a–c), using mechanisms to match the axes of rotation with those of the phalanges. They strictly limit spasticity during rehabilitation. However, the complexity of the design increases as higher finger mobility is sought.
- (ii)
Those that hold only the fingertip (base-to-distal topology,
Figure 1d), with the advantage that their design is simpler, as they do not have the restriction of matching the axes of rotation, so they can be lighter as well. Note that the three degrees of freedom that position and orient the distal phalange (DP) within the plane of flexion of the finger univocally condition the three degrees of freedom provided by the three joints: metacarpophalangeal (MCP), proximal, and distal interphalangeal (PIP, and DIP). However, the movement of the finger is less controlled, regarding the variety of exercises that can be performed by acting on the individual joints.
- (iii)
Those that fit over the entire finger, glove type (
Figure 1e), using flexible and lightweight materials that adapt to the hand (soft robotics). They are less cumbersome and less expensive, but care must be taken in the forces applied.
The choice between these approaches depends on the specific goals of the device and the patient’s needs in terms of rehabilitation. In the case of soft robotics (iii), having no rigid joints to guide torques and forces, the user’s skeleton becomes the guiding structure, and it may not be an appropriate choice in dealing with the patient’s spasticity. The RHEx based on articulated mechanisms guide the movement based on a guiding structure (an articulated linkage that performs the function of guiding the movement) and a guiding chain (a system that transmits the actuation, usually a Bowden cable, electrical, pneumatic, or a system of additional links to the first ones [
15]).
Control for RHEx can be relatively simple if they are aimed at task-specific training, allowing for passive control that overlooks the patient. For case (i), if independent control is desired for each degree of mobility of the hand, individual actuators will be required to adjust and control the movements of each finger independently. However, the design of the exoskeleton can be greatly simplified, and the cost of the device can be reduced by using sub-actuated designs, especially in case (ii), which makes them very economical and easy to transport. In this case, the RHEx may actuate on some degrees of freedom, leaving the others to move passively, i.e., allowing certain joints to freely adopt the position that best suits them, within their anatomical ranges of motion, to achieve the posture demanded by the exoskeleton.
In cases (i) and (ii), the location of the mechanism can be either dorsal (located on the dorsal part of the fingers,
Figure 1b–d), palmar (on the palmar part of the fingers, hardly used because they interfere with interacting with objects [
16]), or lateral (
Figure 1a). Lateral devices leave the palm of the hand free and therefore allow objects to be grasped. In addition, they allow patients to see their hands, which is important for visualizing progress during rehabilitation. In any of the three arrangements, the mechanism is attached to the hand via Velcro
®, flexible straps, or thimbles.
In recent years, different RHEx designs have been proposed in the literature [
2,
14,
15,
17], and there are already some commercial designs for diverse purposes [
16,
18]. There are very few RHEx reported in the literature that reach TRL level 9 [
19,
20], and, even though much research is being carried out on them, they are still far from being a practical solution. In a recent extensive literature search on the design and optimization of exoskeletons (over 722 studies from 2017 to 2023), it was shown that evolutionary computation (EC) methods are the most frequently used (genetic algorithms, particle swarm optimization, differential evolution, and evolutionary strategies) compared to other non-EC [
21]. This outcome is anticipated given EC methods’ numerous attributes, such as the ability to update multiple solutions simultaneously (population-based), their independence from gradient information, rapid convergence, and their capacity to tackle mixed optimization problems, including multimodal and multi-objective scenarios, to optimize different metrics (mainly force transmission (FT), workspace, weight, and size). However, this research found only two studies in which EC techniques were used for hand exoskeletons: one with an assistive application [
22] and another for rehabilitation (RHEx) [
23]. Close to these dates, we also found other four studies using EC for improving an RHEx [
24,
25,
26,
27].
Table 1 summarizes the studies related to the optimization of hand exoskeletons from 2014 to 2024.
Du et al. [
23], and Sarac et al. [
27], used the Single-Objective Genetic Algorithm [
30] to optimize the FT, whereas Iqbal et al. [
24] used a Weight-Based Genetic Algorithm (WBGA) [
21], the simplest classical method of solving multi-objective optimization problems, which considered factors like kinematic mapping, collision avoidance, and a global isotropy index, and FT. Vanteddu et al. [
25] also used WBGA, the cost function being the weighted sum of errors for the end position of the grasp trajectory between the kinematic model and the HUST (Huazhong University of Science and Technology) dataset concerning the joint angles of a natural human finger. Li et al. [
26] proposed a multi-parameter multi-objective optimization method (namely, the Elitist Non-Dominated Sorting Genetic Algorithm (NSGA–II) [
28,
29]) to enhance the three global manipulability measures simultaneously in a wearable index finger rehabilitation exoskeleton.
In those works, which used FT as the metric to observe in EC methods, the improvements achieved in either the method or the results are poorly described: Li et al. [
22] worked with two functions, one to obtain the three contact forces via three phalanges to the object, distributed as evenly as possible, and another to maximize the sum of the forces exerted on the index finger phalanges by the proposed assistive exoskeleton. It is important to note that it only focuses on the objective in the final grasping, not on the entire finger travel as required in rehabilitation therapy. Their EC worked with a population of only 100 individuals, with a stall generation limit set to 500. Du et al. [
23] focused on the transmission angle, a geometrical concept related to FT and mechanism geometry, but they do not detail the improvements achieved after 1000 generations (without detailing the population). Since the objective is to improve FT, it would be much more logical to work on concepts such as mechanical advantage. Iqbal et al. [
24] do not describe their Overall Impact Factor used as a weighted optimization function, and how the FT is included. Sarac et al. [
27] used an optimization function based on the sum of the squared torques applied to the MCP and PIP joints in their design. However, it is unclear how this function accounted for the full range of motion and the characteristics of the population studied. No studies were found using multi-parameter multi-objective optimization methods targeting metrics directly related to optimal FT for RHEx, in which the mechanical advantage is highly variable, and it is important to maintain a proper value for the entire range of motion, not only the final posture.
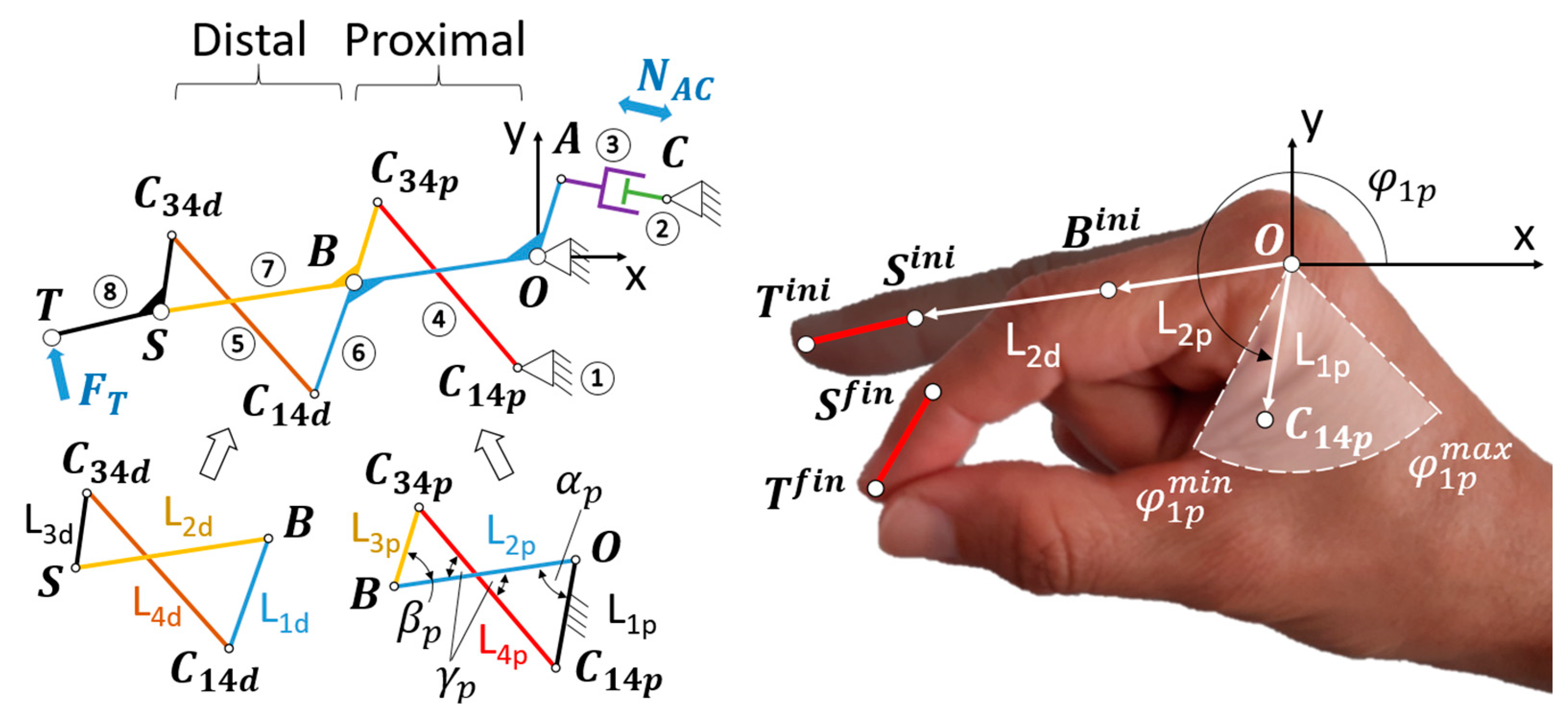
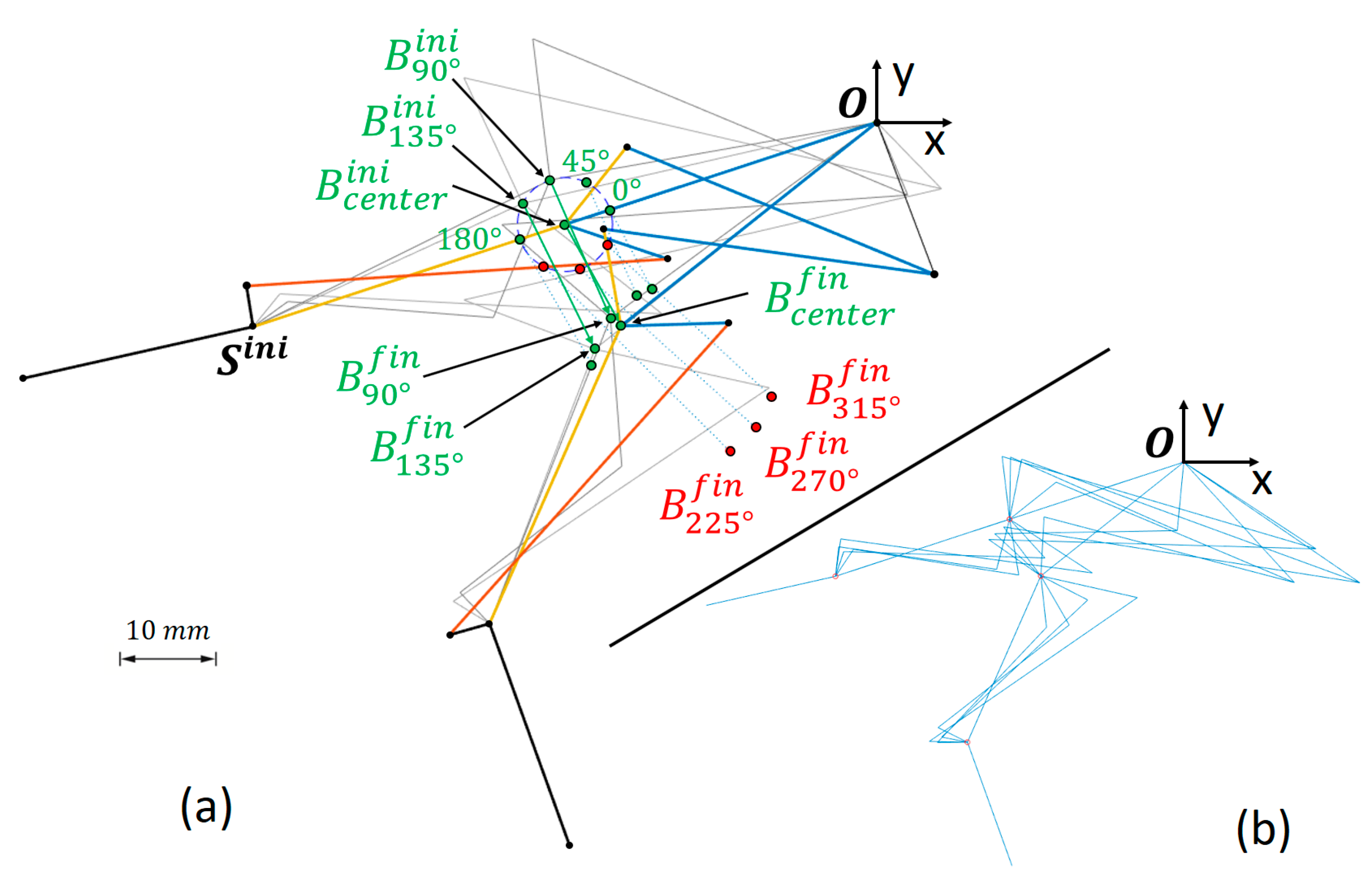
The goal of this work is to design a six-bar linkage intended for implementation in a hand-held RHEx, which can drive the DP from its initial to its final position, mirroring a pulp pinch grasp movement (aka. tip pinch [
42]). This grasp has been documented as the most frequent in ADL [
43,
44]. The study focuses on six-bar mechanisms because this topology is of interest for the conception of RHEx to be placed either on the lateral or on the dorsal side of the index finger, partially mimicking the movement of the phalanges. Extending the design to other fingers would cause a single dorsal four-bar mechanism to interfere with the finger’s dorsum. In the authors’ previous works [
45,
46], a structured exploration of the design space for this linkage was performed to obtain good initial values for subsequent genetic optimization, following a methodology similar to that proposed in [
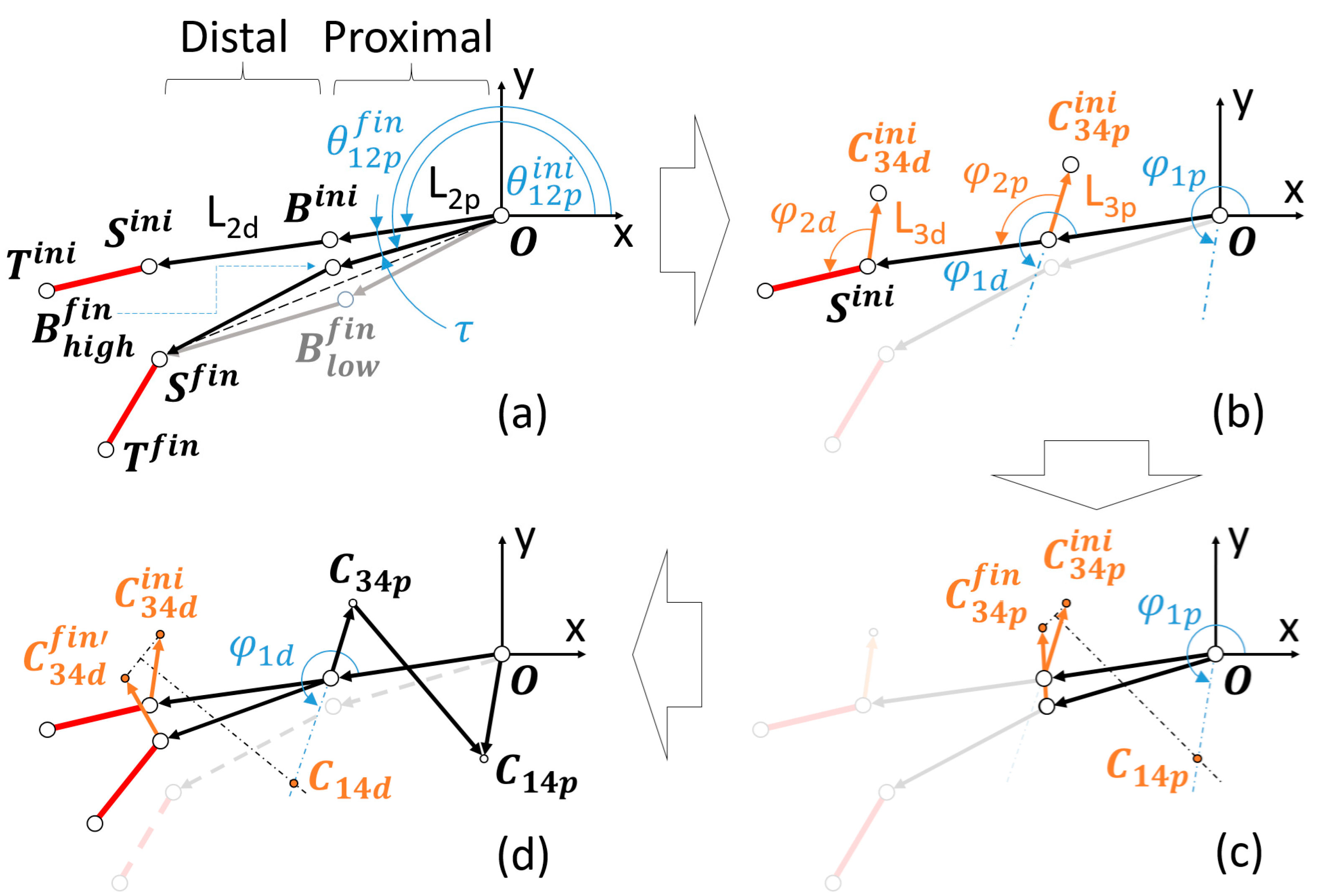
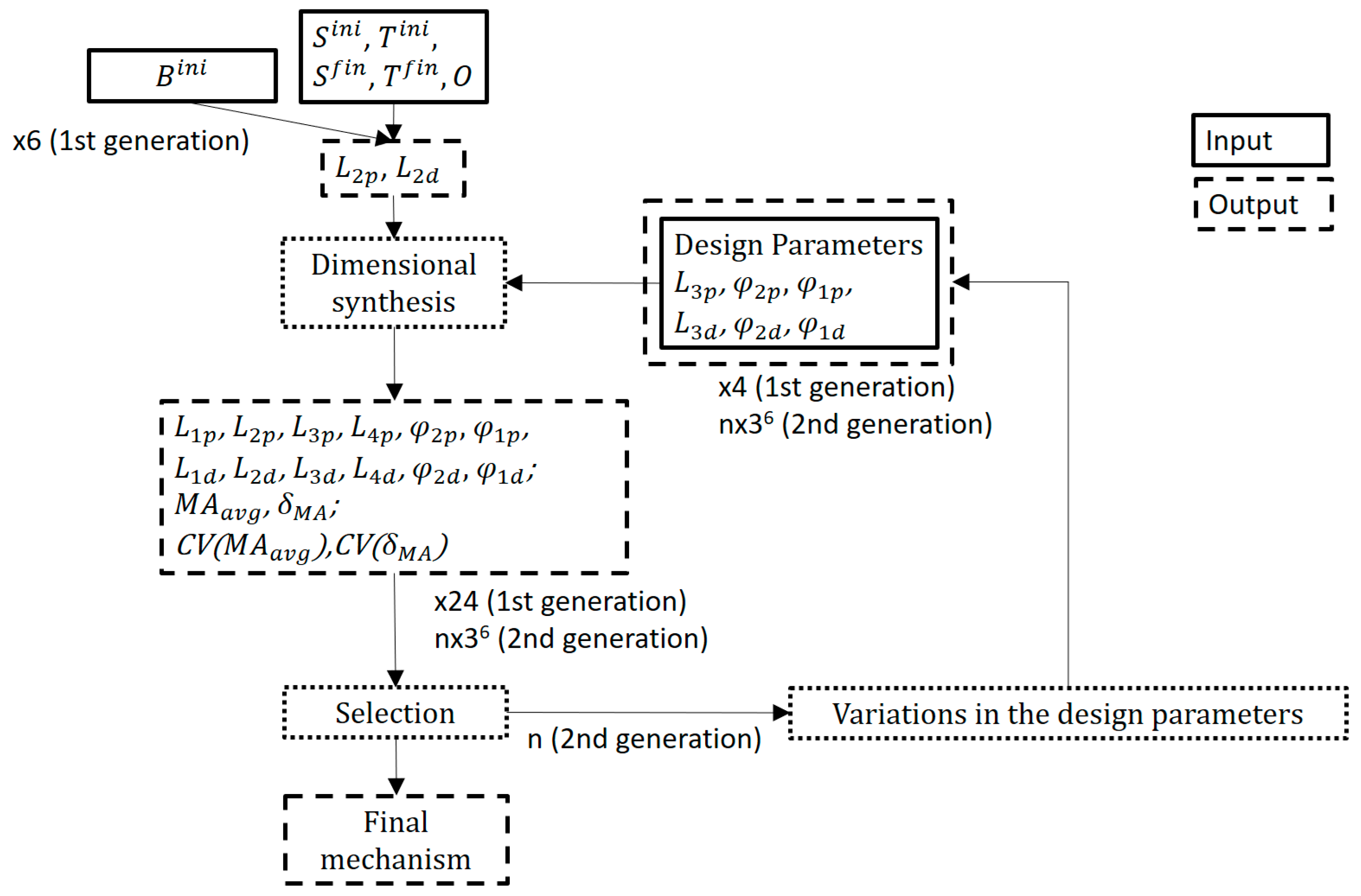
47]. In those works, the design space for this linkage considered 10 free parameters. Three different values were selected for each of these variables, resulting in 59,049 different versions of the six-bar linkage. All these combinations were explored, and only 11,533 were regarded as valid. The maximum mechanical advantage was calculated for each valid solution, which was then used to sort and select the optimal design based on it.
In the present work, and for the optimization, we propose a targeted genetic scheme that reduces the number of generational calculations until an optimal solution is found. This scheme consists of two stages with two different targets, namely: first, the optimal location of a specific joint, and then the optimal dimensions of the linkage. Each one of the stages observed two quality metrics: averaged mechanical advantage along the travel, and its variability. These metrics are often conflicting, meaning improving one may worsen another. The solutions to multi-objective problems are frequently expressed as a set of Pareto optimal solutions, where no solution can be said to be better than another without considering trade-offs. The methodology here proposed lies in the field of ranking-based selection strategies with hierarchical objective prioritization. While it does not directly correspond to a widely recognized standard technique, it can be considered a variation of methods like NSGA-II or SPEA2 (Strength Pareto Evolutionary Algorithm 2) [
48], where objectives are given relative importance instead of being treated entirely equally. According to our knowledge, it has never been applied to optimize the design of RHEx with such metrics, both the mechanical advantage and its variability, both related to FT.
The following section lists the design requirements from the scope of rehabilitation.
Section 2, after meeting the specific requirements of the patient (dimensions and range of movement of the DP) to customize the proposed solution, delves into the mechanism topology selected for the design of the RHEx, as well as the methodology followed for its dimensional synthesis and optimization.
Section 3 shows the results obtained in the current research. The final model is proposed at the end of
Section 3. The last two sections are devoted to discussion and conclusions.
1.1. Design Requirements
In the context of the DERAPPI project [
8], rehabilitators from the Brain Injury Unit of the Hospital La Magdalena (Castellón, Spain) made a detailed list of clinical needs for an RHEx to be integrated into the routine of clinical practice, for the rehabilitation of pulp pinch in subacute stroke patients:
The thumb should be able to perform the opposition movement, or, if in its defect, keep it in opposition to the index finger, to be able to perform the bidigital pulp pinch.
The RHEx should leave the palmar side of the hand and fingers clear so as not to interfere with the sensory stimuli generated when grasping an object and not to excessively favor the flexor pattern of the plegic hand.
The RHEx should leave the wrist joint free to allow for the tenodesis effect in case it is present in the patient, namely, when finger movement involves passive wrist movement.
Leave the pulp of the thumb and index finger free to allow tactile feedback during movement.
The RHEx design must be accessible to different hand sizes.
For the RHEx to be safe in stroke patients, some biomechanical restrictions must also be verified in relation to the forces applied by the device: the use of linear actuators is justified compared to a more economical hydraulic system, since they allow for more detailed control of the movement, joint ranges, and pressure exerted in the pulp pinch movement.
From the observations made, it was concluded that the minimum force required to guide the distal phalanx of the index finger should be approximately (not less than) 5 N (≈0.5 kgf) in a closing sequence of 1 s duration.
As listed above, thumb abduction is required to perform the pulp pinch. In the abducted position, the thumb can oppose the fingertips [
42]. Since including this movement would add complexity and therefore weight and cost to the exoskeleton, it was decided that the exoskeleton structure itself would keep the thumb permanently in opposition to allow the pinch without any specific actuators for it, thus reducing the number of actuators needed without compromising the rehabilitation movement.
4. Discussion
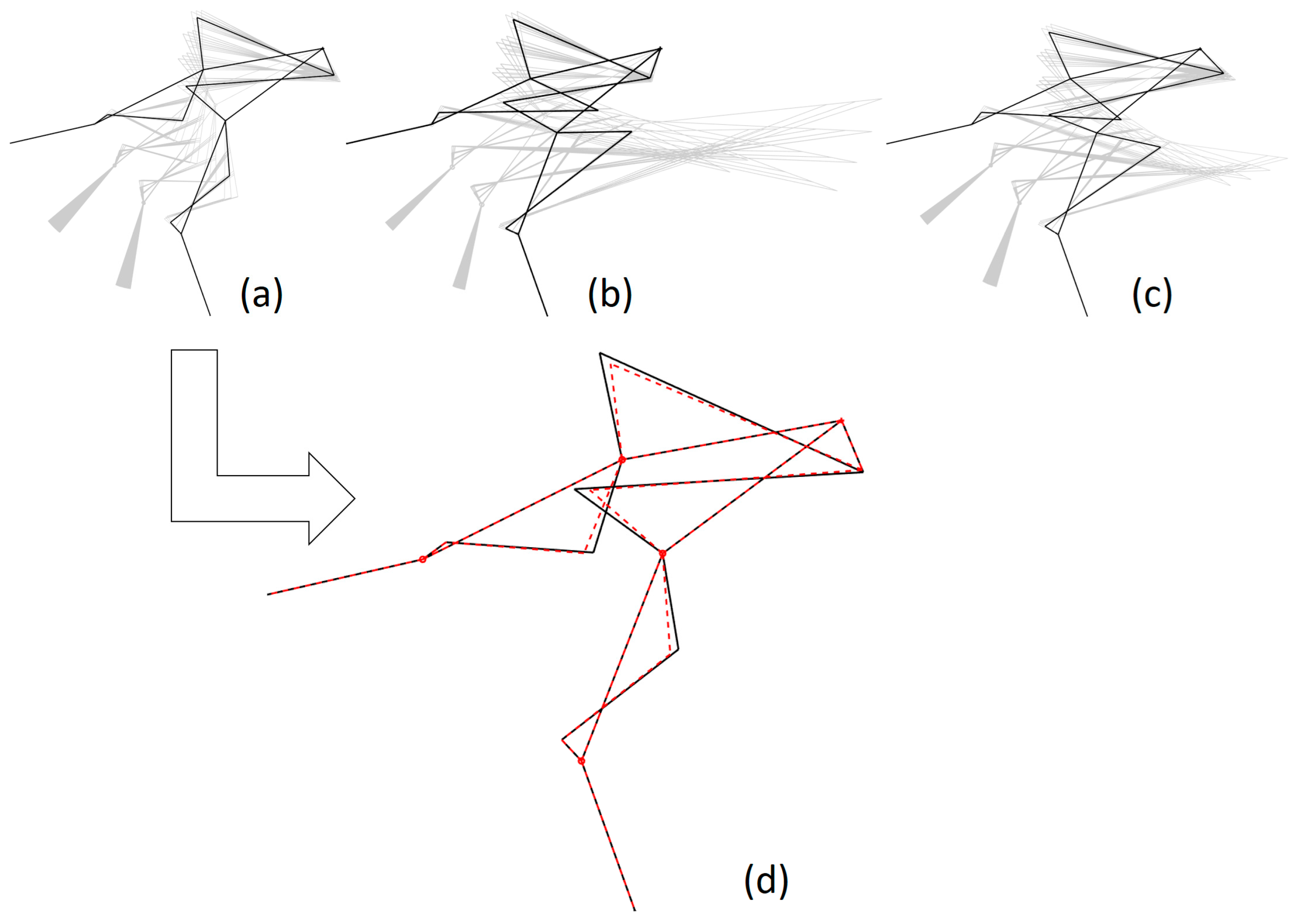
The focus on improving the quality of life and rehabilitation prospects for post-stroke patients has become increasingly significant in recent years. Hand exoskeletons, including assistive ones, should be as compact and lightweight as possible to enhance wearability. Underactuated designs, like the one examined in this study, are well-suited for this goal, as they aim for compact actuator sizes while delivering high output forces (relative to the actuator’s size) and ensuring efficient power transmission through the links. Despite progress, research on exoskeletons with enhanced mechanical advantages and ergonomics still requires revisions and refinements to meet these specific needs. The scanning process, along with the mechanism optimization presented here, are essential tools for developing custom-made, optimal mechanisms tailored to each patient.
The results of this research have focused on a 6-bar topology which is versatile for the design of exoskeletons both for the whole hand (in the case of a dorsal layout) and for index finger rehabilitation, as has been the case (with a more compact lateral layout).
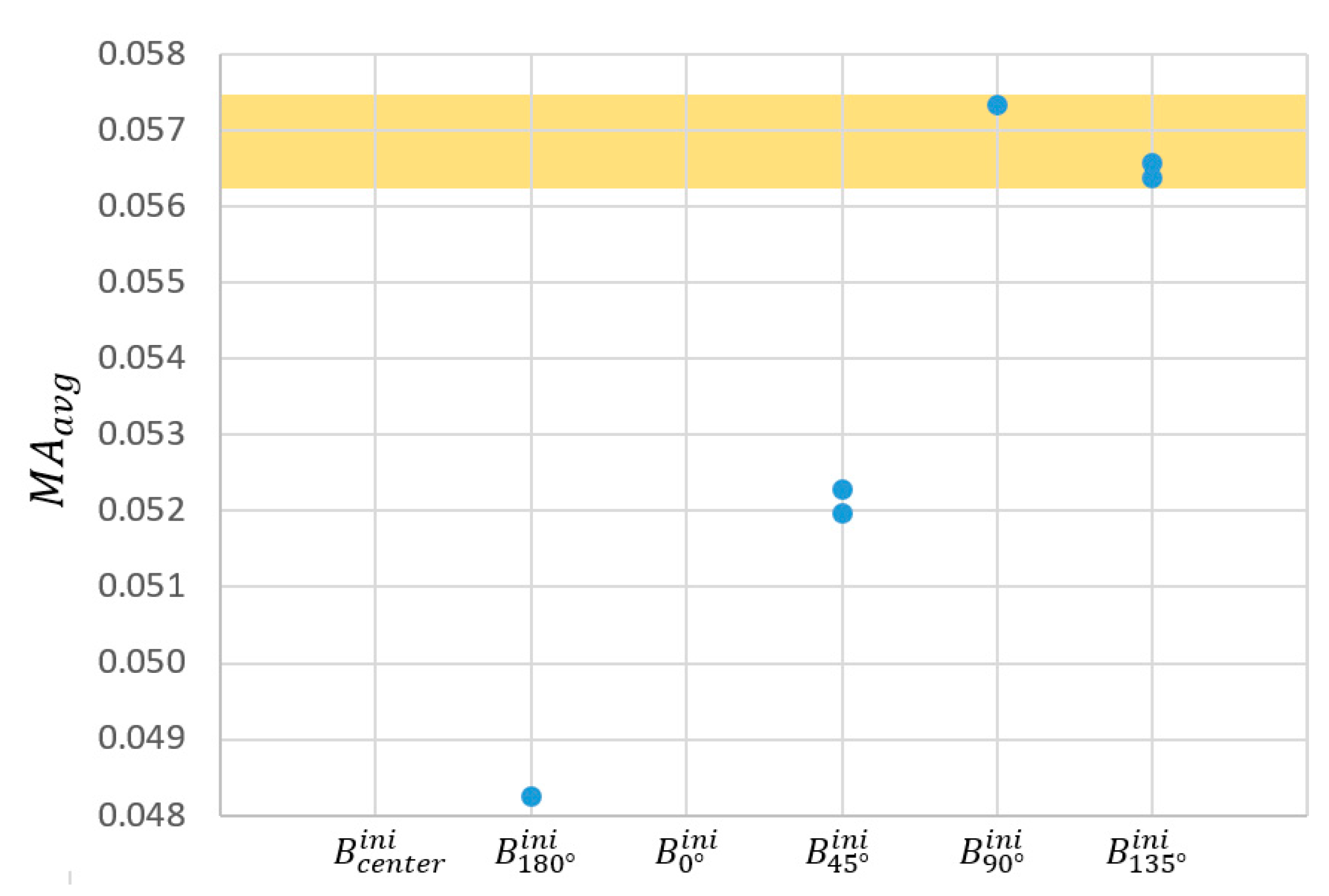
The integration of design and optimization techniques raises an important question: how do engineers determine the most suitable method or algorithm for optimizing different design criteria. To address these challenges, alternative optimization approaches have been developed, including nature-inspired methods from evolutionary computation (EC). The strategy followed in this research provides design procedures applicable to similar exoskeletons. First, the search for the optimal location for the concatenation joint of the four-bar mechanisms (B-joint), and second, the search for correlations on the design variables (the location coordinates of the B-joint included). We have worked with quality metrics that are of interest for the performance of the task, such as
and
. It should be noted that the lack of correlations between the variation of the design parameters and the quality metrics evidenced the high non-linearity of the system. Only the coordinates of the B-joint showed some significance. All things considered, these metrics have allowed us to obtain a mechanism with a quasi-constant
value across its travel, with an average value (
) higher than the result of our previous studies [
45,
46] mentioned earlier (this former design had values of
at the final posture and
at the initial posture, calculated according to Equation (3), with
).
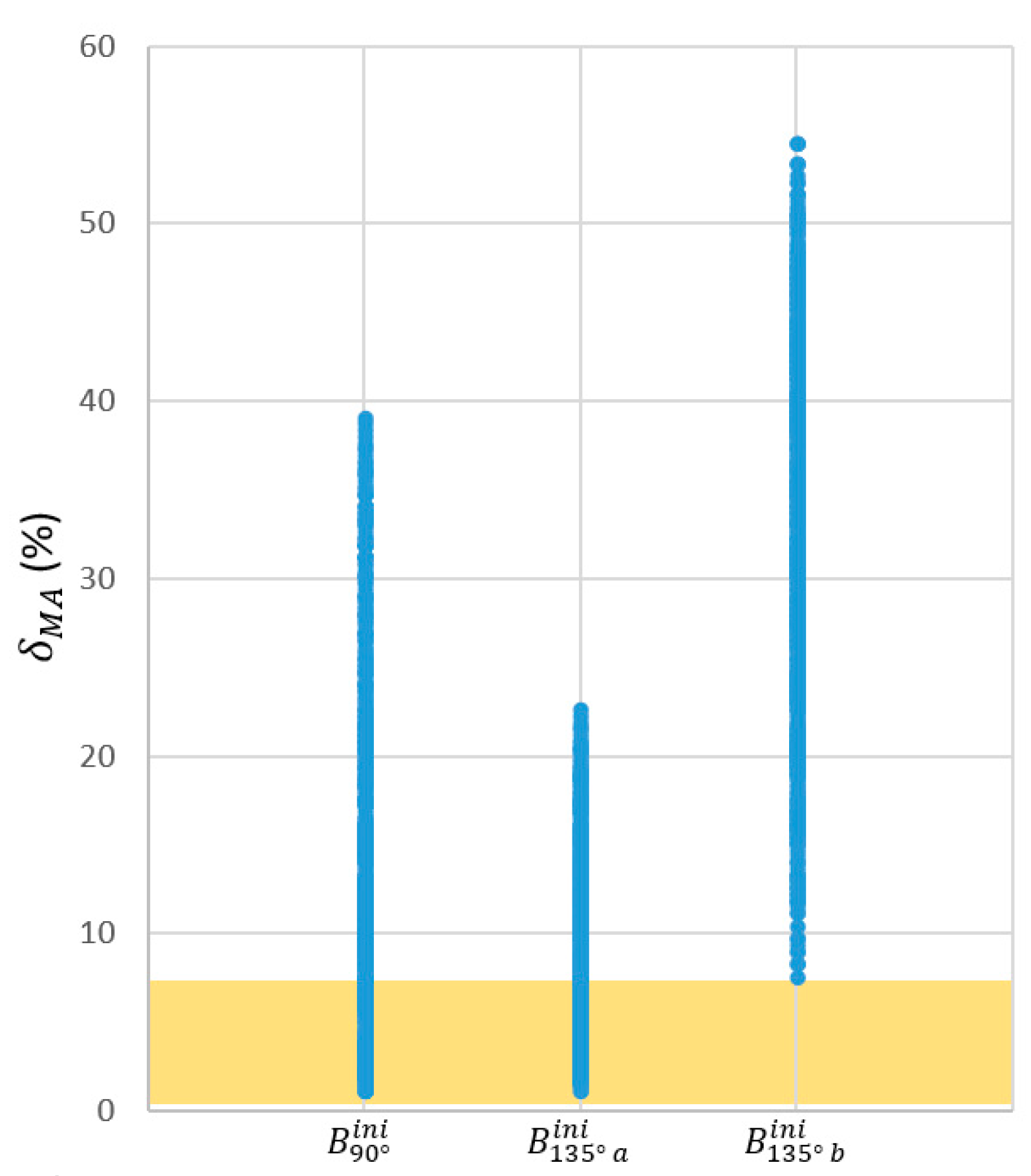
By targeting genetic optimization schemes, we reduced the number of iterative or generational calculations required to reach an optimal solution. In this case, compared to a previous optimization for the same problem [
45,
46], we only evaluated 2211 different mechanisms, instead of 59,049. This signifies the elimination of 96.2% of the cases that were randomly generated in those studies.