Enhanced Range and Endurance Evaluation of a Camber Morphing Wing Aircraft
Abstract
1. Introduction
2. Motivation
3. Problem Statement
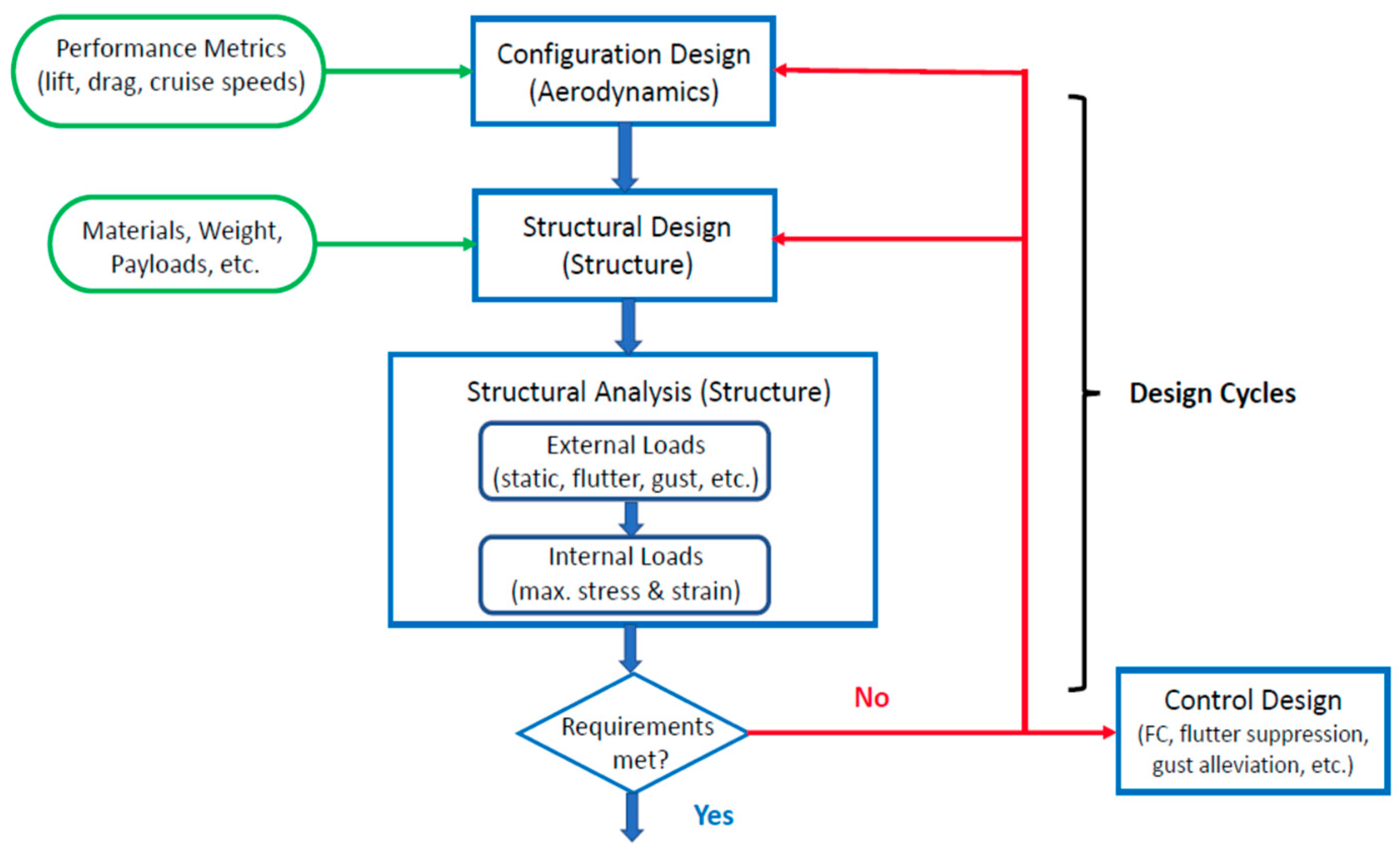
4. Computational Model
5. Methodology
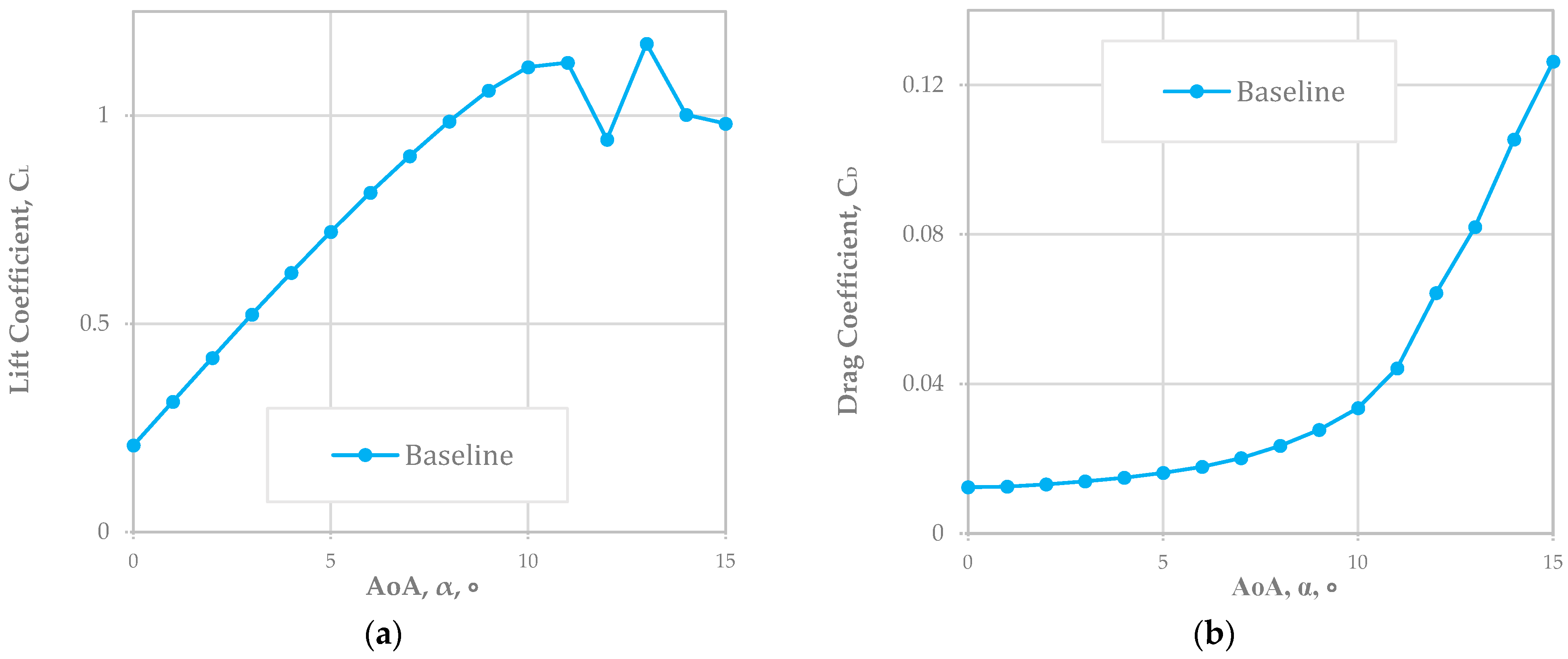
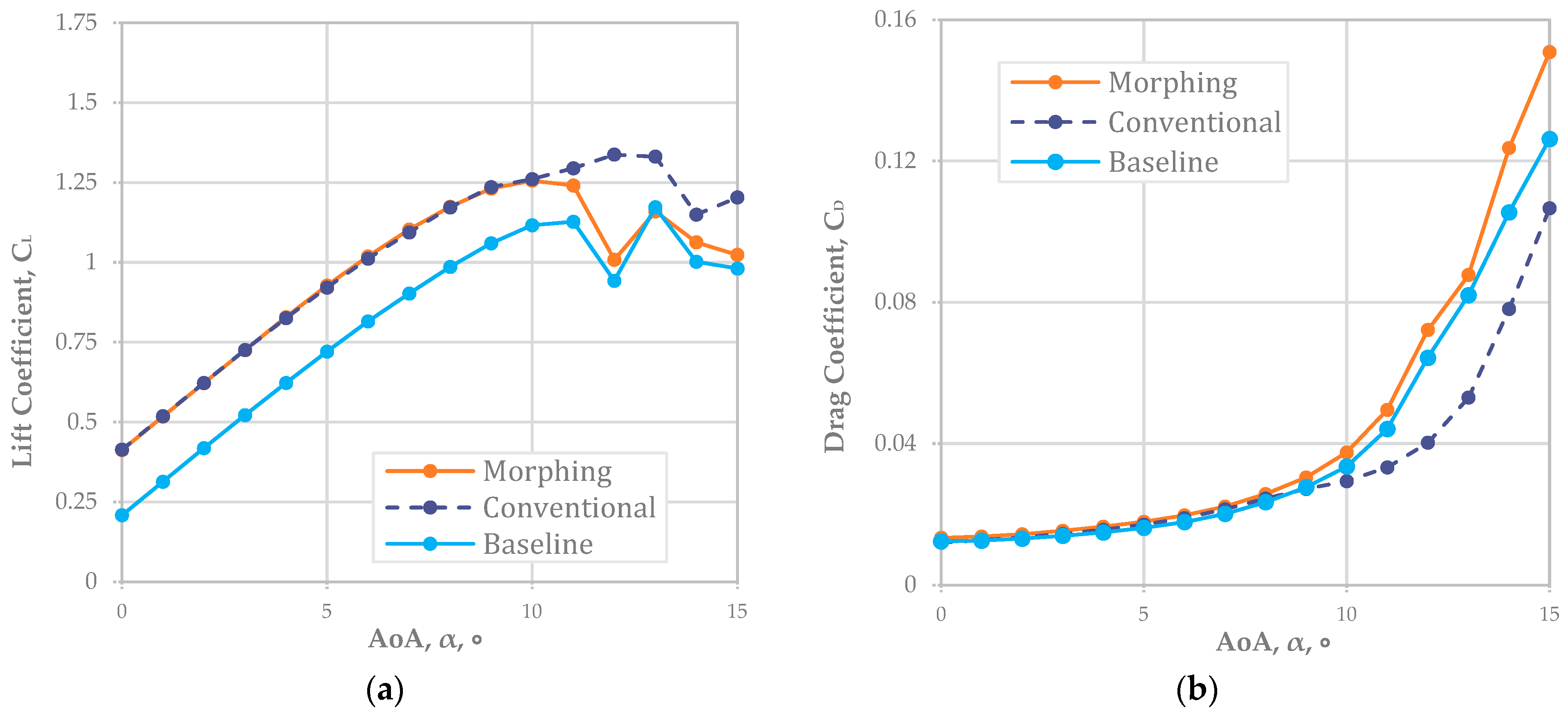
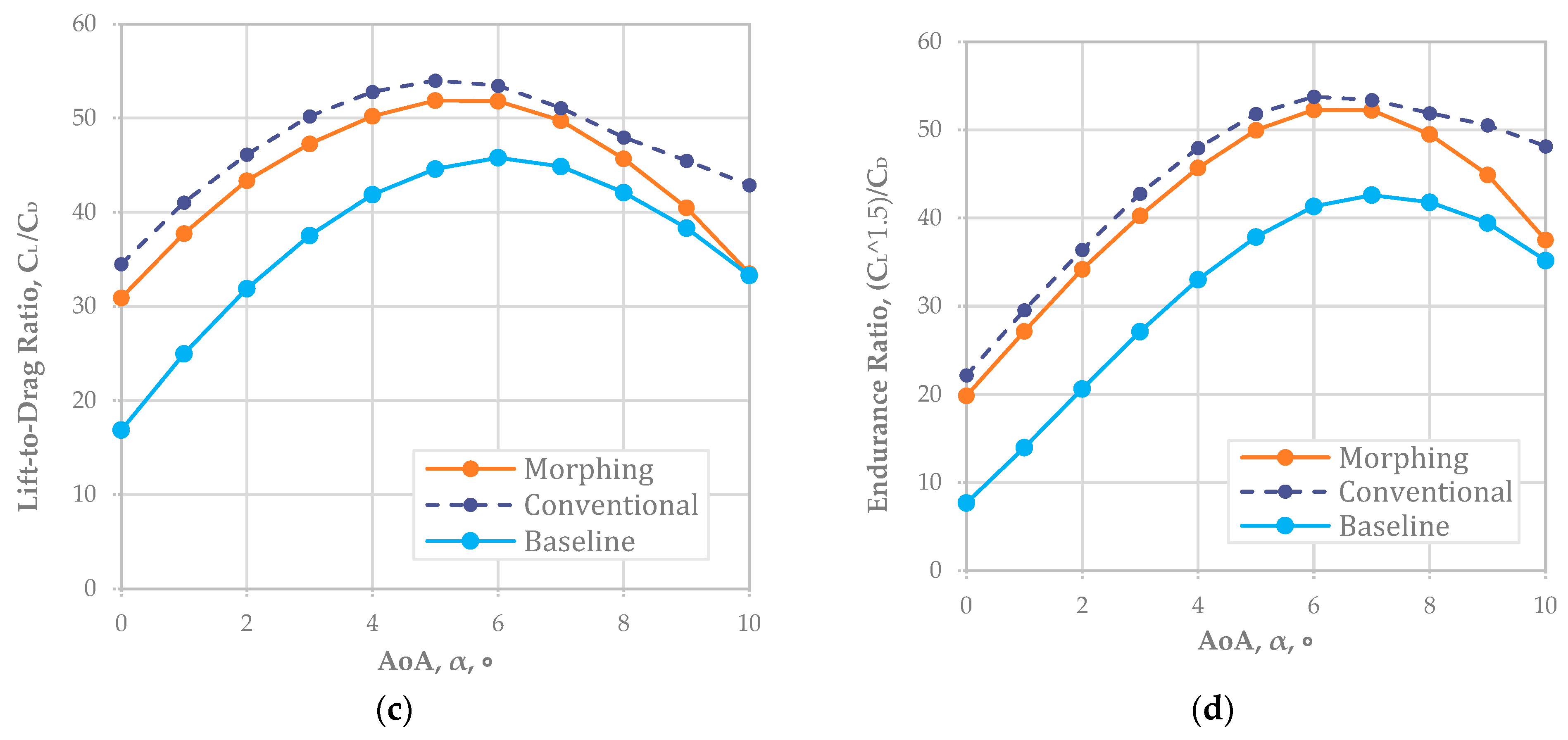
6. Results
7. Summary
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Nomenclature | |
| L | Lift force |
| D | Drag force |
| Lift coefficient | |
| Drag coefficient | |
| AoA | Angle of attack |
| Skin friction coefficient | |
| Distance between the airfoil wall and the first layer | |
| Re | Reynolds number |
| Cruise velocity | |
| Greek Symbols | |
| μ | Dynamic viscosity |
| ρ | Density of air |
| Shear velocity | |
| von Kármán constant | |
| Abbreviations | |
| CFD | Computational fluid dynamics |
| RANS | Reynolds-averaged Navier–Stokes |
| EWT | Enhanced wall treatment |
| NACA | National Advisory Committee for Aeronautics |
References
- Bishop, C.; Erezyilmaz, D.; Flatt, T.; Georgiou, C.; Hadfield, M.; Heyland, A.; Hodin, J.; Jacobs, M.; Maslakova, S.; Pires, A. What is metamorphosis? Integr. Comp. Biol. 2006, 46, 655–661. [Google Scholar] [CrossRef]
- Dodd, M.; Dodd, J. The biology of metamorphosis. Physiol. Amphib. 1976, 3, 467–599. [Google Scholar]
- Harvey, C.; Gamble, L.L.; Bolander, C.R.; Hunsaker, D.F.; Joo, J.J.; Inman, D.J. A review of avian-inspired morphing for UAV flight control. Prog. Aerosp. Sci. 2022, 132, 100825. [Google Scholar] [CrossRef]
- Truman, J.W.; Riddiford, L.M. The origins of insect metamorphosis. Nature 1999, 401, 447–452. [Google Scholar] [CrossRef]
- Sofla, A.; Meguid, S.; Tan, K.; Yeo, W. Shape morphing of aircraft wing: Status and challenges. Mater. Des. 2010, 31, 1284–1292. [Google Scholar] [CrossRef]
- Barbarino, S.; Bilgen, O.; Ajaj, R.M.; Friswell, M.I.; Inman, D.J. A review of morphing aircraft. J. Intell. Mater. Syst. Struct. 2011, 22, 823–877. [Google Scholar] [CrossRef]
- Ameduri, S.; Concilio, A. Morphing wings review: Aims, challenges, and current open issues of a technology. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2020. [Google Scholar] [CrossRef]
- Guo, J.; Zhao, C.; Song, Z. Discussion on research status and key technologies of morphing aircraft. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2021; p. 012021. [Google Scholar]
- Jha, A.K.; Kudva, J.N. Morphing aircraft concepts, classifications, and challenges. In Proceedings of the Smart Structures and Materials 2004: Industrial and Commercial Applications of Smart Structures Technologie, San Diego, CA, USA, 14–18 March 2004; International Society for Optics and Photonics: Bellingham, WA, USA, 2004; pp. 213–224. [Google Scholar]
- Reich, G.; Sanders, B. Introduction to morphing aircraft research. J. Aircr. 2007, 44, 1059. [Google Scholar] [CrossRef]
- Rodriguez, A. Morphing aircraft technology survey. In Proceedings of the 45th AIAA Aerospace Sciences Meeting and Exhibit, Reno, Nevada, 8–11 January 2007; p. 1258. [Google Scholar]
- Tsushima, N.; Tamayama, M. Recent researches on morphing aircraft technologies in Japan and other countries. Mech. Eng. Rev. 2019, 6, 19–00197. [Google Scholar] [CrossRef]
- Weisshaar, T.A. Morphing Aircraft Technology—New Shapes for Aircraft Design; Defense Technical Information Center: Fort Belvoir, VA, USA, 2006. [Google Scholar]
- Weisshaar, T.A. Morphing aircraft systems: Historical perspectives and future challenges. J. Aircr. 2013, 50, 337–353. [Google Scholar] [CrossRef]
- Ajaj, R.M.; Beaverstock, C.S.; Friswell, M.I. Morphing aircraft: The need for a new design philosophy. Aerosp. Sci. Technol. 2016, 49, 154–166. [Google Scholar] [CrossRef]
- Gomez, J.C.; Garcia, E. Morphing unmanned aerial vehicles. Smart Mater. Struct. 2011, 20, 103001. [Google Scholar] [CrossRef]
- Martin, J.; Heyder-Bruckner, J.J.; Remillat, C.; Scarpa, F.; Potter, K.; Ruzzene, M. The hexachiral prismatic wingbox concept. Phys. Status Solidi B 2008, 245, 570–577. [Google Scholar] [CrossRef]
- McGowan, A.-M.R.; Vicroy, D.D.; Busan, R.C.; Hahn, A.S. Perspectives on Highly Adaptive or Morphing Aircraft. In the Symposium, NATO RTO AVT-169, No. RTO-MP-AVT-168, 2009. Available online: https://ntrs.nasa.gov/citations/20090017845 (accessed on 4 January 2023).
- Parker, H. The Parker Variable Camber Wing. Technical Report, No. NACA-TR-77. 1920. Available online: https://digital.library.unt.edu/ark:/67531/metadc65726/ (accessed on 4 January 2023).
- Pettit, G.W.; Robertshaw, H.H.; Inman, D.J. Morphing wings for unmanned aircraft. Smart Mater. Bull. 2001, 2001, 7–12. [Google Scholar] [CrossRef]
- Szodruch, J.; Hilbig, R. Variable wing camber for transport aircraft. Prog. Aerosp. Sci. 1988, 25, 297–328. [Google Scholar] [CrossRef]
- Panagiotou, P.; Yakinthos, K. Aerodynamic efficiency and performance enhancement of fixed-wing UAVs. Aerosp. Sci. Technol. 2020, 99, 105575. [Google Scholar] [CrossRef]
- Previtali, F.; Arrieta, A.F.; Ermanni, P. Performance of a three-dimensional morphing wing and comparison with a conventional wing. AIAA J. 2014, 52, 2101–2113. [Google Scholar] [CrossRef]
- Béguin, B.; Breitsamter, C. Effects of membrane pre-stress on the aerodynamic characteristics of an elasto-flexible morphing wing. Aerosp. Sci. Technol. 2014, 37, 138–150. [Google Scholar] [CrossRef]
- Della Vecchia, P.; Corcione, S.; Pecora, R.; Nicolosi, F.; Dimino, I.; Concilio, A. Design and integration sensitivity of a morphing trailing edge on a reference airfoil: The effect on high-altitude long-endurance aircraft performance. J. Intell. Mater. Syst. Struct. 2017, 28, 2933–2946. [Google Scholar] [CrossRef]
- Kuya, Y.; Ito, R.; Maki, M.; Sawada, K. Numerical Study of Flowfield Around a Multislotted High-Lift Wing. J. Aircr. 2021, 58, 383–389. [Google Scholar] [CrossRef]
- Molinari, G.; Arrieta, A.F.; Guillaume, M.; Ermanni, P. Aerostructural performance of distributed compliance morphing wings: Wind tunnel and flight testing. AIAA J. 2016, 54, 3859–3871. [Google Scholar] [CrossRef]
- Pankonien, A.M.; Faria, C.T.; Inman, D.J. Synergistic smart morphing aileron: Experimental quasi-static performance characterization. J. Intell. Mater. Syst. Struct. 2015, 26, 1179–1190. [Google Scholar] [CrossRef]
- Pecora, R.; Amoroso, F.; Lecce, L. Effectiveness of wing twist morphing in roll control. J. Aircr. 2012, 49, 1666–1674. [Google Scholar] [CrossRef]
- Vale, J.; Leite, A.; Lau, F.; Suleman, A. Aero-structural optimization and performance evaluation of a morphing wing with variable span and camber. J. Intell. Mater. Syst. Struct. 2011, 22, 1057–1073. [Google Scholar] [CrossRef]
- Vasista, S.; Riemenschneider, J.; Van De Kamp, B.; Monner, H.P.; Cheung, R.C.; Wales, C.; Cooper, J.E. Evaluation of a compliant droop-nose morphing wing tip via experimental tests. J. Aircr. 2017, 54, 519–534. [Google Scholar] [CrossRef]
- Bashir, M.; Longtin-Martel, S.; Botez, R.M.; Wong, T. Optimization and Design of a Flexible Droop-Nose Leading-Edge Morphing Wing Based on a Novel Black Widow Optimization Algorithm—Part I. Designs 2022, 6, 10. [Google Scholar] [CrossRef]
- Dexl, F.; Hauffe, A.; Wolf, K. Comparison of structural parameterization methods for the multidisciplinary optimization of active morphing wing sections. Comput. Struct. 2022, 263, 106743. [Google Scholar] [CrossRef]
- Koreanschi, A.; Gabor, O.S.; Acotto, J.; Brianchon, G.; Portier, G.; Botez, R.M.; Mamou, M.; Mebarki, Y. Optimization and design of an aircraft’s morphing wing-tip demonstrator for drag reduction at low speeds, Part II-Experimental validation using Infra-Red transition measurement from Wind Tunnel tests. Chin. J. Aeronaut. 2017, 30, 164–174. [Google Scholar] [CrossRef]
- Liu, B.; Liang, H.; Han, Z.-H.; Yang, G. Surrogate-based aerodynamic shape optimization of a morphing wing considering a wide Mach-number range. Aerosp. Sci. Technol. 2022, 124, 107557. [Google Scholar] [CrossRef]
- Murugan, S.; Woods, B.; Friswell, M. Hierarchical modeling and optimization of camber morphing airfoil. Aerosp. Sci. Technol. 2015, 42, 31–38. [Google Scholar] [CrossRef]
- Andrejašič, M.; Eržen, D.; Costa, E.; Porziani, S.; Biancolini, M.; Groth, C. A mesh morphing based FSI method used in aeronautical optimization applications. In Proceedings of the ECCOMAS Congress, VII European Congress on Computational Methods in Applied Sciences and Engineering, Crete Island, Greece, 5–10 June 2016. [Google Scholar]
- Gabor, O.Ş.; Koreanschi, A.; Botez, R.M. A new non-linear vortex lattice method: Applications to wing aerodynamic optimizations. Chin. J. Aeronaut. 2016, 29, 1178–1195. [Google Scholar] [CrossRef]
- Namgoong, H.; Crossley, W.A.; Lyrintzis, A.S. Aerodynamic optimization of a morphing airfoil using energy as an objective. AIAA J. 2007, 45, 2113–2124. [Google Scholar] [CrossRef]
- Burdette, D.A.; Kenway, G.K.; Lyu, Z.; Martins, J.R. Aerostructural design optimization of an adaptive morphing trailing edge wing. In Proceedings of the 56th AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Kissimmee, FL, USA, 5–9 January 2015; p. 1129. [Google Scholar]
- Keidel, D.; Molinari, G.; Ermanni, P. Aero-structural optimization and analysis of a camber-morphing flying wing: Structural and wind tunnel testing. J. Intell. Mater. Syst. Struct. 2019, 30, 908–923. [Google Scholar] [CrossRef]
- Molinari, G.; Arrieta, A.F.; Ermanni, P. Aero-structural optimization of three-dimensional adaptive wings with embedded smart actuators. AIAA J. 2014, 52, 1940–1951. [Google Scholar] [CrossRef]
- De Gaspari, A.; Riccobene, L.; Ricci, S. Design, manufacturing and wind tunnel validation of a morphing compliant wing. J. Aircr. 2018, 55, 2313–2326. [Google Scholar] [CrossRef]
- Fasel, U.; Keidel, D.; Baumann, L.; Cavolina, G.; Eichenhofer, M.; Ermanni, P. Composite additive manufacturing of morphing aerospace structures. Manuf. Lett. 2020, 23, 85–88. [Google Scholar] [CrossRef]
- Yang, B.; Ouyang, J.; Li, X. Simulation of fiber reinforced composite materials mold filling process and mechanical properties analysis. J. Non-Newton. Fluid Mech. 2011, 166, 1129–1136. [Google Scholar] [CrossRef]
- Somnic, J.; Jo, B.W. Status and Challenges in Homogenization Methods for Lattice Materials. Materials 2022, 15, 605. [Google Scholar] [CrossRef]
- Arrieta, A.F.; Kuder, I.K.; Waeber, T.; Ermanni, P. Variable stiffness characteristics of embeddable multi-stable composites. Compos. Sci. Technol. 2014, 97, 12–18. [Google Scholar] [CrossRef]
- Barbarino, S.; Flores, E.S.; Ajaj, R.M.; Dayyani, I.; Friswell, M.I. A review on shape memory alloys with applications to morphing aircraft. Smart Mater. Struct. 2014, 23, 063001. [Google Scholar] [CrossRef]
- Papadrakakis, M.; Stefanou, G. Multiscale Modeling and Uncertainty Quantification of Materials and Structures; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Sun, J.; Guan, Q.; Liu, Y.; Leng, J. Morphing aircraft based on smart materials and structures: A state-of-the-art review. J. Intell. Mater. Syst. Struct. 2016, 27, 2289–2312. [Google Scholar] [CrossRef]
- Tong, X.; Ge, W.; Sun, C.; Liu, X. Topology optimization of compliant adaptive wing leading edge with composite materials. Chin. J. Aeronaut. 2014, 27, 1488–1494. [Google Scholar] [CrossRef]
- Katagiri, K.; Park, C.S.; Kawakita, S.; Kim, D.; Tamayama, M.; Honda, S.; Sasaki, K.; Yamazaki, M. Mechanical properties of the skeletal structure for UAV morphing wing by using CFRP with applying the electrodeposition resin molding method. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 0865. [Google Scholar]
- Rivero, A.E.; Weaver, P.M.; Cooper, J.E.; Woods, B.K. Structural Modeling of Compliance-Based Camber Morphing Structures Under Transverse Shear Loading. AIAA J. 2020, 58, 4941–4951. [Google Scholar] [CrossRef]
- Jenett, B.; Calisch, S.; Cellucci, D.; Cramer, N.; Gershenfeld, N.; Swei, S.; Cheung, K.C. Digital morphing wing: Active wing shaping concept using composite lattice-based cellular structures. Soft Robot. 2017, 4, 33–48. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Zhang, Q.; Scarpa, F.; Liu, Y.; Leng, J. In-plane elasticity of a novel auxetic honeycomb design. Compos. Part B Eng. 2017, 110, 72–82. [Google Scholar] [CrossRef]
- Zhou, J.; Guan, Z.; Cantwell, W. Scaling effects in the mechanical response of sandwich structures based on corrugated composite cores. Compos. Part B Eng. 2016, 93, 88–96. [Google Scholar] [CrossRef]
- Woods, B.K.; Friswell, M.I. Multi-objective geometry optimization of the Fish Bone Active Camber morphing airfoil. J. Intell. Mater. Syst. Struct. 2016, 27, 808–819. [Google Scholar] [CrossRef]
- Vasista, S.; De Gaspari, A.; Ricci, S.; Riemenschneider, J.; Monner, H.P.; van de Kamp, B. Compliant structures-based wing and wingtip morphing devices. Aircr. Eng. Aerosp. Technol. Int. J. 2016, 88, 311–330. [Google Scholar] [CrossRef]
- Takahashi, H.; Yokozeki, T.; Hirano, Y. Development of variable camber wing with morphing leading and trailing sections using corrugated structures. J. Intell. Mater. Syst. Struct. 2016, 27, 2827–2836. [Google Scholar] [CrossRef]
- Barbarino, S.; Pecora, R.; Lecce, L.; Concilio, A.; Ameduri, S.; De Rosa, L. Airfoil structural morphing based on SMA actuator series: Numerical and experimental studies. J. Intell. Mater. Syst. Struct. 2011, 22, 987–1004. [Google Scholar] [CrossRef]
- Basaeri, H.; Yousefi-Koma, A.; Zakerzadeh, M.R.; Mohtasebi, S.S. Experimental study of a bio-inspired robotic morphing wing mechanism actuated by shape memory alloy wires. Mechatronics 2014, 24, 1231–1241. [Google Scholar] [CrossRef]
- Bil, C.; Massey, K.; Abdullah, E.J. Wing morphing control with shape memory alloy actuators. J. Intell. Mater. Syst. Struct. 2013, 24, 879–898. [Google Scholar] [CrossRef]
- Brailovski, V.; Terriault, P.; Georges, T.; Coutu, D. SMA actuators for morphing wings. Phys. Procedia 2010, 10, 197–203. [Google Scholar] [CrossRef]
- Icardi, U.; Ferrero, L. Preliminary study of an adaptive wing with shape memory alloy torsion actuators. Mater. Des. 2009, 30, 4200–4210. [Google Scholar] [CrossRef]
- Kang, W.-R.; Kim, E.-H.; Jeong, M.-S.; Lee, I.; Ahn, S.-M. Morphing wing mechanism using an SMA wire actuator. Int. J. Aeronaut. Space Sci. 2012, 13, 58–63. [Google Scholar] [CrossRef]
- Karagiannis, D.; Stamatelos, D.; Kappatos, V.; Spathopoulos, T. An investigation of shape memory alloys as actuating elements in aerospace morphing applications. Mech. Adv. Mater. Struct. 2017, 24, 647–657. [Google Scholar] [CrossRef]
- Ko, S.-H.; Bae, J.-S.; Rho, J.-H. Development of a morphing flap using shape memory alloy actuators: The aerodynamic characteristics of a morphing flap. Smart Mater. Struct. 2014, 23, 074015. [Google Scholar] [CrossRef]
- Communier, D.; Botez, R.M.; Wong, T. Design and Validation of a New Morphing Camber System by Testing in the Price—Païdoussis Subsonic Wind Tunnel. Aerospace 2020, 7, 23. [Google Scholar] [CrossRef]
- Rivero, A.E.; Weaver, P.M.; Cooper, J.E.; Woods, B.K. Parametric structural modelling of fish bone active camber morphing aerofoils. J. Intell. Mater. Syst. Struct. 2018, 29, 2008–2026. [Google Scholar] [CrossRef]
- Li, D.; Zhao, S.; Da Ronch, A.; Xiang, J.; Drofelnik, J.; Li, Y.; Zhang, L.; Wu, Y.; Kintscher, M.; Monner, H.P. A review of modelling and analysis of morphing wings. Prog. Aerosp. Sci. 2018, 100, 46–62. [Google Scholar] [CrossRef]
- Chanzy, Q.; Keane, A. Analysis and experimental validation of morphing UAV wings. Aeronaut. J. 2018, 122, 390–408. [Google Scholar] [CrossRef]
- Jo, B.W.; Majid, T. Aerodynamic Analysis of Camber Morphing Airfoils in Transition via Computational Fluid Dynamics. Biomimetics 2022, 7, 52. [Google Scholar] [CrossRef] [PubMed]
- Majid, T.; Jo, B.W. Comparative Aerodynamic Performance Analysis of Camber Morphing and Conventional Airfoils. Appl. Sci. 2021, 11, 10663. [Google Scholar] [CrossRef]
- Kumar, T.; Venugopal, S.; Ramakrishnananda, B.; Vijay, S. Aerodynamic performance estimation of camber morphing airfoils for small unmanned aerial vehicle. J. Aerosp. Technol. Manag. 2020, 12. [Google Scholar] [CrossRef]
- Eguea, J.P.; da Silva, G.P.G.; Catalano, F.M. Fuel efficiency improvement on a business jet using a camber morphing winglet concept. Aerosp. Sci. Technol. 2020, 96, 105542. [Google Scholar] [CrossRef]
- Sato, K.; Yokozeki, T. Aero-structural evaluation of morphing control surface using corrugated panels. Trans. Jpn. Soc. Aeronaut. Space Sci. Aerosp. Technol. Jpn. 2017, 15, a7–a15. [Google Scholar] [CrossRef]
- Alsulami, A.; Akbar, M.; Joe, W.Y. A Comparative Study: Aerodynamics of Morphed Airfoils Using CFD Techniques and Analytical Tools. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Tampa, FL, USA, 3–9 November 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; p. V001T003A010. [Google Scholar]
- Sabri, F.; Elzaabalawy, A.; Meguid, S. Aeroelastic behaviour of a flexible morphing wing design for unmanned aerial vehicle. Acta Mech. 2022, 233, 851–867. [Google Scholar] [CrossRef]
- Gillebaart, E.; De Breuker, R. Low-fidelity 2D isogeometric aeroelastic analysis and optimization method with application to a morphing airfoil. Comput. Methods Appl. Mech. Eng. 2016, 305, 512–536. [Google Scholar] [CrossRef]
- Woods, B.K.; Dayyani, I.; Friswell, M.I. Fluid/structure-interaction analysis of the fish-bone-active-camber morphing concept. J. Aircr. 2015, 52, 307–319. [Google Scholar] [CrossRef]
- Kammegne, M.J.T.; Botez, R.M.; Grigorie, L.T.; Mamou, M.; Mébarki, Y. Proportional fuzzy feed-forward architecture control validation by wind tunnel tests of a morphing wing. Chin. J. Aeronaut. 2017, 30, 561–576. [Google Scholar] [CrossRef]
- Li, D.; Guo, S.; Aburass, T.O.; Yang, D.; Xiang, J. Active control design for an unmanned air vehicle with a morphing wing. Aircr. Eng. Aerosp. Technol. Int. J. 2016, 88, 168–177. [Google Scholar] [CrossRef]
- Shi, R.; Song, J.; Wan, W. Active disturbance rejection control system design for a morphing wing structure. Asian J. Control. 2015, 17, 832–841. [Google Scholar] [CrossRef]
- Keidel, D.; Fasel, U.; Molinari, G.; Ermanni, P. Design, Development, and Structural Testing of a Camber-Morphing Flying Wing Airplane. In Proceedings of the Smart Materials, Adaptive Structures and Intelligent Systems, Snowbird, UT, USA, 18–20 September 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; p. V002T004A013. [Google Scholar]
- Yu, J.; Ma, J. Design and Shear Analysis of an Angled Morphing Wing Skin Module. Appl. Sci. 2022, 12, 3092. [Google Scholar] [CrossRef]
- Alsaidi, B.; Joe, W.Y.; Akbar, M. Computational analysis of 3D lattice structures for skin in real-scale camber morphing aircraft. Aerospace 2019, 6, 79. [Google Scholar] [CrossRef]
- Alsaidi, B.; Joe, W.Y.; Akbar, M. Simplified 2D skin lattice models for multi-axial camber morphing wing aircraft. Aerospace 2019, 6, 90. [Google Scholar] [CrossRef]
- La, S.; Joe, W.Y.; Akbar, M.; Alsaidi, B. Surveys on skin design for morphing wing aircraft: Status and challenges. In Proceedings of the 2018 AIAA Aerospace Sciences Meeting, Kissimmee, FL, USA, 8–12 January 2018; p. 0315. [Google Scholar]
- Wong, A.; Bil, C.; Marino, M. Design and Aerodynamic Performance of a FishBAC Morphing Wing. In Proceedings of the AIAA SCITECH 2022 Forum, San Diego, CA, USA, 3–7 January 2022; p. 1298. [Google Scholar]
- Azzawi, W.A. Development and performance evaluation of a morphing wing design using shape memory polymer and composite corrugated structure. Aust. J. Mech. Eng. 2022. [Google Scholar] [CrossRef]
- Auteri, F.; Savino, A.; Zanotti, A.; Gibertini, G.; Zagaglia, D.; Tekap, Y.B.; Braza, M. Experimental evaluation of the aerodynamic performance of a large-scale high-lift morphing wing. Aerosp. Sci. Technol. 2022, 124, 107515. [Google Scholar] [CrossRef]
- Anderson, J.D.; Bowden, M.L. Introduction to Flight; McGraw-Hill Higher Education: New York, NY, USA, 2005. [Google Scholar]
- Eshelby, M. Aircraft Performance: Theory and Practice; American Institute of Aeronautics and Astronautics, Inc.: Reston, VA, USA, 2000. [Google Scholar]
- Mair, W.A.; Birdsall, D.L. Aircraft Performance; Cambridge University Press: Cambridge, UK, 1996; Volume 5. [Google Scholar]
- Ai, Q.; Kamliya Jawahar, H.; Azarpeyvand, M. Experimental investigation of aerodynamic performance of airfoils fitted with morphing trailing edges. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; p. 1563. [Google Scholar]
- Bowman, J.; Sanders, B.; Weisshaar, T. Evaluating the impact of morphing technologies on aircraft performance. In Proceedings of the 43rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Denver, CO, USA, 22–25 April 2002; p. 1631. [Google Scholar]
- Hui, Z.; Zhang, Y.; Chen, G. Aerodynamic performance investigation on a morphing unmanned aerial vehicle with bio-inspired discrete wing structures. Aerosp. Sci. Technol. 2019, 95, 105419. [Google Scholar]
- Jawahar, H.K.; Ai, Q.; Azarpeyvand, M. Experimental and numerical investigation of aerodynamic performance for airfoils with morphed trailing edges. Renew. Energy 2018, 127, 355–367. [Google Scholar] [CrossRef]
- Joshi, S.; Tidwell, Z.; Crossley, W.; Ramakrishnan, S. Comparison of morphing wing stategies based upon aircraft performance impacts. In Proceedings of the 45th the AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics & Materials Conference, Palm Springs, CA, USA, 19–22 April 2004; p. 1722. [Google Scholar]
- Goetten, F.; Finger, D.; Marino, M.; Bil, C.; Havermann, M.; Braun, C. A review of guidelines and best practices for subsonic aerodynamic simulations using RANS CFD. In Proceedings of the APISAT 2019: Asia Pacific International Symposium on Aerospace Technology, Gold Coast, Australia, 4–6 December 2019; Engineers Australia: Barton, Australia, 2019; p. 227. [Google Scholar]
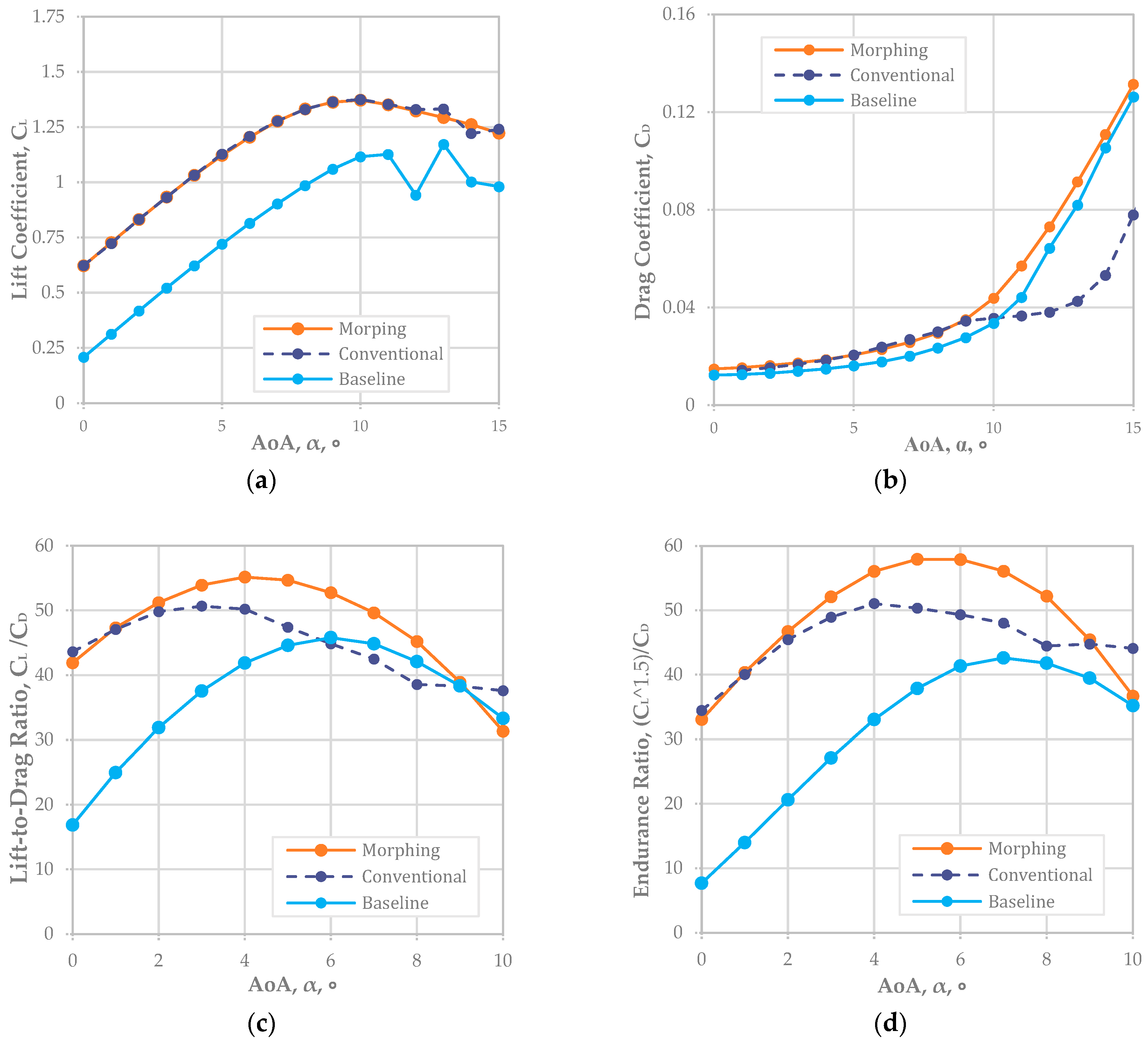
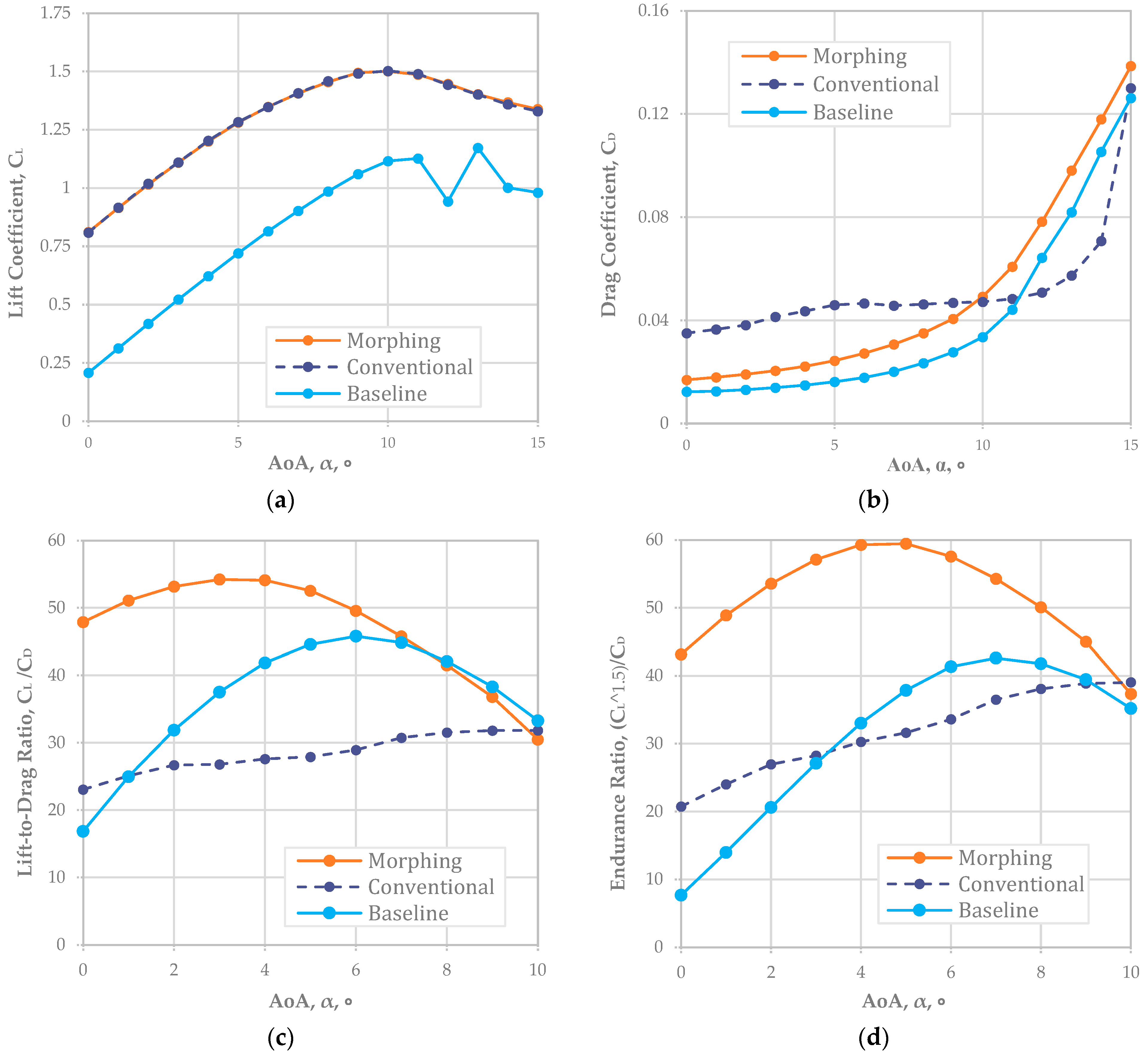
| UAV Model | RQ-7 Shadow | |
|---|---|---|
 | Wing chord | 0.54 m |
| Wingspan | 3.89 m | |
| Cruising speeds | 24.7 m/s | |
| Operating altitude | 2000 m | |
| Density | 1.007 kg/m m3 | |
| Dynamic viscosity | 1.726 × 10−5 Ns/m | |
| Re | 778,179 | |
| Baseline airfoil | NACA 2410 |
| AoA (°) | Morphing Airfoil (NACA 4410) | Conventional Airfoils | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Flap Angle (°) | |||||||||
| 0 | 0.41 | 0.0133 | 30.92 | 19.85 | 0.41 | 0.0120 | 34.49 | 22.18 | 4 |
| 1 | 0.52 | 0.0137 | 37.75 | 27.15 | 0.52 | 0.0126 | 41.04 | 29.56 | 4 |
| 2 | 0.62 | 0.0143 | 43.36 | 34.20 | 0.62 | 0.0135 | 46.14 | 36.41 | 4 |
| 3 | 0.73 | 0.0153 | 47.27 | 40.26 | 0.73 | 0.0145 | 50.20 | 42.77 | 4 |
| 4 | 0.83 | 0.0165 | 50.20 | 45.69 | 0.83 | 0.0156 | 52.79 | 47.96 | 4 |
| 5 | 0.93 | 0.0179 | 51.88 | 49.97 | 0.92 | 0.0171 | 54.00 | 51.82 | 4 |
| 6 | 1.02 | 0.0197 | 51.80 | 52.28 | 1.01 | 0.0189 | 53.45 | 53.76 | 4 |
| 7 | 1.10 | 0.0222 | 49.73 | 52.22 | 1.09 | 0.0214 | 51.06 | 53.40 | 4 |
| 8 | 1.17 | 0.0257 | 45.68 | 49.49 | 1.17 | 0.0244 | 47.95 | 51.90 | 4 |
| 9 | 1.23 | 0.0304 | 40.48 | 44.91 | 1.24 | 0.0272 | 45.47 | 50.54 | 3.7 |
| 10 | 1.26 | 0.0375 | 33.47 | 37.51 | 1.26 | 0.0294 | 42.89 | 48.16 | 2.3 |
| AoA (°) | Morphing Airfoil (NACA 6410) | Conventional Airfoils | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Flap Angle (°) | |||||||||
| 0 | 0.62 | 0.0148 | 41.90 | 33.04 | 0.62 | 0.0143 | 43.61 | 34.45 | 8 |
| 1 | 0.73 | 0.0154 | 47.32 | 40.36 | 0.72 | 0.0154 | 47.07 | 40.02 | 8.1 |
| 2 | 0.83 | 0.0162 | 51.21 | 46.70 | 0.83 | 0.0167 | 49.80 | 45.44 | 8.4 |
| 3 | 0.93 | 0.0173 | 53.91 | 52.09 | 0.93 | 0.0184 | 50.67 | 48.93 | 8.6 |
| 4 | 1.03 | 0.0187 | 55.17 | 56.03 | 1.03 | 0.0206 | 50.21 | 51.04 | 8.95 |
| 5 | 1.12 | 0.0205 | 54.68 | 57.92 | 1.13 | 0.0238 | 47.39 | 50.33 | 9.6 |
| 6 | 1.20 | 0.0228 | 52.74 | 57.88 | 1.21 | 0.0270 | 44.84 | 49.30 | 9.8 |
| 7 | 1.28 | 0.0257 | 49.63 | 56.08 | 1.28 | 0.0301 | 42.49 | 48.02 | 9.8 |
| 8 | 1.33 | 0.0295 | 45.21 | 52.18 | 1.33 | 0.0345 | 38.54 | 44.45 | 9.5 |
| 9 | 1.36 | 0.0350 | 38.90 | 45.42 | 0.62 | 0.0356 | 43.61 | 44.74 | 8 |
| 10 | 1.37 | 0.0438 | 31.32 | 36.68 | 0.72 | 0.0366 | 47.07 | 44.07 | 6.1 |
| AoA (°) | Morphing Airfoil (NACA 8410) | Conventional Airfoils | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Flap Angle (°) | |||||||||
| 0 | 0.81 | 0.0169 | 47.89 | 43.14 | 0.81 | 0.0351 | 23.07 | 20.75 | 17.6 |
| 1 | 0.92 | 0.0179 | 51.08 | 48.88 | 0.92 | 0.0365 | 25.09 | 24.01 | 17.75 |
| 2 | 1.02 | 0.0191 | 53.15 | 53.55 | 1.02 | 0.0382 | 26.70 | 26.96 | 18.05 |
| 3 | 1.11 | 0.0205 | 54.21 | 57.10 | 1.11 | 0.0414 | 26.81 | 28.25 | 18.4 |
| 4 | 1.20 | 0.0222 | 54.10 | 59.27 | 1.20 | 0.0436 | 27.60 | 30.28 | 18.4 |
| 5 | 1.28 | 0.0244 | 52.53 | 59.45 | 1.28 | 0.0460 | 27.92 | 31.63 | 18.3 |
| 6 | 1.35 | 0.0272 | 49.56 | 57.55 | 1.35 | 0.0466 | 28.94 | 33.59 | 18 |
| 7 | 1.41 | 0.0307 | 45.77 | 54.27 | 1.41 | 0.0457 | 30.76 | 36.49 | 16.2 |
| 8 | 1.46 | 0.0351 | 41.51 | 50.07 | 1.46 | 0.0463 | 31.53 | 38.08 | 15 |
| 9 | 1.49 | 0.0406 | 36.81 | 45.00 | 1.49 | 0.0468 | 31.84 | 38.88 | 13.5 |
| 10 | 1.50 | 0.0492 | 30.48 | 37.35 | 1.50 | 0.0472 | 31.85 | 39.04 | 11.1 |
| AoA (°) | Baseline | NACA 4410 | Conventional | Percentage Improvement | NACA 6410 | Conventional | Percentage Improvement | NACA 8410 | Conventional | Percentage Improvement |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 16.86 | 30.92 | 34.49 | −10.4% | 41.90 | 43.61 | −3.9% | 47.89 | 23.07 | 107.6% |
| 1 | 24.97 | 37.75 | 41.04 | −8.0% | 47.32 | 47.07 | 0.5% | 51.08 | 25.09 | 103.6% |
| 2 | 31.89 | 43.36 | 46.14 | −6.0% | 51.21 | 49.80 | 2.8% | 53.15 | 26.70 | 99.0% |
| 3 | 37.54 | 47.27 | 50.20 | −5.8% | 53.91 | 50.67 | 6.4% | 54.21 | 26.81 | 102.2% |
| 4 | 41.87 | 50.20 | 52.79 | −4.9% | 55.17 | 50.21 | 9.9% | 54.10 | 27.60 | 96.0% |
| 5 | 44.59 | 51.88 | 54.00 | −3.9% | 54.68 | 47.39 | 15.4% | 52.53 | 27.92 | 88.2% |
| 6 | 45.80 | 51.80 | 53.45 | −3.1% | 52.74 | 44.84 | 17.6% | 49.56 | 28.94 | 71.3% |
| 7 | 44.85 | 49.73 | 51.06 | −2.6% | 49.63 | 42.49 | 16.8% | 45.77 | 30.76 | 48.8% |
| 8 | 42.09 | 45.68 | 47.95 | −4.7% | 45.21 | 38.54 | 17.3% | 41.51 | 31.53 | 31.6% |
| 9 | 38.32 | 40.48 | 45.47 | −11.0% | 38.90 | 38.30 | 1.6% | 36.81 | 31.84 | 15.6% |
| 10 | 33.30 | 33.47 | 42.89 | −22.0% | 31.32 | 37.58 | −16.7% | 30.48 | 31.85 | −4.3% |
| AoA (°) | Baseline | NACA 4410 | Conventional | Percentage Improvement | NACA 6410 | Conventional | Percentage Improvement | NACA 8410 | Conventional | Percentage Improvement |
|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 7.69 | 19.85 | 22.18 | −10.5% | 33.04 | 34.45 | −4.1% | 43.14 | 20.75 | 107.9% |
| 1 | 13.97 | 27.15 | 29.56 | −8.2% | 40.36 | 40.02 | 0.8% | 48.88 | 24.01 | 103.5% |
| 2 | 20.62 | 34.20 | 36.41 | −6.1% | 46.70 | 45.44 | 2.8% | 53.55 | 26.96 | 98.6% |
| 3 | 27.12 | 40.26 | 42.77 | −5.9% | 52.09 | 48.93 | 6.5% | 57.10 | 28.25 | 102.1% |
| 4 | 33.04 | 45.69 | 47.96 | −4.7% | 56.03 | 51.04 | 9.8% | 59.27 | 30.28 | 95.8% |
| 5 | 37.85 | 49.97 | 51.82 | −3.6% | 57.92 | 50.33 | 15.1% | 59.45 | 31.63 | 88.0% |
| 6 | 41.34 | 52.28 | 53.76 | −2.8% | 57.88 | 49.30 | 17.4% | 57.55 | 33.59 | 71.3% |
| 7 | 42.61 | 52.22 | 53.40 | −2.2% | 56.08 | 48.02 | 16.8% | 54.27 | 36.49 | 48.7% |
| 8 | 41.79 | 49.49 | 51.90 | −4.6% | 52.18 | 44.45 | 17.4% | 50.07 | 38.08 | 31.5% |
| 9 | 39.45 | 44.91 | 50.54 | −11.1% | 45.42 | 44.74 | 1.5% | 45.00 | 38.88 | 15.7% |
| 10 | 35.18 | 37.51 | 48.16 | −22.1% | 36.68 | 44.07 | −16.8% | 37.35 | 39.04 | −4.3% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jo, B.W.; Majid, T. Enhanced Range and Endurance Evaluation of a Camber Morphing Wing Aircraft. Biomimetics 2023, 8, 34. https://doi.org/10.3390/biomimetics8010034
Jo BW, Majid T. Enhanced Range and Endurance Evaluation of a Camber Morphing Wing Aircraft. Biomimetics. 2023; 8(1):34. https://doi.org/10.3390/biomimetics8010034
Chicago/Turabian StyleJo, Bruce W., and Tuba Majid. 2023. "Enhanced Range and Endurance Evaluation of a Camber Morphing Wing Aircraft" Biomimetics 8, no. 1: 34. https://doi.org/10.3390/biomimetics8010034
APA StyleJo, B. W., & Majid, T. (2023). Enhanced Range and Endurance Evaluation of a Camber Morphing Wing Aircraft. Biomimetics, 8(1), 34. https://doi.org/10.3390/biomimetics8010034










