Wind Impact Assessment of a Sour Gas Release in an Offshore Platform
Abstract
1. Introduction
Case Study
- Release pressure and temperature: prel = 50 bar and Trel = 300 K;
- Release hole diameter: drel = 3 cm;
- Released gas mixture mole composition: 99 mol% CH4, 1 mol% H2S;
- Wind velocities: Uref = 2-4-6-8-10 m/s;
- Ambient temperature: Ta = 300 K.
- LFL: lower flammability limit;
- UFL: upper flammability limit;
- IDLH: immediately dangerous to life and health concentration;
- LC50: lethal dose at which 50% of the population is killed in a given time following exposure.
- LFLmix = 4.994%;
- UFLmix = 16.104%.
2. Methodology
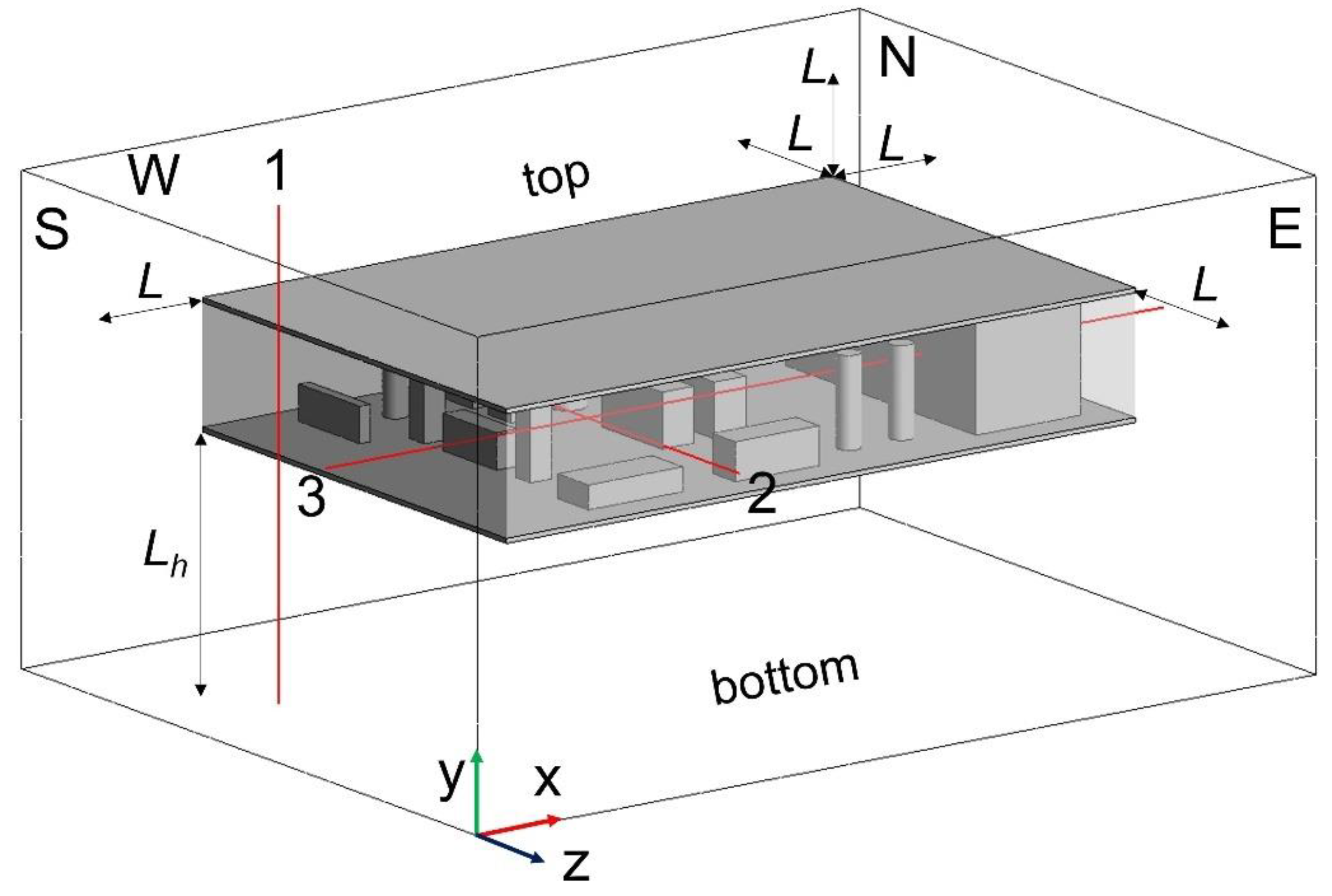
2.1. Wind Field Simulation
- The wind inlet must be imposed at 5H from the body;
- The outlet BCs must be imposed at 15H from the body;
- The height of the domain should be 6H.
- L = H = 5 m;
- L = 2H = 10 m;
- L = 3H = 15 m;
- L = 4H = 20 m.
- (5H + Lx + 15H)·(5H + Lz + 15H)·6H = 468,000 m3,
- (3H + Lx + 3H)·(3H + Lz + 3H)·(10 + H + 3H) = 90,000 m3.
2.2. Wind Sensitivity Analysis
2.2.1. CFD Model Overview: SBAM
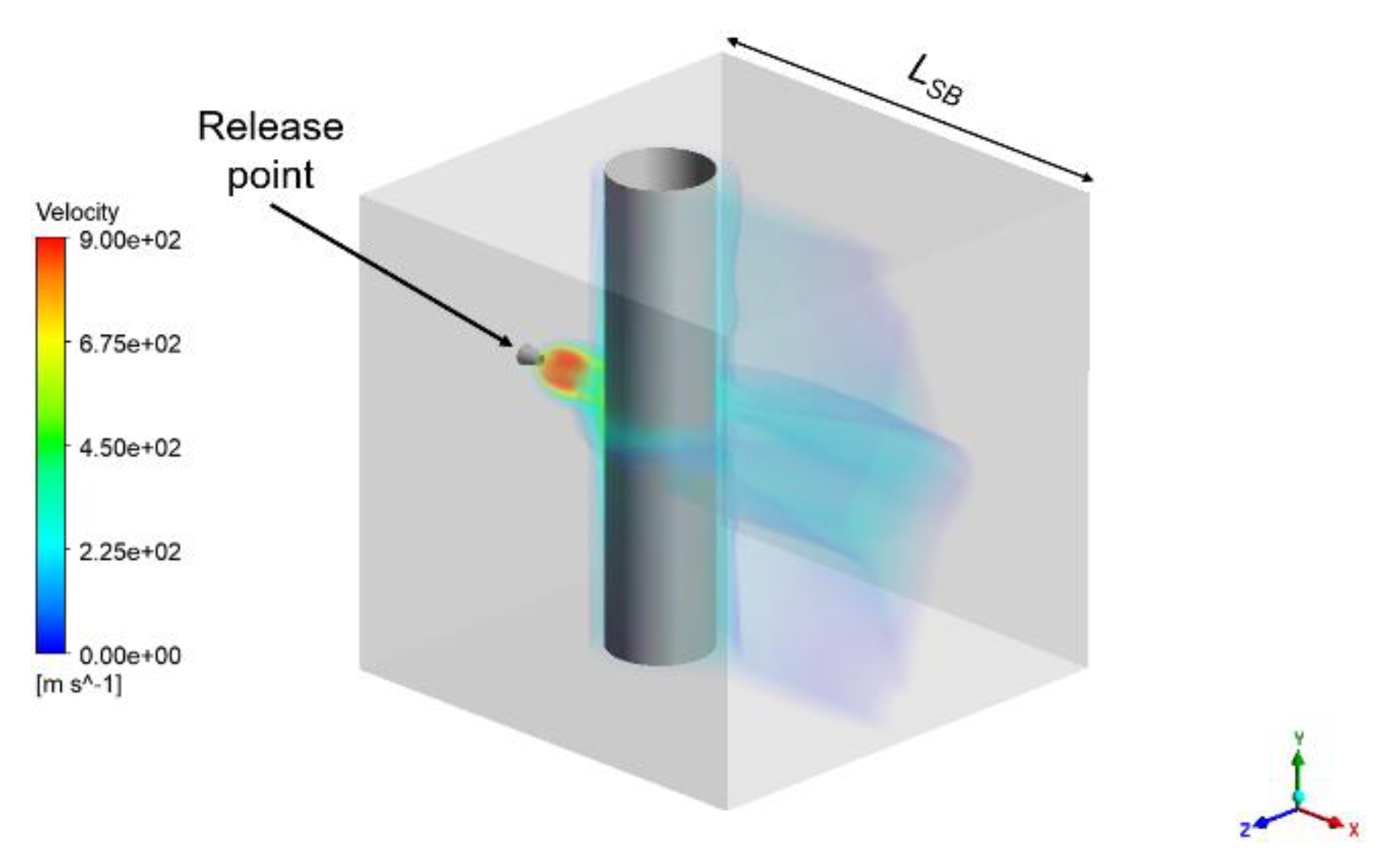
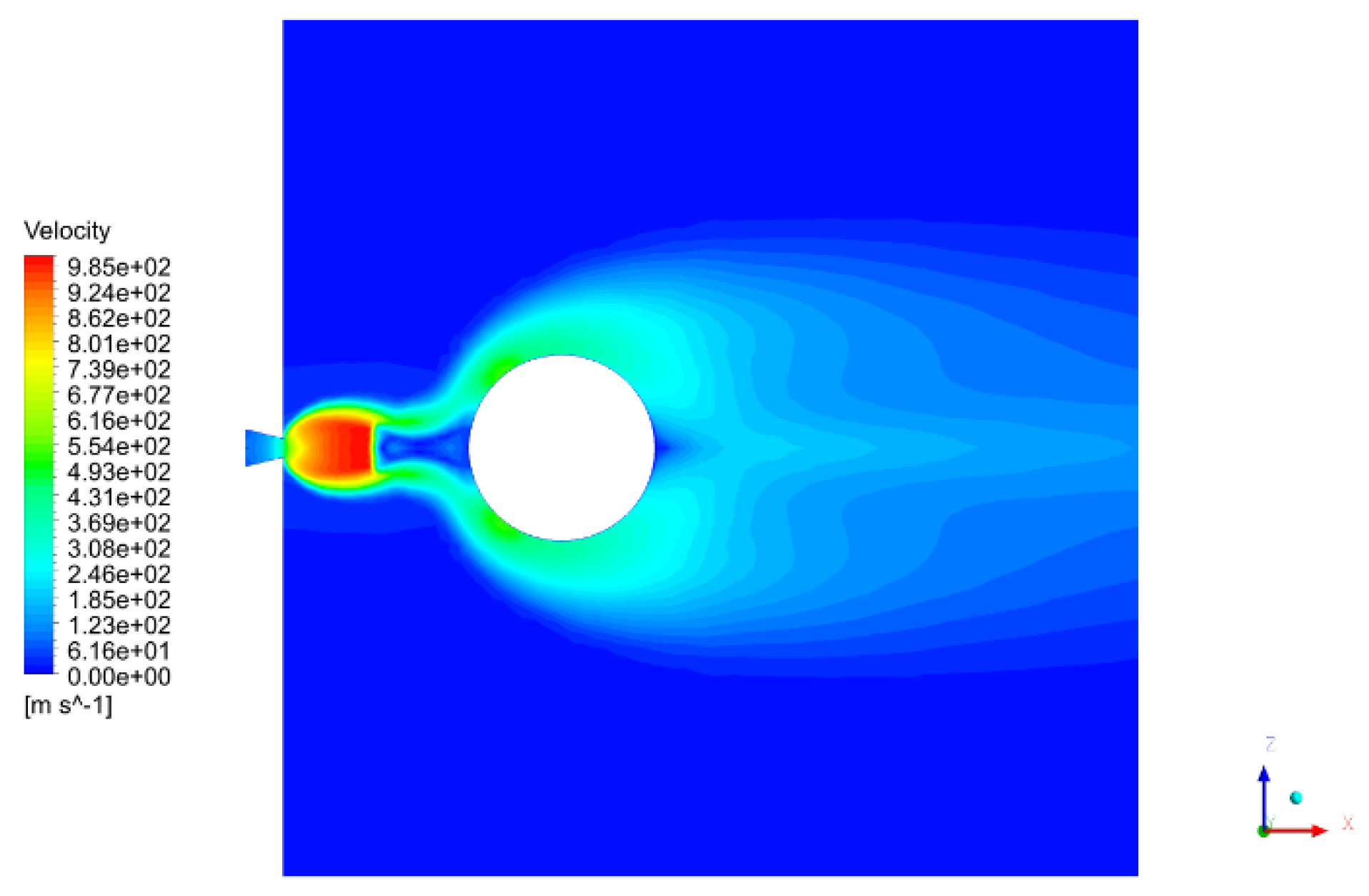
SBAM: Source Box Simulation Setup
SBAM: Dispersion Simulation Setup
3. Results and Discussion
3.1. Source Box Results
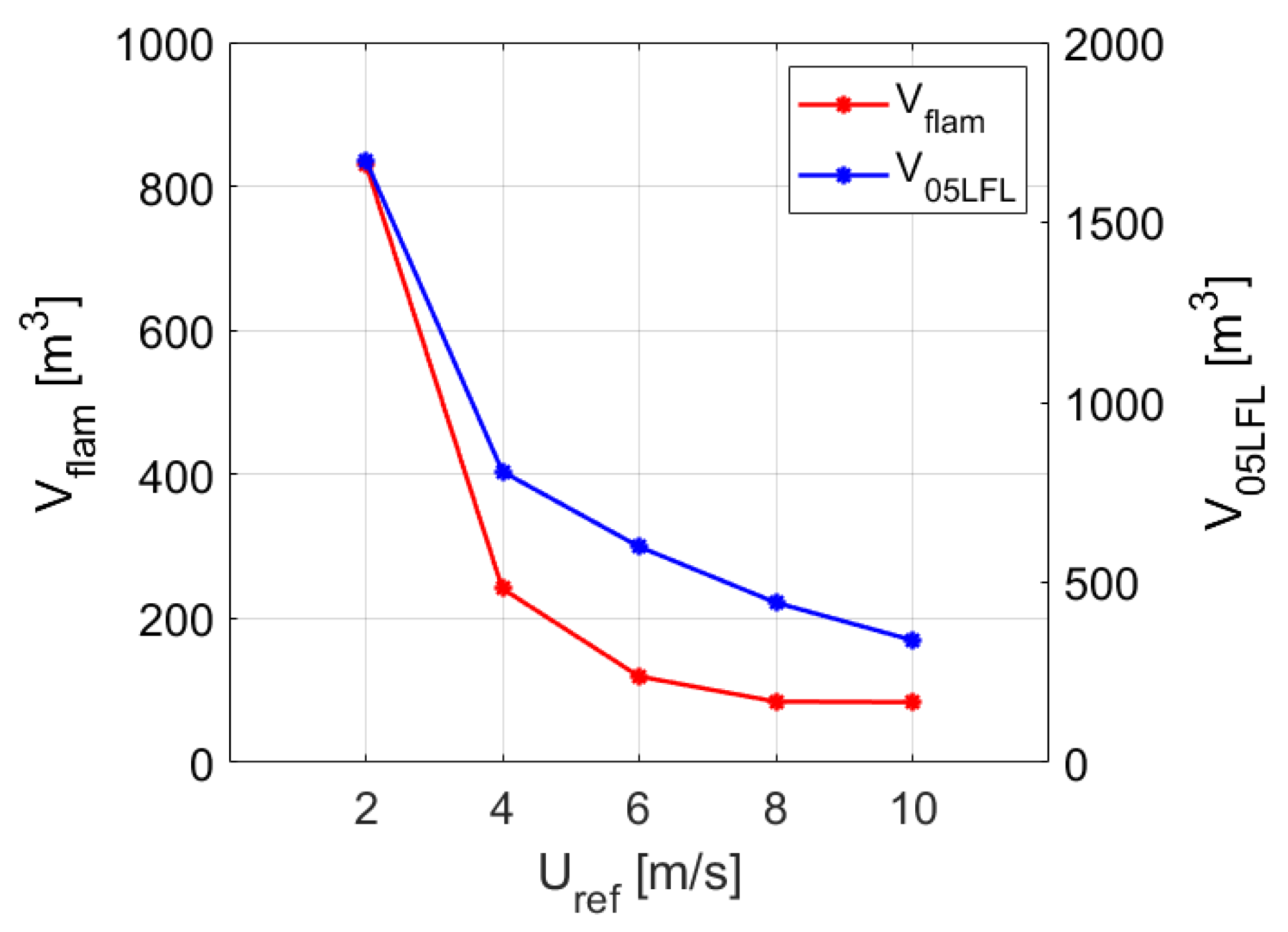
3.2. Dispersion Results
4. Conclusions
- A new criterion for the dimensioning of the wind simulation domain is defined; the volume is reduced by ~80% with respect to the state-of-the-art methodologies.
- The gas dispersion can be simulated in a smaller domain (in this case, in the platform) and not in a larger external box.
- Once the wind field is available, the results can be used to simulate several dispersion simulations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Governing Equations
Appendix A.1.1. Mass Conservation Equation
Appendix A.1.2. Momentum Conservation Equation
Appendix A.1.3. Turbulence Model Equations
Appendix A.1.4. Species Transport Equation
Appendix A.1.5. Energy Equation
Appendix A.1.6. Numerical Methods
Appendix A.2. Grid Features
| Average Velocity | CH4 Mass Fraction | H2S Mass Fraction | |
|---|---|---|---|
| GCI12 | 0.0205 | 0.0003 | 0.0003 |
| GCI23 | 0.0783 | 0.0090 | 0.0090 |
| 1.05 | 0.99 | 0.99 |
| Average Velocity | Flammable Vol. | Toxic Vol. | |
|---|---|---|---|
| GCI12 | 0.0104 | 0.0160 | 10−5 |
| GCI23 | 0.0217 | 0.0348 | 1.5 × 10−4 |
| 1.01 | 1.01 | 1.00 |
References
- Sales, J.; Mushtaq, F.; Christou, M.D.; Nomen, R. Study of major accidents involving chemical reactive substances: Analysis and lessons learned. Process Saf. Environ. Prot. 2007, 85, 117–124. [Google Scholar] [CrossRef]
- Khan, F.I.; Abbasi, S.A. Major accidents in process industries and an analysis of causes and consequences. J. Loss Prev. Process Ind. 1999, 12, 361–378. [Google Scholar] [CrossRef]
- Paté-Cornell, M.E. Risk analysis and risk management for offshore platforms: Lessons from the Piper Alpha accident. J. Offshore Mech. Arct. Eng. 1993, 115, 179–190. [Google Scholar] [CrossRef]
- Necci, A.; Tarantola, S.; Vamanu, B.; Krausmann, E.; Ponte, L. Lessons learned from offshore oil and gas incidents in the Arctic and other ice-prone seas. Ocean Eng. 2019, 185, 12–26. [Google Scholar] [CrossRef]
- Bagheri, M.; Alamdari, A.; Davoudi, M. Quantitative risk assessment of sour gas transmission pipelines using CFD. J. Nat. Gas Sci. Eng. 2016, 31, 108–118. [Google Scholar] [CrossRef]
- Rubright, S.L.M.; Pearce, L.L.; Peterson, J. Environmental toxicology of hydrogen sulfide. Nitric Oxide-Biol. Chem. 2017, 71, 1–13. [Google Scholar] [CrossRef]
- Worden, R.H.; Smalley, P.C.; Barclay, S.A. H2S and diagenetic pyrite in North Sea sandstones: Due to TSR or organic sulphur compound cracking? J. Geochem. Explor. 2003, 78–79, 487–491. [Google Scholar] [CrossRef]
- Zempolich, W.; Negri, A.; Leo, C.; van Ojik, K.; Verdel, A. The Kashagan Discovery: An Example of the Successful Use of a Multidisciplined Approach in Reducing Geologic Risk. Am. Assoc. Pet. Geol. Bull. 2002, 86, 1–2. [Google Scholar] [CrossRef]
- Warner, J.L.; Baskin, D.K.; Hwang, R.J.; Carlson, R.M.K.; Clark, M.E. Geochemical Evidence for Two Stages of Hydrocarbon Emplacement and the Origin of Solid Bitumen in the Giant Tengiz Field, Kazakhstan. In Oil and Gas of the Greater Caspian Area 55; American Association of Petroleum Geologists: Tulsa, OK, USA, 2007. [Google Scholar] [CrossRef]
- Liu, W.; Tenger; Bo, G.; Zhang, Z.; Zhang, J.; Zhang, D.; Fan, M.; Fu, X.; Zheng, L.; Liu, Q. H2S formation and enrichment mechanisms in medium to large scale natural gas fields (reservoirs) in the Sichuan Basin. Pet. Explor. Dev. 2010, 37, 513–522. [Google Scholar] [CrossRef]
- Mi, J.; Zhang, B.; Shen, Z.; Huang, W.; Casalins, A.; Liu, C. The experimental study on H2S generation during thermal recovery process for heavy oil from the Eastern Venezuela Basin. J. Nat. Gas Geosci. 2017, 2, 201–208. [Google Scholar] [CrossRef]
- Li, D.P.; Zhang, L.; Yang, J.W.; Lu, M.X.; Ding, J.H.; Liu, M.L. Effect of H2S concentration on the corrosion behavior of pipeline steel under the coexistence of H2S and CO2. Int. J. Miner. Metall. Mater. 2014, 21, 388–394. [Google Scholar] [CrossRef]
- Layfon, D.W.; Cederwall, R.T. Predicting and Managing the Health Risks of Sour-Gas Wells. JAPCA 1987, 37, 1185–1190. [Google Scholar] [CrossRef]
- OSHA. Hydrogen Sulfide. 2021. Available online: https://www.osha.gov/sites/default/files/publications/hydrogen_sulfide_fact.pdf (accessed on 11 July 2021).
- Zhang, P.; Luo, Q.; Wang, R.; Xu, J. Hydrogen sulfide toxicity inhibits primary root growth through the ROS-NO pathway. Sci. Rep. 2017, 7, 868. [Google Scholar] [CrossRef] [PubMed]
- Torrans, E.L.; Clemens, H.P. Physiological and biochemical effects of acute exposure of fish to hydrogen sulfide. Comp. Biochem. Physiol. Part C Comp. 1982, 71, 183–190. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.J.; Rodi, W. Vertical turbulent buoyant jets: A review of experimental data. NASA Sti/Recon Tech. Rep. A 1980, 80, 23073. [Google Scholar]
- Davidson, G.A. Simultaneous trajectory and dilution predictions from a simple integral plume model. Atmos. Environ. 1989, 23, 341–349. [Google Scholar] [CrossRef]
- The Netherlands Organization of Applied Scientific Research. Methods for the Calculation of Physical Effects (Yellow Book); Publication Series on Dangerous Substances; Ministerie van Verkeer en Waterstaat: Hague, The Netherlands, 2005; p. 870.
- Zamejc, E. API Standard 521 new alternative method to evaluate fire relief for pressure relief device sizing and depressuring system design. J. Loss Prev. Process Ind. 2014, 27, 21–31. [Google Scholar] [CrossRef]
- Qingchun, M.; Laibin, Z. CFD simulation study on gas dispersion for risk assessment: A case study of sour gas well blowout. Saf. Sci. 2011, 49, 1289–1295. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, G.M. Quantitative risk analysis of toxic gas release caused poisoning—A CFD and dose-response model combined approach. Process Saf. Environ. Prot. 2010, 88, 253–262. [Google Scholar] [CrossRef]
- Venetsanos, A.G.; Baraldi, D.; Adams, P.; Heggem, P.S.; Wilkening, H. CFD modelling of hydrogen release, dispersion and combustion for automotive scenarios. J. Loss Prev. Process Ind. 2008, 21, 162–184. [Google Scholar] [CrossRef]
- Choi, J.; Hur, N.; Kang, S.; Lee, E.D.; Lee, K.B. A CFD simulation of hydrogen dispersion for the hydrogen leakage from a fuel cell vehicle in an underground parking garage. Int. J. Hydrogen Energy 2013, 38, 8084–8091. [Google Scholar] [CrossRef]
- Liu, X.; Godbole, A.; Lu, C.; Michal, G.; Venton, P. Source strength and dispersion of CO2 releases from high-pressure pipelines: CFD model using real gas equation of state. Appl. Energy 2014, 126, 56–68. [Google Scholar] [CrossRef]
- Liu, X.; Godbole, A.; Lu, C.; Michal, G.; Venton, P. Study of the consequences of CO2 released from high-pressure pipelines. Atmos. Environ. 2015, 116, 51–64. [Google Scholar] [CrossRef]
- Deng, Y.; Hu, H.; Yu, B.; Sun, D.; Hou, L.; Liang, Y. A method for simulating the release of natural gas from the rupture of high-pressure pipelines in any terrain. J. Hazard. Mater. 2018, 342, 418–428. [Google Scholar] [CrossRef]
- Colombini, C.; Maugeri, G.; Zanon, G.; Rota, R.; Busini, V. Unignited high-pressure methane jet impinging a pipe rack: Practical tools for risk assessment. J. Loss Prev. Process Ind. 2021, 69, 104378. [Google Scholar] [CrossRef]
- Colombini, C.; Carminati, E.; Parisi, A.; Rota, R.; Busini, V. Safety evaluations on unignited high-pressure methane jets impacting a spherical obstacle. J. Loss Prev. Process Ind. 2022, 74, 104631. [Google Scholar] [CrossRef]
- Colombini, C.; Iannantuoni, C.; Rota, R.; Busini, V. Unignited high-pressure methane jet impacting a cylindrical obstacle: An assessment tool for consequences analysis. J. Loss Prev. Process Ind. 2022, 76, 104593. [Google Scholar] [CrossRef]
- Carpignano, A.; Corti, T.; Uggenti, A.C.; Gerboni, R. Modelling of a supersonic accidental release in Oil&Gas offshore: Characterisation of a source box. Geoing. Ambient. Min. 2017, 152, 58–64. [Google Scholar]
- Moscatello, A.; Uggenti, A.C.; Gerboni, R.; Carpignano, A. A novel approach to high-pressure gas releases simulations. J. Loss Prev. Process Ind. 2021, 72, 104531. [Google Scholar] [CrossRef]
- Moscatello, A.; Gerboni, R.; Ledda, G.; Uggenti, A.C.; Piselli, A.; Carpignano, A. CFD gas release model performance evaluation through wind tunnel experiments. J. Loss Prev. Process Ind. 2021, 75, 104715. [Google Scholar] [CrossRef]
- Pappalardo, F.; Moscatello, A.; Ledda, G.; Uggenti, A.C.; Gerboni, R.; Carpignano, A.; di Maio, F.; Mereu, R.; Zio, E. Quantification of Uncertainty in CFD Simulation of Accidental Gas Release for O & G Quantitative Risk Assessment. Energies 2021, 14, 8117. [Google Scholar]
- Lines, I.G.; Deaves, D.M.; Atkins, W.S. Practical modelling of gas dispersion in low wind speed conditions, for application in risk assessment. J. Hazard. Mater. 1997, 54, 201–226. [Google Scholar] [CrossRef]
- Barros, P.L.; Luiz, A.M.; Nascimento, C.A.; Neto, A.T.P.; Alves, J.J.N. On the non-monotonic wind influence on flammable gas cloud from CFD simulations for hazardous area classification. J. Loss Prev. Process Ind. 2020, 68, 104278. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, G.M. Hydrogen sulfide dispersion consequences analysis in different wind speeds: A CFD based approach. In Proceedings of the 2009 International Conference on Energy and Environment Technology (ICEET 2009), Guilin, China, 16–18 October 2009; Volume 3, pp. 365–368. [Google Scholar] [CrossRef]
- Liao, N.; Huang, K.; Chen, L.; Wang, Z.; Wu, J.; Zhang, F. Numerical simulation of gas dispersion during cold venting of natural gas pipelines. Adv. Mech. Eng. 2018, 10, 1687814018755244. [Google Scholar] [CrossRef]
- Nascimento, C.A.; Luiz, A.M.; Barros, P.L.; Neto, A.T.; Alves, J.J. A CFD-based empirical model for hazardous area extent prediction including wind effects. J. Loss Prev. Process Ind. 2021, 71, 104497. [Google Scholar] [CrossRef]
- Vivalda, C.; Gerboni, R.; Carpignano, A. A practical approach to risk-based gas monitoring system design for oil and gas offshore platforms. In Proceedings of the PSAM 14—Probabilistic Safety Assessment and Management, Los Angeles, CA, USA, 16–21 September 2018. [Google Scholar]
- NIOSH. Documentation for Immediately Dangerous to Life or Health Concentration. 2021. Available online: http://niosh.dnacih.com/nioshdbs/idlh/idlhintr.htm (accessed on 11 July 2021).
- OSHA. Hydrogen Sulfide Properties. 2021. Available online: https://www.osha.gov/hydrogen-sulfide/hazards (accessed on 11 July 2021).
- Zlochower, I.A.; Green, G.M. The limiting oxygen concentration and flammability limits of gases and gas mixtures. J. Loss Prev. Process Ind. 2009, 22, 499–505. [Google Scholar] [CrossRef]
- Liao, S.Y.; Cheng, Q.; Jiang, D.M.; Gao, J. Experimental study of flammability limits of natural gas-air mixture. J. Hazard. Mater. 2005, 119, 81–84. [Google Scholar] [CrossRef] [PubMed]
- Abu-Zidan, Y.; Mendis, P.; Gunawardena, T. Optimising the computational domain size in CFD simulations of tall buildings. Heliyon 2021, 7, e06723. [Google Scholar] [CrossRef]
- Revuz, J.; Hargreaves, D.M.; Owen, J.S. On the domain size for the steady-state CFD modelling of a tall building. Wind Struct. Int. J. 2012, 15, 313–329. [Google Scholar] [CrossRef]
- Blocken, B. Computational Fluid Dynamics for urban physics: Importance, scales, possibilities, limitations and ten tips and tricks towards accurate and reliable simulations. Build. Environ. 2015, 91, 219–245. [Google Scholar] [CrossRef]
- ANSYS. ANSYS Fluent User’s Guide. 2018. Available online: https://www.afs.enea.it/project/neptunius/docs/fluent/html/ug/main_pre.htm (accessed on 1 January 2020).
- Wiernga, J. Representative roughness parameters for homogeneous terrain. Bound.-Layer Meteorol. 1993, 63, 323–363. [Google Scholar] [CrossRef]
- IEC. Wind Turbines e Part 1: Design Requirements; Technical Report 61400e1 Ed.3; International Electrotechnical Commission: Geneva, Switzerland, 2005. [Google Scholar]
- Schlichting, H. Boundary-Layer Theory, 7th ed.; McGraw-Hill: New York, NY, USA, 1979; pp. 596–600. [Google Scholar]
- Franquet, E.; Perrier, V.; Gibout, S.; Bruel, P. Free underexpanded jets in a quiescent medium: A review. Prog. Aerosp. Sci. 2015, 77, 25–53. [Google Scholar] [CrossRef]
- Munson, B.R.; Okiishi, T.H.; Huebsch, W.W.; Rothmayer, A.P. Fluid Mechanics; Wiley: Singapore, 2013. [Google Scholar]
- Decreto del Presidente del Consiglio dei Ministri. Linee Guida per la Predisposizione del Piano d’Emergenza Esterna di cui all’Articolo 20, Comma 4, del Decreto Legislativo 17 Agosto 1999, n. 334e. 2005. Available online: https://www.anci.it/wp-content/uploads/linee-guida-piano-emergenza-esterna.pdf (accessed on 1 January 2020).
- Fu, S.; Yan, X.; Zhang, D.; Li, C.; Zio, E. Framework for the quantitative assessment of the risk of leakage from LNG-fueled vessels by an event tree-CFD. J. Loss Prev. Process Ind. 2016, 42, 42–52. [Google Scholar] [CrossRef]
- Menter, F. Zonal two equation kw turbulence models for aerodynamic flows. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993; p. 2906. [Google Scholar]
- Wilcox, D.C. Turbulence Modeling for CFD; DCW Industries: La Canada, CA, USA, 1998; p. 2. [Google Scholar]
- Roache, P.J. Perspective: A Method for Uniform Reporting of Grid Refiniment Studies. J. Fluids Eng. 1994, 116, 405–413. [Google Scholar] [CrossRef]



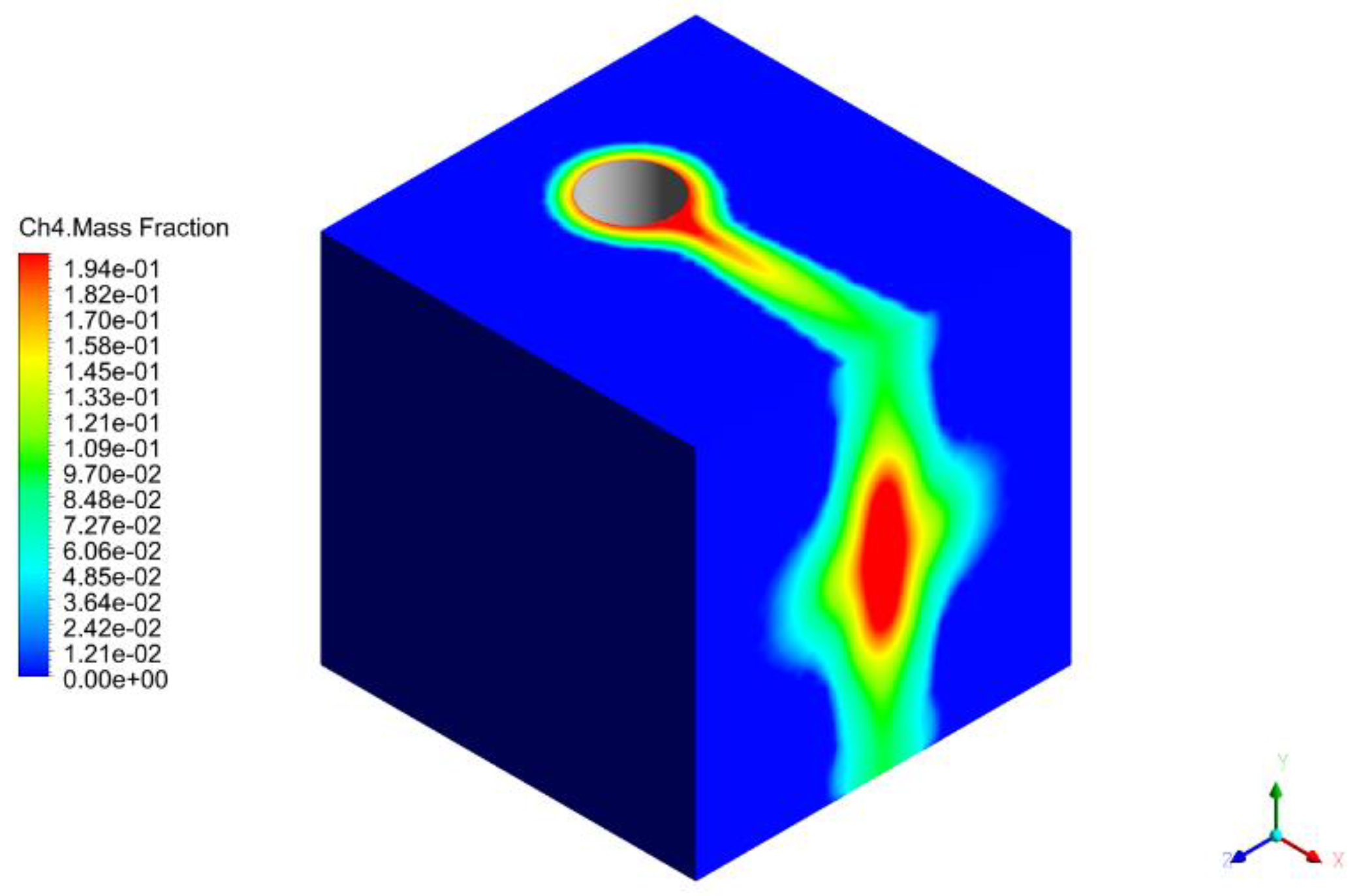
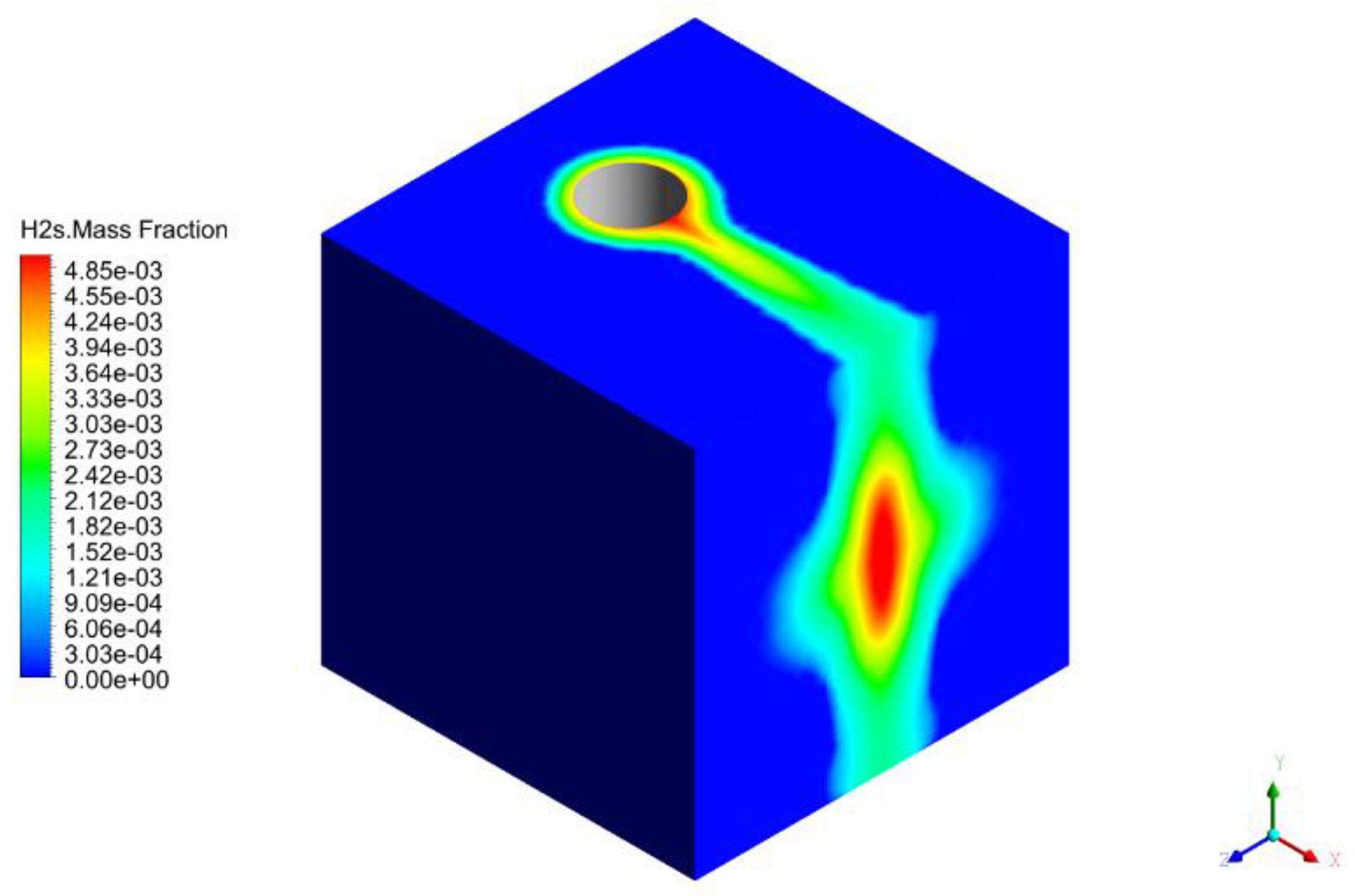




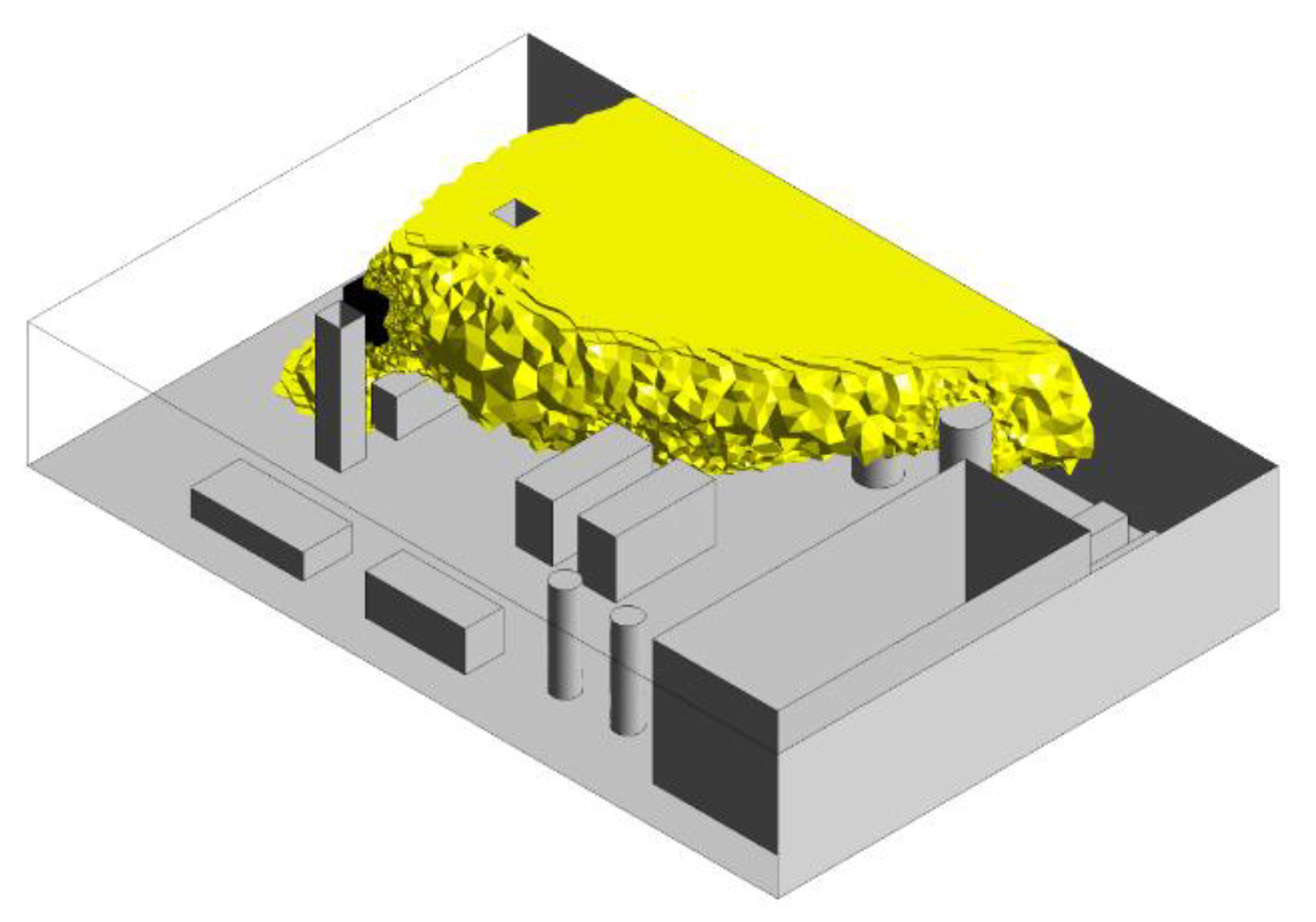
| LFL (mol. conc.) | UFL (mol. conc.) | IDLH (ppm) | LC50 (ppm) | |
|---|---|---|---|---|
| CH4 | 0.05 | 0.16 | / | / |
| H2S | 0.045 | 0.455 | 100 | 713 |
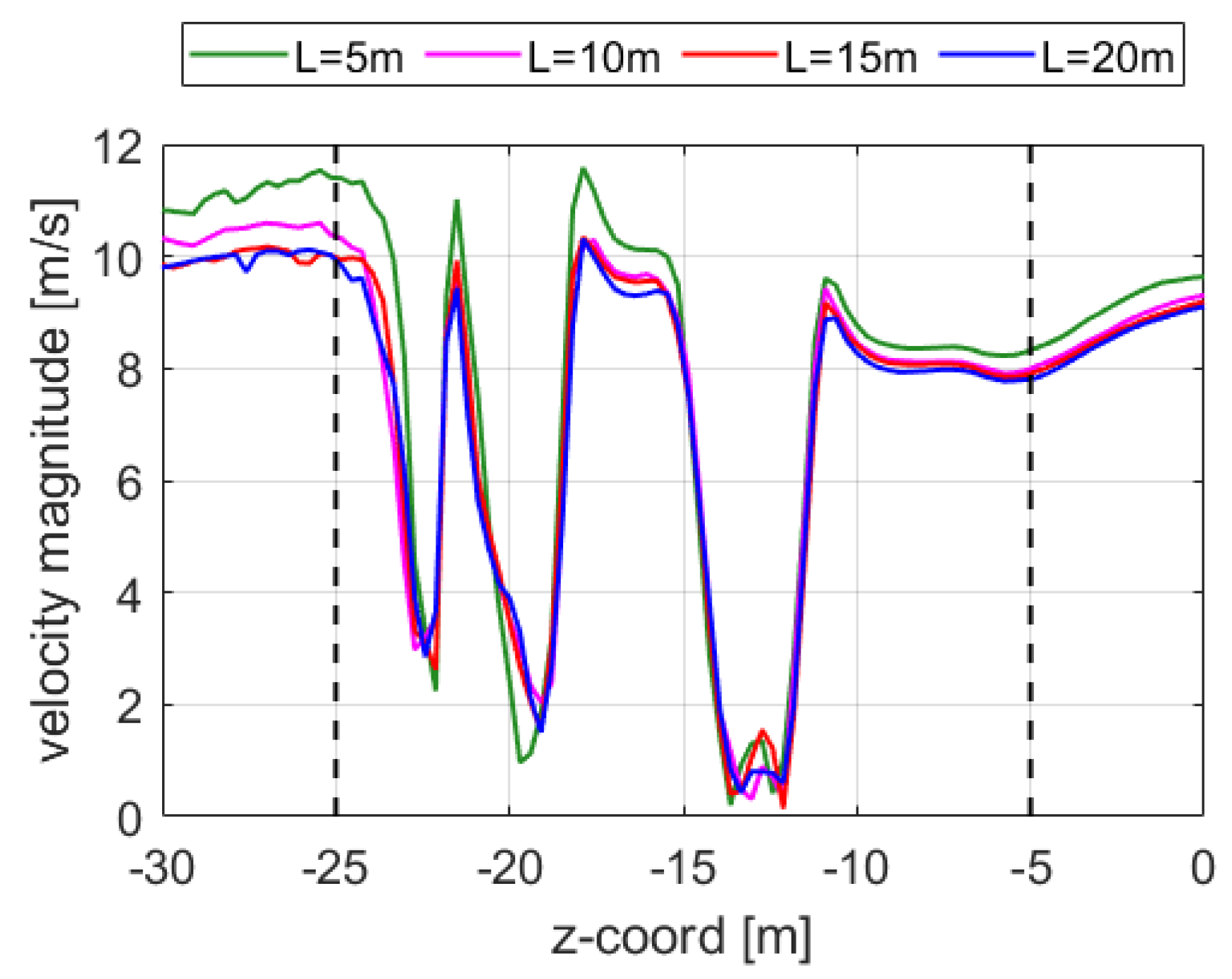
| L = 5 m vs. L = 20 m | L = 10 m vs. L = 20 m | L = 15 m vs. L = 20 m | |
|---|---|---|---|
| Line 1 | 4.47% | 2.46% | 0.87% |
| Line 2 | 9.2% | 2.51% | 1.08% |
| Line 3 | 9.15% | 6.36% | 1.9% |
| Xm (Theory) (m) | Xm (CFD) (m) | Relative Error (%) |
|---|---|---|
| 0.1368 | 0.1300 | 4.97 |
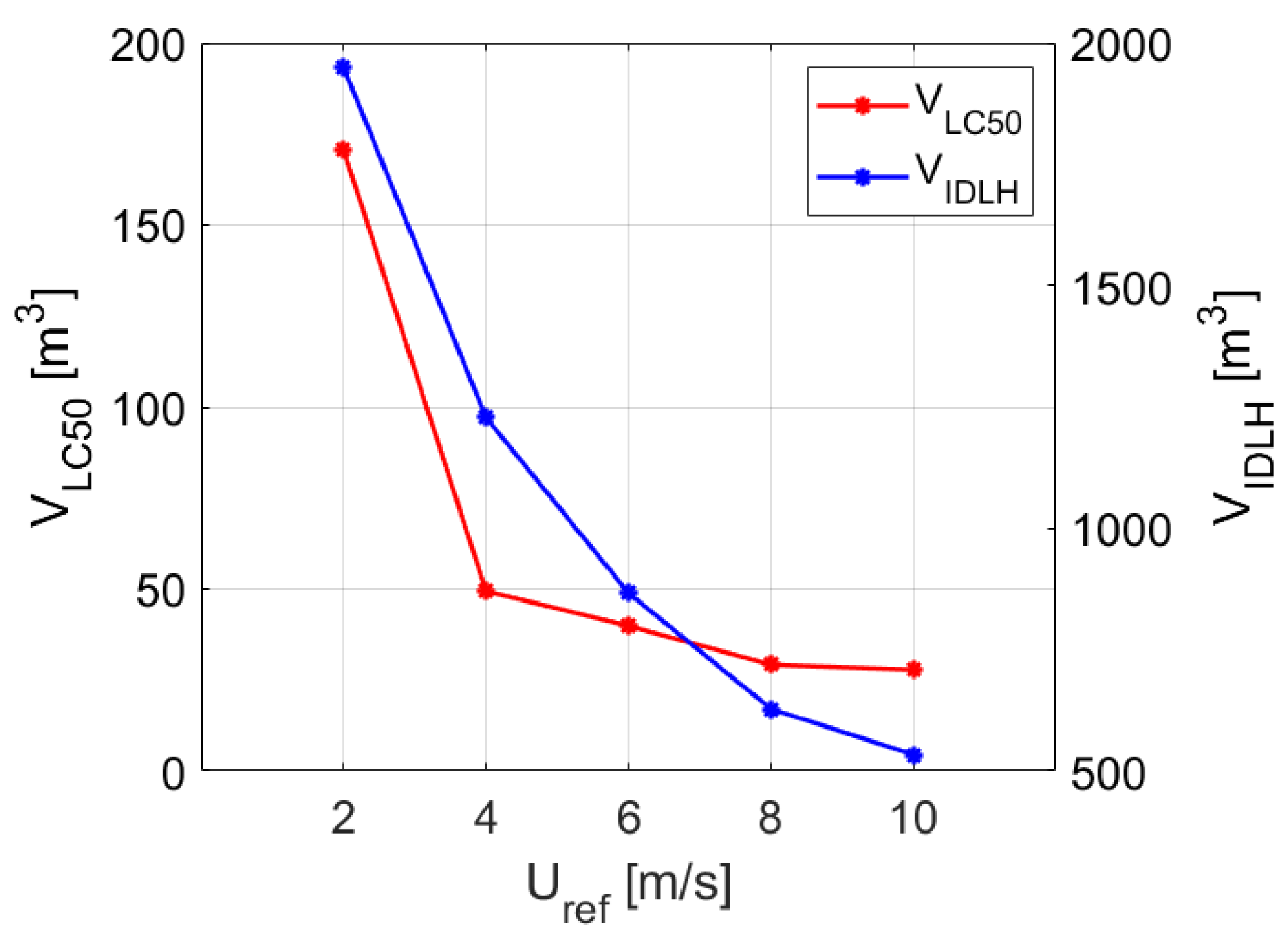
| Uref (m/s) | Vflam (m3) | V05LFL (m3) | VLC50 (m3) | VIDLH (m3) | Σflam (%) | Σ05LFL (%) | ΣLC50 (%) | ΣIDLH (%) |
|---|---|---|---|---|---|---|---|---|
| 2 | 831.36 | 1672.64 | 170.56 | 1948.93 | 32.57 | 65.53 | 6.68 | 76.35 |
| 4 | 241.44 | 807.94 | 49.43 | 1228.09 | 9.46 | 31.65 | 1.94 | 48.11 |
| 6 | 119.48 | 599.21 | 39.85 | 866.60 | 4.68 | 23.47 | 1.56 | 33.95 |
| 8 | 84.58 | 444.05 | 29.18 | 627.97 | 3.31 | 17.40 | 1.14 | 24.60 |
| 10 | 83.77 | 339.64 | 27.81 | 533.67 | 3.28 | 13.31 | 1.09 | 20.91 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moscatello, A.; Ledda, G.; Uggenti, A.C.; Gerboni, R.; Carpignano, A. Wind Impact Assessment of a Sour Gas Release in an Offshore Platform. Safety 2022, 8, 80. https://doi.org/10.3390/safety8040080
Moscatello A, Ledda G, Uggenti AC, Gerboni R, Carpignano A. Wind Impact Assessment of a Sour Gas Release in an Offshore Platform. Safety. 2022; 8(4):80. https://doi.org/10.3390/safety8040080
Chicago/Turabian StyleMoscatello, Alberto, Gianmario Ledda, Anna Chiara Uggenti, Raffaella Gerboni, and Andrea Carpignano. 2022. "Wind Impact Assessment of a Sour Gas Release in an Offshore Platform" Safety 8, no. 4: 80. https://doi.org/10.3390/safety8040080
APA StyleMoscatello, A., Ledda, G., Uggenti, A. C., Gerboni, R., & Carpignano, A. (2022). Wind Impact Assessment of a Sour Gas Release in an Offshore Platform. Safety, 8(4), 80. https://doi.org/10.3390/safety8040080






