Micro- and Nano-Scales Three-Dimensional Characterisation of Softwood
Abstract
:1. Introduction
2. Materials and Methods
2.1. Samples Preparation
2.2. Imaging Setups
2.3. Dynamic Vapour Sorption Analysis
2.4. Methods of Analysis
- (A).
- The wood porosity, due to the presence of the lumen, was defined here as void volume per total volume. By grey value thresholding, the tomographic datasets were first converted into binary datasets composed of voxels containing either cell wall material or air. The “region growing” algorithm available in VGStudio MAX 2.0 software was used as a thresholding method. Starting from the binary images, the wood volume was calculated by counting the number of material (white) voxels for each slice, summing up over all the stacked slices, and multiplying this value by the voxel size.
- (B).
- The wood cell wall deformations were calculated both at the global level (such as during free swelling/shrinkage) and at the local level (as observed during the restraining experiment). In particular, for case 1.b, two ROIs were selected for each sample containing different restraint intensity to the free swelling from the restraining device. In detail, regions of the sample not directly in contact with the PMMA cube surface received a minor restraint to the swelling during moisture adsorption caused by the device; such regions are called “ROIs 1”. Conversely, regions directly in touch with the PMMA cube surface were more subjected to the restraining role of the device: such regions are referred to as “ROIs 2”. The most commonly used transformation method for the calculation of the global deformations is the so-called affine transformation, where changes in position, size and shape of a volume are described. The parameterisation of a 3D affine transformation generally involves 12 parameters (three for defining translation, three for rotation, three for scaling and three for shear). Details are published in [8,10]. A previous work of the author [21] describes the quantitative method implemented to analyse the local deformations in cellular wood tissues when subjected to environmental changes. The algorithm, written in Matlab, employs a free-form deformation model based on B-splines. This algorithm was then used to detect the local strains in bordered pits as well.
3. Results
3.1. Wood Porosity
3.2. Anisotropic Swelling Behaviour of Earlywood and Latewood
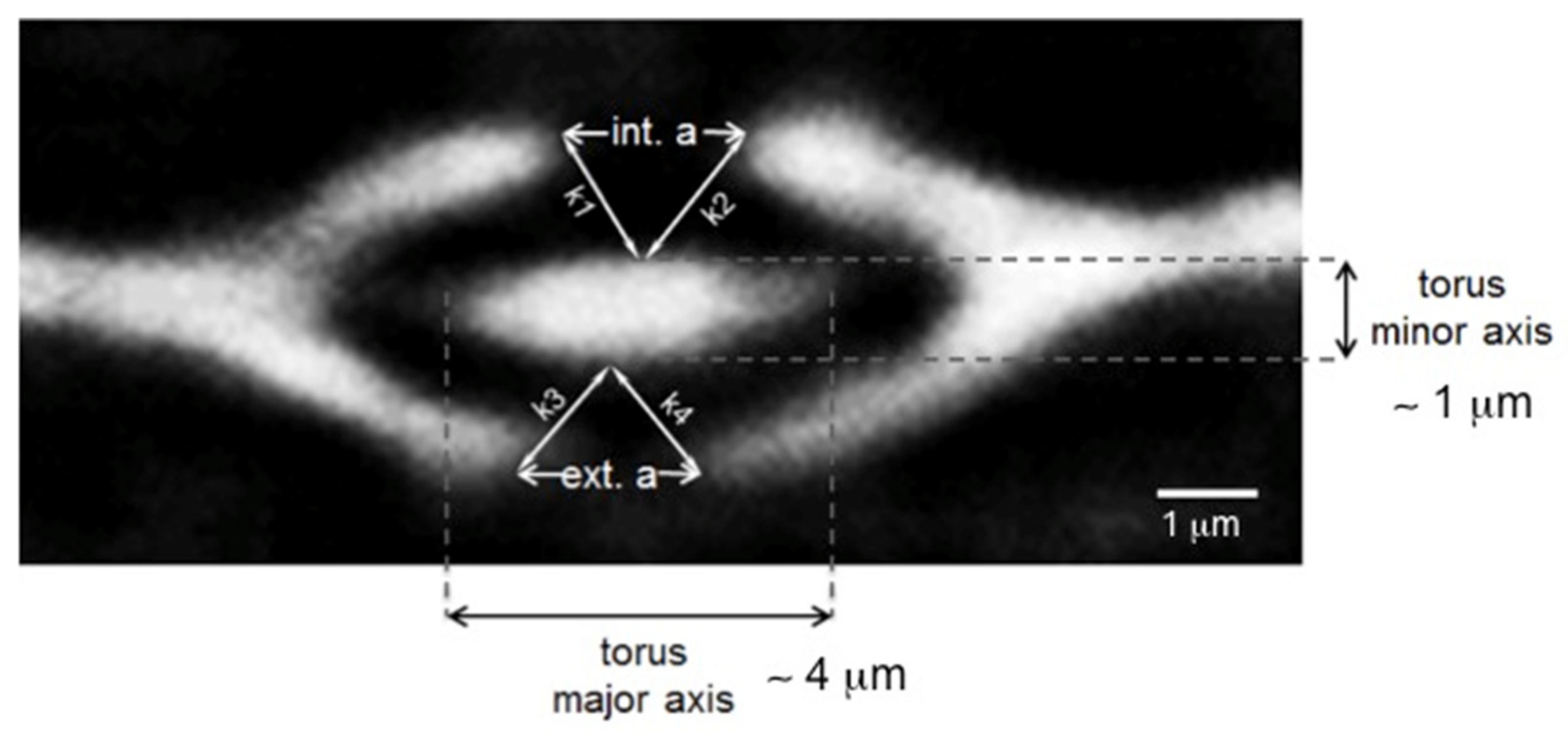
3.3. Anatomy of Bordered Pits in Earlywood Samples
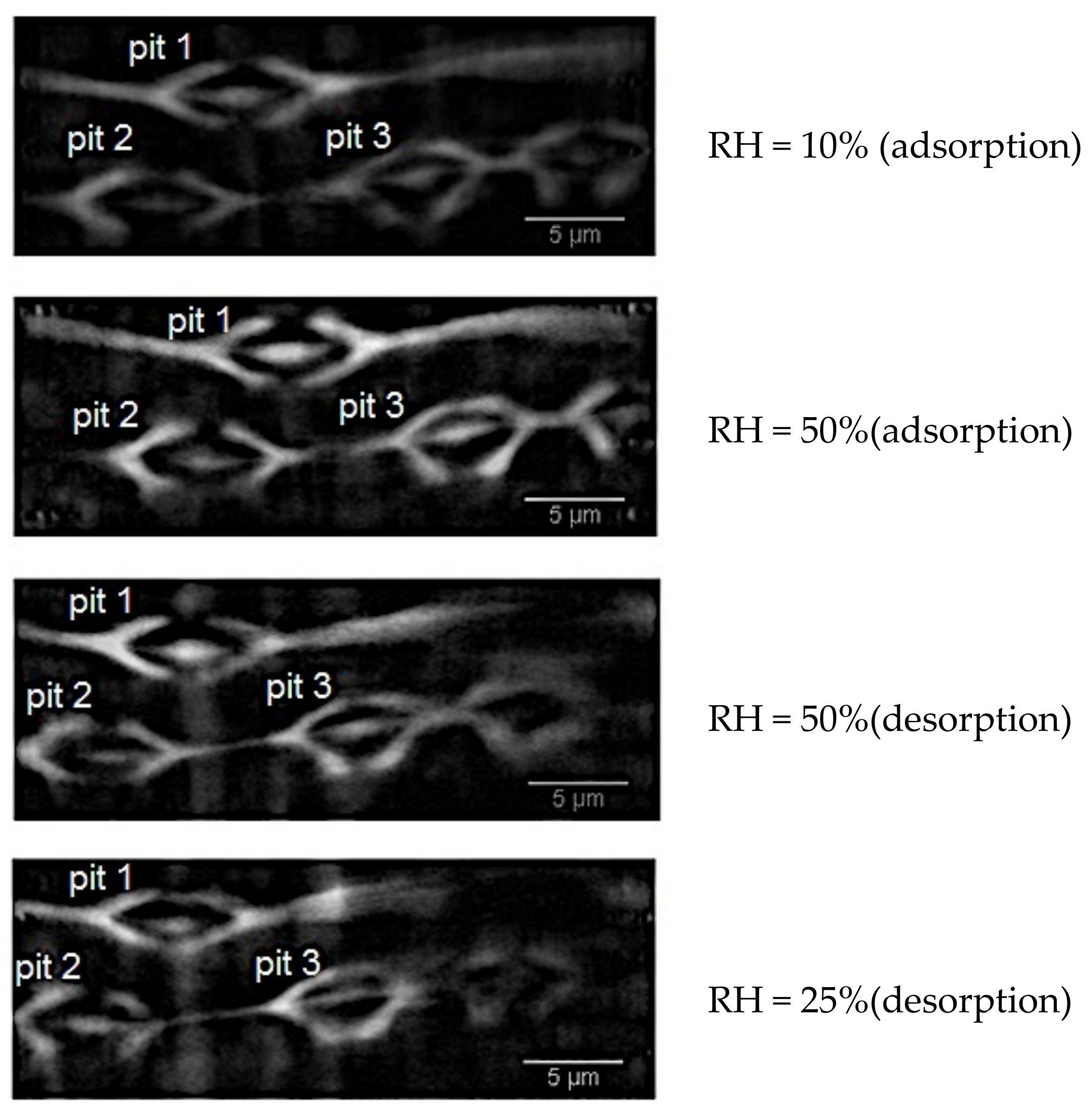
3.4. Behaviour of Bordered Pits during Moisture Sorption
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure & Properties; Pergamon Press: Oxford, UK, 1988. [Google Scholar] [CrossRef]
- Gorbacheva, G.A.; Ugolev, B.N.; Sanaev, V.; Belkovskiy, S.Y.; Gorbachev, S.A. Methods of characterization of memory effect of wood. Pro Ligno 2015, 11, 65–72. [Google Scholar]
- Ugolev, B.N. Wood as a natural smart material. Wood Sci. Technol. 2014, 48, 553–568. [Google Scholar] [CrossRef]
- Murata, K.; Masuda, M. Observation of the Swelling Behavior of Coniferous Cells Using a Confocal Scanning Laser Microscope and a Digital Image Correlation Method. J. Soc. Mater. Sci. Jpn. 2001, 50, 200–205. [Google Scholar] [CrossRef]
- Murata, K.; Masuda, M. Microscopic observation of transverse swelling of latewood tracheid: Effect of macroscopic/mesoscopic structure. J. Wood Sci. 2006, 52, 283–289. [Google Scholar] [CrossRef]
- Gu, H.; Zink-Sharp, A.; Sell, J. Hypothesis on the role of cell wall structure in differential transverse shrinkage of wood. Holz Roh Werkst. 2001, 59, 436–442. [Google Scholar] [CrossRef] [Green Version]
- Sakagami, H.; Matsumura, J.; Oda, K. Shrinkage of Tracheid Cells With Desorption Visualized by Confocal Laser Scanning Microscopy. IAWA J. 2007, 28, 29–37. [Google Scholar] [CrossRef] [Green Version]
- Derome, D.; Griffa, M.; Koebel, M.; Carmeliet, J. Hysteretic swelling of wood at cellular scale probed by phase-contrast X-ray tomography. J. Struct. Biol. 2011, 173, 180–190. [Google Scholar] [CrossRef]
- Derome, D.; Rafsanjani, A.; Patera, A.; Guyer, R.; Carmeliet, J. Hygromorphic behaviour of cellular material: Hysteretic swelling and shrinkage of wood probed by phase contrast X-ray tomography. Philos. Mag. 2012, 92, 3680–3698. [Google Scholar] [CrossRef]
- Patera, A.; Derome, D.; Griffa, M.; Carmeliet, J. Hysteresis in swelling and in sorption of wood tissue. J. Struct. Biol. 2013, 182, 226–234. [Google Scholar] [CrossRef]
- Hernández, R. Influence of moisture sorption history on the swelling of sugar maple wood and some tropical hardwoods. Wood Sci. Technol. 1993, 27, 337–345. [Google Scholar] [CrossRef]
- Thomas, L.H.; Altaner, C.M.; Forsyth, V.T.; Mossou, E.; Kennedy, C.J.; Martel, A.; Jarvis, M.C. Nanostructural deformation of high-stiffness spruce wood under tension. Sci. Rep. 2021, 11, 453. [Google Scholar] [CrossRef]
- Moghaddam, M.S.; Bulcke, J.V.D.; Wålinder, M.; Claesson, P.M.; Van Acker, J.; Swerin, A. Microstructure of chemically modified wood using X-ray computed tomography in relation to wetting properties. Holzforschung 2017, 71, 119–128. [Google Scholar] [CrossRef] [Green Version]
- Patera, A.; Bulcke, J.V.D.; Boone, M.N.; Derome, D.; Carmeliet, J. Swelling interactions of earlywood and latewood across a growth ring: Global and local deformations. Wood Sci. Technol. 2017, 52, 91–114. [Google Scholar] [CrossRef]
- Derome, D.; Zillig, W.; Carmeliet, J. Variation of measured cross-sectional cell dimensions and calculated water vapor permeability across a single growth ring of spruce wood. Wood Sci. Technol. 2011, 46, 827–840. [Google Scholar] [CrossRef]
- Rafsanjani, A.; Stiefel, M.; Jefimovs, K.; Mokso, R.; Derome, D.; Carmeliet, J. Hygroscopic swelling and shrinkage of latewood cell wall micropillars reveal ultrastructural anisotropy. J. R. Soc. Interface 2014, 11, 20140126. [Google Scholar] [CrossRef]
- Patera, A.; Jefimovs, K.; Rafsanjani, A.; Voisard, F.; Mokso, R.; Derome, D.; Carmeliet, J. Micro-Scale Restraint Methodology for Humidity Induced Swelling Investigated by Phase Contrast X-Ray Tomography. Exp. Mech. 2014, 54, 1215–1226. [Google Scholar] [CrossRef]
- Stampanoni, M.; Mokso, R.; Marone, F.; Vila-Comamala, J.; Gorelick, S.; Trtik, P.; Jefimovs, K.; David, C. Hard X-ray 3D phase-contrast nanoimaging. Phys. Rev. B 2010, 81, 140105. [Google Scholar] [CrossRef] [Green Version]
- Marone, F.; Münch, B. Fast reconstruction algorithm dealing with tomography artifacts. In Developments in X-ray Tomography VII, Conference Proceedings, Developments in X-Ray Tomography VII, San Diego, CA, USA, 24 August 2010; International Society for Optics and Photonics: Bellingham, WA, USA, 2010; Volume 7804, p. 780410. [Google Scholar]
- Mokso, R.; Quaroni, L.; Marone, F.; Irvine, S.; Vila-Comamala, J.; Blanke, A.; Stampanoni, M. X-ray mosaic nanotomography of large microorganisms. J. Struct. Biol. 2012, 177, 233–238. [Google Scholar] [CrossRef]
- Patera, A.; Carl, S.; Stampanoni, M.; Derome, D.; Carmeliet, J. A non-rigid registration method for the analysis of local deformations in the wood cell wall. Adv. Struct. Chem. Imaging 2018, 4, 1. [Google Scholar] [CrossRef] [Green Version]
- Abd-Elmoniem, K.Z.; Stuber, M.; Prince, J.L. Direct three-dimensional myocardial strain tensor quantification and tracking using zHARP. Med. Image Anal. 2008, 12, 778–786. [Google Scholar] [CrossRef] [Green Version]
- Yao, J. Shrinkage properties of second-growth southern yellow pine. Wood Sci. Technol. 1969, 3, 25–39. [Google Scholar] [CrossRef]
- Pang, S.; Herritsch, A. Physical properties of earlywood and latewood of Pinus radiata D. Don: Anisotropic shrinkage, equilibrium moisture content and fibre saturation point. Holzforschung 2005, 59, 654–661. [Google Scholar] [CrossRef]
- Patera, A. 3D Experimental Investigation of the Hygro-Mechanical Behaviour of Wood at Cellular and Sub-Cellular Scales. Ph.D. Thesis, ETH Zurich, Zürich, Switzerland, 2014. [Google Scholar]
- Burgert, I.; Fratzl, P. Plants control the properties and actuation of their organs through the orientation of cellulose fibrils in their cell walls. Integr. Comp. Biol. 2009, 49, 69–79. [Google Scholar] [CrossRef]

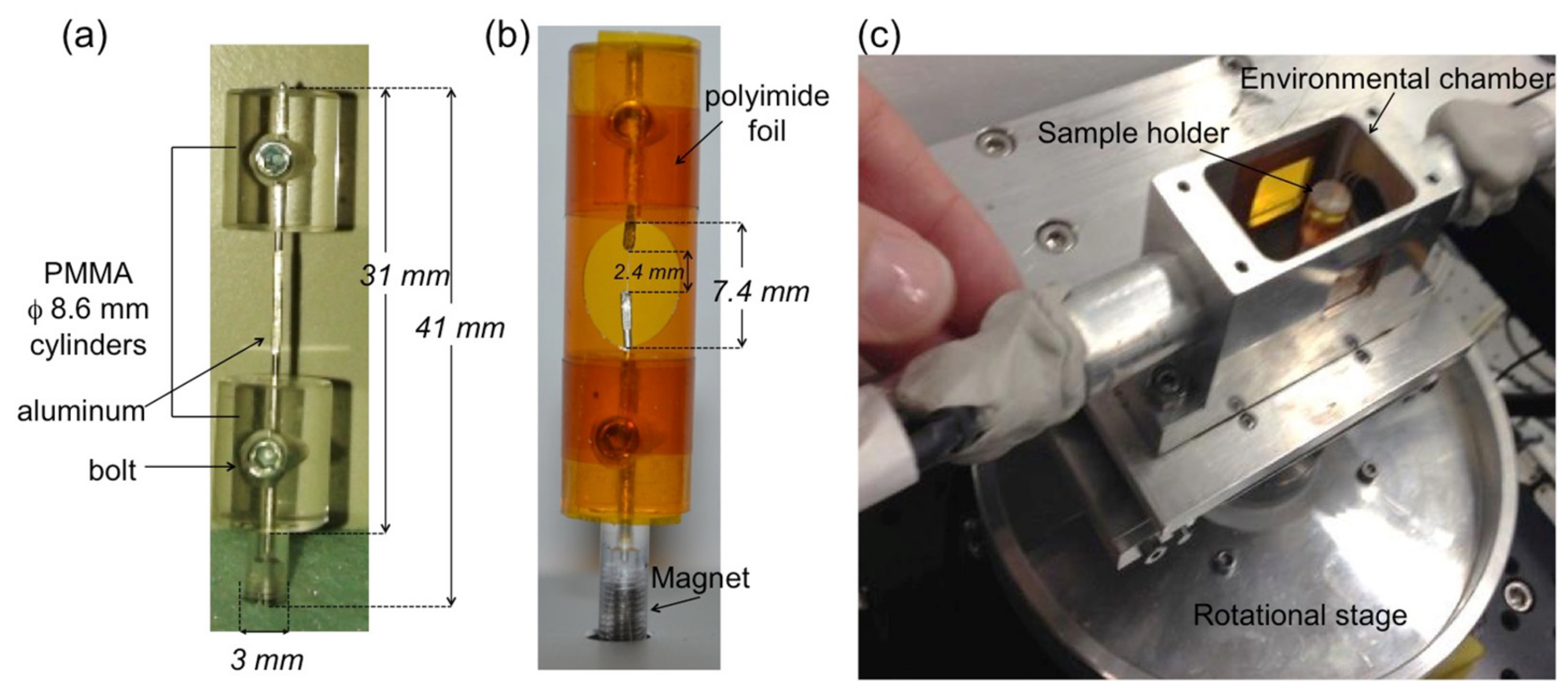
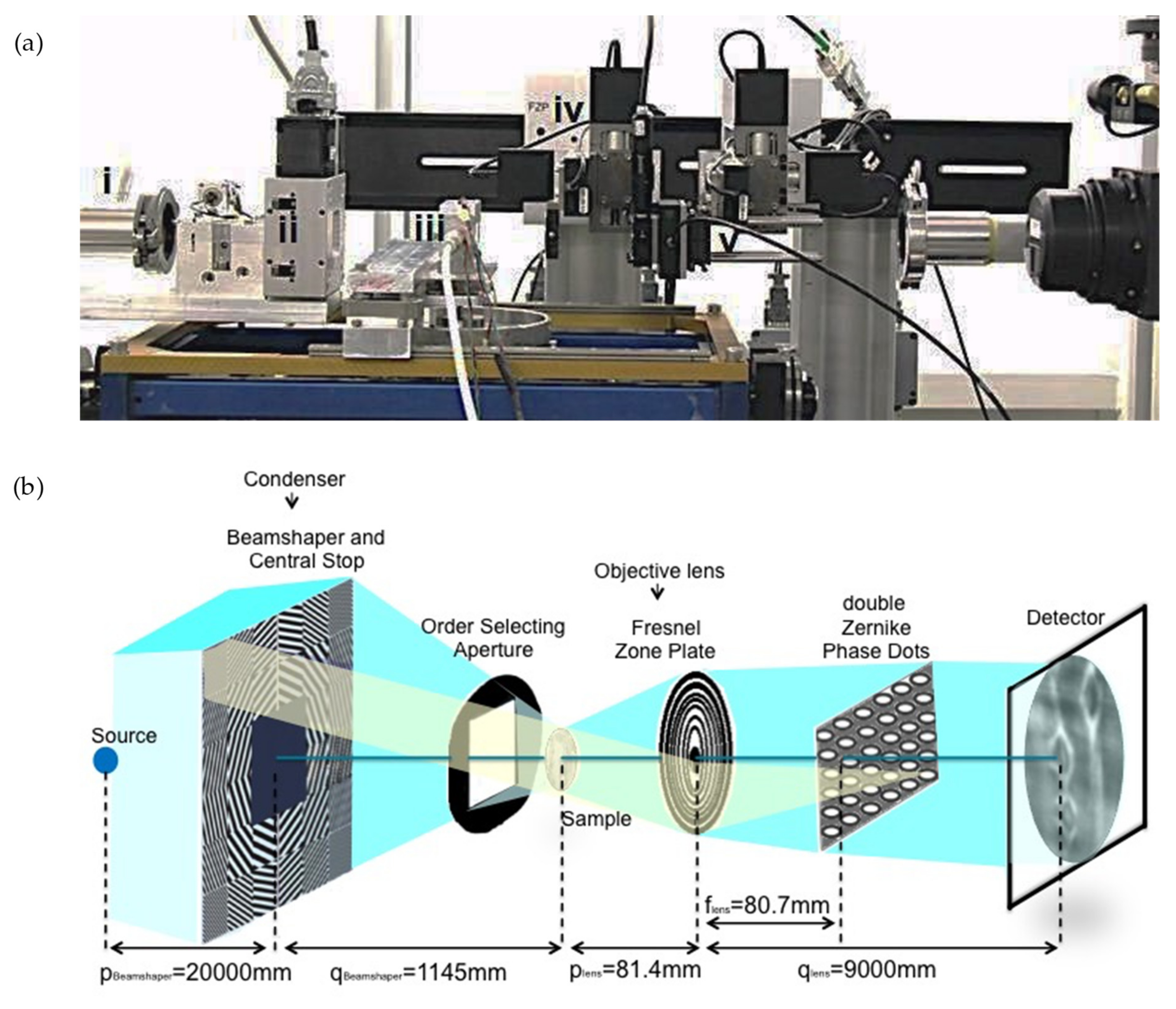

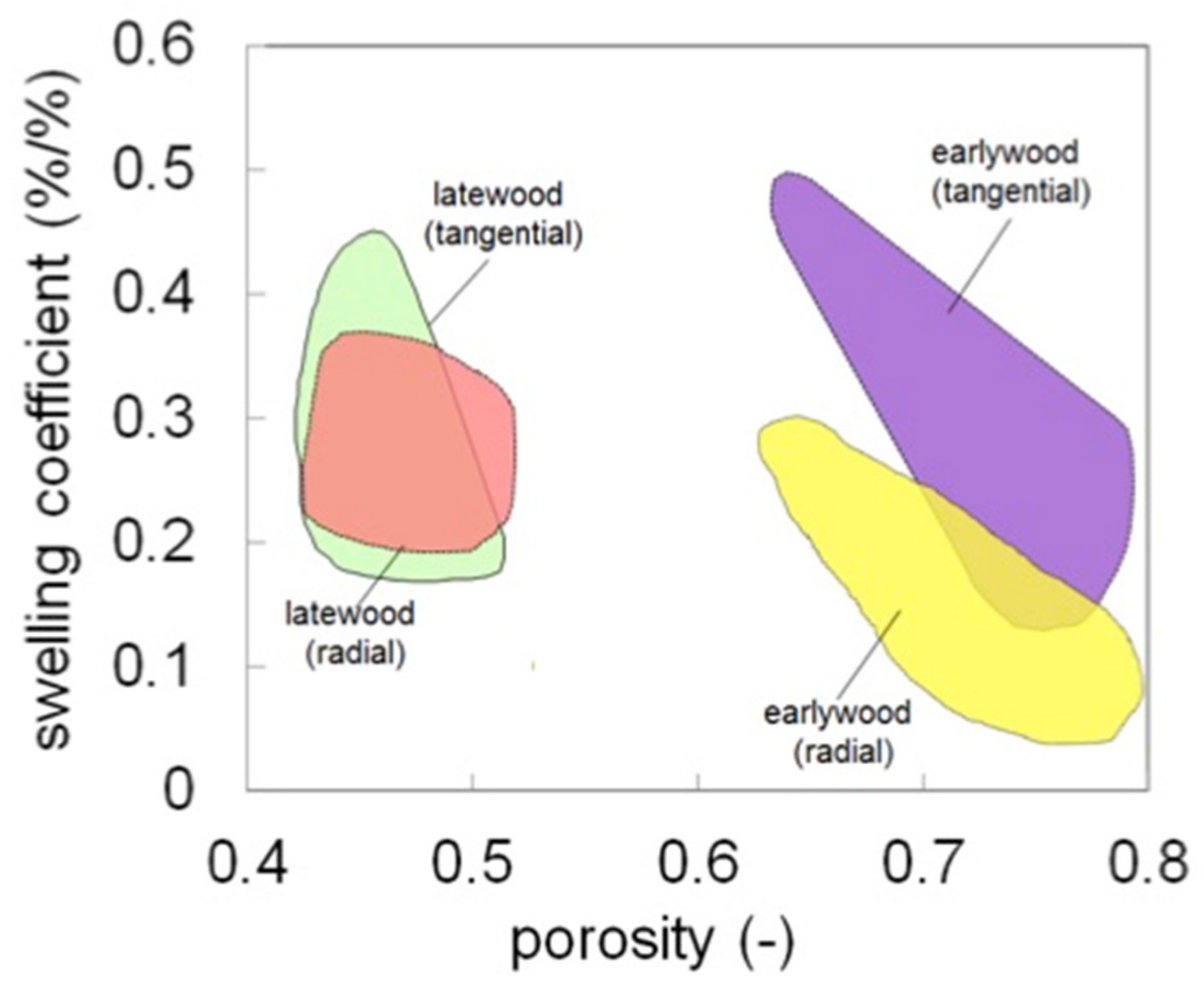
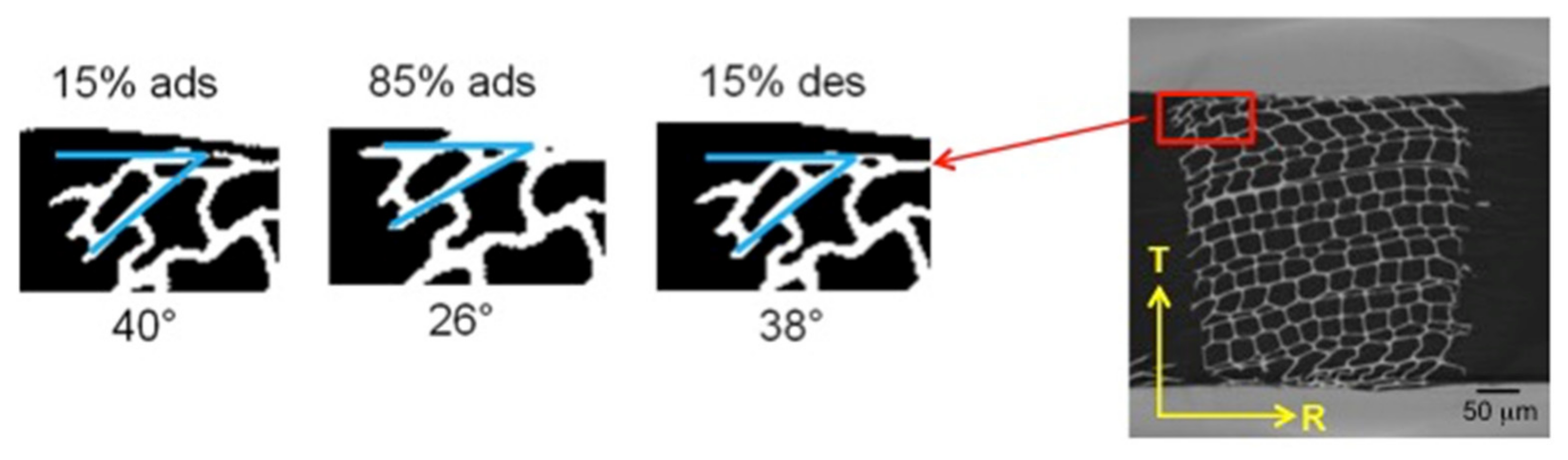



| Study (1) Cases: | Earlywood (EW) | Latewood (LW) | Dimension [T × R × L] |
|---|---|---|---|
| (1.a) | EW1, EW2 | LW1, LW2 | 500 μm × 500 μm × 8 mm |
| (1.b) | EWt | LWt, LWr | 500 μm × 500 μm × 2 mm |
| Sample Name | Sample A | Sample B | Sample C |
|---|---|---|---|
| pits no. | 3 | 1 | 1 |
| initial state | dried | green | dried |
| dimensions | 50 μm × 50 μm × 3 mm | 50 μm × 50 μm × 3 mm | 50 μm × 50 μm × 3 mm |
| Study | Cellular Scale | Sub-Cellular Scale | |
|---|---|---|---|
| Case 1.a | Case 1.b | Case 2 | |
| Detector | PCO 2000 | PCO 2000 | Photonic Science VHR Image Star |
| FOV [μm2 × μm2] | 757 × 757 | 757 × 757 | 12 × 12 * |
| Binning | 1 | 2 | 1 |
| Effective Pixel size [μm] | 0.37 | 0.74 | 0.084 |
| Exposure time [ms] | 75 | 40 | 1200 |
| SDD [mm] | 30 | 20 | see Figure 3 |
| Projections nr. | 1001 | 1001 | 541 |
| X-ray Energy [keV] | 20 | 20 | 12 |
| Case (Cellular Scale) | Sample Name | Porosity [%] |
|---|---|---|
| Case 1.a) | EW1 | 78 |
| EW2 | 64 | |
| LW1 | 50 | |
| LW2 | 45 | |
| Case 1.b) | EWT | 65 |
| LWT | 54 | |
| LWR | 60 |
| Case 1.a) | Case 1.b) | |||
|---|---|---|---|---|
| Anisotropy | Free Swelling | Restrained Swelling | ||
| 1 | 2 | ROI 1 | ROI 2 | |
| EW | 3.10 | 1.70 | EWT:2.40 | EWT:0.25 |
| LW | 1.30 | 1.10 | LWT:0.80 LWR:5.30 | LWT:0.50 |
| State | RH = 10% (ads.) | RH = 50% (ads.) | RH = 50% (des.) | RH = 25% (des.) |
|---|---|---|---|---|
| k1 ± σ [μm] | 1.00 ± 0.02 | 1.20 ± 0.04 | 1.40 ± 0.03 | 1.42 ± 0.04 |
| k2 ± σ [μm] | 1.22 ± 0.05 | 1.36 ± 0.05 | 1.38 ± 0.06 | 1.60 ± 0.08 |
| k3 ± σ [μm] | 1.23 ± 0.18 | 1.08 ± 0.04 | 1.05 ± 0.05 | 1.00 ± 0.04 |
| k4 ± σ [μm] | 1.54 ± 0.05 | 1.20 ± 0.05 | 1.03 ± 0.05 | 0.90 ± 0.05 |
| Measurements | W | D | 2b | 2k |
|---|---|---|---|---|
| Value ± 0.05 [μm] | 3.40 | 16.40 | 4.25 | 4.50 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patera, A.; Bonnin, A.; Mokso, R. Micro- and Nano-Scales Three-Dimensional Characterisation of Softwood. J. Imaging 2021, 7, 263. https://doi.org/10.3390/jimaging7120263
Patera A, Bonnin A, Mokso R. Micro- and Nano-Scales Three-Dimensional Characterisation of Softwood. Journal of Imaging. 2021; 7(12):263. https://doi.org/10.3390/jimaging7120263
Chicago/Turabian StylePatera, Alessandra, Anne Bonnin, and Rajmund Mokso. 2021. "Micro- and Nano-Scales Three-Dimensional Characterisation of Softwood" Journal of Imaging 7, no. 12: 263. https://doi.org/10.3390/jimaging7120263
APA StylePatera, A., Bonnin, A., & Mokso, R. (2021). Micro- and Nano-Scales Three-Dimensional Characterisation of Softwood. Journal of Imaging, 7(12), 263. https://doi.org/10.3390/jimaging7120263






