1. Introduction
Lithium-ion batteries (LIBs) are used in mobile consumer devices (e.g., smartphones, tablets, and laptops) and also in the electrification of powertrains in passenger cars and commercial vehicles. [
1] Compared to other battery technologies, LIBs represent a key technology due to their favorable properties such as the high specific energy density (160–180 Wh/kg) as well as the good long-term stability (SOH over 80% after more than 2000 cycles) [
1,
2,
3,
4,
5,
6,
7]. For the success of electromobility, it is therefore imperative to produce high-quality batteries and to keep production costs as low as possible. Electrolyte filling and forming are relatively cost-intensive process steps at the end of the production chain due to high material costs and long process time [
8]. Thus, precise, fast, and nondestructive quality tests on the dry cell stack contribute to early failure identification and consequently, to cost savings. Besides investigating electrodes, quality tests are also applied to examine the separator quality. The separator is a polymeric membrane, coated with ceramic materials for some applications, that allows the transport of lithium ions while impeding short-cuts between anode and cathode [
9]. Consequently, the separator is functionally crucial for the battery cell, and separator defects may result in a local hot spot as well as lead to a thermal runaway of the cell [
10]. Therefore, quality control as well as detection of defects and poor insulation performance of the separator are essential and have a significant influence on costs, service life, and safety of cells [
11,
12].
In addition to standard quality control done by the materials manufacturers, the separator and the electrodes are typically subject to optical quality control in the cell assembly process [
1,
13,
14]. Most of the approaches are based on online scan camera systems. This enables the detection of defects in the range of a few hundred micrometers in the components and a reliable sorting out of defects outside acceptable ranges. Because of the huge variety of separators, the implementation of vision systems in the production processes causes high manual adaptation efforts. Similarly, defects introduced during the assembly and the production process cannot be identified. For example, melting particles can be generated during the laser cutting process and accumulate on the electrodes. This can result in an electrical short circuit and is, therefore, a safety-critical condition of the battery cell. In this case, the applied optical defect detection is limited due to the different sizes and materials of the particles [
15]. Other nondestructive methods for inspecting the separator for holes and particles are based on ultrasound or airflow [
16,
17]. The ultrasound inspection allows to locate and visualize the defects in flat and thin battery cells, e.g., pouch-cell. However, an ultrasound measurement currently still takes several minutes [
14]. The nondestructive method based on the airflow resistance of a separator can identify holes and cracks since defects have a negative influence on the airflow resistance and enable the air to pass through the separator. This measurement can also be used to identify the quantity and size of the defects. However, it does not cover the electrical insulation performance of the separator [
17].
Partial discharge (PD) is a known process within electrical insulation layers when high-potential stress is applied to the insulating material. Partial discharges are electrical discharges in only a partial area of the insulation. They do not immediately lead to breakdown, but to erosion and aging. PDs increasingly deteriorate the insulation [
18]. It usually starts with voids, cracks, or inclusions and can eventually lead to a complete breakdown of the insulation capacity. While partial discharge is commonly used to inspect electrical equipment, it can be potentially used as an electrical nondestructive test of battery cells to detect defects in the separator layer, e.g., pinholes and particle contamination. Within the dielectric separator material, small air-filled voids can lead to an increased electrical field due to the smaller dielectric constant of air compared with the separator material. If this local electric field strength around the air void surpasses the corona inception voltage, partial discharge is initiated, and high-frequency transient current pulses will appear. Thereby, defects in the separator layer weaken the insulation performance and reduce the electrical voltage where breakdowns occur. As such, partial discharge can be potentially used to test the insulation resistance of the separator. If no breakdown occurs at a preselected voltage, the test is passed [
19]. Nevertheless, there are no standards or legal requirements for carrying out the test, but the insulation must have a dielectric strength specified in the standard [
20]. Various institutions and safety agencies demand a HiPot Test with a voltage of 500 V, so that consumers are not endangered by the products they use.
In summary, no fast and reliable nondestructive electrical quality test for the detection of pinholes and particles deposition is available for battery cell production. To close this gap, we aim to provide an early detection method of separator defects in the battery production and evaluate high-potential tests. For that, partial discharge was measured with a high-potential test on dry battery cell stacks consisting of anode, separator, and cathode layers. The methodology as well as the test requirements and conditions are discussed in
Section 2. Clean reference cell stacks with no particle contamination were compared with cell stacks including metal particles and holes. The failure rate detection was evaluated for both the clean reference cell stacks and the contaminated cell stacks. Various experimental parameters, including the mechanical pressure of the cell stack, were investigated, and an equivalent circuit model was established. Moreover, finite element modeling was developed to analyze the local electrical field strengths of air voids and particles, with the dielectric constant of the separator measured by an accurate electrical probe kit, as described in
Section 3. On this basis, recommendations for the establishment of the high-potential test as a quality test for separators in battery cell production are derived.
3. Results and Discussion
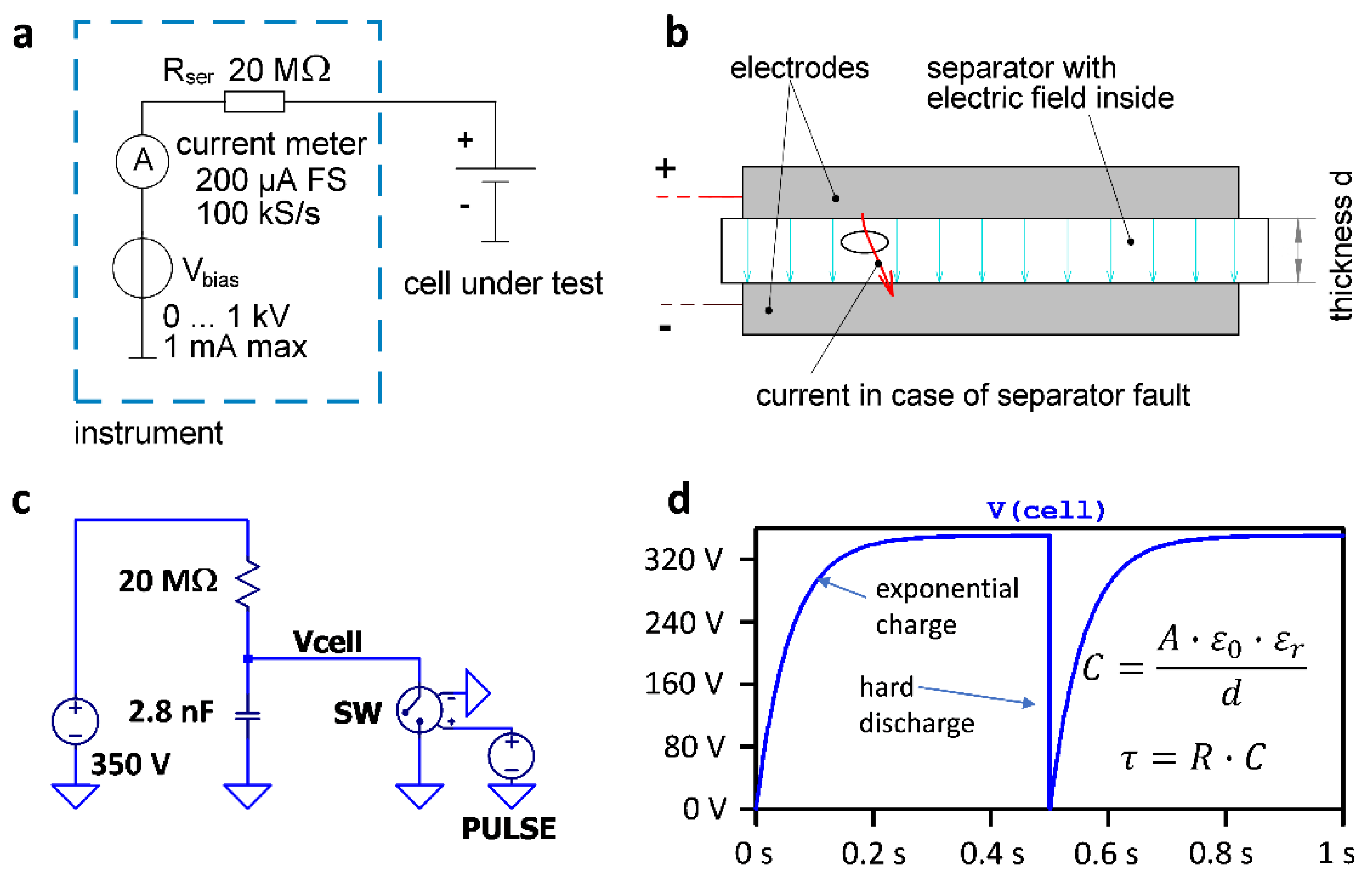
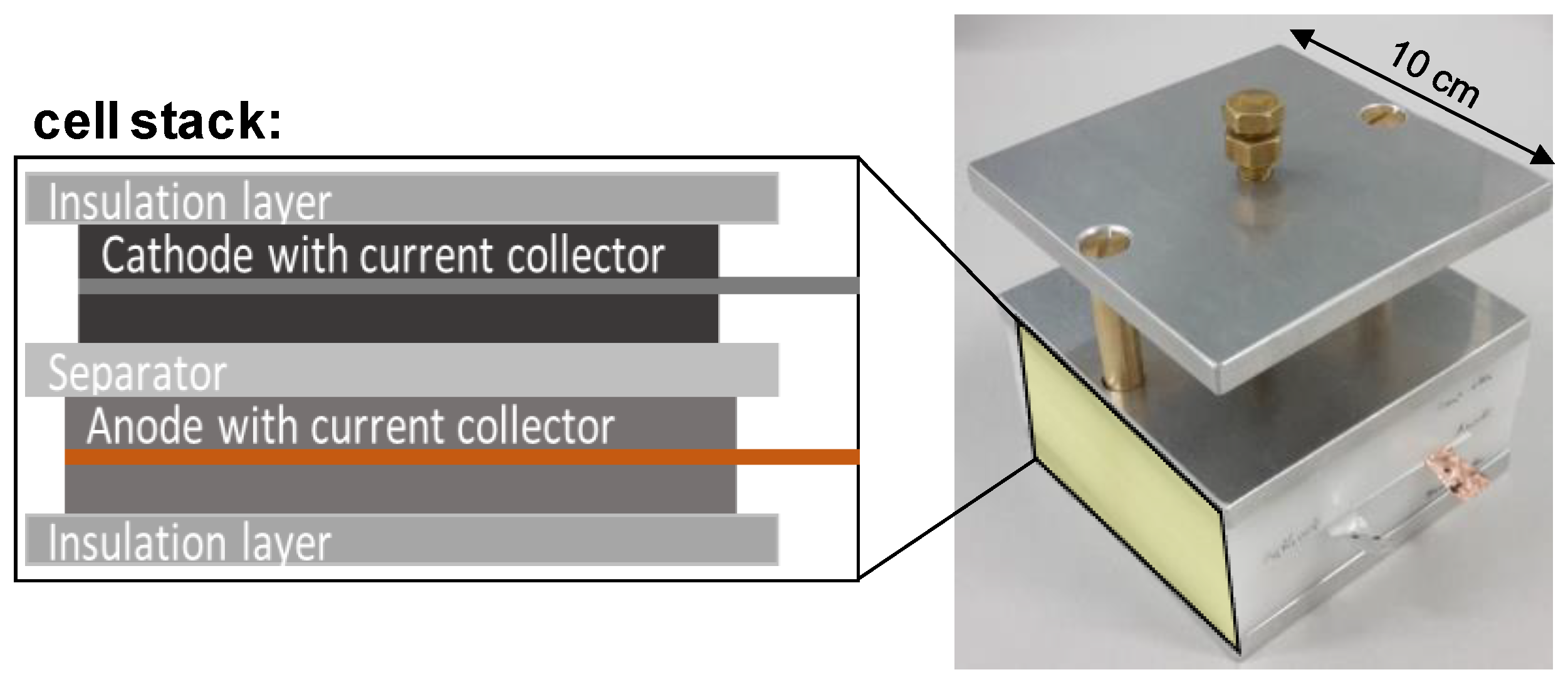
Figure 1a shows the electrical schematic of the measurement setup with a conceptual sketch of the cell stack including a dielectric separator sandwiched between two metallic electrodes and insulation layers (
Figure 1b). The electrodes are highly conductive and form equipotential planes according to the applied voltage, V. The distance, d, of the electrodes is given by the separator thickness, and the electric field strength, E, in volts per meter in the separator is calculated by Equation (6):
In case of a separator insulation defect, a local current path through the separator is created that connects the positive and the negative electrodes. This results in an abrupt discharge of the cell capacitance with a high-current pulse, which is flowing locally in the cell and is not accessible from outside. The current-meter measures the external current on the electrode terminals, which is also flowing through the insulation defect.
Figure 1c shows a SPICE simulation model used to study the transient process of a separator discharge. In the beginning, V
cell charges up exponentially. At a certain time, the discharge pulse occurs and the voltage immediately drops to zero and starts again to rise exponentially with a time constant of τ = 32 ms.
For the experimental discharge measurements, clean cell stacks with defect-free separators and electrodes as well as cell stacks with forced defects were used. We focused on two forced defects that typically can occur also during cell production to determine their effects on the electrical discharge behavior. Firstly, particle contaminations between the separator and the electrodes were introduced. For this, after the clean cell stack was manufactured in the dry room, particles varying from 10 to 20 µm diameter were deposited by hand with laboratory tweezers on the separator and the electrodes [
1,
24]. Secondly, micrometer-sized holes were investigated that arise from particles squeezed between the electrodes and the separator.
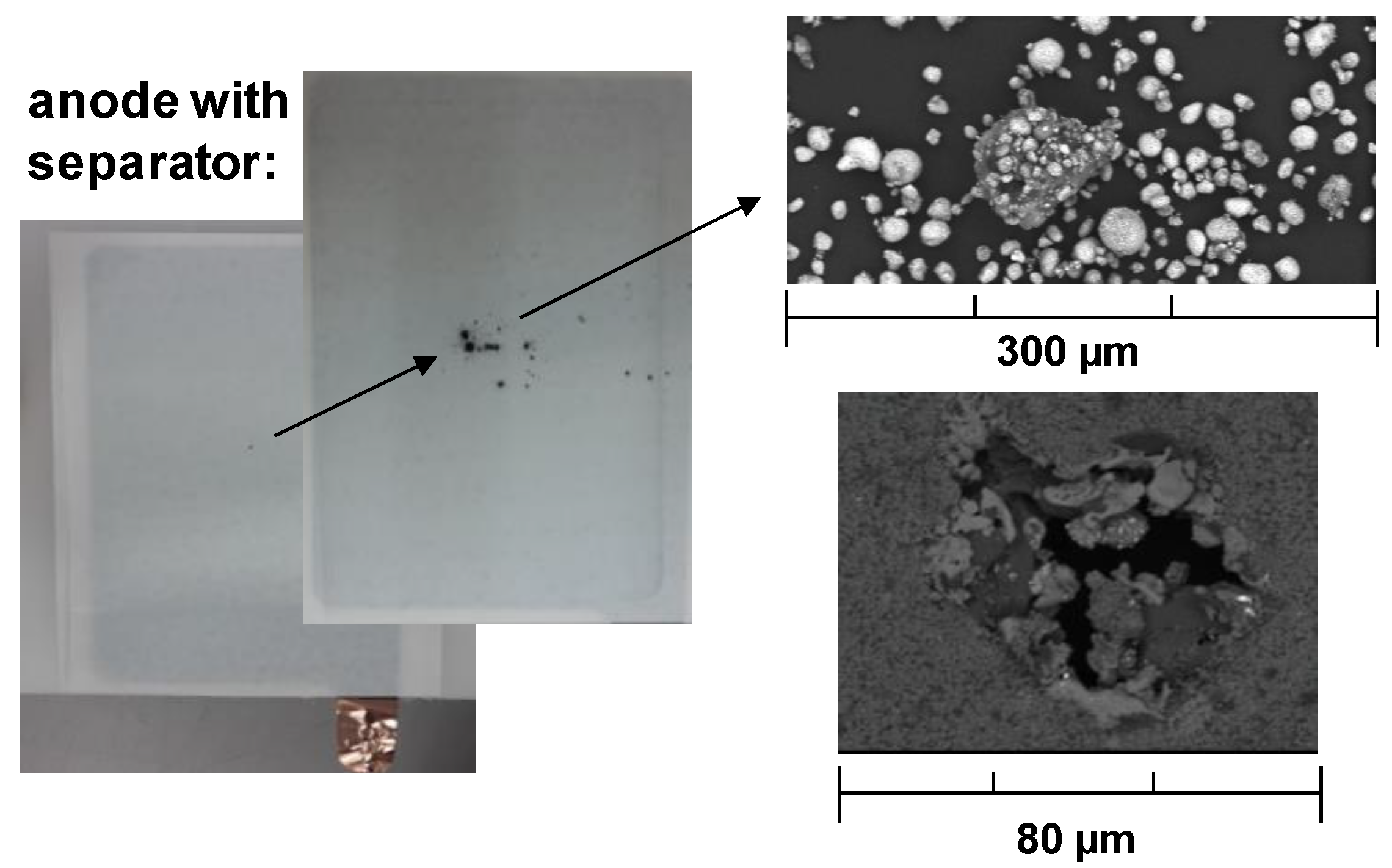
Figure 3 shows optical images (left) and SEM images (right) of the two defect categories before and after the electrical discharge measurements.
The upper right panel in
Figure 3 shows a mixture of small particles (~10–20 µm diameter) and a single large agglomerated particle (~80 µm diameter) placed on the separator and imaged before the discharge measurement. The particles were derived from laser cutting of cathode material. The lower right panel in
Figure 3 shows a hole with a diameter of ~50 µm created during the measurement of cell stacks with particles. In the manufacturing pilot lines, the main process responsible for small-particle contamination is usually the cutting of electrode sheets. When the electrode sheets are cut with a laser, melt splashes can remain on the electrodes or the evaporated collector material can deposit in the form of metal spots. In addition, during punching, chipped particles and burrs can deposit on the electrode [
1]. The particles can then penetrate the separator during cell production and cause electrical contact of the electrodes, causing electrical discharge and shortcuts. Furthermore, small microscopic damages in the separator can develop into larger holes due to the so-called “running mesh effect” caused by mechanical stresses during subsequent manufacturing process steps [
25].
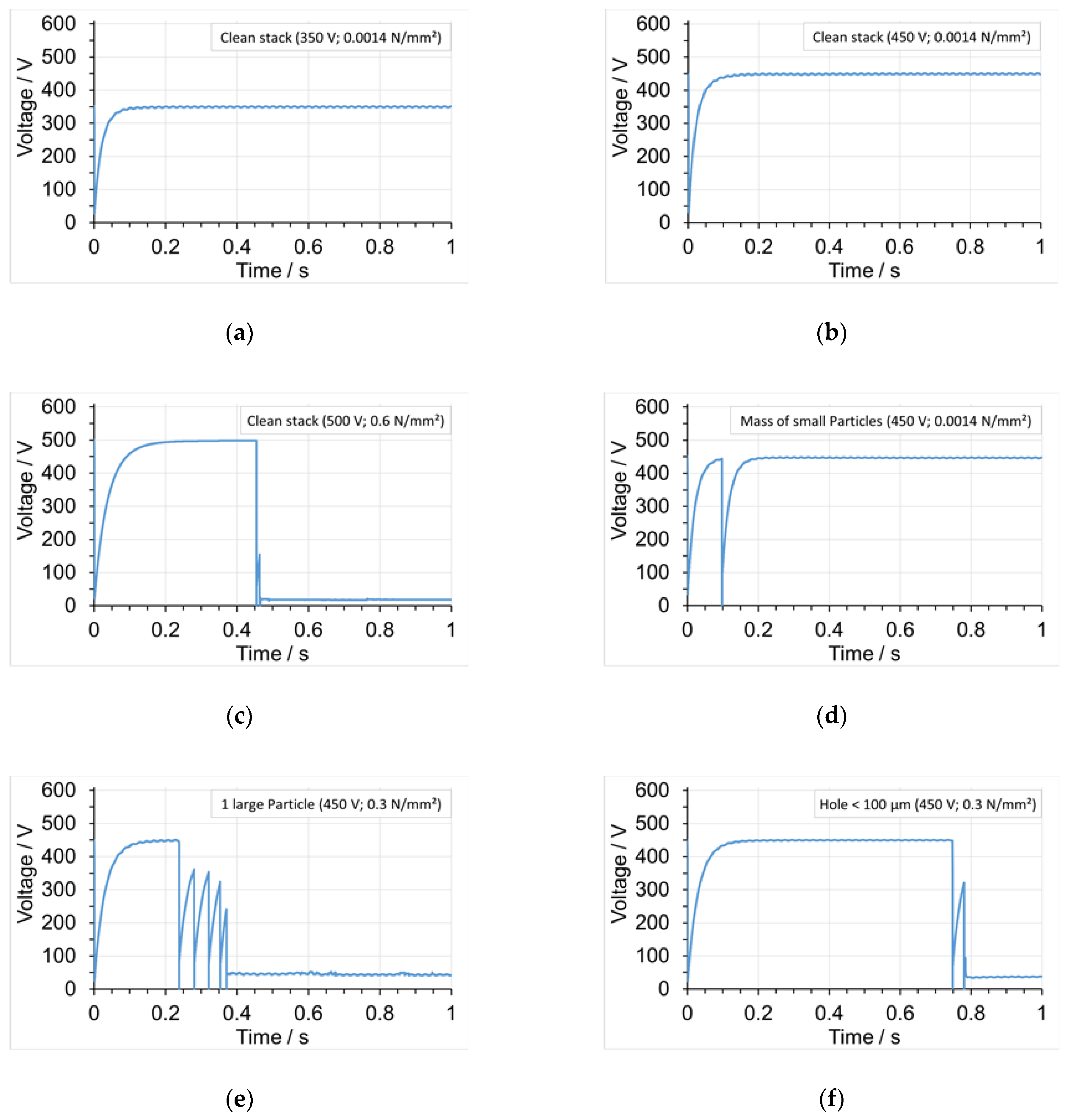
Figure 4 shows different types of electrical discharges measurements on reference cell stacks with no contamination
Figure 4a–c in comparison with cell stacks that have forced defects (particles and holes) at different mechanical pressures and voltages
Figure 4d–f. The forced defects were distinguished between a mass of small particles with 10–20 µm diameter, a single large particle with approximately 80 µm diameter, and holes with diameters of 10–100 µm. The voltage curves of clean cell stacks showed no breakdown at 350 V and 450 V (
Figure 4a,b), while hard discharge (HD) occurred only above 500 V.
Figure 4c shows a clean stack with HD at 500 V and 0.6 N/mm
2 that led to a permanent loss of the electrical insulation performance and no voltage recovery. For cell stacks with forced defects, the breakdown voltage is lower than 500 V.
Figure 4d shows an HD at 450 V with full recovery in a cell stack with a mass of small particles. Here, the cell voltage charged exponentially up to 450 V and, after an HD at 0.1 s, it dropped momentarily to 0 V and the local current in the defect structure increased abruptly (current data not shown).
In full recoveries, the high current leads to a destructive change of the material and, consequently, to a physical disconnection of the defect from the rest of the cell, thereby allowing the cell voltage to recover, in this case, to the original value of 450 V. As such, the hard discharge caused by the defect was only transient and local, while the rest of the cell stack stayed functional. This is similar to the effect of self-healing known for metallic capacitors that can clear a faulty area when a momentary electrical short occurs due to dielectric breakdown under voltage [
26].
Figure 4e shows a more complex HD spectrum arising from a cell stack with a single large particle that was charged up to 450 V. Here, the single large particle led to a series of different defects in the electrode–separator composite that unfold subsequently in various discharge events. The voltage recovery from the individual discharge events occurred only partially, and recharging was possible only up to roughly 350 V in the first three events and 250 V in the last event, before it dropped irreversibly to 50 V. This indicates that several small defects in the separator have led, in the beginning, to minor electrical contacts between the electrodes and the separator, while in the end, the electrical insulation performance was lost to a large extent. In
Figure 4f, an HD is shown arising from a hole when charged up to 450 V. Only briefly was a partial voltage recovery observed, which quickly and irreversibly fell to the remaining small voltage of <50 V, indicating a significant failure in the separator, which caused a major electrical shortcut and complete loss of the insulation performance. After the electrical discharge experiments, some defects were analyzed in detail under SEM, and melt products from the separator material and the NMC cathode materials were identified. Typically, a contamination with a mass of small particles showed more HD events with recovery, whereas cell stacks with holes mostly showed no recovery. For cell stacks with contamination by a single large particle, both types of HD events were observed and statistically, no trend of a defect type was identified.
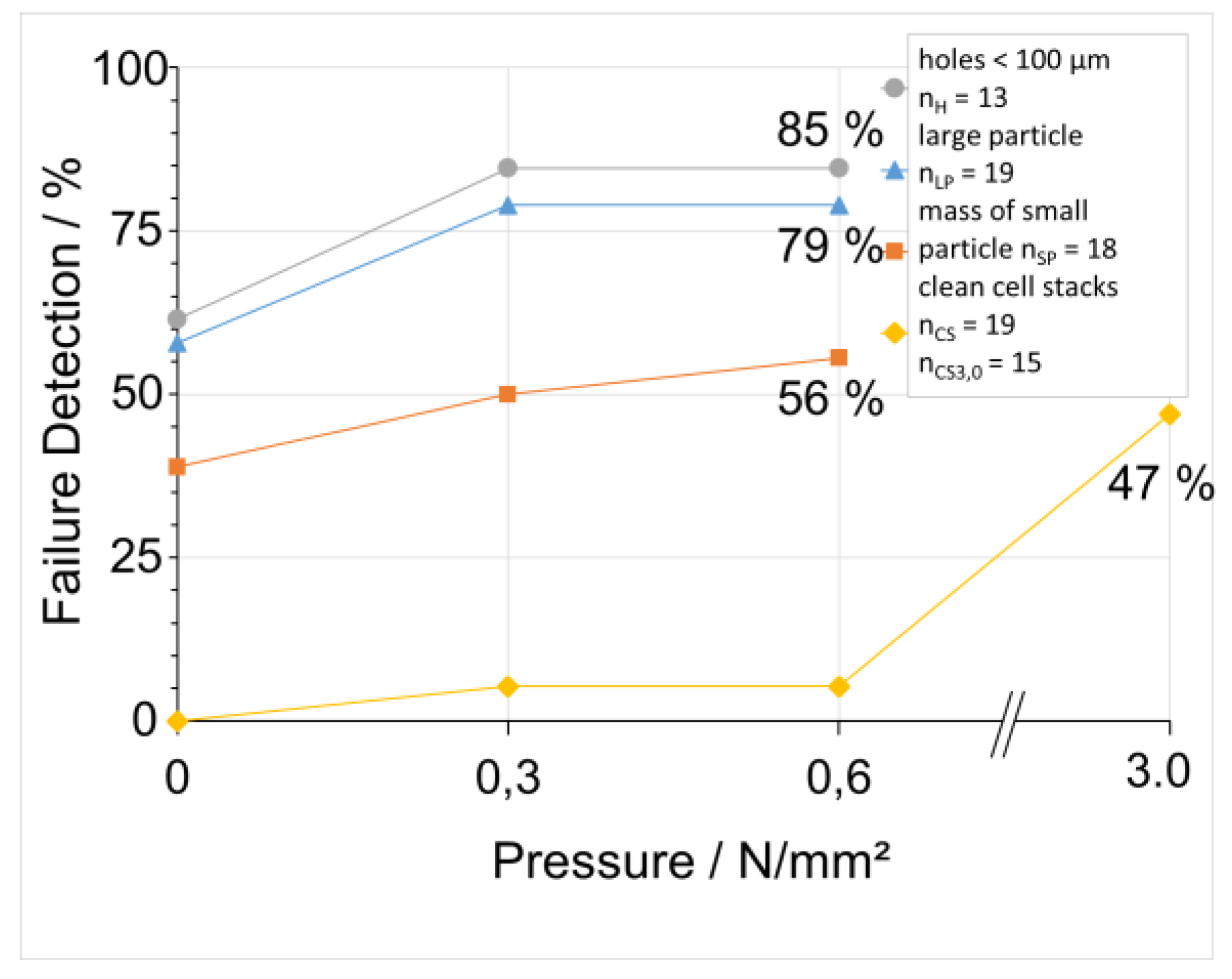
A quantitative assignment of the defect detection rate with respect to the different fault mechanisms and the various mechanical pressures is shown in
Figure 5. The defect detection rate was defined as the probability of observing an individual HD event in a voltage curve, independent of the type of HD. We compared the defect detection rate of clean cell stacks with contaminated cell stacks, including contamination with one large particle, a mass of small particles, and holes. Various cell stacks were prepared, and the number of cell stacks that were used for the experiments is given as inset. For instance, to evaluate the defect detection rate of a clean cell stack, 19 different clean cell stacks were assembled and measured at no pressure, p
I (0.0014 N/mm
2), at pressure p
II (0.3 N/mm
2), at pressure p
III (0.6 N/mm
2), and at pressure p
IV (3 N/mm
2). In the unpressed state, p
I, the failure detection rate was 0% for the clean cell stacks, while it was 40–60% for the cell stacks with defects, with the exact value of the defect detection rate depending on the type of contamination. Typically, the cell stacks with a large particle and holes showed the highest failure detection rates compared to the cell stacks with a mass of small particles. With an increase in the pressure to p
II, an increase in the defect detection rate to 50–85% was observed for all contaminations. Additionally, clean cell stacks showed a slightly increased defect rate of 5% at p
II. At a pressure of p
III, no major change in the defect detection rate was observed, for the contaminated cell stacks or for the clean cell stacks. As expected, for clean cell stacks, the defect detection rate was very low, and only when the pressure load was highly increased to p
IV. Moreover, a significant part (47%) of the clean reference cells showed HD and were damaged. Almost all contaminated cell stacks were damaged at this high pressure (data not shown). Accordingly, a high pressure of 3 N/mm
2 is not recommended for using this method as quality test because also clean cell stacks are destroyed.
A pressure ranging from 0.3 to 0.6 N/mm2 seems to be ideal for detecting contaminations without damaging clean cell stacks, leading therefore to a nondestructive investigation method. There was also a slight variation of the breakdown voltage where HD occurs with respect to the different mechanical pressures, and at 0.3 N/mm2, the mean breakdown voltage was around 420 V. For the test duration and data acquisition time, an optimum of 1 s was identified to keep the stress on the separator lowest. For instance, when repetitive measurements were done, the fault detection rate increased slightly because the stress on the cell stack accumulated.
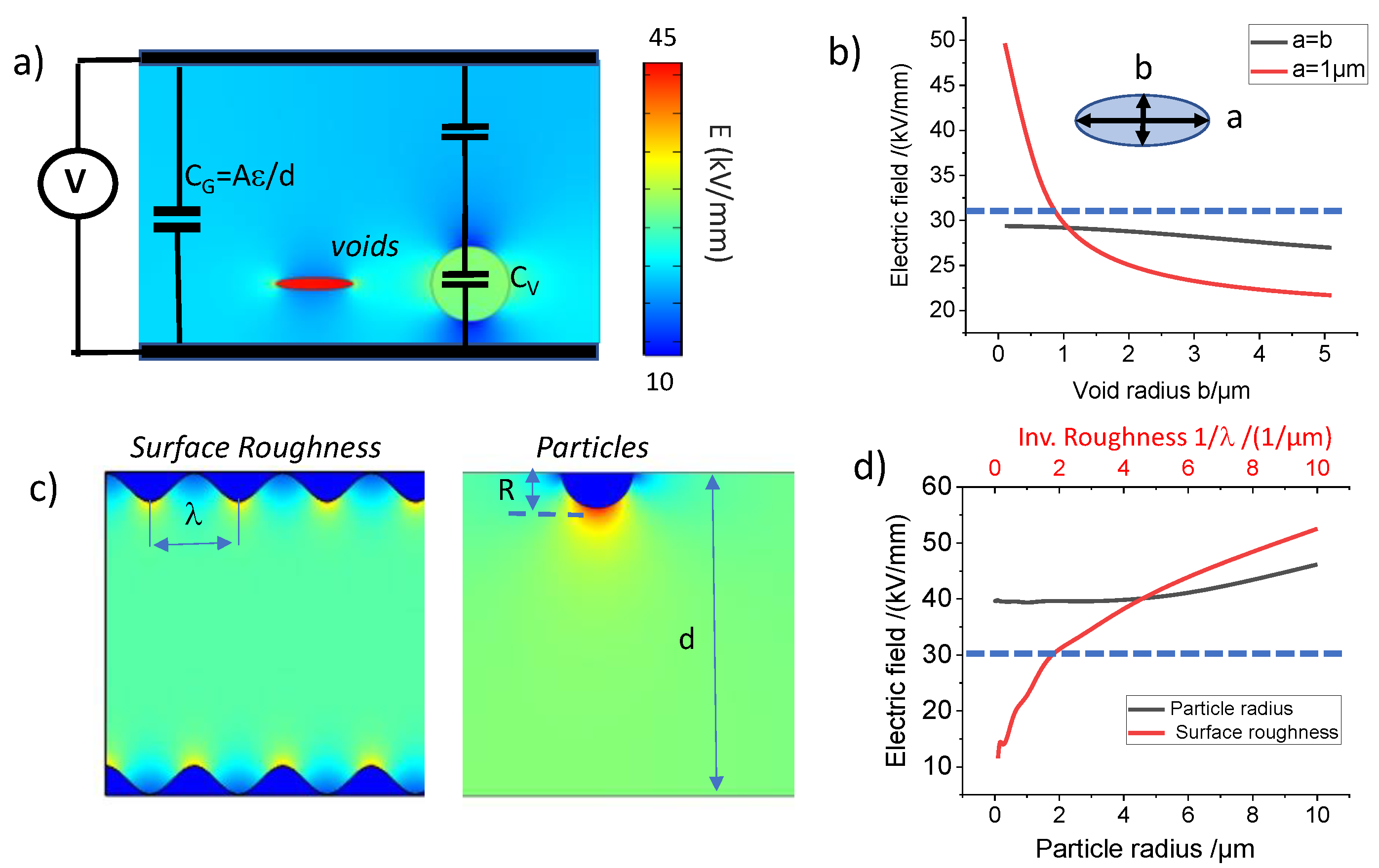
In order to obtain additional physical insights into the HD process, we conducted finite element modeling (FEM) of the defect structures and the corresponding electric fields (E-fields) (
Figure 6). According to the electrostatic fields within the cell stack, the probability of electric discharge is a function of the E-field strength between the two electrodes (
Figure 6a). The total cell stack capacitance (C
G) is described via an ideal parallel-plate capacitor model, C
G = A × e
r/d, with A the electrode area, e
r = 3 the dielectric constant of the separator, and d the thickness of the separator. The separator dielectric constant was measured using the parallel-plate dielectric probe kit [
26]. The voids in the separator, filled with air, decrease locally the dielectric permittivity (e
air = 1) and reduce the overall capacitance (C
V).
Figure 6a shows the calculated E-field distribution in a separator containing air voids, which led to locally increased E-field strengths of up to 20–50 kV/mm. Both the size and the ellipticity of the voids have an influence on the maximum E-field in the separator (
Figure 6b). For instance, the smaller and more elliptic the voids are, the higher is the E-field. A similar effect was observed for the surface roughness of the electrode–separator interface (
Figure 6c, left). The rougher the surface (i.e., the smaller the wavelength λ), the bigger is the E-field strength at the interface (
Figure 6d).
Figure 6c, right panel, shows the influence of a single contamination particle with radius R on the E-fields.
An increasing particle size leads to an effective decrease of the electrode separation distance and therefore, at a certain point, to an increase of the E-field (
Figure 6d). At a certain E-field strength, the probability of an electric breakdown in the separator rises nonlinearly, leading to an abrupt discharge event as observed in the experiments. For polyester thin films, this so-called “dielectric strength” is typically in the range of 20–50 kV/mm [
27]. The FEM results of air voids, electrode surface roughness, and contamination particles lead to E-fields in this range of 20–50 kV/mm, which is similar to the dielectric strength of the separator material used in this study, corroborating the observed failure detection rates of the various contaminated cell stacks.