Combining the Distribution of Relaxation Times from EIS and Time-Domain Data for Parameterizing Equivalent Circuit Models of Lithium-Ion Batteries
Abstract
:1. Introduction
1.1. Motivation
1.2. State of the Art
1.3. Contributions
- Evaluation of frequency- and time-domain measurements:To capture the full dynamic behavior of a cell, both EIS and TDM measurements have been considered in the ECM parameterization process and their DRT computed. Respective measurements in the frequency-domain as well as in the time-domain were performed (Section 2).
- Optimized calculation of the DRT via Tikhonov regularization:Since an accurate DRT is essential for the proposed parameterization process, the L-curve criterion was employed to optimize the calculation of the DRT via Tikhonov regularization. Moreover, the impact of different regularization terms on the calculated DRT was investigated (Section 3).
- Process for direct parameterization of RC elements from the DRT:Instead of using only partial information from the DRT to supplement a conventional fitting algorithm, the full potential of the DRT was exploited by a parameterization process which determines RC parameters directly from the DRT (Section 4).
- Analysis of various data merging approaches:Different methods of combining EIS and TDM data in the parameterization process are presented in this work. One of those methods includes a new way of calculating the DRT using TDM and EIS simultaneously, uniting the steps of DRT calculation and merging frequency- and time-domain data (Section 5).
2. Experimental
2.1. EIS
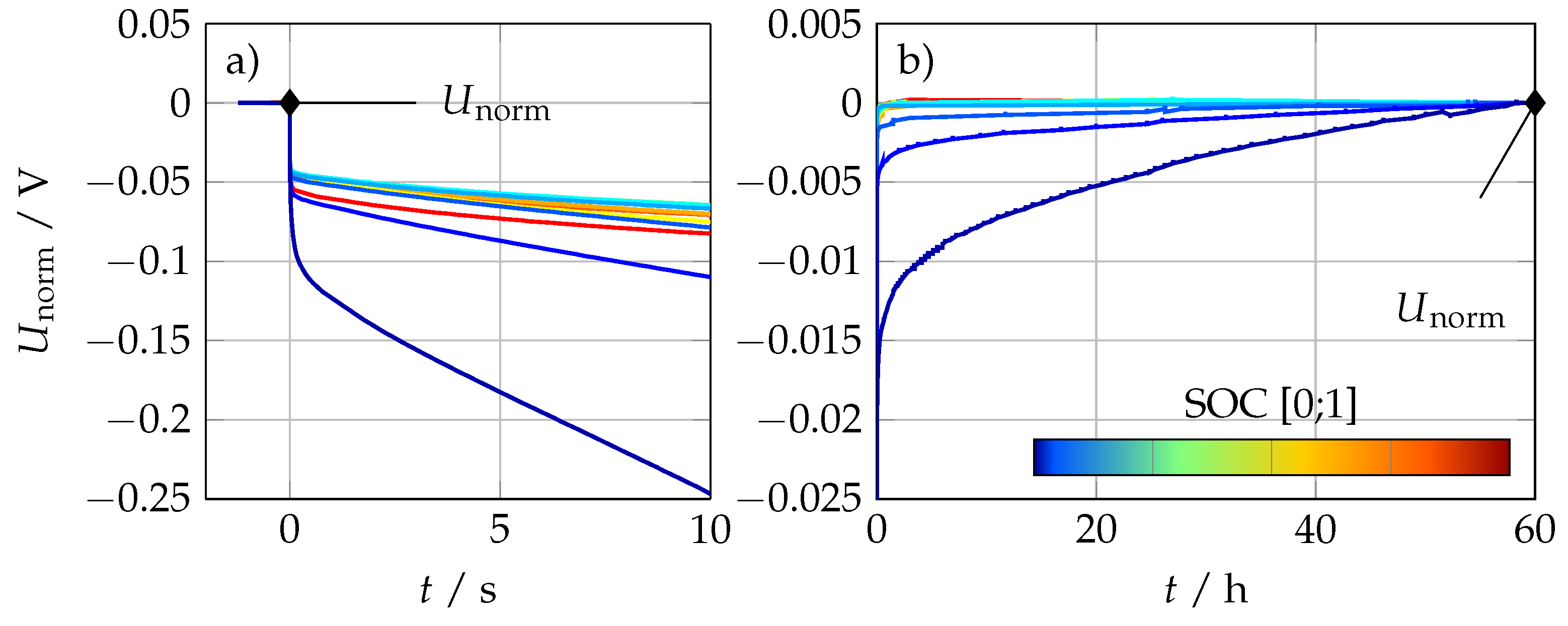
2.2. Time-Domain Measurements (TDMs)
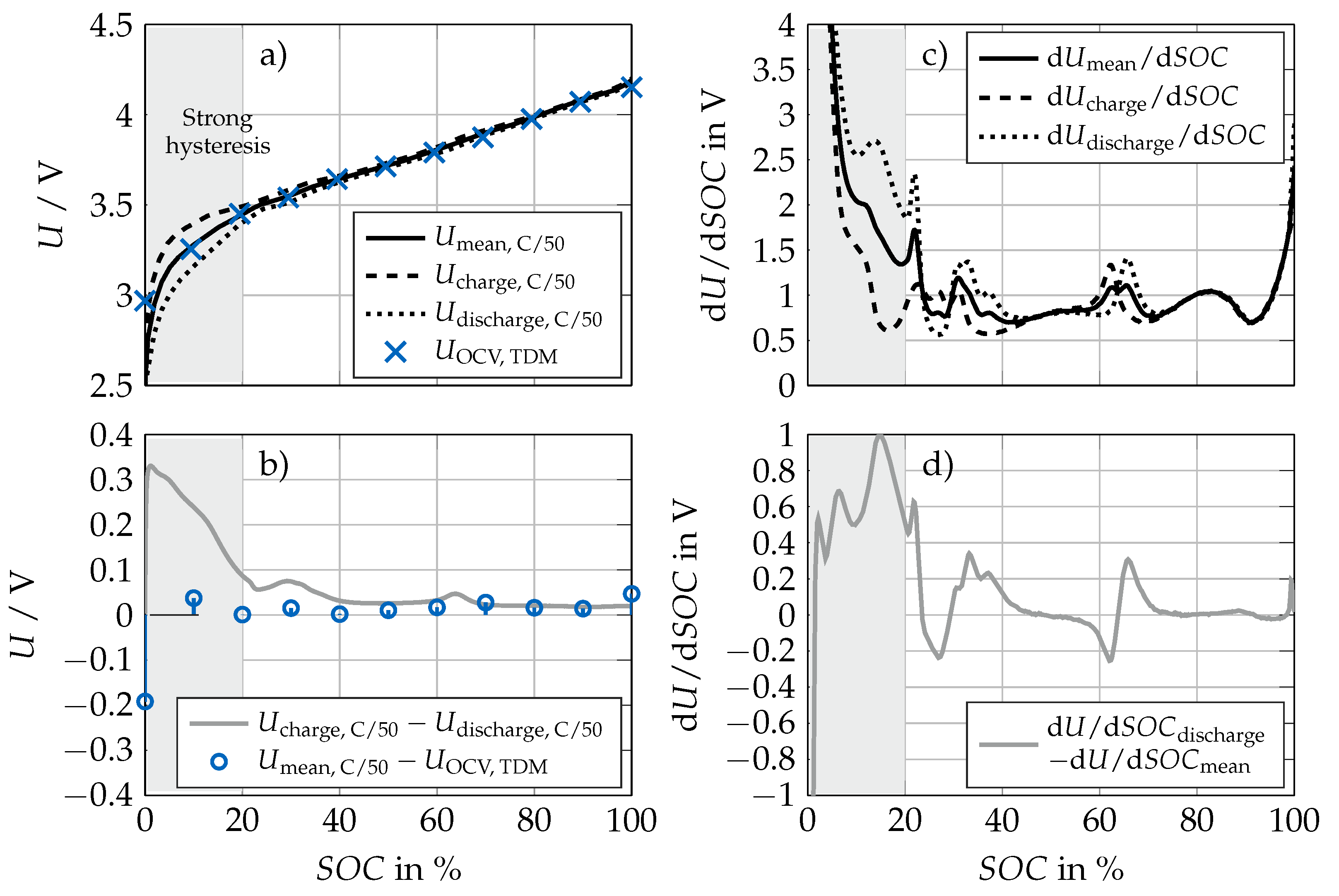
2.3. Open-Circuit Voltage
3. Calculation of the DRT
3.1. DRT Calculated from EIS (EIS-DRT)
3.2. DRT Calculated from Time-Domain Measurements (TDM-DRT)
3.3. Tikhonov Regularization
- The standard form of the Tikhonov regularization is the regularization with a squared norm of the solution itself. Hence, it penalizes high magnitudes of the solution and therefore favors solutions with smaller peaks. has to be set to be the identity matrix such that [29].
- The regularization with the squared norm of the solution’s first derivative penalizes high gradients in the solution and therefore favors solutions with moderate slopes. For this, has to be chosen such that , as shown in Appendix C [27,29].
- The regularization with the squared norm of the solution’s second derivative penalizes high curvatures in the solution and therefore favors flat and smooth solutions. For this, has to be chosen such that , as shown in Appendix C [20,21,25,29].
3.4. L-Curve Method for Optimized Determination of the Regularization Factor
3.5. Defining the Vector of Relaxation Times
4. ECM Parameterization
4.1. Employed ECM
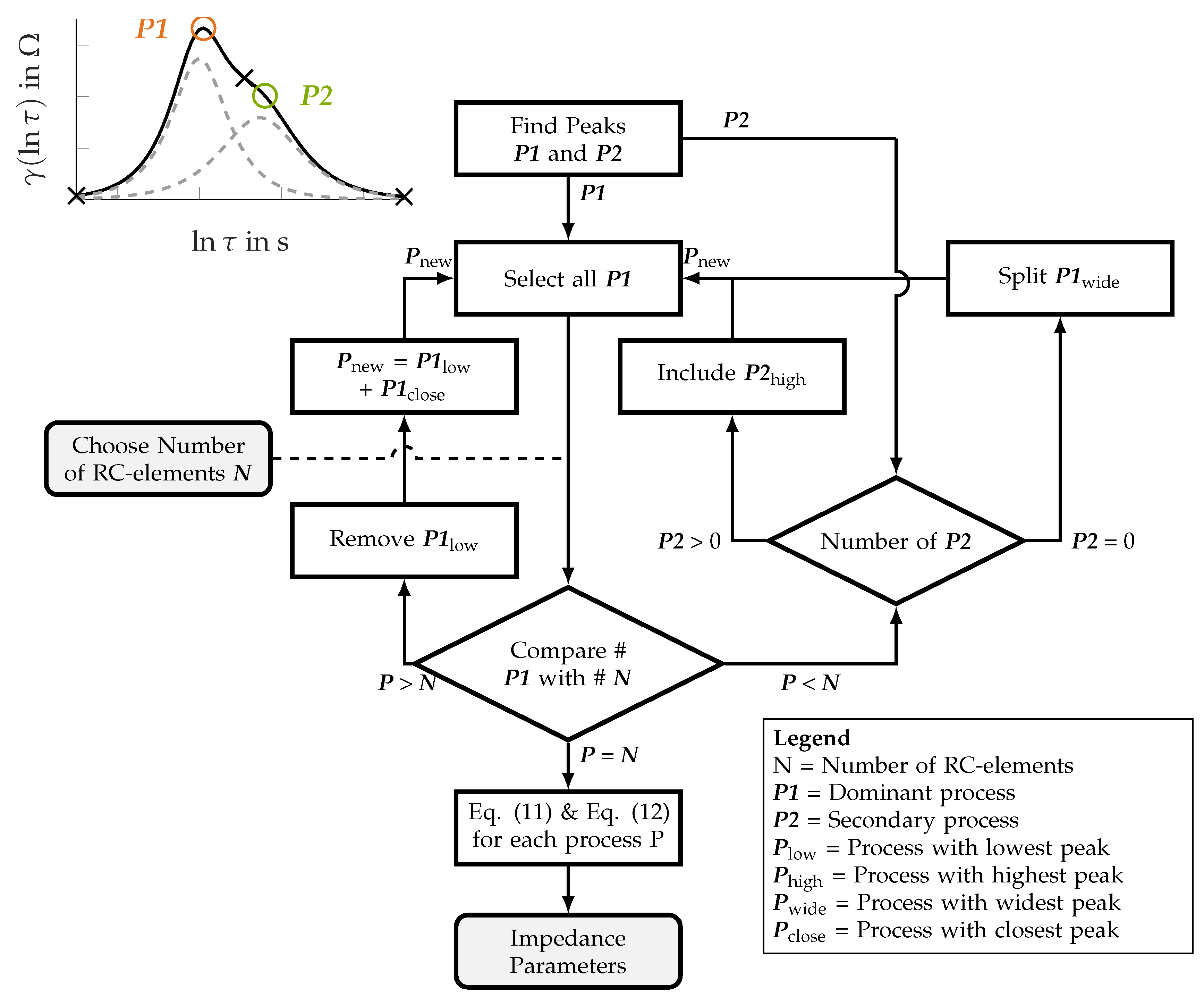
4.2. DRT-Fitting: Direct RC Parameterization from the DRT
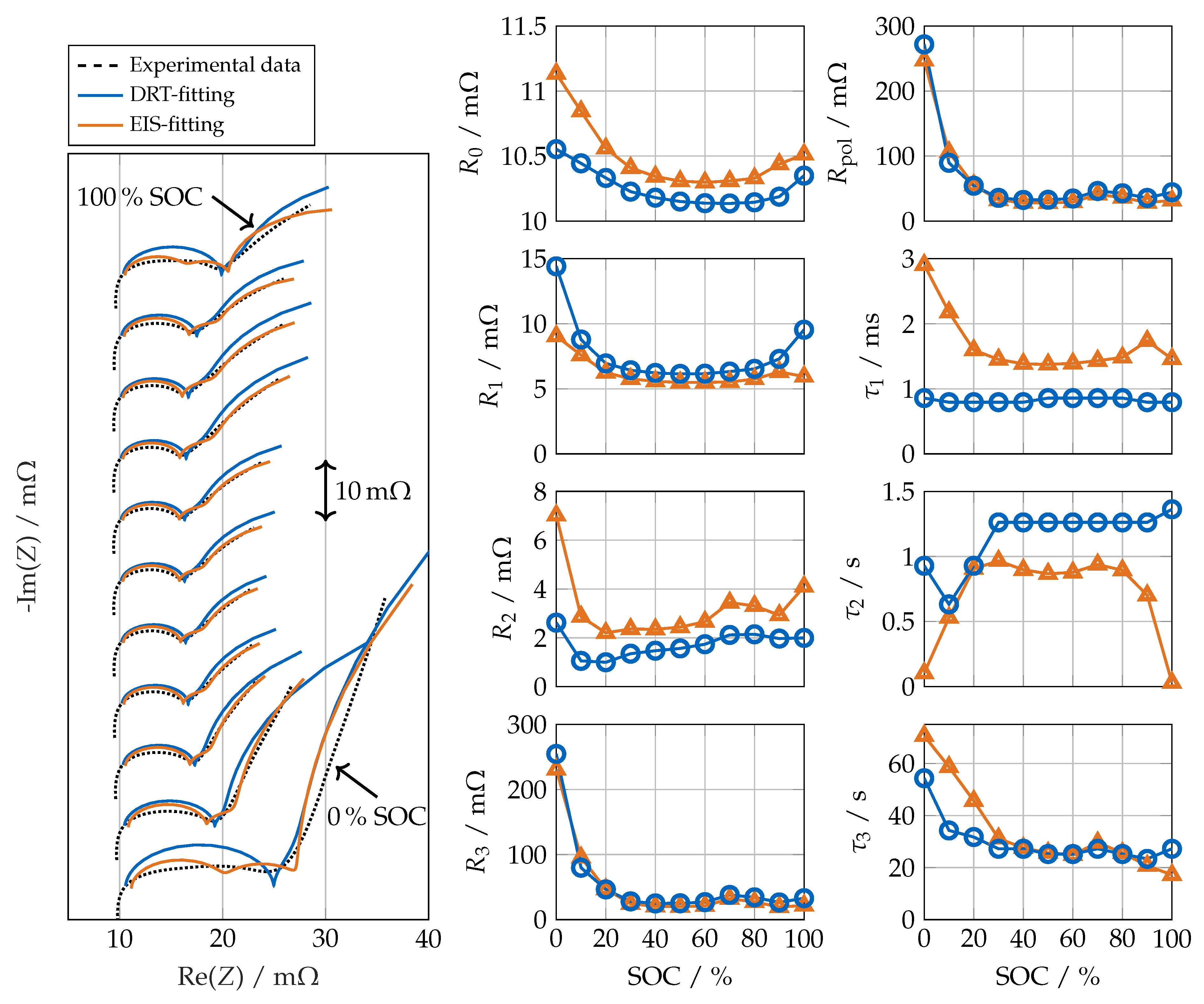
4.3. Comparison to Conventional EIS-Fitting
5. Combination of EIS and TDM
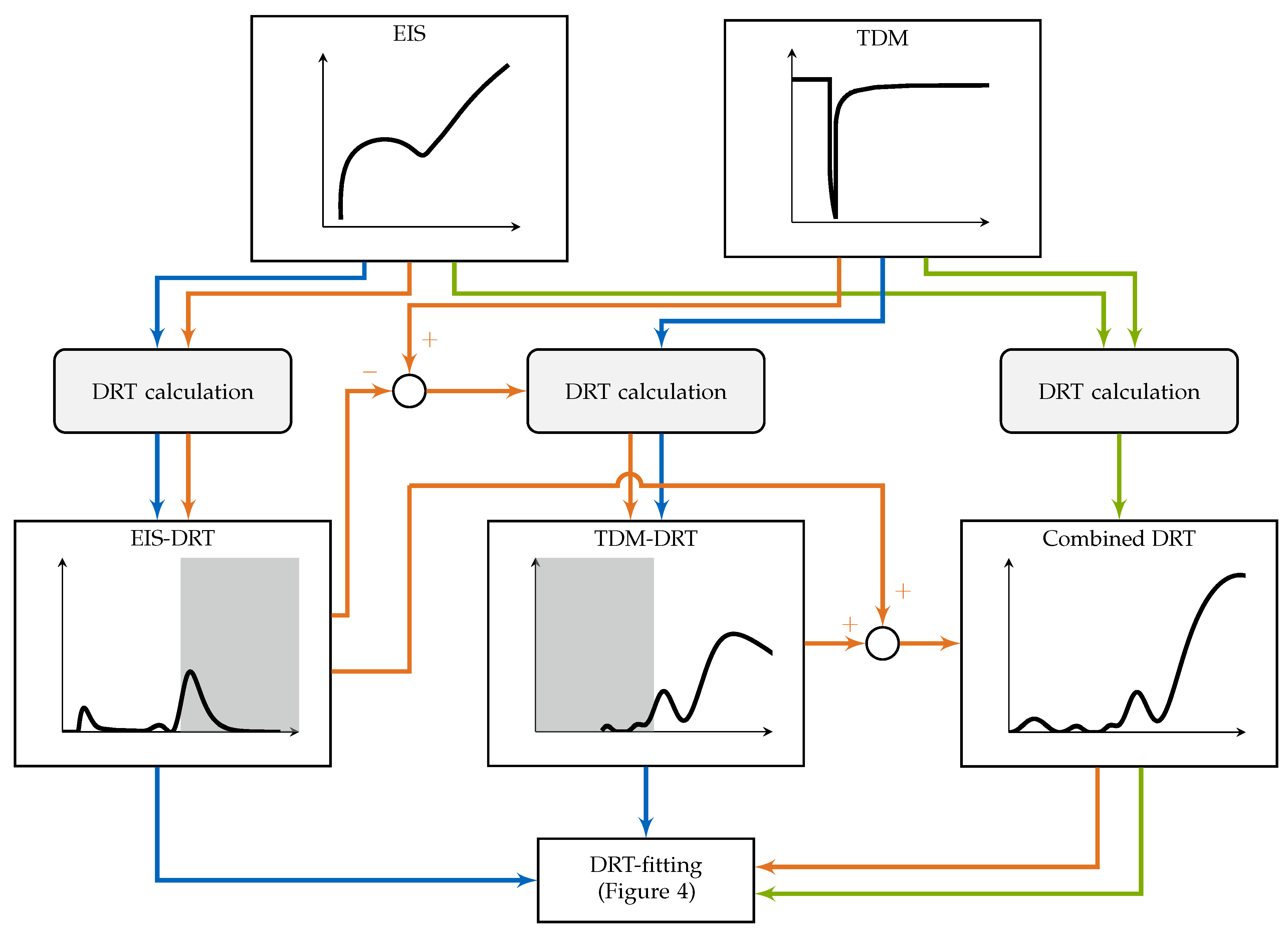
5.1. Merging Approaches
- 1.
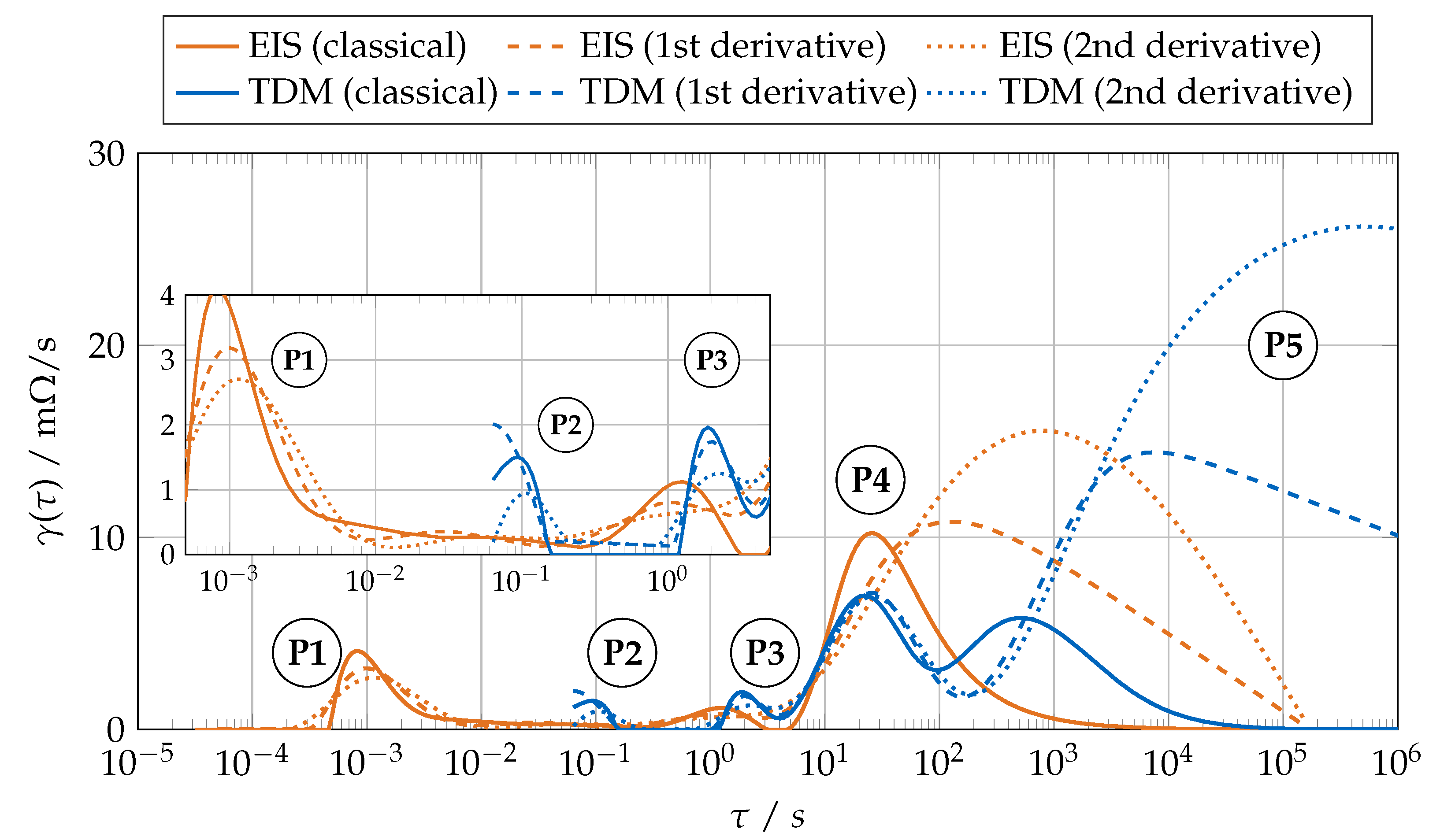
- Separate DRT-fitting (blue):In our first approach, EIS and TDM are used individually to parameterize different RC elements, the allocation of which is defined a priori. With this assumption, EIS and TDM are processed independent from each other. The ohmic resistance and the first RC elements (two in our case) are parameterized purely based on the EIS-DRT, whereas the remaining RC elements (two in our case) are determined from TDM-DRT only. Therefore, relaxation times higher than , with being the highest relaxation time with a local minimum in the EIS-DRT, are ignored in the EIS-DRT. Likewise, the TDM-DRT is only evaluated at . Depending on the sampling frequency, it is difficult to capture high-frequent loss processes during TDM, where meaningless oscillations can occur at low relaxation times close to of the TDM-DRT. This motivates an interconnected approach.
- 2.
- Interconnected DRT-fitting (orange):The goal of our second approach is to use information of EIS measurements in a more intertwined calculation of the TDM-DRT to avoid the described problems of meaningless oscillations at low relaxation times. Since the DRT equals a series of an infinite number of RC elements, the voltage relaxation which is caused by the high-frequency processes of the cell can be calculated from the EIS-DRT:This virtual voltage response is subtracted from the measured voltage of TDM. Since the EIS-DRT is only used to subtract the high-frequency behavior of the cell, the diffusive branch is removed from the impedance spectrum before evaluating Equation (13). Afterwards, the remaining voltage response is used to calculate the TDM-DRT according to Equation (7). Adding EIS-DRT and TDM-DRT finally yields a DRT of the complete system, which is used for the parameterization of all three RC elements. A disadvantage of this rather complex approach is that errors within calculation of the EIS-DRT propagate into the next step and will influence the voltage signal and thus also the TDM-DRT.
- 3.
- Combined DRT-fitting (green):Based on the disadvantages of the first two approaches, we developed a combined DRT-fitting, where the calculation of EIS-DRT and TDM-DRT is performed simultaneously in one single step. This makes decision of a border time constant superfluous and avoids complex interconnected fitting. Defining one system of equations in order to find , such that it best fits both measurements, directly results in a DRT that displays the complete dynamic behavior of the measured cell from high to low frequencies. This can be implemented by merging Equations (3) and (7) toThis equals a summation of the residuals of EIS-DRT and TDM-DRT. The diagonal Matrix applies a weighting between EIS and TDM data to achieve an equal influence of both measurements, the derivation of which can be found in Appendix D. Minimizing Equation (15) yields the DRT of the whole system as well as and and can thus be used to parameterize all ECM parameters at once.
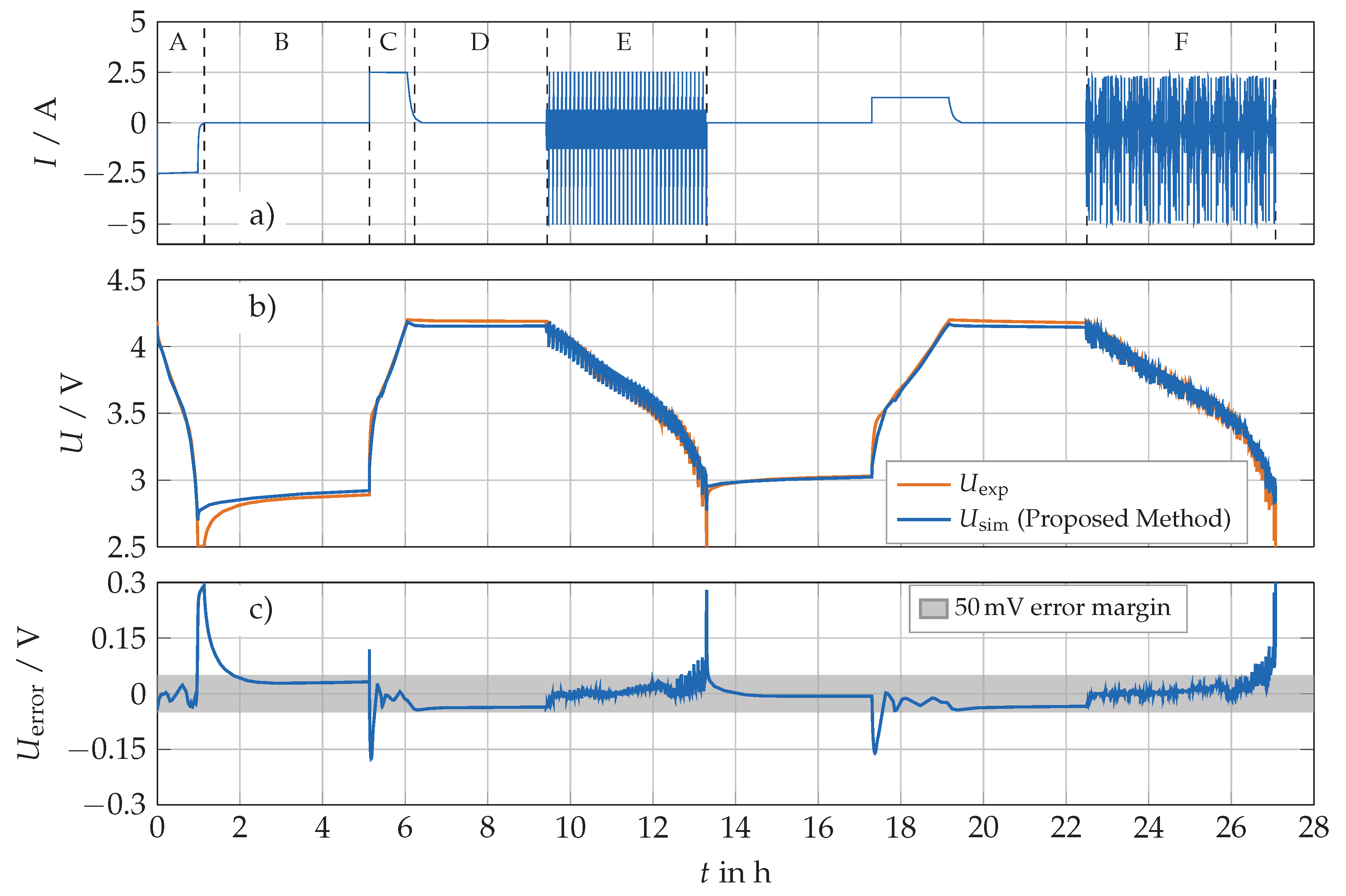
5.2. Validation Results
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BMS | battery management system |
| DRT | distribution of relaxation times |
| DST | dynamic stress test |
| DVA | differential voltage analysis |
| ECM | equivalent circuit model |
| EIS | electrochemical impedance spectoscropy |
| GITT | galvanostatic intermittent titration technique |
| LIB | lithium-ion battery |
| NCA | nickel cobalt aluminium oxide |
| OCV | open-circuit voltage |
| pOCV | pseudo open-circuit voltage |
| RMSE | root mean square error |
| SOAP | state of available power |
| SOC | state of charge |
| SOH | state of health |
| TDM | time-domain measurement |
Appendix A. EIS-DRT
Appendix B. TDM-DRT
Appendix C. Regularization Terms
Appendix D. Weighting of EIS and TDM Data
References
- Wassiliadis, N.; Adermann, J.; Frericks, A.; Pak, M.; Reiter, C.; Lohmann, B.; Lienkamp, M. Revisiting the dual extended Kalman filter for battery state-of-charge and state-of-health estimation: A use-case life cycle analysis. J. Energy Storage 2018, 19, 73–87. [Google Scholar] [CrossRef]
- Birkl, C.R.; Roberts, M.R.; McTurk, E.; Bruce, P.G.; Howey, D.A. Degradation diagnostics for lithium ion cells. J. Power Sources 2017, 341, 373–386. [Google Scholar] [CrossRef]
- Schmitt, J.; Maheshwari, A.; Heck, M.; Lux, S.; Vetter, M. Impedance change and capacity fade of lithium nickel manganese cobalt oxide-based batteries during calendar aging. J. Power Sources 2017, 353, 183–194. [Google Scholar] [CrossRef]
- Zhou, X.; Huang, J.; Pan, Z.; Ouyang, M. Impedance characterization of lithium-ion batteries aging under high-temperature cycling: Importance of electrolyte-phase diffusion. J. Power Sources 2019, 426, 216–222. [Google Scholar] [CrossRef]
- Bruch, M.; Millet, L.; Kowal, J.; Vetter, M. Novel method for the parameterization of a reliable equivalent circuit model for the precise simulation of a battery cell’s electric behavior. J. Power Sources 2021, 490. [Google Scholar] [CrossRef]
- Karger, A.; Wildfeuer, L.; Maheshwari, A.; Wassiliadis, N.; Lienkamp, M. Novel method for the on-line estimation of low-frequency impedance of lithium-ion batteries. J. Energy Storage 2020, 32, 101818. [Google Scholar] [CrossRef]
- Gantenbein, S.; Weiss, M.; Ivers-Tiffée, E. Impedance based time-domain modeling of lithium-ion batteries: Part I. J. Power Sources 2018, 379, 317–327. [Google Scholar] [CrossRef]
- Ciucci, F. Modeling electrochemical impedance spectroscopy. Curr. Opin. Electrochem. 2019, 13, 132–139. [Google Scholar] [CrossRef]
- Waag, W.; Fleischer, C.; Sauer, D.U. On-line estimation of lithium-ion battery impedance parameters using a novel varied-parameters approach. J. Power Sources 2013, 237, 260–269. [Google Scholar] [CrossRef]
- Schmidt, R. Impedance Spectroscopy of Electroceramics. In Ceramic Materials Research Trends; Lin, P.B., Aguiar, R., Eds.; Nova Science Publ.: New York, NY, USA, 2007. [Google Scholar]
- Schönleber, M.; Klotz, D.; Ivers-Tiffée, E. A Method for Improving the Robustness of linear Kramers-Kronig Validity Tests. Electrochim. Acta 2014, 131, 20–27. [Google Scholar] [CrossRef]
- Witzenhausen, H. Elektrische Batteriespeichermodelle: Modellbildung, Parameteridentifikation und Modellreduktion. Ph.D. Thesis, Rheinisch-Westfälischen Technischen Hochschule Aachen, Aachen, Germany, 2017. [Google Scholar]
- Illig, J.; Ender, M.; Chrobak, T.; Schmidt, J.P.; Klotz, D.; Ivers-Tiffée, E. Separation of Charge Transfer and Contact Resistance in LiFePO4-Cathodes by Impedance Modeling. J. Electrochem. Soc. 2012, 159, A952–A960. [Google Scholar] [CrossRef]
- Schmidt, J.P.; Berg, P.; Schönleber, M.; Weber, A.; Ivers-Tiffée, E. The distribution of relaxation times as basis for generalized time-domain models for Li-ion batteries. J. Power Sources 2013, 221, 70–77. [Google Scholar] [CrossRef]
- Sabet, P.S.; Stahl, G.; Sauer, D.U. Non-invasive investigation of predominant processes in the impedance spectra of high energy lithium-ion batteries with Nickel-Cobalt-Aluminum cathodes. J. Power Sources 2018, 406, 185–193. [Google Scholar] [CrossRef]
- Schichlein, H.; Müller, A.C.; Voigts, M.; Krügel, A.; Ivers-Tiffée, E. Deconvolution of electrochemical impedance spectra for the identification of electrode reaction mechanisms in solid oxide fuel cells. J. Appl. Electrochem. 2002, 32, 875–882. [Google Scholar] [CrossRef]
- Hershkovitz, S.; Baltianski, S.; Tsur, Y. Harnessing evolutionary programming for impedance spectroscopy analysis: A case study of mixed ionic-electronic conductors. Solid State Ionics 2011, 188, 104–109. [Google Scholar] [CrossRef]
- Goldammer, E.; Kowal, J. Determination of the Distribution of Relaxation Times by Means of Pulse Evaluation for Offline and Online Diagnosis of Lithium-Ion Batteries. Batteries 2021, 7, 36. [Google Scholar] [CrossRef]
- Lain, M.J.; Brandon, J.; Kendrick, E. Design strategies for high power vs. High energy lithium ion cells. Batteries 2019, 5, 64. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, J.P. Verfahren zur Charakterisierung und Modellierung von Lithium-Ionen Zellen; Karlsruher Institut für Technologie: Karlsruhe, Germany, 2013. [Google Scholar]
- Schmidt, J.P.; Ivers-Tiffée, E. Pulse-fitting—A novel method for the evaluation of pulse measurements, demonstrated for the low frequency behavior of lithium-ion cells. J. Power Sources 2016, 315, 316–323. [Google Scholar] [CrossRef]
- Sturm, J.; Rheinfeld, A.; Zilberman, I.; Spingler, F.; Kosch, S.; Frie, F.; Jossen, A. Modeling and simulation of inhomogeneities in a 18650 nickel-rich, silicon-graphite lithium-ion cell during fast charging. J. Power Sources 2019, 412, 204–223. [Google Scholar] [CrossRef] [Green Version]
- Klotz, D. Characterization and Modeling of Electrochemical Energy Conversion Systems by Impedance Techniques; KIT Scientific Publishing: Karlsruhe, Germany, 2012. [Google Scholar]
- Schichlein, H. Experimentelle Modellbildung für die Hochtemperatur-Brennstoffzelle SOFC; Universität Karlsruhe (TH): Aachen, Germany, 2003. [Google Scholar]
- Wan, T.H.; Saccoccio, M.; Chen, C.; Ciucci, F. Influence of the Discretization Methods on the Distribution of Relaxation Times Deconvolution: Implementing Radial Basis Functions with DRTtools. Electrochim. Acta 2015, 184, 483–499. [Google Scholar] [CrossRef]
- Boukamp, B.A. A Linear Kronig-Kramers Transform Test for Immittance Data Validation. J. Electrochem. Soc. 1995, 142, 1885. [Google Scholar] [CrossRef]
- Saccoccio, M.; Wan, T.H.; Chen, C.; Ciucci, F. Optimal Regularization in Distribution of Relaxation Times applied to Electrochemical Impedance Spectroscopy: Ridge and Lasso Regression Methods—A Theoretical and Experimental Study. Electrochim. Acta 2014, 147, 470–482. [Google Scholar] [CrossRef]
- Tikhonov, A.N.; Goncharsky, A.V.; Hazewinkel, M. Numerical Methods for the Solution of Ill-Posed Problems; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Hahn, M.; Schindler, S.; Triebs, L.C.; Danzer, M.A. Optimized Process Parameters for a Reproducible Distribution of Relaxation Times Analysis of Electrochemical Systems. Batteries 2019, 5, 43. [Google Scholar] [CrossRef] [Green Version]
- Hansen, P.C. Analysis of Discrete Ill-Posed Problems by Means of the L-Curve. SIAM Rev. 1992, 34, 561–580. [Google Scholar] [CrossRef]
- Hansen, P.C. The L-Curve and Its Use in the Numerical Treatment of Inverse Problems. Available online: https://orbit.dtu.dk/en/publications/the-l-curve-and-its-use-in-the-numerical-treatment-of-inverse-pro-2 (accessed on 18 May 2021).
- Choi, M.B.; Shin, J.; Ji, H.I.; Kim, H.; Son, J.W.; Lee, J.H.; Kim, B.K.; Lee, H.W.; Yoon, K.J. Interpretation of Impedance Spectra of Solid Oxide Fuel Cells: L-Curve Criterion for Determination of Regularization Parameter in Distribution Function of Relaxation Times Technique. JOM 2019, 71, 3825–3834. [Google Scholar] [CrossRef]
- Press, W.H. Numerical Recipes in C: The Art of Scientific Computing, 2nd ed.; Reprinted with Corr. to Software Version 2.10; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- The MathWorks Inc. MATLAB MultiStart. 2021. Available online: https://de.mathworks.com/help/gads/multistart.html (accessed on 5 May 2021).
- Reiter, C.; Wassiliadis, N.; Wildfeuer, L.; Wurster, T.; Lienkamp, M. Range Extension of Electric Vehicles through Improved Battery Capacity Utilization: Potentials, Risks and Strategies. In Proceedings of the 2018 21st International Conference on Intelligent Transportation Systems (ITSC), Maui, HI, USA, 4–7 November 2018; pp. 321–326. [Google Scholar] [CrossRef]
- Sonn, V.; Leonide, A.; Ivers-Tiffée, E. Combined deconvolution and CNLS fitting approach applied on the impedance response of technical Ni/ 8YSZ cermet electrodes. J. Electrochem. Soc. 2008, 155, B675–B679. [Google Scholar] [CrossRef]
- Franklin, A.D.; de Bruin, H.J. The fourier analysis of impedance spectra for electroded solid electrolytes. Phys. Status Solidi (a) 1983, 75, 647–656. [Google Scholar] [CrossRef]
- Hust, F.; Witzenhausen, H.; Sauer, D.U. Distribution of relaxation times for lithium-ion batteries. In Proceedings of the 9th International Symposium on Electrochemical Impedance Spectroscopy, Okinawa, Japan, 17–20 June 2013. [Google Scholar]
- Tesler, A.B.; Lewin, D.R.; Baltianski, S.; Tsur, Y. Analyzing results of impedance spectroscopy using novel evolutionary programming techniques. J. Electroceram. 2010, 24, 245–260. [Google Scholar] [CrossRef]
- Tuncer, E.; Macdonald, J.R. Comparison of methods for estimating continuous distributions of relaxation times. J. Appl. Phys. 2006, 99, 074106. [Google Scholar] [CrossRef] [Green Version]
| EIS-Data | TDM-Data | |||
|---|---|---|---|---|
| EIS-Fitting | DRT-Fitting | DRT-Fitting | |||
|---|---|---|---|---|---|
| Measurement | EIS | EIS | EIS and TDM | ||
| Merging Approach | n.a. | n.a. | Separate | Interconnected | Combined |
| Regularization Term | n.a. | x | x/ | x/ | |
| Whole Validation Cycle | 72.56 | 73.14 | 45.18 | 41.52 | 43.38 |
| A: 1 C Discharge | 42.88 | 46.76 | 28.78 | 26.41 | 26.71 |
| B: Relaxation at 0% SOC | 148.12 | 148.17 | 38.86 | 52.44 | 56.87 |
| C: 1 C Charge | 49.61 | 56.85 | 95.08 | 60.87 | 59.63 |
| D: Relaxation at 100% SOC | 37.35 | 37.35 | 36.33 | 36.47 | 36.74 |
| E: DST | 24.14 | 25.63 | 22.65 | 20.59 | 22.16 |
| F: Driving Profile | 31.56 | 32.05 | 23.69 | 25.12 | 27.39 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wildfeuer, L.; Gieler, P.; Karger, A. Combining the Distribution of Relaxation Times from EIS and Time-Domain Data for Parameterizing Equivalent Circuit Models of Lithium-Ion Batteries. Batteries 2021, 7, 52. https://doi.org/10.3390/batteries7030052
Wildfeuer L, Gieler P, Karger A. Combining the Distribution of Relaxation Times from EIS and Time-Domain Data for Parameterizing Equivalent Circuit Models of Lithium-Ion Batteries. Batteries. 2021; 7(3):52. https://doi.org/10.3390/batteries7030052
Chicago/Turabian StyleWildfeuer, Leo, Philipp Gieler, and Alexander Karger. 2021. "Combining the Distribution of Relaxation Times from EIS and Time-Domain Data for Parameterizing Equivalent Circuit Models of Lithium-Ion Batteries" Batteries 7, no. 3: 52. https://doi.org/10.3390/batteries7030052
APA StyleWildfeuer, L., Gieler, P., & Karger, A. (2021). Combining the Distribution of Relaxation Times from EIS and Time-Domain Data for Parameterizing Equivalent Circuit Models of Lithium-Ion Batteries. Batteries, 7(3), 52. https://doi.org/10.3390/batteries7030052






