The Investigation of Spin-Crossover Systems by Raman Spectroscopy: A Review
Abstract
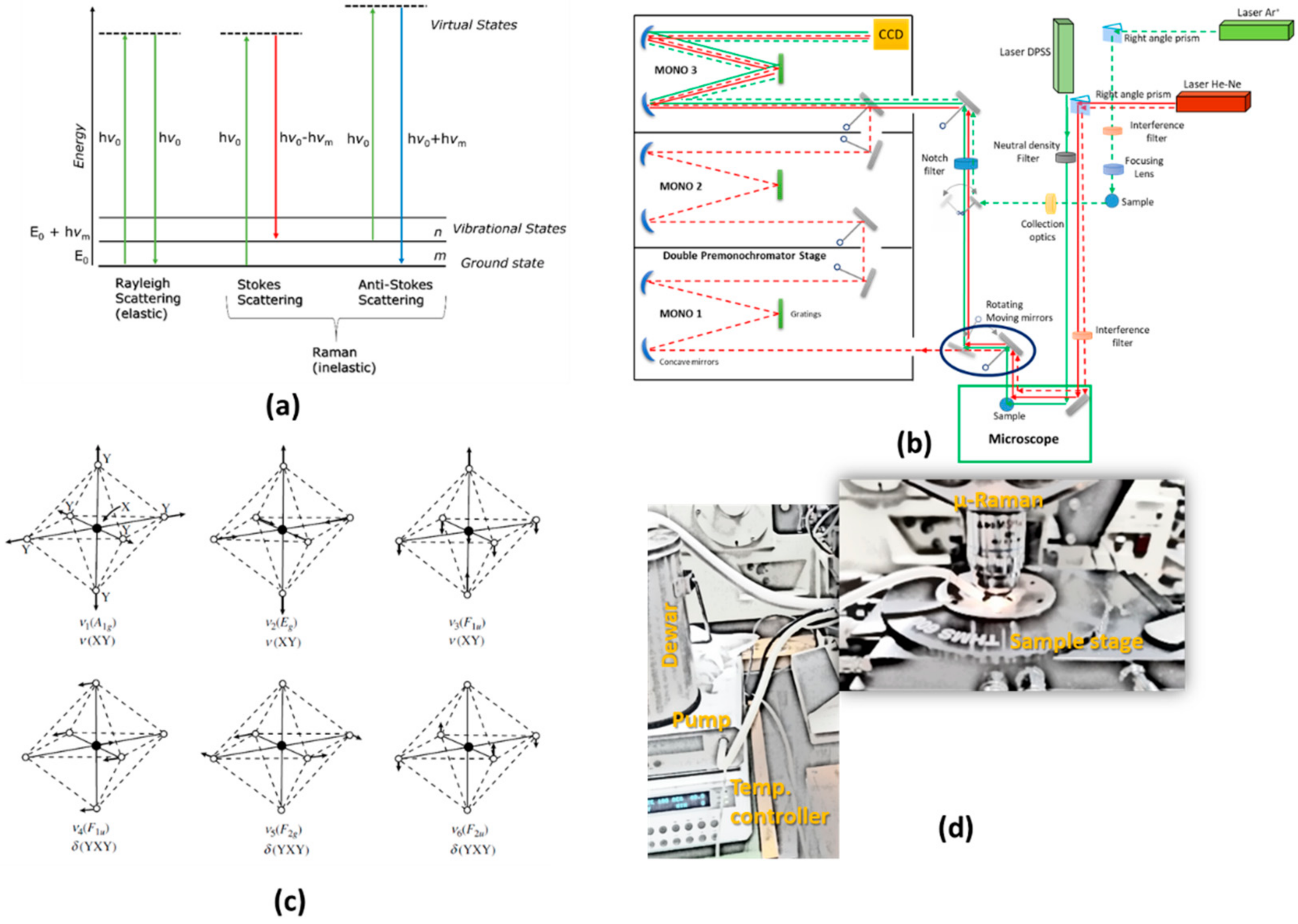
1. Introduction to Raman Spectroscopy: The Impact on Inorganic and Coordination Chemistry
2. The Influence on Molecular Vibrations
3. Conventional Methods for Exploring SCO: The Prevalence of Magnetic Susceptibility Measurements
4. The Investigation of SCO through Raman Spectroscopy in Selected Examples
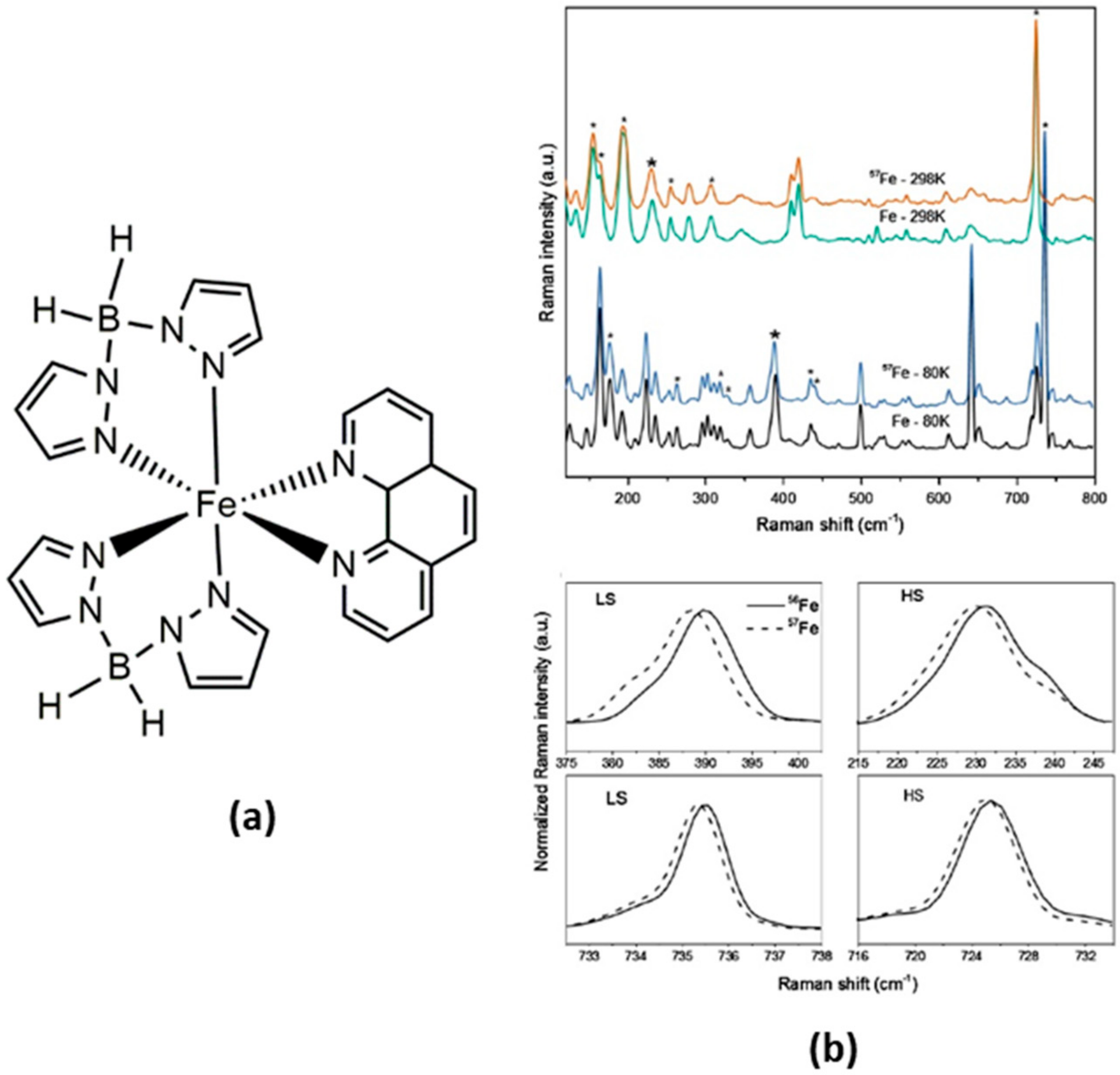
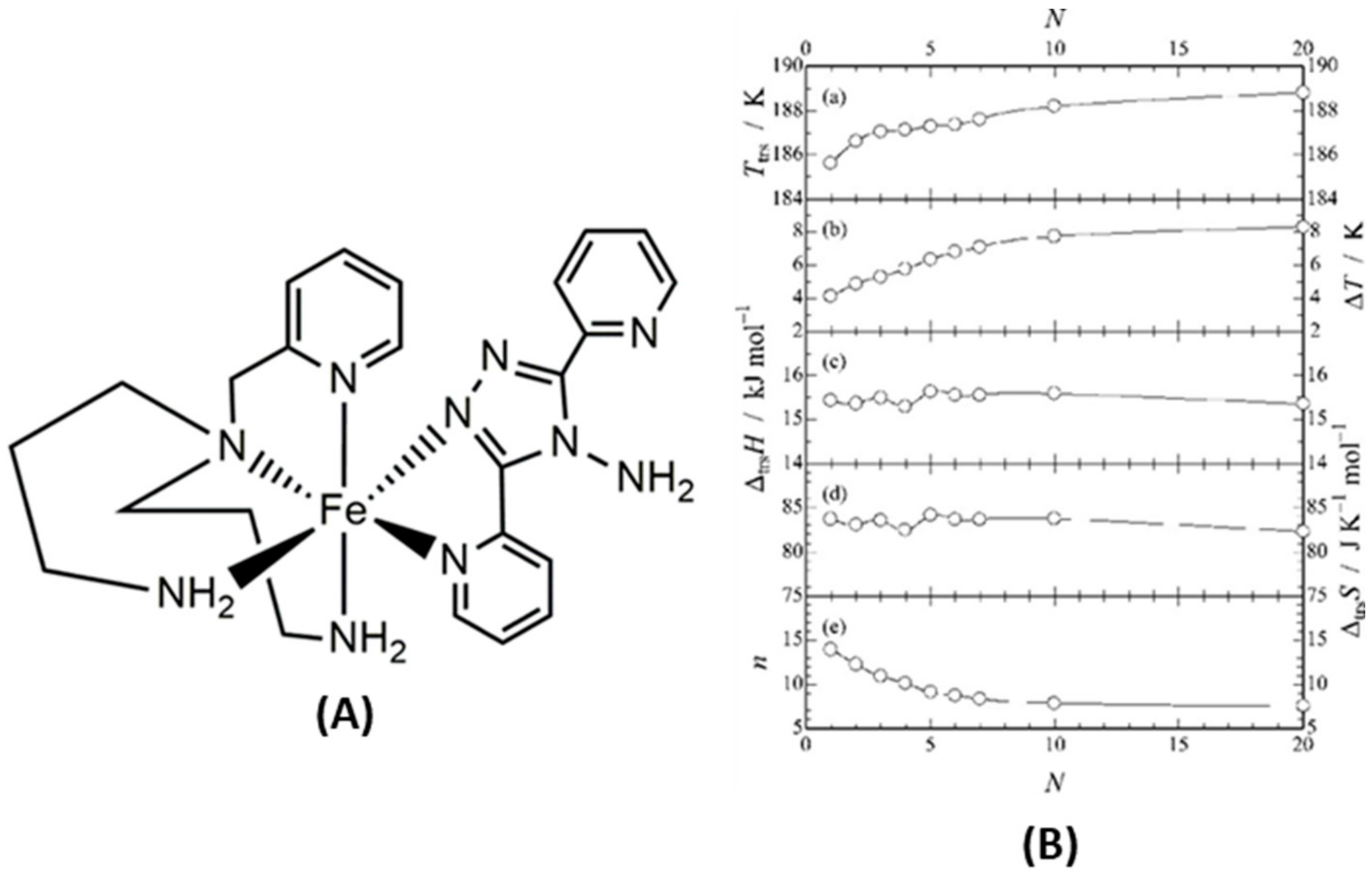
4.1. Fe(II) and Other 3d Mononuclear and Polynuclear Complexes
4.2. 3d/3d’ Metal Organic Frameworks (MOFs)
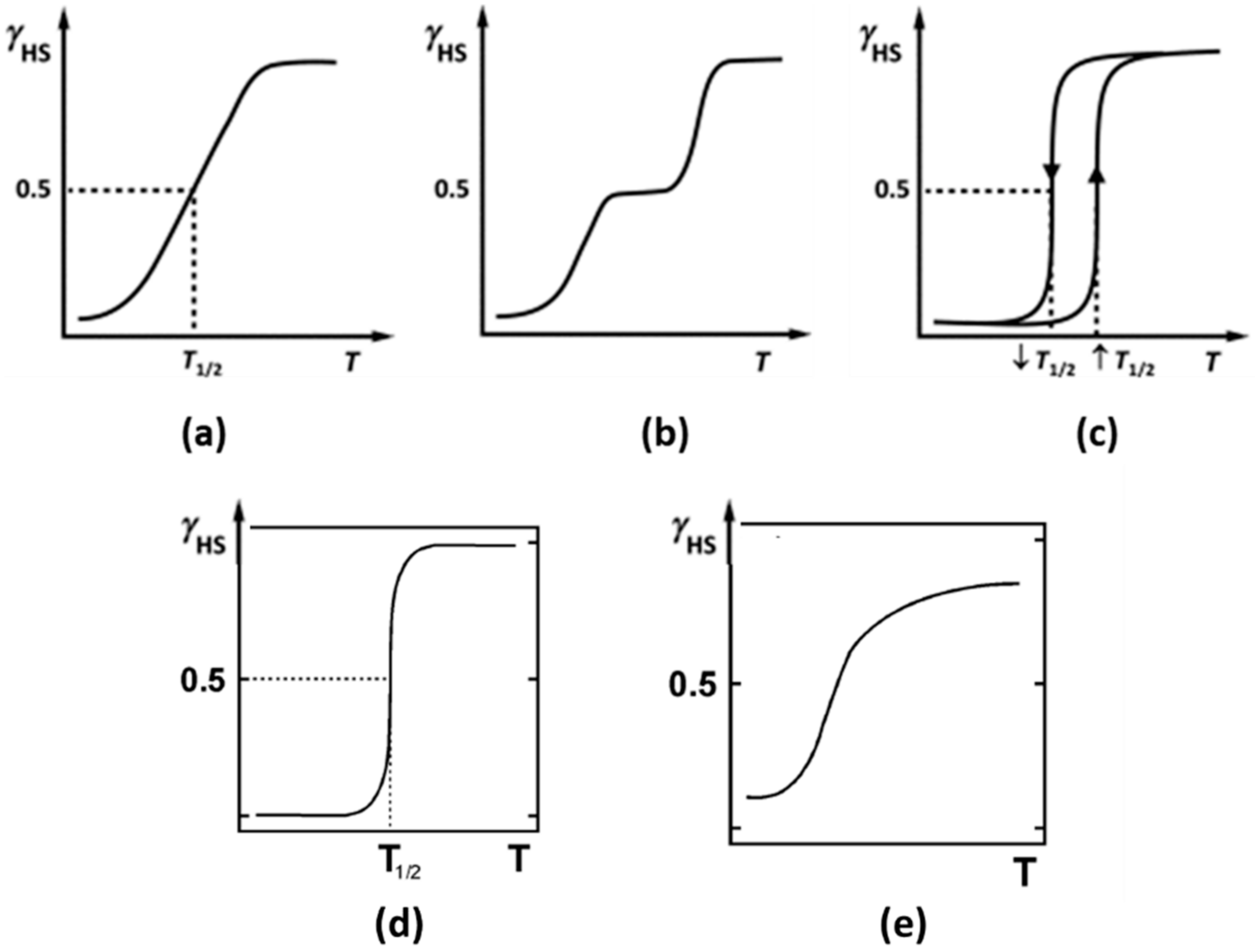
5. In Situ Temperature-Dependent Raman Measurements for Recording the SCO Curve and Calculation of the HS Population
6. Toward the Practical Application of SCO Complexes: Selected Studies in Which Raman Is Involved, Current Trends, Challenges, and Future Perspectives
| Application | Main Features | Raman | Refs. |
|---|---|---|---|
| Memory Devices | Hybrid thin films composed of spin-crossover NPs and CNTs for electrical memory devices | NO | [112] |
| Sensors | Spin-crossover MOFs as gas sensors; suitable reversibility, room-temperature operation, a low limit of detection and linear dynamic range of detection, selectivity | YES | [93] |
| Synergy between magnetic and color properties in spin-crossover material for usage as temperature sensor | NO | [113] | |
| Spin-crossover material acting simultaneously as pressure and temperature sensor | NO | [104] | |
| Incorporation of Spin-Crossover complexes into polymers for tuning of the SCO process and toward their applicability | YES | [28,98,99] | |
| Size and morphology effect of SCO complexes on SCO behavior | YES | [24,96] | |
| MRI Agents | Water-soluble SCO iron(II) NPs with a polyethylene glycol (PEG) coating exhibiting thermally responsive T1/2 values making them appropriate candidates for use as a MRI contrast agent | NO | [114] |
| Silica hybrid, spin-crossover water-soluble nanoparticles as potential candidates for thermally responsive MRI agents | NO | [115] | |
| Multifunctional Materials | Integration of SCO nanoparticles with silver nanowires toward the development of magnetic and conductive bifunctional materials | YES | [111] |
| SCO-based magneto-optical switching materials | YES | [109] | |
| A coordination network comprising of spin-crossover and single-molecule magnet units inducing thermally and photoreversible magnetic and optical properties | NO | [106] | |
| Physical gels exhibiting optical and magnetic properties originating from SCO precursors (photofunctional and biocompatible organogels) | NO | [105] |
Funding
Conflicts of Interest
References
- Orlando, A.; Franceschini, F.; Muscas, C.; Pidkova, S.; Bartoli, M.; Rovere, M.; Tagliaferro, A. A Comprehensive Review on Raman Spectroscopy Applications. Chemosensors 2021, 9, 262. [Google Scholar] [CrossRef]
- Raman, C.V. A new radiation. Proc. Indian Acad. Sci. Sect. A 1953, 37, 333–341. [Google Scholar] [CrossRef]
- Landsberg, G.S. Eine neue Erscheinung bei der Lichtzerstreuung in Krystallen. Naturwissenschaften 1928, 16, 557–558. [Google Scholar]
- Hendra, P.J.; Stratton, P.M. Laser-Raman spectroscopy. Chem. Rev. 1969, 69, 325–344. [Google Scholar] [CrossRef]
- Smith, E.; Dent, G. Introduction, Basic Theory and Principles. In Modern Raman Spectroscopy—A Practical Approach; John Wiley & Sons Ltd.: Chichester, UK, 2005; pp. 1–21. [Google Scholar]
- Thapliyal, V.; Alabdulkarim, M.E.; Whelan, D.R.; Mainali, B.; Maxwell, J.L. A concise review of the Raman spectra of carbon allotropes. Diam. Relat. Mater. 2022, 127, 109180. [Google Scholar] [CrossRef]
- Jones, R.R.; Hooper, D.C.; Zhang, L.; Wolverson, D.; Valev, V.K. Raman Techniques: Fundamentals and Frontiers. Nanoscale Res. Lett. 2019, 14, 231. [Google Scholar] [CrossRef]
- Farber, C.; Li, J.; Hager, E.; Chemelewski, R.; Mullet, J.; Rogachev, A.Y.; Kurouski, D. Complementarity of Raman and Infrared Spectroscopy for Structural Characterization of Plant Epicuticular Waxes. ACS Omega 2019, 4, 3700–3707. [Google Scholar] [CrossRef]
- Auer, B.M.; Skinner, J.L. IR and Raman spectra of liquid water: Theory and interpretation. J. Chem. Phys. 2008, 128, 224511. [Google Scholar] [CrossRef]
- Voyiatzis, G.A.; Kalampounias, A.G.; Papatheodorou, G.N. The structure of molten mixtures of iron (III) chloride with caesium chloride. Phys. Chem. Chem. Phys. 1999, 1, 4797–4803. [Google Scholar] [CrossRef]
- Stammreich, H.; Sans, T.T. Hg-Hg stretching frequencies and bond lengths in mercurous compounds. J. Mol. Struct. 1967, 1, 55–60. [Google Scholar] [CrossRef]
- Tsantis, S.T.; Lada, Z.G.; Tzimopoulos, D.I.; Bekiari, V.; Psycharis, V.; Raptopoulou, C.P.; Perlepes, S.P. Two different coordination modes of the Schiff base derived from ortho-vanillin and 2-(2-aminomethyl)pyridine in a mononuclear uranyl complex. Heliyon 2022, 8, e09705. [Google Scholar] [CrossRef]
- Routzomani, A.; Lada, Z.G.; Angelidou, V.; Raptopoulou, C.P.; Psycharis, V.; Konidaris, K.F.; Chasapis, C.T.; Perlepes, S.P. Confirming the Molecular Basis of the Solvent Extraction of Cadmium (II) Using 2-Pyridyl Oximes through a Synthetic Inorganic Chemistry Approach and a Proposal for More Efficient Extractants. Molecules 2022, 27, 1619. [Google Scholar] [CrossRef]
- North, J.M.; van de Burgt, L.J.; Dalal, N.S. A Raman study of the single molecule magnet Mn12-acetate and analogs. Solid State Commun. 2002, 123, 75–79. [Google Scholar] [CrossRef]
- Browne, W.R. Chapter 8—Resonance Raman spectroscopy and its application in bioinorganic chemistry. In Practical Approaches to Biological Inorganic Chemistry, 2nd ed.; Crichton, R.R., Louro, R.O., Eds.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 275–324. [Google Scholar]
- Choo-Smith, L.P.; Edwards, H.G.M.; Endtz, H.P.; Kros, J.M.; Heule, F.; Barr, H.; Jr, J.S.R.; Bruining, H.A.; Puppels, G.J. Medical applications of Raman spectroscopy: From proof of principle to clinical implementation. Biopolymers 2002, 67, 1–9. [Google Scholar] [CrossRef]
- Baddiel, C.B.; Janz, G.J. Molten thiocyanates: Raman spectra and structure. Trans. Faraday Soc. 1964, 60, 2009–2012. [Google Scholar] [CrossRef]
- Tramer, A. Spectra and structure of thiocyanate complexes. In Theory and Structure of Complex Compounds; JeŻOwska-Trzebiatowska, B., Ed.; Pergamon Press, The Macmillan Company: New York, NY, USA, 1964; pp. 225–228. [Google Scholar]
- Kettle, S.F.A. Physical Inorganic Chemistry: A Coordination Chemistry Approach; Oxford University Press: Oxford, UK, 1998. [Google Scholar]
- Nakamoto, K. Infrared and Raman Spectra of Inorganic and Coordination Compounds Part A, Theory and Applications in Inorganic Chemistry; John Wiley & Sons: Hoboken, NJ, USA, 2009; p. 1. [Google Scholar]
- Drago, R.S. Physical Methods in Inorganic Chemistry; Reinhold Pub. Corp.: New York, NY, USA, 1965. [Google Scholar]
- Suffren, Y.; Rollet, F.-G.; Reber, C. Raman spectroscopy of transition metal complexes: Molecular vibrational frequencies, phase transitions, isomers, and electronic structure. Comments Inorg. Chem. 2011, 32, 246–276. [Google Scholar] [CrossRef]
- Hadjiivanov, K.I.; Panayotov, D.A.; Mihaylov, M.Y.; Ivanova, E.Z.; Chakarova, K.K.; Andonova, S.M.; Drenchev, N.L. Power of Infrared and Raman Spectroscopies to Characterize Metal-Organic Frameworks and Investigate Their Interaction with Guest Molecules. Chem. Rev. 2021, 121, 1286–1424. [Google Scholar] [CrossRef]
- Lada, Z.G.; Andrikopoulos, K.S.; Chrissanthopoulos, A.; Perlepes, S.P.; Voyiatzis, G.A. A Known Iron (II) Complex in Different Nanosized Particles: Variable-Temperature Raman Study of Its Spin-Crossover Behavior. Inorg. Chem. 2019, 58, 5183–5195. [Google Scholar] [CrossRef]
- Lada, Z.G.; Andrikopoulos, K.S.; Polyzou, C.D.; Tangoulis, V.; Voyiatzis, G.A. Monitoring the spin crossover phenomenon of [Fe(2-mpz)2Ni(CN)4] 2D Hofmann-type polymer nanoparticles via temperature-dependent Raman spectroscopy. J. Raman Spectrosc. 2020, 51, 2171–2181. [Google Scholar] [CrossRef]
- Gütlich, P.; Goodwin, H.A. Spin Crossover—An Overall Perspective. In Spin Crossover in Transition Metal Compounds I; Gütlich, P., Goodwin, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 1–47. [Google Scholar]
- Brooker, S. Spin crossover with thermal hysteresis: Practicalities and lessons learnt. Chem. Soc. Rev. 2015, 44, 2880–2892. [Google Scholar] [CrossRef]
- Enriquez-Cabrera, A.; Rapakousiou, A.; Bello, M.P.; Molnár, G.; Salmon, L.; Bousseksou, A. Spin crossover polymer composites, polymers and related soft materials. Coord. Chem. Rev. 2020, 419, 213396. [Google Scholar] [CrossRef]
- Molnár, G.; Rat, S.; Salmon, L.; Nicolazzi, W.; Bousseksou, A. Spin Crossover Nanomaterials: From Fundamental Concepts to Devices. Adv. Mater. 2018, 30, 1703862. [Google Scholar] [CrossRef]
- Li, H.; Peng, H. Recent advances in self-assembly of spin crossover materials and their applications. Curr. Opin. Colloid Interface Sci. 2018, 35, 9–16. [Google Scholar] [CrossRef]
- Sakaida, S.; Otsubo, K.; Maesato, M.; Kitagawa, H. Crystal Size Effect on the Spin-Crossover Behavior of {Fe(py)2[Pt(CN)4]} (py = Pyridine) Monitored by Raman Spectroscopy. Inorg. Chem. 2020, 59, 16819–16823. [Google Scholar] [CrossRef]
- Reeves, M.G.; Tailleur, E.; Wood, P.A.; Marchivie, M.; Chastanet, G.; Guionneau, P.; Parsons, S. Mapping the cooperativity pathways in spin crossover complexes. Chem. Sci. 2021, 12, 1007–1015. [Google Scholar] [CrossRef]
- Vela, S.; Paulsen, H. Cooperativity in Spin Crossover Systems. An Atomistic Perspective on the Devil’s Staircase. Inorg. Chem. 2018, 57, 9478–9488. [Google Scholar] [CrossRef]
- Kipgen, L.; Bernien, M.; Ossinger, S.; Nickel, F.; Britton, A.J.; Arruda, L.M.; Naggert, H.; Luo, C.; Lotze, C.; Ryll, H.; et al. Evolution of cooperativity in the spin transition of an iron (II) complex on a graphite surface. Nat. Commun. 2018, 9, 2984. [Google Scholar] [CrossRef]
- Gütlich, P.; Goodwin, H.A. (Eds.) Spin Crossover in Transition Metal Compounds; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Halcrow, M.A. (Ed.) Spin-Crossover Materials; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Gütlich, P.; Garcia, Y.; Goodwin, H.A. Spin crossover phenomena in Fe (II) complexes. Chem. Soc. Rev. 2000, 29, 419–427. [Google Scholar] [CrossRef]
- Bousseksou, A.; McGarvey, J.J.; Varret, F.; Real, J.A.; Tuchagues, J.-P.; Dennis, A.C.; Boillot, M.L. Raman spectroscopy of the high- and low-spin states of the spin crossover complex Fe(phen)2(NCS)2: An initial approach to estimation of vibrational contributions to the associated entropy change. Chem. Phys. Lett. 2000, 318, 409–416. [Google Scholar] [CrossRef]
- Batschelet, W.H.; Rose, N.J. Laser Raman spectroscopic studies of metal.alpha.-diimine complexes. 1. Imine stretching frequencies and bonding implications. Inorg. Chem. 1983, 22, 2078–2083. [Google Scholar] [CrossRef]
- Feltham, H.L.C.; Johnson, C.; Elliott, A.B.S.; Gordon, K.C.; Albrecht, M.; Brooker, S. “Tail” Tuning of Iron (II) Spin Crossover Temperature by 100 K. Inorg. Chem. 2015, 54, 2902–2909. [Google Scholar] [CrossRef] [PubMed]
- Miyazaki, Y.; Nakamoto, T.; Ikeuchi, S.; Saito, K.; Inaba, A.; Sorai, M.; Tojo, T.; Atake, T.; Matouzenko, G.S.; Zein, S.; et al. Spin Crossover Phenomenon Accompanying Order−Disorder Phase Transition in the Ligand of [FeII(DAPP)(abpt)](ClO4)2 Compound (DAPP = Bis(3-aminopropyl)(2-pyridylmethyl)amine, abpt = 4-Amino-3,5-bis(pyridin-2-yl)-1,2,4-triazole) and Its Successive Self-Grinding Effect. J. Phys. Chem. B 2007, 111, 12508–12517. [Google Scholar] [PubMed]
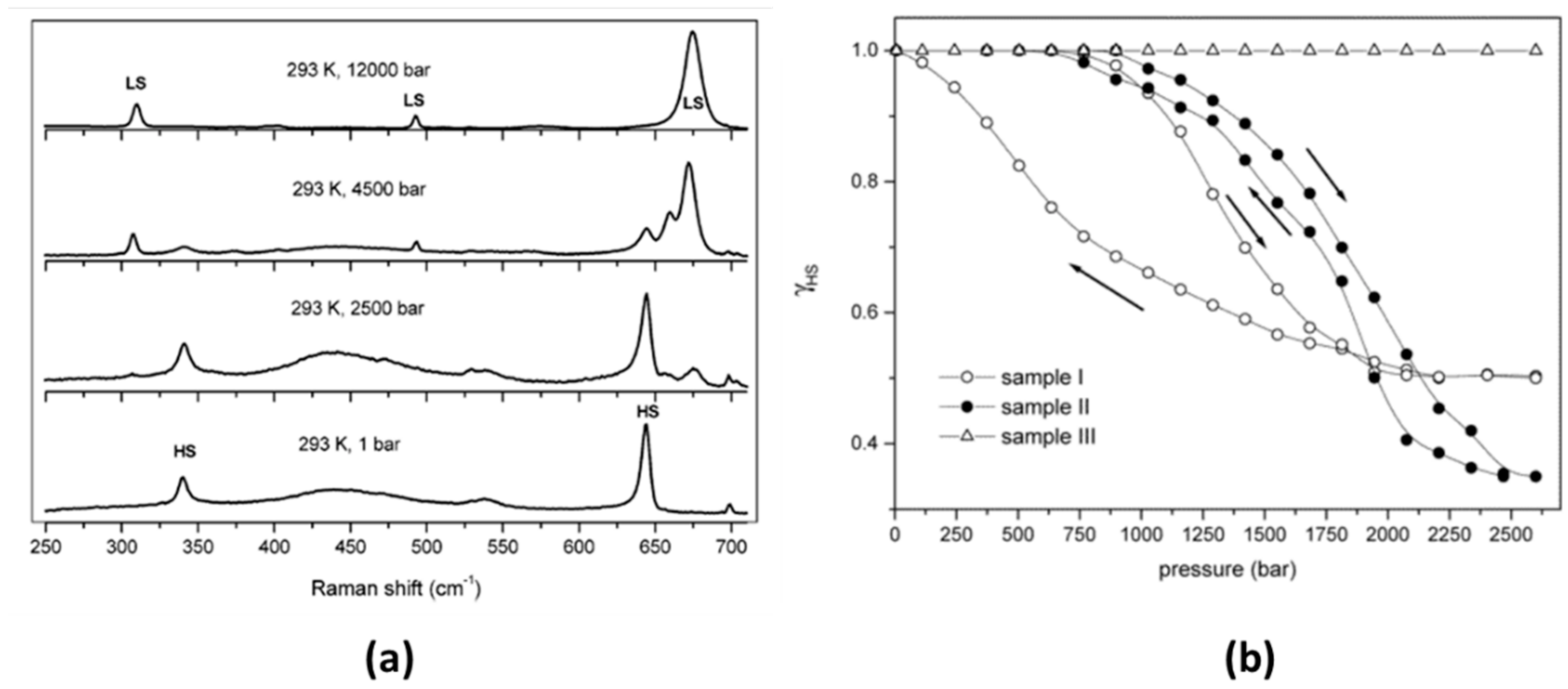
- Molnár, G.; Niel, V.; Real, J.-A.; Dubrovinsky, L.; Bousseksou, A.; McGarvey, J.J. Raman Spectroscopic Study of Pressure Effects on the Spin-Crossover Coordination Polymers Fe(Pyrazine)[M(CN)4]·2H2O (M = Ni, Pd, Pt). First Observation of a Piezo-Hysteresis Loop at Room Temperature. J. Phys. Chem. B 2003, 107, 3149–3155. [Google Scholar] [CrossRef]
- Moussa, N.O.; Molnár, G.; Ducros, X.; Zwick, A.; Tayagaki, T.; Tanaka, K.; Bousseksou, A. Decoupling of the molecular spin-state and the crystallographic phase in the spin-crossover complex [Fe(ptz)6](BF4)2 studied by Raman spectroscopy. Chem. Phys. Lett. 2005, 402, 503–509. [Google Scholar] [CrossRef]
- Molnár, G.; Niel, V.; Gaspar, A.B.; Real, J.-A.; Zwick, A.; Bousseksou, A.; McGarvey, J.J. Vibrational Spectroscopy of Cyanide-Bridged, Iron(II) Spin-Crossover Coordination Polymers: Estimation of Vibrational Contributions to the Entropy Change Associated with the Spin Transition. J. Phys. Chem. B 2002, 106, 9701–9707. [Google Scholar] [CrossRef]
- Craig, G.A.; Costa, J.S.; Roubeau, O.; Teat, S.J.; Shepherd, H.J.; Lopes, M.; Molnár, G.; Bousseksou, A.; Aromí, G. High-temperature photo-induced switching and pressure-induced transition in a cooperative molecular spin-crossover material. Dalton Trans. 2014, 43, 729–737. [Google Scholar] [CrossRef]
- McAllen Public Utility Water Plants. Available online: https://mcallenpublicutility.com/departments/water-systems/water-plants/ (accessed on 2 March 2022).
- Larionova, J.; Salmon, L.; Guari, Y.; Tokarev, A.; Molvinger, K.; Molnár, G.; Bousseksou, A. Towards the Ultimate Size Limit of the Memory Effect in Spin-Crossover Solids. Angew. Chem. Int. Ed. 2008, 47, 8236–8240. [Google Scholar] [CrossRef]
- Raza, Y.; Volatron, F.; Moldovan, S.; Ersen, O.; Huc, V.; Martini, C.; Brisset, F.; Gloter, A.; Stéphan, O.; Bousseksou, A.; et al. Matrix-dependent cooperativity in spin crossover Fe(pyrazine)Pt(CN)4 nanoparticles. Chem. Commun. 2011, 47, 11501–11503. [Google Scholar] [CrossRef]
- Tokarev, A.; Long, J.; Guari, Y.; Larionova, J.; Quignard, F.; Agulhon, P.; Robitzer, M.; Molnár, G.; Salmon, L.; Bousseksou, A. Spin crossover polysaccharide nanocomposites. New J. Chem. 2013, 37, 3420–3432. [Google Scholar] [CrossRef]
- Available online: https://ec.europa.eu/environment/soil/soil_policy_en.htm (accessed on 11 June 2020).
- Ronayne, K.L.; Paulsen, H.; Höfer, A.; Dennis, A.C.; Wolny, J.A.; Chumakov, A.I.; Schünemann, V.; Winkler, H.; Spiering, H.; Bousseksou, A.; et al. Vibrational spectrum of the spin crossover complex [Fe(phen)2(NCS)2] studied by IR and Raman spectroscopy, nuclear inelastic scattering and DFT calculations. Phys. Chem. Chem. Phys. 2006, 8, 4685–4693. [Google Scholar] [CrossRef]
- Wolny, J.A.; Diller, R.; Schünemann, V. Vibrational Spectroscopy of Mono- and Polynuclear Spin-Crossover Systems. Eur. J. Inorg. Chem. 2012, 2012, 2635–2648. [Google Scholar] [CrossRef]
- Tuchagues, J.-P.; Bousseksou, A.; Molnár, G.; McGarvey, J.J.; Varret, F. The Role of Molecular Vibrations in the Spin Crossover Phenomenon. In Spin Crossover in Transition Metal Compounds III; Gütlich, P., Goodwin, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 84–103. [Google Scholar]
- Wolny, J.A.; Schünemann, V.; Németh, Z.; Vankó, G. Spectroscopic techniques to characterize the spin state: Vibrational, optical, Mössbauer, NMR, and X-ray spectroscopy. Comptes Rendus Chim. 2018, 21, 1152–1169. [Google Scholar] [CrossRef]
- Molnár, G.; Mikolasek, M.; Ridier, K.; Fahs, A.; Nicolazzi, W.; Bousseksou, A. Molecular Spin Crossover Materials: Review of the Lattice Dynamical Properties. Ann. Phys. 2019, 531, 1900076. [Google Scholar] [CrossRef]
- Enamullah, M.; Linert, W.; Gutmann, V. Vibrational spectroscopy on iron (II) spin-crossover complexes with 4-substituted 2,6-bis (benzimidazol-2′-yl) pyridine. Vib. Spectrosc. 1995, 9, 265–271. [Google Scholar] [CrossRef]
- Wolny, J.A.; Paulsen, H.; Trautwein, A.X.; Schünemann, V. Density functional theory calculations and vibrational spectroscopy on iron spin-crossover compounds. Coord. Chem. Rev. 2009, 253, 2423–2431. [Google Scholar] [CrossRef]
- Urakawa, A.; van Beek, W.; Monrabal-Capilla, M.; Galán-Mascarós, J.R.; Palin, L.; Milanesio, M. Combined, Modulation Enhanced X-ray Powder Diffraction and Raman Spectroscopic Study of Structural Transitions in the Spin Crossover Material [Fe(Htrz)2(trz)](BF4). J. Phys. Chem. C 2011, 115, 1323–1329. [Google Scholar] [CrossRef]
- Bedoui, S.; Molnár, G.; Bonnet, S.; Quintero, C.; Shepherd, H.J.; Nicolazzi, W.; Salmon, L.A. Bousseksou, Raman spectroscopic and optical imaging of high spin/low spin domains in a spin crossover complex. Chem. Phys. Lett. 2010, 499, 94–99. [Google Scholar] [CrossRef]
- Guillaume, F.; Tobon, Y.A.; Bonhommeau, S.; Létard, J.-F.; Moulet, L.; Freysz, E. Photoswitching of the spin crossover polymeric material [Fe(Htrz)2(trz)](BF4) under continuous laser irradiation in a Raman scattering experiment. Chem. Phys. Lett. 2014, 604, 105–109. [Google Scholar] [CrossRef]
- Sorai, M.; Seki, S. Phonon coupled cooperative low-spin 1A1high-spin 5T2 transition in [Fe(phen)2(NCS)2] and [Fe(phen)2(NCSe)2] crystals. J. Phys. Chem. Solids 1974, 35, 555–570. [Google Scholar] [CrossRef]
- Roubeau, O.; Castro, M.; Burriel, R.; Haasnoot, J.G.; Reedijk, J. Calorimetric Investigation of Triazole-Bridged Fe (II) Spin-Crossover One-Dimensional Materials: Measuring the Cooperativity. J. Phys. Chem. B 2011, 115, 3003–3012. [Google Scholar] [CrossRef]
- Brehm, G.; Reiher, M.; Schneider, S. Estimation of the Vibrational Contribution to the Entropy Change Associated with the Low- to High-Spin Transition in Fe(phen)2(NCS)2 Complexes: Results Obtained by IR and Raman Spectroscopy and DFT Calculations. J. Phys. Chem. A 2002, 106, 12024–12034. [Google Scholar] [CrossRef]
- Collet, E.; Guionneau, P. Structural analysis of spin-crossover materials: From molecules to materials. C. R. Chim. 2018, 21, 1133–1151. [Google Scholar] [CrossRef]
- Craze, A.R.; Marjo, C.E.; Li, F. A complementary characterisation technique for spin crossover materials; the application of X-ray photoelectron spectroscopy for future device applications. Dalton Trans. 2022, 51, 428–441. [Google Scholar] [CrossRef] [PubMed]
- Chong, C.; Berini, B.; Boukheddaden, K.; Codjovi, E.; Linarès, J.; Garcia, Y.; Naik, A.D.; Varret, F. Characterization of spin crossover crystal surface by AFM. Phys. Status Solidi a 2010, 207, 1227–1231. [Google Scholar] [CrossRef]
- Cini, A.; Poggini, L.; Chumakov, A.I.; Rüffer, R.; Spina, G.; Wattiaux, A.; Duttine, M.; Gonidec, M.; Fittipaldi, M.; Rosa, P.; et al. Synchrotron-based Mössbauer spectroscopy characterization of sublimated spin crossover molecules. Phys. Chem. Chem. Phys. 2020, 22, 6626–6637. [Google Scholar] [CrossRef]
- Gütlich, P. Spin Crossover—Quo Vadis? Eur. J. Inorg. Chem. 2013, 2013, 581–591. [Google Scholar] [CrossRef]
- Collet, E.; Azzolina, G.; Ichii, T.; Guerin, L.; Bertoni, R.; Moréac, A.; Cammarata, M.; Daro, N.; Chastanet, G.; Kubicki, J.; et al. Lattice phonon modes of the spin crossover crystal [Fe(phen)2(NCS)2] studied by THz, IR, Raman spectroscopies and DFT calculations. Eur. Phys. J. B 2019, 92, 12. [Google Scholar] [CrossRef]
- Brady, C.; Callaghan, P.L.; Ciunik, Z.; Coates, C.G.; Døssing, A.; Hazell, A.; McGarvey, J.J.; Schenker, S.; Toftlund, H.; Trautwein, A.X.; et al. Molecular Structure and Vibrational Spectra of Spin-Crossover Complexes in Solution and Colloidal Media: Resonance Raman and Time-Resolved Resonance Raman Studies. Inorg. Chem. 2004, 43, 4289–4299. [Google Scholar] [CrossRef]
- Brady, C.; McGarvey, J.J.; McCusker, J.K.; Toftlund, H.; Hendrickson, D.N. Time-Resolved Relaxation Studies of Spin Crossover Systems in Solution. In Spin Crossover in Transition Metal Compounds III; Gütlich, P., Goodwin, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 1–22. [Google Scholar]
- Smeigh, A.L.; Creelman, M.; Mathies, R.A.; McCusker, J.K. Femtosecond Time-Resolved Optical and Raman Spectroscopy of Photoinduced Spin Crossover: Temporal Resolution of Low-to-High Spin Optical Switching. J. Am. Chem. Soc. 2008, 130, 14105–14107. [Google Scholar] [CrossRef]
- Rat, S.; Mikolasek, M.; Costá, J.S.; Chumakov, A.I.; Nicolazzi, W.; Molnár, G.; Salmon, L.; Bousseksou, A. Raman and nuclear inelastic scattering study of the lattice dynamics of the [Fe(H2B(pz)2)2(phen)] spin crossover complex. Chem. Phys. Lett. 2016, 653, 131–136. [Google Scholar] [CrossRef]
- Takemoto, J.H.; Hutchinson, B. Low-frequency infrared spectra of complexes which exhibit magnetic crossover. I. Iron (II) complexes of 1,10-phenanthroline and 2,2′-bipyridine. Inorg. Chem. 1973, 12, 705–708. [Google Scholar] [CrossRef]
- Pálfi, V.K.; Guillon, T.; Paulsen, H.; Molnár, G.; Bousseksou, A. Isotope effects on the vibrational spectra of the Fe(Phen)2(NCS)2 spin-crossover complex studied by density functional calculations. C. R. Chim. 2005, 8, 1317–1325. [Google Scholar] [CrossRef]
- Suryadevara, N.; Mizuno, A.; Spieker, L.; Salamon, S.; Sleziona, S.; Maas, A.; Pollmann, E.; Heinrich, B.; Schleberger, M.; Wende, H.; et al. Structural Insights into Hysteretic Spin-Crossover in a Set of Iron(II)-2,6-bis(1H-Pyrazol-1-yl)Pyridine) Complexes. Chem. A Eur. J. 2022, 28, e202103853. [Google Scholar]
- Garcia, Y.; Niel, V.; Muñoz, M.C.; Real, J.A. Spin Crossover in 1D, 2D and 3D Polymeric Fe (II) Networks. In Spin Crossover in Transition Metal Compounds I; Gütlich, P., Goodwin, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 229–257. [Google Scholar]
- Ni, Z.-P.; Liu, J.-L.; Hoque, M.N.; Liu, W.; Li, J.-Y.; Chen, Y.-C.; Tong, M.-L. Recent advances in guest effects on spin-crossover behavior in Hofmann-type metal-organic frameworks. Coord. Chem. Rev. 2017, 335, 28–43. [Google Scholar] [CrossRef]
- Bartual-Murgui, C.; Akou, A.; Thibault, C.; Molnár, G.; Vieu, C.; Salmon, L.; Bousseksou, A. Spin-crossover metal–organic frameworks: Promising materials for designing gas sensors. J. Mater. Chem. C 2015, 3, 1277–1285. [Google Scholar] [CrossRef]
- Bousseksou, A.; Molnár, G.; Salmon, L.; Nicolazzi, W. Molecular spin crossover phenomenon: Recent achievements and prospects. Chem. Soc. Rev. 2011, 40, 3313–3335. [Google Scholar] [CrossRef]
- Dîrtu, M.; Schmit, F.; Naik, A.D.; Rusu, I.; Rotaru, A.; Rackwitz, S.; Wolny, J.A.; Schünemann, V.; Spinu, L.; Garcia, Y. Two-Step Spin Transition in a 1D FeII 1,2,4-Triazole Chain Compound. Chem. A Eur. J. 2015, 21, 5843–5855. [Google Scholar] [CrossRef]
- Bousseksou, A.; Molnár, G.; Real, J.A.; Tanaka, K. Spin crossover and photomagnetism in dinuclear iron (II) compounds. Coord. Chem. Rev. 2007, 251, 1822–1833. [Google Scholar] [CrossRef]
- Schmidt, S.O.; Naggert, H.; Buchholz, A.; Brandenburg, H.; Bannwarth, A.; Plass, W.; Tuczek, F. Thermal and Light-Induced Spin Transitions of FeII Complexes with 4- and 5-(Phenylazo)-2,2′-bipyridine Ligands: Intra- vs. Intermolecular Effects. Eur. J. Inorg. Chem. 2016, 2016, 2175–2186. [Google Scholar] [CrossRef]
- Harding, D.J.; Harding, P.; Phonsri, W. Spin crossover in iron (III) complexes. Coord. Chem. Rev. 2016, 313, 38–61. [Google Scholar] [CrossRef]
- Hayami, S.; Gu, Z.-z.; Yoshiki, H.; Fujishima, A.; Sato, O. Iron (III) Spin-Crossover Compounds with a Wide Apparent Thermal Hysteresis around Room Temperature. J. Am. Chem. Soc. 2001, 123, 11644–11650. [Google Scholar] [CrossRef] [PubMed]
- Griffin, M.; Shakespeare, S.; Shepherd, H.J.; Harding, C.J.; Létard, J.-F.; Desplanches, C.; Goeta, A.E.; Howard, J.A.K.; Powell, A.K.; Mereacre, V.; et al. A Symmetry-Breaking Spin-State Transition in Iron (III). Angew. Chem. Int. Ed. 2011, 50, 896–900. [Google Scholar] [CrossRef] [PubMed]
- Bertoni, R.; Lorenc, M.; Laisney, J.; Tissot, A.; Moréac, A.; Matar, S.F.; Boillot, M.L.; Collet, E. Femtosecond spin-state photo-switching dynamics in an FeIII spin crossover solid accompanied by coherent structural vibrations. J. Mater. Chem. C 2015, 3, 7792–7801. [Google Scholar] [CrossRef]
- Martinho, P.N.; Brendan, G.; Harris, M.M.; Lemma, T.; Naik, A.D.; Müller-Bunz, H.; Keyes, T.E.; Garcia, Y.; Morgan, G.G. Cooperative Spin Transition in a Mononuclear Manganese(III)Complex. Angew. Chem. Int. Ed. 2012, 124, 12765–12769. [Google Scholar] [CrossRef]
- Pandurangan, K.; Carter, A.B.; Martinho, P.N.; Gildea, B.; Lemma, T.; Shi, S.; Sultan, A.; Keyes, T.E.; Müller-Bunz, H.; Morgan, G.G. Steric Quenching of Mn (III) Thermal Spin Crossover: Dilution of Spin Centers in Immobilized Solutions. Magnetochemistry 2022, 8, 8. [Google Scholar] [CrossRef]
- Létard, J.-F.; Guionneau, P.; Goux-Capes, L. Towards Spin Crossover Applications. In Spin Crossover in Transition Metal Compounds III; Gütlich, P., Goodwin, H.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2004; pp. 221–249. [Google Scholar]
- Forestier, T.; Mornet, S.; Daro, N.; Nishihara, T.; Mouri, S.-i.; Tanaka, K.; Fouché, O.; Freysz, E.; Létard, J.-F. Nanoparticles of ironm (II) spin-crossover. Chem. Commun. 2008, 4327–4329. [Google Scholar] [CrossRef]
- Siddiqui, S.A.; Domanov, O.; Schafler, E.; Vejpravova, J.; Shiozawa, H. Synthesis and size-dependent spin crossover of coordination polymer [Fe(Htrz)2(trz)](BF4). J. Mater. Chem. C 2021, 9, 1077–1084. [Google Scholar] [CrossRef]
- Tissot, A.; Kesse, X.; Giannopoulou, S.; Stenger, I.; Binet, L.; Rivière, E.; Serre, C. A spin crossover porous hybrid architecture for potential sensing applications. Chem. Commun. 2019, 55, 194–197. [Google Scholar] [CrossRef]
- Hosoya, K.; Kitazawa, T.; Takahashi, M.; Takeda, M.; Meunier, J.-F.; Molnár, G.; Bousseksou, A. Unexpected isotope effect on the spin transition of the coordination polymer Fe(C5H5N)2[Ni(CN)4]. Phys. Chem. Chem. Phys. 2003, 5, 1682–1688. [Google Scholar] [CrossRef]
- Hernández, E.M.; Zheng, S.; Shepherd, H.J.; Yufit, D.S.; Ridier, K.; Bedoui, S.; Nicolazzi, W.; Velázquez, V.; Bonnet, S.; Molnár, G.; et al. Spatially Resolved Investigation and Control of the Bistability in Single Crystals of the [Fe(bbpya) (NCS)2] Spin Crossover Complex. J. Phys. Chem. C 2016, 120, 27608–27617. [Google Scholar] [CrossRef][Green Version]
- Lada, Z.G.; Chrissanthopoulos, A.; Perlepes, S.P.; Andrikopoulos, K.S.; Voyiatzis, G.A. Wet-chemistry assembly of one-dimensional nanowires: Switching characteristics of a known spin-crossover iron (II) complex through Raman spectroscopy. Chem. Commun. 2022, 58, 521–524. [Google Scholar] [CrossRef] [PubMed]
- Attwood, M.; Akutsu, H.; Martin, L.; Blundell, T.J.; le Maguere, P.; Turner, S.S. Exceptionally high temperature spin crossover in amide-functionalised 2,6-bis(pyrazol-1-yl)pyridine iron (II) complex revealed by variable temperature Raman spectroscopy and single crystal X-ray diffraction. Dalton Transact. 2021, 50, 11843–11851. [Google Scholar] [CrossRef] [PubMed]
- Lada, Z.G.; Andrikopoulos, K.S.; Mathioudakis, G.N.; Piperigkou, Z.; Karamanos, N.; Perlepes, S.P.; Voyiatzis, G.A. Tuning the Spin-Crossover Behaviour in Fe(II) Polymeric Composites for Food Packaging Applications. Magnetochemistry 2022, 8, 16. [Google Scholar] [CrossRef]
- Lada, Z.G.; Beobide, A.S.; Mathioudakis, G.N.; Voyiatzis, G.A. Fe (II) Spin Crossover/Polymer Hybrid Materials: Investigation of the SCO Behavior via Temperature-Dependent Raman Spectroscopy, Physicochemical Characterization and Migration Release Study. Molecules 2021, 26, 201. [Google Scholar] [CrossRef] [PubMed]
- Hiiuk, V.M.; Shylin, S.I.; Barakhtii, D.D.; Korytko, D.M.; Kotsyubynsky, V.O.; Rotaru, A.; Shova, S.; Gural’skiy, I.y.A. Two-Step Spin Crossover in Hofmann-Type Coordination Polymers [Fe(2-phenylpyrazine)2{M(CN)2}2] (M = Ag, Au). Inorg. Chem. 2022, 61, 2093–2104. [Google Scholar] [CrossRef]
- Volatron, F.; Catala, L.; Rivière, E.; Gloter, A.; Stéphan, O.; Mallah, T. Spin-Crossover Coordination Nanoparticles. Inorg. Chem. 2008, 47, 6584–6586. [Google Scholar] [CrossRef]
- Sakaida, S.; Otsubo, K.; Otake, K.-i.; Kawaguchi, S.; Maesato, M.; Kitagawa, S.; Kitagawa, H. Surface morphology-induced spin-crossover-inactive high-spin state in a coordination framework. Chem. Commun. 2021, 57, 1462–1465. [Google Scholar] [CrossRef]
- Maskowicz, D.; Sawczak, M.; Ghosh, A.C.; Grochowska, K.; Jendrzejewski, R.; Rotaru, A.; Garcia, Y.; Śliwiński, G. Spin crossover and cooperativity in nanocrystalline [Fe(pyrazine)Pt(CN)4] thin films deposited by matrix-assisted laser evaporation. Appl. Surface Sci. 2021, 541, 148419. [Google Scholar] [CrossRef]
- Jureschi, C.-M.; Linares, J.; Boulmaali, A.; Dahoo, P.R.; Rotaru, A.; Garcia, Y. Pressure and Temperature Sensors Using Two Spin Crossover Materials. Sensors 2016, 16, 187. [Google Scholar] [CrossRef]
- Roubeau, O.; Colin, A.; Schmitt, V.; Clérac, R. Thermoreversible Gels as Magneto-Optical Switches. Angew. Chem. Int. Ed. 2004, 43, 3283–3286. [Google Scholar] [CrossRef]
- Ababei, R.; Pichon, C.; Roubeau, O.; Li, Y.-G.; Bréfuel, N.; Buisson, L.; Guionneau, P.; Mathonière, C.; Clérac, R. Rational Design of a Photomagnetic Chain: Bridging Single-Molecule Magnets with a Spin-Crossover Complex. J. Am. Chem. Soc. 2013, 135, 14840–14853. [Google Scholar] [CrossRef] [PubMed]
- Gaspar, A.B.; Ksenofontov, V.; Seredyuk, M.; Gütlich, P. Multifunctionality in spin crossover materials. Coord. Chem. Rev. 2005, 249, 2661–2676. [Google Scholar] [CrossRef]
- Ge, J.-Y.; Chen, Z.; Zhang, L.; Liang, X.; Su, J.; Kurmoo, M.; Zuo, J.-L. A Two-Dimensional Iron (II) Coordination Polymer with Synergetic Spin-Crossover and Luminescent Properties. Angew. Chem. Int. Ed. 2019, 58, 8789–8793. [Google Scholar] [CrossRef]
- Kumar, K.S.; Ruben, M. Emerging trends in spin crossover (SCO) based functional materials and devices. Coord. Chem. Rev. 2017, 346, 176–205. [Google Scholar] [CrossRef]
- Devid, E.J.; Martinho, P.N.; Kamalakar, M.V.; Šalitroš, I.; Prendergast, Ú.; Dayen, J.-F.; Meded, V.; Lemma, T.; González-Prieto, R.; Evers, F.; et al. Spin Transition in Arrays of Gold Nanoparticles and Spin Crossover Molecules. ACS Nano 2015, 9, 4496–4507. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.-H.; Wang, Y.-X.; Han, W.-K.; Zhu, W.; Li, T.; Li, Z.; Ren, X.; Gu, Z.-G. Integrating spin-crossover nanoparticles with silver nanowires: Toward magnetic and conductive bifunctional nanomaterials. New J. Chem. 2017, 41, 10062–10068. [Google Scholar] [CrossRef]
- Tanaka, D.; Aketa, N.; Tanaka, H.; Horike, S.; Fukumori, M.; Tamaki, T.; Inose, T.; Akai, T.; Toyama, H.; Sakata, O.; et al. Facile preparation of hybrid thin films composed of spin-crossover nanoparticles and carbon nanotubes for electrical memory devices. Dalton Trans. 2019, 48, 7074–7079. [Google Scholar] [CrossRef] [PubMed]
- Lapresta-Fernández, A.; Titos-Padilla, S.; Herrera, J.M.; Salinas-Castillo, A.; Colacio, E.; Vallvey, L.F.C. Photographing the synergy between magnetic and colour properties in spin crossover material [Fe(NH2trz)3](BF4)2: A temperature sensor perspective. Chem. Commun. 2013, 49, 288–290. [Google Scholar] [CrossRef]
- Tsukiashi, A.; Min, K.S.; Kitayama, H.; Terasawa, H.; Yoshinaga, S.; Takeda, M.; Lindoy, L.F.; Hayami, S. Application of spin-crossover water soluble nanoparticles for use as MRI contrast agents. Sci. Rep. 2018, 8, 14911. [Google Scholar] [CrossRef]
- Gkolfi, P.; Tsivaka, D.; Tsougos, I.; Vassiou, K.; Malina, O.; Polaskova, M.; Polyzou, C.D.; Chasapis, C.T.; Tangoulis, V. A facile approach to prepare silica hybrid, spin-crossover water-soluble nanoparticles as potential candidates for thermally responsive MRI agents. Dalton Trans. 2021, 50, 13227–13231. [Google Scholar] [CrossRef]

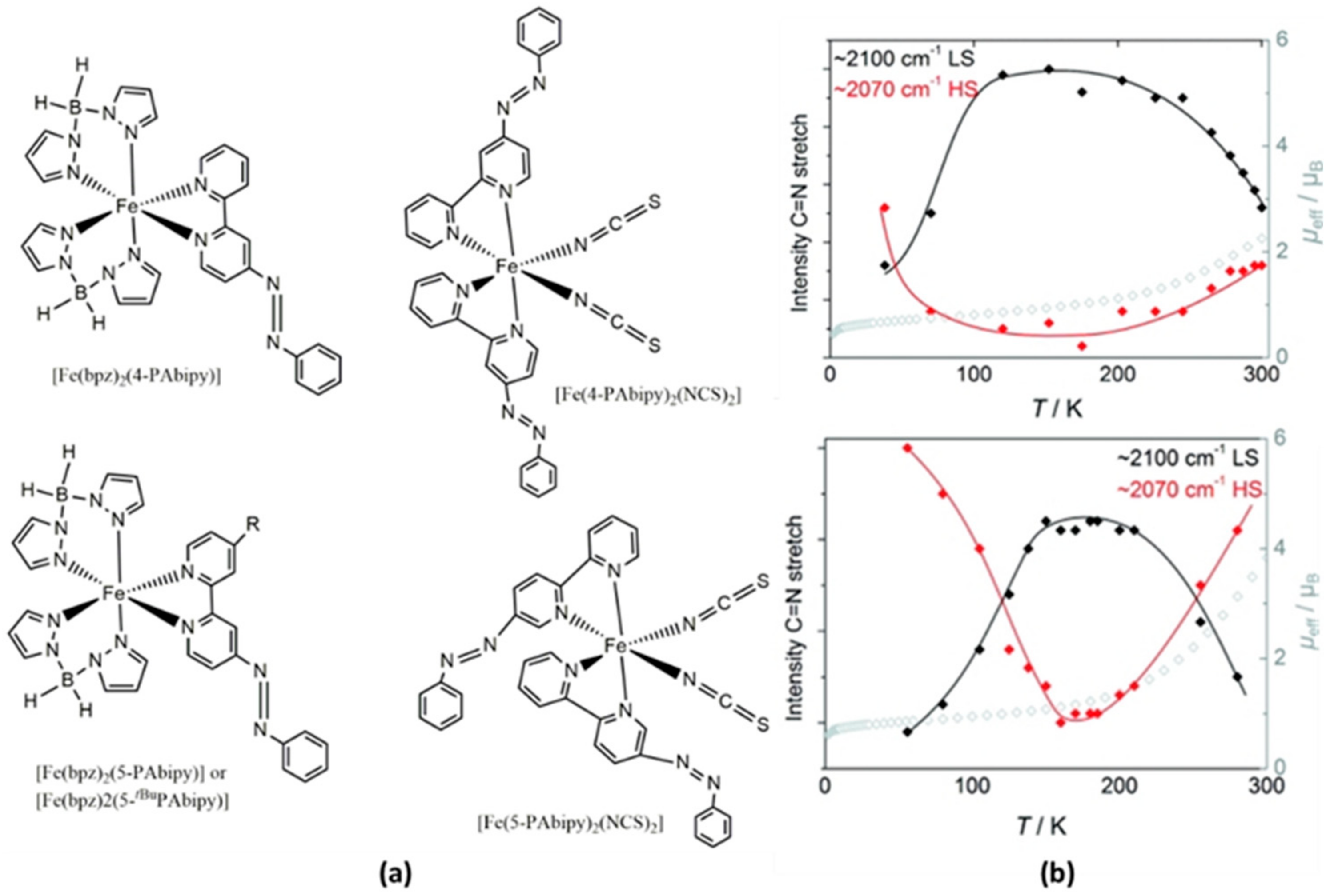
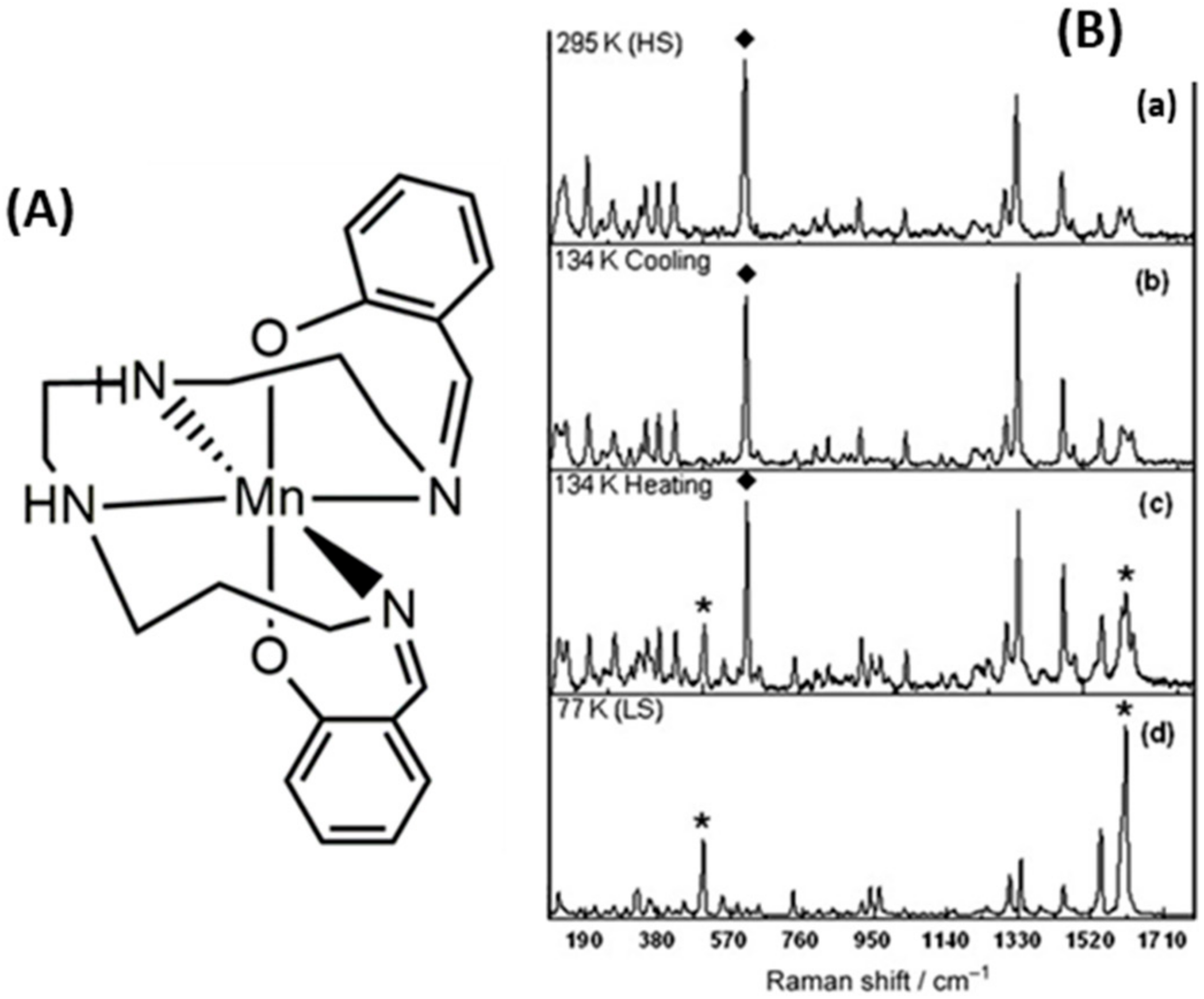

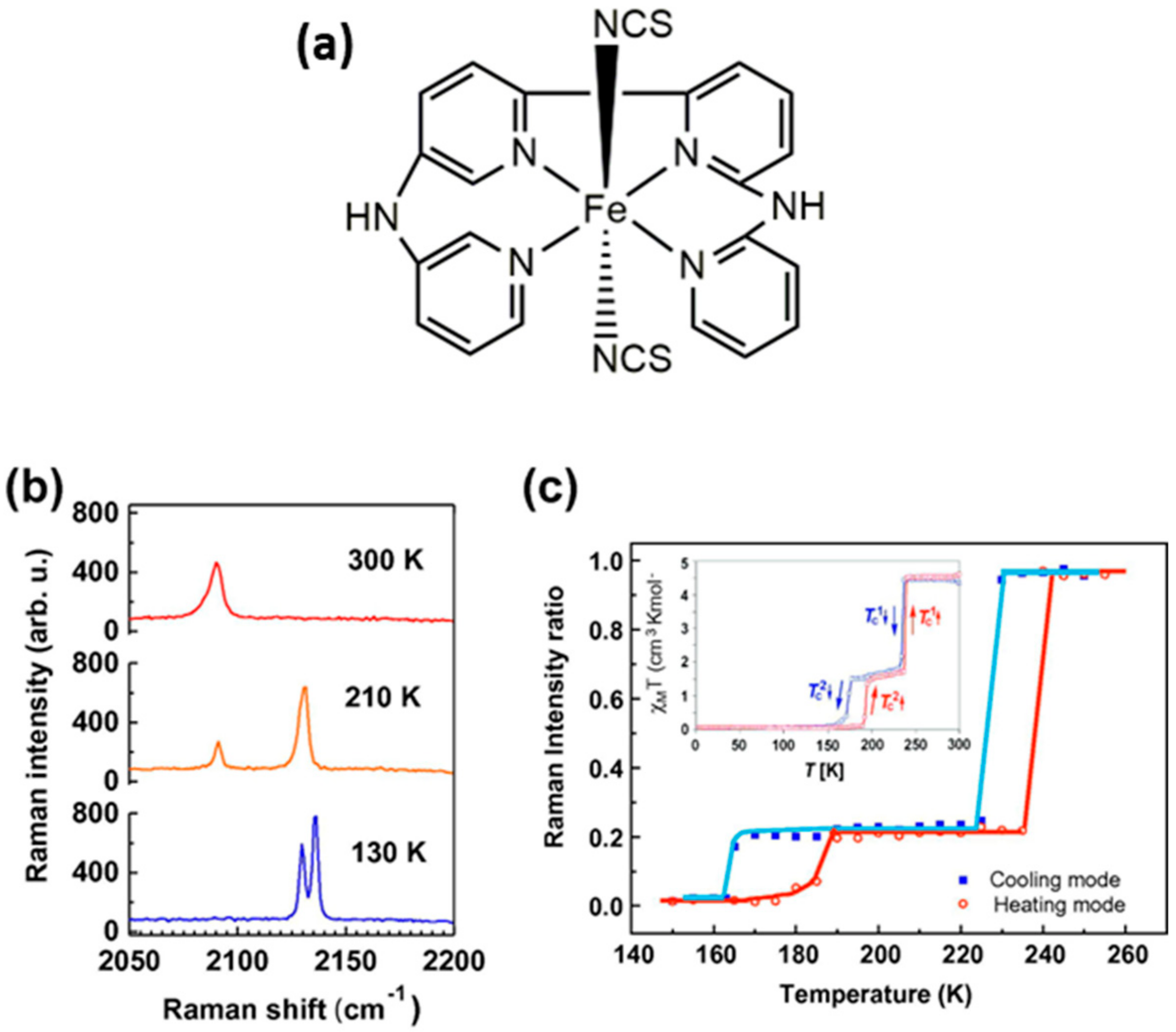
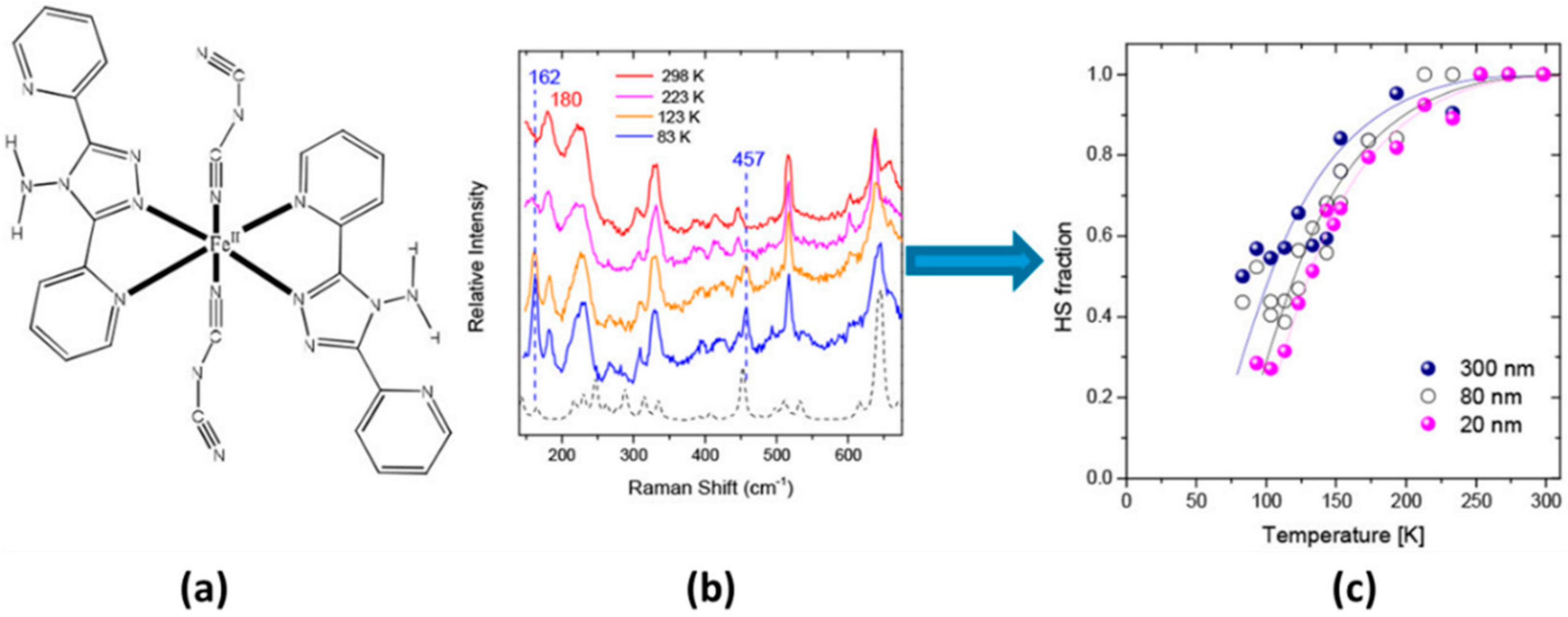


| Method | Main Features | Advantages | Disadvantages | SCO Characteristics Being Recorded |
|---|---|---|---|---|
| Magnetic susceptibility (Principal technique) | Variation of magnetic susceptibility as a function of temperature due to drastic transition from a strongly paramagnetic HS state to a weakly paramagnetic or even diamagnetic LS state | High sensitivity and accuracy, spin-state variations under various external perturbations (pressure, temperature, light irradiation) | Operation at cryogenic temperatures, bulk technique | T1/2 values, curve of SCO transition, calculation of HS population |
| 57Fe Mössbauer | Evaluate separate spin states during the SCO process based on spectral parameters | High sensitivity, characterization of electronic structure, and molecular structure modifications | Only for iron SCO complexes being limited to the 57-Fe isotope leading to necessicity of istopic enrichment in some cases, bulk technique | T1/2 values, curve of SCO transition, calculation of HS population |
| X-rays | Peak splitting for HS/LS components and intensities increase/decrease proportionally to the macroscopic spin conversion level | Insight on electronic and molecular structure | Limitation to study crystals that do not decompose upon transition, bulk technique | T1/2 values, curve of SCO transition, calculation of HS population |
| DSC | Monitoring of the phase transition taking place during the SCO process | Can also facilitate the determination of the ΔH and ΔS occurring during SCO event | Limited to temperature-induced SCO, can be utilized in a specific temperature window, bulk technique | T1/2 values, ΔS, ΔH calculation of the phase transition |
| UV/Vis | Visible change in color due to the usual shift of the charge transfer transition during the SCO to lower energy at low temperatures | Ultrafast time-dependent UV–vis spectroscopy together with X-rays–has been used to characterize the photoinduced kinetics, comparing the dynamical processes in solid to that observed in solution | Usually small changes in UV/Vis band during SCO | T1/2 values, curve of SCO transition, calculation of HS population |
| Infrared | Vibrational pattern upon spin switching | A quick and efficient way of monitoring LIESST effect, time-dependent IR may provide unique information on the dynamics of SCO systems | Spin marker bands of metal–ligand stretchings require spectra in the far-IR region (<400 cm−1); not easily accessible | T1/2 values, curve of SCO transition, calculation of HS population, dynamic properties |
| Raman | Monitoring of the structural differentiations and phase transition taking place during the SCO process | High sensitivity, characterization of structure, and molecular structure modifications can also facilitate the determination of ΔS occurring during SCO event; μ-Raman allows the study of a specific spot of sample (non-bulk) | Preresonant Raman effect, laser-induced heat effects (induce of transition), laser induce LIEST | T1/2 values, curve of SCO transition, calculation of HS population, ΔS, ΔH calculation of the phase transition |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lada, Z.G. The Investigation of Spin-Crossover Systems by Raman Spectroscopy: A Review. Magnetochemistry 2022, 8, 108. https://doi.org/10.3390/magnetochemistry8090108
Lada ZG. The Investigation of Spin-Crossover Systems by Raman Spectroscopy: A Review. Magnetochemistry. 2022; 8(9):108. https://doi.org/10.3390/magnetochemistry8090108
Chicago/Turabian StyleLada, Zoi G. 2022. "The Investigation of Spin-Crossover Systems by Raman Spectroscopy: A Review" Magnetochemistry 8, no. 9: 108. https://doi.org/10.3390/magnetochemistry8090108
APA StyleLada, Z. G. (2022). The Investigation of Spin-Crossover Systems by Raman Spectroscopy: A Review. Magnetochemistry, 8(9), 108. https://doi.org/10.3390/magnetochemistry8090108






