EPR Spectroscopy of Cu(II) Complexes: Prediction of g-Tensors Using Double-Hybrid Density Functional Theory
Abstract
1. Introduction
2. Methodology
2.1. Benchmark Set of Copper Complexes
2.2. Overview of Double Hybrid Density Functionals
3. Results and Discussion
3.1. Evaluation Criteria
3.2. Performance of Functionals
4. Conclusions
5. Computational Details
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Karlin, K.D.; Tyeklár, Z. Bioinorganic Chemistry of Copper; Springer: Dordrecht, The Netherlands, 2013; ISBN 978-94-011-6875-5. [Google Scholar]
- Kaim, W.; Schwederski, B.; Klein, A. Bioinorganic Chemistry: Inorganic Elements in the Chemistry of Life: An Introduction and Guide, 2nd ed.; Inorganic Chemistry: A Wiley Series of Advanced Textbooks; Wiley: Chichester, UK, 2013; ISBN 978-0-470-97524-4. [Google Scholar]
- Festa, R.A.; Thiele, D.J. Copper: An Essential Metal in Biology. Curr. Biol. 2011, 21, R877–R883. [Google Scholar] [CrossRef] [PubMed]
- Solomon, E.I.; Heppner, D.E.; Johnston, E.M.; Ginsbach, J.W.; Cirera, J.; Qayyum, M.; Kieber-Emmons, M.T.; Kjaergaard, C.H.; Hadt, R.G.; Tian, L. Copper Active Sites in Biology. Chem. Rev. 2014, 114, 3659–3853. [Google Scholar] [CrossRef]
- Belle, C.; Rammal, W.; Pierre, J. Sulfur Ligation in Copper Enzymes and Models. J. Inorg. Biochem. 2005, 99, 1929–1936. [Google Scholar] [CrossRef] [PubMed]
- MacPherson, I.S.; Murphy, M.E.P. Type-2 Copper-Containing Enzymes. Cell. Mol. Life Sci. 2007, 64, 2887–2899. [Google Scholar] [CrossRef]
- Pretzler, M.; Rompel, A. What Causes the Different Functionality in Type-III-Copper Enzymes? A State of the Art Perspective. Inorg. Chim. Acta 2018, 481, 25–31. [Google Scholar] [CrossRef]
- Peisach, J.; Blumberg, W.E. Structural Implications Derived from the Analysis of Electron Paramagnetic Resonance Spectra of Natural and Artificial Copper Proteins. Arch. Biochem. Biophys. 1974, 165, 691–708. [Google Scholar] [CrossRef]
- Solomon, E.I.; Hare, J.W.; Dooley, D.M.; Dawson, J.H.; Stephens, P.J.; Gray, H.B. Spectroscopic Studies of Stellacyanin, Plastocyanin, and Azurin. Electronic Structure of the Blue Copper Sites. J. Am. Chem. Soc. 1980, 102, 168–178. [Google Scholar] [CrossRef]
- Gewirth, A.A.; Cohen, S.L.; Schugar, H.J.; Solomon, E.I. Spectroscopic and Theoretical Studies of the Unusual EPR Parameters of Distorted Tetrahedral Cupric Sites: Correlations to x-Ray Spectral Features of Core Levels. Inorg. Chem. 1987, 26, 1133–1146. [Google Scholar] [CrossRef]
- Shadle, S.E.; Penner-Hahn, J.E.; Schugar, H.J.; Hedman, B.; Hodgson, K.O.; Solomon, E.I. X-Ray Absorption Spectroscopic Studies of the Blue Copper Site: Metal and Ligand K-Edge Studies to Probe the Origin of the EPR Hyperfine Splitting in Plastocyanin. J. Am. Chem. Soc. 1993, 115, 767–776. [Google Scholar] [CrossRef]
- Andersson, K.K.; Schmidt, P.P.; Katterle, B.; Strand, K.R.; Palmer, A.E.; Lee, S.-K.; Solomon, E.I.; Gräslund, A.; Barra, A.-L. Examples of High-Frequency EPR Studies in Bioinorganic Chemistry. J. Biol. Inorg. Chem. 2003, 8, 235–247. [Google Scholar] [CrossRef]
- de Almeida, K.J.; Rinkevicius, Z.; Hugosson, H.W.; Ferreira, A.C.; Ågren, H. Modeling of EPR Parameters of Copper(II) Aqua Complexes. Chem. Phys. 2007, 332, 176–187. [Google Scholar] [CrossRef]
- Kaupp, M.; Bühl, M.; Malkin, V.G. (Eds.) Calculation of NMR and EPR Parameters: Theory and Applications, 1st ed.; Wiley: Hoboken, NJ, USA, 2004; ISBN 978-3-527-30779-1. [Google Scholar]
- Sinnecker, S.; Neese, F. Theoretical Bioinorganic Spectroscopy. In Atomistic Approaches in Modern Biology; Reiher, M., Ed.; Topics in Current Chemistry; Springer: Berlin/Heidelberg, Germany, 2007; Volume 268, pp. 47–83. ISBN 978-3-540-38082-5. [Google Scholar]
- Neese, F. Prediction of Molecular Properties and Molecular Spectroscopy with Density Functional Theory: From Fundamental Theory to Exchange-Coupling. Coord. Chem. Rev. 2009, 253, 526–563. [Google Scholar] [CrossRef]
- Orio, M.; Pantazis, D.A. Successes, Challenges, and Opportunities for Quantum Chemistry in Understanding Metalloenzymes for Solar Fuels Research. Chem. Commun. 2021, 57, 3952–3974. [Google Scholar] [CrossRef] [PubMed]
- Remenyi, C.; Reviakine, R.; Kaupp, M. Density Functional Study of EPR Parameters and Spin-Density Distribution of Azurin and Other Blue Copper Proteins. J. Phys. Chem. B 2007, 111, 8290–8304. [Google Scholar] [CrossRef] [PubMed]
- Sinnecker, S.; Neese, F. QM/MM Calculations with DFT for Taking into Account Protein Effects on the EPR and Optical Spectra of Metalloproteins. Plastocyanin as a Case Study. J. Comput. Chem. 2006, 27, 1463–1475. [Google Scholar] [CrossRef]
- Ames, W.M.; Larsen, S.C. DFT Calculations of the EPR Parameters for Cu(Ii) DETA Imidazole Complexes. Phys. Chem. Chem. Phys. 2009, 11, 8266. [Google Scholar] [CrossRef]
- Ames, W.M.; Larsen, S.C. Density Functional Theory Investigation of EPR Parameters for Tetragonal Cu(II) Model Complexes with Oxygen Ligands. J. Phys. Chem. A 2009, 113, 4305–4312. [Google Scholar] [CrossRef]
- Courtade, G.; Ciano, L.; Paradisi, A.; Lindley, P.J.; Forsberg, Z.; Sørlie, M.; Wimmer, R.; Davies, G.J.; Eijsink, V.G.H.; Walton, P.H.; et al. Mechanistic Basis of Substrate–O2 Coupling within a Chitin-Active Lytic Polysaccharide Monooxygenase: An Integrated NMR/EPR Study. Proc. Natl. Acad. Sci. USA 2020, 117, 19178–19189. [Google Scholar] [CrossRef]
- Bissaro, B.; Streit, B.; Isaksen, I.; Eijsink, V.G.H.; Beckham, G.T.; DuBois, J.L.; Røhr, Å.K. Molecular Mechanism of the Chitinolytic Peroxygenase Reaction. Proc. Natl. Acad. Sci. USA 2020, 117, 1504–1513. [Google Scholar] [CrossRef]
- Theibich, Y.A.; Sauer, S.P.A.; Leggio, L.L.; Hedegård, E.D. Estimating the Accuracy of Calculated Electron Paramagnetic Resonance Hyperfine Couplings for a Lytic Polysaccharide Monooxygenase. Comput. Struct. Biotechnol. J. 2021, 19, 555–567. [Google Scholar] [CrossRef]
- Singh, S.K.; Atanasov, M.; Neese, F. Challenges in Multireference Perturbation Theory for the Calculations of the g -Tensor of First-Row Transition-Metal Complexes. J. Chem. Theory Comput. 2018, 14, 4662–4677. [Google Scholar] [CrossRef] [PubMed]
- Sciortino, G.; Lubinu, G.; Maréchal, J.-D.; Garribba, E. DFT Protocol for EPR Prediction of Paramagnetic Cu(II) Complexes and Application to Protein Binding Sites. Magnetochemistry 2018, 4, 55. [Google Scholar] [CrossRef]
- Zhou, C.; Wu, D.; Gagliardi, L.; Truhlar, D.G. Calculation of the Zeeman Effect for Transition-Metal Complexes by Multiconfiguration Pair-Density Functional Theory. J. Chem. Theory Comput. 2021, 17, 5050–5063. [Google Scholar] [CrossRef] [PubMed]
- Ames, W.M.; Larsen, S.C. Insight into the Copper Coordination Environment in the Prion Protein through Density Functional Theory Calculations of EPR Parameters. J. Biol. Inorg. Chem. 2009, 14, 547–557. [Google Scholar] [CrossRef]
- Kumar, S.; Sharma, R.P.; Venugopalan, P.; Witwicki, M.; Ferretti, V. Synthesis, Characterization, Single Crystal X-Ray Structure, EPR and Theoretical Studies of a New Hybrid Inorganic-Organic Compound [Cu(Hdien)2(H2O)2](Pnb)4·4H2O and Its Structural Comparison with Related [Cu(En)2(H2O)2](Pnb)2. J. Mol. Struct. 2016, 1123, 124–132. [Google Scholar] [CrossRef]
- Atanasov, M.; Daul, C.A.; Rohmer, M.-M.; Venkatachalam, T. A DFT Based Ligand Field Study of the EPR Spectra of Co(II) and Cu(II) Porphyrins. Chem. Phys. Lett. 2006, 427, 449–454. [Google Scholar] [CrossRef][Green Version]
- Ames, W.M.; Larsen, S.C. DFT Calculations of EPR Parameters for Copper(II)-Exchanged Zeolites Using Cluster Models. J. Phys. Chem. A 2010, 114, 589–594. [Google Scholar] [CrossRef]
- Vancoillie, S.; Malmqvist, P.-Å.; Pierloot, K. Calculation of EPR g Tensors for Transition-Metal Complexes Based on Multiconfigurational Perturbation Theory (CASPT2). Chem. Phys. Chem. 2007, 8, 1803–1815. [Google Scholar] [CrossRef]
- Bolvin, H. An Alternative Approach to the G-Matrix: Theory and Applications. Chem. Eur. J. Chem. Phys. 2006, 7, 1575–1589. [Google Scholar] [CrossRef]
- Sayfutyarova, E.R.; Chan, G.K.-L. Electron Paramagnetic Resonance G-Tensors from State Interaction Spin-Orbit Coupling Density Matrix Renormalization Group. J. Chem. Phys. 2018, 148, 184103. [Google Scholar] [CrossRef]
- Sayfutyarova, E.R.; Chan, G.K.-L. A State Interaction Spin-Orbit Coupling Density Matrix Renormalization Group Method. J. Chem. Phys. 2016, 144, 234301. [Google Scholar] [CrossRef] [PubMed]
- Gómez-Piñeiro, R.J.; Pantazis, D.A.; Orio, M. Comparison of Density Functional and Correlated Wave Function Methods for the Prediction of Cu(II) Hyperfine Coupling Constants. ChemPhysChem 2020, 21, 2667–2679. [Google Scholar] [CrossRef] [PubMed]
- Mardirossian, N.; Head-Gordon, M. Thirty Years of Density Functional Theory in Computational Chemistry: An Overview and Extensive Assessment of 200 Density Functionals. Mol. Phys. 2017, 115, 2315–2372. [Google Scholar] [CrossRef]
- Perdew, J.P. Jacob’s Ladder of Density Functional Approximations for the Exchange-Correlation Energy. In AIP Conference Proceedings; AIP: Antwerp, Belgium, 2001; Volume 577, pp. 1–20. [Google Scholar] [CrossRef]
- Kaupp, M.; Reviakine, R.; Malkina, O.L.; Arbuznikov, A.; Schimmelpfennig, B.; Malkin, V.G. Calculation of Electronic G-Tensors for Transition Metal Complexes Using Hybrid Density Functionals and Atomic Meanfield Spin-Orbit Operators. J. Comput. Chem. 2002, 23, 794–803. [Google Scholar] [CrossRef] [PubMed]
- Fritscher, J.; Hrobárik, P.; Kaupp, M. Computational Studies of Electron Paramagnetic Resonance Parameters for Paramagnetic Molybdenum Complexes. 1. Method Validation on Small and Medium-Sized Systems. J. Phys. Chem. B 2007, 111, 4616–4629. [Google Scholar] [CrossRef]
- Fritscher, J.; Hrobárik, P.; Kaupp, M. Computational Studies of EPR Parameters for Paramagnetic Molybdenum Complexes. II. Larger Mo V Systems Relevant to Molybdenum Enzymes. Inorg. Chem. 2007, 46, 8146–8161. [Google Scholar] [CrossRef]
- Gohr, S.; Hrobárik, P.; Repiský, M.; Komorovský, S.; Ruud, K.; Kaupp, M. Four-Component Relativistic Density Functional Theory Calculations of EPR g—and Hyperfine-Coupling Tensors Using Hybrid Functionals: Validation on Transition-Metal Complexes with Large Tensor Anisotropies and Higher-Order Spin–Orbit Effects. J. Phys. Chem. A 2015, 119, 12892–12905. [Google Scholar] [CrossRef]
- Munzarová, M.; Kaupp, M. A Critical Validation of Density Functional and Coupled-Cluster Approaches for the Calculation of EPR Hyperfine Coupling Constants in Transition Metal Complexes. J. Phys. Chem. A 1999, 103, 9966–9983. [Google Scholar] [CrossRef]
- Schattenberg, C.J.; Maier, T.M.; Kaupp, M. Lessons from the Spin-Polarization/Spin-Contamination Dilemma of Transition-Metal Hyperfine Couplings for the Construction of Exchange-Correlation Functionals. J. Chem. Theory Comput. 2018, 14, 5653–5672. [Google Scholar] [CrossRef]
- Goerigk, L.; Grimme, S. Double-Hybrid Density Functionals: Double-Hybrid Density Functionals. WIREs Comput. Mol. Sci. 2014, 4, 576–600. [Google Scholar] [CrossRef]
- Martin, J.M.L.; Santra, G. Empirical Double-Hybrid Density Functional Theory: A ‘Third Way’ in Between WFT and DFT. Isr. J. Chem. 2020, 60, 787–804. [Google Scholar] [CrossRef]
- Grimme, S. Semiempirical Hybrid Density Functional with Perturbative Second-Order Correlation. J. Chem. Phys. 2006, 124, 034108. [Google Scholar] [CrossRef] [PubMed]
- Radoń, M. Revisiting the Role of Exact Exchange in DFT Spin-State Energetics of Transition Metal Complexes. Phys. Chem. Chem. Phys. 2014, 16, 14479–14488. [Google Scholar] [CrossRef] [PubMed]
- Pinter, B.; Chankisjijev, A.; Geerlings, P.; Harvey, J.N.; De Proft, F. Conceptual Insights into DFT Spin-State Energetics of Octahedral Transition-Metal Complexes through a Density Difference Analysis. Chem. Eur. J. 2018, 24, 5281–5292. [Google Scholar] [CrossRef]
- Cremer, D. Density Functional Theory: Coverage of Dynamic and Non-Dynamic Electron Correlation Effects. Mol. Phys. 2001, 99, 1899–1940. [Google Scholar] [CrossRef]
- Tran, V.A.; Neese, F. Double-Hybrid Density Functional Theory for g-Tensor Calculations Using Gauge Including Atomic Orbitals. J. Chem. Phys. 2020, 153, 054105. [Google Scholar] [CrossRef]
- Alipour, M. How Well Can Parametrized and Parameter-Free Double-Hybrid Approximations Predict Response Properties of Hydrogen-Bonded Systems? Dipole Polarizabilities of Water Nanoclusters as a Working Model. J. Phys. Chem. A 2013, 117, 4506–4513. [Google Scholar] [CrossRef]
- Kossmann, S.; Kirchner, B.; Neese, F. Performance of Modern Density Functional Theory for the Prediction of Hyperfine Structure: Meta-GGA and Double Hybrid Functionals. Mol. Phys. 2007, 105, 2049–2071. [Google Scholar] [CrossRef]
- Witwicki, M.; Walencik, P.K.; Jezierska, J. How Accurate Is Density Functional Theory in Predicting Spin Density? An Insight from the Prediction of Hyperfine Coupling Constants. J. Mol. Model. 2020, 26, 10. [Google Scholar] [CrossRef]
- Dittmer, A.; Stoychev, G.L.; Maganas, D.; Auer, A.A.; Neese, F. Computation of NMR Shielding Constants for Solids Using an Embedded Cluster Approach with DFT, Double-Hybrid DFT, and MP2. J. Chem. Theory Comput. 2020, 16, 6950–6967. [Google Scholar] [CrossRef]
- Scholl, H.J.; Huettermann, J. ESR and ENDOR of Copper(II) Complexes with Nitrogen Donors: Probing Parameters for Prosthetic Group Modeling of Superoxide Dismutase. J. Phys. Chem. 1992, 96, 9684–9691. [Google Scholar] [CrossRef]
- Yordanov, N.D.; Stankova, M.; Shopov, D. EPR Study of Bis(8-Quinolinethiolato) Copper(II) and Bis(8-Quinolinolato) Copper(II) Complexes. Chem. Phys. Lett. 1976, 39, 174–176. [Google Scholar] [CrossRef]
- Kuźniarska-Biernacka, I.; Kurzak, K.; Kurzak, B.; Jezierska, J. Spectrochemical Properties of Noncubical Transition Metal Complexes in Solutions. XV. Solution Properties of Bis (Salicylideneaniline)Copper(II). J. Solut. Chem. 2003, 32, 719–741. [Google Scholar] [CrossRef]
- Folli, A.; Ritterskamp, N.; Richards, E.; Platts, J.A.; Murphy, D.M. Probing the Structure of Copper(II)-Casiopeina Type Coordination Complexes [Cu(O-O)(N-N)]+ by EPR and ENDOR Spectroscopy. J. Catal. 2021, 394, 220–227. [Google Scholar] [CrossRef]
- Uçar, İ.; Bulut, A.; Büyükgüngör, O. Synthesis, Crystal Structure, EPR and Electrochemical Studies of Copper(II) Dipicolinate Complex with 2,2′-Dipyridylamine Ligand. J. Phys. Chem. Solids 2007, 68, 2271–2277. [Google Scholar] [CrossRef]
- Ritterskamp, N.; Sharples, K.; Richards, E.; Folli, A.; Chiesa, M.; Platts, J.A.; Murphy, D.M. Understanding the Coordination Modes of [Cu(Acac)2(Imidazole)n=1,2] Adducts by EPR, ENDOR, HYSCORE, and DFT Analysis. Inorg. Chem. 2017, 56, 11862–11875. [Google Scholar] [CrossRef] [PubMed]
- Ali, M.A.; Mirza, A.H.; Fereday, R.J.; Butcher, R.J.; Fuller, J.M.; Drew, S.C.; Gahan, L.R.; Hanson, G.R.; Moubaraki, B.; Murray, K.S. Synthetic, EPR Spectroscopic, Magnetic and X-Ray Crystallographic Structural Studies on Copper(II) Complexes of the Tridentate N2S Donor Ligand Formed from 6-Methyl-2-Formylpyridine and S-Methyldithiocarbazate (Hmpsme). Inorg. Chim. Acta 2005, 358, 3937–3948. [Google Scholar] [CrossRef]
- Seena, E.B.; Kurup, M.R.P. Spectral and Structural Studies of Mono- and Binuclear Copper(II) Complexes of Salicylaldehyde N(4)-Substituted Thiosemicarbazones. Polyhedron 2007, 26, 829–836. [Google Scholar] [CrossRef]
- West, D.X.; Salberg, M.M.; Bain, G.A.; Liberta, A.E.; Valdés-Martínez, J.; Hernández-Ortega, S. Binuclear Copper(II) Complexes of 5-Nitrosalicylaldehyde N(3)-Substituted Thiosemicarbazones. Transit. Met. Chem. 1996, 21, 206–212. [Google Scholar] [CrossRef]
- García-Tojal, J.; García-Orad, A.; Serra, J.L.; Pizarro, J.L.; Lezama, L.; Arriortua, M.I.; Rojo, T. Synthesis and Spectroscopic Properties of Copper(II) Complexes Derived from Thiophene-2-Carbaldehyde Thiosemicarbazone. Structure and Biological Activity of [Cu(C6H6N3S2)2]. J. Inorg. Biochem. 1999, 75, 45–54. [Google Scholar] [CrossRef]
- Bharadwaj, P.K.; Potenza, J.A.; Schugar, H.J. Characterization of [Dimethyl N,N′-Ethylenebis(L-Cysteinato)(2-)-S,S’]Copper(II), a Stable Copper(II) Aliphatic Dithiolate. J. Am. Chem. Soc. 1986, 108, 1351–1352. [Google Scholar] [CrossRef]
- Suzuki, Y.; Fujii, S.; Tominaga, T.; Yoshimoto, T.; Yoshimura, T.; Kamada, H. The Origin of an EPR Signal Observed in Dithiocarbamate-Loaded Tissues. Biochim. Biophys. Acta Gen. Subj. 1997, 1335, 242–245. [Google Scholar] [CrossRef]
- Glass, R.S.; Steffen, L.K.; Swanson, D.D.; Wilson, G.S.; de Gelder, R.; de Graaff, R.A.G.; Reedijk, J. Bis(Trithiacyclononane)Metal(II) Compounds and Jahn-Teller Distortions from Octahedral Geometry, Electrochemistry, Spectroscopy, and Crystal Structures of the Copper Bis(Tetrafluoroborate) Bis(Acetonitrile) Complex at 177 K and the Cadmium Bis(Tetrafluoroborate) and Copper Bis(Tetrafluoroborate) Bis(Nitromethane) Complexes at 300 K. Inorg. Chim. Acta 1993, 207, 241–252. [Google Scholar] [CrossRef]
- Schwabe, T.; Grimme, S. Towards Chemical Accuracy for the Thermodynamics of Large Molecules: New Hybrid Density Functionals Including Non-Local Correlation Effects. Phys. Chem. Chem. Phys. 2006, 8, 4398. [Google Scholar] [CrossRef]
- Karton, A.; Tarnopolsky, A.; Lamère, J.-F.; Schatz, G.C.; Martin, J.M.L. Highly Accurate First-Principles Benchmark Data Sets for the Parametrization and Validation of Density Functional and Other Approximate Methods. Derivation of a Robust, Generally Applicable, Double-Hybrid Functional for Thermochemistry and Thermochemical Kinetics. J. Phys. Chem. A 2008, 112, 12868–12886. [Google Scholar] [CrossRef]
- Tarnopolsky, A.; Karton, A.; Sertchook, R.; Vuzman, D.; Martin, J.M.L. Double-Hybrid Functionals for Thermochemical Kinetics. J. Phys. Chem. A 2008, 112, 3–8. [Google Scholar] [CrossRef]
- Brémond, É.; Sancho-García, J.C.; Pérez-Jiménez, Á.J.; Adamo, C. Communication: Double-Hybrid Functionals from Adiabatic-Connection: The QIDH Model. J. Chem. Phys. 2014, 141, 031101. [Google Scholar] [CrossRef]
- Brémond, E.; Adamo, C. Seeking for Parameter-Free Double-Hybrid Functionals: The PBE0-DH Model. J. Chem. Phys. 2011, 135, 024106. [Google Scholar] [CrossRef]
- Brémond, É.; Savarese, M.; Pérez-Jiménez, Á.J.; Sancho-García, J.C.; Adamo, C. Range-Separated Double-Hybrid Functional from Nonempirical Constraints. J. Chem. Theory Comput. 2018, 14, 4052–4062. [Google Scholar] [CrossRef]
- Brémond, É.; Pérez-Jiménez, Á.J.; Sancho-García, J.C.; Adamo, C. Range-Separated Hybrid Density Functionals Made Simple. J. Chem. Phys. 2019, 150, 201102. [Google Scholar] [CrossRef]
- Kozuch, S.; Gruzman, D.; Martin, J.M.L. DSD-BLYP: A General Purpose Double Hybrid Density Functional Including Spin Component Scaling and Dispersion Correction. J. Phys. Chem. C 2010, 114, 20801–20808. [Google Scholar] [CrossRef]
- Kozuch, S.; Martin, J.M.L. DSD-PBEP86: In Search of the Best Double-Hybrid DFT with Spin-Component Scaled MP2 and Dispersion Corrections. Phys. Chem. Chem. Phys. 2011, 13, 20104. [Google Scholar] [CrossRef] [PubMed]
- Iikura, H.; Tsuneda, T.; Yanai, T.; Hirao, K. A Long-Range Correction Scheme for Generalized-Gradient-Approximation Exchange Functionals. J. Chem. Phys. 2001, 115, 3540–3544. [Google Scholar] [CrossRef]
- Yanai, T.; Tew, D.P.; Handy, N.C. A New Hybrid Exchange–Correlation Functional Using the Coulomb-Attenuating Method (CAM-B3LYP). Chem. Phys. Lett. 2004, 393, 51–57. [Google Scholar] [CrossRef]
- Chai, J.-D.; Head-Gordon, M. Long-Range Corrected Hybrid Density Functionals with Damped Atom–Atom Dispersion Corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615. [Google Scholar] [CrossRef]
- Casanova-Páez, M.; Dardis, M.B.; Goerigk, L. ΩB2PLYP and ΩB2GPPLYP: The First Two Double-Hybrid Density Functionals with Long-Range Correction Optimized for Excitation Energies. J. Chem. Theory Comput. 2019, 15, 4735–4744. [Google Scholar] [CrossRef]
- Casanova-Páez, M.; Goerigk, L. Time-Dependent Long-Range-Corrected Double-Hybrid Density Functionals with Spin-Component and Spin-Opposite Scaling: A Comprehensive Analysis of Singlet–Singlet and Singlet–Triplet Excitation Energies. J. Chem. Theory Comput. 2021, 17, 5165–5186. [Google Scholar] [CrossRef]
- Chai, J.-D.; Head-Gordon, M. Long-Range Corrected Double-Hybrid Density Functionals. J. Chem. Phys. 2009, 131, 174105. [Google Scholar] [CrossRef]
- Medvedev, M.G.; Bushmarinov, I.S.; Sun, J.; Perdew, J.P.; Lyssenko, K.A. Density Functional Theory Is Straying from the Path toward the Exact Functional. Science 2017, 355, 49–52. [Google Scholar] [CrossRef]
- Kepp, K.P. Comment on “Density Functional Theory Is Straying from the Path toward the Exact Functional”. Science 2017, 356, 496. [Google Scholar] [CrossRef]
- Savarese, M.; Brémond, É.; Ciofini, I.; Adamo, C. Electron Spin Densities and Density Functional Approximations: Open-Shell Polycyclic Aromatic Hydrocarbons as Case Study. J. Chem. Theory Comput. 2020, 16, 3567–3577. [Google Scholar] [CrossRef] [PubMed]
- Sharkas, K.; Toulouse, J.; Savin, A. Double-Hybrid Density-Functional Theory Made Rigorous. J. Chem. Phys. 2011, 134, 064113. [Google Scholar] [CrossRef] [PubMed]
- Neese, F.; Wennmohs, F.; Becker, U.; Riplinger, C. The ORCA Quantum Chemistry Program Package. J. Chem. Phys. 2020, 152, 224108. [Google Scholar] [CrossRef]
- Becke, A.D. Density-Functional Exchange-Energy Approximation with Correct Asymptotic Behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron Density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Becke, A.D. A New Mixing of Hartree–Fock and Local Density-functional Theories. J. Chem. Phys. 1993, 98, 1372–1377. [Google Scholar] [CrossRef]
- Groom, C.R.; Bruno, I.J.; Lightfoot, M.P.; Ward, S.C. The Cambridge Structural Database. Acta Crystallogr. B Struct. Sci. Cryst. Eng. Mater. 2016, 72, 171–179. [Google Scholar] [CrossRef]
- Van Lenthe, E.; Baerends, E.J.; Snijders, J.G. Relativistic Regular Two-component Hamiltonians. J. Chem. Phys. 1993, 99, 4597–4610. [Google Scholar] [CrossRef]
- van Lenthe, E.; Baerends, E.J.; Snijders, J.G. Relativistic Total Energy Using Regular Approximations. J. Chem. Phys. 1994, 101, 9783–9792. [Google Scholar] [CrossRef]
- Neese, F. An Improvement of the Resolution of the Identity Approximation for the Formation of the Coulomb Matrix. J. Comput. Chem. 2003, 24, 1740–1747. [Google Scholar] [CrossRef]
- Neese, F.; Wennmohs, F.; Hansen, A.; Becker, U. Efficient, Approximate and Parallel Hartree–Fock and Hybrid DFT Calculations. A ‘Chain-of-Spheres’ Algorithm for the Hartree–Fock Exchange. Chem. Phys. 2009, 356, 98–109. [Google Scholar] [CrossRef]
- Pantazis, D.A.; Chen, X.-Y.; Landis, C.R.; Neese, F. All-Electron Scalar Relativistic Basis Sets for Third-Row Transition Metal Atoms. J. Chem. Theory Comput. 2008, 4, 908–919. [Google Scholar] [CrossRef] [PubMed]
- Weigend, F.; Ahlrichs, R. Balanced Basis Sets of Split Valence, Triple Zeta Valence and Quadruple Zeta Valence Quality for H to Rn: Design and Assessment of Accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297. [Google Scholar] [CrossRef] [PubMed]
- Weigend, F. Accurate Coulomb-Fitting Basis Sets for H to Rn. Phys. Chem. Chem. Phys. 2006, 8, 1057. [Google Scholar] [CrossRef] [PubMed]
- Adamo, C.; Barone, V. Toward Reliable Density Functional Methods without Adjustable Parameters: The PBE0 Model. J. Chem. Phys. 1999, 110, 6158–6170. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Heß, B.A.; Marian, C.M.; Wahlgren, U.; Gropen, O. A Mean-Field Spin-Orbit Method Applicable to Correlated Wavefunctions. Chem. Phys. Lett. 1996, 251, 365–371. [Google Scholar] [CrossRef]
- Neese, F. Efficient and Accurate Approximations to the Molecular Spin-Orbit Coupling Operator and Their Use in Molecular g-Tensor Calculations. J. Chem. Phys. 2005, 122, 034107. [Google Scholar] [CrossRef]
- Neese, F. Calculation of the Zero-Field Splitting Tensor on the Basis of Hybrid Density Functional and Hartree-Fock Theory. J. Chem. Phys. 2007, 127, 164112. [Google Scholar] [CrossRef]

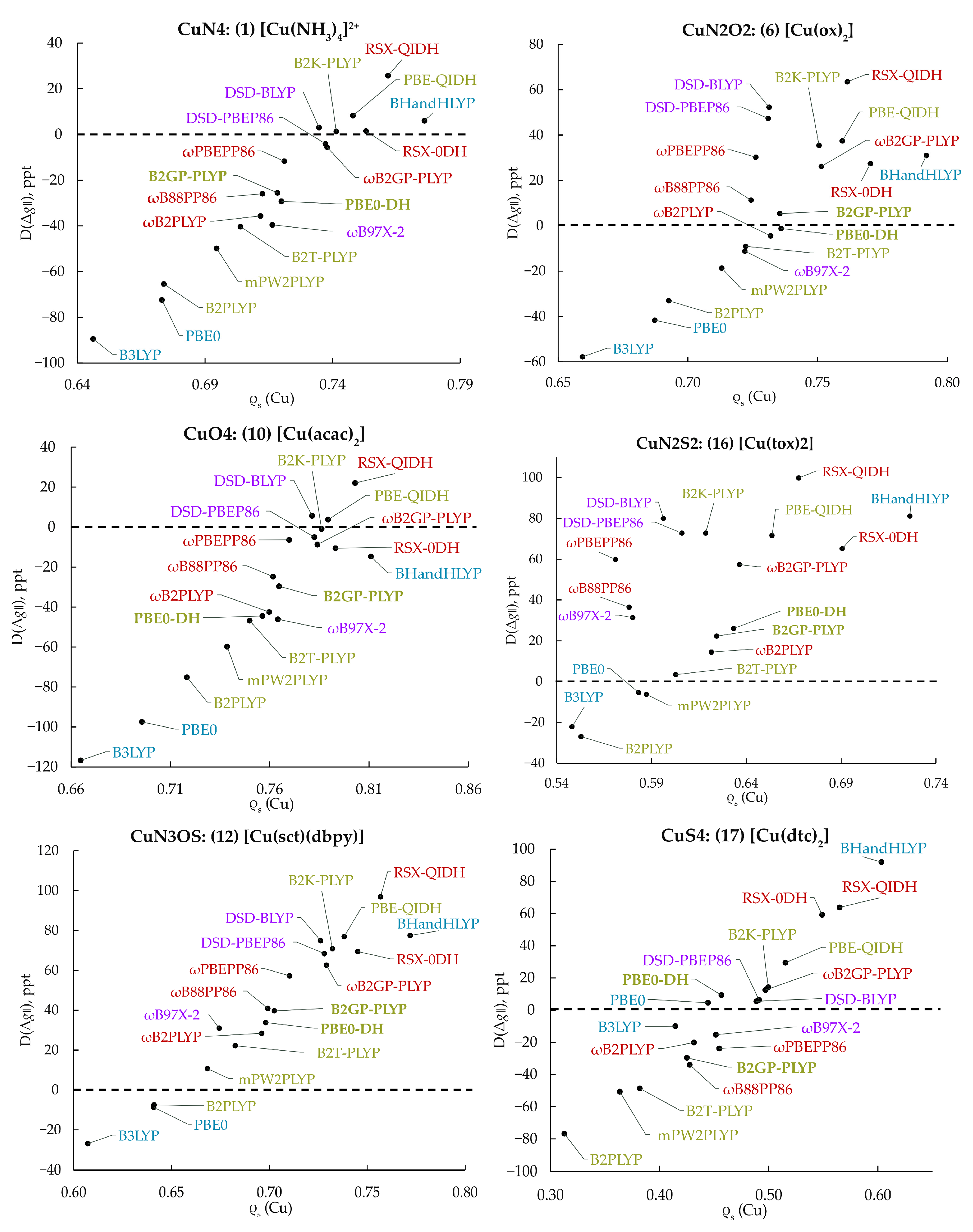
| Complex | Coord. Core | Ref. | ||||
|---|---|---|---|---|---|---|
| 1 | [Cu(NH3)4]2+ | N4 | 45 | 45 | 239 | [56] |
| 2 | [Cu(py)4]2+ | 51 | 51 | 261 | [56] | |
| 3 | [Cu(iz)4]2+ | 45 | 45 | 260 | [56] | |
| 4 | [Cu(en)2]2+ | 39 | 39 | 203 | [56] | |
| 5 | [Cu(gly)2] | N2O2 | 50 | 50 | 265 | [56] |
| 6 | [Cu(ox)2] | 50 | 50 | 200 | [57] | |
| 7 | [Cu(sac)2] | 48 | 48 | 238 | [58] | |
| 8 | [Cu(acac)(bpy)]+ | 48 | 55 | 251 | [59] | |
| 9 | [Cu(dpa)(dpc)] | N3O2 | 60 | 60 | 252 | [60] |
| 10 | [Cu(acac)2] | O4 | 58 | 58 | 283 | [61] |
| 11 | [Cu(mpsme)(NCS)] | N3S | 48 | 48 | 203 | [62] |
| 12 | [Cu(sct)(dbpy)] | N3OS | 54 | 54 | 165 | [63] |
| 13 | [Cu(spt)(DMF)] | NO2S | 35 | 46 | 201 | [64] |
| 14 | [Cu(tct)2] | N2S2 | 27 | 88 | 112 | [65] |
| 15 | [Cu(eLcys)] | 37 | 37 | 124 | [66] | |
| 16 | [Cu(tox)2] | 42 | 42 | 136 | [57] | |
| 17 | [Cu(dtc)2] | S4 | 23 | 23 | 83 | [67] |
| 18 | [Cu(ttcn)2]2+ | S8 | 25 | 25 | 112 | [68] |
| Functional | ||||||
|---|---|---|---|---|---|---|
| B2PLYP | 0.53 | - | 0.73 | 0.27 | - | - |
| mPW2PLYP | 0.55 | - | 0.75 | 0.25 | - | - |
| B2GP-PLYP | 0.65 | - | 0.64 | 0.36 | - | - |
| B2K-PLYP | 0.72 | - | 0.58 | 0.42 | - | - |
| B2T-PLYP | 0.60 | - | 0.69 | 0.31 | - | - |
| PBE-QIDH | 0.69 | - | 0.67 | 0.33 | - | - |
| PBE0-DH | 0.50 | - | 0.875 | 0.125 | - | - |
| DSD-BLYP | 0.75 | - | 0.53 | - | 0.46 | 0.60 |
| DSD-PBEP86 | 0.72 | - | 0.44 | - | 0.51 | 0.36 |
| ωB2PLYP | 0.53 | 0.30 | 0.73 | 0.27 | - | - |
| ωB2GP-PLYP | 0.65 | 0.27 | 0.64 | 0.36 | - | - |
| RSX-QIDH | 0.69 | 0.27 | 0.67 | 0.33 | - | - |
| RSX-0DH | 0.50 | 0.33 | 0.875 | 0.125 | - | - |
| ωB88PP86 | 0.65 | 0.20 | 0.58 | 0.42 | - | - |
| ωPBEPP86 | 0.70 | 0.18 | 0.68 | 0.48 | - | - |
| ωB97X-2 | 0.63(6) | 0.30 | 1.00 | 1.00 | 0.44(7) | 0.52(9) |
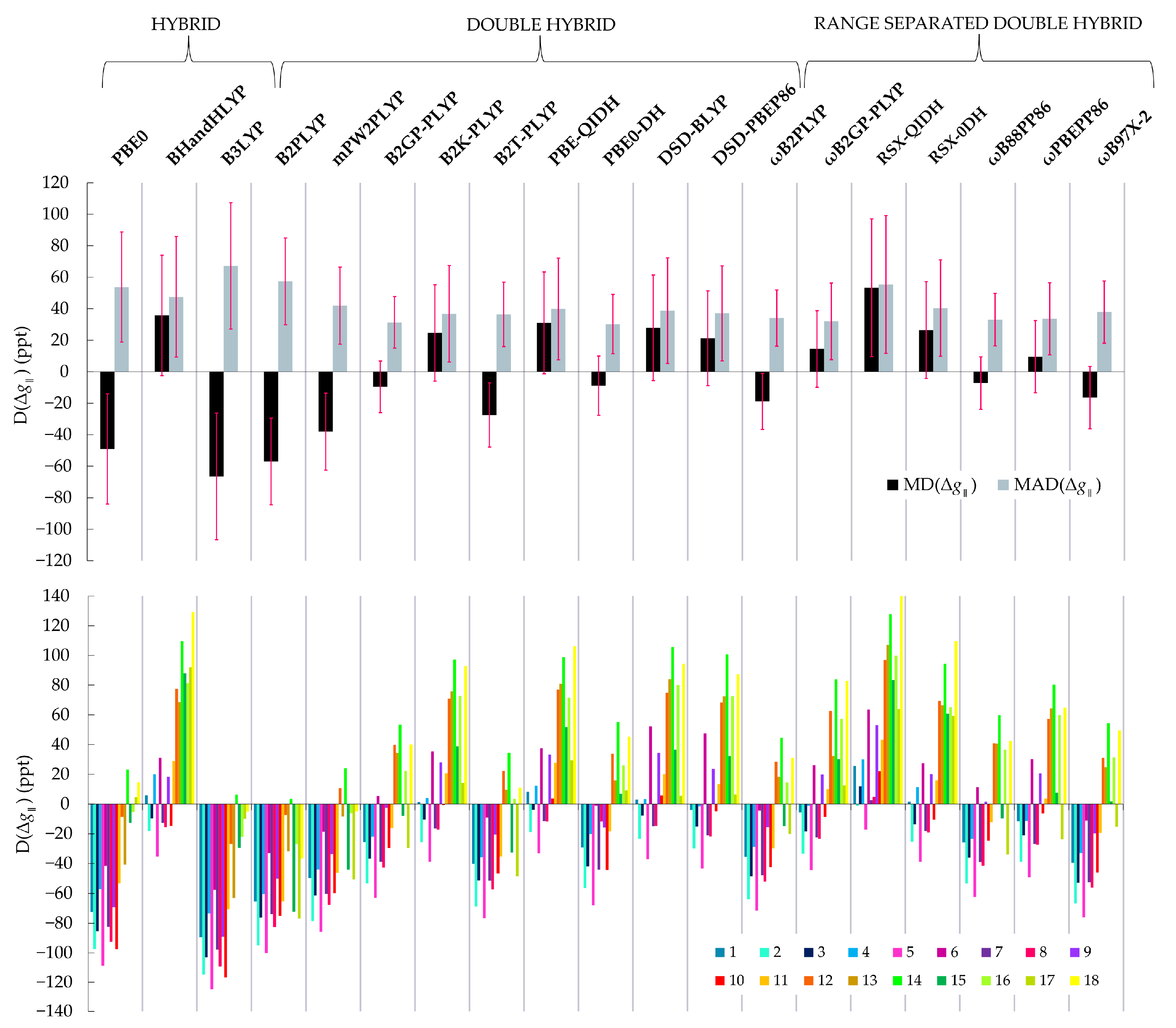
| Functional | MD | SDD | MAD | MAD | SDAD | MAPD | MAPD |
|---|---|---|---|---|---|---|---|
| PBE0 | −49 | 41 | 6 | 54 | 35 | 14 | 24 |
| BHandHLYP | 36 | 49 | 19 | 48 | 38 | 49 | 35 |
| B3LYP | −66 | 41 | 9 | 67 | 40 | 19 | 30 |
| B2PLYP | −57 | 28 | 12 | 57 | 28 | 31 | 30 |
| mPW2PLYP | −38 | 30 | 8 | 42 | 24 | 19 | 21 |
| B2GP-PLYP | −10 | 34 | 7 | 31 | 16 | 18 | 18 |
| B2K-PLYP | 25 | 41 | 8 | 37 | 31 | 21 | 24 |
| B2T-PLYP | −27 | 31 | 7 | 36 | 20 | 19 | 19 |
| PBE-QIDH | 31 | 41 | 10 | 40 | 32 | 25 | 27 |
| PBE0-DH | −9 | 35 | 6 | 31 | 19 | 15 | 17 |
| DSD-BLYP | 28 | 43 | 8 | 39 | 34 | 21 | 25 |
| DSD-PBEP86 | 21 | 43 | 7 | 37 | 30 | 20 | 24 |
| ωB2PLYP | −19 | 33 | 6 | 34 | 18 | 17 | 18 |
| ωB2GP-PLYP | 14 | 37 | 7 | 32 | 24 | 19 | 21 |
| RSX-QIDH | 53 | 46 | 15 | 55 | 44 | 37 | 39 |
| RSX-0DH | 26 | 43 | 14 | 40 | 31 | 35 | 28 |
| ωB88PP86 | −7 | 36 | 7 | 33 | 17 | 19 | 19 |
| ωPBEPP86 | 10 | 40 | 7 | 34 | 23 | 20 | 21 |
| ωB97X-2 | −16 | 39 | 7 | 38 | 20 | 19 | 20 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Drosou, M.; Mitsopoulou, C.A.; Orio, M.; Pantazis, D.A. EPR Spectroscopy of Cu(II) Complexes: Prediction of g-Tensors Using Double-Hybrid Density Functional Theory. Magnetochemistry 2022, 8, 36. https://doi.org/10.3390/magnetochemistry8040036
Drosou M, Mitsopoulou CA, Orio M, Pantazis DA. EPR Spectroscopy of Cu(II) Complexes: Prediction of g-Tensors Using Double-Hybrid Density Functional Theory. Magnetochemistry. 2022; 8(4):36. https://doi.org/10.3390/magnetochemistry8040036
Chicago/Turabian StyleDrosou, Maria, Christiana A. Mitsopoulou, Maylis Orio, and Dimitrios A. Pantazis. 2022. "EPR Spectroscopy of Cu(II) Complexes: Prediction of g-Tensors Using Double-Hybrid Density Functional Theory" Magnetochemistry 8, no. 4: 36. https://doi.org/10.3390/magnetochemistry8040036
APA StyleDrosou, M., Mitsopoulou, C. A., Orio, M., & Pantazis, D. A. (2022). EPR Spectroscopy of Cu(II) Complexes: Prediction of g-Tensors Using Double-Hybrid Density Functional Theory. Magnetochemistry, 8(4), 36. https://doi.org/10.3390/magnetochemistry8040036








