Modeling of the Particle Build-Up Evolution on a Single-Wire Magnetic Capture from Axial Stream Flow
Abstract
1. Introduction
- (1)
- Determination of the effective length that this particle can capture on the magnetized wire, based on the motion equation of the particle around the wire.
- (2)
- Establishment and solution of the kinetic equation of the build-up profile, which determines the evolution of the particles captured and accumulated on the wire.
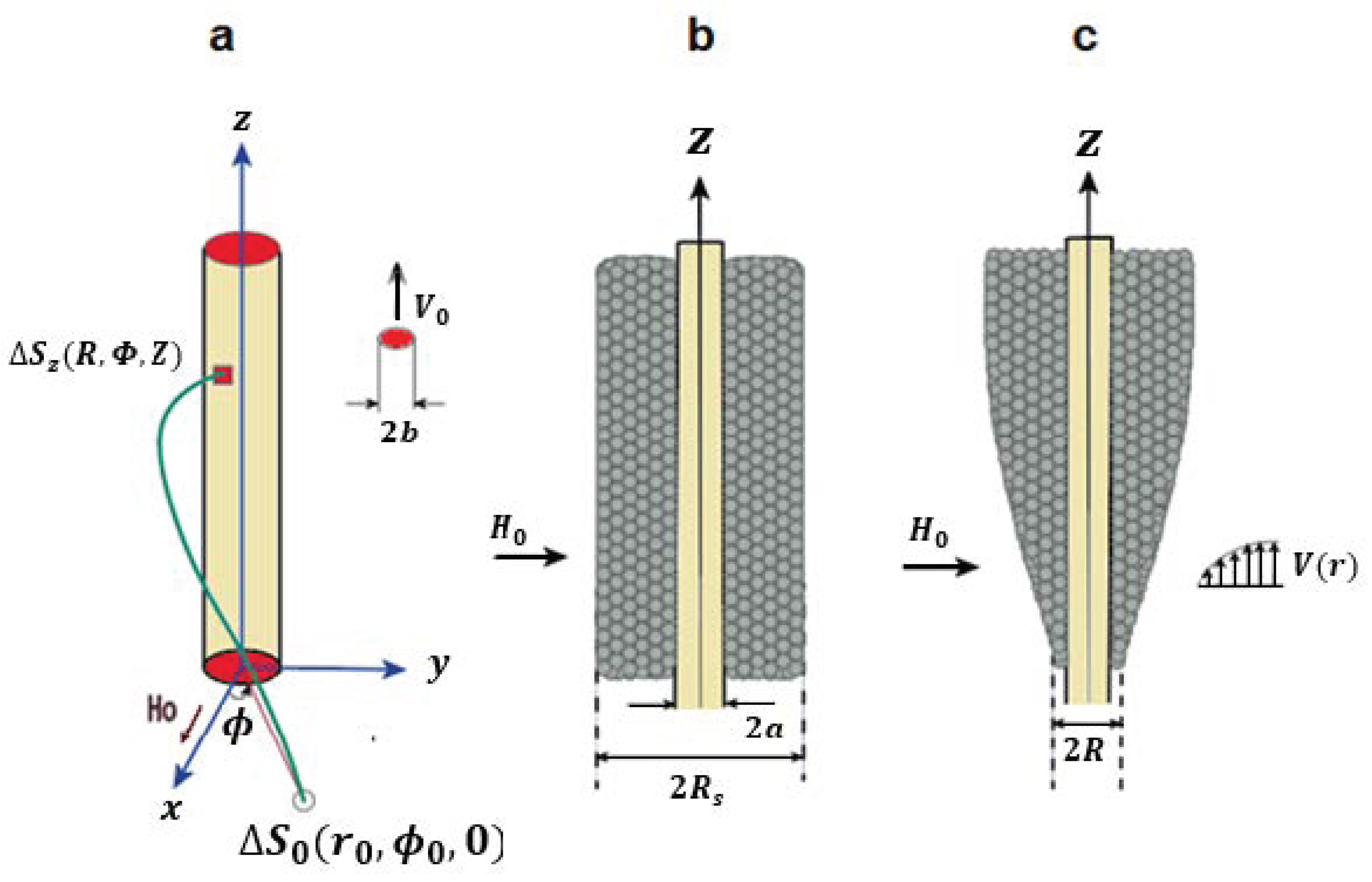
2. Formulation of the Problem
- i.
- Determining the HGMS performance by defining the area of the particle’s capture region (active region) or the capture radius on the magnetized ferromagnetic wire.
- ii.
- Determining the capture saturation radius and HGMS performance from the initial accumulation profile of the particles deposited on the magnetized wire.
- (a)
- The initial accumulation of particles captured on the magnetized wire occurs in regions of the highest magnetic field gradient (). The accumulation of particles in these regions of the magnetized wire is predominantly aggregation during the initial phase of deposition processes. Deposition of the captured particles on the wire remains predominant until the saturation radius () is formed. During this process, if the particle captured in the active region breaks for any reason (turbulence, drag, etc.), this particle can be drifting and captured in another position on the wire, or the breaking events of the captured particles are very weak.
- (b)
- Detachment and divergence events of the particles are more dominant at the level of the saturation profile of the particles formed on the magnetized wire and in the region above it (). This is a critical point for HGMS performance, after which the performance becomes minimal or negligible. The time required for this condition to occur is also expressed as the safe operating time of the separator.
- (a)
- The solution model of the differential equation expressing both aggregation and breaking away of particles captured in the active region. This model is generally constructed from nonlinear integro-differential equations based on population balance [70].
- (b)
- The solution model with the Fokker–Planck equation as a stochastic event observed with both capture and detachment processes at the same time in turbulent flow of particles captured in the active region.
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Watson, J.H. Magnetic filtration. J. Appl. Phys. 1973, 44, 4209–4213. [Google Scholar] [CrossRef]
- Oberteuffer, J. Magnetic separation: A review of principles, devices, and applications. IEEE Trans. Magn. 1974, 10, 223–238. [Google Scholar] [CrossRef]
- Oder, R. High gradient magnetic separation theory and applications. IEEE Trans. Magn. 1976, 12, 428–435. [Google Scholar] [CrossRef]
- Gerber, R.; Birss, R.R. High Gradient Magnetic Separation; Research Studies Press Division of John Wiley & Sons, Ltd.: London, UK, 1983; p. 209. [Google Scholar]
- Karmazin, V.; Karmazin, V. Magnetic Methods of beneficiation; Nedra Publishing: Moscow, Russia, 1984. [Google Scholar]
- Svoboda, J. Magnetic Techniques for the Treatment of Materials; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Kelland, D. High gradient magnetic separation applied to mineral beneficiation. IEEE Trans. Magn. 1973, 9, 307–310. [Google Scholar] [CrossRef]
- Parker, M.R. The physics of magnetic separation. Contemp. Phys. 1977, 18, 279–306. [Google Scholar] [CrossRef]
- Kolm, H.; Oberteuffer, J.; Kelland, D. High-gradient magnetic separation. Sci. Am. 1975, 233, 46–55. [Google Scholar] [CrossRef]
- Luborsky, F.; Drummond, B. High gradient magnetic separation: Theory versus experiment. IEEE Trans. Magn. 1975, 11, 1696–1700. [Google Scholar] [CrossRef]
- Yavuz, C.T.; Prakash, A.; Mayo, J.; Colvin, V.L. Magnetic separations: From steel plants to biotechnology. Chem. Eng. Sci. 2009, 64, 2510–2521. [Google Scholar] [CrossRef]
- Lindner, J.; Nirschl, H. A hybrid method for combining High-Gradient Magnetic Separation and centrifugation for a continuous process. Sep. Purif. Technol. 2014, 131, 27–34. [Google Scholar] [CrossRef]
- Gómez-Pastora, J.; Xue, X.; Karampelas, I.H.; Bringas, E.; Furlani, E.P.; Ortiz, I. Analysis of separators for magnetic beads recovery: From large systems to multifunctional microdevices. Sep. Purif. Technol. 2017, 172, 16–31. [Google Scholar] [CrossRef]
- Kefeni, K.K.; Mamba, B.B.; Msagati, T.A. Application of spinel ferrite nanoparticles in water and wastewater treatment: A review. Sep. Purif. Technol. 2017, 188, 399–422. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, C.; Wei, W. Industry applications of magnetic separation based on nanoparticles: A review. Int. J. Appl. Electromagn. Mech. 2019, 60, 281–297. [Google Scholar] [CrossRef]
- Melville, D.; Paul, F.; Roath, S. Direct magnetic separation of red cells from whole blood. Nature 1975, 255, 706. [Google Scholar] [CrossRef]
- Paul, F.; Melville, D.; Roath, S.; Warhurst, D. A bench top magnetic separator for malarial parasite concentration. IEEE Trans. Magn. 1981, 17, 2822–2824. [Google Scholar] [CrossRef]
- Zborowski, M.; Chalmers, J.J. Magnetic Cell Separation; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Häfeli, U.; Schütt, W.; Teller, J.; Zborowski, M. Scientific and Clinical Applications of Magnetic Carriers; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Šafařík, I.; Šafaříková, M. Use of magnetic techniques for the isolation of cells. J. Chromatogr. B Biomed. Sci. Appl. 1999, 722, 33–53. [Google Scholar] [CrossRef]
- Setchell, C.H. Magnetic separations in biotechnology—A review. J. Chem. Technol. Biotechnol. 1985, 35, 175–182. [Google Scholar] [CrossRef]
- Leong, S.S.; Yeap, S.P.; Lim, J. Working principle and application of magnetic separation for biomedical diagnostic at high-and low-field gradients. Interface Focus 2016, 6, 20160048. [Google Scholar] [CrossRef] [PubMed]
- Kelland, D.R. Magnetic separation of nanoparticles. IEEE Trans. Magn. 1998, 34, 2123–2125. [Google Scholar] [CrossRef]
- He, J.; Huang, M.; Wang, D.; Zhang, Z.; Li, G. Magnetic separation techniques in sample preparation for biological analysis: A review. J. Pharm. Biomed. Anal. 2014, 101, 84–101. [Google Scholar] [CrossRef]
- David, A.E.; Cole, A.J.; Chertok, B.; Park, Y.S.; Yang, V.C. A combined theoretical and in vitro modeling approach for predicting the magnetic capture and retention of magnetic nanoparticles in vivo. J. Control. Release 2011, 152, 67–75. [Google Scholar] [CrossRef]
- Abbasov, T. Some Actual Problems of Magnetic Filters With Magnetized Packed Beds and Comparative Analysis of Filter Performances. IEEE Trans. Magn. 2019, 55, 1–12. [Google Scholar] [CrossRef]
- Birss, R.; Gerber, R.; Parker, M.; Sheerer, T. Theory and performance of axial magnetic filters in laminar flow conditions. IEEE Trans. Magn. 1978, 14, 389–391. [Google Scholar] [CrossRef]
- Birss, R.; Gerber, R.; Parker, M. Theory and design of axially ordered filters for high intensity magnetic separation. IEEE Trans. Magn. 1976, 12, 892–894. [Google Scholar] [CrossRef]
- Gerber, R. Theory of particle capture in axial filters for high gradient magnetic separation. J. Phys. D Appl. Phys. 1978, 11, 2119. [Google Scholar] [CrossRef]
- Uchiyama, S.; Takayasu, M.; Kurinobu, S. Performance of Parallel Stream Type Magnetic Filter for High Gradient Magnetic Separation. Trans. Inst. Electr. Eng. Jpn. B 1977, 97, 459–466. [Google Scholar] [CrossRef]
- Uchiyama, S.; Kondo, S.; Takayasu, M.; Eguchi, I. Performance of parallel stream type magnetic filter for HGMS. IEEE Trans. Magn. 1976, 12, 895–897. [Google Scholar] [CrossRef]
- Uchiyama, S.; Kurinobu, S.; Kumazawa, M.; Takayasu, M. Magnetic particle buildup processes in parallel stream type HGMS filter. IEEE Trans. Magn. 1977, 13, 1490–1492. [Google Scholar] [CrossRef]
- Zheng, X.; Xue, Z.; Wang, Y.; Zhu, G.; Lu, D.; Li, X. Modeling of particle capture in high gradient magnetic separation: A review. Powder Technol. 2019, 352, 159–169. [Google Scholar] [CrossRef]
- Friedlaender, F.; Takayasu, M.; Rettig, J.; Kentzer, C. Particle flow and collection process in single wire HGMS studies. IEEE Trans. Magn. 1978, 14, 1158–1164. [Google Scholar] [CrossRef]
- Friedlaender, F.; Takayasu, M. A study of the mechanisms of particle buildup on single ferromagnetic wires and spheres. IEEE Trans. Magn. 1982, 18, 817–821. [Google Scholar] [CrossRef]
- Friedlaender, F.; Takayasu, M.; Rettig, J.; Kentzer, C. Studies of single wire parallel stream type HGMS. IEEE Trans. Magn. 1978, 14, 404–406. [Google Scholar] [CrossRef]
- Hoffmann, H.; Neudel, M.; Schewe, H.; Reffle, J. Trajectories of para-and freeomagnetic particles in HGMS filters with axial configurations. Appl. Phys. 1981, 24, 229–232. [Google Scholar] [CrossRef]
- Badescu, V.; Murariu, V.; Rotariu, O.; Rezlescu, N. A new modeling of the initial buildup evolution on a wire in an axial HGMF filter. J. Magn. Magn. Mater. 1996, 163, 225–231. [Google Scholar] [CrossRef]
- Wang, Y.; Xue, Z.; Zheng, X.; Lu, D.; Li, X.; Chu, H. Study on favorable matrix aspect ratio for maximum particle capture in axial high gradient magnetic separation. Miner. Eng. 2019, 135, 48–54. [Google Scholar] [CrossRef]
- Gurevich, A.; Beklemisheva, A.V.; Levada, E.; Rodionova, V.; Panina, L.V. Ferromagnetic Microwire Systems as a High-Gradient Magnetic Field Source for Magnetophoresis. IEEE Magn. Lett. 2020, 11, 1–5. [Google Scholar] [CrossRef]
- Rezlescu, N.; Murariu, V.; Rotariu, O.; Badescu, V. Capture modeling for an axial high gradient magnetic separation filter with a bounded flow field. Powder Technol. 1995, 83, 259–264. [Google Scholar] [CrossRef]
- Xue, Z.; Wang, Y.; Zheng, X.; Lu, D.; Li, X. Particle capture of special cross-section matrices in axial high gradient magnetic separation: A 3D simulation. Sep. Purif. Technol. 2020, 237, 116375. [Google Scholar] [CrossRef]
- Zheng, X.; Wang, Y.; Lu, D. Particle capture of elliptic cross-section matrices for parallel stream high gradient magnetic separation. COMPEL Int. J. Comput. Math. Electr. Electron. Eng. 2016, 35, 187–199. [Google Scholar] [CrossRef]
- Zheng, X.; Wang, Y.; Lu, D.; Li, X.; Li, S.; Chu, H. Comparative study on the performance of circular and elliptic cross-section matrices in axial high gradient magnetic separation: Role of the applied magnetic induction. Miner. Eng. 2017, 110, 12–19. [Google Scholar] [CrossRef]
- Kramer, A.; Janssen, J.; Perenboom, J. Single-wire HGMS of colloidal particles: The evolution of concentration profiles. IEEE Trans. Magn. 1990, 26, 1858–1860. [Google Scholar] [CrossRef][Green Version]
- Takayasu, M.; Gerber, R.; Friedlaender, F. The collection of strongly magnetic particles in HGMS. J. Magn. Magn. Mater. 1983, 40, 204–214. [Google Scholar] [CrossRef]
- Abbasov, T.; Herdem, S.; Köksal, M. Particle capture in axial magnetic filters with power law flow model. J. Phys. D Appl. Phys. 1999, 32, 1097. [Google Scholar] [CrossRef]
- Köksal, M.; Abbasov, T.; Herdem, S. Mathematical modeling of the magnetic filtration processes. Int. J. Appl. Electromagn. Mech. 2003, 18, 227–234. [Google Scholar] [CrossRef]
- Hournkumnuard, K.; Natenapit, M. Diffusive capture of magnetic particles by an assemblage of random cylindrical collectors. Sep. Sci. Technol. 2008, 43, 3448–3460. [Google Scholar] [CrossRef]
- Hournkumnuard, K.; Natenapit, M. The capture of micro-particles by random cylindrical wires in axial magnetic filters. Sep. Sci. Technol. 2013, 48, 2234–2242. [Google Scholar] [CrossRef]
- Choomphon-anomakhun, N.; Ebner, A.D.; Natenapit, M.; Ritter, J.A. Simulation of dynamic magnetic particle capture and accumulation around a ferromagnetic wire. J. Magn. Magn. Mater. 2017, 428, 493–505. [Google Scholar] [CrossRef]
- Abbasov, T.; Gögebakan, V.; Karadağ, T. Particle capture modeling for an axial magnetic filter with a bounded non-Newtonian flow field. Powder Technol. 2016, 291, 223–228. [Google Scholar] [CrossRef]
- Schroen, K.; van Dinther, A.; Stockmann, R. Particle migration in laminar shear fields: A new basis for large scale separation technology? Sep. Purif. Technol. 2017, 174, 372–388. [Google Scholar] [CrossRef]
- Abbasov, T.; Bilgili, H.; Sarımeşeli Paçacı, A. Quasi-Newtonian Approach determination of velocity profile for the fully developed axial power law fluid flow in concentric annuli. Asia-Pac. J. Chem. Eng. 2021, 16, e2710. [Google Scholar] [CrossRef]
- Bilgili, H.; Abbasov, T. An Overview of Blood Analogues Used in Bioengineering (in Turkish). J. Inonu Univ. Health Serv. Vocat. Sch. 2020, 8, 946–963. [Google Scholar]
- Paul, F.; Melville, D.; Roath, S. Inviscid approximation trajectories in high gradient magnetic separation. IEEE Trans. Magn. 1982, 18, 792–795. [Google Scholar] [CrossRef]
- Gerber, R.; Takayasu, M.; Friedlaender, F. Generalization of HGMS theory: The capture of ultra-fine particles. IEEE Trans. Magn. 1983, 19, 2115–2117. [Google Scholar] [CrossRef]
- Gerber, R. Magnetic filtration of ultra-fine particles. IEEE Trans. Magn. 1984, 20, 1159–1164. [Google Scholar] [CrossRef]
- Takayasu, M.; Gerber, R.; Friedlaender, F. Magnetic separation of submicron particles. IEEE Trans. Magn. 1983, 19, 2112–2114. [Google Scholar] [CrossRef]
- Fletcher, D. Fine particle high gradient magnetic entrapment. IEEE Trans. Magn. 1991, 27, 3655–3677. [Google Scholar] [CrossRef]
- Yaniv, I.; Lin, I.J.; Zimmels, Y. Hydrostatic levitation of particles in electric and magnetic fields. Sep. Sci. Technol. 1979, 14, 557–570. [Google Scholar] [CrossRef]
- Luborsky, F.; Drummond, B. Buildup of particles on fibers in a high field-high gradient separator. IEEE Trans. Magn. 1976, 12, 463–465. [Google Scholar] [CrossRef]
- Murariu, V.; Rezlescu, N.; Rotariu, O.; Badescu, V. Concentration influences on recovery in a high gradient magnetic separation axial filter. IEEE Trans. Magn. 1998, 34, 695–699. [Google Scholar] [CrossRef]
- Zheng, X.; Wang, Y.; Lu, D. Study on buildup of fine weakly magnetic minerals on matrices in high gradient magnetic separation. Physicochem. Probl. Miner. Processing 2017, 53, 94–109. [Google Scholar]
- Murariu, V.; Rezlescu, N.; Rotariu, O.; Badescu, V. The effect of suspension concentration on the buildup evolution in a HGMF-axial magnetic filter. Eur. Phys. J. Appl. Phys. 1998, 1, 39–44. [Google Scholar] [CrossRef]
- Sobecki, C.; Zhang, J.; Wang, C. Dynamics of a Pair of Paramagnetic Janus Particles under a Uniform Magnetic Field and Simple Shear Flow. Magnetochemistry 2021, 7, 16. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, C. Numerical study of lateral migration of elliptical magnetic microparticles in microchannels in uniform magnetic fields. Magnetochemistry 2018, 4, 16. [Google Scholar] [CrossRef]
- Shapiro, M.; Shalom, A. Particle buildup on a single rod in magnetic capture from axisymmetric flows. Physicochem. Hydrodyn. 1988, 10, 215–228. [Google Scholar]
- Badescu, V.; Rezlescu, N. On the possibility of HGMS filtration in the turbulent flow regime. Powder Technol. 1992, 73, 93–94. [Google Scholar] [CrossRef]
- Lasheras, J.C.; Eastwood, C.; Martınez-Bazán, C.; Montanes, J. A review of statistical models for the break-up of an immiscible fluid immersed into a fully developed turbulent flow. Int. J. Multiph. Flow 2002, 28, 247–278. [Google Scholar] [CrossRef]
- Herdem, S.; Abbasov, T.; Köksal, M. Modelling of the buildup process of particles in pores of high-gradient magnetic filters using the Fokker-Planck equation. J. Phys. D Appl. Phys. 1999, 32, 3146. [Google Scholar] [CrossRef]
- Gardiner, C.W. Handbook of Stochastic Methods, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Kelbaliyev, G.; Tagiyev, D.B.; Rasulov, S.R. Transport Phenomena in Dispersed Media; CRC Press: Boca Raton, FL, USA, 2019. [Google Scholar]
- McNeese, W.; Wankat, P.; Friedlaender, F.; Nakano, T.; Takayasu, M. Viscosity effects in single wire HGMS studies. IEEE Trans. Magn. 1979, 15, 1520–1522. [Google Scholar] [CrossRef]
- Takayasu, M.; Friedlaender, F.; Jeong, K.; Petrakis, L. High gradient magnetic separation II: Single wire studies of shale oils. IEEE Trans. Magn. 1982, 18, 1695–1697. [Google Scholar] [CrossRef]
- Cowen, C.; Friedlaender, F.; Jaluria, R. Single wire model of high gradient magnetic separation processes II. IEEE Trans. Magn. 1976, 12, 898–900. [Google Scholar] [CrossRef]
- Cowen, C.; Friedlaender, F.; Jaluria, R. Single wire model of high gradient magnetic separation processes I. IEEE Trans. Magn. 1976, 12, 466–470. [Google Scholar] [CrossRef]
- Kurinobu, S. Buildup of magnetic flocs containing plankton on a single wire. IEEE Trans. Magn. 1987, 23, 2770–2772. [Google Scholar] [CrossRef]
- Liu, Q.; Fridlaender, F. Selective collection of non-magnetic rutile and quartz by means of a magnetic reagent by HGMS. IEEE Trans. Magn. 1994, 30, 4668–4670. [Google Scholar]
- Wankat, P.; Hwang, J.; Beckemeyer, D.; Friedlaender, F. Removal of paramagnetic particles from single wire HGMS. IEEE Trans. Magn. 1984, 20, 1177–1179. [Google Scholar] [CrossRef]
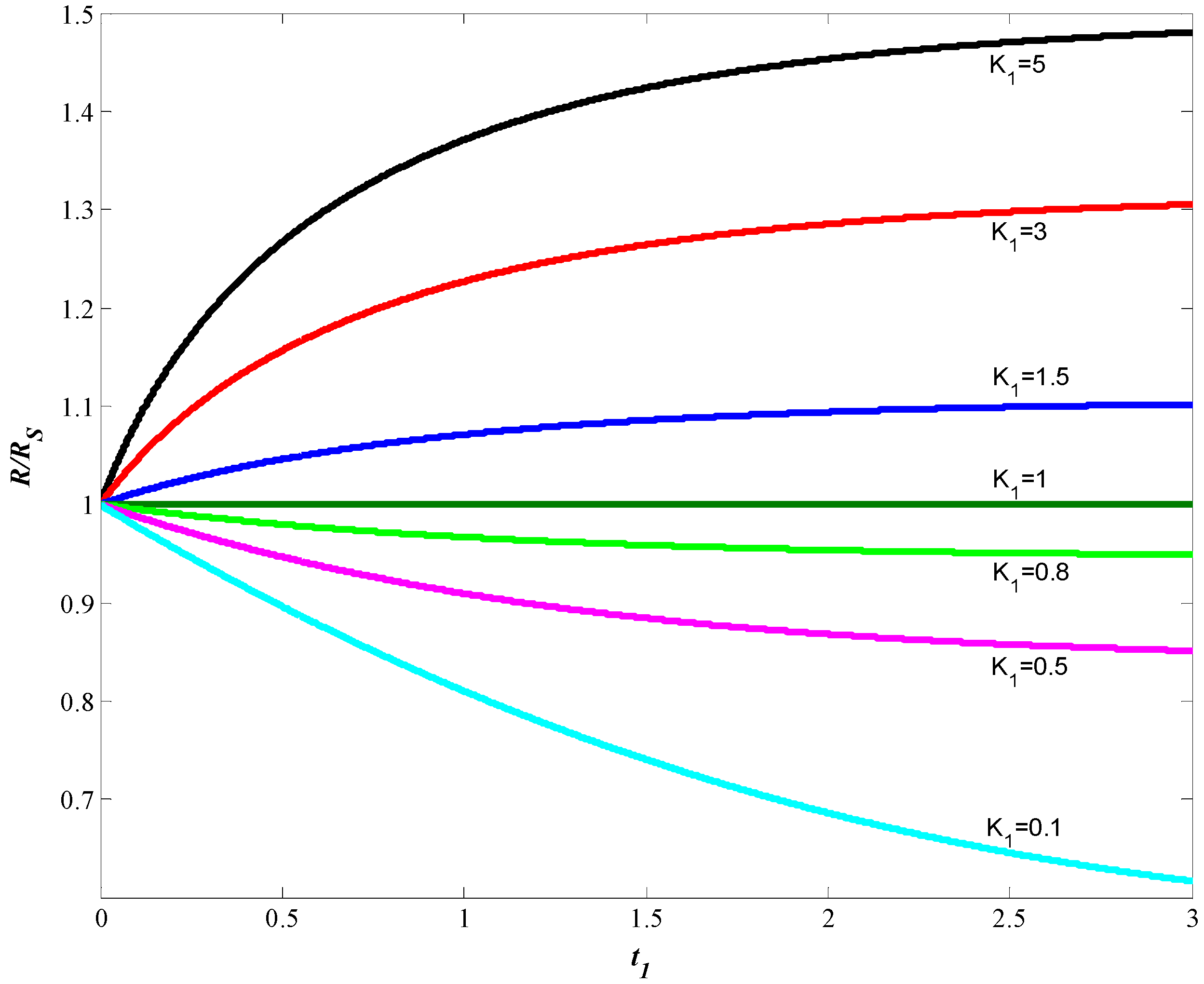
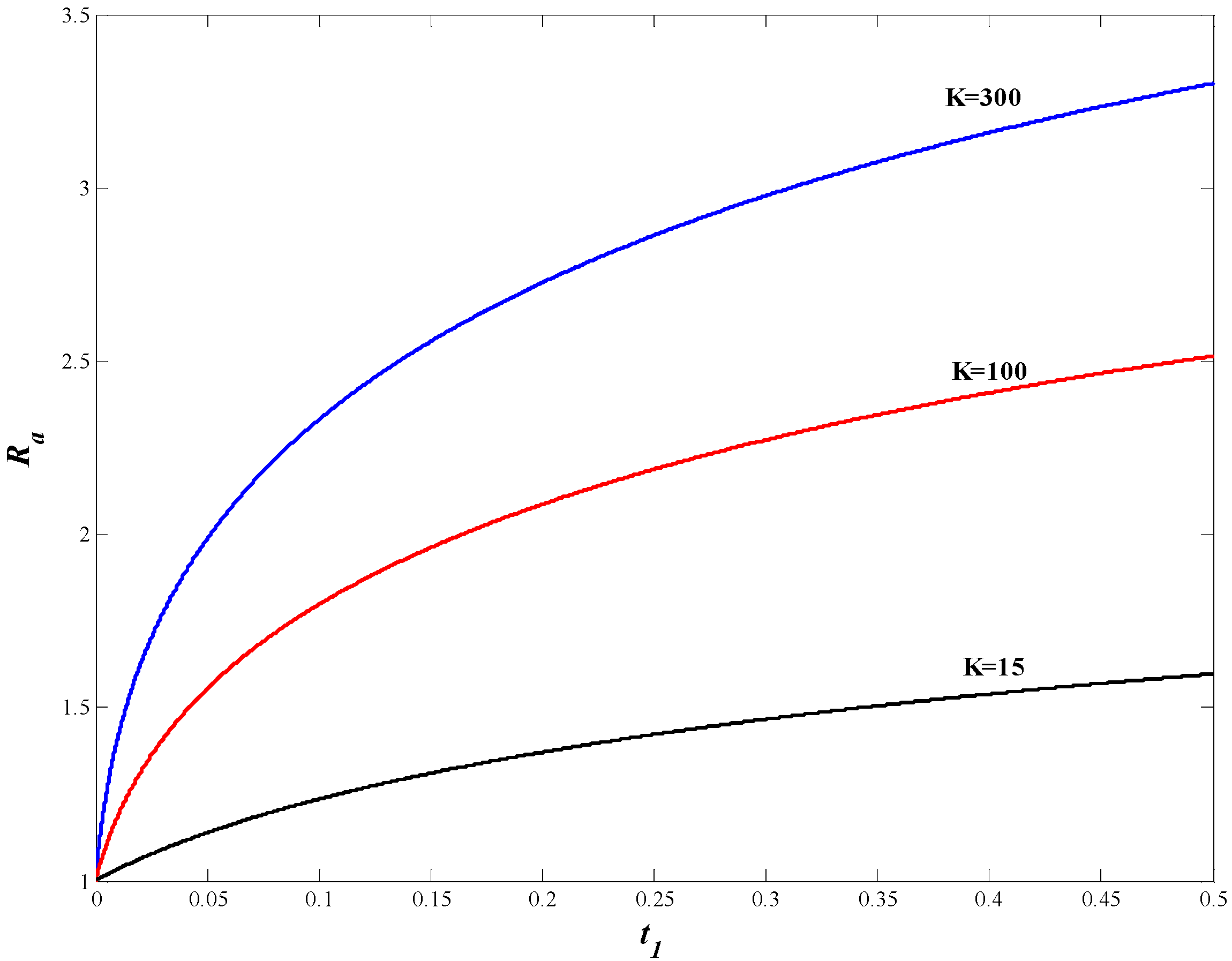
 : particles
: particles  : particles and theoretical curve calculated based on Equation (8), , ( is “travelling time” of capturing particles [32,38]).
: particles and theoretical curve calculated based on Equation (8), , ( is “travelling time” of capturing particles [32,38]).

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bilgili, H.; Keleş, C.; Abbasov, T. Modeling of the Particle Build-Up Evolution on a Single-Wire Magnetic Capture from Axial Stream Flow. Magnetochemistry 2022, 8, 15. https://doi.org/10.3390/magnetochemistry8020015
Bilgili H, Keleş C, Abbasov T. Modeling of the Particle Build-Up Evolution on a Single-Wire Magnetic Capture from Axial Stream Flow. Magnetochemistry. 2022; 8(2):15. https://doi.org/10.3390/magnetochemistry8020015
Chicago/Turabian StyleBilgili, Hatice, Cemal Keleş, and Teymuraz Abbasov. 2022. "Modeling of the Particle Build-Up Evolution on a Single-Wire Magnetic Capture from Axial Stream Flow" Magnetochemistry 8, no. 2: 15. https://doi.org/10.3390/magnetochemistry8020015
APA StyleBilgili, H., Keleş, C., & Abbasov, T. (2022). Modeling of the Particle Build-Up Evolution on a Single-Wire Magnetic Capture from Axial Stream Flow. Magnetochemistry, 8(2), 15. https://doi.org/10.3390/magnetochemistry8020015







