Engineered Magnetization Dynamics of Magnonic Nanograting Filters
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
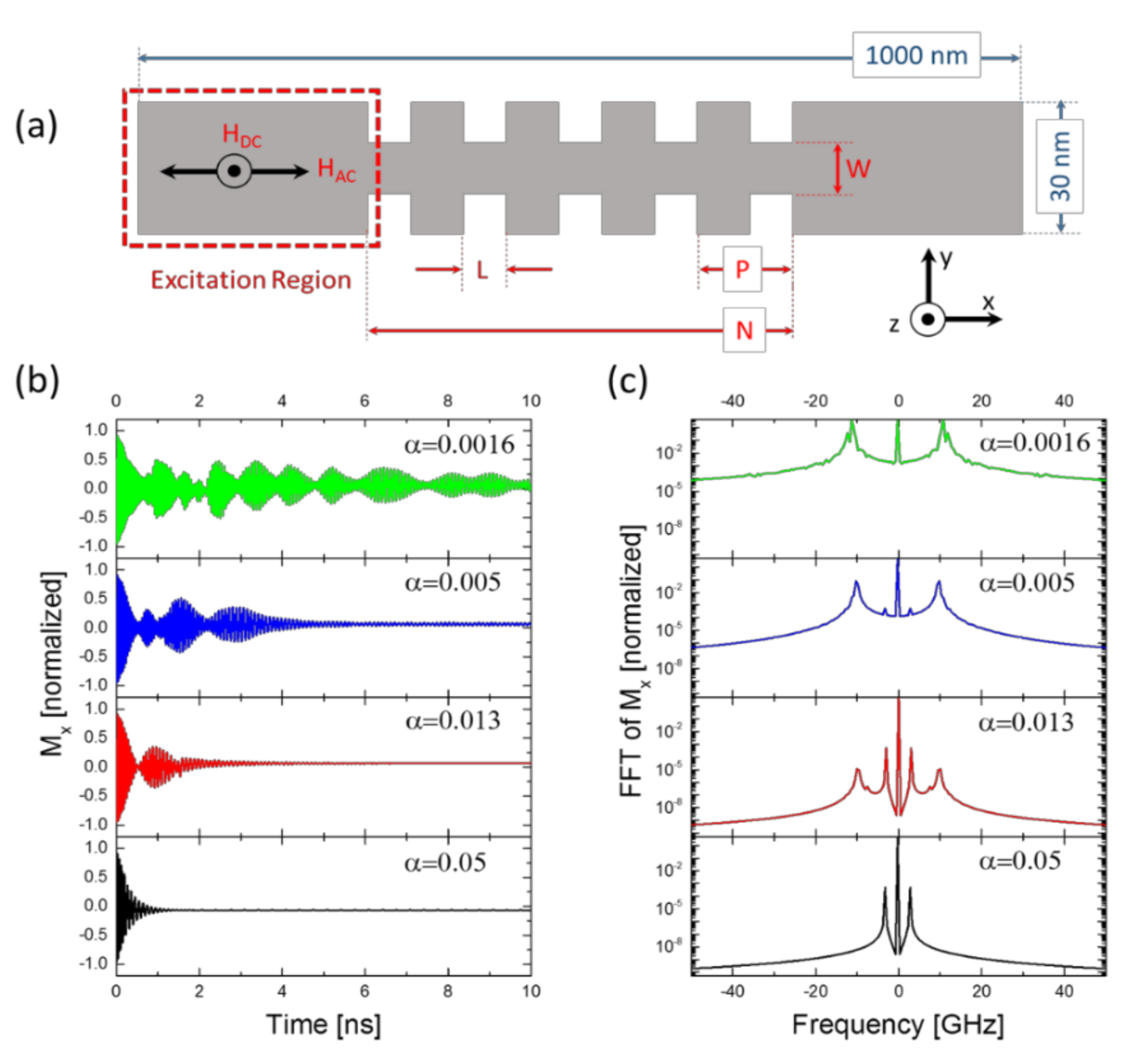
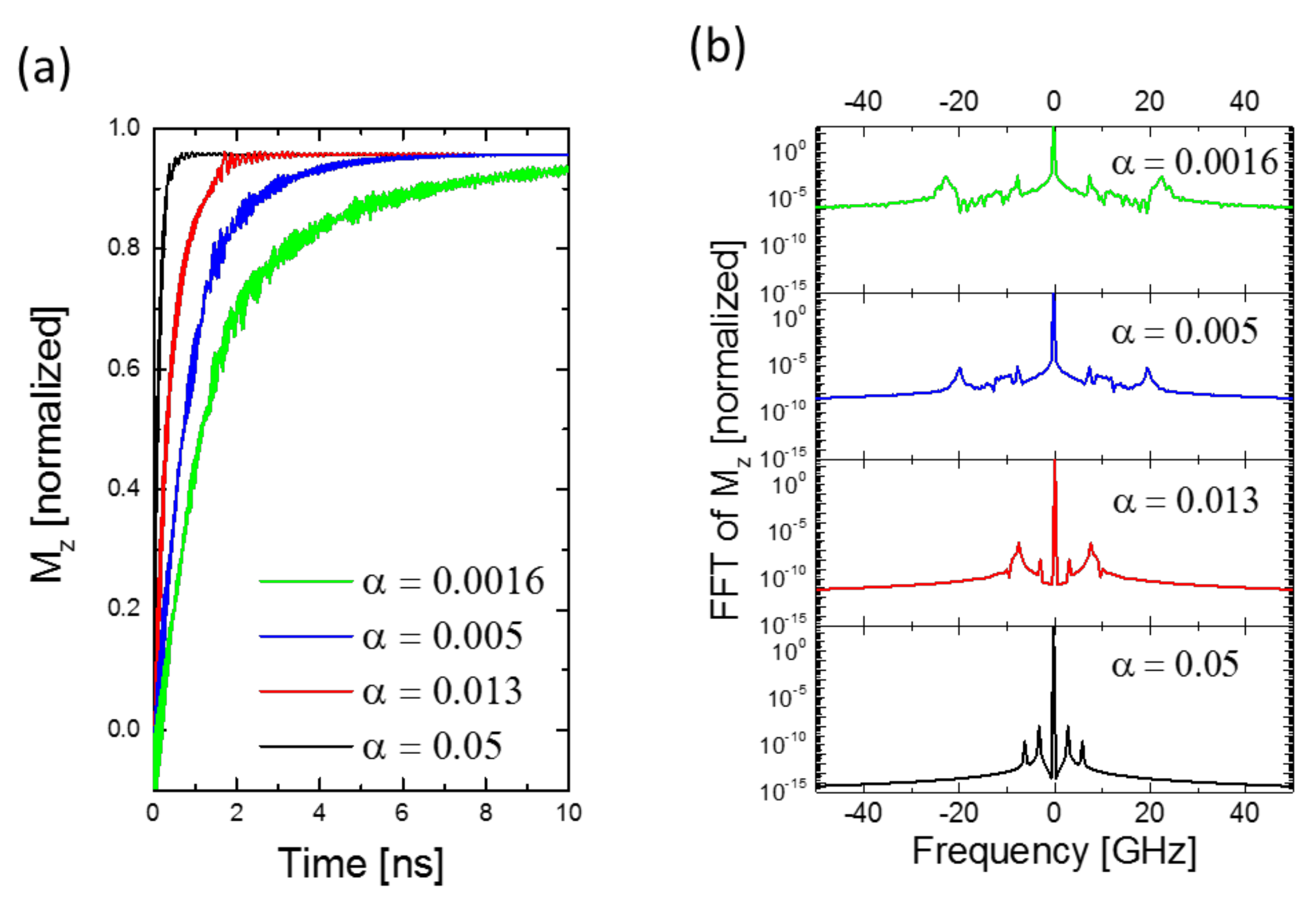
3.1. Effect of Damping, and Ms on Mx and Mz Transient and Steady State Responses
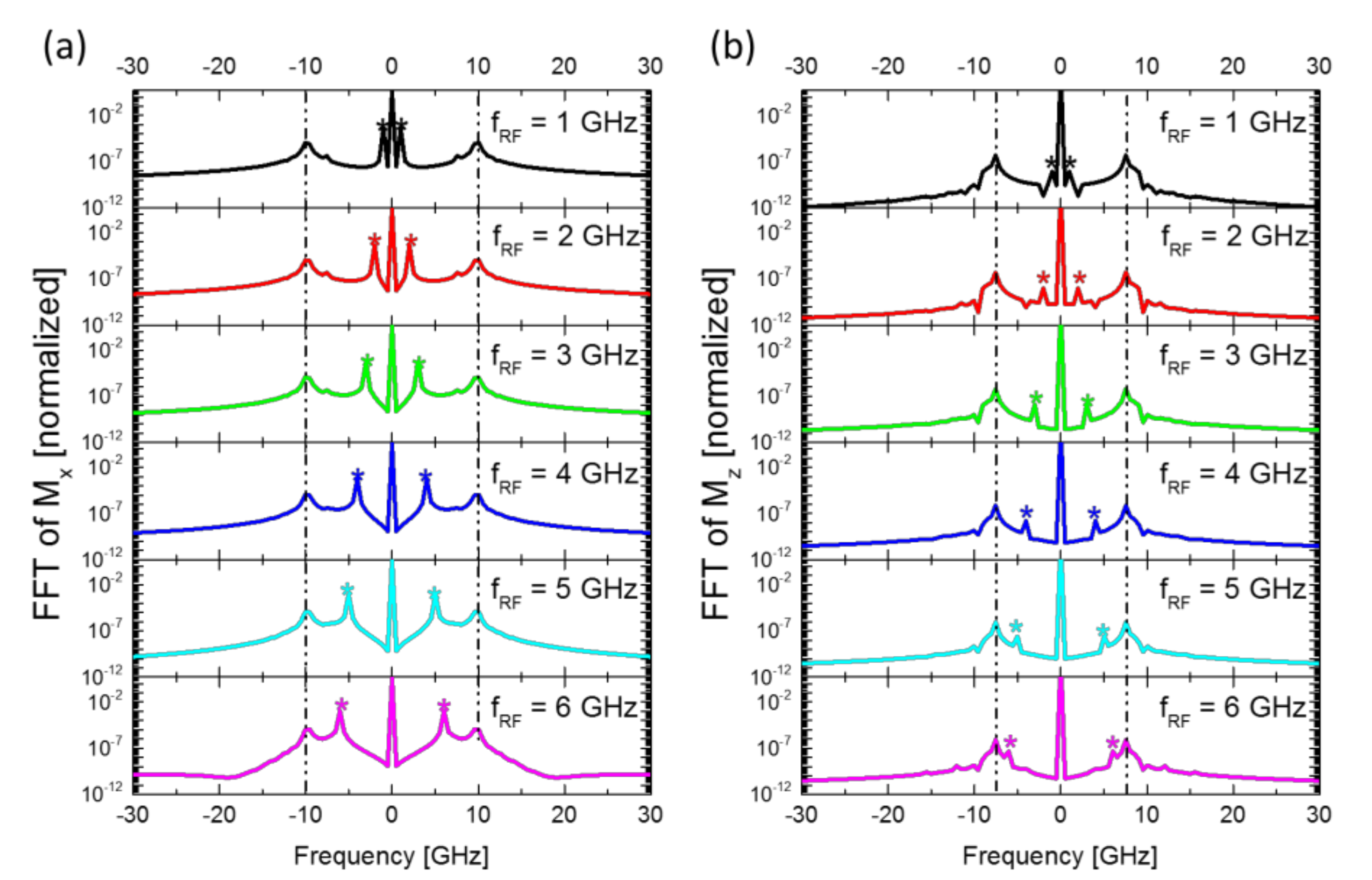
3.2. Effect of Frequency of RF Signal on Mx and Mz Steady State Responses
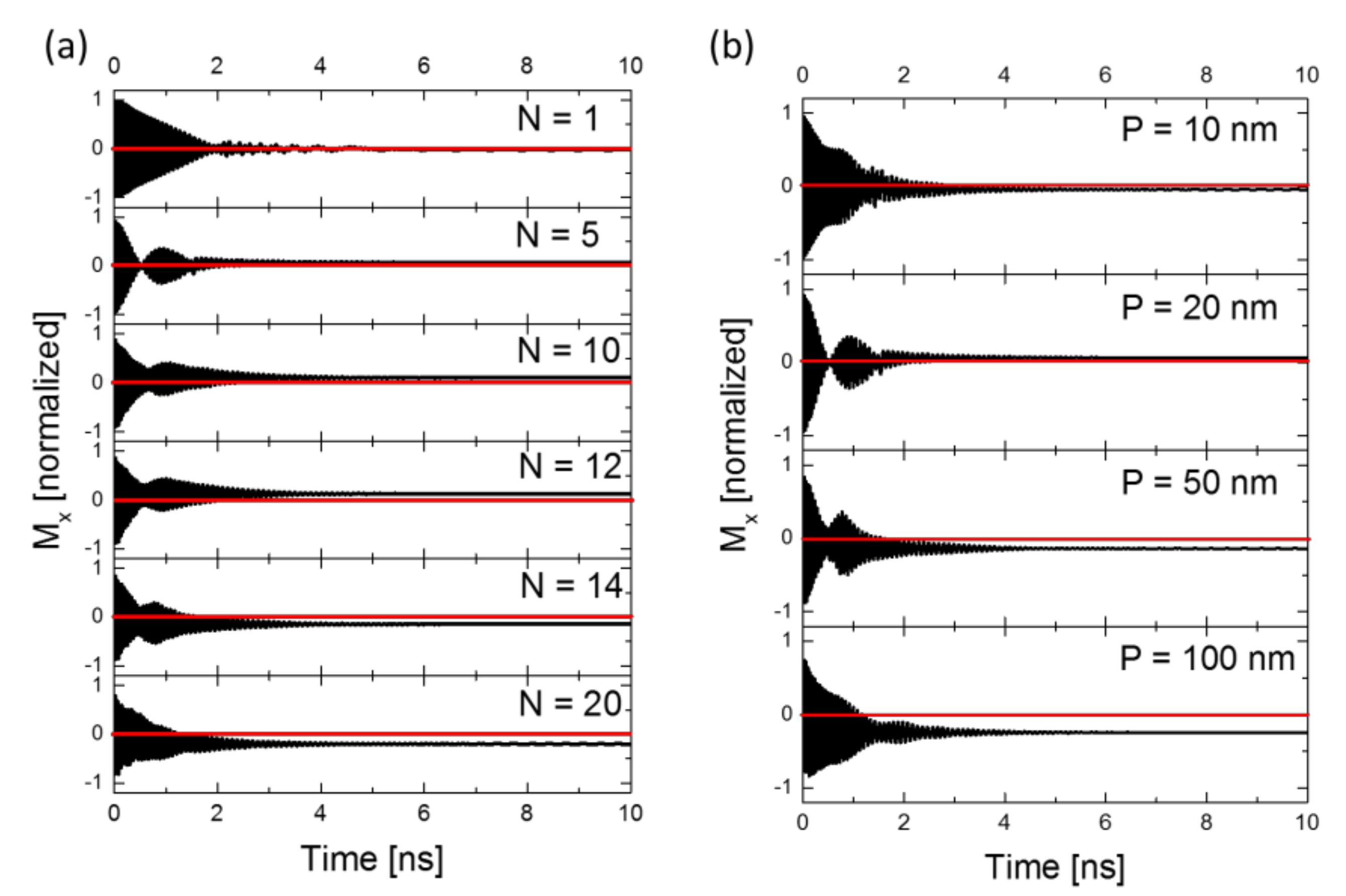
3.3. Effect of Nanograting Structure Geometry on Mx and Mz Responses
3.3.1. Periodicity (N) and Period Length (P)
3.3.2. Filter Response for W (nm)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chumak, A.V.; Serga, A.A.; Hillebrands, B.; Kostylev, M.P. Scattering of backward spin waves in a one-dimensional magnonic crystal. Appl. Phys. Lett. 2008, 93, 022508. [Google Scholar] [CrossRef]
- Deng, J.; Zhu, M.; Luo, J.; Zheng, H.; Zheng, P.; Feng, C.; Luo, J. Modulation of magnonic bands of dipole-exchange spin waves in fishbone-like yttrium iron garnet nanostrip magnonic crystal waveguides. J. Phys. D Appl. Phys. 2020, 53, 315001. [Google Scholar] [CrossRef]
- Sheshukova, S.E.; Beginin, E.N.; Sadovnikov, A.V.; Sharaevsky, Y.P.; Nikitov, S.A. Multimode propagation of magnetostatic waves in a width-modulated yttrium-iron-garnet waveguide. IEEE Magn. Lett. 2014, 5, 1–4. [Google Scholar] [CrossRef]
- Haldar, A.; Kumar, D.; Adeyeye, A.O. A reconfigurable waveguide for energy-efficient transmission and local manipulation of information in a nanomagnetic device. Nat. Nanotechnol. 2016, 11, 437–443. [Google Scholar] [CrossRef]
- Lisenkov, I.; Kalyabin, D.; Osokin, S.; Klos, J.W.; Krawczyk, M.; Nikitov, S. Nonreciprocity of edge modes in 1D magnonic crystal. J. Magn. Magn. Mater. 2015, 378, 313–319. [Google Scholar] [CrossRef]
- Ustinov, A.B.; Drozdovskii, A.V.; Kalinikos, B.A. Multifunctional nonlinear magnonic devices for microwave signal processing. Appl. Phys. Lett. 2010, 96, 142513. [Google Scholar] [CrossRef]
- Gubbiotti, G.; Zhou, X.; Haghshenasfard, Z.; Cottam, M.G.; Adeyeye, A.O.; Kostylev, M. Interplay between intra- and inter-nanowires dynamic dipolar interactions in the spin wave band structure of Py/Cu/Py nanowires. Sci. Rep. 2019, 9. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.K.; Zhang, V.L.; Lim, H.S.; Ng, S.C.; Kuok, M.H.; Jain, S.; Adeyeye, A.O. Observation of frequency band gaps in a one-dimensional nanostructured magnonic crystal. Appl. Phys. Lett. 2009, 94, 083112. [Google Scholar] [CrossRef]
- Ciubotaru, F.; Chumak, A.V.; Grigoryeva, N.Y.; Serga, A.A.; Hillebrands, B. Magnonic band gap design by the edge modulation of micro-sized waveguides. J. Phys. D Appl. Phys. 2012, 45, 255002. [Google Scholar] [CrossRef]
- Kim, S.-K.; Lee, K.-S.; Han, D.-S. A gigahertz-range spin-wave filter composed of width-modulated nanostrip magnonic-crystal waveguides. Appl. Phys. Lett. 2009, 95, 082507. [Google Scholar] [CrossRef]
- Lee, K.-S.; Han, D.-S.; Kim, S.-K. Physical origin and generic control of magnonic band gaps of dipole-exchange spin waves in width-modulated nanostrip waveguides. Phys. Rev. Lett. 2009, 102, 127202. [Google Scholar] [CrossRef]
- Wang, Q.; Zhong, Z.; Jin, L.; Tang, X.; Bai, F.; Zhang, H.; Beach, G.S.D. Design of nanostrip magnonic crystal waveguides with a single magnonic band gap. J. Magn. Magn. Mater. 2013, 340, 23–26. [Google Scholar] [CrossRef]
- Ciubotaru, F.; Chumak, A.V.; Obry, B.; Serga, A.A.; Hillebrands, B. Magnonic band gaps in waveguides with a periodic variation of the saturation magnetization. Phys. Rev. B 2013, 88, 134406. [Google Scholar] [CrossRef]
- Frey, P.; Nikitin, A.A.; Bozhko, D.A.; Bunyaev, S.A.; Kakazei, G.N.; Ustinov, A.B.; Kalinikos, B.A.; Ciubotaru, F.; Chumak, A.V.; Wang, Q.; et al. Reflection-less width-modulated magnonic crystal. Commun. Phys. 2020, 3, 1–7. [Google Scholar] [CrossRef]
- Goto, T.; Shimada, K.; Nakamura, Y.; Uchida, H.; Inoue, M. One-dimensional magnonic crystal with Cu stripes for forward volume spin waves. Phys. Rev. Appl. 2019, 11, 014033. [Google Scholar] [CrossRef]
- Ma, F.; Lim, H.; Zhang, V.; Ng, S.; Kuok, M. Magnonic band structure investigation of one-dimensional bi-component magnonic crystal waveguides. Nanoscale Res. Lett. 2012, 7, 498. [Google Scholar] [CrossRef]
- Lutsev, L.V.; Dubovoy, V.A.; Stognij, A.I.; Novitskii, N.N.; Mozharov, A.M.; Mukhin, I.S.; Ketsko, V.A. Spin-wave filters based on thin Y3Fe5O12 films on Gd3Ga5O12 and Si substrates for microwave applications. J. Appl. Phys. 2020, 127, 183903. [Google Scholar] [CrossRef]
- Fallarino, L.; Madami, M.; Duerr, G.; Grundler, D.; Gubbiotti, G.; Tacchi, S.; Carlotti, G. Propagation of spin waves excited in a permalloy film by a finite-ground coplanar waveguide: A combined phase-sensitive micro-focused brillouin light scattering and micromagnetic study. IEEE Trans. Magn. 2013, 49, 1033–1036. [Google Scholar] [CrossRef]
- Mohseni, M.; Wang, Q.; Heinz, B.; Kewenig, M.; Schneider, M.; Kohl, F.; Lägel, B.; Dubs, C.; Chumak, A.V.; Pirro, P. Controlling the nonlinear relaxation of quantized propagating magnons in nanodevices. Phys. Rev. Lett. 2021, 126. [Google Scholar] [CrossRef]
- Carter, R.L.; Owens, J.M.; Smith, C.V.; Reed, K.W. Ion-implanted magnetostatic wave reflective array filters. J. Appl. Phys. 1982, 53, 2655–2657. [Google Scholar] [CrossRef]
- Divinskiy, B.; Urazhdin, S.; Demokritov, S.O.; Demidov, V.E. Controlled nonlinear magnetic damping in spin-Hall nano-devices. Nat. Commun. 2019, 10, 1–7. [Google Scholar] [CrossRef]
- Kim, Y.M.; Han, S.H.; Kim, H.J.; Choi, D.; Kim, K.H.; Kim, J. Thickness effects on magnetic properties and ferromagnetic resonance in Co–Ni–Fe–N soft magnetic thin films. J. Appl. Phys. 2002, 91, 8462. [Google Scholar] [CrossRef]
- Richardson, D.; Kalinikos, B.A.; Carr, L.D.; Wu, M. Spontaneous exact spin-wave fractals in magnonic crystals. Phys. Rev. Lett. 2018, 121, 107204. [Google Scholar] [CrossRef] [PubMed]
- Lakshmanan, M. The fascinating world of the Landau–Lifshitz–Gilbert equation: An overview. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2011, 369, 1280–1300. [Google Scholar] [CrossRef] [PubMed]
- Kruglyak, V.V.; Hicken, R.J. Magnonics: Experiment to prove the concept. J. Magn. Magn. Mater. 2006, 306, 191–194. [Google Scholar] [CrossRef]
- Duflou, R.; Ciubotaru, F.; Vaysset, A.; Heyns, M.; Sorée, B.; Radu, I.P.; Adelmann, C. Micromagnetic simulations of magnetoelastic spin wave excitation in scaled magnetic waveguides. Appl. Phys. Lett. 2017, 111, 192411. [Google Scholar] [CrossRef]
- Klingler, S.; Pirro, P.; Brächer, T.; Leven, B.; Hillebrands, B.; Chumak, A.V. Spin-wave logic devices based on isotropic forward volume magnetostatic waves. Appl. Phys. Lett. 2015, 106, 212406. [Google Scholar] [CrossRef]
- Prabhakar, A.; Stancil, D. Propagation Characteristics and Excitation of Dipolar Spin Waves. In Spin Waves-Theory and Applications; Springer: Berlin, Germany, 2009; p. 169. [Google Scholar]
- Ding, J.; Liu, C.; Zhang, Y.; Erugu, U.; Quan, Z.; Yu, R.; McCollum, E.; Mo, S.; Yang, S.; Ding, H.; et al. Nanometer-thick yttrium iron garnet films with perpendicular anisotropy and low damping. Phys. Rev. Appl. 2020, 14, 014017. [Google Scholar] [CrossRef]
- Fujii, T.; Uchiyama, S.; Masuda, M.; Sakaki, Y. On the origin of uniaxial anisotropy in permalloy thin films. IEEE Trans. Magn. 1968, 4, 515–519. [Google Scholar] [CrossRef]
- Labrune, M.; Miltat, J. Wall structures in ferro/antiferromagnetic exchange-coupled bilayers: A numerical micromagnetic approach. J. Magn. Magn. Mater. 1995, 151, 231–245. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yagan, R.; Katmis, F.; Onbaşlı, M.C. Engineered Magnetization Dynamics of Magnonic Nanograting Filters. Magnetochemistry 2021, 7, 81. https://doi.org/10.3390/magnetochemistry7060081
Yagan R, Katmis F, Onbaşlı MC. Engineered Magnetization Dynamics of Magnonic Nanograting Filters. Magnetochemistry. 2021; 7(6):81. https://doi.org/10.3390/magnetochemistry7060081
Chicago/Turabian StyleYagan, Rawana, Ferhat Katmis, and Mehmet C. Onbaşlı. 2021. "Engineered Magnetization Dynamics of Magnonic Nanograting Filters" Magnetochemistry 7, no. 6: 81. https://doi.org/10.3390/magnetochemistry7060081
APA StyleYagan, R., Katmis, F., & Onbaşlı, M. C. (2021). Engineered Magnetization Dynamics of Magnonic Nanograting Filters. Magnetochemistry, 7(6), 81. https://doi.org/10.3390/magnetochemistry7060081





