Next Article in Journal
Microstructure Evolution of Ag/TiO2 Thin Film
Next Article in Special Issue
Numerical Optimization of a Single Bunch of NiTi Wires to Be Placed in an Elastocaloric Experimental Device: Preliminary Results
Previous Article in Journal
New Mononuclear Mn(III) Complexes with Hydroxyl-Substituted Hexadentate Schiff Base Ligands
Previous Article in Special Issue
Electrocaloric Cooling: A Review of the Thermodynamic Cycles, Materials, Models, and Devices
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Magnetochemistry
Volume 7
Issue 1
10.3390/magnetochemistry7010013
magnetochemistry-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Tuning the Magnetocaloric Properties of the La(Fe,Si)13 Compounds by Chemical Substitution and Light Element Insertion

by
Valérie Paul-Boncour
* and
Lotfi Bessais
Univ Paris Est Creteil, CNRS, ICMPE, UMR 7182, 2 rue Henri Dunant, 94320 Thiais, France
*
Author to whom correspondence should be addressed.
Magnetochemistry 2021, 7(1), 13; https://doi.org/10.3390/magnetochemistry7010013
Submission received: 15 December 2020 / Revised: 11 January 2021 / Accepted: 12 January 2021 / Published: 14 January 2021
(This article belongs to the Special Issue Magnetocaloric and Caloric Materials for Solid-State Cooling)
Browse Figures
Versions Notes

Abstract

:
LaFe 13 − x Si x compounds exhibit a giant magnetocaloric effect and they are considered as a good magnetocaloric working substance for an environmentally friendly cooling technique. Nevertheless as the Curie temperature T C is around 200 K, it is necessary to tune T C near room temperature for magnetic refrigeration. In this work we present a review of the various methods of synthesis and shaping of the LaFe 13 − x Si x type compounds as well as the influence of chemical substitution, light element insertion or combination of both on T C , magnetic entropy and adiabatic temperature variation ( Δ S M and Δ T ad ), and stability upon cycling. The advantages and drawbacks of each method of preparation and type of element substitution/insertion are discussed. The implementation of these NaZn 13 type materials in active magnetic refrigerator is presented and their performances are compared to that of Gd in prototypes.

1. Introduction

Classical refrigeration technologies are using refrigerants that deplete the ozone layer and contribute to global warming, and will be therefore forbidden by different climate protocols. Alternative refrigerants present also various drawbacks (flammable, toxic). Therefore, it is worth developing new refrigeration technologies without environmental problems [1,2], such as magnetic refrigeration that is based on the magnetocaloric effect. The development of efficient magnetocaloric materials has become challenging since the discovery of a giant magnetocaloric effect (MCE) near room temperature (RT) in Gd(Ge,Si) 5 , MnAs 1 − x Sb x and FeRh based alloys [3,4,5,6,7,8,9,10,11].
Since that time, intensive studies have yielded the discovery of several families of materials, among which the La(Fe,Si) 13 system, which displays a giant magnetocaloric effect, and is considered as a good magnetocaloric material for magnetic refrigeration. This system is particularly interesting, because it has relatively cheap, abundant, and non-toxic constituents. However several questions should be solved to use this material for magnetic refrigeration: (i) the synthesis and shaping of the material should be fast, not too expensive, and appropriate for an industrial scaling, (ii) the chemical composition should be adjusted to reach room temperature, as La(Fe,Si) 13 Curie temperature is close to 200 K, while keeping a giant MCE effect, and (iii) the compounds should remain stable upon a large number of thermomagnetic cycles. In this paper, we first propose a review of the main works that have been undertaken to solve these different challenges. The different methods of synthesis and shaping will be presented and then compared. The influence of both chemical substitution of La, Fe, and Si by other elements as well as the insertion of light elements (H, C) will be presented to show the advantages and drawbacks of each solutions.
We have developed a synthesis method combining high energy ball milling with a subsequent short annealing treatment in order to obtain a single-phase compound. Besides, a reactive Spark Plasma Sintering (SPS) method has been used in order to produce and sinter a powdered sample in one single step. The carbon atoms were inserted by solid/solid reaction, while the hydrogenation of the parent intermetallic La(Fe,Si,Co) 13 alloys was carried out using a Sievert method. A study of our own experimental results will then be presented, which combines Fe for Co substitution with the hydrogen insertion in order to give an example of a strategy developed to obtain performant magnetocaloric materials. The insertion of light elements (H, C) or/and cobalt for iron substitution are used in order to improve the Curie temperature near room temperature for magnetic refrigeration applications. The tuning of the Curie temperature is explained by a combination of the structural and electronic properties. The advantages and disadvantages of each type of element insertion are discussed and the misunderstanding on interstitial site is clarified.

2. Sample Synthesis

Condition of Synthesis of La(Fe 1 − X Si x ) 13 Type Alloys

Contrary to LaCo 13 , the binary LaFe 13 compound does not form, but the 1:13 phase can be stabilized by a partial Si for Fe substitution. The study of the ternary La-Fe-Si ternary phase diagram at 1173, 1373, and 1573 K [12,13] showed that the LaFe 13 − x Si x compounds only exist in a very narrow range of composition (with La/(Fe+Si) =1/14) and crystallize either in the cubic NaZn 13 type structure (phase τ 1 ) or the tetragonal Ce 2 Ni 17 Si 9 -type structure (phase τ 2 ). At 1200 K, τ 1 is single phase for 0.08 ≤ x ≤ 0.21 and τ 2 for 0.25 ≤ x ≤ 0.40 . Both of the phases coexist between 0.21 and 0.25. The Si concentration at the boundaries varies with the equilibrium temperature. The NaZn 13 phase forms via a peritectic reaction at T = 1665 K:
LFeSi + LLa + LaFe2Si2 → τ1 or α−Fe + LLa + LaFe2Si2 → τ1
The as-cast alloys are constituted by a mixture of α − Fe(Si) and LaFeSi (formed from the liquid L La phase upon cooling) and an appropriate thermal treatment is necessary for obtaining the NaZn 13 τ 1 -phase via a diffusion process [14,15,16]. The single phase NaZn 13 samples can be synthesized by arc or induction melting with a further annealing treatment at temperatures between 1273 and 1423 K and during several days or weeks [11,12]. It is necessary to take the risk of La oxidation into account and an excess of La is generally added in order to avoid the formation of secondary phases [17]. The chemical purity of each element, their preparation into a glove box, and the quality of the atmosphere upon melting and annealing (secondary vacuum or Argon pressure) are crucial to obtain single phase compounds. The annealing time can be shortened by increasing the annealing temperature. In ref [18] the NaZn 13 was obtained for an arc-melted sample annealed 1 h at 1573 K, but some α -Fe was still present. However, in order to prepare a large quantity of sample for magnetic refrigeration application, the too long duration or too high temperature of the annealing treatment remains an economical drawback. Therefore, to reduce the annealing time several alternative synthesis methods have been developed. They are mainly based to a reduction of the grain size and an intimate contact between the various phases obtained as cast to shorten the diffusion path. Among these methods, one can find melt-spinning [19,20,21,22,23], melt extraction [24], drop-tube solidification [25], and solid state sintering [26]. Gas atomization, followed by an annealing treatment of 1 hour at 1323 K, has been developed by Erasteel Company, in order to produce batches of 500 kg of alloys for industrial applications [27]. Another industrial alternative was developed by the Vacuumschmelze Company [28,29], based on powder metallurgy using reactive sintering.
Our group has developed a high energy ball milling method starting with a mixture of LaSi alloys, α − Fe and Si. A ball milling of only 1 hour, followed by an annealing treatment of 30 min at 1423 K, were sufficient for obtaining 95 % of the NaZn 13 phase with very small quantities of α − (Fe,Si), La 2 O 3 and La(OH) 3 [30]. A comparison of the results that were obtained by these different techniques can be found in [31].
Furthermore, it is also necessary to shape the magnetocaloric material in appropriate form as a function of the design of the magnetic refrigeration device. Several methods have been investigated, like extrusion after mixing with an epoxy polymer [27], thermal decomposition and recombination (TDR) process [29], and selective laser melting [32]. Erasteel has been chosen to mix the powder that was obtained from gas atomization with epoxy, whereas Vacuumschmelze has developed the TDR process in order to shape their materials in specific form. They prepare an alloy containing a mixture of α − Fe and LaFeSi, which can be easily cut without breaking and then process to the annealing treatment in order to obtain the NaZn 13 phase.
Our group also succeeded in obtaining a NaZn 13 sample with a density that is very close to that of bulk sample by combining high energy ball milling with reactive spark plasma sintering (SPS). It was possible to directly obtain the NaZn 13 phase by sintering the non-annealed BM sample at 1273 K with a heating rate of 100 K/min [31]. The best results were obtained under Ar atmosphere with a final step of 15 min at 1273 K [33].

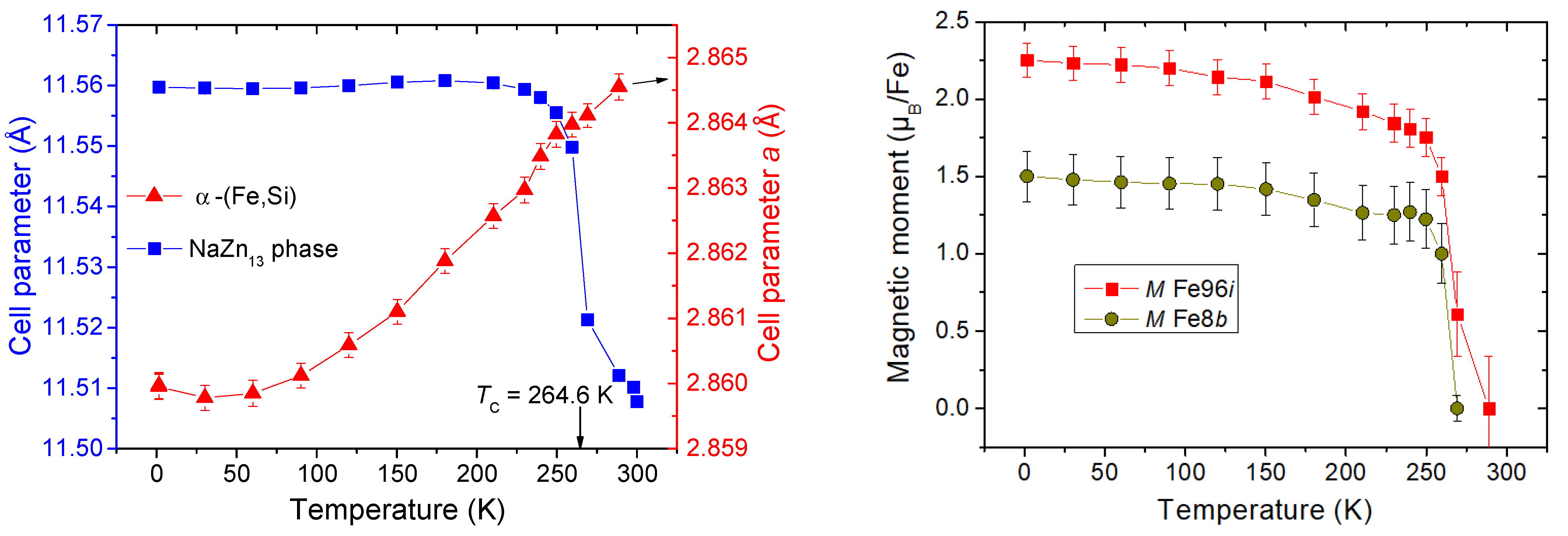
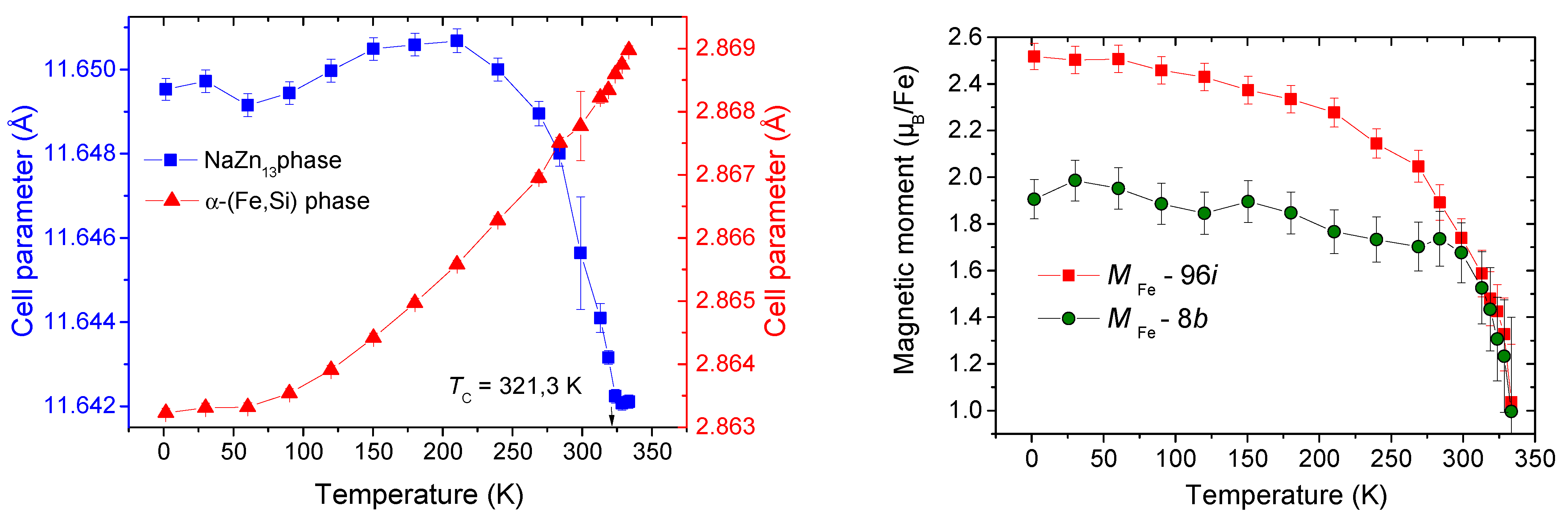
3. Properties of the La(Fe 1 − X Si x ) 13 Compounds

Table 1 reports the crystallographic data of both τ 1 (NaZn 13 ) and τ 2 (Ce 2 Ni 17 Si 9 ) phases. The crystal structure of the NaZn 13 phase is shown in Figure 1. The evolution of the cell parameters versus the Si content have been detailed in [34]. The cubic cell parameter slightly decreases versus Si content ( a ≈ 11.46 Å). The tetragonal distortion corresponds to an expansion along the c axis and an intermediate tetragonal τ 2 ′ phase is found as the distortion progressively increases. The cell volume decrease becomes steeper upon the tetragonal distortion for a Si concentration larger than 20 at.%.
All of the La(Fe 1 − x Si x ) 13 compounds are ferromagnetic. The Curie temperature T C monotonously increases from 200 to 260 K for 0.1 < x < 0.21 , and then decreases abruptly reaching 60 K for x = 0.34 [34]. The spontaneous magnetization decreases linearly versus Si content with a discontinuity at the τ 2 ′ − τ 2 transition. The evolution of the magnetic entropy variation Δ S M at the transition of the τ 1 phase was studied in [30]. It showed that the Δ S M peak was larger and higher for x = 0.108 ( Δ S M max = 32.6 J/kgK) and broadens progressively as the Si content increases. This indicates a diminution of the first order character of the transition. As the magnetic properties of the τ 2 phase are not suitable for magnetocaloric application and they will not be discussed in the following. Thermal expansion measurements for the τ 1 phase showed that the cell volume decreases sharply at T C , thus confirming the first order character of the ferromagnetic-paramagnetic transition [35]. Above T C , an itinerant electron metamagnetic (IEM) behavior is observed. The linear decrease of T C under applied pressure confirms its strong dependence versus cell volume variation, characteristic of the magnetovolume behavior of IEM compounds.
The NaZn 13 compounds show hysteresis that yields energy loss upon magnetic cycles due to the first order character of the metamagnetic FM-PM transition. Therefore, several studies have been undertaken in order to reduce the hysteresis [36,37,38,39]. It was found that the hysteresis increases with the field sweep rates, but it can be reduced by playing on the sample shape to lower the demagnetization coefficient. Indeed, the hysteresis is smaller for thin or porous samples and fragments when compared to bulk samples. The hysteresis can be also significantly reduced when the applied field is parallel to the smaller dimension of the sample. The presence of local chemical inhomogeneity’s also play a role in the local magnetic properties [40].
Therefore, La(Fe 1 − x Si x ) 13 compounds with the NaZn 13 structure are very interesting for their giant magnetocaloric effect and other advantages (moderate cost, environmental friendly), but their Curie temperature remains too low for room temperature magnetic refrigeration. Therefore, many works have been undertaken to reach an operating room temperature range while keeping a giant MCE: the substitution of La or Fe, insertion of light elements (H, C), or combination of both. The main and most interesting results will be described in the following part of this paper.

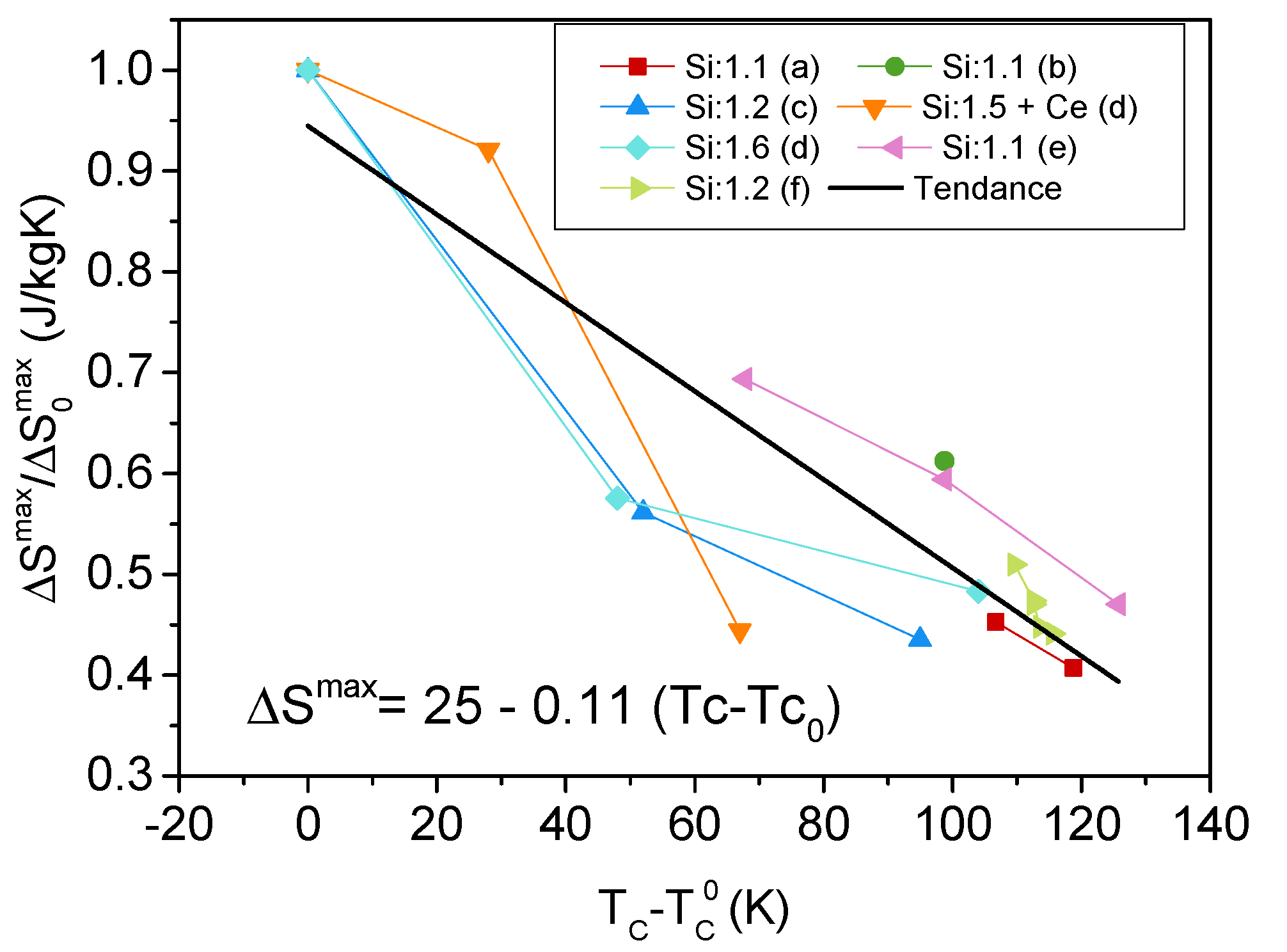
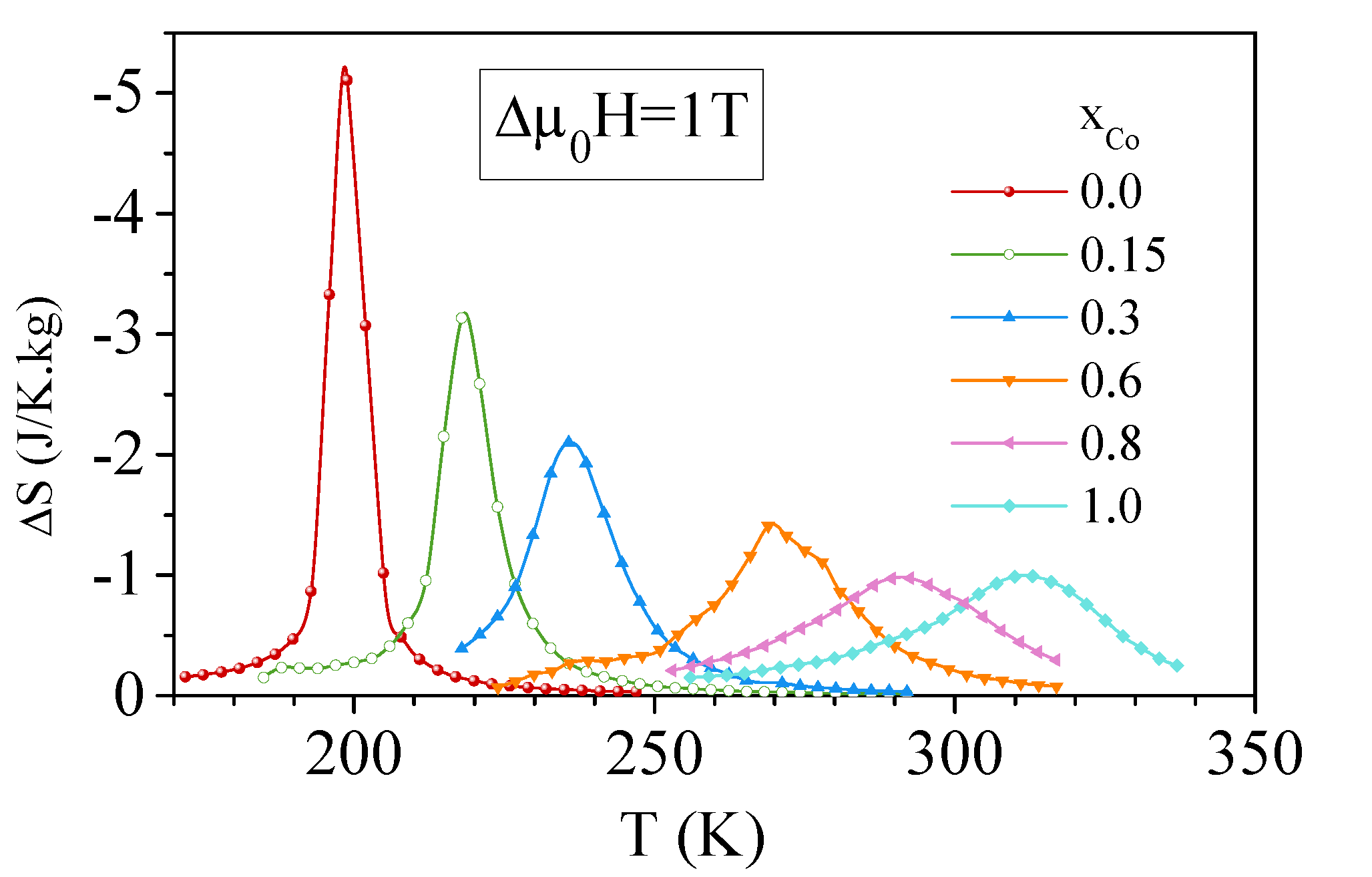
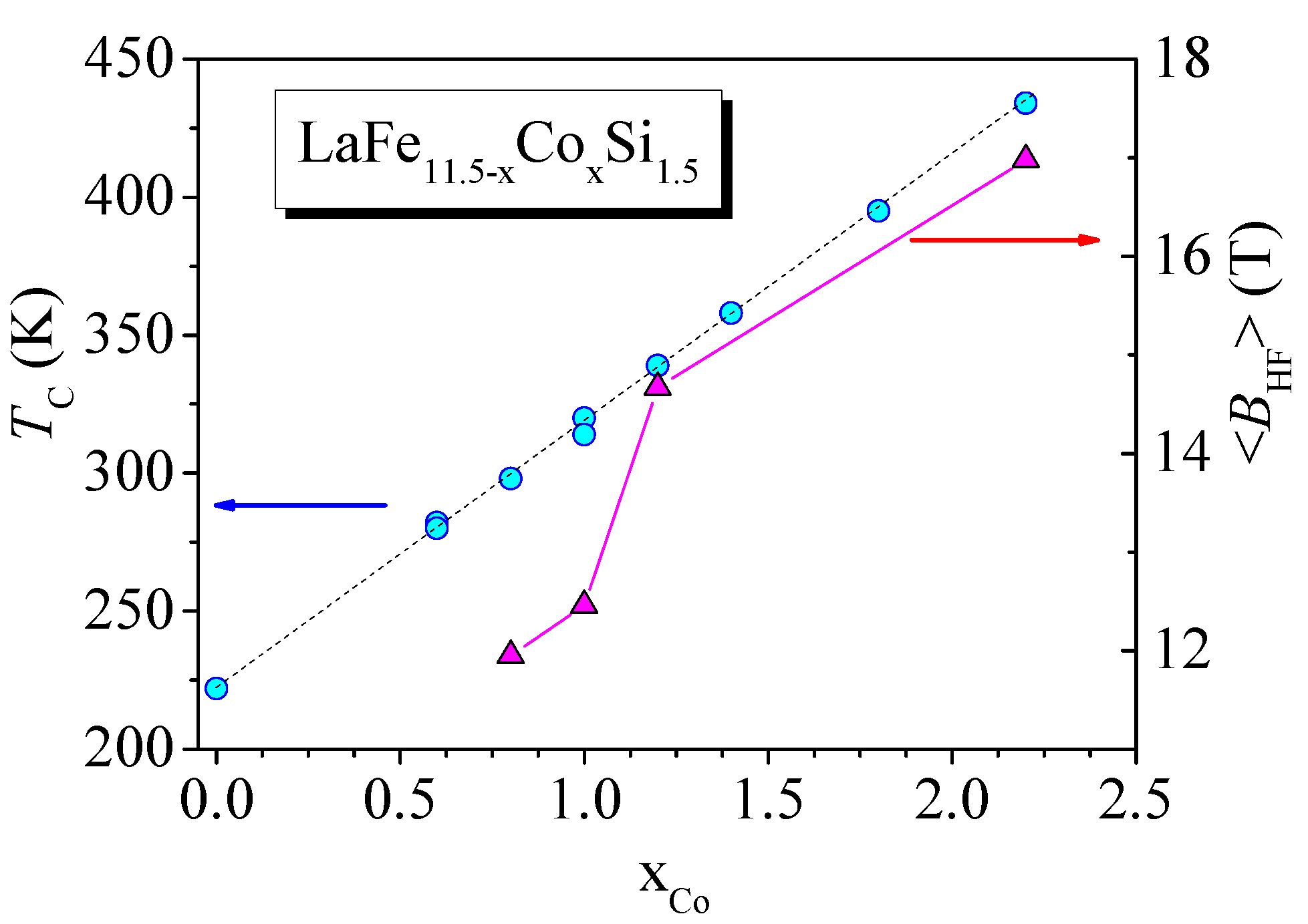
4. Effect of Iron Substitution in La(FeSiM) 13 Compounds

The Curie temperature of La(Fe,Si) 13 alloys was found to be much lower than the LaCo 13 alloy (1318 K) [41]. The main reason is that Fe-Fe interactions are much weaker than the Co-Co interactions in NaZn 13 -based systems. This fact can explain the increase of T C in the Co-substituted La-Fe-Si alloys, which was attributed to the strong Co-Fe and Co-Co exchange interactions.
Hu et al. [42], Liu et al. [21], Katter et al. [28], Bjork et al. [43], and Hansen et al. [44] have substituted cobalt for iron atoms, while preserving the first-order magnetic phase transition from paramagnetic to ferromagnetic state at T C in order to increase the Curie temperature of LaFe 13 − x Si x . The obtained compound, LaFe 11.2 Co 0.7 Si 1.1 presents a very large magnetic entropy change Δ S M = 20.3 J/kgK under a magnetic field change Δ H = 5 T at T C = 274 K. The main reason of this large Δ S M is the huge negative lattice expansion at T C . In addition, the LaFe 11.2 Co 0.7 Si 1.1 compound exhibits a small temperature hysteresis. At the Curie temperature, the unit cell parameter decreases sharply as the structure stay cubic with F m 3 ¯ c space group. The unit cell parameter in the paramagnetic state was smaller than in the ferromagnetic one, with a change of 0.43% for this magnetic state transition.
For LaFe 11.2 Si 1.8 compound Hu et al. [45] substituted Fe by Co and studied La(Fe 1 − x Co x ) 11.2 Si 1.8 ( x = 0 − 0.08 ) compounds, that they prepared by arc melting and subsequent annealing at 1323 K. They found that the Curie temperature derived from the ZFC-FC magnetization increases from 222 K for x = 0 to 307 K for x = 0.08 , while the magnetic entropy change decreases from 13 J/kg K for x = 0 to 8 J/kg K for x = 0.08 under magnetic field variation of 0 − 5 T. The later value of Δ S M is just smaller than Gd at the same magnetic field changes 0 − 5 T. Another interesting result observed is the disappearance of the field induced itinerant electron metamagnetic transition with cobalt content; indeed, Hu et al. [45] underlined that the substitution of iron by cobalt induced a disappearance of the asymmetrical broadening of Δ S M peak.
Figure 2 highlights the fact that Si content has much less influence on T C when considering a Co substituted compound. It shows that the increase of T C is linear with the substitution rate and it reaches about 310 K for x = 1 . The increase of T C is due to the addition of new 3 d electrons by the cobalt, which modifies the density of states of the material.
Figure 3 shows a passage from first-order toward second-order magnetic phase transition visible through the quick decrease of Δ S M max with T C . An increase in T C of 100 K due to the Co substitution results in a decrease of Δ S M max of 13 J/kg.K. The decrease of Δ S M max is much less than in the case of the silicon content change. Therefore, it is preferable to use Co substitution to increase T C . The decrease of Δ S M max due to a second-order transition is compensated by the widening of Δ S M . In this case, the Relative Cooling Power (RCP) appears to be unchanged. On the other hand, a more spread out transition in working temperature can be efficient for applications.
Paul-Boncour et al. [52] studied the influence of cobalt substitution for LaFe 11.5 − x Co x Si 1.5 compounds ( x ≤ 2.2 ), prepared by ball milling and one of either short annealing treatment or reactive Spark Plasma Sintering (SPS). Their magnetic properties have been investigated while using magnetic measurements, differential scanning calorimetry, and Mössbauer spectroscopy. Figure 4a shows the cell volume at 293 K plotted versus Co content. The annealed and sintered powder display close cell volumes for a given x Co content. We observe a critical range with a cell volume jump, which can be related to the change of magnetic order, depending on whether samples are above or below T C , in the ferromagnetic state as the cell volume decreases versus Co content.
The Curie temperature was estimated either from the maxima of d M / d T or d V / d T derivative curves or by the maxima of the DSC peaks. Figure 4b shows the good agreement obtained between these different methods. T C increases linearly versus x Co content according to the following relation: T C = 226 + 97 × x Co .
Δ S M max curves versus temperature for LaFe 11.5 − x Co x Si 1.5 compounds with μ 0 Δ H of 1 T are presented in Figure 5. T C shift to larger temperature and a decrease of Δ S M max are clearly observed as the Co content increases.
The 57 Fe Mössbauer spectra that were collected at room temperature, for x = 0.8, 1, 1.2, and 2.2, have been refined with several ferromagnetic sextets for both inequivalent 8 b and 96 i iron sites. The obtained weighted average hyperfine field < B HF > , together with T C , versus x Co content, are shown in Figure 6. As the Co content becomes larger, an increase of the mean Fe local moment is observed. It is worth noticing that the Co substitution increases both T C and the iron local moment.
Gebara et al. [53] have studied LaFe 11.8 − x Co x Si 1.2 alloys ( x = 0.52 , 0.66 , 0.8 , 0.94 , 1.08 ) composites that were prepared by high energy ball milling showing the evolution of lattice constant, the Curie temperature, magnetic entropy change, and the evolution of the Fe Mössbauer spectra. Surprisingly, the magnetic entropy changes are not very sensitive to the Co content in their composites.
The substitution of Fe by the Z elements (Z = V, Cr, Ni, Cu) in the La(Fe 1 − x Z x ) 11.4 Si 1.6 system can lead to a strong influence on the electron concentration on the magnetic and magnetocaloric properties [54]. This is due to the properties of itinerant metamagnets that are very sensitive to the 3 d electron density at the Fermi level. For this motivation, Pathak et al. [54] have studied the possibility of substituting Fe by other 3 d metals (V, Cr, Ni, Cu).
For Z = V and at low Z substitution rates, in La(Fe 1 − x Z x ) 11.4 Si 1.6 , a loss of the magnetocaloric effect and an increase in T C are observed, T C = 211 K for x = 0 increases up to 223 K for x = 0.01 , and Δ S M max decreases from 15 to 8 J/kg.K. On the contrary, a substitution of Fe by Mn in La(Fe 1 − x Mn x ) 11.7 Si 1.3 [55] shows a decrease in T C , as well as a decrease of Δ S M max .

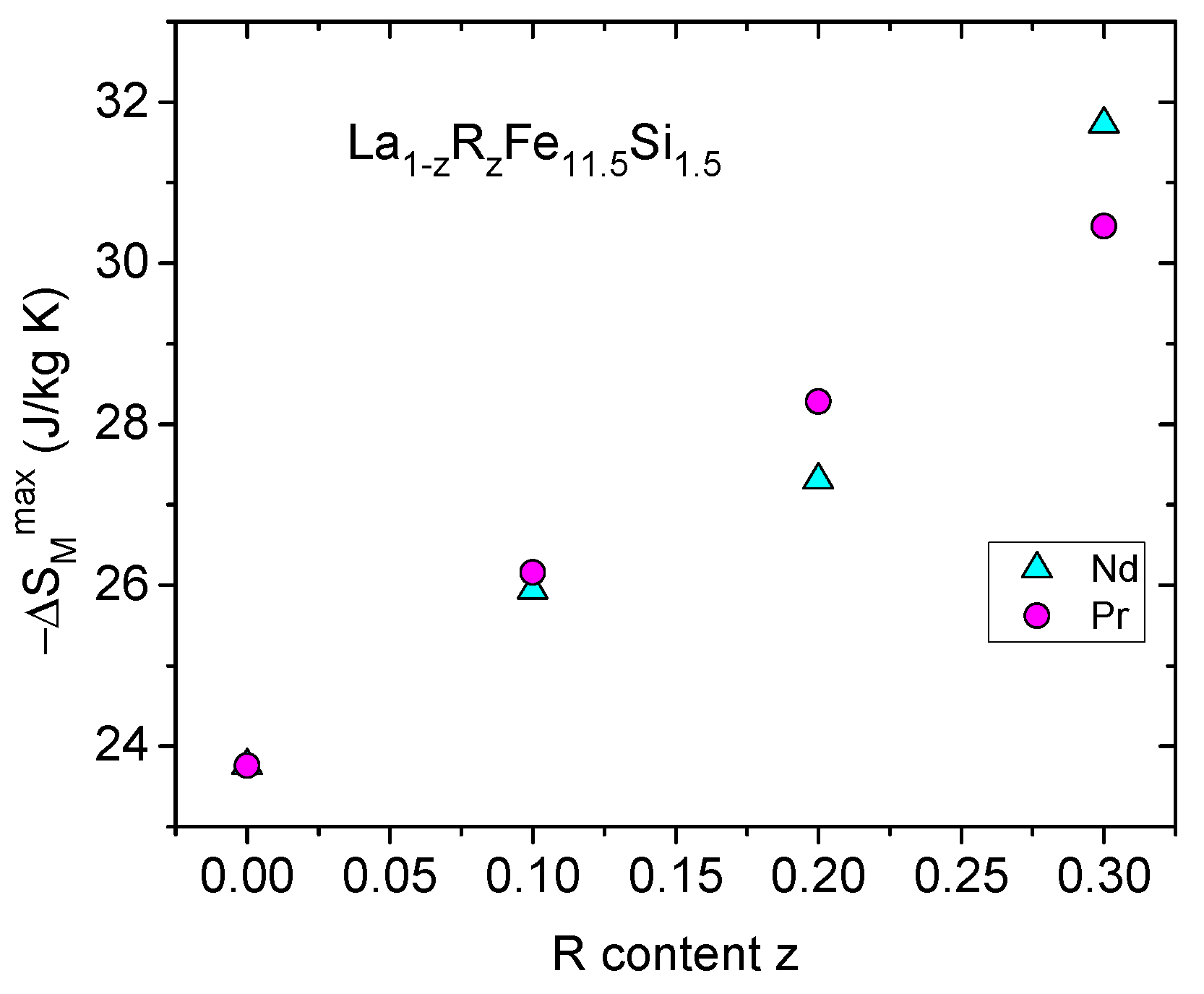
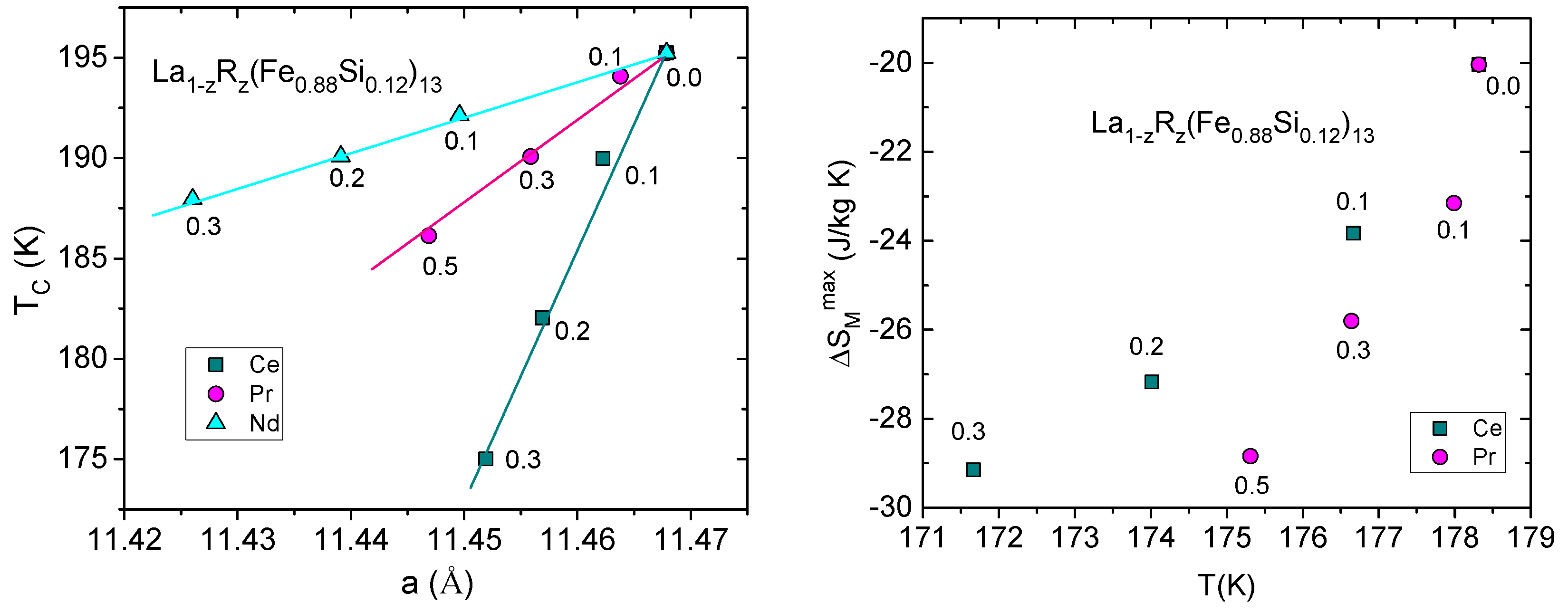
5. Effect of La Substitution in La 1 − Z R z (Fe,Si) 13

The substitution of La by another rare earth atom influences the structural and magnetic properties of the material. Anh et al. [56] performed a systematic study on the effect of Nd substitution on magnetocaloric effect in La 1 − z Nd z Fe 11.44 Si 1.56 ( z = 0 − 0.4 ). They found a decrease of the unit cell parameter and Δ S M max with an increasing Nd content.
Fujita et al. [57,58] and Fujieda et al. [59,60] studied the effect of partial substitution of La in La(Fe 0.88 Si 0.12 ) 13 compound. Figure 7 (Left) shows that the unit cell parameter and T C linearly decrease with Ce/Pr content; these results were mainly attributed to the magnetovolume effect [59,60].
In addition, Δ S M max increases with the substitution rate (Figure 8). We can see that the effect is more contrasted with Ce atoms, which can be tetravalent. In an alloy, its average valence is therefore greater than three and its average ionic radius is smaller than for other rare earths.
The effect of substituting La by another rare earth atom on the unit cell parameter, T C , and MCE was also studied by Shen et al. [61,62] for (R = Ce, Pr, Nd) and by P. Gebara and J. Kovac [63,64] for (R = Dy, Ho).
The maximum value of Δ S M max was found to increase from 23.7 J/(kg.K) for z = 0 to 32.0 J/(kg.K) for z = 0.3 in La 1 − z Nd z Fe 11.5 Si 1.5 , for a field change μ 0 Δ H of 0-5 T, but this comes with a change of the hysteresis loss from 21.2 J/kg to 77.5 J/kg. Moreover, the relative cooling power (RCP) is enhanced by the partial substitution of Nd [61,62]. On the other hand, the substitution of La by Ho or Dy causes a decrease of magnetic entropy change [63,64].

6. Effect of Light Element Insertion in La(FeSi) 13 X

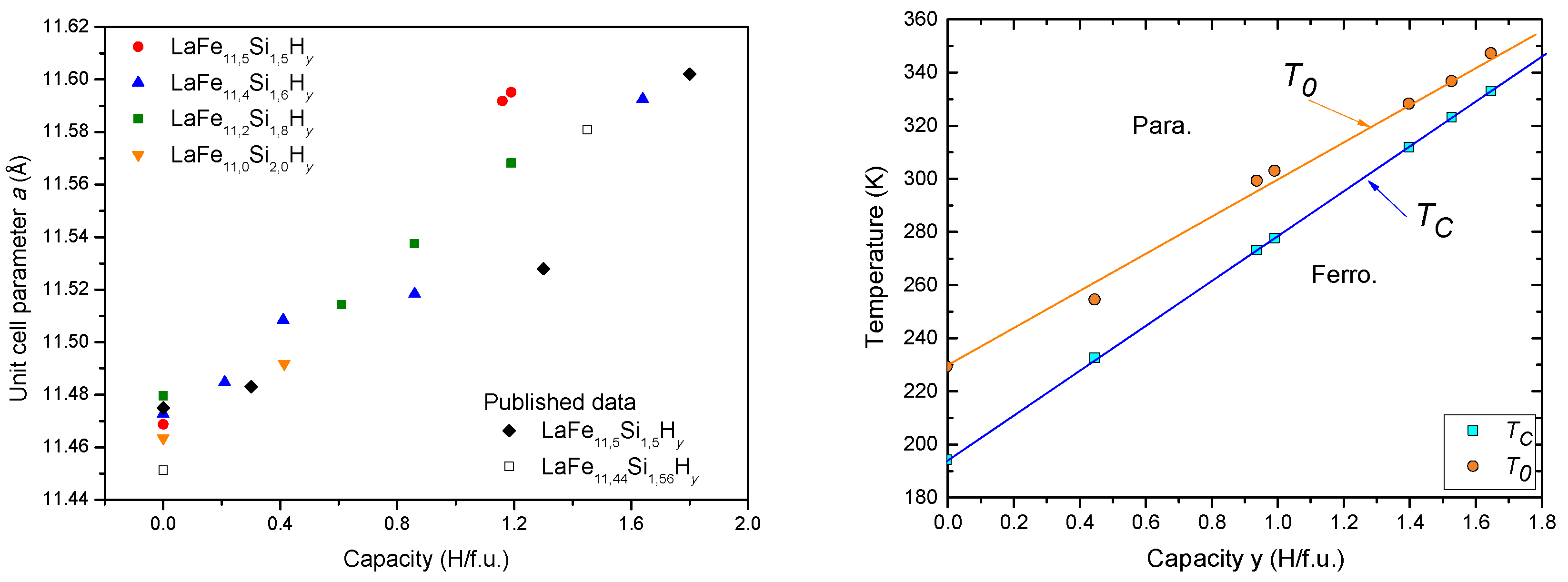
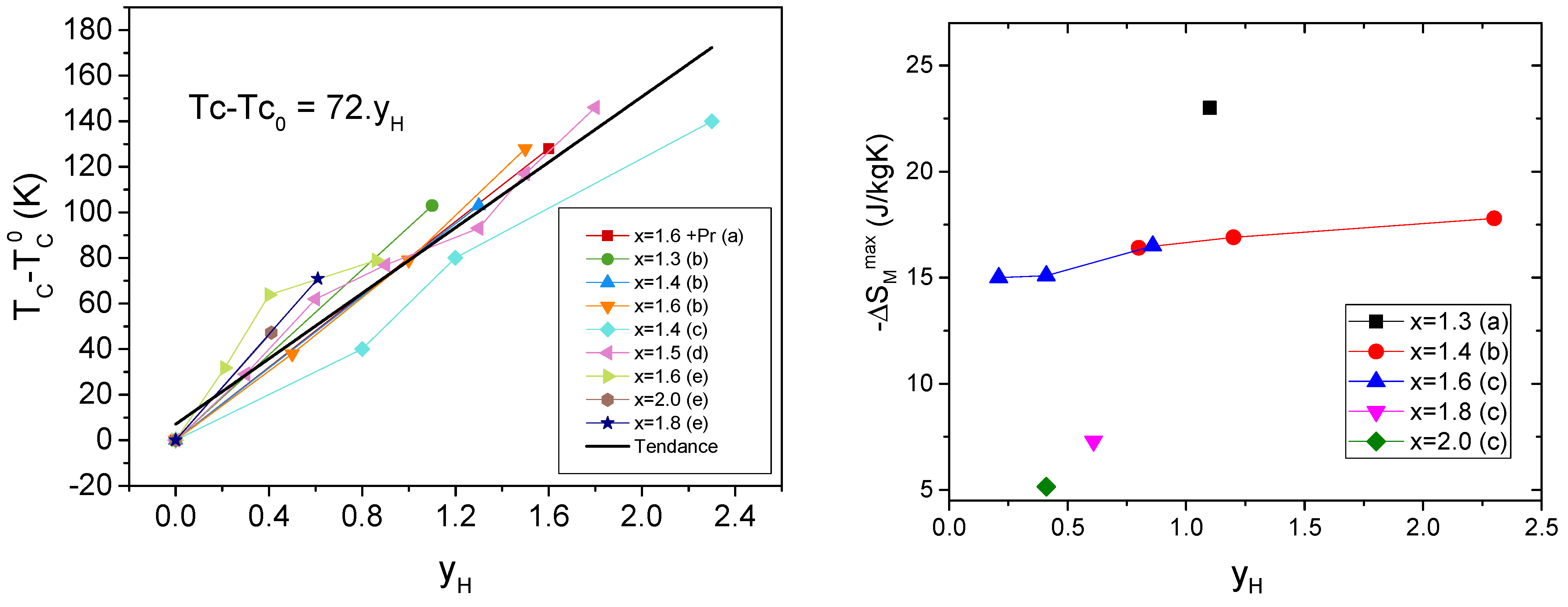
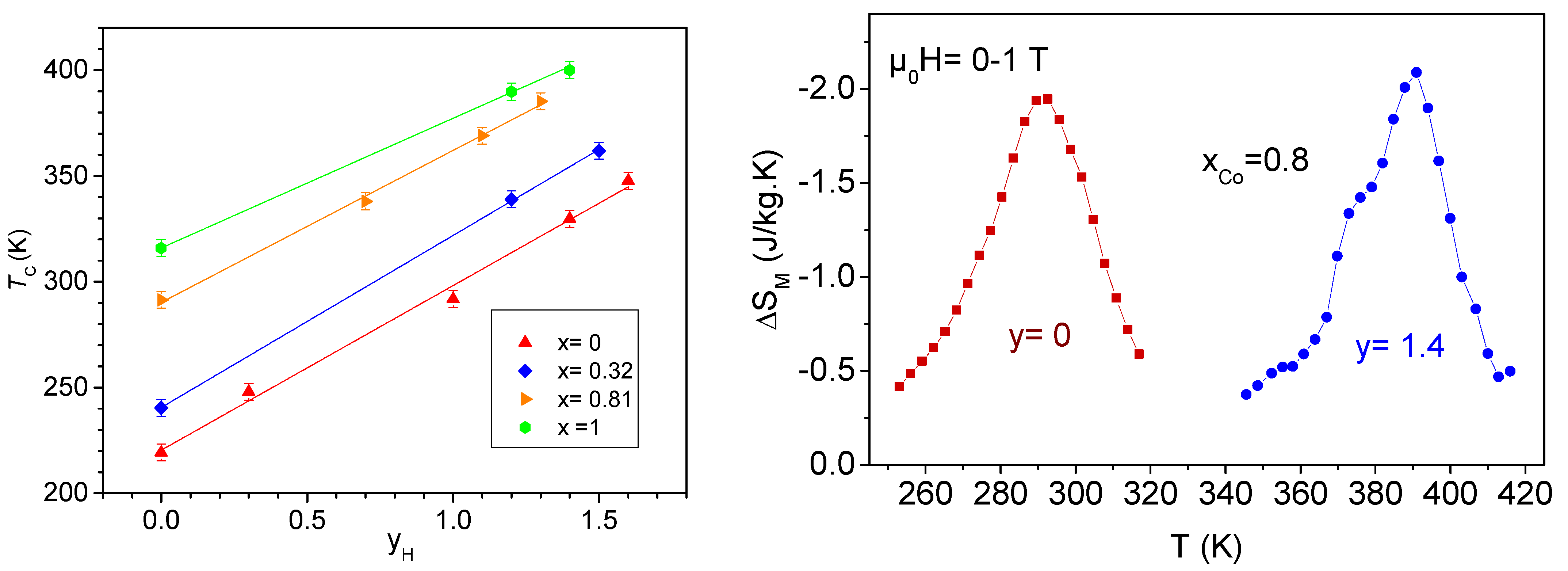
6.1. Hydrogen Insertion

Fujieda et al. [65] and Fujita et al. [35] have shown that the insertion of hydrogen atoms (H) into La(Fe 0.88 Si 0.12 ) 13 significantly increases the Curie temperature, which increases linearly with the hydrogen content while maintaining the first order character of the transition. They have controlled the hydrogen concentration by changing both the annealing temperature and the hydrogen gas pressure. Fujieda et al. [65] adjusted T C to around room temperature (278 K) by hydrogen absorption. Fujita et al. [35] showed that, due to the increase of T 0 , temperature at which the IEM transition disappears, this phenomenon appears above the Curie temperature, Figure 9 (Right). The disappearance of the IEM transition reflects the suppression of the renormalization effect due to the cell volume expansion of the La(Fe 0.88 Si 0.12 ) 13 H y compounds through magnetovolume effects.
Phejar et al. [68] found that the unit cell parameter a increases linearly with hydrogen content, independent of the composition of the parent compound; this result is in agreement with previous results [66,67] (Figure 9-Left). In order to follow the evolution of the crystallographic and magnetic parameters as a function of the temperature, they have performed NPD experiments between 1.5 and 300 K. In order to have a smaller incoherent background and, therefore, a better signal/noise ratio, the hydrogen is replaced by deuterium (D). The La(Fe 0.88 Si 0.12 ) 13 D 0.7 compound exhibited a magnetostrictive effect around T C , resulting in a contraction of the cell parameter at the transition, which is the result of the IEM transition, as shown in Figure 10 (left). The cell parameter of the α -(Fe,Si) inclusions increases, as expected, from a thermal expansion behavior without anomaly at T C . A different behavior has been found by Gebara et al. [69], where an anomalous behavior of α -Fe impurity cell parameter was observed at T C in La(Fe,Si) 13 compounds with La substituted by Ce, Pr, Ho, or Fe by Mn. This means that micro-strains are negligible in the deuteride. Figure 10 (right) shows the magnetic moments of Fe { 96 i } and Fe { 8 b } atoms in the LaFe 11.5 Si 1.5 D 0.7 compound.
Neutron powder diffraction (NDP) experiments have been performed to solve the structure and localize the interstitial site In order to remove ambiguity concerning the interstitial occupied by hydrogen (deuterium) or carbon (24d or 48f site) [68]. Paul-Boncour et al. [68,70] have refined the NDP patterns while using the Rietveld method and have found that the interstitial D(H) was located on the 48f site (Figure 11); this result is in perfect agreement with previous work [67].
Mandal et al. [71] showed that they have succeeded in tuning the Curie temperature from 199 to 346 K, with the hydrogen content without changing significantly Δ S M max value.
Figure 12 (Left) shows the evolution of the Curie temperature for materials in which hydrogen has been inserted as a function of the insertion rate y H taken from different works [35,68,71,72]. Figure 12 (Right) shows the evolution of Δ S M max versus the insertion rate y H for LaFe 13 − x Si x H y [35,68,71]. According to this figure, it can be noticed that, for the Si content 1.3 ≤ x ≤ 1.6 , we obtain a first-order magnetic transition, which results in a larger Δ S M max than for the compositions x ≥ 1.8 , which represent a second-order magnetic transition. Moreover, for x = 1.4 and 1.6, Δ S M max is almost constant for the different hydrogen insertion rates y H .

6.2. Carbon Insertion

Another possibility for increasing unit cell parameters and, thus, the Curie temperature of the material by magneto-volumic effect is the insertion of carbon atoms into the interstitial sites of the structure. This carbon insertion induces a decrease in Δ S M . The insertion of carbon in the material changes the electronic state and, thus, the magnetic properties of the material. The decrease of Δ S M as a function of T C is of the same order as that obtained by the substitution of iron by cobalt: a decrease in entropy of 19 J/kg.K for an increase in T C of 100 K. Thus, it seems that, in this case, the transition also goes from first order to second order.
Li et al. [73] studied the insertion of carbon influences the phase formation, T C , and magnetic entropy change of LaFe 11.7 Si 1.3 . The unit cell parameter a increases with C content, T C increases from 194 to 225 K, and Δ S M was equal to 27.5 J.kg − 1 K − 1 .
Phejar et al. [68] studied the effect of the carbonation of LaFe 13 − x Si x compounds. LaFe 11.5 Si 1.5 C 0.7 compound displays a magnetostrictive effect around T C and a classical thermal expansion for the α -(Fe,Si) impurities as for the deuteride, as shown in Figure 13 (left). Figure 13 (right) shows the evolution of the magnetic moments versus the temperature. The magnetic moment decrease is not as abrupt when compared to the hydrides, which is due to a weakening of the first order transition.

7. Combination of Substitution and Light Element Insertion

Hydrogen insertion in La(Fe 1 − x Si x ) 13 compounds allow for maintaining a large magnetic entropy variation with T C at room temperature (RT). However, several studies have shown that, for H composition lower than 1.5 H/f.u. and with T C close to room temperature, there is a splitting into two hydride phases with low and high H concentrations after few hours or weeks at RT [55,74,75]. The sample is no more homogeneous and the magnetic entropy variation is split in two peaks. In order to avoid this segregation, it is safer to prepare saturated hydrides, but their Curie temperature is around 330–340 K. This temperature can be reduced to RT by partial Mn for Fe substitution for a fully hydrogenated hydride. In this case, the hydride remains stable without desorption or segregation versus time or upon magnetic cycles, despite T C being near RT. A systematic study on the influence of Mn for Fe substitution [76] has revealed that it is possible to vary T C between 260 and 350 K ( 0.1 ≤ x Mn ≤ 0.4 Mn/f.u; Si concentrations: 1.2 and 1.4 Si/f.u. and 1.77 ≤ y H ≤ 1.97 H/f.u.). The drawback is a significant reduction of the Δ S M and Δ T ad values as the Mn content increases significantly [77], but this Mn substitution remains interesting to vary T C in a narrow temperature range, as, in this case, the reduction of Δ S M is limited [78].
Another alternative is to partially substitute La by Ce [79], as, beside the reduction of T C , there is an increase of the MCE effect versus the rate of Ce for La substitution. However, it was found that, in this case, the hydrogenation significantly reduces the MCE effect as compared to that of the parent alloys, which is less beneficial than expected. The influence of Ce substitution for carbides was also investigated, but, despite the larger magnetovolume effect of C versus H insertion, the reduction of Δ S M was more pronounced [50]. Hai et al. [80,81] studied the influence of both C and H insertion on Ce substituted alloys on the hydrogenation kinetic by in-situ neutron diffraction. The preferential insertion of C in 24 d sites introduces a cell distortion and modifies some specific Fe-Fe distances. The presence of C is found to influence the H insertion and to slow down the hydrogenation kinetic. The presence of Ce substituted to La also modifies the kinetic of reaction due to its smaller atomic radius.
Further studies have been undertaken to combine both Ce for La and Mn for Fe substitution with hydrogen insertion [27,82,83]. An adjustment of the rate of both Ce and Mn in La 0.9 Ce 0.1 Fe 11.7 − x Mn x Si 1.3 hydrides allows for maintaining a significant MCE effect near RT. The influence of the bonding with epoxy [27,82] was found to improve the mechanical properties and slightly reduce the MCE effect. High hydrogen pressure sintering on La 0.9 Ce 0.1 Fe 11.7 − x Mn x Si 1.3 hydrides [83] showed a beneficial effect for a pressure of 40 MPa. However, an applied pressure of 50 MPa induced the precipitation of α -Fe reducing the MCE effect.
Beside these works, the influence of Co insertion on the magnetocaloric properties of hydrides has been investigated [70]. The aim was not to maintain T C near RT, but rather to increase it for heat pump applications above room temperature. T C is found to increase linearly versus Co content and H concentration for LaFe 10.5 − x Co x Si 1.5 H y compounds (Figure 14 (Left)), but the d T C / d y slope changes versus Co concentration. T C can be expressed as a function of x and y while taking a negative mixing term into account:
T C ( x , y ) = 210 + 100 · x + 83 · y − 18 · x · y
For x = 1 and y = 1.5 , T C = 400 K, whereas it would have been 450 K without the negative exchange term.
The Co substitution induces a reduction of the magnetic entropy variation, whereas it remain almost constant before and after hydrogenation, as shown for x Co = 0.8 and y = 1.4 (Figure 14 (Right)).

8. Implementation in Active Magnetic Regenerators

The optimization of the chemical composition by the substitution or/and light element insertion in La(Fe,Si) 13 compounds has been reviewed in previous part. But magnetic refrigeration requires also the use of an active magnetic regenerator (AMR) to increase the temperature span ( Δ T span ) by generating a temperature gradient between the hot and cold sources [84]. Many prototypes have been tested while using Gd or its alloys as reference refrigerants in AMR [85]. Although Gd offer many advantages (stability, easy to machine, large Δ T ad , the absence of thermal hysteresis, large thermal conductivity), its elevated cost and limited global reserve constitutes a serious drawback for a broad public application. Therefore, it is necessary to test and optimize other promising magnetocaloric material, such as La(Fe,Si) 13 in AMR. The performances of different regenerators using Gd and La(Fe,Co,Si) 13 material have been compared and discussed in [86,87,88,89]. In all of these prototypes, the AMR were fixed and the magnetic field mobile (rotation of permanent magnets arranged in Halbach cylinder or reciprocating movement). One of the best geometries to favor heat exchange is thin parallel plates (0.25–1 mm) that are separated by a small gap [87,90]. Compared to Gd, which presents broad Δ S M and Δ T ad peaks, La(Fe,Co,Si) 13 compounds display higher but narrower peaks due to their first order transition. In addition, due to its smaller specific heat, Gd has a two time larger Δ T ad than La(Fe,Co,Si) 13 for a field variation around 1 T. To expand the Δ T span , the use of a composite or several plates of La(Fe,Co,Si) 13 compounds with different T C , obtained by varying the Co content, have been therefore tested. Thin plates prepared by TDR method and purchased by Vacuumschmelze GmbH and Co were used in several prototypes [86,87,88]. Other tests were performed with an epoxy-bonded plates [89]. Better performances were obtained while using regenerators with at least four different compositions that were arranged to optimize the thermal gradient, as compared to plates with only one or two different compositions.
Balli et al. [86] built a prototype with two AMRs that are alternatively magnetized and demagnetized in order to reduce the magnetic forces acting on the magnetic refrigerant. The applied magnetic field is parallel to the plates to limit the demagnetization field which is corrected. Working with two AMR, one with Gd and the other with a La(Fe,Co,Si) 13 composite, they obtained a comparable maximum Δ T span of 14 and 16 K for LaFeSi-based and Gd AMR respectively. They have tested the corrosion behavior of La-Fe-Co-Si compounds in different solutions of water with an additional corrosion inhibitor and a silicon oil. The best resistance to corrosion was obtained with 3% of noxal anti-oxidant. Because water has a larger specific heat ( C p = 4.2 J/g K) than Si oil ( C p = 1.6 J/g K), it is more favorable as fluid heat transfer. The study of the corrosion of Gd and La(Fe,Co,Si) 13 in various heat conducting fluids is detailed in Forchelet et al. [91].
Legait et al. [88] compared the performance of Gd, a manganite oxide (Pr 0.65 Sr 0.35 MnO 3 ) and La(Fe,Co) 13 − x Si x compounds under a wide range of fluidic and magnetic operating conditions. Their devices do not use a thermal exchanger, therefore it only provides non-load temperature spans. They have followed the influence of non-dimensional numbers (utilization ratio U and volume ratio V * ) and physical properties, such as thermal conductivity. They observed that each regenerator is most efficient over a specific domain of utilization. A material with low thermal conductivity (manganite) is more efficient at low frequency, whereas the materials with large thermal conductivity are better at a high frequency.
Tusek et al. [87] have tested their AMR with a device that is equipped with a reciprocating magnet and a heat exchanger to investigate the cooling load under different Δ T span . They have observed that the maximum Δ T span varies versus the utilization factor U and the operating frequency P. It is larger for LaFeSi-based AMR when compared to Gd at low U values (moderate Δ T span ), whereas that of Gd increases for larger U values. The maximum Δ T span also depends on the hot side temperature and it is maximum at 35 ∘ C for Gd, 27 ∘ C for a two-layered, and 43 ∘ C for a seven-layered La(Fe,Co,Si) 13 AMR
AMR was also prepared with epoxy-bonded plates that were constituted of a composite of La(Fe,Co) 13 − x Si x powder in a polymer matrix pressed into thin plates [89]. This AMR yields smaller Δ T span than with sintered plates, but it has very good mechanical properties, as it remains stable without significant changes over 90,000 cycles. Because the AMR was constituted with two-layered plates (two different T C ), further improvements can be expected by preparing a AMR with four-layered plates. Beside these experimental results, numerical investigations have been performed through a two-dimensional (2D) model of an AMR refrigerator for various magnetocaloric materials [92,93]. The best performance in terms of temperature spans and coefficient of performance (COP) were obtained for Gd, Gd 5 Si 2 Ge 2 , La(Fe,Mn,Si) 13 H y , and La(Fe,Co,Si) 13 compounds as compared to MnFe(P,As) and manganite oxide.
The thickness of the plates as well as the spacing between the plates, the T C distribution, the intensity of the magnetic field, and the operating frequencies are among the important parameters for optimizing in order to obtain the best cooling performances. Therefore, the properties of AMR with La(Fe,Co,Si) 13 material should be optimized to reach as good AMR performances as Gd. These materials remain very promising for magnetic refrigeration near room temperature application due to their many advantages in term of cost and global reserve.

9. Conclusions

In this review, we have presented the main works dealing with the magnetocaloric effect of LaFe 13 − x Si x . The different methods of synthesis and shaping were presented and compared. The influence of both chemical substitution of La, Fe, and Si by other elements, as well as the insertion of light elements (H, C), were presented to show the advantages and disadvantages of each solutions. Fe for Co substitution or H insertion are considered to be the most efficient way to use these materials as refrigerant near room temperature. A combination of metal substitution and light element insertion is another alternative to adjust their working conditions. La(Fe,Si) 13 type compounds have been implemented in active magnetic regenerators and their performance in magnetic refrigerator prototype when compared with that of Gd.

Author Contributions

Conceptualization, V.P.-B. and L.B.; methodology, V.P.-B. and L.B.; validation, V.P.-B. and L.B.; formal analysis, V.P.-B. and L.B.; investigation, V.P.-B. and L.B.; data curation, V.P.-B. and L.B.; writing—original draft preparation, V.P.-B. and L.B.; writing—review and editing, V.P.-B. and L.B.; visualization, V.P.-B. and L.B. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data available on request due to restrictions eg privacy or ethical.

Acknowledgments

The work of our group was done in the frame of the PhD thesis of M. Phejar and A. Patissier, as well as the master work of K. Nakouri.

Conflicts of Interest

The authors declare no conflict of interest.

Abbreviations

AMRActive Magnetic Regenerator
COPCoefficient of Performance
DSCDifferential Scanning Calorimetry
FMFerromagnetic
IEMItinerant Electron Metamagnetic
MCEMagnetocaloric effect
NPDNeutron Powder Diffraction
PMParamagnetic
RTRoom Temperature
SPSSpark Plasma Sintering
T C Curie Temperature
TDRThermal Decomposition and Recombination
RCPRelative Cooling Power
ZFC-FCZero Field Cooled - Field Cooled

References

  1. Kitanovski, A.; Tusek, J.; Tomc, U.; Plaznik, U.; Ozbolt, M.; Poredos, A. Magnetocaloric Energy Conversion, From Theory to Applications; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
  2. Aprea, C.; Greco, A.; Maiorino, A.; Masselli, C. The employment of caloric-effect materials for solid-state heat pumping. Int. J. Refrig. 2020, 109, 1–11. [Google Scholar] [CrossRef]
  3. Nikitin, S.A.; Myalikgulyev, G.; Tishin, A.M.; Annaorazov, M.P.; Asatryan, K.A.; Tyurin, A.L. The magnetocaloric effect in Fe49Rh51 compound. Phys. Lett. A 1990, 148, 363–366. [Google Scholar] [CrossRef]
  4. Pecharsky, V.K.; Gschneidner, K.A. Giant Magnetocaloric Effect in Gd5(Si2Ge2). Phys. Rev. Lett. 1997, 78, 4494. [Google Scholar] [CrossRef]
  5. Wada, H.; Tomekawa, S.; Shiga, M. Magnetocaloric properties of a first-order magnetic transition system ErCo2. Cryogenics 1999, 39, 915–919. [Google Scholar] [CrossRef]
  6. Wada, H.; Tanabe, Y. Giant magnetocaloric effect of MnAs1-xSbx. Appl. Phys. Lett. 2001, 79, 3302–3304. [Google Scholar] [CrossRef]
  7. Valiev, E.; Gimaev, R.; Zverev, V.; Kamilov, K.; Pyatakov, A.; Kovalev, B.; Tishin, A. Application of the exchange-striction model for the calculation of the FeRh alloys magnetic properties. Intermetallics 2019, 108, 81–86. [Google Scholar] [CrossRef]
  8. Gimaev, R.R.; Vaulin, A.A.; Gubkin, A.F.; Zverev, V.I. Peculiarities of Magnetic and Magnetocaloric Properties of Fe-Rh Alloys in the Range of Antiferromagnet-Ferromagnet Transition. Phys. Metals Metallogr. 2020, 121, 823–850. [Google Scholar] [CrossRef]
  9. Sanchez-Valdas, C.F.; Gimaev, R.R.; Lopez-Cruz, M.; Llamazares, J.L.S.; Zvereve, V.I.; Tishin, A.M.; Carvalho, A.M.G.; Aguiar, D.J.M.; Mudryk, Y.; Pecharsky, V.K. The effect of cooling rate on magnetothermal properties of Fe49Rh51. J. Magn. Magn. Mater. 2020, 498, 166130. [Google Scholar] [CrossRef]
  10. Zarkevich, N.A.; Zverev, V.I. Viable Materials with a Giant Magnetocaloric Effect. Crystals 2020, 10, 815. [Google Scholar] [CrossRef]
  11. Zverev, V.I.; Gimaev, R.R.; Miyanaga, T.; Vaulin, A.A.; Gubkin, A.F.; Kovalev, B.B.; dos Santos, A.M.; Lovell, E.; Cohen, L.F.; Zarkevich, N.A. Peculiarities of the phase transformation dynamics in bulk FeRh based alloys from magnetic and structural measurements. J. Magn. Magn. Mater. 2021, 522, 167560. [Google Scholar] [CrossRef]
  12. Raghavan, V. Fe-La-Si (Iron-Lanthanum-Silicon). J. Phase Equilib. 2001, 22, 158. [Google Scholar] [CrossRef]
  13. Niitsu, K.; Kainuma, R. Phase equilibria in the Fe-La-Si ternary system. Intermetallics 2012, 20, 160–169. [Google Scholar] [CrossRef]
  14. Liu, X.D.; Liu, X.B.; Altounian, Z.; Tu, G.H. Microstructures of (Fe0.88Co0.12)82La7Si11 prepared by arc-melting/melt spinning and subsequent annealing. Appl. Phys. A Mater. 2006, 82, 339–343. [Google Scholar] [CrossRef]
  15. Fujita, A.; Koiwai, S.; Fujieda, S.; Fukamichi, K.; Kobayashi, T.; Tsuji, H.; Kaji, S.; Saito, A.T. Magnetocaloric effect in spherical La(FexSi1-x)13 and their hydrides for active magnetic regenerator-type refrigerator. J. Appl. Phys. 2009, 105, 07A936. [Google Scholar] [CrossRef]
  16. Fujita, A.; Yako, H. Stability of metallic, magnetic and electronic states in NaZn13-type La(FexSi1-x)13 magnetocaloric compounds. Scripta Mater. 2012, 67, 578–583. [Google Scholar] [CrossRef]
  17. Gebara, P.; Pawlik, P.; Skorvanek, I.; Bednarcik, J.; Marcin, J.; Michalik, S.; Donges, J.; Wyslocki, J.J.; Michalski, B. Effect of Al content on the order of phase transition and magnetic entropy change in LaFe11Co0.8(Si1-xAlx)1.2 alloys. J. Magn. Magn. Mater. 2014, 372, 201–207. [Google Scholar] [CrossRef]
  18. Liu, T.; Chen, Y.G.; Tang, Y.B.; Xiao, S.F.; Zhang, E.Y.; Wang, J.W. Structure and magnetic properties of shortly high temperature annealing LaFe11.6Si1.4 compound. J. Alloys Compd. 2009, 475, 672–675. [Google Scholar] [CrossRef]
  19. Gutfleisch, O.; Yan, A.; Muller, K.H. Large magnetocaloric effect in melt-spun LaFe13-xSix. J. Appl. Phys. 2005, 97, 10M305. [Google Scholar] [CrossRef]
  20. Liu, X.B.; Liu, X.D.; Altounian, Z.; Tu, G.H. Phase formation and structure in rapidly quenched La(Fe0.88Co0.12)13-xSix alloys. J. Alloys Compd. 2005, 397, 120–125. [Google Scholar] [CrossRef]
  21. Liu, X.B.; Liu, X.D.; Altounian, Z. Phase formation and magnetocaloric effect in rapidly quenched La(Fe1-xCox)11.4Si1.6. J. Appl. Phys. 2005, 98, 113904. [Google Scholar] [CrossRef]
  22. Yan, A. Structure and magnetocaloric effect in melt-spun La (Fe,Si)13 and MnFePGe compounds. Rare Met. 2006, 25, 544–549. [Google Scholar] [CrossRef]
  23. Lyubina, J.; Gutfleisch, O.; Kuzmin, M.D.; Richter, M. La(Fe,Si)13-based magnetic refrigerants obtained by novel processing routes. J. Magn. Magn. Mater. 2008, 320, 2252–2258. [Google Scholar] [CrossRef]
  24. Dong, J.D.; Yan, A.R.; Liu, J. Microstructure and magnetocaloric properties of melt-extracted La-Fe-Si microwires. J. Magn. Magn. Mater. 2014, 357, 73–76. [Google Scholar] [CrossRef]
  25. Liu, J.; Zhang, P.N.; Dai, F.P.; Yan, A.R. A new approach to prepare spherical La-Fe-Si-Co magnetocaloric refrigerant particles. Scripta Mater. 2013, 69, 485–488. [Google Scholar] [CrossRef]
  26. Fujita, A.; Nakayama, Y.; Kano, M.; Matsunami, D. Improvement of low-field magnetic entropy change by increasing Fe concentration in solid-state reactive sintered La(FexSi1-x)13. J. Alloys Compd. 2014, 601, 158–161. [Google Scholar] [CrossRef]
  27. Mayer, C.; Dubrez, A.; Pierronnet, M.; Vikner, P. Towards the large scale production of (La1-zCez)(Fe1-x-yMnySix)13H-n products for room temperature refrigeration. Phys. Status Solidi C. 2014, 11, 1059–1063. [Google Scholar] [CrossRef]
  28. Katter, M.; Zellmann, V.; Reppel, G.W.; Uestuener, K. Magnetocaloric Properties of La(Fe,Co,Si)13 Bulk Material Prepared by Powder Metallurgy. IEEE Trans. Magn. 2008, 44, 3044–3047. [Google Scholar] [CrossRef]
  29. Katter, M.; Zellmann, V.; Barcza, A. Sintering behavior and thermally induced decomposition and recomposition processe of LaFe13-x-yCoxSiy. In Proceedings of the Fourth IIF-IIR International Conference on Magnetic Refrigeration at Room Temperature, Thermag IV, IIF-IIR, Baotou, China, 23–27 August 2010. [Google Scholar]
  30. Phejar, M.; Paul-Boncour, V.; Bessais, L. Structural and magnetic properties of magnetocaloric LaFe13-xSix compounds synthesized by high energy ball-milling. Intermetallics 2010, 18, 2301–2307. [Google Scholar] [CrossRef]
  31. Patissier, A.; Paul-Boncour, V. Fast synthesis of LaFe13-xSix magnetocaloric compounds by reactive Spark Plasma Sintering. J. Alloys Compd. 2015, 645, 143–150. [Google Scholar] [CrossRef]
  32. Moore, J.D.; Klemm, D.; Lindackers, D.; Grasemann, S.; Trager, R.; Eckert, J.; Lober, L.; Scudino, S.; Katter, M.; Barcza, A.; et al. Selective laser melting of La(Fe,Co,Si)13 geometries for magnetic refrigeration. J. Appl. Phys. 2013, 114, 043907. [Google Scholar] [CrossRef]
  33. Paul-Boncour, V.; Pattisier, A.; Nakouri, K.; Bessais, L. Magnetocaloric compounds prepared by reactive spark plasma sintering. In Proceedings of the 7th International Conference on Caloric Cooling (Thermag VII), Turin, Italy, 11–14 September 2016. [Google Scholar]
  34. Niitsu, K.; Fujieda, S.; Fujita, A.; Kainuma, R. Microstructure and magnetic properties of as-quenched cubic and tetragonal La(Fe1-xSix)13 compounds. J. Alloys Compd. 2013, 578, 220–227. [Google Scholar] [CrossRef]
  35. Fujita, A.; Fujieda, S.; Hasegawa, Y.; Fukamichi, K. Itinerant-electron metamagnetic transition and large magnetocaloric effects in La(FexSi1-x)13 compounds and their hydrides. Phys. Rev. B 2003, 67, 104416. [Google Scholar] [CrossRef] [Green Version]
  36. Moore, D.; Morrison, K.; Sandeman, K.G.; Katter, M.; Cohen, L.F. Reducing extrinsic hysteresis in first-order La(Fe,Co,Si)13 magnetocaloric systems. Appl. Phys. Lett. 2009, 95, 252504. [Google Scholar] [CrossRef] [Green Version]
  37. Yako, H.; Fujieda, S.; Fujita, A.; Fukamichi, K. Influence of Demagnetization Effect on the Kinetics of the Itinerant Electron Metamagnetic Transition in Magnetic Refrigerant La(Fe0.88Si0.12)13. IEEE Trans. Magn. 2011, 47, 2482–2485. [Google Scholar] [CrossRef]
  38. Lovell, E.; Pereira, A.M.; Caplin, A.D.; Lyubina, J.; Cohen, L.F. Dynamics of the First-Order Metamagnetic Transition in Magnetocaloric La(Fe,Si)13: Reducing Hysteresis. Adv. Energy Mater. 2015, 5, 1401639. [Google Scholar] [CrossRef]
  39. Cohen, L.F. Contributions to Hysteresis in Magnetocaloric Materials. Phys. Status Solidi B 2018, 255, 1700317. [Google Scholar] [CrossRef]
  40. Lovell, E.; Morrison, K.; Pereira, A.M.; Caplin, A.D.; Gutfleisch, O.; Cohen, L.F. Scanning Hall Probe Imaging of LaFe13-xSix. Adv. Sci. Tech. 2014, 93, 219–224. [Google Scholar] [CrossRef]
  41. Ido, H.; Sohn, J.C.; Pourarian, F.; Cheng, S.F.; Wallace, W.E. Magnetic properties of LaCo13-based systems. J. Appl. Phys. 1990, 67, 4978. [Google Scholar] [CrossRef]
  42. Hu, F.X.; Shen, B.G.; Sun, J.R.; Wang, G.J.; Cheng, Z.H. Very large magnetic entropy change near room temperature in LaFe11.2Co0.7Si1.1. Appl. Phys. Lett. 2002, 80, 826–828. [Google Scholar] [CrossRef]
  43. Bjork, R.; Bahl, C.R.H.; Katter, M. Magnetocaloric properties of LaFe13-x-yCoxSiy and commercial grade Gd. J. Magn. Magn. Mater. 2010, 322, 3882–3888. [Google Scholar] [CrossRef] [Green Version]
  44. Hansen, B.R.; Kuhn, L.T.; Bahl, C.R.H.; Lundberg, M.; Ancona-Torres, C.; Katter, M. Properties of magnetocaloric La(Fe,Co,Si)13 produced by powder metallurgy. J. Magn. Magn. Mater. 2010, 322, 3447–3454. [Google Scholar] [CrossRef]
  45. Hu, F.X.; Qian, X.L.; Sun, J.R.; Wang, G.J.; Zhang, X.X.; Cheng, Z.H.; Shen, B.G. Magnetic entropy change and its temperature variation in compounds La(Fe1-xCox)11.2Si1.8. J. Appl. Phys. 2002, 92, 3620–3623. [Google Scholar] [CrossRef]
  46. Balli, M.; Fruchart, D.; Sari, O.; Gignoux, D.; Huang, J.H.; Hu, J.; Egolf, P.W. Direct measurement of the magnetocaloric effect on La(Fe13-x-yCoy)Six. J. Appl. Phys. 2009, 106, 023902. [Google Scholar] [CrossRef]
  47. Ilyn, M.; Tishin, A.; Hu, F.; Gao, J.; Sun, J.; Shen, B. Magnetocaloric properties of the LaFe11.7Si1.3 and LaFe11.2Co0.7Si1.1 systems. J. Magn. Magn. Mater. 2005, 290, 712. [Google Scholar] [CrossRef]
  48. Yan, A.; Muller, K.H.; Gutfleish, O. Magnetocaloric properties of the LaFe11.7Si1.3 and LaFe11.2Co0.7Si1.1 systems. J. Alloys Compd. 2008, 450, 18. [Google Scholar] [CrossRef]
  49. Dong, Q.Y.; Zhang, H.W.; Zhao, T.Y.; Sun, J.R.; Shen, B.G. Realization of a small hysteresis loss and a large magnetic entropy change in NaZn13-type La-Fe-Si compound. Solid State Commun. 2008, 147, 266–270. [Google Scholar] [CrossRef]
  50. Zhang, H.; Shen, J.; Xu, Z.Y.; Zheng, X.Q.; Hu, F.X.; Sun, J.R.; Shen, B.G. Simultaneous enhancements of Curie temperature and magnetocaloric effects in the La1-xCexFe11.5Si1.5Cy compounds. J. Magn. Magn. Mater. 2013, 324, 484–487. [Google Scholar] [CrossRef]
  51. Hu, F.X.; Gao, J.; Qian, X.L.; Ilyn, M.; Tishin, A.M.; Sun, J.R.; Shen, B.G. Magnetocaloric effect in itinerant electron metamagnetic systems La(Fe1-xCox)11.9Si1.1. J. Appl. Phys. 2005, 97, 10M303. [Google Scholar] [CrossRef] [Green Version]
  52. Paul-Boncour, V.; Nakouri, K.; Pattisier, A.; Bessais, L. Fast synthesis and magnetocaloric properties of La(Fe, Co, Si)13 compounds and their hydrides. In Proceedings of the 8th International Conference on Caloric Cooling (Thermag VIII), Darmstadt, Germany, 16–20 September 2018; pp. 593–598. [Google Scholar]
  53. Gebara, P.; Pawlik, P. Broadening of temperature working range inmagnetocaloric La(Fe,Co,Si)13- based multicomposite. J. Magn. Magn. Mater. 2017, 442, 145–151. [Google Scholar] [CrossRef]
  54. Pathak, A.K.; Basnyat, P.; Dubenko, I.; Stadler, S.; Ali, N. Influence of the small substitution of Z=Ni, Cu, Cr, V for Fe on the magnetic, magnetocaloric, and magnetoelastic properties of LaFe11.4Si1.6. J. Magn. Magn. Mater. 2010, 322, 692–697. [Google Scholar] [CrossRef]
  55. Barcza, A.; Katter, M.; Zellmann, V.; Russek, S.; Jacobs, S.; Zimm, C. Stability and Magnetocaloric Properties of Sintered La(Fe,Mn,Si)13Hz Alloys. IEEE Trans. Magn. 2011, 47, 3391–3394. [Google Scholar] [CrossRef]
  56. Anh, D.T.K.; Thuy, N.P.; Duc, N.H.; Nhien, T.T.; Nong, N.V. Magnetism and magnetocaloric effect in La1-yNdy(Fe0.88Si0.12)13 compounds. J. Magn. Magn. Mater. 2003, 262, 427–431. [Google Scholar]
  57. Fujita, A.; Fujieda, S.; Fukamichi, K. Control of Magnetocaloric Effects by Partial Substitution in Itinerant-Electron Metamagnetic La(FexSi1-x)13 for Application to Magnetic Refrigeration. IEEE Trans. Magn. 2009, 45, 2620–2625. [Google Scholar] [CrossRef]
  58. Fujita, A.; Fujieda, S.; Fukamichi, K. Changes in electronic states and magnetic free energy in La1-zCez(FexSi1-x )13 magnetic refrigerants. J. Phys. D Appl. Phys. 2011, 44, 064013. [Google Scholar] [CrossRef]
  59. Fujieda, S.; Fujita, A.; Fukamichi, K. Enhancements of magnetocaloric effects in La(Fe0.90Si0.10)13 and its hydride by partial substitution of Ce for La. Mater. Trans. 2004, 45, 3228–3231. [Google Scholar] [CrossRef]
  60. Fujieda, S.; Fujita, A.; Fukamichi, K.; Hirano, N.; Nagaya, S. Large magnetocaloric effects enhanced by partial substitution of Ce for La in La(Fe0.88Si0.12)13 compound. J. Alloys Compd. 2006, 408, 1165–1168. [Google Scholar] [CrossRef]
  61. Shen, B.G.; Sun, J.R.; Hu, F.X.; Zhang, H.W.; Cheng, Z.H. Recent Progress in Exploring Magnetocaloric Materials. Adv. Mater. 2009, 21, 4545–4564. [Google Scholar] [CrossRef] [Green Version]
  62. Shen, J.; Li, Y.X.; Sun, J.R.; Shen, B.G. Effect of R substitution on magnetic properties and magnetocaloric effects of La1-xRxFe11.5Si1.5 compounds with R=Ce, Pr and Nd. Chin. Phys. B 2009, 18, 2058–2062. [Google Scholar]
  63. Gebara, P.; Kovac, J. Magnetocaloric effect of the LaFe11.2Co0.7Si1.1 modified by partial substitution of La by Pr or Ho. Mater. Des. 2017, 129, 111–115. [Google Scholar] [CrossRef]
  64. Gebara, P.; Kovac, J. The influence of partial substitution of La by Dy on structure and thermomagnetic properties of the LaFe11.0Co0.7Si1.3 alloy. J. Magn. Magn. Mater. 2018, 454, 298–303. [Google Scholar] [CrossRef]
  65. Fujieda, S.; Fujita, A.; Fukamichi, K.; Yamazaki, Y.; Iijima, Y. Giant isotropic magnetostriction of itinerant-electron metamagnetic La(Fe0.88Si0.12)13Hy. Appl. Phys. Lett. 2001, 79, 653–655. [Google Scholar] [CrossRef]
  66. Chen, Y.F.; Wang, F.; Shen, B.G.; Wang, G.J.; Sun, J.R. Magnetism and magnetic entropy change of LaFe11.6Si1.4Cx (x = 0–0.6) interstitial compounds. J. Appl. Phys. 2003, 93, 1323–1325. [Google Scholar] [CrossRef]
  67. Rosca, M.; Balli, M.; Fruchart, D.; Gignoux, D.; Hill, E.K.; Miraglia, S.; Ouladdiaf, B.; Wolfers, P. Neutron diffraction study of LaFe11.31Si1.69 and LaFe11.31Si1.69H1.45 compounds. J. Alloys Compd. 2010, 490, 50–55. [Google Scholar] [CrossRef]
  68. Phejar, M.; Paul-Boncour, V.; Bessais, L. Investigation on structural and magnetocaloric properties of LaFe13-xSix(H,C)y compounds. J. Solid State Chem. 2016, 233, 95–102. [Google Scholar] [CrossRef]
  69. Gebara, P.; Cesnek, M.; Bednarcik, J. Anomalous behavior of thermal expansion of α-Fe impurities in the La(Fe,Co,Si)13- based alloys modified by Mn or selected lanthanides (Ce, Pr, Ho). Curr. Appl. Phys. 2019, 19, 188–192. [Google Scholar] [CrossRef]
  70. Paul-Boncour, V.; Nakouri, K.; Bessais, L. Optimization of Magnetocaloric Properties of Ball-Milled La(Fe,Co,Si)13(H,C)y. In Proceedings of the TMS 2019 148th Annual Meeting and Exhibition, San Antonio, TX, USA, 10–14 March 2019. [Google Scholar]
  71. Mandal, K.; Pal, D.; Gutfleisch, O.; Kerschi, P.; Müller, K. Magnetocaloric effect in reactively-milled LaFe11.57Si1.43Hy intermetalic compounds. J. Appl. Phys. 2007, 102, 053806. [Google Scholar] [CrossRef]
  72. Zhao, J.L.; Shen, J.; Hu, F.X.; Li, Y.X.; Sun, J.R.; Shen, B.G. Reduction of magnetic hysteresis loss in La0.5Pr0.5Fe11.4Si1.6Hx hydrides with large magnetocaloric effects. J. Appl. Phys. 2011, 107, 113911. [Google Scholar] [CrossRef]
  73. Li, J.Q.; Liu, F.S.; Ao, W.Q.; Zhuang, Y.H.; Zhou, K.W. Influence of carbon on the giant magnetocaloric effect of LaFe11.7Si1.3. Rare Met. 2006, 25, 556–561. [Google Scholar] [CrossRef]
  74. Wang, Z.C.; He, L.H.; Cuevas, F.; Shen, M.L.J.; Wang, F.W. Hydrogenation, structure and magnetic properties of La(Fe0.91Si0.09)13 hydrides and deuterides. Chin. Phys. B 2011, 20, 067502. [Google Scholar] [CrossRef]
  75. Baumfeld, O.L.; Gercsi, Z.; Krautz, M.; Gutfleisch, O.; Sandeman, K.G. The dynamics of spontaneous hydrogen segregation in LaFe13-xSixHy. J. Appl. Phys. 2014, 115, 203905. [Google Scholar] [CrossRef] [Green Version]
  76. Krautz, M.; Skokov, K.; Gottschall, T.; Teixeira, C.S.; Waske, A.; Liu, J.; Schultz, J.L.; Gutfleisch, O. Systematic investigation of Mn substituted La(Fe,Si)13 alloys and their hydrides for room-temperature magnetocaloric application. J. Alloys Compd. 2014, 598, 27–32. [Google Scholar] [CrossRef]
  77. Piazzi, M.; Bennati, C.; Curcio, C.; Kuepferling, M.; Basso, V. Modeling specific heat and entropy change in La(Fe-Mn-Si)13-H compounds. J. Magn. Magn. Mater. 2016, 400, 349–355. [Google Scholar] [CrossRef]
  78. Morrison, K.; Sandeman, K.G.; Cohen, L.F.; Sasso, C.P.; Basso, V.; Barcza, A.; Katter, M.; Moore, J.D.; Skokov, K.P.; Gutfleisch, O. Evaluation of the reliability of the measurement of key magnetocaloric properties: A round robin study of La(Fe,Si,Mn)Hδ conducted by the SSEEC consortium of European laboratories. Int. J. Refrig. Rev. Int. Froid 2012, 35, 1528–1536. [Google Scholar] [CrossRef] [Green Version]
  79. Mu, L.; Huang, J.; Zhang, W.; Liu, C.; Wang, G.; Zhao, Z. Influence of partial substitution of cerium for lanthanum on magnetocaloric properties of La1-xCexFe11.44Si1.56 and their hydrides. J. Rare Earth 2014, 32, 1135–1139. [Google Scholar] [CrossRef]
  80. Hai, X.Y.; Mayer, C.; Colin, C.V.; Miraglia, S. In-situ neutron investigation of hydrogen absorption kinetics in La(Fe1-xSix)13 magnetocaloric alloys for room-temperature refrigeration application. J. Magn. Magn. Mater. 2016, 400, 344–348. [Google Scholar] [CrossRef]
  81. Hai, X.Y.; Porcher, F.; Mayer, C.; Miraglia, S. Structural effects in the interstitial solid solution system (La,Ce)(Fe,Si)13CxH: Correlation with hydrogenation kinetics. J. Appl. Phys. 2018, 123, 7. [Google Scholar] [CrossRef]
  82. Xia, W.; Huang, J.; Sun, N.; Lui, C.; Ou, Z.; Song, L. Influence of powder bonding on mechanical properties and magnetocaloric effects of La0.9Ce0.1(Fe,Mn)11.7Si1.3H1.8. J. Alloys Compd. 2015, 635, 124–128. [Google Scholar] [CrossRef]
  83. Sun, N.; Zhang, Y.; Zhao, X.; Guo, J.; Cheng, J.; Huang, J.; Zhang, Z. Microstructure, mechanical and magnetocaloric properties of bulk La0.9Ce0.1Fe11.7-xMnxSi1.3 hydrides prepared by high-hydrogen-pressure sintering. J. Magn. Magn. Mater. 2020, 495, 165889. [Google Scholar] [CrossRef]
  84. Brown, G.V. Magnetic heat pumping near room-temperature. J. Appl. Phys. 1976, 47, 3673. [Google Scholar] [CrossRef] [Green Version]
  85. Rowe, A.; Tura, A. Experimental investigation of a three-material layered active magnetic regenerator. Int. J. Refrig. 2006, 29, 1286–1293. [Google Scholar] [CrossRef]
  86. Balli, M.; Sari, O.; Zamni, L.; Mahmed, C.; Forchelet, J. Implementation of La(Fe, Co)13-xSix materials in magnetic refrigerators: Practical aspects. Mater. Sci. Eng. B Adv. 2012, 177, 629–634. [Google Scholar] [CrossRef]
  87. Tusek, J.; Kitanovski, A.; Poredos, A. Geometrical optimization of packed-bed and parallel-plate active magnetic regenerators. Int. J. Refrig. 2013, 36, 1456–1464. [Google Scholar] [CrossRef]
  88. Legait, U.; Guillou, F.; Kedous-Lebouc, A.; Hardy, V.; Almanza, M. An experimental comparison of four magnetocaloric regenerators using three different materials. Int. J. Refrig. 2014, 37, 147–155. [Google Scholar] [CrossRef]
  89. Pulko, B.; Tusek, J.; Moore, J.D.; Weise, B.; Skokov, K.; Mityashkin, O.; Kitanovski, A.; Favero, C.; Fajfar, P.; Gutfleisch, O.; et al. Epoxy-bonded La-Fe-Co-Si magnetocaloric plates. J. Magn. Magn. Mater. 2015, 375, 65–73. [Google Scholar] [CrossRef]
  90. Vasile, C.; Muller, C. Innovative design of a magnetocaloric system. Int. J. Refrig. 2006, 29, 1318–1326. [Google Scholar] [CrossRef]
  91. Forchelet, J.; Zamni, L.; El Alami, S.E.; Hu, J.; Balli, M.; Sari, O. Corrosion behavior of gadolinium and La-Fe-Co-Si compounds in various heat conducting fluids. Int. J. Refrig. 2014, 37, 307–313. [Google Scholar] [CrossRef]
  92. Aprea, C.; Greco, A.; Maiorino, A.; Masselli, C. Analyzing the energetic performances of AMR regenerator working with different magnetocaloric materials: Investigations and viewpoints. Int. J. Heat Technol. 2017, 35, S383–S390. [Google Scholar] [CrossRef]
  93. Aprea, C.; Greco, A.; Maiorino, A.; Masselli, C. The environmental impact of solid-state materials working in an active caloric refrigerator compared to a vapor compression cooler. Int. J. Heat Technol. 2018, 36, 1155–1162. [Google Scholar] [CrossRef]
Magnetochemistry 07 00013 g001 550
Figure 1. The crystal structure of the cubic NaZn 13 phase. The La atom occupy the 8 a sites (Na), whereas the Fe atoms are located on the 8 b and 96 i sites (Zn). In the right figure: one Fe ( 8 b ) atom is surrounded by 10 Fe ( 96 i ) and 8 La ( 8 a ). The Si atoms are substituted in the 96 i position.
Figure 1. The crystal structure of the cubic NaZn 13 phase. The La atom occupy the 8 a sites (Na), whereas the Fe atoms are located on the 8 b and 96 i sites (Zn). In the right figure: one Fe ( 8 b ) atom is surrounded by 10 Fe ( 96 i ) and 8 La ( 8 a ). The Si atoms are substituted in the 96 i position.
Magnetochemistry 07 00013 g001
Magnetochemistry 07 00013 g002 550
Figure 2. Curie temperature evolution of compounds for which Fe has been partially substituted by Co (a) [46], (b) [47], (c) [48], (d) [49], (e) [18], (f) [50], (g) [21], and (h) [51].
Figure 2. Curie temperature evolution of compounds for which Fe has been partially substituted by Co (a) [46], (b) [47], (c) [48], (d) [49], (e) [18], (f) [50], (g) [21], and (h) [51].
Magnetochemistry 07 00013 g002
Magnetochemistry 07 00013 g003 550
Figure 3. Relative magnetic entropy evolution as a function of T C for materials in which Fe iron has been substituted by Co, compared to the compound without Co. (a) [46], (b) [47], (c) [48], (d) [49], (e) [18], and (f) [50].
Figure 3. Relative magnetic entropy evolution as a function of T C for materials in which Fe iron has been substituted by Co, compared to the compound without Co. (a) [46], (b) [47], (c) [48], (d) [49], (e) [18], and (f) [50].
Magnetochemistry 07 00013 g003
Magnetochemistry 07 00013 g004 550
Figure 4. (a) Unit cell volume vs. Co content, (b) T C vs. Co content, for LaFe 11.5 − x Co x Si 1.5 [52].
Figure 4. (a) Unit cell volume vs. Co content, (b) T C vs. Co content, for LaFe 11.5 − x Co x Si 1.5 [52].
Magnetochemistry 07 00013 g004
Magnetochemistry 07 00013 g005 550
Figure 5. Magnetic Δ S M variations for different x Co content for LaFe 11.5 − x Co x Si 1.5 [52].
Figure 5. Magnetic Δ S M variations for different x Co content for LaFe 11.5 − x Co x Si 1.5 [52].
Magnetochemistry 07 00013 g005
Magnetochemistry 07 00013 g006 550
Figure 6. Evolution of the Curie temperature T C and the weighted average hyperfine field < B HF > versus x Co content [52].
Figure 6. Evolution of the Curie temperature T C and the weighted average hyperfine field < B HF > versus x Co content [52].
Magnetochemistry 07 00013 g006
Magnetochemistry 07 00013 g007 550
Figure 7. (Left) Evolution of T C vs. unit cell parameter, (Right) Δ S M max vs. T C , for La 1 − z R z (Fe 0.88 Si 0.12 ) 13 (R = Ce, Pr, Nd) [57,58,59,60,61,62].
Figure 7. (Left) Evolution of T C vs. unit cell parameter, (Right) Δ S M max vs. T C , for La 1 − z R z (Fe 0.88 Si 0.12 ) 13 (R = Ce, Pr, Nd) [57,58,59,60,61,62].
Magnetochemistry 07 00013 g007
Magnetochemistry 07 00013 g008 550
Figure 8. Δ S M max vs. R content z, for La 1 − z R z Fe 11.5 Si 1.5 (R = Nd, Pr) [61,62].
Figure 8. Δ S M max vs. R content z, for La 1 − z R z Fe 11.5 Si 1.5 (R = Nd, Pr) [61,62].
Magnetochemistry 07 00013 g008
Magnetochemistry 07 00013 g009 550
Figure 9. (Left) Unit cell parameter a vs. hydrogen content [66,67]. (Right) Magnetic phase diagram of LaFe 11.44 Si 1.56 H y . The IEM transition occurs between T 0 (the temperature at which the IEM transition disappears) and T C [35].
Figure 9. (Left) Unit cell parameter a vs. hydrogen content [66,67]. (Right) Magnetic phase diagram of LaFe 11.44 Si 1.56 H y . The IEM transition occurs between T 0 (the temperature at which the IEM transition disappears) and T C [35].
Magnetochemistry 07 00013 g009
Magnetochemistry 07 00013 g010 550
Figure 10. (Left) Evolution of the deuteride LaFe 11.5 Si 1.5 D 0.7 cell parameter versus temperature derived from neutron diffraction. (Right) Magnetic moments of Fe atoms versus temperature, in the 96 i and 8 b crystallographic sites [68].
Figure 10. (Left) Evolution of the deuteride LaFe 11.5 Si 1.5 D 0.7 cell parameter versus temperature derived from neutron diffraction. (Right) Magnetic moments of Fe atoms versus temperature, in the 96 i and 8 b crystallographic sites [68].
Magnetochemistry 07 00013 g010
Magnetochemistry 07 00013 g011 550
Figure 11. The unit cell of LaFe 11.5 Si 1.5 D 0.7 projected along the ( a , c ) plane.
Figure 11. The unit cell of LaFe 11.5 Si 1.5 D 0.7 projected along the ( a , c ) plane.
Magnetochemistry 07 00013 g011
Magnetochemistry 07 00013 g012 550
Figure 12. (Left) Evolution of the Curie temperature for materials in which hydrogen has been inserted vs. the insertion rate y H (a) [72], (b) [35], (c) [71], (d) [68], and (e) [70]. (Right) Δ S M max vs. the insertion rate y H for LaFe 13 − x Si x H y (a) [35], (b) [71], and (c) [68].
Figure 12. (Left) Evolution of the Curie temperature for materials in which hydrogen has been inserted vs. the insertion rate y H (a) [72], (b) [35], (c) [71], (d) [68], and (e) [70]. (Right) Δ S M max vs. the insertion rate y H for LaFe 13 − x Si x H y (a) [35], (b) [71], and (c) [68].
Magnetochemistry 07 00013 g012
Magnetochemistry 07 00013 g013 550
Figure 13. Unit cell parameter a vs. temperature for LaFe 11.5 Si 1.5 C 0.7 (left). Magnetic moments of Fe atoms vs. temperature, in the 96 i and 8 b crystallographic sites (right). The results are obtained from neutron diffraction [68].
Figure 13. Unit cell parameter a vs. temperature for LaFe 11.5 Si 1.5 C 0.7 (left). Magnetic moments of Fe atoms vs. temperature, in the 96 i and 8 b crystallographic sites (right). The results are obtained from neutron diffraction [68].
Magnetochemistry 07 00013 g013
Magnetochemistry 07 00013 g014 550
Figure 14. (Left) Evolution of T C versus H concentration for different Co rates, (Right) Comparison of Δ S M for LaFe 9.7 Co 0.8 Si 1.5 H y ( y = 0 and 1.4) and a field variation 0-1 T.
Figure 14. (Left) Evolution of T C versus H concentration for different Co rates, (Right) Comparison of Δ S M for LaFe 9.7 Co 0.8 Si 1.5 H y ( y = 0 and 1.4) and a field variation 0-1 T.
Magnetochemistry 07 00013 g014
Table
Table 1. Crystallographic data of the La(Fe,Si) 13 phases [34].
Table 1. Crystallographic data of the La(Fe,Si) 13 phases [34].
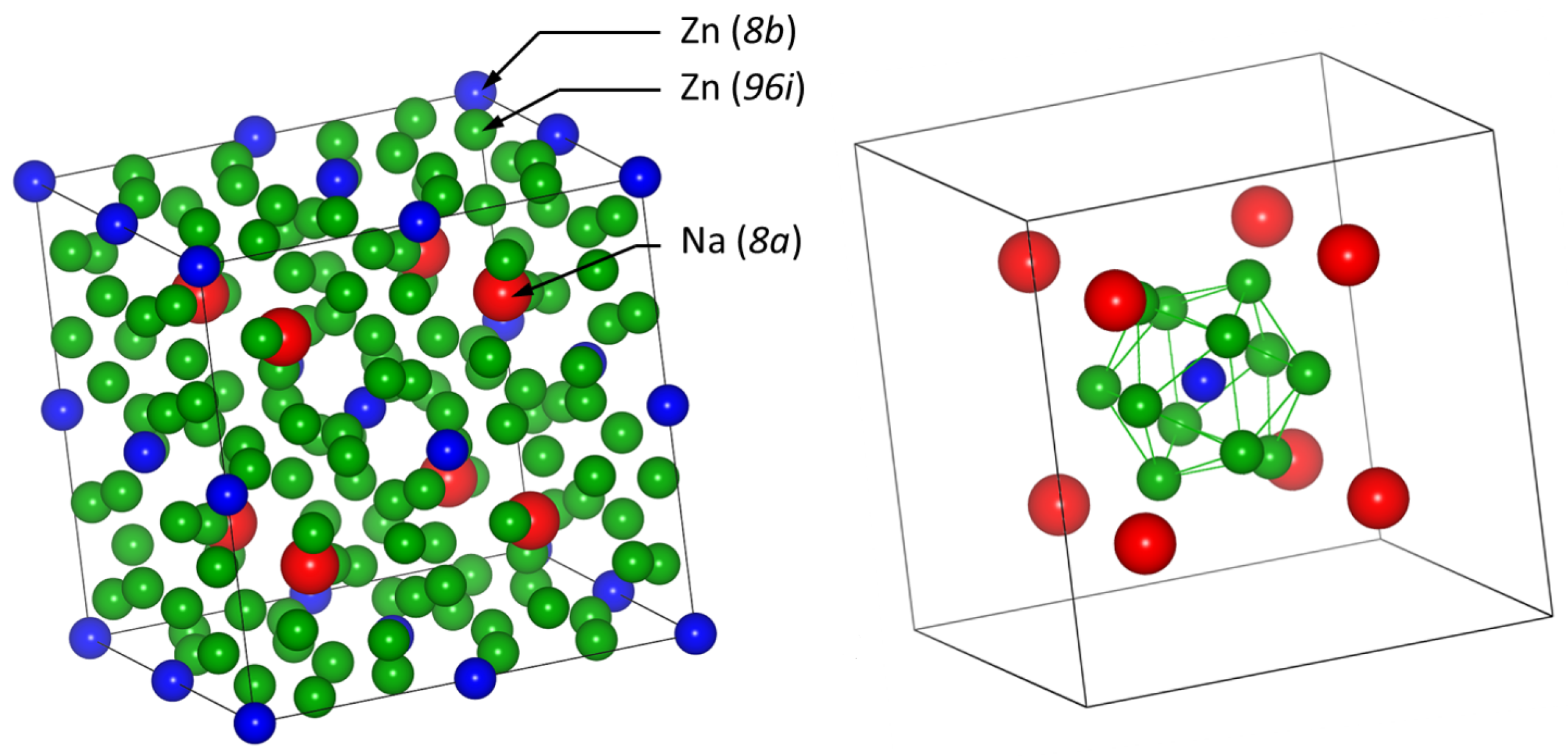
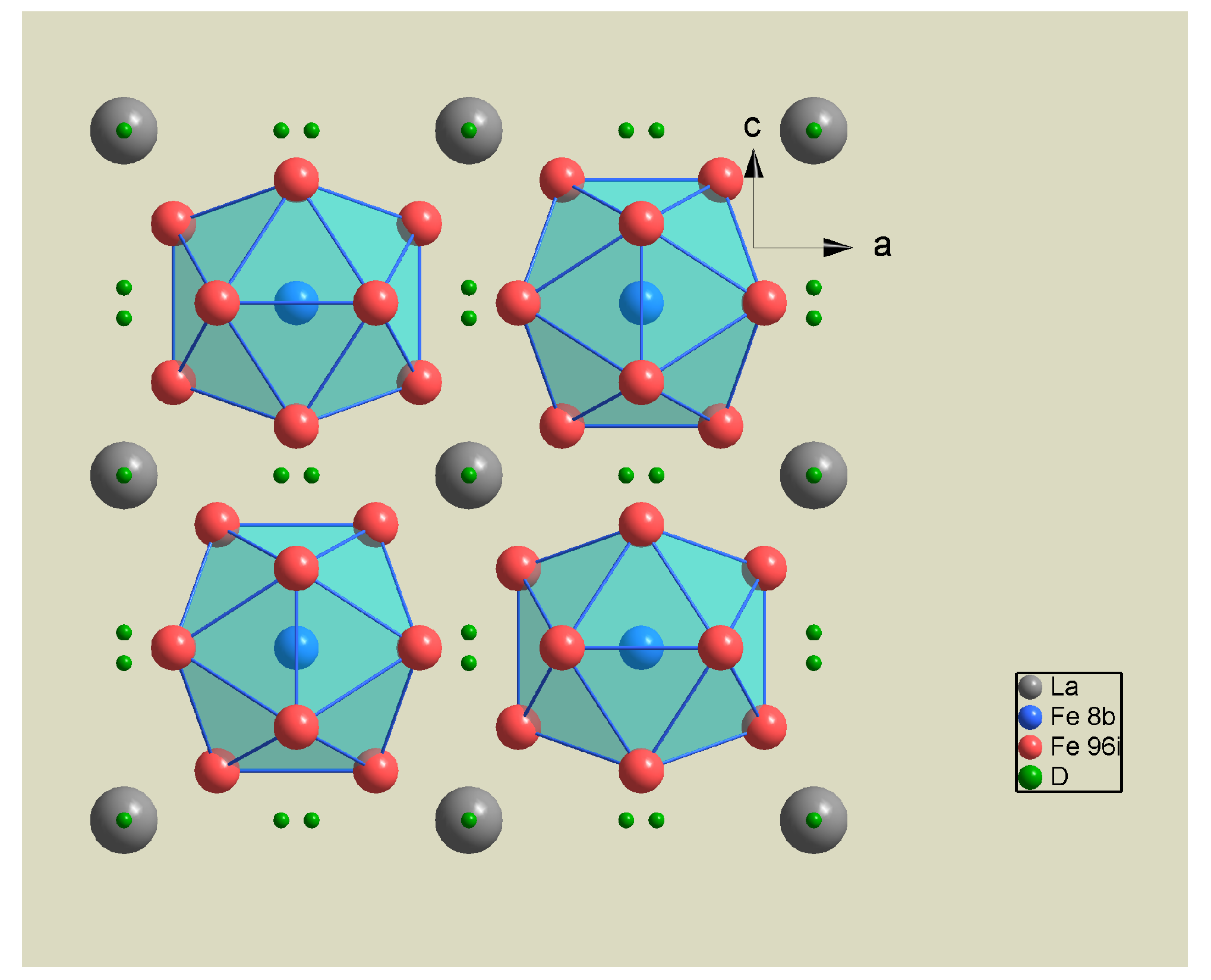
PhasePearsonSpace GroupAtm.Wyck.Sym.Position
NaZn 13 cF112 F m 3 ¯ c La 8 a 4321/4, 1/4, 1/4
Fe 8 b m 3 ¯ 0, 0, 0
Fe, Si 96 i m . . 0, 0.1806, 0.1192
Ce 2 Ni 17 Si 9 tI56 I 4 / m c m La 4 a 4220, 0, 1/4
Fe1 4 d m . m m 0, 1/2, 0
Fe2 16 k m . . 0.2024, 0.0691, 0
Fe3 16 l . . m 0.1294, 0.6294, 0.1832
Si 16 l * . . m 0.33, 0.83, 0.118
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Paul-Boncour, V.; Bessais, L. Tuning the Magnetocaloric Properties of the La(Fe,Si)13 Compounds by Chemical Substitution and Light Element Insertion. Magnetochemistry 2021, 7, 13. https://doi.org/10.3390/magnetochemistry7010013

AMA Style

Paul-Boncour V, Bessais L. Tuning the Magnetocaloric Properties of the La(Fe,Si)13 Compounds by Chemical Substitution and Light Element Insertion. Magnetochemistry. 2021; 7(1):13. https://doi.org/10.3390/magnetochemistry7010013

Chicago/Turabian Style

Paul-Boncour, Valérie, and Lotfi Bessais. 2021. "Tuning the Magnetocaloric Properties of the La(Fe,Si)13 Compounds by Chemical Substitution and Light Element Insertion" Magnetochemistry 7, no. 1: 13. https://doi.org/10.3390/magnetochemistry7010013

APA Style

Paul-Boncour, V., & Bessais, L. (2021). Tuning the Magnetocaloric Properties of the La(Fe,Si)13 Compounds by Chemical Substitution and Light Element Insertion. Magnetochemistry, 7(1), 13. https://doi.org/10.3390/magnetochemistry7010013

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop