Slow Relaxation of Magnetization and Magnetocaloric Effects in One-Dimensional Oxamato-Based Lanthanide(III) Coordination Polymers †
Abstract
:1. Introduction
2. Experimental Section
2.1. General Information
2.2. Synthesis
2.3. Crystallographic Data Collection and Refinement
2.4. Magnetic Measurements
3. Results
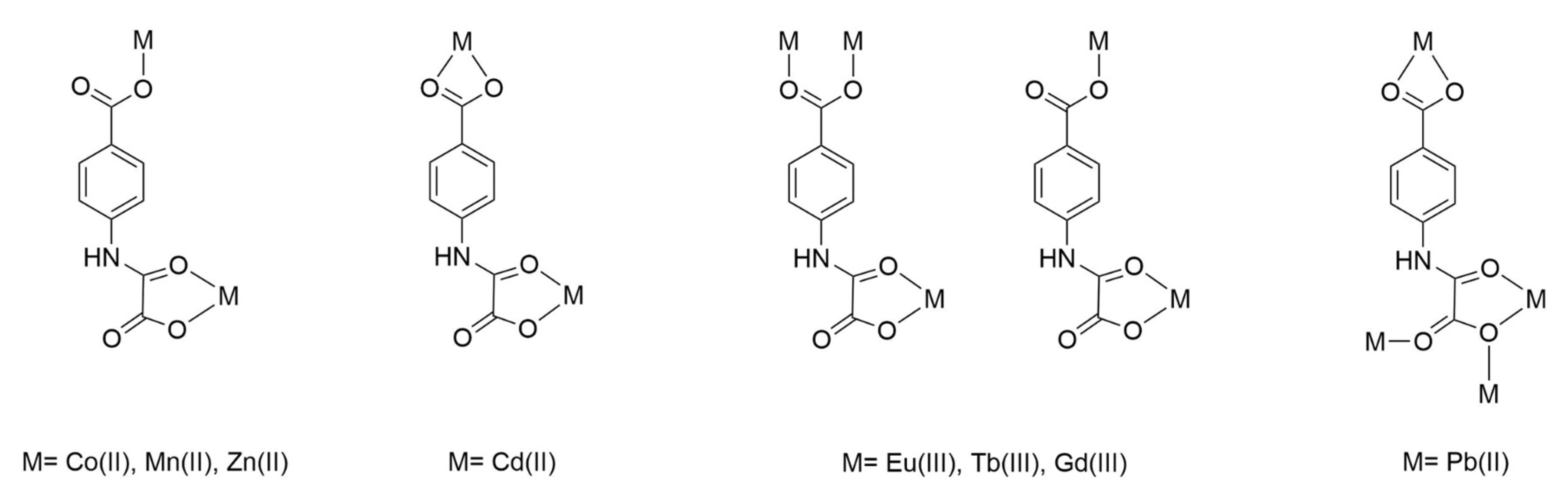
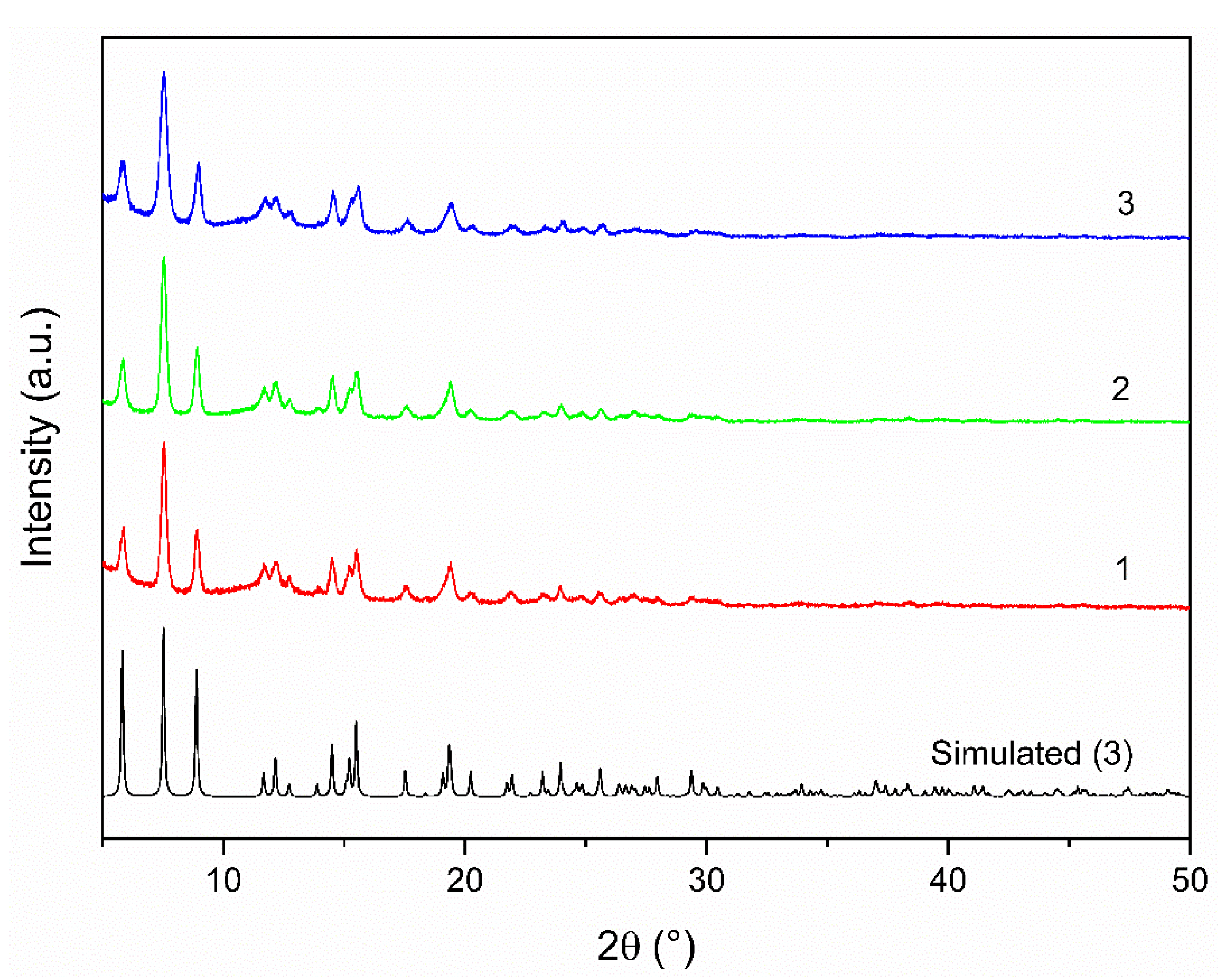
3.1. Syntheses and General Physicochemical Characterization of 1–3
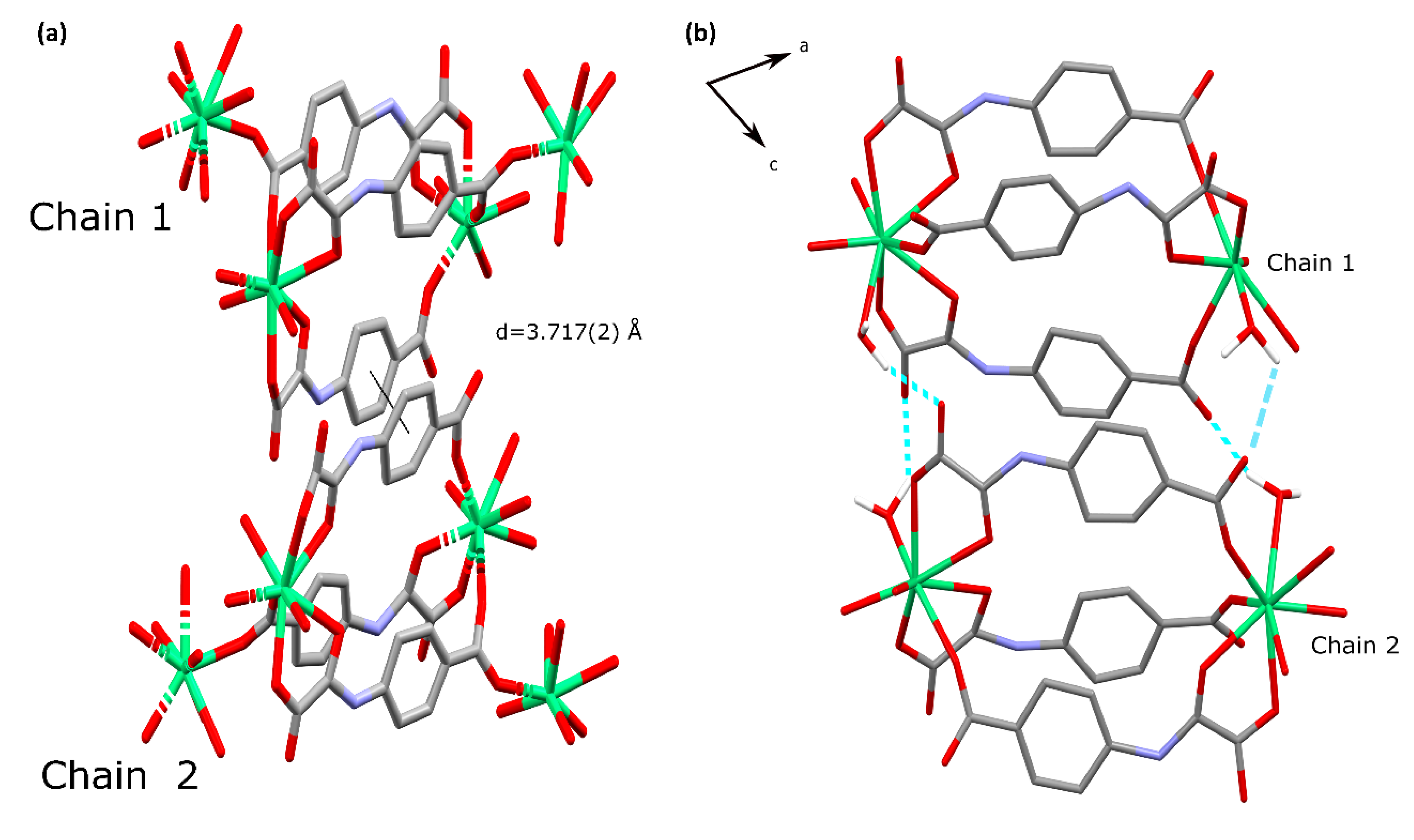
3.2. Description of the Structure of 3
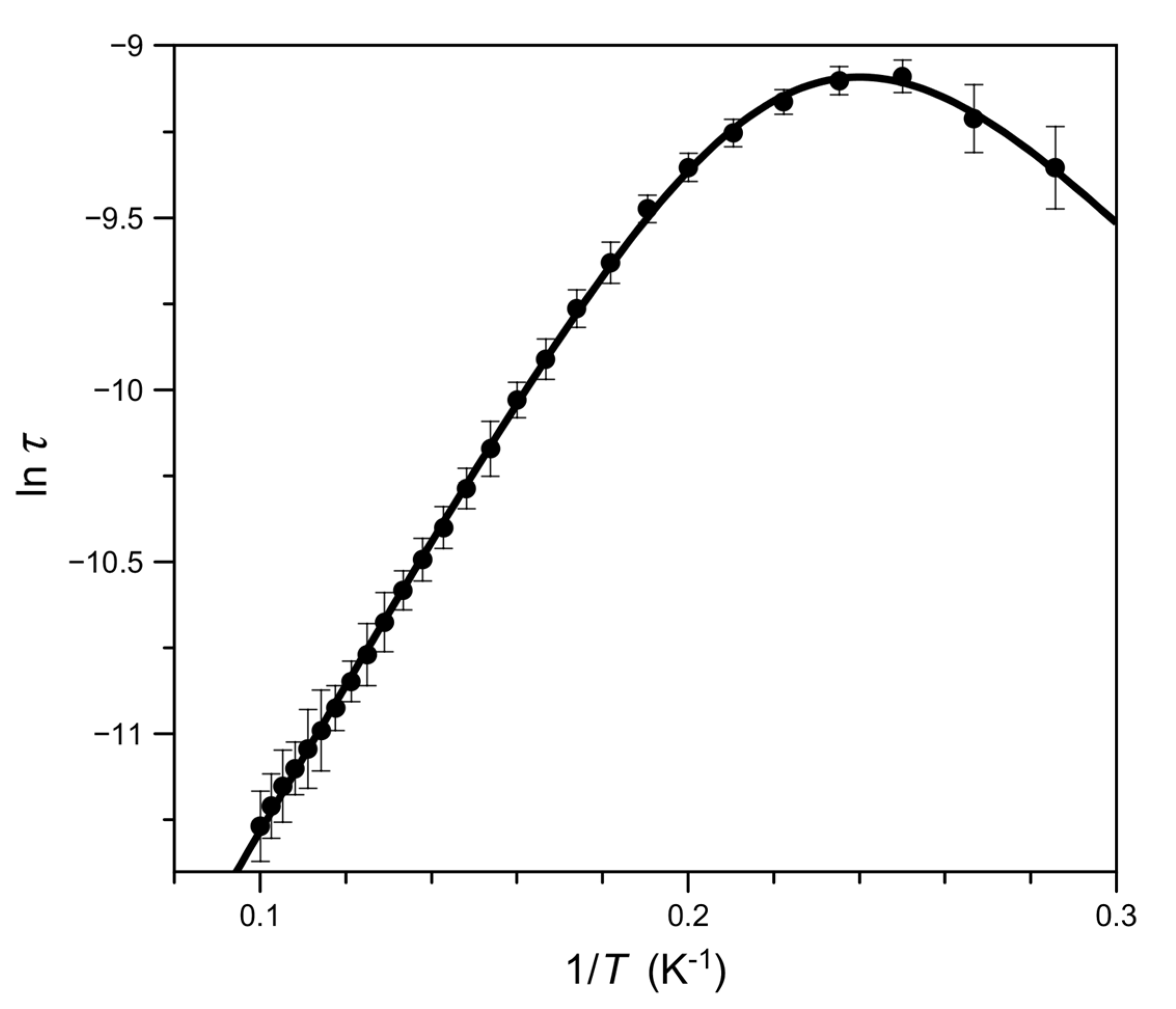
3.3. Magnetic Properties of 1–3
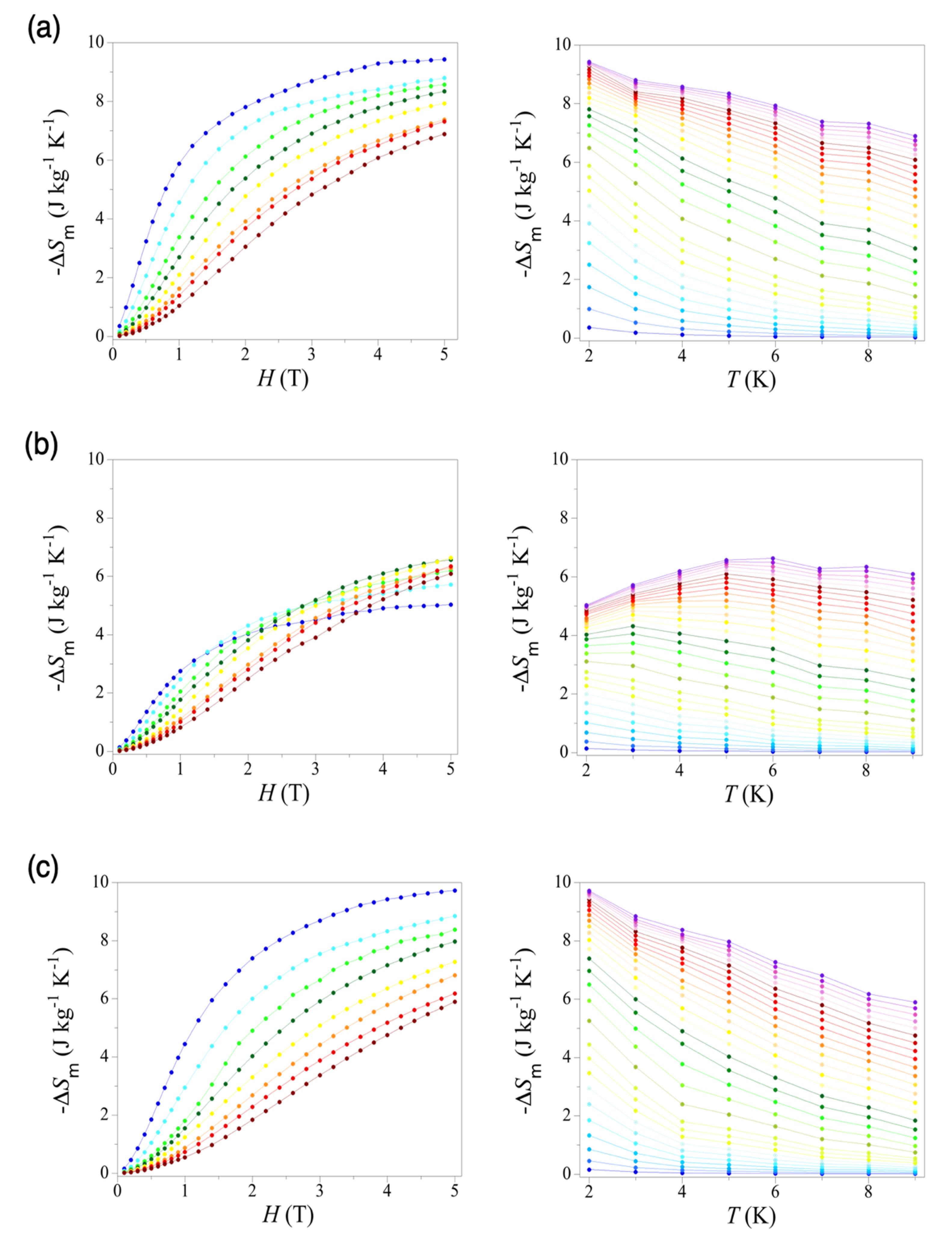
3.4. Magnetocaloric Properties of 1–3
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, T.; Lin, E.; Peng, Y.L.; Chen, Y.; Cheng, P.; Zhang, Z. Rational Design and Synthesis of Ultramicroporous Metal-Organic Frameworks for Gas Separation. Coord. Chem. Rev. 2020, 423, 213485. [Google Scholar] [CrossRef]
- Wang, C.; Liu, X.; Keser Demir, N.; Chen, J.P.; Li, K. Applications of Water Stable Metal–Organic Frameworks. Chem. Soc. Rev. 2016, 45, 5107–5134. [Google Scholar] [CrossRef]
- Liu, J.Q.; Luo, Z.D.; Pan, Y.; Kumar Singh, A.; Trivedi, M.; Kumar, A. Recent Developments in Luminescent Coordination Polymers: Designing Strategies, Sensing Application and Theoretical Evidences. Coord. Chem. Rev. 2020, 406, 213145. [Google Scholar] [CrossRef]
- Zheng, Y.-Z.; Zheng, Z.; Chen, X.-M. A Symbol Approach for Classification of Molecule-Based Magnetic Materials Exemplified by Coordination Polymers of Metal Carboxylates. Coord. Chem. Rev. 2014, 258–259, 1–15. [Google Scholar] [CrossRef]
- Journaux, Y.; Ferrando-Soria, J.; Pardo, E.; Ruiz-Garcia, R.; Julve, M.; Lloret, F.; Cano, J.; Li, Y.; Lisnard, L.; Yu, P.; et al. Design of Magnetic Coordination Polymers Built from Polyoxalamide Ligands: A Thirty Year Story. Eur. J. Inorg. Chem. 2018, 2018, 228–247. [Google Scholar] [CrossRef]
- Leong, W.L.; Vittal, J.J. One-Dimensional Coordination Polymers: Complexity and Diversity in Structures, Properties, and Applications. Chem. Rev. 2011, 111, 688–764. [Google Scholar] [CrossRef]
- Marin, R.; Brunet, G.; Murugesu, M. Shining New Light on Multifunctional Lanthanide Single-Molecule Magnets. Angew. Chem. Int. Ed. 2021, 60, 1728–1746. [Google Scholar] [CrossRef]
- Edelmann, F.T. Lanthanide Amidinates and Guanidinates in Catalysis and Materials Science: A Continuing Success Story. Chem. Soc. Rev. 2012, 41, 7657. [Google Scholar] [CrossRef]
- Ferrando-Soria, J.; Pasán, J.; Ruiz-Pérez, C.; Journaux, Y.; Julve, M.; Lloret, F.; Cano, J.; Pardo, E. Spin Control in Oxamato-Based Manganese(II)-Copper(II) Coordination Polymers with Brick-Wall Layer Architectures. Inorg. Chem. 2011, 50, 8694–8696. [Google Scholar] [CrossRef]
- Ferrando-Soria, J.; Grancha, T.; Julve, M.; Cano, J.; Lloret, F.; Journaux, Y.; Pasán, J.; Ruiz-Pérez, C.; Pardo, E. Ligand Effects on the Dimensionality of Oxamato-Bridged Mixed-Metal Open-Framework Magnets. Chem. Commun. 2012, 48, 3539. [Google Scholar] [CrossRef]
- Grancha, T.; Acosta, A.; Cano, J.; Ferrando-Soria, J.; Seoane, B.; Gascon, J.; Pasán, J.; Armentano, D.; Pardo, E. Cation Exchange in Dynamic 3D Porous Magnets: Improvement of the Physical Properties. Inorg. Chem. 2015, 54, 10834–10840. [Google Scholar] [CrossRef]
- Abhervé, A.; Grancha, T.; Ferrando-Soria, J.; Clemente-León, M.; Coronado, E.; Waerenborgh, J.C.; Lloret, F.; Pardo, E. Spin-Crossover Complex Encapsulation within a Magnetic Metal-Organic Framework. Chem. Commun. 2016, 52, 7360–7363. [Google Scholar] [CrossRef]
- Fortea-Pérez, F.R.; Vallejo, J.; Julve, M.; Lloret, F.; De Munno, G.; Armentano, D.; Pardo, E. Slow Magnetic Relaxation in a Hydrogen-Bonded 2D Array of Mononuclear Dysprosium(III) Oxamates. Inorg. Chem. 2013, 52, 4777–4779. [Google Scholar] [CrossRef]
- Vaz, R.C.A.; Esteves, I.O.; Oliveira, W.X.C.; Honorato, J.; Martins, F.T.; da Silva Júnior, E.N.; Valente, D.d.C.A.; Cardozo, T.M.; Horta, B.A.C.; Mariano, D.L.; et al. Lanthanide(III)-Oxamato Complexes Containing Nd3+ and Ho3+: Crystal Structures, Magnetic Properties, and Ab Initio Calculations. CrystEngComm 2022, 24, 6628–6641. [Google Scholar] [CrossRef]
- Vaz, R.C.A.; Esteves, I.O.; Oliveira, W.X.C.; Honorato, J.; Martins, F.T.; Marques, L.F.; Dos Santos, G.L.; Freire, R.O.; Jesus, L.T.; Pedroso, E.F.; et al. Mononuclear Lanthanide(III)-Oxamate Complexes as New Photoluminescent Field-Induced Single-Molecule Magnets: Solid-State Photophysical and Magnetic Properties. Dalton Trans. 2020, 49, 16106–16124. [Google Scholar] [CrossRef]
- da Cunha, T.T.; Barbosa, V.M.M.; Oliveira, W.X.C.; Pinheiro, C.B.; Pedroso, E.F.; Nunes, W.C.; Pereira, C.L.M. Slow Magnetic Relaxation in Mononuclear Gadolinium(III) and Dysprosium(III) Oxamato Complexes. Polyhedron 2019, 169, 102–113. [Google Scholar] [CrossRef]
- da Silveira, C.O.C.; Oliveira, W.X.C.; da Silva Júnior, E.N.; Alvarenga, M.E.; Martins, F.T.; Gatto, C.C.; Pinheiro, C.B.; Pedroso, E.F.; Silva, J.P.O.; Marques, L.F.; et al. Photoluminescence and Magnetic Properties of Isostructural Europium(III), Gadolinium(III) and Terbium(III) Oxamate-Based Coordination Polymers. Dalton Trans. 2024, 53, 14995–15009. [Google Scholar] [CrossRef]
- Maciel, J.W.; de Souza, J.J.S.; Valdo, A.K.; Martins, F.T.; Guimarães, F.F.; de Santana, R.C.; Maia, L.J.Q.; Cangussu, D. Investigating Solid-State Luminescent Properties of New Cadmium(II) and Lead(II) Oxamato-Based Coordination Polymers. J. Braz. Chem. Soc. 2024, 35, e-20240072. [Google Scholar] [CrossRef]
- De Oliveira Maciel, J.W.; Lemes, M.A.; Valdo, A.K.; Rabelo, R.; Martins, F.T.; Queiroz Maia, L.J.; De Santana, R.C.; Lloret, F.; Julve, M.; Cangussu, D. Europium(III), Terbium(III), and Gadolinium(III) Oxamato-Based Coordination Polymers: Visible Luminescence and Slow Magnetic Relaxation. Inorg. Chem. 2021, 60, 6176–6190. [Google Scholar] [CrossRef]
- Maciel, J.W.; Kalinke, L.H.G.; Valdo, A.K.; Martins, F.T.; Rabelo, R.; Moliner, N.; Cano, J.; Julve, M.; Lloret, F.; Cangussu, D. New Metal-Organic Systems with a Functionalized Oxamate-Type Ligand and MnII, FeII, CuII and ZnII. J. Braz. Chem. Soc. 2019, 30, 2413–2429. [Google Scholar] [CrossRef]
- Oliveira, T.L.; Kalinke, L.H.G.; Mascarenhas, E.J.; Castro, R.; Martins, F.T.; Sabino, J.R.; Stumpf, H.O.; Ferrando, J.; Julve, M.; Lloret, F.; et al. Cobalt(II) and Copper(II) Assembling through a Functionalized Oxamate-Type Ligand. Polyhedron 2014, 81, 105–114. [Google Scholar] [CrossRef]
- Sheldrick, G.M. Crystal Structure Refinement with SHELXL. Acta Crystallogr. C Struct. Chem. 2015, 71, 3–8. [Google Scholar] [CrossRef]
- Spek, A.L. Single-Crystal Structure Validation with the Program PLATON. J. Appl. Crystallogr. 2003, 36, 7–13. [Google Scholar] [CrossRef]
- Macrae, C.F.; Sovago, I.; Cottrell, S.J.; Galek, P.T.A.; McCabe, P.; Pidcock, E.; Platings, M.; Shields, G.P.; Stevens, J.S.; Towler, M.; et al. Mercury 4.0: From Visualization to Analysis, Design and Prediction. J. Appl. Crystallogr. 2020, 53, 226–235. [Google Scholar] [CrossRef]
- Farrugia, L.J. ORTEP-3; School of Chemistry, University of Glasgow: Glasgow, Scotland, 2012. [Google Scholar]
- Farrugia, L.J. WinGX and ORTEP for Windows: An Update. J. Appl. Crystallogr. 2012, 45, 849–854. [Google Scholar] [CrossRef]
- Gruber, J.B.; Zandi, B.; Valiev, U.V.; Rakhimov, S.A. Energy Levels of Dy3+(4f 9) in Orthoaluminate Crystals. J. Appl. Phys. 2003, 94, 1030–1034. [Google Scholar] [CrossRef]
- Caspers, H.H.; Rast, H.E.; Fry, J.L. Absorption, Fluorescence, and Energy Levels of Ho3+ in LaF3. J. Chem. Phys. 1970, 53, 3208–3216. [Google Scholar] [CrossRef]
- Krupke, W.F.; Grdbee, J.B. Absorption and Fluorescence Spectra of Er3+(4f11) in LaF3. J. Chem. Phys. 1963, 39, 1024–1030. [Google Scholar] [CrossRef]
- Rajnák, C.; Boča, R. Reciprocating Thermal Behavior in the Family of Single Ion Magnets. Coord. Chem. Rev. 2021, 436, 213808. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and Absorption in Dielectrics I. Alternating Current Characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- Sahu, P.K.; Konar, S. Dysprosium (III) Based One-Dimensional Zigzag Chain Exhibiting Slow Magnetic Relaxation. Inorganica Chim. Acta 2024, 572, 122255. [Google Scholar] [CrossRef]
- Orts-Arroyo, M.; Rabelo, R.; Carrasco-Berlanga, A.; Moliner, N.; Cano, J.; Julve, M.; Lloret, F.; De Munno, G.; Ruiz-García, R.; Mayans, J.; et al. Field-Induced Slow Magnetic Relaxation and Magnetocaloric Effects in an Oxalato-Bridged Gadolinium(III)-Based 2D MOF. Dalton Trans. 2021, 50, 3801–3805. [Google Scholar] [CrossRef] [PubMed]
- El Alouani Dahmouni, N.; Orts-Arroyo, M.; Sanchis-Perucho, A.; Moliner, N.; Mayans, J.; Pacheco, M.; Castro, I.; De Munno, G.; Marino, N.; Ruiz-García, R.; et al. Magnetocaloric Efficiency Tuning through Solvent-Triggered 3D to 2D Interconversion in Holmium(III)-Based Dynamic MOFs. Chem. Commun. 2024, 60, 7451–7454. [Google Scholar] [CrossRef] [PubMed]
- Daudin, B.; Lagnier, R.; Salce, B. Thermodynamic Properties of the Gadolinium Gallium Garnet, Gd3Ga5O12, between 0.05 and 25 K. J. Magn. Magn. Mater. 1982, 27, 315–322. [Google Scholar] [CrossRef]
- Numazawa, T.; Kamiya, K.; Okano, T.; Matsumoto, K. Magneto Caloric Effect in (DyxGd+)3Ga5O12 for Adiabatic Demagnetization Refrigeration. Physica B Condens. Matter 2003, 329–333, 1656–1657. [Google Scholar] [CrossRef]
- Saines, P.J.; Paddison, J.A.M.; Thygesen, P.M.M.; Tucker, M.G. Searching beyond Gd for Magnetocaloric Frameworks: Magnetic Properties and Interactions of the Ln(HCO2)3 Series. Mater. Horiz. 2015, 2, 528–535. [Google Scholar] [CrossRef]
- Dixey, R.J.C.; Saines, P.J. Optimization of the Magnetocaloric Effect in Low Applied Magnetic Fields in LnOHCO3 Frameworks. Inorg. Chem. 2018, 57, 12543–12551. [Google Scholar] [CrossRef]
- Doheny, P.W.; Cassidy, S.J.; Saines, P.J. Investigations of the Magnetocaloric and Thermal Expansion Properties of the Ln3(Adipate)4.5(DMF)2(Ln = Gd-Er) Framework Series. Inorg. Chem. 2022, 61, 4957–4964. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Arauzo, A.; Giner Planas, J.; Bartolomé, E. Magnetic Properties and Magnetocaloric Effect of Ln = Dy, Tb Carborane-Based Metal–Organic Frameworks. Dalton Trans. 2024, 53, 8969–8979. [Google Scholar] [CrossRef]
- Li, Z.; Arauzo, A.; Roscini, C.; Planas, J.G.; Bartolomé, E. Multifunctional Self-Refrigerated Multivariate {GdLn} (Ln = Dy, Tb, Tb/Eu) Metal–Organic Frameworks. J. Mater. Chem. A Mater. 2024, 12, 21971–21986. [Google Scholar] [CrossRef]
- Herrero, A.; Aseguinolaza, I.R.; Oleaga, A.; Garcia-Adeva, A.J.; Apiñaniz, E.; Garshev, A.V.; Yapaskurt, V.O.; Morozkin, A.V. Selecting Optimal R6TX2 Intermetallics (R = Gd, Tb, Dy; T = Mn, Fe, Co, Ni; X = Sb, Te) for Magnetic Refrigeration. Dalton Trans. 2023, 52, 5780–5797. [Google Scholar] [CrossRef]
- Kleinhans, M.; Eibensteiner, K.; Leiner, J.C.; Resch, C.; Worch, L.; Wilde, M.A.; Spallek, J.; Regnat, A.; Pfleiderer, C. Magnetocaloric Properties of R3Ga5O12 (R=Tb, Gd, Nd, Dy). Phys. Rev. Appl. 2023, 19, 014038. [Google Scholar] [CrossRef]


| 3 | |
|---|---|
| Empirical formula | C27H25N3O20Er2 |
| Formula weight | 1046.02 |
| Crystal system | Monoclinic |
| Space group | Cc |
| a/Å | 24.290(4) |
| b/Å | 19.873(4) |
| c/Å | 7.4327(13) |
| β/° | 104.998(5) |
| V/Å3 | 3465.7(11) |
| Z | 4 |
| T/K | 296 |
| Radiation | Mo Kα |
| Wavelength/Å | 0.71073 |
| ρ/g cm−3 | 2.005 |
| μ//mm−1 | 4.897 |
| Symmetry factor (Rint) | 0.1171 |
| Completeness to θmax/% | 98.70 |
| F(000) | 2016.0 |
| Refined parameters | 272 |
| Reflections collected | 6351 |
| R a, wR b [I > 2s(I)] | 0.1087, 0.3155 |
| R a, wR b (tall data) | 0.1502 |
| S c | 1.127 |
| ρmax and ρmin/eÅ−1 | 2.3400/–4.2300 |
| CCDC | 2417034 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maciel, J.W.; Kalinke, L.H.G.; Rabelo, R.; Alvarenga, M.E.; Martins, F.T.; Moliner, N.; Cangussu, D. Slow Relaxation of Magnetization and Magnetocaloric Effects in One-Dimensional Oxamato-Based Lanthanide(III) Coordination Polymers. Magnetochemistry 2025, 11, 23. https://doi.org/10.3390/magnetochemistry11040023
Maciel JW, Kalinke LHG, Rabelo R, Alvarenga ME, Martins FT, Moliner N, Cangussu D. Slow Relaxation of Magnetization and Magnetocaloric Effects in One-Dimensional Oxamato-Based Lanthanide(III) Coordination Polymers. Magnetochemistry. 2025; 11(4):23. https://doi.org/10.3390/magnetochemistry11040023
Chicago/Turabian StyleMaciel, Jhonny W., Lucas H. G. Kalinke, Renato Rabelo, Meiry E. Alvarenga, Felipe Terra Martins, Nicolás Moliner, and Danielle Cangussu. 2025. "Slow Relaxation of Magnetization and Magnetocaloric Effects in One-Dimensional Oxamato-Based Lanthanide(III) Coordination Polymers" Magnetochemistry 11, no. 4: 23. https://doi.org/10.3390/magnetochemistry11040023
APA StyleMaciel, J. W., Kalinke, L. H. G., Rabelo, R., Alvarenga, M. E., Martins, F. T., Moliner, N., & Cangussu, D. (2025). Slow Relaxation of Magnetization and Magnetocaloric Effects in One-Dimensional Oxamato-Based Lanthanide(III) Coordination Polymers. Magnetochemistry, 11(4), 23. https://doi.org/10.3390/magnetochemistry11040023








