pH-Based Control Strategies for the Nitrification of High-Ammonium Wastewaters
Abstract
1. Introduction
2. Materials and Methods
2.1. Experiments
2.2. Apparatus and Instruments
3. Results and Discussion
3.1. Determining the pH Characteristics of Nitrification
3.2. Batch Systems
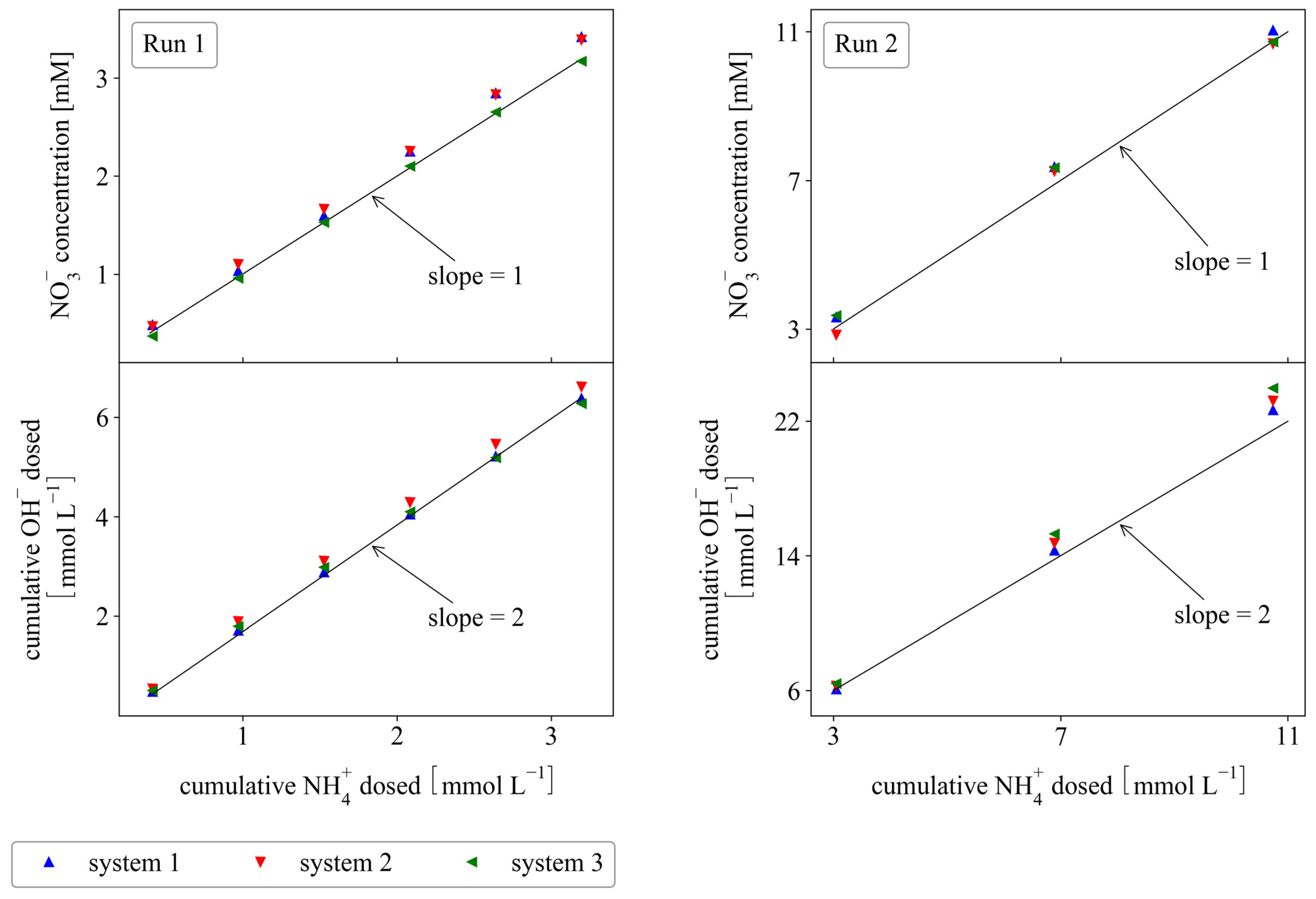
3.3. Fed-Batch Systems
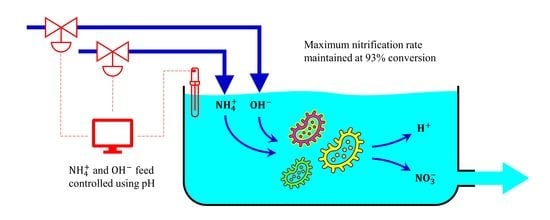
3.4. Continuous Systems
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Erisman, J.W.; Sutton, M.A.; Galloway, J.; Klimont, Z.; Winiwarter, W. How a century of ammonia synthesis changed the world. Nat. Geosci. 2008, 1, 636–639. [Google Scholar] [CrossRef]
- Kanter, D.R.; Bartolini, F.; Kugelberg, S.; Leip, A.; Oenema, O.; Uwizeye, A. Nitrogen pollution policy beyond the farm. In Nature Food; Springer Nature: Berlin/Heidelberg, Germany, 2020. [Google Scholar] [CrossRef]
- Castellar, J.A.C.; Formosa, J.; Fernández, A.I.; Jové, P.; Bosch, M.G.; Morató, J.; Arias, C.A. Cork as a sustainable carbon source for nature-based solutions treating hydroponic wastewaters–Preliminary batch studies. Sci. Total. Environ. 2019, 650, 267–276. [Google Scholar] [CrossRef]
- Gagnon, V.; Maltais-Landry, G.; Puigagut, J.; Chazarenc, F.; Brisson, J. Treatment of hydroponics wastewater using constructed wetlands in winter conditions. Water Air Soil Pollut. 2010, 212, 483–490. [Google Scholar] [CrossRef]
- Prystay, W.; Lo, K.V. Treatment of greenhouse wastewater using constructed wetlands. J. Environ. Sci. Health Part. B Pestic. Food Contam. Agric. Wastes 2001, 36, 341–353. [Google Scholar] [CrossRef]
- Rufí-Salís, M.; Calvo, M.J.; Petit-Boix, A.; Villalba, G.; Gabarrell, X. Exploring nutrient recovery from hydroponics in urban agriculture: An environmental assessment. Resour. Conserv. Recycl. 2020, 155. [Google Scholar] [CrossRef]
- Saxena, P.; Bassi, A. Removal of nutrients from hydroponic greenhouse effluent by alkali precipitation and algae cultivation method. J. Chem. Technol. Biotechnol. 2013, 88, 858–863. [Google Scholar] [CrossRef]
- Chan-Pacheco, C.R.; Valenzuela, E.I.; Cervantes, F.J.; Quijano, G. Novel biotechnologies for nitrogen removal and their coupling with gas emissions abatement in wastewater treatment facilities. In Science of the Total Environment; Elsevier, B.V.: Amsterdam, The Netherlands, 2021. [Google Scholar] [CrossRef]
- Ronga, D.; Setti, L.; Salvarani, C.; De Leo, R.; Bedin, E.; Pulvirenti, A.; Francia, E. Effects of solid and liquid digestate for hydroponic baby leaf lettuce (Lactuca sativa L.) cultivation. Sci. Hortic. 2019, 244, 172–181. [Google Scholar] [CrossRef]
- Möller, K.; Müller, T. Effects of anaerobic digestion on digestate nutrient availability and crop growth: A review. Eng. Life Sci. 2012, 12, 242–257. [Google Scholar] [CrossRef]
- Hachiya, T.; Sakakibara, H. Interactions between nitrate and ammonium in their uptake, allocation, assimilation, and signaling in plants. J. Exp. Bot. 2017, 68, 2501–2512. [Google Scholar] [CrossRef] [PubMed]
- Bergstrand, K.J.; Asp, H.; Hultberg, M. Utilizing anaerobic digestates as nutrient solutions in hydroponic production systems. Sustainability 2020, 12, 10076. [Google Scholar] [CrossRef]
- Neal, J.; Wilkie, A.C. Anaerobic Digester Effluent as Fertilizer for Hydroponically Grown Tomatoes. J. Undergrad. Res. 2014, 15, 1–5. [Google Scholar]
- Stoknes, K.; Scholwin, F.; Krzesiński, W.; Wojciechowska, E.; Jasińska, A. Efficiency of a novel “Food to waste to food” system including anaerobic digestion of food waste and cultivation of vegetables on digestate in a bubble-insulated greenhouse. Waste Manag. 2016, 56, 466–476. [Google Scholar] [CrossRef] [PubMed]
- Grand View Research. Hydroponics Market Size, Share & Trends Analysis Report by Type (Aggregate Systems, Liquid Systems), by Crops (Tomatoes, Lettuce, Peppers, Cucumbers, Herbs), by Region, and Segment Forecasts 2019–2025. Available online: https://www.grandviewresearch.com/industry-analysis/hydroponics-market (accessed on 17 October 2021).
- Kumar, R.R.; Cho, J.Y. Reuse of hydroponic waste solution. Environ. Sci. Pollut. Res. 2014, 21, 9569–9577. [Google Scholar] [CrossRef]
- Pelayo, L.O.; Hultberg, M.; Bergstrand, K.J.; Larsson-Jönsson, H.; Caspersen, S.; Asp, H. Biogas Digestate in Vegetable Hydroponic Production: pH Dynamics and pH Management by Controlled Nitrification. Waste Biomass Valorization 2021, 12, 123–133. [Google Scholar] [CrossRef]
- Pitts, M.; Stutte, G. Computer model of hydroponics nutrient solution pH control using ammonium. Life Support Biosph. Sci. Int. J. Earth Space 1999, 6, 73–85. [Google Scholar]
- Hoagland, D.R.; Arnon, D.I. The Water-Culture Method for Growing Plants without Soil; University of California, College of Agriculture, Agricultural Experiment Station: Berkeley, CA, USA, 1938. [Google Scholar]
- Botheju, D.; Svalheim, O.; Bakke, R. Digestate Nitrification for Nutrient Recovery. Open Waste Manag. J. 2010, 3, 1–12. [Google Scholar] [CrossRef]
- Edgar, M.; Levi, A.; Percy, C.; Gaddafi, L. Analysis of nitrifying microbial community for organic hydroponics. Afr. J. Microbiol. Res. 2018, 12, 1–8. [Google Scholar] [CrossRef][Green Version]
- Quinlan, A.V. Prediction of the optimum pH for ammonia-N oxidation by nitrosomonas Europaea in well-aerated natural and domestic-waste waters. Water Res. 1984, 18, 561–566. [Google Scholar] [CrossRef]
- Madigan, M.T.; Martinko, J.M.; Parker, J. Brock Biology of Microorganisms, 10th ed.; Pearson Education, Inc.: Upper Saddle River, NJ, USA, 2003; p. 1019. [Google Scholar]
- Norton, J.; Ouyang, Y. Controls and adaptive management of nitrification in agricultural soils. Front. Microbiol. 2019, 10, 1931. [Google Scholar] [CrossRef]
- Andreottola, G.; Foladori, P.; Ragazzi, M. On-line control of a SBR system for nitrogen removal from industrial wastewater. Water Sci. Technol. 2001, 43, 93–100. [Google Scholar] [CrossRef]
- Hajsardar, M.; Borghei, S.M.; Hassani, A.H.; Takdastan, A. Simultaneous ammonium and nitrate removal by a modified intermittently aerated sequencing batch reactor (SBR) with multiple filling events. Pol. J. Chem. Technol. 2016, 18, 72–80. [Google Scholar] [CrossRef]
- Kim, H.; Hao, O.J. pH and Oxidation-Reduction Potential Control Strategy for Optimization of Nitrogen Removal in an Alternating Aerobic-Anoxic System All use subject to JSTOR Terms and Conditions pH and Control Nitrogen Strategy Removal Potential of for Optimization in an A. Water Environ. Res. 2001, 73, 95–102. [Google Scholar] [CrossRef]
- Kim, D.J.; Lee, D.I.; Keller, J. Effect of temperature and free ammonia on nitrification and nitrite accumulation in landfill leachate and analysis of its nitrifying bacterial community by FISH. Bioresour. Technol. 2006, 97, 459–468. [Google Scholar] [CrossRef] [PubMed]
- Cytryn, E.; Levkovitch, I.; Negreanu, Y.; Dowd, S.; Frenk, S.; Silber, A. Impact of short-term acidification on nitrification and nitrifying bacterial community dynamics in soilless cultivation media. Appl. Environ. Microbiol. 2012, 78, 6576–6582. [Google Scholar] [CrossRef]
- Tabatabaei, S.; Fatemi, L.; Fallahi, E. Effect of ammonium: Nitrate ratio on yield, calcium concentration, and photosynthesis rate in strawberry. J. Plant. Nutr. 2006, 29, 1273–1285. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
van Rooyen, I.L.; Brink, H.G.; Nicol, W. pH-Based Control Strategies for the Nitrification of High-Ammonium Wastewaters. Fermentation 2021, 7, 319. https://doi.org/10.3390/fermentation7040319
van Rooyen IL, Brink HG, Nicol W. pH-Based Control Strategies for the Nitrification of High-Ammonium Wastewaters. Fermentation. 2021; 7(4):319. https://doi.org/10.3390/fermentation7040319
Chicago/Turabian Stylevan Rooyen, Ignatius Leopoldus, Hendrik Gideon Brink, and Willie Nicol. 2021. "pH-Based Control Strategies for the Nitrification of High-Ammonium Wastewaters" Fermentation 7, no. 4: 319. https://doi.org/10.3390/fermentation7040319
APA Stylevan Rooyen, I. L., Brink, H. G., & Nicol, W. (2021). pH-Based Control Strategies for the Nitrification of High-Ammonium Wastewaters. Fermentation, 7(4), 319. https://doi.org/10.3390/fermentation7040319