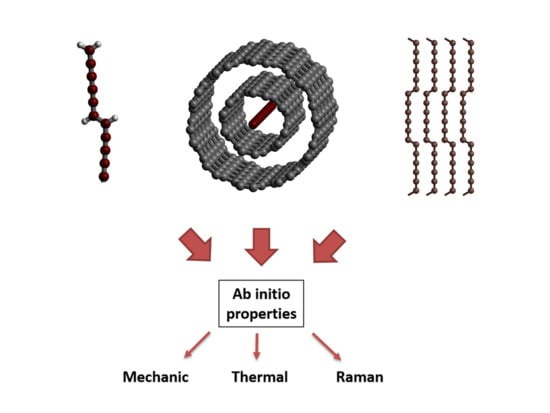
Structure and Properties of Chained Carbon: Recent Ab Initio Studies
Abstract
:1. Introduction
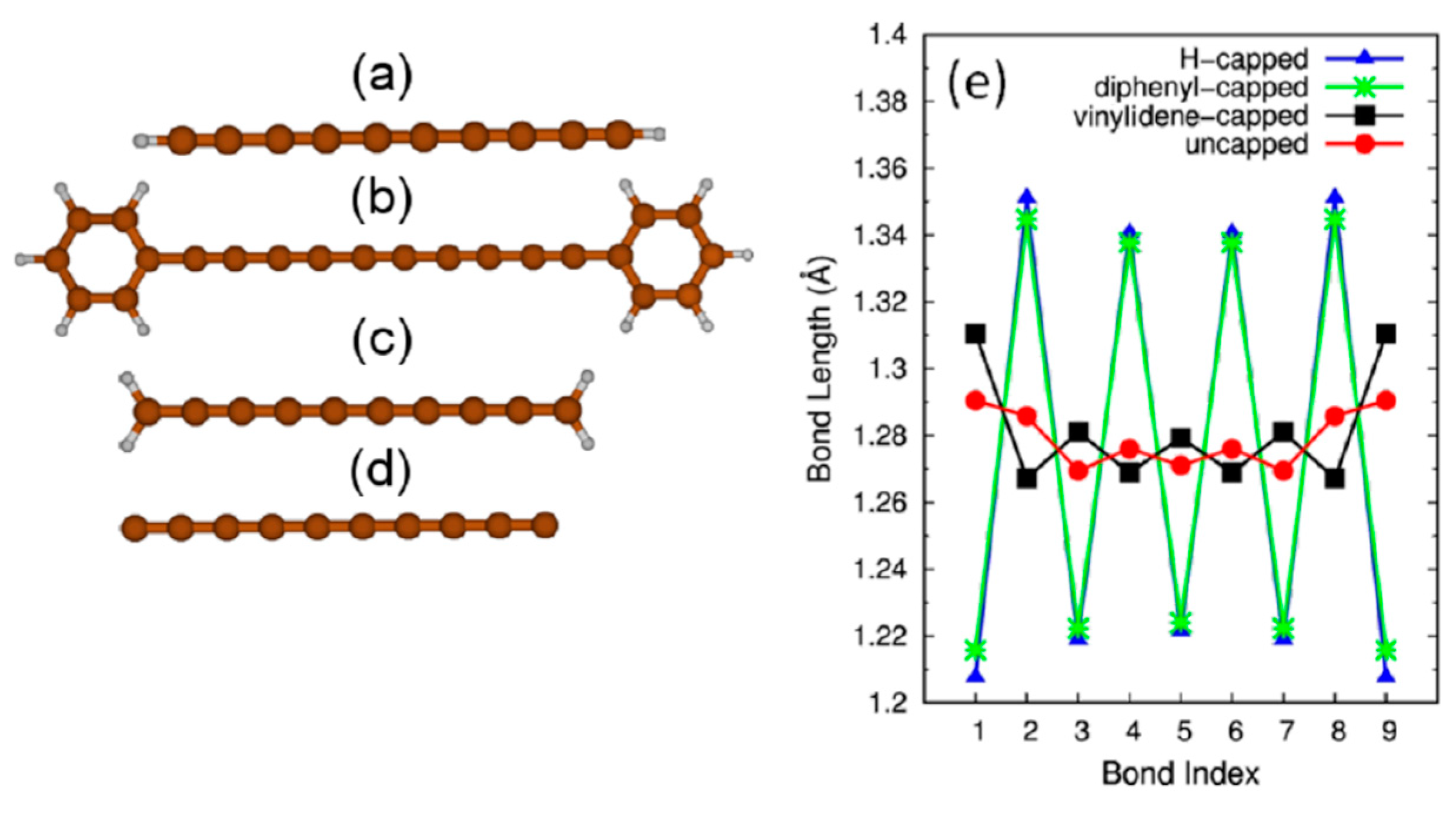
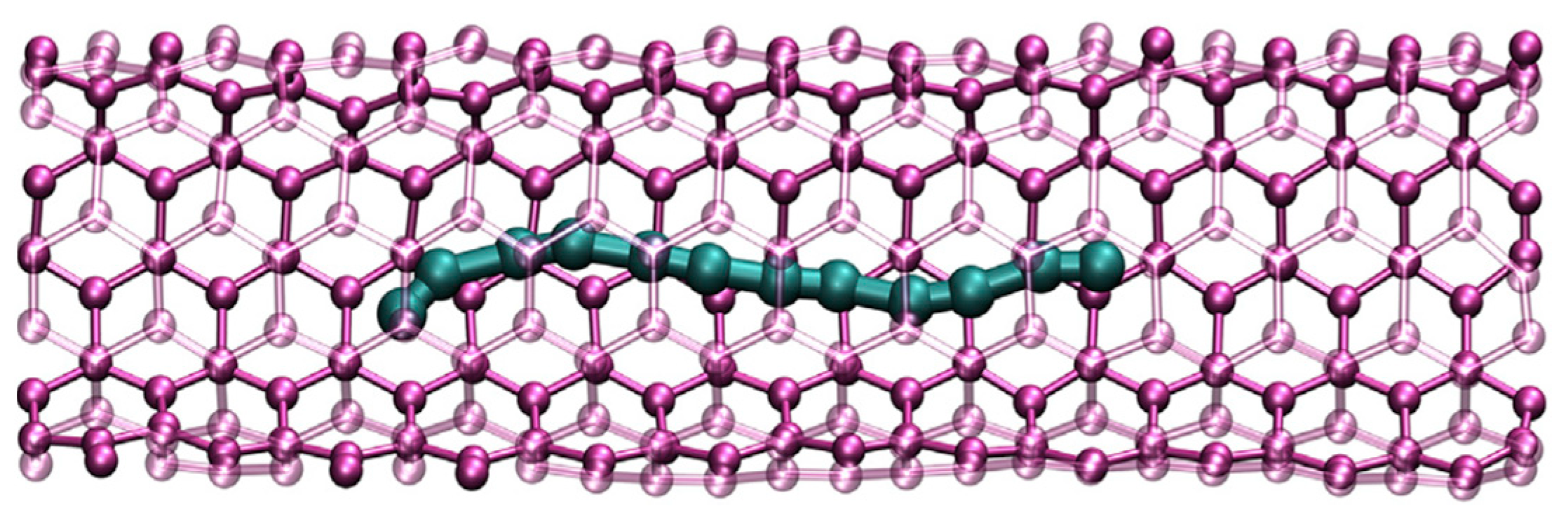
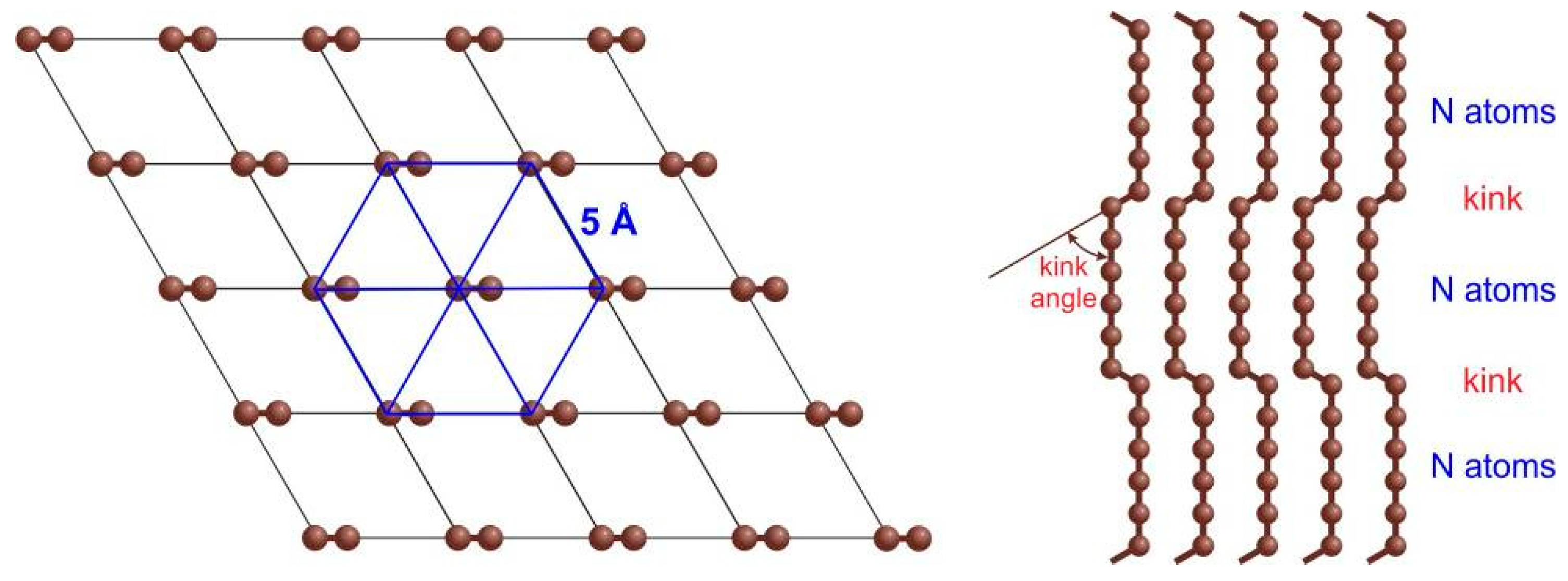
2. Mechanical Properties
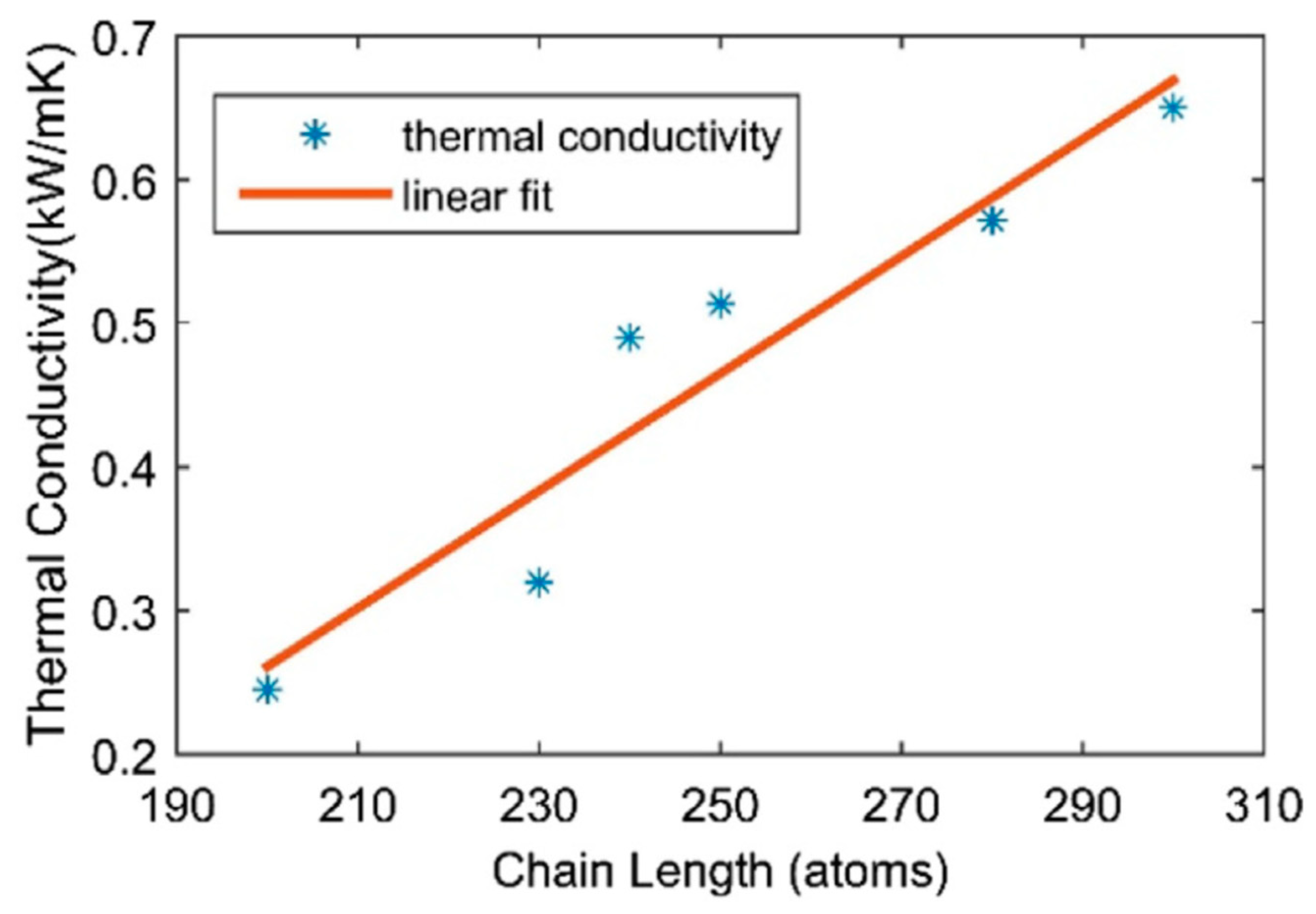
3. Thermal Conductivity
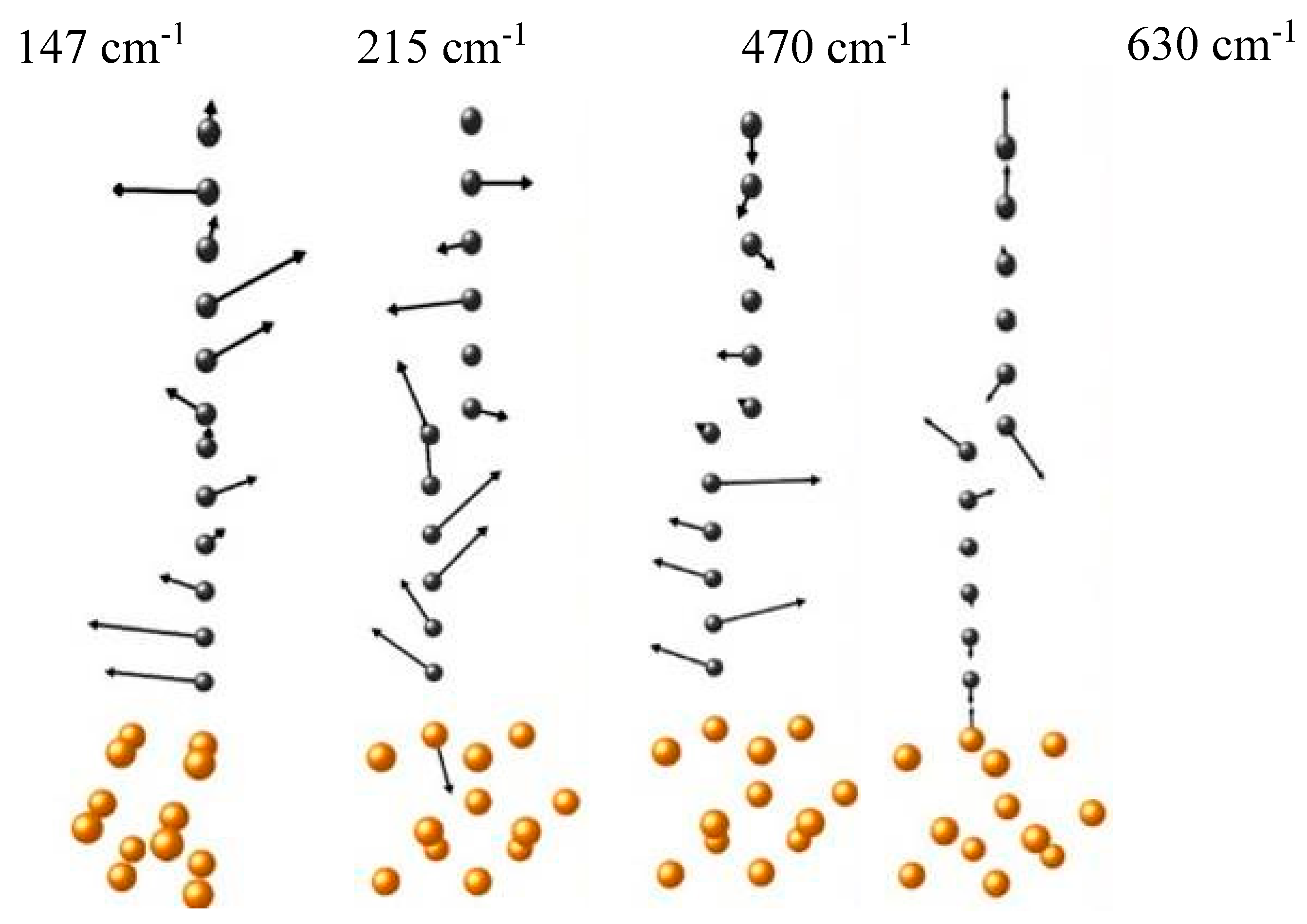
4. Electronic Properties, Raman Response, and Superconductivity
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Casari, C.S.; Milani, A. Carbyne: From the elusive allotrope to stable carbon atom wires. MRS Commun. 2018, 8, 207–219. [Google Scholar] [CrossRef]
- Timoshevskii, A.; Kotrechko, S.; Matviychuk, Y. Atomic structure and mechanical properties of carbyne. Phys. Rev. B - Condens. Matter Mater. Phys. 2015, 91, 1–7. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, G.; Zhang, Y.W. Tunable mechanical and thermal properties of one-dimensional carbyne chain: Phase transition and microscopic dynamics. J. Phys. Chem. C. 2015, 119, 24156–24164. [Google Scholar] [CrossRef]
- Muller, S.E.; Nair, A.K. Carbyne as a fiber in metal-matrix nanocomposites: A first principle study. Comput. Mater. Sci. 2019, 159, 187–193. [Google Scholar] [CrossRef]
- Casari, C.S.; Tommasini, M.; Tykwinski, R.R.; Milani, A. Carbon-atom wires: 1-D systems with tunable properties. Nanoscale 2016, 8, 4414–4435. [Google Scholar] [CrossRef] [PubMed]
- Tongay, S.; Senger, R.T.; Dag, S.; Ciraci, S. Ab-initio electron transport calculations of carbon based string structures. Phys. Rev. Lett. 2004, 93, 2–5. [Google Scholar] [CrossRef] [PubMed]
- Milani, A.; Tommasini, M.; Russo, V.; Bassi, A.L.; Lucotti, A.; Cataldo, F.; Casari, C.S. Raman spectroscopy as a tool to investigate the structure and electronic properties of carbon-atom wires. Beilstein, J. Nanotechnol. 2015, 6, 480–491. [Google Scholar] [CrossRef] [Green Version]
- Shi, L.; Rohringer, P.; Suenaga, K.; Niimi, Y.; Kotakoski, J.; Meyer, J.C.; Peterlik, H.; Wanko, M.; Cahangirov, S.; Rubio, A.; et al. Confined linear carbon chains as a route to bulk carbyne. Nat. Mater. 2016, 15, 634–639. [Google Scholar] [CrossRef]
- Shi, L.; Rohringer, P.; Wanko, M.; Rubio, A.; Waßerroth, S.; Reich, S.; Cambré, S.; Wenseleers, W.; Ayala, P.; Pichler, T. Electronic band gaps of confined linear carbon chains ranging from polyyne to carbyne. Phys. Rev. Mater. 2017, 1, 1–7. [Google Scholar] [CrossRef]
- Deng, Y.; Cranford, S.W. Thermal conductivity of 1D carbyne chains. Comput. Mater. Sci. 2017, 129, 226–230. [Google Scholar] [CrossRef]
- Deng, Y.; Cranford, S.W. Mapping temperature and confinement dependence of carbyne formation within carbon nanotubes. Carbon N. Y. 2019, 141, 209–217. [Google Scholar] [CrossRef]
- Flood, P.; Babaev, V.; Khvostov, V.; Novikov, N.; Guseva, M. Carbon Material with a Highly Ordered Linear-Chain Structure. In Polyynes Synthesis, Properties, and Applications; Cataldo, F., Ed.; Taylor & Francis Group: Didcot, UK,, 2005; pp. 219–252. [Google Scholar] [CrossRef]
- Buntov, E.A.; Zatsepin, A.F.; Guseva, M.B.; Ponosov, Y.S. 2D-ordered kinked carbyne chains: DFT modeling and Raman characterization. Carbon N. Y. 2017, 117, 271–278. [Google Scholar] [CrossRef]
- Kaiser, K.; Scriven, L.M.; Schulz, F.; Gawel, P.; Gross, L.; Anderson, H.L. An sp-hybridized molecular carbon allotrope, cyclo[18] carbon. Science 2019, eaay1914. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Artyukhov, V.I.; Lee, H.; Xu, F.; Yakobson, B.I. Carbyne from First Principles: Chain of C Atoms, a Nanorod or a Nanorope. ACS Nano. 2013, 7, 10075–10082. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vojta, M. Reports on Progress in Physics Related content. Rep. Prog. Phys. 1996, 59, 1665–1735. [Google Scholar] [CrossRef]
- Kocsis, A.J.; Yedama, N.A.R.; Cranford, S.W. Confinement and controlling the effective compressive stiffness of carbyne. Nanotechnology. 2014, 25, 335709. [Google Scholar] [CrossRef] [PubMed]
- Van Duin, A.C.T.; Dasgupta, S.; Lorant, F.; Goddard, W.A. ReaxFF: A reactive force field for hydrocarbons. J. Phys. Chem. A. 2001, 105, 9396–9409. [Google Scholar] [CrossRef]
- Mirzaeifar, R.; Qin, Z.; Buehler, M.J. Tensile strength of carbyne chains in varied chemical environments and structural lengths. Nanotechnology. 2014, 25, 371001. [Google Scholar] [CrossRef] [PubMed]
- Kotrechko, S.; Mikhailovskij, I.; Mazilova, T.; Sadanov, E.; Timoshevskii, A.; Stetsenko, N.; Matviychuk, Y. Mechanical properties of carbyne: Experiment and simulations. Nanoscale Res. Lett. 2015, 10, 24. [Google Scholar] [CrossRef]
- Wong, C.H.; Buntov, E.A.; Rychkov, V.N.; Guseva, M.B.; Zatsepin, A.F. Simulation of chemical bond distributions and phase transformation in carbon chains. Carbon N. Y. 2017, 114, 106–110. [Google Scholar] [CrossRef] [Green Version]
- Müller-Plathe, F. A simple nonequilibrium molecular dynamics method for calculating the thermal conductivity. J. Chem. Phys. 1997, 106, 6082–6085. [Google Scholar] [CrossRef]
- Zhan, H.; Gu, Y. Thermal conduction of one-dimensional carbon nanomaterials and nanoarchitectures. Chin. Phys. B. 2018, 27, 038103. [Google Scholar] [CrossRef]
- Mazzolari, T.M.; Manini, N. Thermal formation of carbynes. J. Phys. Condens. Matter. 2014, 26, 215302. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ming, C.; Meng, F.X.; Chen, X.; Zhuang, J.; Ning, X.J. Tuning the electronic and optical properties of monatomic carbon chains. Carbon N. Y. 2013, 68, 487–492. [Google Scholar] [CrossRef]
- Mostaani, E.; Monserrat, B.; Drummond, N.D.; Lambert, C.J. Quasiparticle and excitonic gaps of one-dimensional carbon chains. Phys. Chem. Chem. Phys. 2016, 18, 14810–14821. [Google Scholar] [CrossRef] [Green Version]
- Pino, T.; Ding, H.; Güthe, F. Electronic spectra of the chains HC2nH (n=8-13) in the gas phase. J. Chem. Phys. 2001, 114, 2208–2212. [Google Scholar] [CrossRef]
- Agarwal, N.R.; Lucotti, A.; Fazzi, D.; Tommasini, M.A.T.T.E.O.; Castiglioni, C.; Chalifoux, W.A.; Tykwinski, R.R. Structure and chain polarization of long polyynes investigated with infrared and Raman spectroscopy. J. Raman Spectrosc. 2013, 44, 1398–1410. [Google Scholar] [CrossRef]
- Eisler, S.; Slepkov, A.D.; Elliott, E.; Luu, T.; McDonald, R.; Hegmann, F.A.; Tykwinski, R.R. Polyynes as a model for carbyne: Synthesis, physical properties, and nonlinear optical response. J. Am. Chem. Soc. 2005, 127, 2666–2676. [Google Scholar] [CrossRef]
- Moura, L.G.; Malard, L.M.; Carneiro, M.A.; Venezuela, P.; Capaz, R.B.; Nishide, D.; Achiba, Y.; Shinohara, H.; Pimenta, M.A. Charge transfer and screening effects in polyynes encapsulated inside single-wall carbon nanotubes. Phys. Rev. B Condens. Matter Mater. Phys. 2009, 80, 10–13. [Google Scholar] [CrossRef]
- Nishide, D.; Wakabayashi, T.; Sugai, T.; Kitaura, R.; Kataura, H.; Achiba, Y.; Shinohara, H. Raman spectroscopy of size-selected linear polyyne molecules C2nH2 (n = 4–6) encapsulated in single-wall carbon nanotubes. J. Phys. Chem. C. 2007, 111, 5178–5183. [Google Scholar] [CrossRef]
- Jorio, A.; Souza Filho, A.G. Raman Studies of Carbon Nanostructures. Annu. Rev. Mater. Res. 2016, 46, 357–382. [Google Scholar] [CrossRef]
- Buntov, E.A.; Zatsepin, A.F.; Slesarev, A.I.; Shchapova, Y.V.; Challinger, S.; Baikie, I. Effect of thickness and substrate type on the structure and low vacuum photoemission of carbyne-containing films. Carbon N. Y. 2019, 152, 388–395. [Google Scholar] [CrossRef]
- Balakrishnan, J.; Koon, G.K.W.; Jaiswal, M.; Neto, A.C.; Özyilmaz, B. Colossal enhancement of spin-orbit coupling in weakly hydrogenated graphene. Nat. Phys. 2013, 9, 284–287. [Google Scholar] [CrossRef]
- Prazdnikov, Y. A Simplified Approach to the Problems of Room-Temperature Superconductivity. J. Mod. Phys. 2015, 06, 396–402. [Google Scholar] [CrossRef] [Green Version]
- Wong, C.H.; Buntov, E.A.; Guseva, M.B.; Kasimova, R.E.; Rychkov, V.N.; Zatsepin, A.F. Superconductivity in ultra-thin carbon nanotubes and carbyne-nanotube composites: An ab-initio approach. Carbon N. Y. 2017, 125, 509–515. [Google Scholar] [CrossRef] [Green Version]
- Wong, C.H.; Lortz, R.; Buntov, E.A.; Kasimova, R.E.; Zatsepin, A.F. A theoretical quest for high temperature superconductivity on the example of low-dimensional carbon structures. Sci. Rep. 2017, 7, 15815. [Google Scholar] [CrossRef] [PubMed] [Green Version]




© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buntov, E.; Zatsepin, A.; Kitayeva, T.; Vagapov, A. Structure and Properties of Chained Carbon: Recent Ab Initio Studies. C 2019, 5, 56. https://doi.org/10.3390/c5030056
Buntov E, Zatsepin A, Kitayeva T, Vagapov A. Structure and Properties of Chained Carbon: Recent Ab Initio Studies. C. 2019; 5(3):56. https://doi.org/10.3390/c5030056
Chicago/Turabian StyleBuntov, Evgeny, Anatoly Zatsepin, Tatiana Kitayeva, and Alexander Vagapov. 2019. "Structure and Properties of Chained Carbon: Recent Ab Initio Studies" C 5, no. 3: 56. https://doi.org/10.3390/c5030056
APA StyleBuntov, E., Zatsepin, A., Kitayeva, T., & Vagapov, A. (2019). Structure and Properties of Chained Carbon: Recent Ab Initio Studies. C, 5(3), 56. https://doi.org/10.3390/c5030056