Degradation Kinetics, Mechanisms, and Antioxidant Activity of PCL-Based Scaffolds with In Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls
Abstract
1. Introduction
2. Materials and Methods
2.1. Scaffold Preparation and Enzymatic Degradation
2.1.1. Zero-Order Kinetics
2.1.2. First-Order Kinetics
2.1.3. Higuchi Model
2.1.4. Korsmeyer–Peppas Model
2.1.5. Contracting Volume Model
2.2. Thermal Properties
2.3. Hydrolysis of Ester Groups
2.4. Antioxidant Potential
3. Results and Discussion
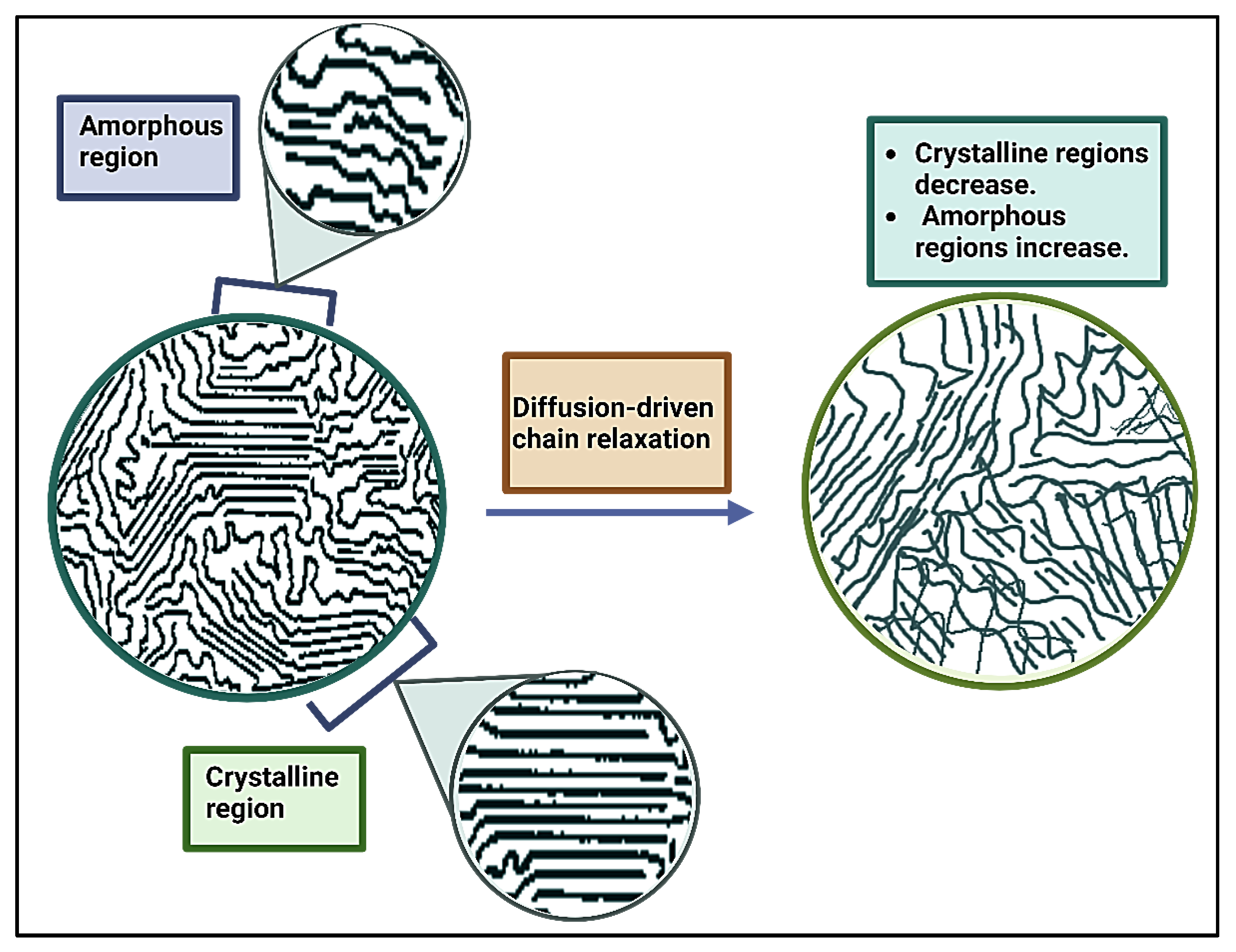
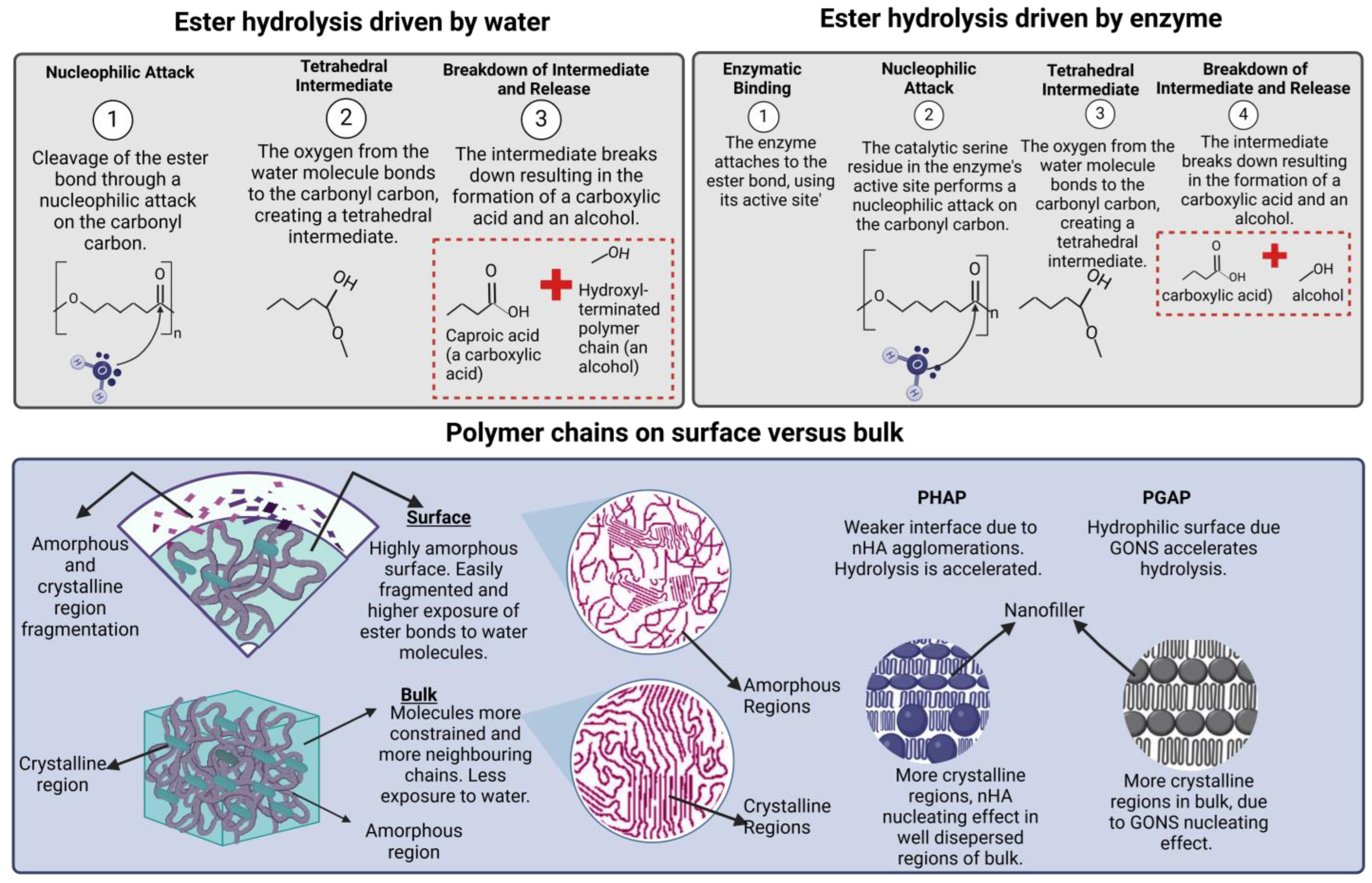
3.1. Enzymatic Degradation
3.2. Thermal Behavior
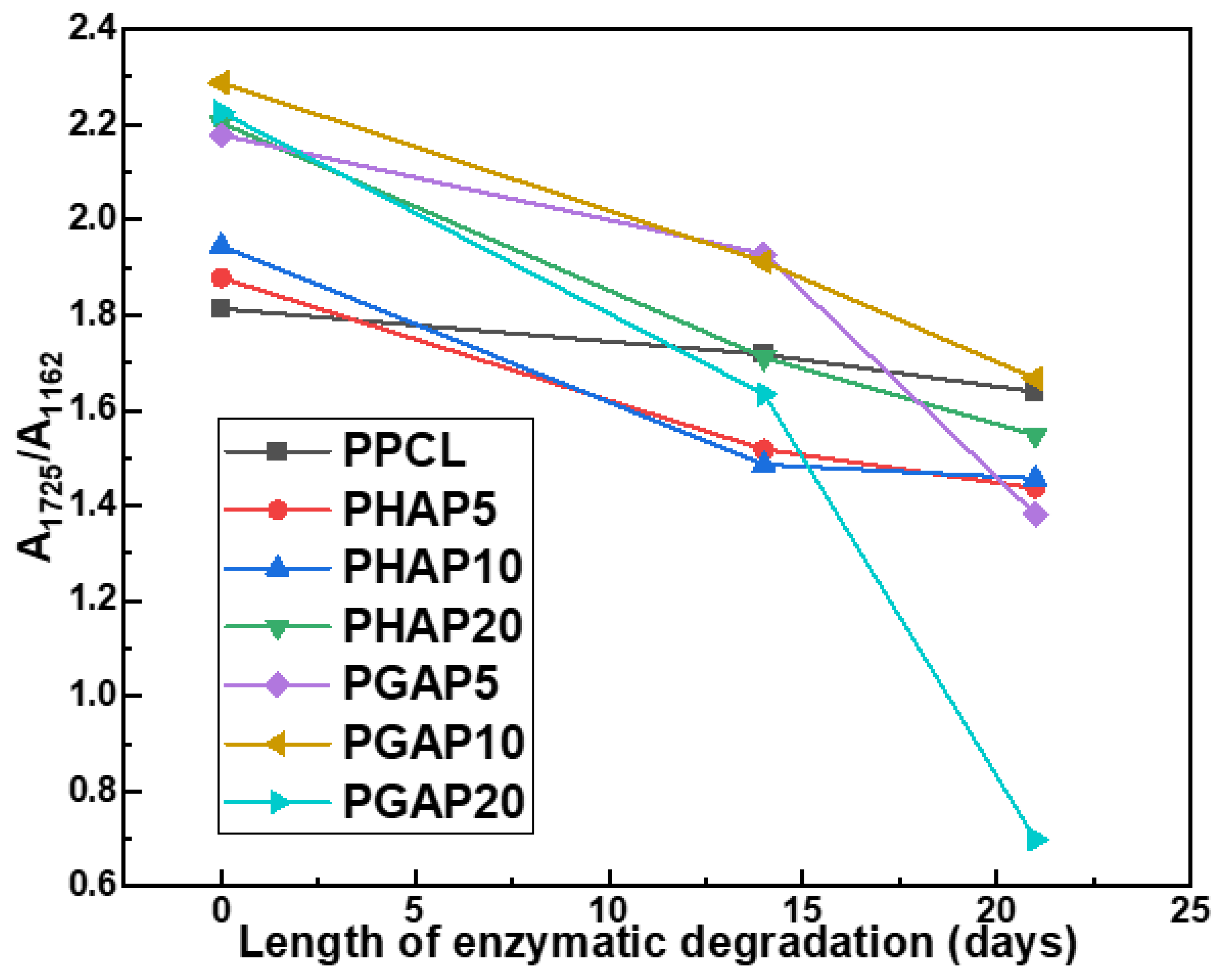
3.3. Hydrolysis of Ester Group
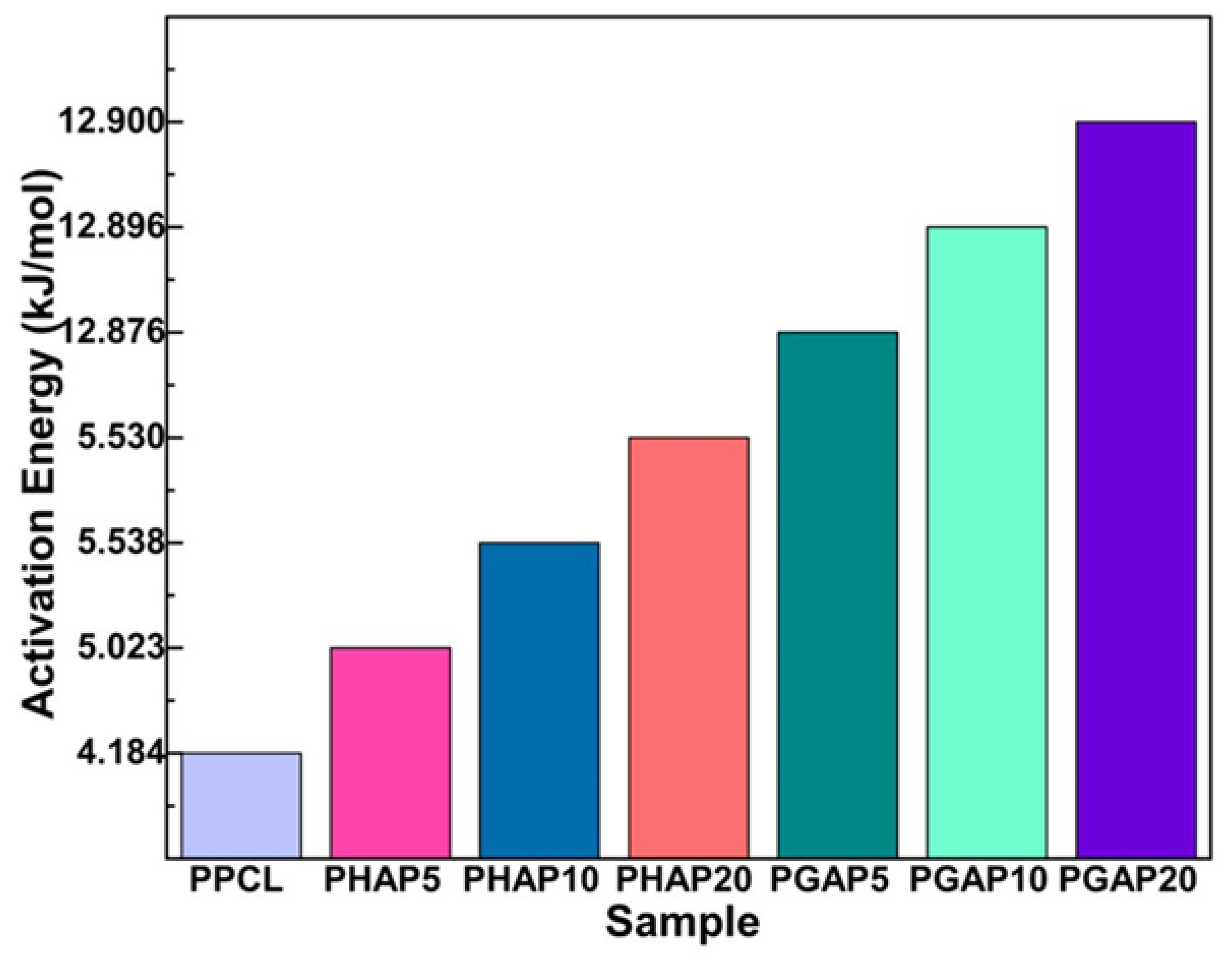
3.4. Activation Energy
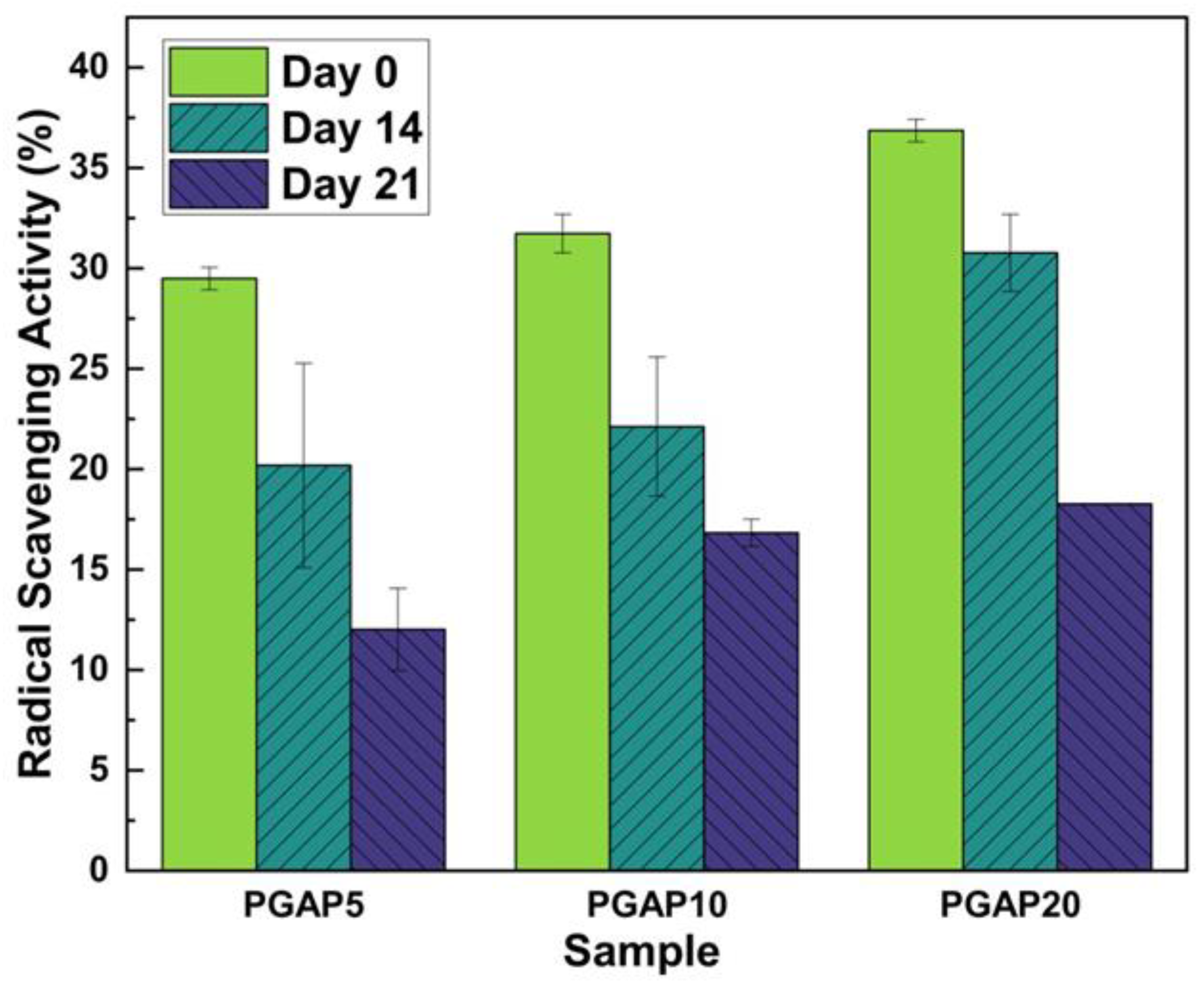
3.5. Antioxidant Properties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Daskalakis, E.; Hassan, M.H.; Omar, A.M.; Acar, A.A.; Fallah, A.; Cooper, G.; Weightman, A.; Blunn, G.; Koc, B.; Bartolo, P. Accelerated Degradation of Poly-ε-caprolactone Composite Scaffolds for Large Bone Defects. Polymers 2023, 15, 670. [Google Scholar] [CrossRef]
- Tajvar, S.; Hadjizadeh, A.; Samandari, S.S. Scaffold degradation in bone tissue engineering: An overview. Int. Biodeterior. Biodegrad. 2023, 180, 105599. [Google Scholar] [CrossRef]
- Salmasi, S.; Nayyer, L.; Seifalian, A.M.; Blunn, G.W. Nanohydroxyapatite Effect on the Degradation, Osteoconduction and Mechanical Properties of Polymeric Bone Tissue Engineered Scaffolds. Open Orthop. J. 2016, 10, 900–919. [Google Scholar] [CrossRef]
- Hegyesi, N.; Hodosi, E.; Polyák, P.; Faludi, G.; Balogh-Weiser, D.; Pukánszky, B. Controlled degradation of poly-ε-caprolactone for resorbable scaffolds. Colloids Surf. B Biointerfaces 2020, 186, 110678. [Google Scholar] [CrossRef] [PubMed]
- Rodenas-Rochina, J.; Vidaurre, A.; Castilla Cortázar, I.; Lebourg, M. Effects of hydroxyapatite filler on long-term hydrolytic degradation of PLLA/PCL porous scaffolds. Polym. Degrad. Stab. 2015, 119, 121–131. [Google Scholar] [CrossRef]
- Bartnikowski, M.; Dargaville, T.R.; Ivanovski, S.; Hutmacher, D.W. Degradation mechanisms of polycaprolactone in the context of chemistry, geometry and environment. Prog. Polym. Sci. 2019, 96, 1–20. [Google Scholar] [CrossRef]
- Sivalingam, G.; Karthik, R.; Madras, G. Kinetics of thermal degradation of poly(ε-caprolactone). J. Anal. Appl. Pyrolysis 2003, 70, 631–647. [Google Scholar] [CrossRef]
- Fiuza, C.; Polak-Kraśna, K.; Antonini, L.; Petrini, L.; Carroll, O.; Ronan, W.; Vaughan, T.J. An experimental investigation into the physical, thermal and mechanical degradation of a polymeric bioresorbable scaffold. J. Mech. Behav. Biomed. Mater. 2022, 125, 104955. [Google Scholar] [CrossRef] [PubMed]
- Weir, N.A.; Buchanan, F.J.; Orr, J.F.; Dickson, G.R. Degradation of poly-L-lactide. Part 1: In vitro and in vivo physiological temperature degradation. Proc. Inst. Mech. Eng. H 2004, 218, 307–319. [Google Scholar] [CrossRef] [PubMed]
- Mahomed, A. Ageing processes of biomedical polymers in the body. In Durability and Reliability of Medical Polymers; Elsevier: Amsterdam, The Netherlands, 2012; pp. 164–182. [Google Scholar] [CrossRef]
- Fagali, N.S.; A Madrid, M.; Maceda, B.T.P.; Fernández, M.E.L.; Puerto, R.M.L.; de Mele, M.F.L. Effect of degradation products of iron-bioresorbable implants on the physiological behavior of macrophages in vitro. Metallomics 2020, 12, 1841–1850. [Google Scholar] [CrossRef]
- Cerqueni, G.; Scalzone, A.; Licini, C.; Gentile, P.; Mattioli-Belmonte, M. Insights into oxidative stress in bone tissue and novel challenges for biomaterials. Mater. Sci. Eng. C 2021, 130, 112433. [Google Scholar] [CrossRef] [PubMed]
- Yao, H.; Huang, Y.; Li, X.; Li, X.; Xie, H.; Luo, T.; Chen, J.; Chen, Z. Underlying mechanisms of reactive oxygen species and oxidative stress photoinduced by graphene and its surface-functionalized derivatives. Environ. Sci. Nano 2020, 7, 782–792. [Google Scholar] [CrossRef]
- Liang, X.; Yang, X.; Liu, J.; Tu, L.; Wei, W.; Wang, H.; Wu, M.; Cai, L.; Zheng, Y.; Chen, Y. ROS-scavenging bioactive scaffold orchestrates bone regeneration for osteoporotic bone defect repair. Compos. B Eng. 2024, 281, 111528. [Google Scholar] [CrossRef]
- Hou, Y.; Wang, W.; Bartolo, P. The effect of graphene and graphene oxide induced reactive oxygen species on polycaprolactone scaffolds for bone cancer applications. Mater. Today Bio 2024, 24, 100886. [Google Scholar] [CrossRef] [PubMed]
- Mambiri, L.T.; Broussard, G.; Smith, J.; Depan, D. In-Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls for Modulated Physicochemical Properties of Poly (Caprolactone) Composites. Macromol 2024, 4, 285–303. [Google Scholar] [CrossRef]
- Mansur, H.S.; Costa, H.S.; Mansur, A.A.P.; Pereira, M. 3D-macroporous hybrid scaffolds for tissue engineering: Network design and mathematical modeling of the degradation kinetics. Mater. Sci. Eng. C 2012, 32, 404–415. [Google Scholar] [CrossRef]
- García, A.V.; Serrano, N.J.; Sanahuja, A.B.; Garrigós, M.C. Novel antioxidant packaging films based on poly(ε-caprolactone) and almond skin extract: Development and effect on the oxidative stability of fried almonds. Antioxidants 2020, 9, 629. [Google Scholar] [CrossRef]
- Kumar, V.; Tang, X. New Horizons in Nanofiller-Based Polymer Composites II. Polymers 2023, 15, 4259. [Google Scholar] [CrossRef] [PubMed]
- Azani, M.-R.; Hassanpour, A. High-performance polymer nanocomposites: Advanced fabrication methods and critical insights. J. Polym. Res. 2024, 31, 168. [Google Scholar] [CrossRef]
- Sima, W.; Tang, X.; Sun, P.; Yang, M.; Yuan, T.; Shi, Z.; Yang, H.; Deng, Q. Role of a 3D Nanoskeleton in Hindering Electrical Tree Growth in Nanocomposites: Insights from in Situ Self-Fluorescence Imaging. ACS Macro Lett. 2023, 12, 866–873. [Google Scholar] [CrossRef]
- Sharma, M.; Chauhan, P.; Jangid, N.K.; Sharma, R. Recent Advances in Nanofillers for Multidisciplinary Applications of Polymer Nanocomposites. In Handbook of Nanofillers; Springer Nature: Singapore, 2024; pp. 1–20. [Google Scholar] [CrossRef]
- Plota, A.; Masek, A. Lifetime prediction methods for degradable polymeric materials—A short review. Materials 2020, 13, 4507. [Google Scholar] [CrossRef]
- Castilla-Cortázar, I.; Vidaurre, A.; Marí, B.; Campillo-Fernández, A.J. Morphology, Crystallinity, and Molecular Weight of Poly(ε-caprolactone)/Graphene Oxide Hybrids. Polymers 2019, 11, 1099. [Google Scholar] [CrossRef] [PubMed]
- Biscaia, S.; Silva, J.C.; Moura, C.; Viana, T.; Tojeira, A.; Mitchell, G.R.; Pascoal-Faria, P.; Ferreira, F.C.; Alves, N. Additive Manufactured Poly(ε-caprolactone)-graphene Scaffolds: Lamellar Crystal Orientation, Mechanical Properties and Biological Performance. Polymers 2022, 14, 1669. [Google Scholar] [CrossRef]
- Wu, D.; Lin, D.; Zhang, J.; Zhou, W.; Zhang, M.; Zhang, Y.; Wang, D.; Lin, B. Selective Localization of Nanofillers: Effect on Morphology and Crystallization of PLA/PCL Blends. Macromol. Chem. Phys. 2011, 212, 613–626. [Google Scholar] [CrossRef]
- Ajala, O.; Werther, C.; Nikaeen, P.; Singh, R.P.; Depan, D. Influence of graphene nanoscrolls on the crystallization behavior and nano-mechanical properties of polylactic acid. Polym. Adv. Technol. 2019, 30, 1825–1835. [Google Scholar] [CrossRef]
- Richaud, E.; Derue, I.; Gilormini, P.; Verdu, J.; Vaulot, C.; Coquillat, M.; Desgardin, N.; Vandenbrouke, A. Plasticizer effect on network structure and hydrolytic degradation. Eur. Polym. J. 2015, 69, 232–246. [Google Scholar] [CrossRef]
- Blasi, P.; D’Souza, S.S.; Selmin, F.; DeLuca, P.P. Plasticizing effect of water on poly(lactide-co-glycolide). J. Control. Release 2005, 108, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Catania, F.; Marras, E.; Giorcelli, M.; Jagdale, P.; Lavagna, L.; Tagliaferro, A.; Bartoli, M. A Review on Recent Advancements of Graphene and Graphene-Related Materials in Biological Applications. Appl. Sci. 2021, 11, 614. [Google Scholar] [CrossRef]
- Shin, S.R.; Li, Y.-C.; Jang, H.L.; Khoshakhlagh, P.; Akbari, M.; Nasajpour, A.; Zhang, Y.S.; Tamayol, A.; Khademhosseini, A. Graphene-based materials for tissue engineering. Adv. Drug Deliv. Rev. 2016, 105, 255–274. [Google Scholar] [CrossRef] [PubMed]
- Ganguly, S.; Sengupta, J. Graphene-Based Nanofiller Fabrication: Opportunities and Challenges. In Handbook of Nanofillers; Springer Nature: Singapore, 2024; pp. 1–22. [Google Scholar] [CrossRef]
- Jodati, H.; Yilmaz, B.; Evis, Z. In vitro and in vivo properties of graphene-incorporated scaffolds for bone defect repair. Ceram. Int. 2021, 47, 29535–29549. [Google Scholar] [CrossRef]
- Kamatchi, T.; Saravanan, R.; Rangappa, S.M.; Siengchin, S. Effect of filler content and size on the mechanical properties of graphene-filled natural fiber-based nanocomposites. Biomass Convers. Biorefinery 2023, 13, 11311–11320. [Google Scholar] [CrossRef]
- Ou, L.; Tan, X.; Qiao, S.; Wu, J.; Su, Y.; Xie, W.; Jin, N.; He, J.; Luo, R.; Lai, X.; et al. Graphene-Based Material-Mediated Immunomodulation in Tissue Engineering and Regeneration: Mechanism and Significance. ACS Nano 2023, 17, 18669–18687. [Google Scholar] [CrossRef] [PubMed]
- Florien, N.; Gupta, S.; Poria, R.; Chaudhary, D.; Poria, R.; Rawat, T. Structure and Electrochemical Properties of Graphene, Derivatives, and Its Nanocomposites. In Electrochemical Exfoliation of Graphene and Its Derivatives. Engineering Materials; Springer: Singapore, 2024; pp. 113–136. [Google Scholar] [CrossRef]
- Moharana, S.; Kar, S.K.; Mishra, M.K.; Mahaling, R.N. Synthesis and Properties of Graphene and Graphene Oxide-Based Polymer Composites. In Surface Engineering of Graphene; Springer: Berlin/Heidelberg, Germany, 2019; pp. 175–201. [Google Scholar] [CrossRef]
- Langueh, C.; Changotade, S.; Ramtani, S.; Lutomski, D.; Rohman, G. Combination of in vitro thermally-accelerated ageing and Fourier-Transform Infrared spectroscopy to predict scaffold lifetime. Polym. Degrad. Stab. 2021, 183, 109454. [Google Scholar] [CrossRef]
- Bahrami, S.; Solouk, A.; Mirzadeh, H.; Seifalian, A.M. Electroconductive polyurethane/graphene nanocomposite for biomedical applications. Compos. B Eng. 2019, 168, 421–431. [Google Scholar] [CrossRef]
- Klimova, N.S.; Pereborova, N.V.; Makarov, A.G. Activation Energy of Deformation Processes in Polymer Textile Materials. Fibre Chemistry 2021, 53, 76–81. [Google Scholar] [CrossRef]
- Noroozi, N.; Thomson, J.A.; Noroozi, N.; Schafer, L.L.; Hatzikiriakos, S.G. Viscoelastic behaviour and flow instabilities of biodegradable poly (ε-caprolactone) polyesters. Rheol. Acta 2012, 51, 179–192. [Google Scholar] [CrossRef]
- Arcenegui-Troya, J.; Sánchez-Jiménez, P.E.; Perejón, A.; Pérez-Maqueda, L.A. Determination of the activation energy under isothermal conditions: Revisited. J. Therm. Anal. Calorim. 2023, 148, 1679–1686. [Google Scholar] [CrossRef]
- Tripathy, D.; Gadtya, A.S.; Sahu, B.B.; Moharana, S. Surface Engineering of Graphene-Based Polymeric Composites for Energy Storage Devices. In Emerging Nanodielectric Materials for Energy Storage; Springer: Berlin/Heidelberg, Germany, 2024; pp. 269–303. [Google Scholar] [CrossRef]
- Phuoc, T.X.; Chen, R.H. Modeling the effect of particle size on the activation energy and ignition temperature of metallic nanoparticles. Combust. Flame 2012, 159, 416–419. [Google Scholar] [CrossRef]
- Khan, N.S.; Shah, Z.; Shutaywi, M.; Kumam, P.; Thounthong, P. A comprehensive study to the assessment of Arrhenius activation energy and binary chemical reaction in swirling flow. Sci. Rep. 2020, 10, 7868. [Google Scholar] [CrossRef] [PubMed]
- Yu, W.; Sisi, L.; Haiyan, Y.; Jie, L. Progress in the functional modification of graphene/graphene oxide: A review. RSC Adv. 2020, 10, 15328–15345. [Google Scholar] [CrossRef]
- Singh, A.; Satheeshkumar, P.K. Reactive Oxygen Species (ROS) and ROS Scavengers in Plant Abiotic Stress Response. In Stress Biology in Photosynthetic Organisms; Springer Nature: Singapore, 2024; pp. 41–63. [Google Scholar] [CrossRef]
- Wang, Y.; Ji, D.; Chen, T.; Li, B.; Zhang, Z.; Qin, G.; Tian, S. Production, Signaling, and Scavenging Mechanisms of Reactive Oxygen Species in Fruit–Pathogen Interactions. Int. J. Mol. Sci. 2019, 20, 2994. [Google Scholar] [CrossRef]
- Bhaloo, A.; Nguyen, S.; Lee, B.H.; Valimukhametova, A.; Gonzalez-Rodriguez, R.; Sottile, O.; Dorsky, A.; Naumov, A.V. Doped Graphene Quantum Dots as Biocompatible Radical Scavenging Agents. Antioxidants 2023, 12, 1536. [Google Scholar] [CrossRef] [PubMed]
- Qiu, Y.; Wang, Z.; Owens, A.C.E.; Kulaots, I.; Chen, Y.; Kane, A.B.; Hurt, R.H. Antioxidant chemistry of graphene-based materials and its role in oxidation protection technology. Nanoscale 2014, 6, 11744–11755. [Google Scholar] [CrossRef] [PubMed]
- Świderek, K.; Velasco-Lozano, S.; Galmés M, À.; Olazabal, I.; Sardon, H.; López-Gallego, F.; Moliner, V. Mechanistic studies of a lipase unveil effect of pH on hydrolysis products of small PET modules. Nat. Commun. 2023, 14, 3556. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Camilli, L.; Caridad, J.M.; Santos, J.E.; Liu, Y. Spontaneous adsorption of ions on graphene at the electrolyte–graphene interface. Appl. Phys. Lett. 2020, 117, 203102. [Google Scholar] [CrossRef]
- Mohanan, N.; Wong, C.H.; Budisa, N.; Levin, D.B. Characterization of Polymer Degrading Lipases, LIP1 and LIP2 From Pseudomonas chlororaphis PA23. Front. Bioeng. Biotechnol. 2022, 10, 854298. [Google Scholar] [CrossRef] [PubMed]
- Soozanipour, A.; Taheri-Kafrani, A. Enzyme Immobilization on Functionalized Graphene Oxide Nanosheets: Efficient and Robust Biocatalysts. Methods Enzymol. 2018, 609, 371–403. [Google Scholar] [CrossRef] [PubMed]
- Sadiq, H.; Sadiq, H.; Sohail, A.; Basit, A.; Akhtar, N.; Batool, K.; Hisaindee, S.; Asghar, L. Assessment of antioxidant activity of pure graphene oxide (GO) and composite V2O5/GO using DPPH radical and H2O2 scavenging assays. J. Solgel Sci. Technol. 2023, 108, 840–849. [Google Scholar] [CrossRef]
- Ramakrishna TR, B.; Nalder, T.D.; Yang, W.; Marshall, S.N.; Barrow, C.J. Controlling enzyme function through immobilisation on graphene, graphene derivatives and other two dimensional nanomaterials. J. Mater. Chem. B 2018, 6, 3200–3218. [Google Scholar] [CrossRef]
- Losada-Garcia, N.; Berenguer-Murcia, A.; Cazorla-Amorós, D.; Palomo, J. Efficient Production of Multi-Layer Graphene from Graphite Flakes in Water by Lipase-Graphene Sheets Conjugation. Nanomaterials 2019, 9, 1344. [Google Scholar] [CrossRef]
- Freije García, F.; García Liñares, G. Use of Lipases as a Sustainable and Efficient Method for the Synthesis and Degradation of Polymers. J. Polym. Environ. 2024, 32, 2484–2516. [Google Scholar] [CrossRef]
- Chen, M.; Wu, H.; Li, Z.; Wu, K.; Jiao, Y.; Zhou, C. Synthesis of chitin/graphene oxide composite aerogel beads for lipase immobilization. J. Porous Mater. 2020, 27, 549–554. [Google Scholar] [CrossRef]




| R2 Zero-Order | R2 First-Order | R2 Higuchi | R2 Korsmeyer | R2 Contracting Volume | Best Fit Model | |
|---|---|---|---|---|---|---|
| PPCL | 0.72 | 0.72 | 0.55 | 0.87 | 0.69 | Zero Order/Korsmeyer |
| PHAP5 | 0.68 | 0.75 | 0.45 | 0.89 | 0.79 | Korsmeyer |
| PHAP10 | 0.83 | 0.83 | 0.63 | 0.92 | 0.70 | Korsmeyer |
| PHAP20 | 0.68 | 0.68 | 0.45 | 0.92 | 0.60 | Korsmeyer |
| PGAP5 | 0.74 | 0.74 | 0.55 | 0.93 | 0.65 | Korsmeyer |
| PGAP10 | 0.49 | 0.49 | 0.31 | 0.98 | 0.57 | Korsmeyer |
| PGAP20 | 0.68 | 0.68 | 0.46 | 0.96 | 0.52 | Korsmeyer |
| k4 (Korsmeyer) | n (Korsmeyer) | |
|---|---|---|
| PPCL | 5.1 × 10−3 | 1.38 |
| PHAP5 | 2.0 × 10−4 | 2.37 |
| PHAP10 | 9.0 × 10−4 | 1.90 |
| PHAP20 | 1.0 × 10−6 | 3.76 |
| PGAP5 | 9.4 × 10−5 | 2.53 |
| PGAP10 | 6.1 × 10−5 | 2.63 |
| PGAP20 | 2.0 × 10−5 | 2.93 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mambiri, L.T.; Depan, D. Degradation Kinetics, Mechanisms, and Antioxidant Activity of PCL-Based Scaffolds with In Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls. C 2025, 11, 5. https://doi.org/10.3390/c11010005
Mambiri LT, Depan D. Degradation Kinetics, Mechanisms, and Antioxidant Activity of PCL-Based Scaffolds with In Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls. C. 2025; 11(1):5. https://doi.org/10.3390/c11010005
Chicago/Turabian StyleMambiri, Lillian Tsitsi, and Dilip Depan. 2025. "Degradation Kinetics, Mechanisms, and Antioxidant Activity of PCL-Based Scaffolds with In Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls" C 11, no. 1: 5. https://doi.org/10.3390/c11010005
APA StyleMambiri, L. T., & Depan, D. (2025). Degradation Kinetics, Mechanisms, and Antioxidant Activity of PCL-Based Scaffolds with In Situ Grown Nanohydroxyapatite on Graphene Oxide Nanoscrolls. C, 11(1), 5. https://doi.org/10.3390/c11010005









